Open Gromov-Witten Invariants from the Augmentation Polynomial
Abstract
1. Introduction
1.1. Statement of Main Results
1.2. Organization of the Paper
2. Open String Mirror Symmetry and the Augmentation Polynomial
2.1. The Mirror of
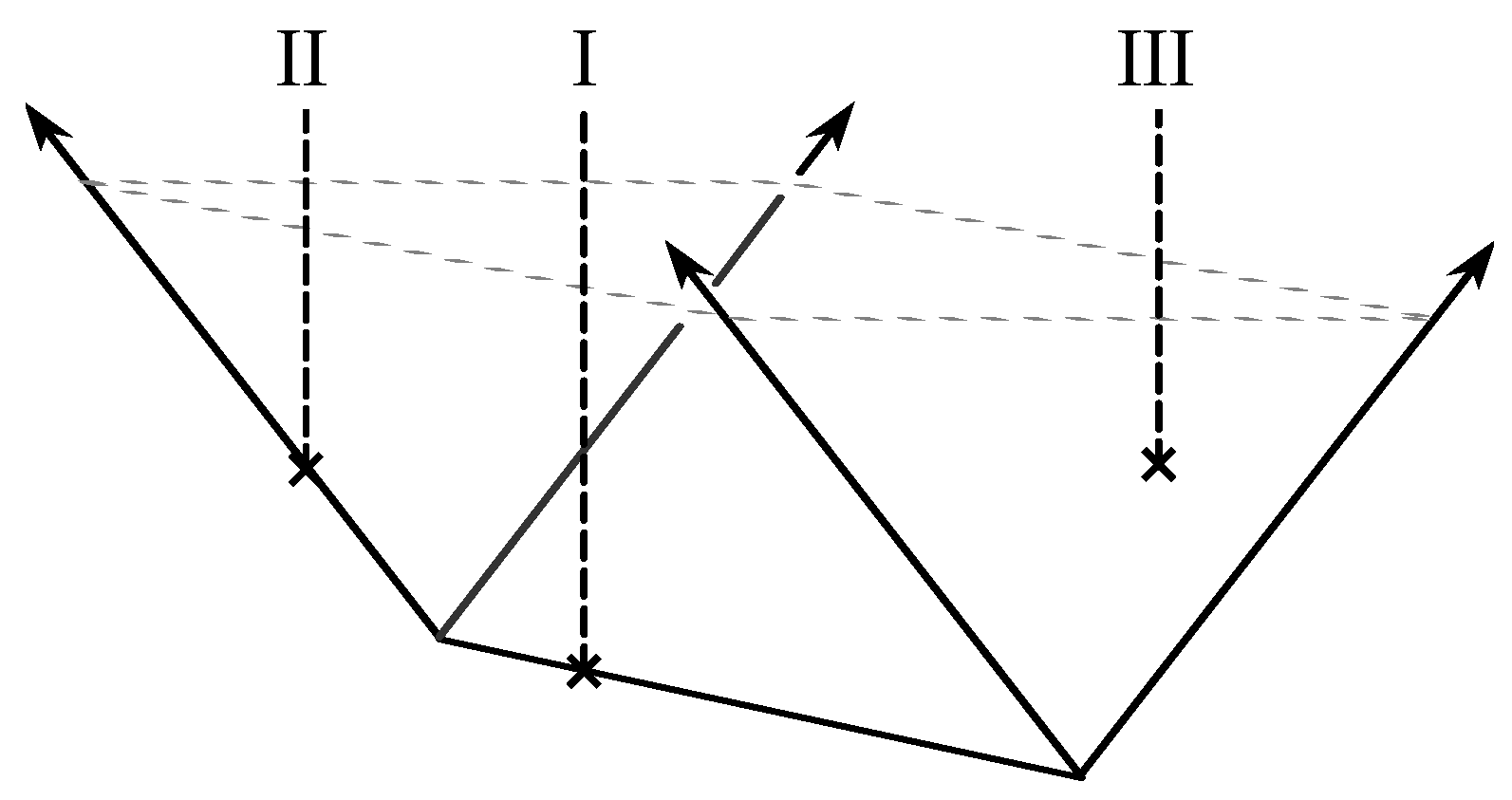
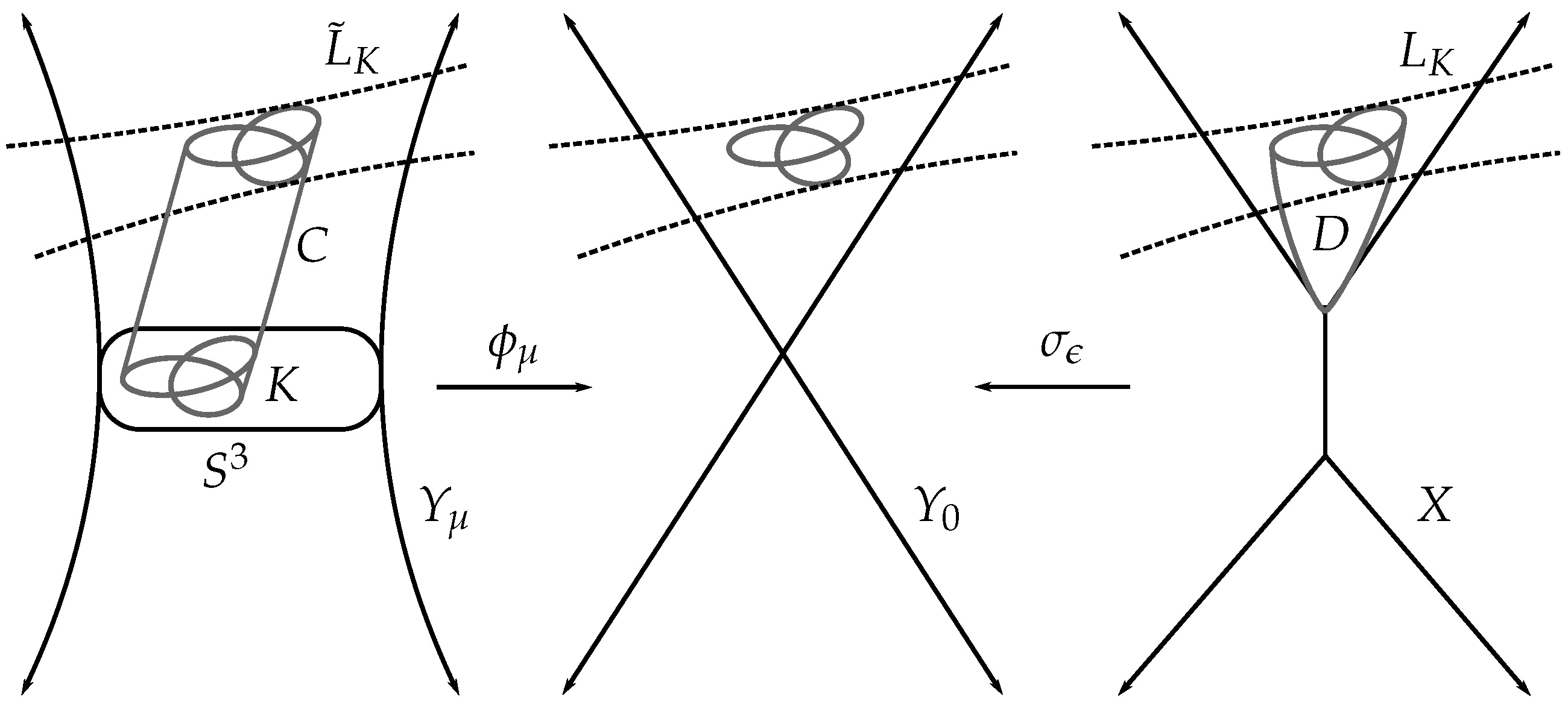
2.2. Knots and the Conifold Transition
2.3. Open Gromov-Witten Invariants and the Augmentation Polynomial
3. Non-Toric Examples
3.1. The (Figure-Eight) Knot
3.2. The (Three-Twist) Knot
4. Recovering the Augmentation Polynomial
4.1. The Unknot
4.2. The Torus Knot
5. Conclusions
Acknowledgments
Conflicts of Interest
Appendix A. Proof of Lemma 1
References
- Candelas, P.; de la Ossa, X.C.; Green, P.S.; Parkes, L. A pair of Calabi-Yau manifolds as an exactly soluble superconformal theory. Nuclear Phys. B 1991, 359, 21–74. [Google Scholar] [CrossRef]
- Witten, E. Mirror manifolds and topological field theory. In Essays on Mirror Manifolds; International Press: Hong Kong, China, 1992; pp. 120–158. [Google Scholar]
- Gopakumar, R.; Vafa, C. On the gauge theory/geometry correspondence. Adv. Theor. Math. Phys. 1999, 3, 1415–1443. [Google Scholar] [CrossRef]
- Ooguri, H.; Vafa, C. Knot invariants and topological strings. Nuclear Phys. B 2000, 577, 419–438. [Google Scholar] [CrossRef]
- Kontsevich, M. Enumeration of rational curves via torus actions. In The Moduli Space of Curves (Texel Island, 1994); Birkhäuser Boston: Boston, MA, USA, 1995; Volume 129, pp. 335–368. [Google Scholar]
- Graber, T.; Pandharipande, R. Localization of virtual classes. Invent. Math. 1999, 135, 487–518. [Google Scholar] [CrossRef]
- Chiang, T.M.; Klemm, A.; Yau, S.T.; Zaslow, E. Local mirror symmetry: Calculations and interpretations. Adv. Theor. Math. Phys. 1999, 3, 495–565. [Google Scholar] [CrossRef]
- Klemm, A.; Zaslow, E. Local mirror symmetry at higher genus. arXiv, 1999; arXiv:hep-th/9906046. [Google Scholar]
- Witten, E. Quantum field theory and the Jones polynomial. Commun. Math. Phys. 1989, 121, 351–399. [Google Scholar] [CrossRef]
- Diaconescu, D.E.; Shende, V.; Vafa, C. Large N duality, Lagrangian cycles, and algebraic knots. Commun. Math. Phys. 2013, 319, 813–863. [Google Scholar] [CrossRef]
- Aganagic, M.; Vafa, C. Large N duality, mirror symmetry, and a Q-deformed A-polynomial for knots. arXiv, 2012; arXiv:1204.4709 [hep-th]. [Google Scholar]
- Aganagic, M.; Ekholm, T.; Ng, L.; Vafa, C. Topological strings, D-model, and knot contact homology. Adv. Theor. Math. Phys. 2014, 18, 827–956. [Google Scholar]
- Ng, L. A topological introduction to knot contact homology. arXiv, 2012; arXiv:1210.4803 [math.GT]. [Google Scholar]
- Strominger, A.; Yau, S.T.; Zaslow, E. Mirror symmetry is T-duality. Nuclear Phys. B 1996, 479, 243–259. [Google Scholar] [CrossRef]
- Aganagic, M.; Vafa, C. Mirror symmetry, D-branes and counting holomorphic discs. arXiv, 2000; arXiv:hep-th/0012041. [Google Scholar]
- Hori, K.; Vafa, C. Mirror symmetry. arXiv, 2000; arXiv:hep-th/0002222. [Google Scholar]
- Aganagic, M.; Klemm, A.; Vafa, C. Disk instantons, mirror symmetry and the duality web. Z. Naturforsch. A 2002, 57, 1–28. [Google Scholar] [CrossRef]
- Katz, S.; Liu, C.C.M. Enumerative geometry of stable maps with Lagrangian boundary conditions and multiple covers of the disc. Adv. Theor. Math. Phys. 2001, 5, 1–49. [Google Scholar] [CrossRef]
- Labastida, J.; Mariño, M.; Vafa, C. Knots, links and branes at large N. J. High Energy Phys. 2001, 2000. [Google Scholar] [CrossRef]
- Brini, A.; Eynard, B.; Mariño, M. Torus knots and mirror symmetry. Ann. Henri Poincaré 2012, 13, 1873–1910. [Google Scholar] [CrossRef]
- Jockers, H.; Klemm, A.; Soroush, M. Torus knots and the topological vertex. Lett. Math. Phys. 2014, 104, 953–989. [Google Scholar] [CrossRef]
- Ekholm, T.; Etnyre, J.; Ng, L.; Sullivan, M. Knot contact homology. Geom. Topol. 2013, 17, 975–1112. [Google Scholar] [CrossRef]
- Gu, J.; Jockers, H.; Klemm, A.; Soroush, M. Knot invariants from topological recursion on augmentation varieties. Commun. Math. Phys. 2015, 336, 987–1051. [Google Scholar] [CrossRef]
- Neitzke, A.; Walcher, J. background independence and the open topological string wavefunction. arXiv, 2007; arXiv:0709.2390 [hep-th]. [Google Scholar]
- Graber, T.; Zaslow, E. Open-string Gromov-Witten invariants: calculations and a mirror “theorem”. In Orbifolds in Mathematics and Physics (Madison, WI, 2001); American Mathematical Society: Providence, RI, USA, 2002; Volume 310, pp. 107–121. [Google Scholar]
- Givental, A. Equivariant Gromov-Witten invariants. Internat. Math. Res. Notices 1996, 1996, 613–663. [Google Scholar] [CrossRef]
- Brini, A.; Cavalieri, R. Open orbifold Gromov–Witten invariants of []: Localization and Mirror Symmetry. arXiv, 2010; arXiv:1007.0934 [math.AG]. [Google Scholar]
- Brini, A. Open topological strings and integrable hierarchies: remodeling the A-model. Commun. Math. Phys. 2012, 312, 735–780. [Google Scholar] [CrossRef]
- Cox, D.A.; Katz, S. Mirror symmetry and algebraic geometry. In Mathematical Surveys and Monographs; American Mathematical Society: Providence, RI, USA, 1999; Volume 68. [Google Scholar]
- Coates, T.; Corti, A.; Iritani, H.; Tseng, H.H. Computing genus-zero twisted Gromov-Witten invariants. Duke Math. J. 2009, 147, 377–438. [Google Scholar] [CrossRef]
- Brini, A. (Imperial College London). Personal communication, 2016.
| 2 | 4 | 14 | 60 | |
| 1 | 0 | 186 |
| 2 | 4 | 14 | 60 | |
| 1 | 0 | 186 |
| 0 | 4 | |||
| 2 | 1 | 0 | ||
| 8 |
| 1 | 2 | |||
| 0 | 4 | |||
| 2 | 1 | 0 | 14 | |
| 8 |
© 2017 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahowald, M. Open Gromov-Witten Invariants from the Augmentation Polynomial. Symmetry 2017, 9, 232. https://doi.org/10.3390/sym9100232
Mahowald M. Open Gromov-Witten Invariants from the Augmentation Polynomial. Symmetry. 2017; 9(10):232. https://doi.org/10.3390/sym9100232
Chicago/Turabian StyleMahowald, Matthew. 2017. "Open Gromov-Witten Invariants from the Augmentation Polynomial" Symmetry 9, no. 10: 232. https://doi.org/10.3390/sym9100232
APA StyleMahowald, M. (2017). Open Gromov-Witten Invariants from the Augmentation Polynomial. Symmetry, 9(10), 232. https://doi.org/10.3390/sym9100232




