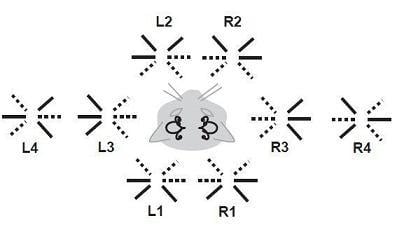
Symmetries of the Central Vestibular System: Forming Movements for Gravity and a Three-Dimensional World
Abstract
:1. Sensorimotor Integration and the Central Vestibular System
2. Anatomy, Physiology, and Behavior
3. Multimodal Sensory Organization
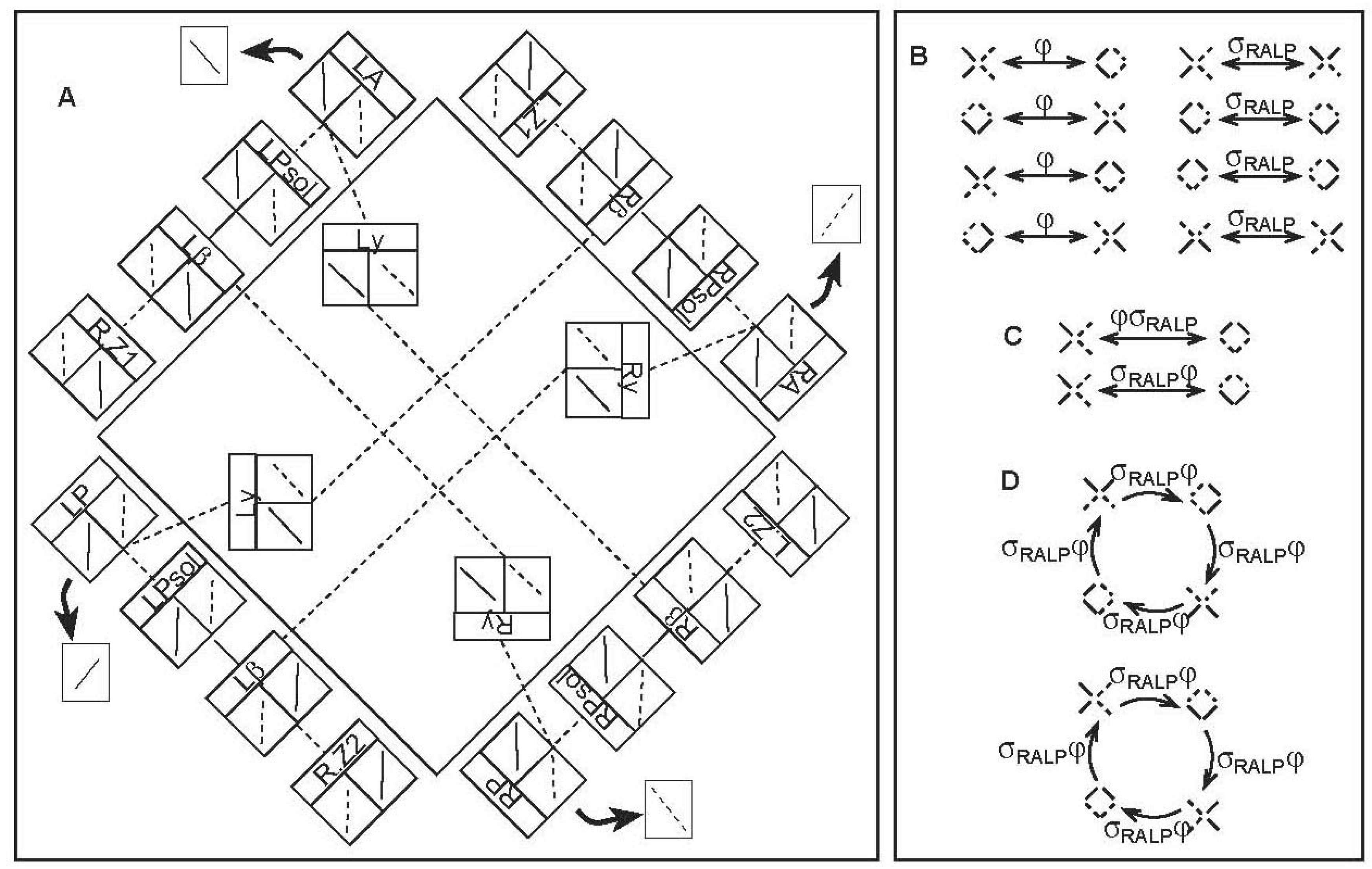
4. Pathway Symmetries
4.1. A Canal-Neck Pathway
4.2. Canal Pathway to the Uvula-Nodulus (CVOUN Pathway)
5. Sensorimotor States, Pathway Symmetries, and Causal Logic
Acknowledgments
References and Notes
- Goldberg, J.M.; Fernández, C. The Vestibular System. In Handbook of Physiology—The Nervous System; Brookhart, J.M., Mountcastle, V.B., Darian-Smith, I., Eds.; American Physiological Society: Bethesda, MD, USA, 1984; Volume III, pp. 977–1022. [Google Scholar]
- Barmack, N.H. Central Vestibular System: Vestibular Nuclei and Posterior Cerebellum. Brain Res. Bull. 2003, 60, 511–541. [Google Scholar] [CrossRef]
- Boyle, R.; Belton, T.; McCrea, R.A. Responses of Identified Vestibulospinal Neurons to Voluntary and Reflex Eye and Head Movements in the Alert Squirrel Monkey. Ann. NY Acad Sci. 1996, 781, 244–263. [Google Scholar] [CrossRef] [PubMed]
- McCrea, R.A.; Gdowski, G.T.; Boyle, R.; Belton, T. Firing Behavior of Vestibular Neurons during Active and Passive Head Movements: Vestibulo-Spinal and Other Non-Eye-Movement Related Neurons. J. Neurophys. 1999, 82, 416–428. [Google Scholar] [CrossRef] [PubMed]
- Roy, J.E.; Cullen, K.E. Selective processing of vestibular reafference during self-generated head motion. J. Neurosci. 2001, 21, 2131–2142. [Google Scholar] [CrossRef] [PubMed]
- Roy, J.E.; Cullen, K.E. Dissociating Self-Generated from Passive Applied Head Motion: Neural Mechanisms in the Vestibular Nuclei. J. Neurosci. 2004, 24, 2102–2111. [Google Scholar] [CrossRef] [PubMed]
- Cullen, K.E. Sensory signals during active versus passive movement. Curr. Opin. Neurobiol. 2004, 14, 698–706. [Google Scholar] [CrossRef] [PubMed]
- McCluskey, M.K.; Cullen, K.E. Eye, Head, and Body Coordination during Large Gaze Shifts in Rhesus Monkeys: Movement Kinematics and the Influence of Posture. J. Neurophysiol. 2007, 97, 2976–2991. [Google Scholar] [CrossRef]
- Simpson, J.I. The Accessory Optic System. Ann. Rev. Neurosc. 1984, 7, 13–41. [Google Scholar] [CrossRef]
- Giolli, R.A.; Blanks, R.H.; Lui, F. The Accessory Optic System: Basic Organization with an Update on Connectivity, Neurochemistry, and Function. Prog. Brain Res. 2005, 151, 407–440. [Google Scholar]
- Hanes, D.A.; McCollum, G. Cognitive-Vestibular Interactions: A Review of Patient Difficulties and Possible Mechanisms. J. Vest. Res. 2006, 16, 75–91. [Google Scholar]
- Fukushima, K. Corticovestibular Interactions: Anatomy, Electrophysiology, and Functional Considerations. Exp. Brain Res. 1997, 117, 1–16. [Google Scholar] [CrossRef] [PubMed]
- Brandt, T.; Schnautzer, F.; Hamilton, D.A.; Brüning, R.; Markowitsch, H.J.; Kalla, R.; Darlington, C.; Smith, P.; Strupp, M. Vestibular Loss Causes Hippocampal Atrophy and Impaired Spatial Memory in Humans. Brain 2005, 128, 2732–2741. [Google Scholar] [CrossRef] [PubMed]
- Smith, P.F.; Horii, A.; Russell, N.; Bilkey, D.K.; Zheng, Y.; Liu, P.; Kerr, D.S.; Darlington, C.L. The Effects of Vestibular Lesions on Hippocampal Function in Rats. Prog. Neurobiol. 2005, 75, 391–405. [Google Scholar] [CrossRef] [PubMed]
- McCollum, G.; Boyle, R. Rotations in a Vertebrate Setting: Evaluation of the Symmetry Group of the Disynaptic Canal-neck Projection. Biol. Cybern. 2004, 90, 203–217. [Google Scholar] [CrossRef] [PubMed]
- Foster, I.Z.; Hanes, D.A.; Barmack, N.H.; McCollum, G. Spatial Symmetries in Vestibular Projections to the Uvula-Nodulus. Biol. Cybern. 2007, 96, 439–453. [Google Scholar] [CrossRef] [PubMed]
- McCollum, G. Spatial Symmetry Groups as Sensorimotor Guidelines. J. Vest. Res. 2007, 17, 347–359. [Google Scholar]
- Lackner, J.R.; DiZio, P. Altered Sensory-Motor Control of the Head as an Etiological Factor in Space-Motion Sickness. Percept. Motor Skills 1989, 68, 784–786. [Google Scholar] [CrossRef]
- DiZio, P.; Lackner, J.R. Sensorimotor Aspects of High-Speed Artificial Gravity: III. Sensorimotor Adaptation. J. Vest. Res. 2002–2003, 12, 291–299. [Google Scholar]
- Clément, G.; Fraysse, M.J.; Deguine, O. Mental Representation of Space in Vestibular Patients with Otolithic or Rotatory Vertigo. Neurorep. 2009, 20, 457–461. [Google Scholar] [CrossRef]
- Lakoff, G.; Johnson, M. Metaphors We Live By; University of Chicago Press: Chicago, IL, USA, 2003. [Google Scholar]
- Llinás, R.R. I of the Vortex; MIT Press: Cambridge, MA, USA, 2001. [Google Scholar]
- Crosby, E.C. Comparative Aspects of Cerebellar Morphology. In Neurobiology of Cerebellar Evolution and Development; Llinás, R., Ed.; American Medical Association: Chicago, IL, USA, 1969. [Google Scholar]
- Bressloff, P.C.; Cowan, J.D.; Golubitsky, M.; Thomas, P.J.; Wiener, M.C. Geometric Visual Hallucinations, Euclidean Symmetry, and the Functional Architecture of Striate Cortex. Phil. Trans. R. Soc. Lond. B 2001, 356, 299–330. [Google Scholar] [CrossRef]
- Bressloff, P.C.; Cowan, J.D. A Spherical Model of Orientation and Spatial-Frequency Tuning in a Cortical Hypercolumn. Phil. Trans. R. Soc. Lond. B 2002, 357, 1643–1667. [Google Scholar] [CrossRef] [PubMed]
- Bressloff, P.C. Spontaneous Symmetry Breaking in Self-Organizing Neural Fields. Biol. Cybern. 2005, 93, 256–274. [Google Scholar] [CrossRef]
- Chastain, E.; Liu, Y. Firing Fields of Dorsocaudal Medial Entorhinal Cortex as a Context-Independent Spatial Map; Number 06-02; Robotics Institute Technical Report: Pittsburgh, PA, USA, January 2006. [Google Scholar]
- Chastain, E.; Liu, Y. Quantified Symmetry for Entorhinal Spatial Maps. Neurocomputing 2007, 70, 1723–1727. [Google Scholar] [CrossRef]
- Rock, I. The Perception of Movement. In Indirect Perception; Rock, I., Palmer, S., Eds.; MIT Press: Cambridge, MA, USA, 1996. [Google Scholar]
- Gregory, R.L. Knowledge in Perception and Illusion. Phil. Trans. R. Soc. Lond. B 1997, 352, 1121–1128. [Google Scholar] [CrossRef] [PubMed]
- Holly, J.E.; McCollum, G. Constructive Perception of Self-Motion. J. Vest. Res. 2008, 18, 249–266. [Google Scholar]
- Golubitsky, M.; Stewart, I. The Symmetry Perspective: From Equilibrium to Chaos in Phase Space and Physical Space; Birkhauser Verlag: Basel, Switzerland, 2002. [Google Scholar]
- Llinás, R.R. The Intrinsic Electrophysiological Properties of Mammalian Neurons: Insights into Central Nervous System Function. Science 1988, 242, 1654–1664. [Google Scholar] [CrossRef] [PubMed]
- Llinás, R.R.; Ribary, U.; Jeanmonod, D.; Kronberg, E.; Mitra, P.P. Thalamocortical Dysrhythmia: A Neurological and Neuropsychiatric Syndrome Characterized by Magnetoencephalography. Proc. Nat. Acad. Sci. USA 1999, 96, 15222–15227. [Google Scholar] [CrossRef] [PubMed]
- Varela, F.; Lachaux, J.-P.; Rodriguez, E.; Martinerie, J. The Brainweb: Phase Synchronization and Large-Scale Integration. Nat. Rev. Neurosci. 2001, 2, 229–239. [Google Scholar] [CrossRef]
- Golubitsky, M.; Shiau, L.J.; Stewart, I. Spatiotemporal Symmetries in the Disynaptic Canal-Neck Projection. SIAM J. Appl. Math. 2007, 67, 1396–1417. [Google Scholar] [CrossRef]
- Carroll, S.B. Evo-Devo and an Expanding Evolutionary Synthesis: A Genetic Theory of Morphological Evolution. Cell 2008, 134, 25–36. [Google Scholar] [CrossRef]
- Wagner, G.P.; Pavlicev, M.; Cheverud, J.M. The Road to Modularity. Nat. Rev. Genetics 2007, 8, 921–931. [Google Scholar] [CrossRef] [PubMed]
- Wagner, W.; Wagner, G.P. Examining the Modularity Concept in Evolutionary Psychology: The Level of Genes, Mind, and Culture. J. Cult. Evol. Psychol. 2003, 1, 135–165. [Google Scholar] [CrossRef]
- Uzun-Coruhlu, H.; Curthoys, I.S.; Jones, A.S. Attachment of the Utricular and Saccular Maculae to the Temporal Bone. Hearing Res. 2007, 233, 77–85. [Google Scholar] [CrossRef]
- Fritsch, B.; Beisel, K.W.; Jones, K.; Fariñas, I.; Maklad, A.; Lee, J.; Reichardt, L.F. Development and Evolution of Inner Ear Sensory Epithelia and their Development. J. Neurobiol. 2002, 53, 143–156. [Google Scholar] [CrossRef] [PubMed]
- Graf, W.M. Vestibular System. In Evolution of Nervous Systems: A Comprehensive Reference; Kaas, J.H., Krubitzer, L.A., Eds.; Academic Press: Amsterdam, The Netherland, 2007. [Google Scholar]
- Graf, W.; Klam, F. Le Système Vestibulaire: Anatomic Fontionelle et Comparée, Evolution et Développement. C. R. Palevol 2006, 5, 637–655. [Google Scholar] [CrossRef]
- Mach, E. Grundlinien der Lehre von den Bewegungsempfindungen (1875); Young, L.R.; Henn, V.; Scherberger, H., Translators; Leipzig: Engelmann, IL, USA, 2001. [Google Scholar]
- Shinoda, Y.; Sugiuchi, Y.; Futami, T.; Ando, N.; Kawasaki, T. Input Patterns and Pathways from Six Semicircular Canals to Motoneurons of Neck Muscles. I. The Multifidus Muscle Group. J. Neurophysiol. 1994, 72, 2691–702. [Google Scholar] [CrossRef] [PubMed]
- Shinoda, Y.; Sugiuchi, Y.; Futami, T.; Kakei, S.; Izawa, Y.; Na, J. Four Convergent Patterns of Input from the Six Semicircular Canals to Motoneurons of Different Neck Muscles in the Upper Cervical Cord. Ann. NY Acad. Sci. 1996, 781, 264–75. [Google Scholar] [CrossRef] [PubMed]
- Shinoda, Y.; Sugiuchi, Y.; Futami, T.; Ando, N.; Yagi, J. Input Patterns and Pathways from Six Semicircular Canals to Motoneurons of Neck Muscles. II. The Longissimus and Semispinalis Muscle Groups. J. Neurophysiol. 1997, 72, 2691–702. [Google Scholar] [CrossRef]
- Goldstein, H. Classical Mechanics, 2nd ed.; Addison-Wesley Publishing Company: Reading, MA, USA, 1980. [Google Scholar]
- Lackner, J.R.; DiZio, P. Vestibular, Proprioceptive, and Haptic Contributions to Spatial Orientation. Annu. Rev. Psychol. 2005, 56, 115–47. [Google Scholar] [CrossRef] [PubMed]
- Lewis, E.R.; Leverenz, E.L.; Bialek, W.S. The Vertebrate Inner Ear; CRC Press: Boca Raton, FL, USA, 1985. [Google Scholar]

© 2010 by the authors; licensee MDPI, Basel, Switzerland. This article is an Open Access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
McCollum, G.; Hanes, D.A. Symmetries of the Central Vestibular System: Forming Movements for Gravity and a Three-Dimensional World. Symmetry 2010, 2, 1544-1558. https://doi.org/10.3390/sym2031544
McCollum G, Hanes DA. Symmetries of the Central Vestibular System: Forming Movements for Gravity and a Three-Dimensional World. Symmetry. 2010; 2(3):1544-1558. https://doi.org/10.3390/sym2031544
Chicago/Turabian StyleMcCollum, Gin, and Douglas A. Hanes. 2010. "Symmetries of the Central Vestibular System: Forming Movements for Gravity and a Three-Dimensional World" Symmetry 2, no. 3: 1544-1558. https://doi.org/10.3390/sym2031544
APA StyleMcCollum, G., & Hanes, D. A. (2010). Symmetries of the Central Vestibular System: Forming Movements for Gravity and a Three-Dimensional World. Symmetry, 2(3), 1544-1558. https://doi.org/10.3390/sym2031544