Hartee Fock Symmetry Breaking Effects in La2CuO4: Hints for connecting the Mott and Slater Pictures and Pseudogap Prediction
Abstract
:1. Introduction
2. Rotational Invariant Hartree-Fock Method
2.1. , and symmetry restrictions
3. Tight Binding Electron Model: "Removing Symmetries"
3.1. Model for the Cu-O planes
3.2. Translations on the sublattices
3.3. Tight-Binding basis
4. Matrix Problem and Solutions
4.1. Tight-Binding representation
4.2. Maximally translational symmetric solutions
4.3. Insulating and antiferromagnetic solutions
4.4. Paramagnetic solution showing a pseudogap
5. Conclusions
- The isolator gap magnitude diminishes with the increasing of the screening constant .
- The antiferromagnetic structure the HF orbitals increases when the states approach the Fermi level. In addition, the size of the outlying region in which antiferromagnetism persists depends on the screening created by the effective environment . That is, by increasing screening, the size of the antiferromagnetic region reduces. Thus, the idea arises that after doping with holes (that is, solving for HF solutions not at half filling condition as it is done here) the antiferromagnetic zone, which is precisely concentrated near the Fermi level could be annihilated, producing in this way a phase transition to a non globally magnetically ordered ground state. This possibility indicates a way to describe the normal state properties of the HTc superconductors through a simple HF study.
- The magnetic moments per cell which are evaluated show a modular value of 0.67 , which is close to the measured moments in LaCuO and interestingly almost coincide with the measured result of 0.68 for the Cu sites in the 3D solid CuO [34].
- The magnitude of the predicted maximal value of the pseudogap is of the order of 100 . This result is close to the range 100-200 which is experimentally detected through ARPES for the pseudogap in the zero doping limit for LaCuO [15,35,36,37]. The comparison of the filled band spectra of the IAF and PPG states suggests that under a relatively small doping the energy of both states could evolve to coincidence. A decreasing evolution with doping can be expected from future temperature and doping dependent HF pseudogap evaluations. Thus, assuming that the maximal value of the obtained pseudogap describes the experimentally determined pseudogap temperature , a description of the observed decaying behavior of for increasing doping is suggested. [15].
- Similarly as it happens for the antiferromagnetic character of the IAF ground state, the difference between the one particle energies of IAF and the PPG (PM) solutions is larger for orbitals closer to the Fermi surface. That is, it happens for the electrons with more energy and consequently the first to disappear under doping with holes. Therefore, this outcome further supports the possibility to describe a crossing of the energies of the IAF and PPG states under doping.k
- The pseudogap magnitude diminishes with the increasing of the screening constant . This property, and the fact that the set of parameters were non univocally fixed (to reproduce Matheiss results for the single band crossing the Fermi level in [22]) leads to the opportunity of a better determination of the parameters to match a larger set of observed physical properties of LaCuO.
- At the limit considered here, the states PM and PPG were identical. The difference between them only appears at the excitations of the system. Thus, the removal of some symmetry restrictions defines new properties for unoccupied single particle states. It seems feasible that it could be possible to obtain a gap instead of a pseudogap in other materials, even in the absence of magnetic order. Such an outcome could show the ability of a properly formulated HF description to describe general kinds of Mott insulators, being or not magnetically ordered.
- To generalize the discussion in order to introduce the doping with holes and temperature as new parameters. This will allow to investigate the effects of these parameters on the determined HF states. Of particular interest in a first stage appears the study of the crossing of the energies per particle of the IAF and PPG states under doping. As above remarked, this point could determine the AF destruction phase transition. Furthermore, the mean field study for even larger dopings could shed light on the superconducting phase change.
- To compute the temperature and doping dependent electron Green function of the system, and use it to evaluate the effective polarization of the LaCuO in the obtained states.
- With the polarization results in hand, it could be possible to attempt solving the Bethe-Salpeter equation for two holes in the HF ground state, to find whether or not it is possible to decide about the existence of preformed Cooper Pairs in the HF model under finite doping and temperature. The possibility for their existence was suggested by the results of Ref. [46], in which it was argued that a strong 2D-screening of the Coulomb interaction is created by a half filled band of tight binding electrons.
- Finally, we intend to follow the hints given by the ideas exposed here to clarify the debate between the Mott and Slater pictures in connection with the electronic structure of transition metal oxides.
Acknowledgements
References
- Mott, N.F. The Basis of the Electron Theory of Metals, with Special Reference to the Transition Metals. Proc. Phys. Soc. 1949, A62, 416–422. [Google Scholar] [CrossRef]
- Slater, J.C. A Simplification of the Hartree-Fock Method. Phys. Rev. 1951, 81, 385–390. [Google Scholar] [CrossRef]
- Mott, N.F.; Peierls, R. Discussion of the Paper by de Boer and Verwey. Proc. Phys. Soc. 1937, A49, 72–73. [Google Scholar] [CrossRef]
- Hubbard, F. Electron Correlations in Narrow Energy Bands. Proc. R. Soc. 1963, A276, 238–257. [Google Scholar]
- Anderson, P.W. The Resonating Valence Bond State in La2CuO4 and Superconductivity. Science 1987, 235, 1196–1198. [Google Scholar] [CrossRef] [PubMed]
- Anderson, P.W. New Approach to the Theory of Superexchange Interactions. Phys. Rev. 1959, 115, 2–13. [Google Scholar] [CrossRef]
- Kohn, W. Theory of the Insulating State. Phys. Rev. 1964, 133, A171–A181. [Google Scholar] [CrossRef]
- Brinkman, W.F.; Rice, T.M. Application of Gutzwiller’s Variational Method to the Metal-Insulator Transition. Phys. Rev. 1970, B2, 4302–4304. [Google Scholar] [CrossRef]
- Gutzwiller, M. Effect of Correlation on the Ferromagnetism of Transition Metals. Phys. Rev. 1964, 134, A923–A941. [Google Scholar] [CrossRef]
- Gutzwiller, M. Correlation of Electrons in a Narrow S Band. Phys. Rev. 1965, 137, A1726–A1735. [Google Scholar] [CrossRef]
- Imada, M. Metal-Insulator Transitions. Rev. Mod. Phys. 1998, 70, 1039–1263. [Google Scholar] [CrossRef]
- Dagotto, E. Correlated Electrons in High-temperature Superconductors. Rev. Mod. Phys. 1994, 66, 763–840. [Google Scholar] [CrossRef]
- Yanase, Y.; Jujo, T.; Nomura, T.; Ikeda, H.; Hotta, T.; Yamada, K. Theory of Superconductivity in Strongly Correlated Electron Systems. Phys. Rep. 2003, 387, 1–149. [Google Scholar] [CrossRef]
- Van Harlingen, D.J. Phase-sensitive Tests of the Symmetry of the Pairing State in the High-temperature Superconductors: Evidence for dx2−y2 Symmetry. Rev. Mod. Phys. 1995, 67, 515–535. [Google Scholar] [CrossRef]
- Damascelli, A.; Hussain, Z.; Shen, Z-X. Angle-resolved Photoemission Studies of the Cuprate Superconductors. Rev. Mod. Phys. 2003, 75, 473–541. [Google Scholar] [CrossRef]
- Bednorz, J.G.; Müller, K.A. Perovskite-type Oxides-The New Approach to High-Tc Superconductivity. Rev. Mod. Phys. 1987, 60, 473–541. [Google Scholar] [CrossRef]
- Burns, G. High-Temperature Superconductivity; Academic Press: New York, NY, USA, 1992. [Google Scholar]
- Almasan, C.; Maple, M.B. Chemistry of High Temperature Superconductors; World Scientific: Singapore, 1991. [Google Scholar]
- Mott, N.F. Metal-Insulator Transition; Taylor and Francis: Philadelphia, USA, 1990. [Google Scholar]
- Fradkin, E. Field Theories of Condensed Matter; Addison Wesley Publishing Company: Redwood City, CA, USA, 1991. [Google Scholar]
- Terakura, K.; Williams, A.R.; Oguchi, T.; Kubler, J. Transition-Metal Monoxides: Band or Mott Insulators. Phys. Rev. Lett. 1984, 52, 1830–1833. [Google Scholar] [CrossRef]
- Mattheiss, L.F. Electronic Band Properties and Superconductivity in La2−yXyCuO4. Phys. Rev. Lett. 1987, 58, 1028–1030. [Google Scholar] [CrossRef]
- Kohn, W.; Sham, L.J. Self-Consistent Equations Including Exchange and Correlation Effects. Phys. Rev. 1965, 140, A1133–A1138. [Google Scholar] [CrossRef]
- Cabo-Bizet, A.; Cabo Montes de Oca, A. Spontaneous Symmetry Breaking Approach to La2CuO4 Properties: Hints for Matching the Mott and Slater Pictures. Phys. Lett. 2009, A 373, 1865–1869. [Google Scholar] [CrossRef]
- Dirac, P.A.M. Note on Exchange Phenomena in the Thomas Atom. Proc. Cambridge. Phil. Soc. 1930, 26, 376–385. [Google Scholar] [CrossRef]
- Kubler, J.; Hock, K.-H.; Sticth, J.; Williams, A. R. Density Functional Theory of Non-collinear Magnetism. J. Phys. F: Met. Phys. 1988, 18, 469–483. [Google Scholar] [CrossRef]
- Nordstrom, L.; Singh, J. S. Noncollinear Intra-atomic Magnetism. Phys. Rev. Lett. 1996, 76, 4420–4423. [Google Scholar] [CrossRef] [PubMed]
- Oda, T.; Pasquarello, A.; Car, R. Fully Unconstrained Approach to Noncollinear Magnetism: Application to Small Fe Clusters. Phys. Rev. Lett. 1998, 80, 3622–3625. [Google Scholar] [CrossRef]
- Bylander, D. M.; Kleinman, L. Full Potential ab initio Calculations of Spiral Spin Density Waves in fcc Fe. Phys. Rev. 1998, B 58, 9207–9211. [Google Scholar] [CrossRef]
- Gebauer, R.; Serra, S.; Chiarotti, G.L.; Scandolo, S.; Baroni, S.; Tosatti, E. Noncolinear Spin Polarization from Frustrated Antiferromagnetism: A Possible Scenario for Molecular Oxygen at High Pressure. Phys. Rev. 2000, B 61, 6145–6149. [Google Scholar] [CrossRef]
- Fukutome, H. Unrestricted Hartree-Fock Theory and its Applications to Molecules and Chemical Reactions. Int. J. Quantum Chem. 1981, 20, 955–1065. [Google Scholar] [CrossRef]
- Fazekas, P. Lecture Notes on Electron Correlation and Magnetism; World Scientific: Singapore, 1999. [Google Scholar]
- Slater, J.C. Quantum Theory of Atomic Structure; Dover Publications Inc.: Mineola, NY, USA; Volume v. 2, 1960. [Google Scholar]
- Pickett, W.E. Electronic Structure of the High-temperature Oxide Superconductors. Rev. Mod. Phys. 1989, 61, 433–512. [Google Scholar] [CrossRef]
- Ino, A.; Mizokawa, T.; Kobayashi, K.; Fujimori, A.; Sasagawa, T.; Kimura, T.; Kishio, K.; Tamasaku, K.; Eisaki, H.; Uchida, S. Doping Dependent Density of States and Pseudogap Behavior in La2−xSrxCuO4. Phys. Rev. Lett. 1998, 81, 2124–2127. [Google Scholar] [CrossRef]
- Ino, A.; Kim, C.; Nakamura, M.; Yoshida, T.; Mizokawa, T.; Shen, Z.-X.; Kakeshita, T.; Eisaki, H.; Uchida, S. Doping-dependent Evolution of the Electronic Structure of La(2−x)SrxCuO4 in the Superconducting and Metallic Phases. Phys. Rev. 2002, B 65, 094504(1-11). [Google Scholar]
- Yoshida, T.; Zhou, X.J.; Nakamura, M.; Kellar, S.A.; Bogdanov, P.V.; Lu, E.D.; Lanzara, A.; Hussain, Z.; Ino, A.; Mizokawa, T.; Fujimori, A.; Eisaki, H.; Kim, C.; Shen, Z.-X.; Kakeshita, T.; Uchida, S. Electron Like Fermi Surface and Remnant (π,0) Feature in Overdoped La1.78Sr0.22CuO4. Phys. Rev. 2001, B 63, 220501(4). [Google Scholar]
- Loktev, V.M.; Quick, R.M.; Sharapov, S.G. Phase Fluctuations and Pseudogap Phenomena. Phys. Rep. 2001, 349, 1–123. [Google Scholar] [CrossRef]
- Gabovich, A.M.; Voitenko, A.I.; Ausloos, M. Charge- and Spin-density Waves in Existing Superconductors: Competition Between Cooper Pairing and Peierls or Excitonic Intabilities. Phys. Rep. 2002, 367, 583–709. [Google Scholar] [CrossRef]
- Powel, B.J. An Introduction to Effective Low-Energy Hamiltonians in Condensed Matter Physics and Chemistry. arXiv:0906.1640v6.2009; physics.chem-ph. [Google Scholar]
- Blaizot, J-P.; Ripka, G. Quantum Theory of Finite Systems; MIT Press: Cambridge, Massachusetts, USA, 1986. [Google Scholar]
- Yannouleas, C.; Landman, U. Symmetry Breaking and Quantum Correlations in Finite Systems: Studies of Quantum Dots and Ultracold Bose Gases and Related Nuclear and Chemical Bonds. Rep. Prog. Phys. 2007, 70, 2067–2148. [Google Scholar] [CrossRef]
- Yannouleas, C.; Landman, U. Spontaneous Symmetry Breaking in Single and Molecular Quantum Dots. Phys. Rev. Lett. 1999, 82, 5325–5328. [Google Scholar] [CrossRef]
- Szabo, A.; Ostlund, N. Modern Quantum Chemistry: Introduction to Advanced Electronic Structure Theory; Dover Publications Inc.: Mineola, New York, USA, 1989. [Google Scholar]
- Fetter, A.L.; Walecka, J.D. Quantum Theory of Many Particle Physics; McGraw-Hill, Inc.: New York, NY, USA, 1971. [Google Scholar]
- Ponce, Y.V.; Oliva, D.; Cabo, A. About the Role of 2D Screening in High Temperature Superconductivity. Phys. Lett. 2006, A353, 255–268. [Google Scholar] [CrossRef]
A.. Matrix elements
A.1.. Brackets notation
A.2.. Dimensionless definitions
A.3.. Other definitions and properties
A.4.. Matrix Elements
A.5.. Reducing the order of some integrals
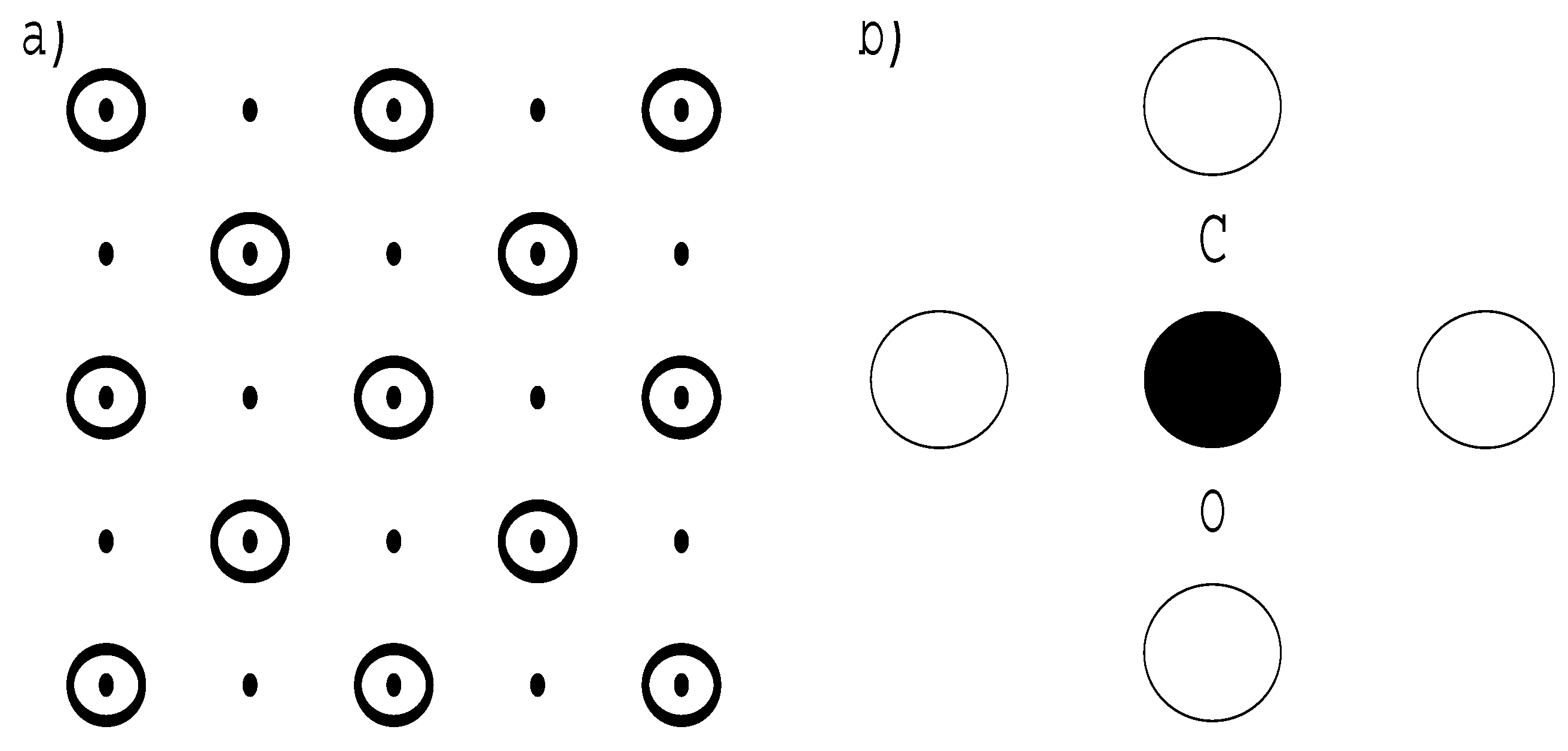

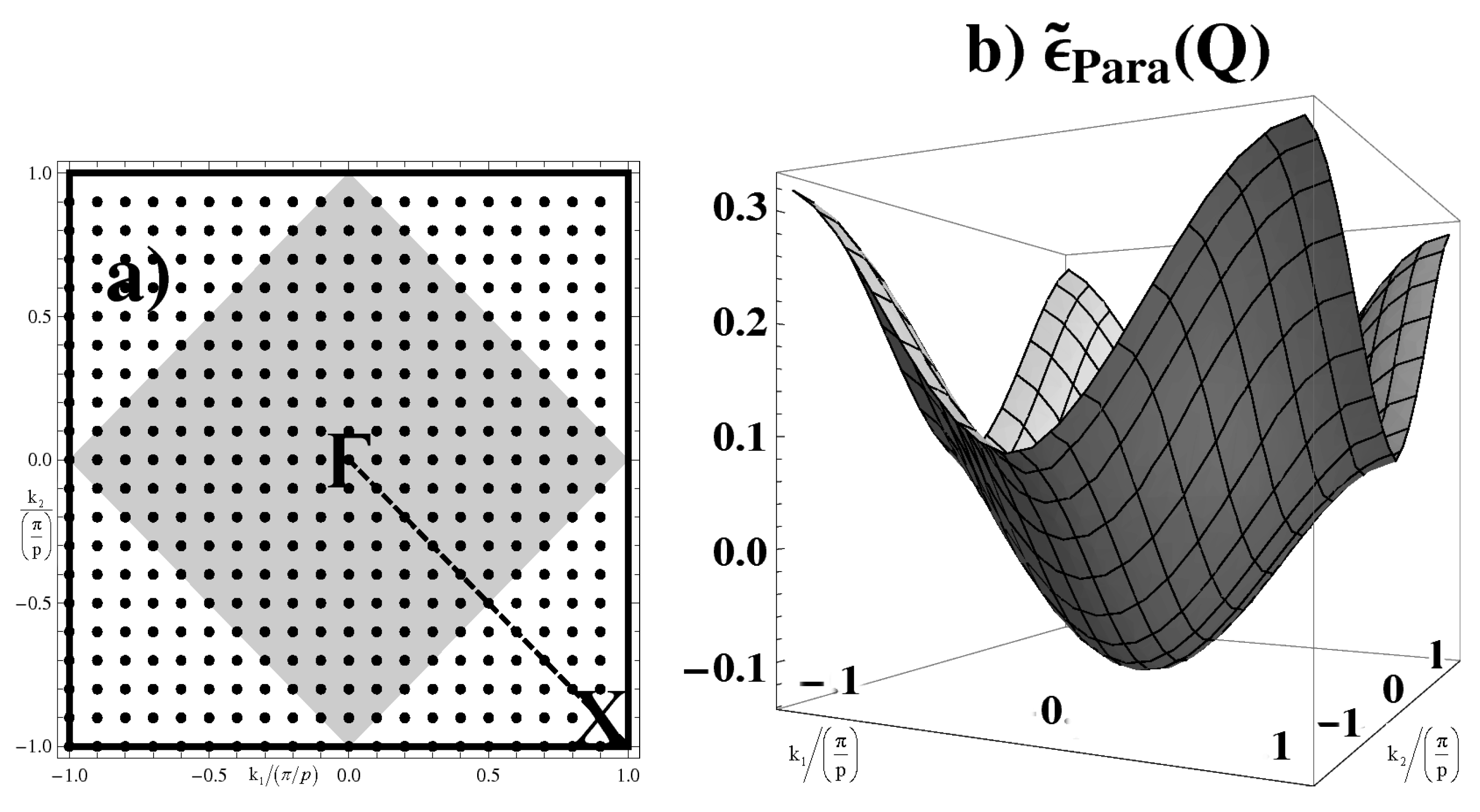
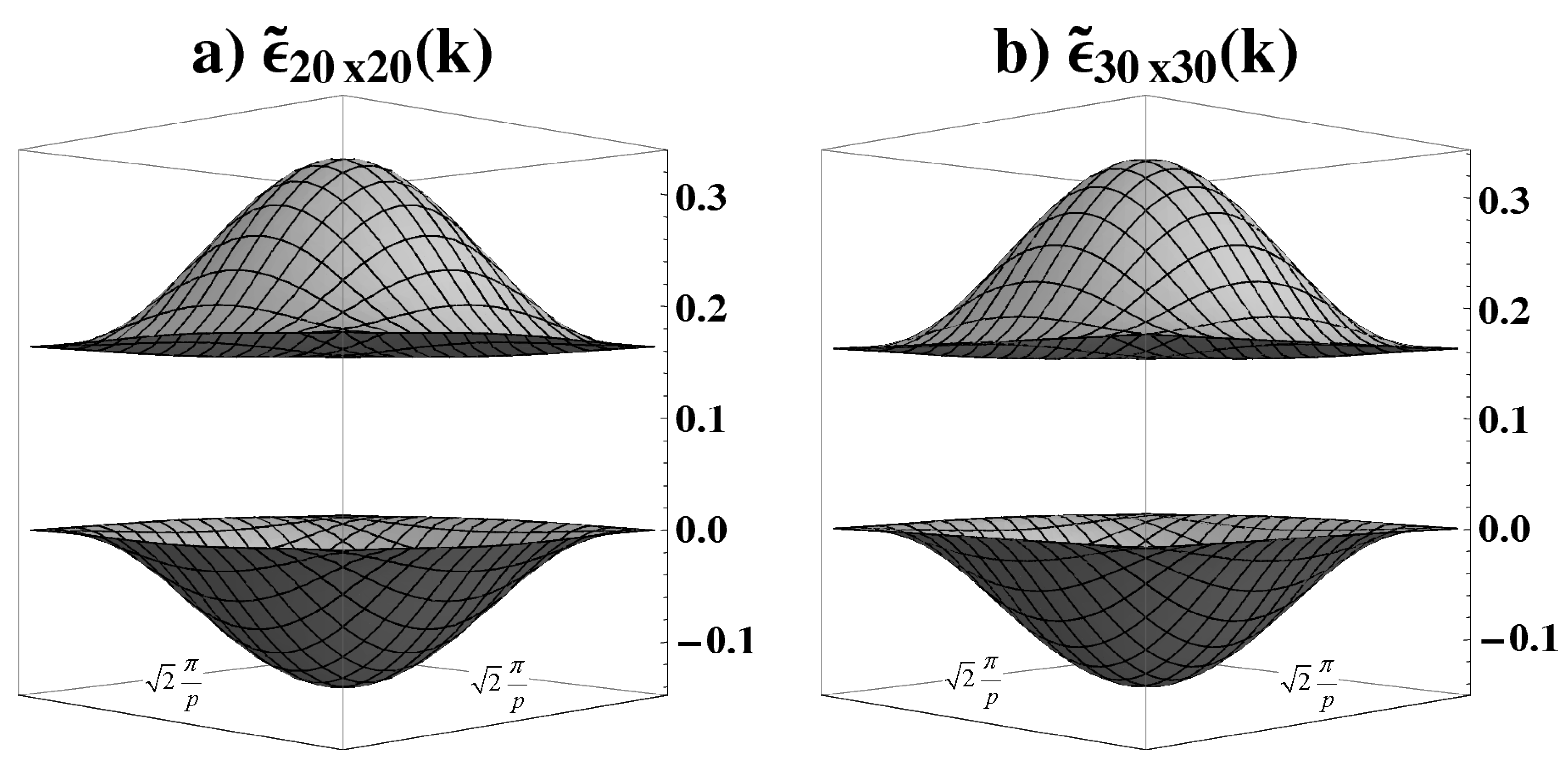
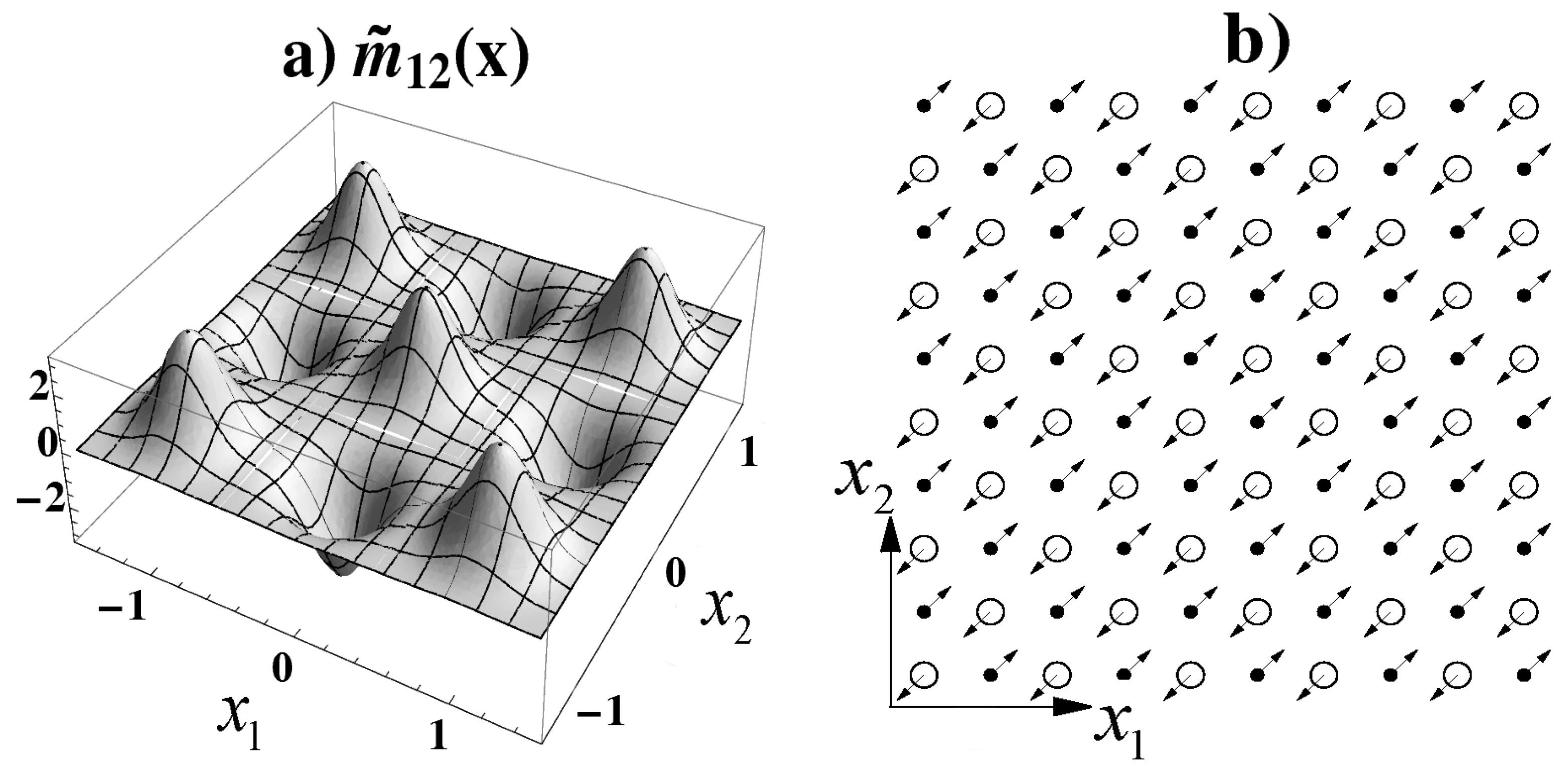
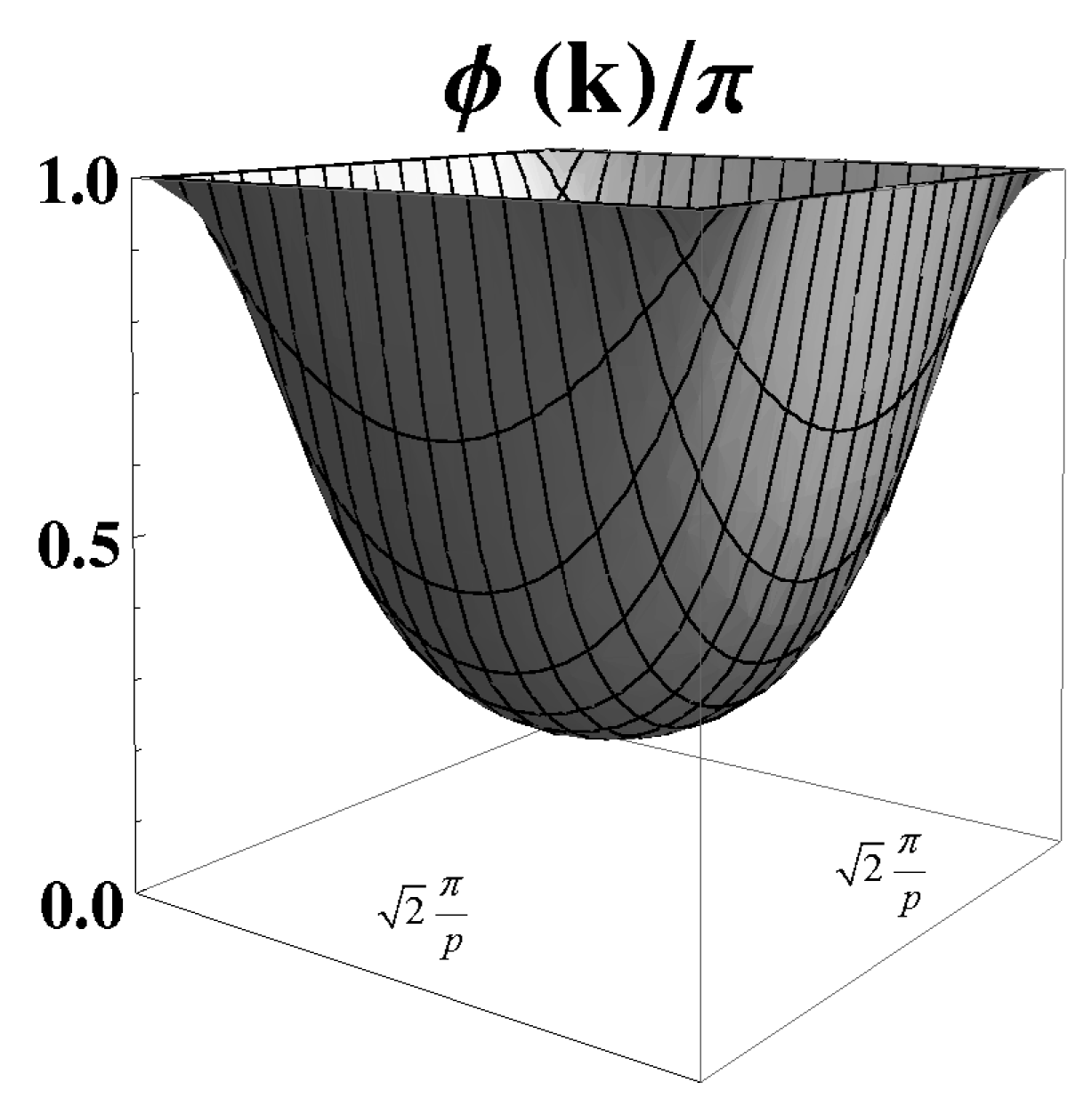
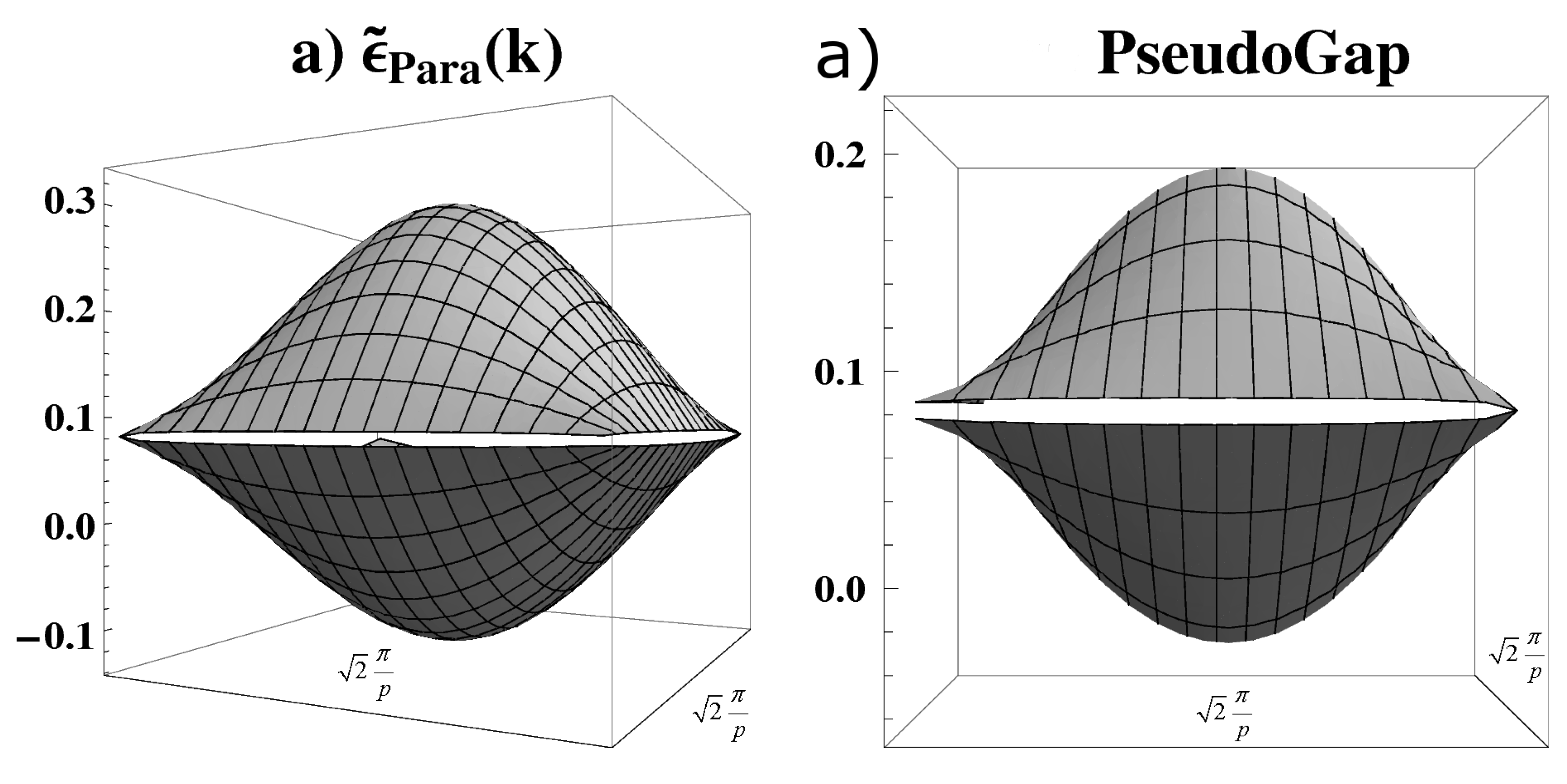
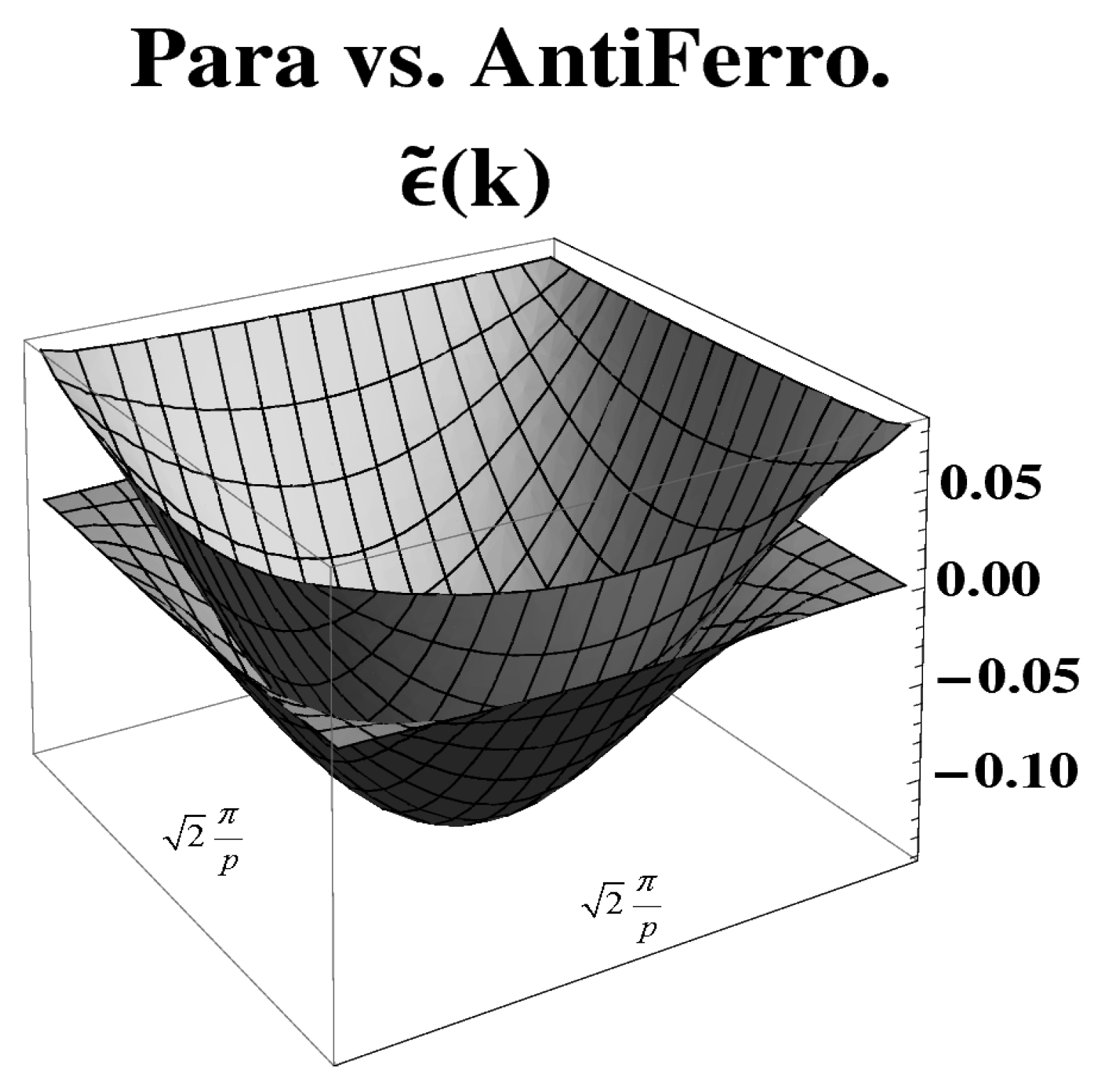
| State | IAF | PM | PPG |
|---|---|---|---|
| E (eV) | 0.0 | +0.076 | +0.076 |
© 2010 by the authors; licensee Molecular Diversity Preservation International, Basel, Switzerland. This article is an open-access article distributed under the terms and conditions of the Creative Commons Attribution license http://creativecommons.org/licenses/by/3.0/.
Share and Cite
Cabo-Bizet, A.; De Oca, A.C.M. Hartee Fock Symmetry Breaking Effects in La2CuO4: Hints for connecting the Mott and Slater Pictures and Pseudogap Prediction. Symmetry 2010, 2, 388-417. https://doi.org/10.3390/sym2010388
Cabo-Bizet A, De Oca ACM. Hartee Fock Symmetry Breaking Effects in La2CuO4: Hints for connecting the Mott and Slater Pictures and Pseudogap Prediction. Symmetry. 2010; 2(1):388-417. https://doi.org/10.3390/sym2010388
Chicago/Turabian StyleCabo-Bizet, Alejandro, and Alejandro Cabo Montes De Oca. 2010. "Hartee Fock Symmetry Breaking Effects in La2CuO4: Hints for connecting the Mott and Slater Pictures and Pseudogap Prediction" Symmetry 2, no. 1: 388-417. https://doi.org/10.3390/sym2010388
APA StyleCabo-Bizet, A., & De Oca, A. C. M. (2010). Hartee Fock Symmetry Breaking Effects in La2CuO4: Hints for connecting the Mott and Slater Pictures and Pseudogap Prediction. Symmetry, 2(1), 388-417. https://doi.org/10.3390/sym2010388




