Flexible Piezoresistive Sensor with High Stability Based on GO@PDMS-PU Porous Structure

Abstract

In recent years, flexible piezoresistive sensors based on polydimethylsiloxane (PDMS) matrix materials have developed rapidly, showing broad application prospects in fields such as human motion monitoring, electronic skin, and intelligent robotics. However, achieving a balance between structural durability and fabrication simplicity remains challenging. Traditional methods for preparing PDMS flexible substrates with high porosity and high stability often require complex, costly processes. Breaking through the constraints of conventional material systems, this study innovatively combines the high elasticity of polydimethylsiloxane (PDMS) with the stochastically distributed porous topology of a sponge-derived biotemplate through biomimetic templating replication technology, fabricating a heterogeneous composite system with an architecturally asymmetric spatial network. After 5000 loading cycles, uncoated samples experienced a thickness reduction of 7.0 mm, while PDMS-coated samples showed minimal thickness changes (2.0–3.0 mm), positively correlated with curing agent content (5:1 to 20:1). The 5:1 ratio sample demonstrated exceptional mechanical stability. As evidenced, the PDMS film-encapsulated architecturally asymmetric spatial network demonstrates superior stress dissipation efficacy, effectively mitigating stress concentration phenomena inherent to symmetric configurations that induce matrix fracture, thereby achieving optimal mechanical stability. Compared to the pre-test resistance distribution of 10–248 Ω, after 5000 cyclic loading cycles, the uncoated samples exhibited a narrowed resistance range of 10–50 Ω, while PDMS-coated samples maintained a broader resistance range (10–240 Ω) as the curing agent ratio increased (from 20:1 to 5:1), demonstrating that increasing the curing agent ratio helps maintain conductive network stability. The 5:1 ratio sample displayed the lowest resistance variation rate attenuation—only 3% after 5000 cycles (vs. 80% for uncoated samples)—and consistently minimal attenuation at all stages, validating superior electrical stability. Under 0–6 kPa pressure, the 5:1 ratio device maintained a linear sensitivity of 0.157 kPa⁻¹, outperforming some existing works. Human motion monitoring experiments further confirmed its reliable signal output. Furthermore, the architecturally asymmetric spatial network of the device enables superior conformability to complex curvilinear geometries, leveraging its structural anisotropy to achieve seamless interfacial adaptation. By synergistically optimizing material composition and structural design, this study provides a novel technical method for developing highly durable flexible electronic devices.

1. Introduction

With the development of informatization and intelligentization in modern life, the demand for flexible sensors in wearable monitoring devices and human–machine interaction continues to grow. Flexible piezoresistive sensors, which operate based on the piezoresistive effect, detect external forces by inducing deformation in their sensitive materials. This deformation alters the distribution and contact state of internal conductive components, resulting in predictable changes in electrical resistance. Such sensors are favored for their simple structure, low fabrication cost, and ease of operation [1,2]. A critical component of flexible piezoresistive sensors is the integration of conductive materials with highly compliant substrate materials. The choice of substrate directly determines the sensor’s tensile/compressive performance, flexibility, and durability, as flexible substrates enable a wide range of deformations. Unlike traditional medical devices and wearable healthcare electronics, flexible pressure sensors demonstrate superior safety and comfort in human-integrated applications due to their exceptional flexibility and highly conformal contact with measured surfaces. These sensors can be safely and comfortably attached to human skin or even implanted within the body, enabling real-time and accurate monitoring of various physiological states. This capability provides valuable early detection and diagnostic foundations for disease prevention and health management [3,4,5]. Vaezi et al. [6] presented a wearable antenna which was fabricated on a completely flexible substrate for medical application, with circular polarization, high-gain, and low-profile features. It provides more than 75% measured bandwidth around the 2.4 GHz ISM band with an excellent circular gain.

Commonly used substrates include polymer materials such as polydimethylsiloxane (PDMS) [7,8,9], polyimide (PI) [10], polyurethane (PU) [11,12], thermoplastic polyurethane (TPU) [13], and polyethylene terephthalate (PET) [14]. Zhao et al. [15] prepared pressure sensors based on a solution of styrene–butadiene–styrene (SBS) and reduced graphene oxide (rGO) dispersion. The prepared sensors exhibit fast and slightly destructive resilience after unloading at low compressive strains, along with high electrical conductivity and sensitivity, and short signal response and recovery times. Wang et al. [16] developed a multifunctional composite sensor enabled by the combination of ecoflex, spacer fabric, and graphene-based aerogel. It showed a pressure sensitivity of 0.125 kPa⁻¹ at the pressure range of 0~15 kPa, with the advantage of a simple structure, large pressure strain, high sensitivity, and ease of fabrication. Yang et al. [17] constructed a strain-insensitive stretchable pressure sensor with a serpentine nested structure, fabricated through laser engraving of graphene. The obtained sensor demonstrated high sensitivity (1.41 × 10⁻¹ kPa⁻¹), a broad sensing range (0−50 kPa), a short response time (100 ms), a low detection limit (100 Pa), and good cyclic stability (3000@10 kPa). These polymeric substrates endow sensors with excellent stretchability and adaptability. Among them, PDMS stands out due to its low elastic modulus, high elasticity, moldability, transparency, thermal stability, and corrosion resistance, making it the most widely adopted substrate for flexible sensors. To fabricate 3D PDMS architectures, methods such as sacrificial templating, 3D printing, and chemical foaming are commonly employed to create foam-like or spongy structures [18,19,20,21,22,23].

Sacrificial templating is currently the most widely reported method for preparing PDMS-based active materials. This approach involves creating composite materials using pore-forming agents (templates) such as salt (NaCl) or sugar, followed by template removal to yield porous structures. For instance, Song et al. [24] proposed a bio-inspired hierarchical gradient architecture (HGA) using NaCl as a sacrificial template. They constructed a four-layer gradient structure by stepwise curing of CNTs/PDMS composites combined with a PLA mold to form hexagonal arrays, ultimately dissolving NaCl to obtain porous materials. Mu et al. [25] fabricated a PDMS/carbon nanocapsule (PDMS/CNCs) composite with dual-scale porous structures via templating. A mixture of NaCl/CNCs powder was blade-coated onto a PDMS prepolymer surface, and subsequent curing and ultrasonic leaching of NaCl templates generated a composite film featuring both CNC-derived nanoscale cavities and microscale pores. This sensor synergized the high conductivity and mechanical strength of CNCs with PDMS flexibility, achieving wide-range pressure detection and high sensitivity, thus addressing the trade-off between sensitivity and range in traditional flexible sensors. Jung et al. [26] developed a 3D compressible porous CNT/PDMS composite using a sugar templating method. A homogeneous mixture of sugar, deionized water, and CNTs was evaporated at room temperature to form partially fused sugar cubes. These cubes were infiltrated with PDMS precursor, cured, and then dissolved in water, followed by air-drying to finalize the porous structure. Chen et al. [27] engineered ridge-like microporous PDMS sponges via sugar templating. The sponges were modified with polydopamine (PDA) to interface Ag nanoparticles (AgNPs) and reduced graphene oxide (rGO) conductive layers, followed by perfluorosilane treatment to impart superhydrophobicity. The resulting piezoresistive sensor exhibited a sensitivity of 0.0132 kPa⁻¹ within 0–40 kPa, with its unique microstructure and superhydrophobicity enhancing environmental adaptability for broad-range pressure monitoring. Zhai et al. [28] employed a sugar template–vacuum impregnation strategy to fabricate a CB/PDMS porous foam active layer. PDMS prepolymer was infiltrated into a sugar skeleton, cured, and then dissolved to form a 3D porous matrix. Ultrasonic-assisted loading of carbon black (CB) established a synergistic surface/bulk conductive network. Iglio et al. [29] utilized sugar templates to produce porous PDMS foam, followed by drop-casting CNT ink to create nanostructured CNT surface networks. Lo et al. [30] reported a multi-stimuli-responsive sensor capable of distinguishing pressure, strain, and temperature. This device used a low-cost dip-coating process to deposit poly (3,4-ethylenedioxythiophene)/polystyrene sulfonate (PEDOT:PSS) onto sugar-templated porous PDMS sponges. Wang et al. [31] coated multilayer graphene onto the inner walls of porous nickel (Ni) foam, embedded it in PDMS, etched away the Ni, and deposited gold (Au) nanoparticles to create a pressure-sensitive film. Dai et al. [32] addressed the sensitivity–mechanical performance imbalance caused by thick, low-porosity PDMS sponges by designing a bamboo-inspired hierarchical porous CNFs/PDMS foam (CPF). Using Ni foam as a template, a dual-step dip-coating/spin-coating/curing process with CNFs/PDMS suspension mimicked bamboo-like gradient pores. Acid etching removed the Ni, yielding a bio-inspired interpenetrating network. This sensor maintained stability over 10,000 cycles, overcoming the sensitivity–reliability trade-off.

However, there is a main contradiction for the current structural designs of PDMS-based flexible piezoresistive sensors: although high-porosity PDMS substrates exhibit excellent flexibility, achieving both porous architectures and high stability relies on complex fabrication processes (e.g., template etching, directional foaming), resulting in high costs and scalability limitations. Balancing structural durability with manufacturing simplicity has become the central challenge for advancing PDMS-based flexible piezoresistive sensors toward practical applications. In recent years, bio-inspired structural design has offered novel insights for optimizing flexible electronic devices. Natural systems, such as spider webs and honeycombs, unify high strength and resilience through synergistic interactions between hierarchical porous structures and elastic matrices. Inspired by this mechanism, we propose a bio-inspired composite construction strategy employing sponge-templated replication technology, which synergistically integrates the stochastically organized 3D porous topology of marine-derived biotemplates with the hyperelastic characteristics of polydimethylsiloxane (PDMS). Through precision replication of the sponge template’s pore structural hierarchy, this methodology engineers PDMS-based composite systems that simultaneously exhibit asymmetric spatial network percolation and coupled mechano-electronic stability, thereby transcending the performance constraints of conventional monolithic material systems.

2. Materials and Methods

2.1. Computer Simulation

The finite element method (FEM) was employed to systematically investigate the mechanical properties of porous-structured matrix materials with varying elastic modulus parameters, aiming to evaluate the influence of elastic modulus on the substrate’s performance.

2.1.1. Establishment of Finite Element Model

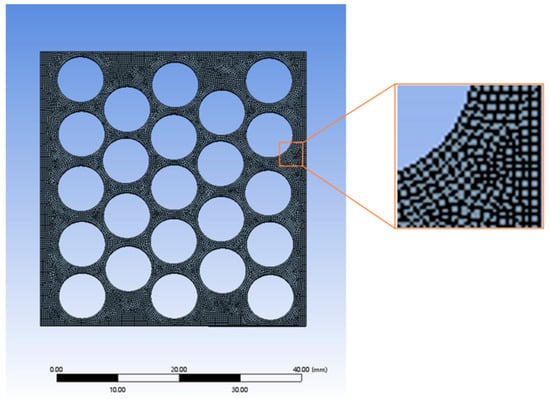
To investigate the mechanical properties of the matrix material, a static structural analysis was performed in ANSYS Workbench (version 2021R2, https://www.ansys.com/) [17,33]. Since the deformations of the piezoresistive sensor under load are significantly larger in the longitudinal (compressive) and transverse (tensile) directions compared to the out-of-plane direction, a 2D model was adopted to balance computational efficiency. The finite element model is illustrated in Figure 1. The model dimensions were set to 45 mm × 45 mm, with a uniform pore diameter of 3 mm to simplify calculations. The analysis object was defined as a flexible body, and its material parameters are listed in

Table 1. Material parameters.

Density (kg/m³)	Tensile Strength (MPa)	Open Porosity (%)	Elastic Modulus (MPa)	Poisson’s Ratio
15~60	0.2~1	80	60	0.3
			70
			80
			90

.

The element type was selected as quadrilateral-dominated, with a mesh size of 0.5 mm, resulting in 9816 quadrilateral-dominant elements and 31,954 nodes. Quadrilateral elements were primarily distributed near the model edges, while triangular elements were concentrated around the pores. To prevent mesh penetration during compression or tension, self-contact settings were applied to the pore structures, where both target and contact geometries were assigned to the same pore. A penalty-based frictional contact algorithm was employed with a friction coefficient of 0.2. Boundary conditions were set to fix the model’s bottom surface, while a controlled load was applied to the top surface to achieve 45% longitudinal deformation for four groups of models with varying elastic moduli. For tensile testing, equal and opposite loads were applied to the left and right ends of the model to ensure 45% transverse deformation across all groups. This configuration avoided computational termination caused by excessive mesh distortion beyond 45% strain.

2.1.2. Finite Element Results Analysis

In the static structural analysis, identical strain and deformation magnitudes were observed across materials with different elastic moduli due to consistent model deformation. However, the resulting stresses varied significantly depending on material parameters. Figure 2 illustrates the stress distribution diagrams of materials with different elastic moduli during compressive and tensile deformation processes. When the elastic modulus was 60 MPa, the overall compressive stress during deformation was 34 MPa, while the tensile stress reached 41 MPa. Increasing the elastic modulus to 70 MPa resulted in compressive and tensile stresses of 39 MPa and 48 MPa, respectively. At 80 MPa, these values further rose to 45 MPa (compression) and 55 MPa (tension). For the highest tested modulus of 90 MPa, the stresses reached 51 MPa under compression and 62 MPa under tension. The results demonstrate that stress during both compression and tension increases proportionally with elastic modulus. Under compressive loading, stress rose from 34 MPa to 51 MPa (a 50% increase), while tensile stress increased from 41 MPa to 62 MPa (a 51% increase), as shown in Figure 3. These findings indicate that materials with higher elastic moduli generate greater stress under identical deformation conditions, reflecting stronger resistance to deformation and enhanced structural durability. Consequently, improving the elastic modulus of the matrix material can effectively enhance the structural stability of the sensor.

2.2. Fabrication Methods

2.2.1. Experimental Materials

PDMS prepolymer and curing agent (Dow Corning 184), commercial polyurethane (PU) sponge (Guangzhou Jijie Commercial Trading Development Co., Ltd., Guangzhou, China), graphene powder (Shenzhen Suiheng Technology Co., Ltd., Shenzhen, China), natural graphite powder, NaNO₃, concentrated sulfuric acid, KMnO₄, 30% H₂O₂, ethanol, and deionized water were used in this study. (The above-mentioned chemicals are from Aladdin Co., Ltd., Shanghai, China).

2.2.2. Material Preparation Process

• Preparation of composite material for PDMS-PU sponge

PDMS solvent and curing agent were mixed in ratios of 5:1, 10:1, and 20:1, respectively, where the higher the ratio of curing agent, the greater the hardness of the material. After thoroughly stirring the mixed solution, the vacuum extraction method was used to remove bubbles. The PU sponge was immersed into the PDMS solution and thoroughly squeezed to ensure full contact between the sponge’s pores and the PDMS. Subsequently, the PDMS-coated PU sponge was removed from the solution and left to stand in a tray for several hours. Under gravitational force, excess PDMS solution drained away, fully restoring the sponge’s porous structure while leaving a thin PDMS film coating on the PU sponge. Then, it was dried at 80 °C for 2 h in a vacuum oven.

Since the curing agent ratio studied in this work showed minimal influence on the adhesion between PDMS and the PU sponge, the PDMS film thickness remained consistent across samples prepared using this process.

2.

Preparation of Graphene Oxide Conductive Material

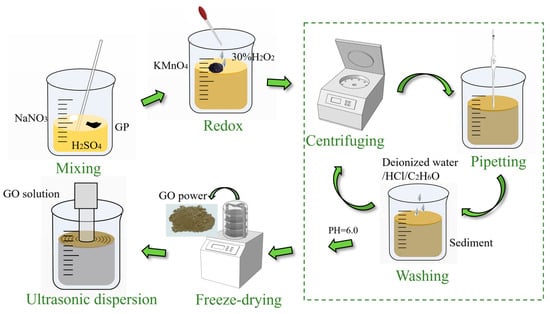
This study adopted a simplified preparation method by modifying and refining the traditional Hummers method. Through controlled experimental conditions and rationally designed preparation processes, the oxidation degree of graphene oxide (GO) was regulated to obtain high-quality products. A schematic diagram of the graphene oxide preparation process using the improved Hummers method is illustrated in Figure 4.

During the low-temperature mixing stage, 1 g of natural graphite powder, 2 g of sodium nitrate, and 25 mL of concentrated sulfuric acid were added to a beaker and thoroughly stirred to prepare for subsequent oxidation. In the oxidation stage, 4 g of ground potassium permanganate was slowly added under continuous stirring, followed by 24 h of stirring at room temperature. For the dilution stage, 50 mL of deionized water was first introduced while maintaining the temperature below 50 °C with 2 h of stirring, and then 150 mL of deionized water was added at once for further mixing. During the reduction stage, 10 mL of 30% hydrogen peroxide was dripped to eliminate residual potassium permanganate until gas evolution ceased and bright yellow precipitates formed, after which stirring was stopped and the mixture was left undisturbed for 24 h. In the separation and washing stage, 1000 mL each of hydrochloric acid, ethanol, and deionized water were prepared; the product was washed via centrifugation, repeatedly centrifuged, and rinsed with deionized water until the supernatant reached a pH of approximately 6.0, yielding a dark yellow viscous graphene oxide solution. For freeze-drying, the sample was lyophilized, crushed into powder for storage, and finally prepared into a graphene oxide conductive solution.

3.

GO@PDMS-PU Composite-Based Flexible Piezoresistive Sensor

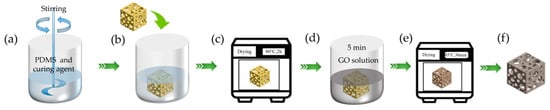
At room temperature, the samples were immersed in a graphene oxide (GO) solution for 5 min to uniformly coat the surfaces and pores. Excess solution was drained under gravity, and the samples were dried in a drying oven at 85 °C for 30 min. Figure 5 shows the flowchart for the preparation process of the GO@PDMS-PU-based flexible piezoresistive sensor, while Figure 6 presents the photograph of the porous composite-based flexible piezoresistive sensor (70 mm × 70 mm × 2 mm).

2.3. Testing and Characterization Methods

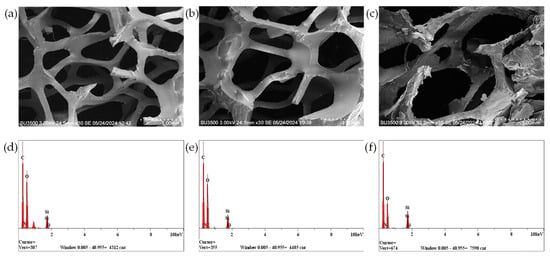
As shown in Figure 7a, the samples were sputter-coated with 5 nm gold and analyzed using a field-emission scanning electron microscope (FE-SEM, SU3500, Hitachi High-Tech Corporation, Tokyo, Japan) for morphological observation. Compositional analysis was performed using energy-dispersive X-ray spectroscopy (EDS, IXRF system, Hitachi High-Tech Corporation, Tokyo, Japan).

The mechanical properties of the flexible piezoresistive sensor were evaluated using a computer-controlled universal testing machine (ZQ-990B, Dongguan ZhiQu Precision Instruments Co., Ltd., Dongguan, China; Figure 7b, left). Electrical performance was measured with a high-precision multimeter (Keysight 34465A, Keysight Technologies, Santa Rosa, CA, USA; Figure 7b, right). The tensile–compression testing and electrical characterization setup are illustrated in Figure 7b. During testing, custom-made electrodes were placed on the upper and lower surfaces of the sensor. The sensor and electrodes were mounted on the testing machine platform, with the electrodes connected to the multimeter. Prior to testing, the starting point was defined as the position where the pressure head exerted zero force on the sensor. Cyclic compression tests were then conducted under dynamic loading, with applied loads and indenter speeds adjusted according to sample specifications.

3. Results and Discussion

This section analyzes the micro-morphology, composition, mechanical–electrical stability, and sensitivity of the prepared GO@PDMS-PU composite-based flexible piezoresistive sensors with different PDMS solvent-to-curing agent ratios.

3.1. Micro-Morphology and Composition Analysis

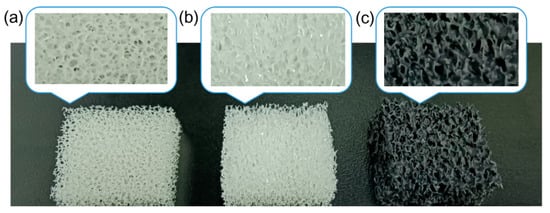
Figure 8a shows the SEM image of the untreated PU sponge sample. Figure 8b displays the SEM image of the PDMS-coated PU sponge semi-finished product (prior to conductive material coating). Although the PU sponge was wrapped with a PDMS film, the structure and morphology of the sample remained consistent with the original PU sponge due to the thin PDMS layer, exhibiting interconnected hexagons of varying sizes and a relatively smooth surface. Figure 8c presents the SEM image of the finished product after the dipping process. While the structural morphology showed no significant changes compared to earlier stages, the surface became rough. This roughness primarily arose from the flake-like stacking of graphene oxide (GO) on the surface and pores of the sample, with partial filling of internal cavities.

Figure 8d illustrates the composition of the PU sponge sample, primarily consisting of C, O, Si, and trace impurities. Figure 8e shows the composition of the PDMS-coated PU sponge. Since the outermost layer was PDMS, the main components remained C, O, and Si, with a notable increase in carbon content. After the dipping process (GO coating), Figure 8f reveals that the PDMS film was fully covered by GO. The elemental composition aligned with GO characteristics, dominated by C, O, and Si, along with minimal traces of impurities. Notably, the carbon content increased further post-treatment.

3.2. Mechanical Performance Analysis

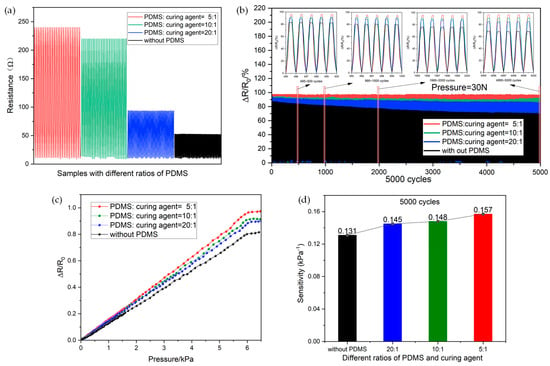
The mechanical properties were evaluated primarily using a universal testing machine for data acquisition. The test data were systematically analyzed and organized. The mechanical performance of the samples was tested under cyclic loading conditions. In the mechanical performance testing, due to the high flexibility of the matrix material and its thickness, the pores of samples were completely closed under a 30 N force. Therefore, 30 N was selected as the cyclic loading condition. At this load level, the samples demonstrated a stable input–output relationship.

Figure 9 illustrates the deformation curves of samples with different PDMS solvent-to-curing agent ratios under cyclic loading at 30 N after 5000 cycles. Experimental results demonstrate that the PDMS ratio significantly influences the material’s resistance to cyclic deformation, with the 5:1 (solvent/curing agent) ratio sample exhibiting the most superior mechanical stability.

After 5000 cyclic loading cycles, the uncoated (no PDMS) sample exhibited a deformation of 13.0 mm. As the curing agent ratio increased, the deformation decreased progressively: 12.5 mm for the 20:1 ratio sample, 11.0 mm for the 10:1 ratio sample, and 8.8 mm for the 5:1 ratio sample, which demonstrated the lowest deformation and stable anti-deformation capability.

In terms of recovery capacity, the thickness of the uncoated sample decreased by 7.0 mm compared to its pre-test state after 5000 cycles. In contrast, PDMS-coated samples showed significantly smaller thickness changes (2.0–3.0 mm), with these changes positively correlated to the curing agent content (5:1 to 20:1). Notably, the 5:1 ratio sample exhibited a thickness change of less than 2.0 mm, achieving a 25-fold improvement in recovery capacity over uncoated samples.

Evidently, as the curing agent proportion increases, the material’s anti-deformation capability and recovery capacity exhibit a pronounced upward trend, highlighting the enhanced mechanical stability of PDMS coatings under long-term cyclic loading. This divergence in mechanical response may relate to the crosslinking density of PDMS: the dense crosslinked network formed at the 5:1 ratio effectively disperses stress, whereas higher solvent ratios lead to internal stress concentration and accumulation of plastic deformation. The latest research indicates that the architecturally asymmetric spatial network inherent in the synthesized specimens demonstrates superior stress delocalization capacity [34], effectively mitigating stress concentration-induced fracture propagation observed in symmetric architectures. Experimental validation confirms that systematic optimization of PDMS stoichiometry serves as a robust strategy to enhance mechanical stability coefficients by modulating crosslinking density gradient distribution, thereby overcoming the performance ceiling of homogeneous material systems.

3.3. Electrical Performance Analysis

Figure 10 illustrates the evolution of electrical characteristics for samples with different PDMS solvent-to-curing agent ratios under cyclic loading at 30 N over 5000 cycles. A dual-dimensional analysis of resistance variation rate and distribution range reveals that the PDMS encapsulation layer significantly enhances the electrical stability of the material.

Compared to the pre-test resistance distribution of 10–248 Ω after 5000 cyclic loading cycles, the uncoated samples exhibited a narrowed resistance range of 10–50 Ω, while PDMS-coated samples demonstrated significantly stabilized resistance distributions: 10–95 Ω for the 20:1 ratio, 10–220 Ω for the 10:1 ratio, and 10–240 Ω for the optimal 5:1 ratio (as shown in Figure 10a). This gradient variation in resistance distribution indicates that due to the inherent characteristics of the asymmetric network structure, increasing the curing agent proportion in the PDMS matrix (corresponding to lower solvent-to-curing agent ratios) more effectively preserves the conductive network of the composite under cyclic loading, thereby maintaining more stable electrical performance under mechanical stress. From the attenuation trend of resistance variation rate (Figure 10b), the 5:1 ratio sample exhibited the highest stability: after 5000 cycles, its resistance variation rate attenuation was only 3%, significantly lower than the nearly 80% attenuation observed in uncoated samples.

3.4. Sensitivity Analysis

After 5000 pressure cycles (0–6 kPa), the sensing performance of samples with different PDMS solvent-to-curing agent ratios exhibited significant differences, as shown in Figure 10c,d. Experimental data indicate that as the PDMS solvent-to-curing agent ratio was progressively optimized from 20:1 to 5:1, both the resistance variation rate and sensitivity of the sensors increased systematically: the uncoated sample demonstrated an 80% resistance variation rate and a sensitivity of 0.131 kPa⁻¹; the PDMS-coated 20:1 ratio sample showed improved performance (89% resistance variation rate and 0.145 kPa⁻¹ sensitivity); the 10:1 ratio sample further enhanced these values to 91% and 0.148 kPa⁻¹, respectively; while the optimized 5:1 ratio sample achieved the highest performance with a 96% resistance variation rate and 0.157 kPa⁻¹ sensitivity, representing improvements of approximately 20% and 17%, respectively, compared to the uncoated sample. This trend suggests that reducing the PDMS solvent ratio (i.e., increasing the curing agent content) effectively enhances the mechanical stability and pressure-responsive characteristics of the sensing material. Among all ratios, the 5:1 sample demonstrated the most outstanding pressure sensing performance in long-term cyclic testing. This research has certain advantages compared to some similar works (as shown in Table 2). In this study, the sensitivity and cycling stability of the sensor were synergistically improved by adjusting the curing agent content in the PDMS coating from a ratio of 20:1 to 5:1. However, further increasing the curing agent content resulted in a continued enhancement of mechanical stability but reduced sensitivity performance. This degradation in sensitivity was attributed to the excessively rigid PDMS coating caused by the higher curing agent content and the altered adhesion strength between the conductive materials and the PDMS coating. In practical applications, the balance between sensitivity and mechanical stability must be carefully optimized according to specific operational requirements.

Table 2. Comparison of sensor sensitivity in related works.

Research Work	Materials	Sensitivity (kPa−1)	Stable Cycle Times
Reference [35]	MWNT-rGO@PU	0.022~0.088	5000
Reference [36]	CNTs/TPU/SR	0.013~0.032	1000
Reference [37]	PDMS/CNT	0.111	10,000
Reference [38]	CMF/CNTs	0.11	3000
Reference [39]	PU@AgNWs@SLG	0.009~0.011	3000
Reference [40]	PDMS/PDA/MWCNT	0.020~0.202	8000
Reference [41]	GNPs/PDMS	0.0602	5000
Reference [42]	Graphene/PDMS	0.075	2000
Reference [43]	CFSCs/PDMS	0.01	800
This work	GO@PDMS-PU	0.131–0.157	5000

Table 2. Comparison of sensor sensitivity in related works.

Research Work	Materials	Sensitivity (kPa−1)	Stable Cycle Times
Reference [35]	MWNT-rGO@PU	0.022~0.088	5000
Reference [36]	CNTs/TPU/SR	0.013~0.032	1000
Reference [37]	PDMS/CNT	0.111	10,000
Reference [38]	CMF/CNTs	0.11	3000
Reference [39]	PU@AgNWs@SLG	0.009~0.011	3000
Reference [40]	PDMS/PDA/MWCNT	0.020~0.202	8000
Reference [41]	GNPs/PDMS	0.0602	5000
Reference [42]	Graphene/PDMS	0.075	2000
Reference [43]	CFSCs/PDMS	0.01	800
This work	GO@PDMS-PU	0.131–0.157	5000

4. Applications in Human Motion Monitoring

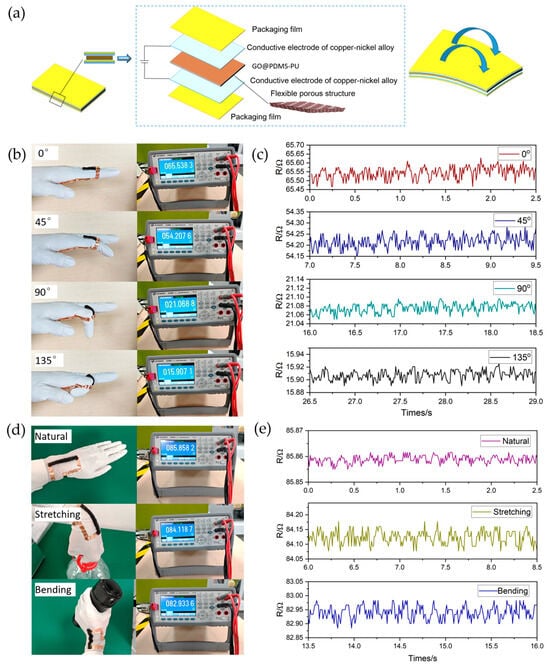
Figure 11 demonstrates the application of flexible sensors in monitoring finger flexion and wrist articulation. Given the complex curvilinear geometries of human joint interfaces, the asymmetric architecture achieves superior conformal contact fidelity through strain-field adaptive reconfiguration, enabling seamless integration with dynamic biomechanical surfaces. Figure 11a illustrates the bending deformation mechanism of the sensor. Figure 11b and Figure 11c display the resistance output values and electrical signal variation trends under different finger bending angles, respectively. Experimental data show that the sensor’s resistance decreases nonlinearly with increasing bending angles: it measures 65.5 Ω in a relaxed state, decreases to 54.2 Ω at 45°, sharply reduces to 21 Ω at 90°, and slows to 15.9 Ω at 135°. This behavior originates from the biaxial deformation mechanism of the material during bending: tensile strain occurs along the finger’s longitudinal direction, while compressive strain is generated at the joint region. Notably, when bending reaches 90°, the microstructural pores within the sensor approach their closure limit, leading to a significant reduction in the resistance variation rate during further bending to 135°, which highlights the device’s dynamic response characteristics.

Figure 11d,e illustrate the resistance output values and electrical signal variation trends under different tensile deformation states of the wrist. In this experiment, the sensor was fixed with one end attached below the wrist and the other end on the back of the hand. The resistance signal measured 85.8 Ω in the relaxed wrist state. When lifting a weight, the hand underwent clockwise bending, but the piezoresistive material experienced minimal structural deformation, resulting in a relatively subtle signal change (84.1 Ω). Conversely, when grasping a water cup, the hand bent counterclockwise, inducing significant morphological changes in the piezoresistive material, which led to a pronounced signal variation (82.9 Ω).

Leveraging the advantages of the PDMS-PU sponge composite—high flexibility, excellent fatigue resistance, and sensitive responsiveness—this study developed a wearable monitoring system tailored for rehabilitation patients. The system operates via multimodal sensing: Lifting motions trigger tensile deformation of the material, causing a positive resistance change. Bending motions induce structural bending and pore collapse, generating a stepwise resistance decrease. When the signal deviates from the baseline beyond a preset threshold, the system activates real-time alerts to prevent secondary injuries from overexertion. This technology can be extended to monitor multidimensional motions, such as torsional motions of the waist and flexion–extension of joints, enabling the construction of a full-body biomechanical map through a distributed sensor network. This framework provides a quantitative assessment basis for personalized rehabilitation training.

The design of the GO@PDMS-PU sponge flexible piezoresistive sensor is based on a highly elastic PU sponge, with its flexibility and environmental adaptability enhanced by a PDMS coating. Due to the porous structure and high elasticity of the PU sponge, which enables it to withstand large-scale deformations, and the chemical stability of PDMS that mitigates aging of the substrate material caused by environmental factors (e.g., humidity, temperature), the GO@PDMS-PU sponge sensor demonstrates high mechanical stability and environmental adaptability during use, particularly excelling in cycling durability and electrical performance retention. However, long-term usage may lead to sensitivity degradation due to microcracks in the conductive material or wear of the conductive layer. Future efforts should focus on introducing nano-reinforced conductive materials to further improve the mechanical strength and conductivity of the conductive components.

5. Conclusions

This study successfully fabricated a GO@PDMS-PU composite-based flexible piezoresistive sensor with exceptional mechanical/electrical stability and high sensitivity by optimizing the PDMS solvent-to-curing agent ratio, validating its potential for human motion monitoring. Key findings are summarized as follows:

Ratio Optimization and Mechanical Performance: The PDMS ratio critically governs the material’s resistance to cyclic deformation. Due to the asymmetric structure’s ability to more effectively distribute stress, the 5:1 (PDMS solvent/curing agent) ratio sample demonstrated superior anti-fatigue properties, achieving a deformation of 8.8 mm after 5000 cycles under 30 N loading, representing a 32% reduction compared to uncoated samples (13.0 mm). Moreover, PDMS-coated samples exhibited significantly reduced thickness variation. The 5:1 ratio sample showed minimal thickness change (<2 mm), with recovery capacity improved by 250% over uncoated samples.

Electrical Stability and Sensitivity Enhancement: With the asymmetric structure, the sensor’s electrical stability was improved significantly by the PDMS coating. Compared to the untested sample’s resistance range (10–248 Ω), the 5:1 ratio sample maintained a resistance distribution of 10–240 Ω after 5000 cycles, with a minimal attenuation of resistance variation rate (3%), a marked improvement over uncoated samples (10–50 Ω). Sensitivity was optimized from 0.131 kPa⁻¹ to 0.157 kPa⁻¹, surpassing some existing studies and overcoming the performance limitations of conventional piezoresistive materials.

Validation in Wearable Applications: The device’s asymmetric structure enables better conformability to complex curved surfaces, and the sensor demonstrated distinct response characteristics in human motion monitoring. Finger bending induced a stepwise resistance decrease (e.g., resistance dropped sharply to 15.9 Ω when bending from a relaxed state to 135°), while wrist motions triggered resistance increases (e.g., resistance decreased from 85.8 Ω to 82.9 Ω during lifting or bending). These linear responses originated from the dynamic closure limit of the material’s internal porous structure. The developed multimodal sensing system accurately captured physiological signals such as finger bending and wrist movements, enabling real-time alerts via preset thresholds and offering a quantifiable, highly reliable solution for rehabilitation monitoring. By synergistically optimizing material ratios and structural design, this work provides new insights for developing high-performance flexible sensors.