1. Introduction
Fractional calculus extends classical calculus from integer-order to fractional-order and has been widely applied in various fields of science, technology, and engineering, as evidenced by published articles [
1,
2,
3].
Due to the widespread applicability of Riemann–Liouville fractional integrals, researchers have devoted efforts to various generalizations, such as the
k-Riemann–Liouville fractional integrals [
4], the (
k,
s)-Riemann–Liouville fractional integrals [
5], and many others [
6,
7,
8].
Furthermore, to overcome the limitation of the conformable fractional derivative [
9], which fails to return to the original function as the fractional order approaches zero, Anderson et al. proposed a modification by using proportional derivatives in [
10] as
Inspired by [
10], Jarad et al. redefined a generalized proportional fractional derivative in [
11] as
They also defined the proportional integral related to
as
This definition incorporates an exponential function in the kernel of the fractional operator.
Moreover, the generalized proportional
-Riemann–Liouville fractional integrals introduced by Rashid et al. [
12] and Jarad et al. [
13] have further advanced the development of proportional derivatives and Riemann–Liouville fractional integrals.
Meanwhile, fractional calculus plays a crucial role in numerical integral estimation. Against this background, Sarikaya et al. [
14] were the first to establish Hermite–Hadamard inequalities using Riemann–Liouville fractional integrals as follows:
Since then, convex analysis related to the concept of symmetry in fractional calculus has been widely applied to the study of Hermite–Hadamard inequalities. Among them, Vivas-Cortez et al. [
15] established some new Hermite–Hadamard–Mercer-type inequalities by using the
k-Riemann–Liouville fractional integral, which further developed the application of Hermite–Hadamard inequalities in fractional calculus.
Notable examples include those derived using
k-Riemann–Liouville fractional integrals [
16], (
,
k)-Riemann–Liouville fractional integrals [
17], Riemann–Liouville fractional integrals defined on the plane
[
18], and generalized proportional Riemann–Liouville fractional integrals [
19].
Compared to classical calculus, the multiplicative calculus introduced by Grossman and Katz [
20] provides a more natural framework for modeling exponential growth and decay processes commonly observed in nature and socioeconomics. Özcan et al. extended these studies by exploring Hermite–Hadamard type inequalities for various multiplicative convex functions. Specifically, they examined inequalities for multiplicative preinvex function [
21], multiplicative
p-convex function [
22], multiplicative harmonically convex function [
23], and exponentially multiplicative convex function [
24] within the framework of integer-order calculus.
Furthermore, based on the multiplicative convexity of differentiable functions, researchers have also explored midpoint and trapezoidal inequalities [
25], Ostrowski-type inequalities [
26], dual Simpson-type inequalities [
27], and Newton-type inequalities [
28], among others.
With the advancement of fractional calculus, researchers nowadays begin to explore the theory of multiplicative fractional inequalities. In this regard, Budak and Özçelik [
29] established Hermite–Hadamard type inequality for multiplicative Riemann–Liouville fractional integrals as follows:
Furthermore, the generalized multiplicative fractional integral operator introduced by Kashuri et al. [
30] can lead to the derivation of interesting fractional integral operators. The Hermite–Hadamard type inequalities obtained within this framework can effectively promote the research progress of inequalities in the context of multiplicative fractional integrals. Similarly, Du and Peng [
31] obtained Hermite–Hadamard type inequalities via multiplicative Riemann–Liouville fractional integrals, thus enabling the research on the Hermite–Hadamard inequalities in the realm of multiplicative fractional integrals to reach a new level and achieve further breakthroughs. Moreover, Zhang et al. [
32] explored Hermite–Hadamard type inequalities for multiplicative
-convex functions via multiplicative
k-Riemann–Liouville fractional integrals. Peng et al. [
33] investigated symmetric Hermite–Hadamard inequalities with multiplicative fractional integrals containing an exponential kernel, and Zhou and Du [
34] analyzed Hermite–Hadamard inequalities for multiplicative
k-Hadamard fractional integrals. These works significantly advance the study of Hermite–Hadamard inequalities in multiplicative fractional integrals.
In conclusion, existing research on Hermite–Hadamard-type inequalities has predominantly concentrated on the additive setting. In contrast, studies focusing on multiplicative fractional integrals, particularly in the context of generalized proportional operators, remain relatively limited.
Motivated by this gap and inspired by the relevant literature—especially references [
30,
31]—this paper investigates two key aspects:
- (i)
The concept of the multiplicative -convex function, which generalizes both classical convexity and multiplicative convexity by incorporating a strictly monotonic transformation function and a geometric mean-based structure. This framework is naturally compatible with applications in information theory and economics, where multiplicative behavior frequently arises. For instance, in information theory, it supports information measurement models involving logarithmic means, while in economics, it provides a theoretical basis for analyzing investment decisions based on geometric mean returns in risk models.
- (ii)
The construction of the multiplicative generalized proportional -Riemann–Liouville fractional integral, which leverages logarithmic and exponential functions to define an integration process in a multiplicative setting. This operator is particularly well-suited for positive-valued functions and processes involving proportional transformations. The presence of a scalable parameter and a transformation function allows flexible control over memory effects and scaling behavior, enabling more accurate modeling of non-local phenomena, heterogeneous media, and adaptive systems.
Within this framework, the generalized Hermite–Hadamard-type inequalities not only offer refined bounds for function median estimations but also effectively capture non-local multiplicative characteristics of functions. Moreover, they serve as powerful analytical tools with potential applications in the estimation of solutions to fractional differential equations, inequality analysis in information theory, entropy and uncertainty quantification, risk assessment and reliability modeling, and distribution fitting in statistical and engineering models, etc.
The paper follows this structure: following the introduction,
Section 2 presents the relevant definitions and theorems required for this paper.
Section 3 rigorously defines multiplicative
-convex functions and elaborates on their analytical properties and some inequalities.
Section 4 introduces multiplicative generalized proportional
-Riemann–Liouville fractional integrals and establishes their continuity, semigroup properties and integrability criteria, and so on. Building on the fractional operators,
Section 5 derives Hermite–Hadamard type inequalities for multiplicative
-convex functions.
Section 6 proves a fractional Hermite–Hadamard identity, which serves as the foundation for subsequent Hermite–Hadamard type inequalities. Finally, we summarize key contributions and discuss their broader implications.
3. Properties of the Multiplicative -Convex Functions
In this section, we begin by introducing a generalized notion of convexity, namely multiplicative -convexity. We demonstrate that this class not only generalizes several known types of multiplicative convex functions, but also satisfies key structural properties and inequalities that are essential in the context of multiplicative analysis.
Definition 6. A function is said to be a multiplicatively σ-convex function with respect to a strictly monotonic continuous function σ, for all , and , the following inequality holds:where is monotonic and continuous, and the range of σ corresponds to the domain of . Remark 1. Now, we discuss several special cases.
- 1.
If , then Definition 6 become a multiplicative convex function given in [42], - 2.
If , then Definition 6 become a multiplicative geometric convex function given in [42], - 3.
If , then Definition 6 can become multiplicative harmonic convexity as considered in [23], - 4.
If , , then Definition 6 can become the concept of multiplicatively p-convex function as considered in [22].
Next, we present some properties and inequalities of multiplicative -convex functions.
Proposition 1. We state the following as true.
- 1.
If f and g are multiplicative σ-convex functions, then is a multiplicatively σ-convex function,
- 2.
For any , if f is a multiplicative σ-convex function, then is also a multiplicatively σ-convex function,
- 3.
For any , if f is a multiplicative σ-convex function, then is also a multiplicative σ-convex function,
- 4.
A function f is a multiplicative σ-convex function, if and only if, is a σ-convex function,
- 5.
If f is a multiplicative σ-convex function, then for and weights with , the following inequality holds:
Proof. The proof of the above property is straightforward, so we omit it. □
Proposition 2. Let be an arbitrary family of multiplicatively σ-convex functions, where . Then, the function is a multiplicative σ-convex function.
Proof. For all
and
, we obtain
The proof is completed. □
Proposition 3. Let be a σ-convex function, is a multiplicatively convex function, then the function is a multiplicative σ-convex function.
Proof. For all
and
, the following holds:
This completes the proof. □
Proposition 4. If is a monotonically increasing convex function and is a multiplicative σ-convex function, then is also a multiplicative σ-convex function.
Proof. By considering the multiplicative
-convexity of
f, and combining it with the convexity and monotonicity of
g, we obtain
This completes the proof. □
Proposition 5. Suppose is monotonically decreasing and is a family of monotonically increasing bijective mappings parameterized by , where is also monotonically increasing with respect to λ. If for , f satisfiesthen f also satisfies Proof. Since
is monotonically increasing with respect to
and
, for any
, we have
Also, since
is monotonically increasing, we further obtain
Since
f is a monotonically decreasing function, we obtain
From the given condition, we finally obtain
The proposition is proved. □
Proposition 6. Suppose that σ is a strictly monotonic increasing and continuous function. If is a multiplicative σ-convex function, with and , and there exists a positive number m, such that for any , , then the function f is bounded on the interval .
Proof. For any
, since
is a strictly monotonic increasing and continuous function, there exists a unique
, such that
Due to the multiplicative
-convexity of
f, when we set
, we can obtain
At the same time, according to the known conditions, we have
; therefore, on the interval
, we have
. □
Proposition 7. Let be a multiplicative σ-convex function, and be a strictly monotonic continuous bijective function. For any , and . When we let and , then is a multiplicative convex function on .
Proof. For any
,
(
) and
, according to the definition of
, we obtain
It is also known that:
Since
and
, we have
. □
Example 1. Let , where is a convex function and σ is a strictly monotonic continuous function. When we let and , we obtain Remark 2. Let , where , and , where . Arbitrarily take and . Let and . Then, Remark 3. Let for , and , where . For any , , and , let , . Then, Next, for simplicity, we use the following notations to represent the logarithmic means of two non-negative numbers ().
Generalized logarithmic mean:
Theorem 2. Assume that f, g: are multiplicative σ-convex functions. Then, for any , , with , we can obtain Proof. When
,
and
, the following inequality holds:
Therefore, based on the multiplicative
-convexity of
f and
g, we can use the above-mentioned inequality to obtain
This completes the proof. □
Theorem 3. Assume that f, g: are monotonically increasing multiplicative σ-convex functions. For any with , we can obtain the following inequality: Proof. Since
f and
g are monotonically increasing multiplicative
-convex functions, we know that:
Using the rearrangement inequality, we can obtain
Taking the natural logarithm of both sides of the above inequality and integrating with respect to
t over the interval
, we have
Now, taking the exponential of both sides of the inequality, we can obtain
This completes the proof. □
4. Multiplicative Generalized Proportional -Riemann–Liouville Fractional Calculus
The multiplicative generalized proportional -Riemann–Liouville fractional integral operator proposed in this study effectively unifies and extends existing integral forms. By introducing a proportional parameter and a monotonic function , it provides greater flexibility and adaptability in analyzing multiplicative nonlocal behaviors. This operator not only enriches the theoretical framework of multiplicative fractional calculus but also offers practical potential in various applied contexts.
Definition 7. Suppose that is a finite interval of the real line . Let be a function that is positive on , and let σ be an increasing and positive monotonic function on with a continuous derivative on . The multiplicative left-sided and right-sided generalized proportional σ-Riemann–Liouville fractional integrals of order with , and the proportionality index , are defined as follows: Remark 4. Now, we discuss several special cases.
- 1.
If , , then Definition 7 become multiplicative Riemann–Liouville fractional integrals given in [43], - 2.
If , , then Definition 7 become multiplicative Hadamard fractional integrals given in [34].
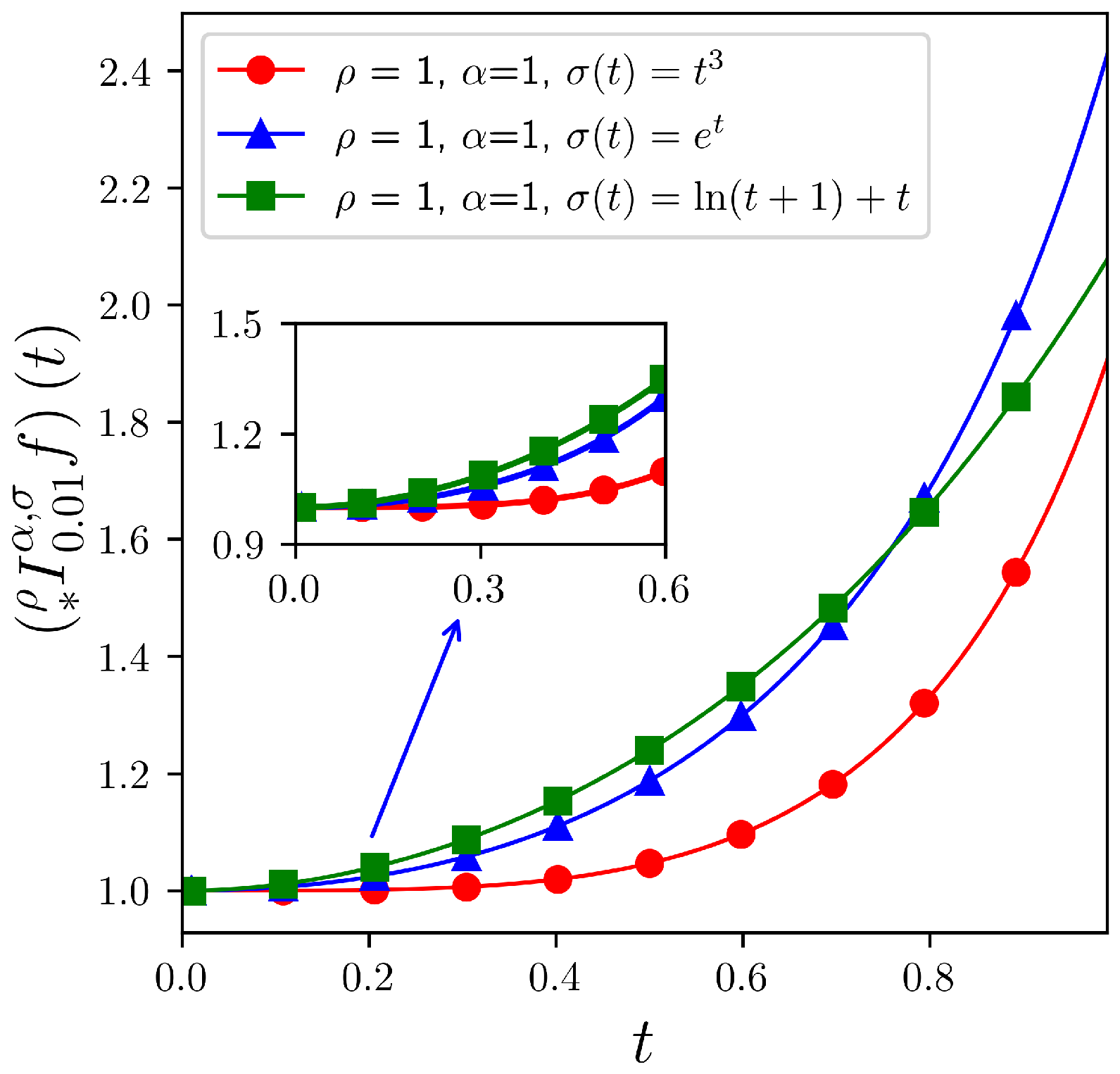
Remark 5. For the convenience of expression below, the quantities are defined as follows: Example 2. Next, the performance of the left-sided operator as formulated in Definition 7, focusing on its behavior when applied to separate functions on the interval . Taking , the following holds: Using graphical visualization, the simulation results presented in
Figure 1,
Figure 2 and
Figure 3 indicate that the core parameters
,
, and the specific forms of the function
exert a significant influence on the behavior of the left-sided operator
. In particular, under the given conditions, smaller values of
and
correspond to a faster growth rate of the integral of the function
f with respect to the variable
t. Furthermore, among the considered cases, when
is taken as an exponential function, the growth of the integral is the most rapid, highlighting the sensitivity of the operator’s behavior to the choice of transformation function
.
Next, we conduct a deeper examination of the properties of the integral operator .
Theorem 4. Suppose that the function with is continuous and integrable. Then, for any and , the function is continuously defined on .
Proof. Assume
with
, we have
Note that for a fixed
, the function
is continuous with respect to
t, and when
, we have
Moreover, since
is continuously integrable, by the Dominated Convergence Theorem, we obtain
Similarly, because
f is continuous (so
is bounded),
, and
is bounded on the compact set, we have
Therefore, the overall estimated term tends to zero, indicating that:
that is, the conclusion holds. □
Theorem 5. Let be a continuous function, where . Also, let α, and . Then, for all , the following equality holds: Proof. By adopting the definition of the operator
, while leveraging the continuity of the function
f and Dirichlet’s formula, we can obtain
Through the substitution of variable
, we obtain
Substituting Equation (
2) into Equation (
1), we have
By reversing the values of
and
in Equation (
3), the second equation can be proven. □
Theorem 6. Suppose that , , and . Then, for all , the following equation holds: Proof. Through the substitution of variable
, we can obtain
This completes the proof. □
Theorem 7. Let f, be integrable functions, where . For and , the operator has multiplicative linearity. Proof. By leveraging the continuity of functions
f and
g, we obtain
This completes the proof. □
Theorem 8. Assume that the function is integrable on , where and is a bounded mapping on . σ is a Lipschitz mapping and a monotonically increasing positive function on , and has a continuous derivative on . Then, for any , , is also a Lipschitz mapping on .
Proof. Since
is a bounded function, there exists a constant
M, such that
. Assume that
. Using the monotonic increasing property of
, we know that
; therefore, we have
where
Since
is a monotonically increasing positive function on
and has a continuous derivative
on
, we know that
and
. For any
, let
on
. Then,
satisfies the conditions of the Lagrange mean value theorem, that is, we obtain
Since
is a Lipschitz function on
, for
, There exist positive constants
,
, and
L such that
and
. Then
and
; therefore, we obtain
This establishes the desired result and the proof is complete. □
To prove the boundedness of in the space for , it is essential to re-examine the definition of the norm .
Definition 8 ([
44])
. Let and let σ be an increasing, positive and monotone function on with a continuous derivative for . For , the space is defined as the set of all real-valued, Lebesgue measurable functions f on and its norm is given by Theorem 9. Suppose that the function is integrable. Given , , and . Then is bounded in , and Proof. For
and
, we have
Using the fact that
and taking into account the generalized Minkowski inequality ([
45]), we have
By employing the well-known Hölder inequality with
, we can obtain
This completes the proof. □
Remark 6. The operator yields results that are similar to those presented above.
5. The Hermite–Hadamard Inequality of the Multiplicative Fractional Integrals
The following presents the derivation of Hermite–Hadamard type inequalities for multiplicative generalized proportional -Riemann–Liouville fractional integrals via multiplicative -convex functions. Compared with the classical multiplicative Riemann–Liouville operator, the generalized form introduced here provides broader generality and greater applicability, thereby extending the scope and flexibility of related integral inequalities.
Definition 9 ([
19])
. For , the confluent hypergeometric function admits an integral representation given by Theorem 10. Let f be a positive function that is multiplicatively σ-convex on with , σ is a monotonically increasing positive function on , and has a continuous derivative on . For and , the following Hermite–Hadamard inequality for multiplicative generalized proportional σ-Riemann–Liouville fractional integrals holds: Proof. Since
f is a positive and multiplicatively
-convex function on
, we can obtain
After the inequality (
4) is multiplied by
and the resulting inequality is integrated with respect to
t over the interval
, we obtain
Moreover, because
Therefore, we have
Thus, we can obtain
Since
f is a multiplicatively
-convex function on the interval
, we obtain
As a consequence, the following inequality holds:
After the inequality (
5) is multiplied on both sides by
and the resulting inequality is integrated with respect to
t over the interval
, we have
Therefore, we can obtain
Thus, the desired assertion is proven, completing the proof. □
Corollary 1. Taking into account Theorem 10, when , Budak and Özçelik [29] establish the Hermite–Hadamard inequality for multiplicatively convex functions as follows: Corollary 2. Taking into account Theorem 10, when , , Zhou and Du [34] examined the multiplicative Hadamard fractional integrals related to Hermite–Hadamard inequalities as follows: Corollary 3. Taking into account Theorem 10, when , we establish the Hermite–Hadamard inequality for integer-order integrals of multiplicative σ-convex functions. Corollary 4. Suppose that for , the functions are positive and multiplicatively σ-convex on , where . Then, Proof. Since the functions () are positive and multiplicatively -convex on the interval with , their product is also positive and multiplicatively -convex. Therefore, by applying Theorem 10 to the function , we obtain the desired result. □
As illustrated in Example 1, a function of the form , where is convex and is a strictly monotonic and continuous function, qualifies as a multiplicative -convex function. Building upon this foundation, we employ the numerical examples presented in Remarks 2 and 3 to provide a numerical verification of the validity of Theorem 10.
Example 3. Let , , , . Then . Also, assume , . Here, Theorem 10 is equivalent to Based on Example 3, we consider the parameter ranges
and
. Using graphical visualization techniques, we present
Figure 4, which illustrates the plots of three functions corresponding to the left-hand side, middle term, and right-hand side of inequality (
6). From
Figure 4, it is evident that variations in the parameters
and
result in distinct changes in the shapes and trajectories of the graphs. These changes highlight the significant impact of the parameters on the behavior of the inequality, emphasizing their essential role in shaping the underlying functional relationships.
Remark 7. In Example 3, when assuming , then the fractional integral in Theorem 10 becomes the Hadamard fractional integral. Here, Example 3 is tantamount to In contrast to Example 3, we now disregard the influence of the proportional parameter
as discussed in Remark 7, and consider the variable
. Using graphical visualization,
Figure 5 illustrates the graphs of the three functions corresponding to the left-hand side, middle, and right-hand side of inequality (
7). This comparison highlights the significant regulatory role of the parameter
. As previously illustrated in
Figure 4, incorporating
introduces increased complexity and variability in the functional behavior. In contrast, omitting
results in a simplified relationship among the functions, revealing its pivotal influence on the dynamic structure of the inequality.
Remark 8. In Example 3, when we assume , the fractional integral in Theorem 10 is transformed into the proportional integral, and consequently, Example 3 is changed into To further investigate the isolated influence of the proportional parameter
, we fix all other variables and allow
to vary within the interval
. Utilizing graphical visualization,
Figure 6 presents the plots of the three functions derived from inequality (
8). As observed from
Figure 6, when the effect of the fractional order
is disregarded, the relationships among the three functions exhibit a trend toward stabilization as
increases. This behavior indirectly highlights the underlying influence that the parameter
exerts on the dynamics of the system. A comparison with
Figure 4 further reveals that the joint modulation of
and
plays a crucial role in shaping the functional behavior, demonstrating that their combined effects contribute significantly to the regulation and complexity of the system.
Example 4. Let , , , . Then . Let , . Then, Theorem 10 becomes:According to the above results, we can obtain Following the same approach as in Example 4, we perform an additional graphical analysis to further explore the behavior of the Hermite–Hadamard-type inequality given in Equation (
9). In this case, we allow the fractional order
and the proportional parameter
to vary simultaneously. As shown in
Figure 7, the plots represent the three components of inequality (
9), corresponding respectively to the left-hand side, middle term, and right-hand side. The visualization illustrates how different combinations of
and
affect the shapes and relative positions of the function curves. This example reaffirms the significant role played by the parameters
and
in regulating the inequality bounds. The observed changes in the trend and dispersion of the graphs indicate the flexibility and applicability of the proposed integral operator and function class in capturing multiplicative non-local behaviors under varying parameter regimes.
From Examples 3 and 4, it is evident that both the fractional order and the proportional parameter play a crucial role in regulating the behavior of the integral operator. Specifically, the integral value consistently remains bounded between the left-hand and right-hand sides of the inequality, in alignment with the theoretical predictions established in Theorem 10. This empirical observation provides strong numerical support for the validity and robustness of the derived Hermite–Hadamard-type inequality under the proposed integral framework.
6. Identities and the Associated Hermite–Hadamard-Type Inequalities
This section is primarily divided into two parts. In the first part, we establish a Hermite–Hadamard-type identity for the proposed multiplicative generalized proportional fractional integral. Building upon this foundation, the second part derives a Hermite–Hadamard-type inequality for multiplicative fractional integrals by thoroughly investigating the multiplicative -convexity of functions. These results not only generalize the classical Hermite–Hadamard inequality but also extend its applicability within the framework of multiplicative fractional calculus.
To simplify the subsequent results, we introduce the following definition.
Definition 10 ([
46])
. For any real numbers and t, , the λ-incomplete gamma function is defined as follows:wherewhen , we obtain The following is the multiplicative generalized proportional fractional Hermite–Hadamard type identity, which is a key tool for deriving the subsequent results.
Lemma 1. Assume that is a multiplicative differentiable function on with , such that . If is multiplicative integrable on , then the following identity holds:whereand Proof. Let
and
, we can obtain
Similarly, we obtain
Multiplying the four equalities (
), we have
The proof is completed. □
Theorem 11. Let be an increasing multiplicative differentiable function on with . And let be a multiplicative σ-convex function, where is a monotonically increasing positive function and has a continuous derivative on J. If we assume , , then by Proposition 7, is a multiplicative convex function on . Therefore, is a convex function on , where . Then, the following inequality holds: Proof. Taking advantage of Lemma 1, we can obtain
In terms of the power-mean inequality, we obtain
Using the convexity of
and the monotonic increase of
f, we have
Similarly, we obtain
and
and
Also, it follows that:
For the convenience of expression below, the quantities are defined as follows:
If the formulas from Equations (
11)–(
16) are applied to the inequality (
10), we can obtain
Using the fact that
for
with
, we have
This ends the proof. □
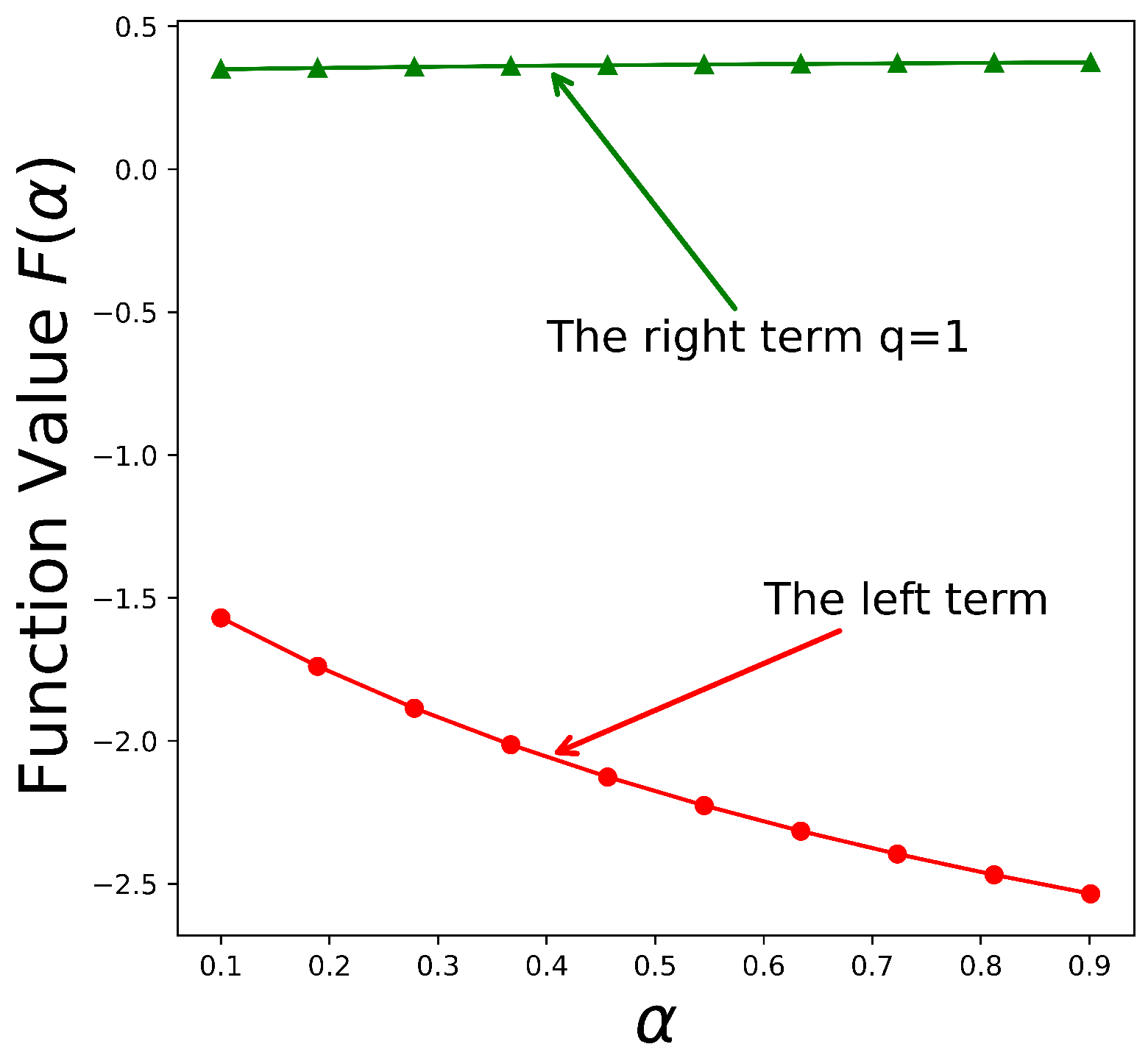
Corollary 5. When in Theorem 11, Du and Peng [31] obtain Hermite–Hadamard type inequalities via multiplicative Riemann–Liouville fractional integrals. Remark 9. When we take , , in Theorem 11, it yields the following inequalitywhich is presented by Du and Peng in [31]. Example 5. Let , , the function is convex with . Thus, all the requirements of Theorem 11 are met. If letting , , and taking for all , then, we can obtain the left-hand side of the inequality stated in Theorem 11:and the right portion is equivalent toFrom the above result, the following inequality can be further established: By employing numerical estimation and graphical visualization techniques, the results presented in
Table 1,
Figure 8 and
Figure 9 demonstrate notable disparities between the left- and right-hand sides of the inequality. Specifically, the left-hand term remains negative and exhibits a continuous decline as
increases, indicating a monotonic downward trend. In contrast, the right-hand term increases with
, showing an opposing trend that suggests the possibility of equilibrium under certain parameter conditions. Furthermore, as the parameter
q increases, the right-hand term consistently remains positive and continues to grow. These numerical findings are in strong agreement with the theoretical predictions outlined in Theorem 11, thereby reinforcing the validity of the proposed inequality.
Remark 10. Considering the estimation of bound on the multiplicative right-sided fractional integrals defined as By making use of Remark 10, we can obtain similar results, which will be omitted here.
7. Conclusions
This study investigates multiplicative -convex functions and their interactions with multiplicative generalized proportional -Riemann–Liouville fractional integrals, where denotes a strictly monotonic and continuous function. Within this framework, we systematically establish Hermite–Hadamard-type inequalities, rigorously validating their analytical soundness and operational domain.
To substantiate the theoretical results, several illustrative examples are provided, demonstrating their practical relevance and applicability. By flexibly adjusting the monotonic function , fine-tuning the proportionality parameter , and appropriately selecting the fractional order , we explore the proposed mathematical constructs from multiple perspectives. This parametric flexibility yields a wide range of meaningful and insightful outcomes.
However, due to the exponential kernel structure inherent in the multiplicative fractional integral, the associated analytical expressions become relatively intricate. As such, this paper does not yet include more refined estimations of the function median in the derived Hermite–Hadamard inequalities.
Despite this limitation, the study offers important insights for the continued development of integral inequalities in the setting of multiplicative calculus, particularly those involving multiplicative fractional operators. The methodologies and findings presented herein are expected to foster further research in this direction. As a natural extension, we propose the exploration of broader classes of multiplicative fractional inequalities and their potential applications.