Fractional and Higher Integer-Order Moments for Fractional Stochastic Differential Equations
Abstract
1. Introduction
2. Preliminaries
- 1.
- for all .
- 2.
- for all .
- 3.
- for all .
- 4.
- has continuous sample paths -a.s.
3. Main Results
4. Numerical Simulation
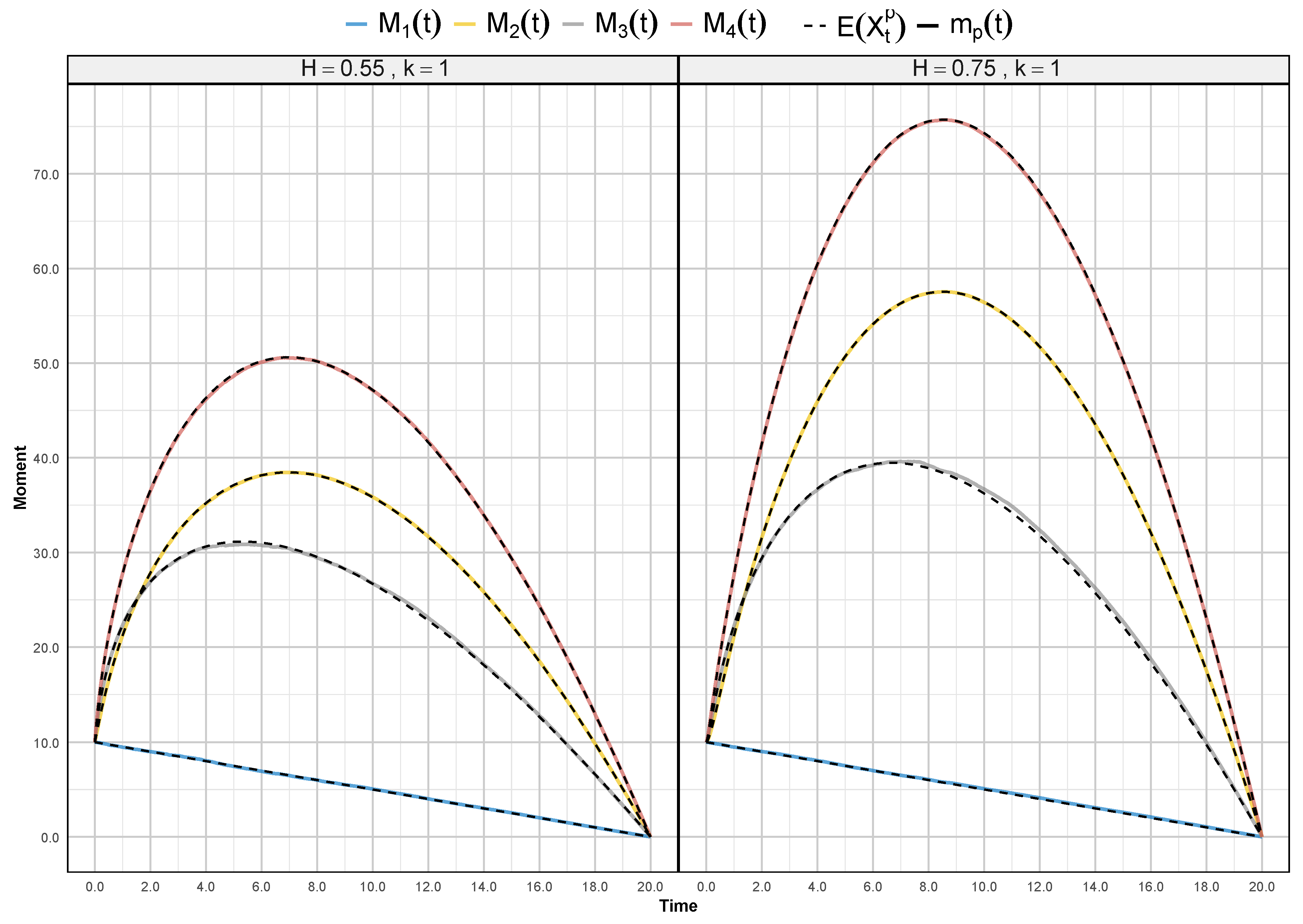
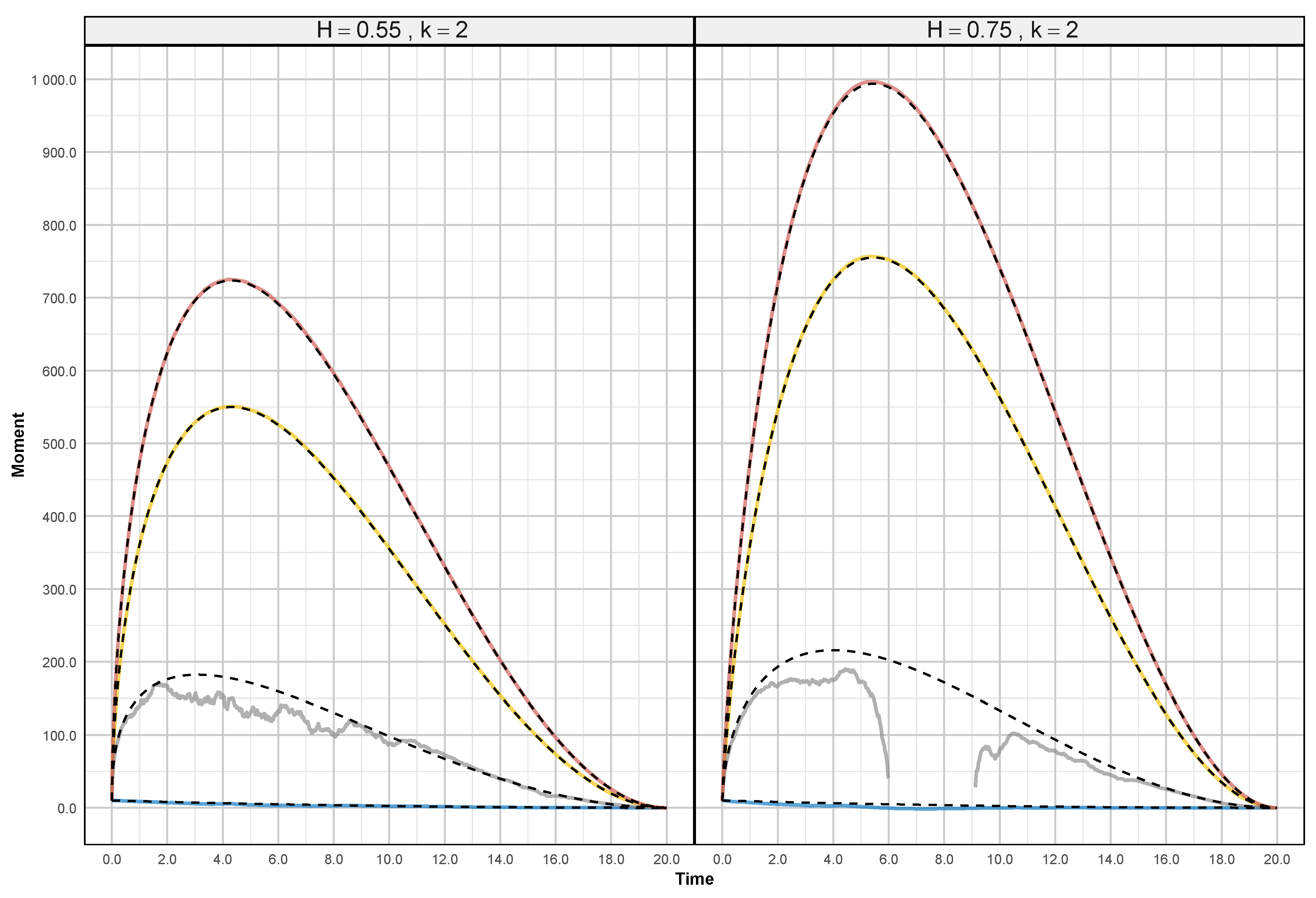
4.1. Linear Time-Dependent Function
4.2. Non-Linear Time-Dependent Function
4.3. Fractional Brownian Bridge Process
4.4. Fractional Ornstein–Uhlenbeck Process
- Function: , with .
- Parameters: , , , .
5. Conclusions and Perspectives
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Klebaner, F.C. Introduction to Stochastic Calculus with Applications, 2nd ed.; Imperial College Press: London, UK, 2005. [Google Scholar] [CrossRef]
- Allen, E. Mathematical Modelling: Theory and Applications. In Modeling with Itô Stochastic Differential Equations, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2007; Volume 22. [Google Scholar] [CrossRef]
- Jedrzejewski, F. Modèles Aléatoires et Physique Probabiliste; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar] [CrossRef]
- Freidlin, M.I.; Wentzell, A.D. Grundlehren der mathematischen Wissenschaften. In Random Perturbations of Dynamical Systems; Springer: Berlin/Heidelberg, Germany, 2012; Volume 260. [Google Scholar] [CrossRef]
- Živorad, T.; Sandev, T.; Metzler, R.; Dubbeldam, J. Generalized space–time fractional diffusion equation with composite fractional time derivative. Phys. A Stat. Mech. Its Appl. 2012, 391, 2527–2542. [Google Scholar] [CrossRef]
- Lange, K. Statistics for Biology and Health. In Mathematical and Statistical Methods for Genetic Analysis, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar] [CrossRef]
- Han, X.; Kloeden, P.E. Probability Theory and Stochastic Modelling. In Random Ordinary Differential Equations and Their Numerical Solution, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2017; Volume 85. [Google Scholar] [CrossRef]
- Panik, M.J. Stochastic Differential Equations: An Introduction with Applications in Population Dynamics Modeling; John Wiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar] [CrossRef]
- Atangana, A.; İgret Araz, S. Industrial and Applied Mathematics. In Fractional Stochastic Differential Equations: Applications to Covid-19 Modeling, 1st ed.; Springer Nature: Singapore, 2022. [Google Scholar] [CrossRef]
- Rolski, T.; Schmidli, H.; Schmidt, V.; Teugels, J. Stochastic Processes for Insurance and Finance; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar] [CrossRef]
- Iacus, S.M. Option Pricing and Estimation of Financial Models with R; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar] [CrossRef]
- Nguyen Tien, D. Fractional stochastic differential equations with applications to finance. J. Math. Anal. Appl. 2013, 397, 334–348. [Google Scholar] [CrossRef]
- Rostek, S.; Schöbel, R. A note on the use of fractional Brownian motion for financial modeling. Econ. Model. 2013, 30, 30–35. [Google Scholar] [CrossRef]
- Mackevicius, V. Stochastic Models of Financial Mathematics, 1st ed.; Elsevier: Amsterdam, The Netherlands, 2016. [Google Scholar] [CrossRef]
- Primak, S.; Kontorovich, V.; Lyandres, V. Stochastic Methods and Their Applications to Communications, Stochastic Differential Equations Approach; John Wiley & Sons: Hoboken, NJ, USA, 2004. [Google Scholar] [CrossRef]
- Henderson, D.; Plaschko, P. Stochastic Differential Equations in Science and Engineering; World Scientific: Singapore, 2006. [Google Scholar] [CrossRef]
- Kolmogorov, A.N. Wienersche spiralen und einige andere interessante kurven in hilbertscen raum, cr (doklady). Acad. Sci. URSS 1940, 26, 115–118. [Google Scholar]
- Mandelbrot, B.B.; Ness, J.W.V. Fractional Brownian Motions, Fractional Noises and Applications. SIAM Rev. 1968, 10, 422–437. [Google Scholar] [CrossRef]
- Maslowski, B.; Nualart, D. Evolution equations driven by a fractional Brownian motion. J. Funct. Anal. 2003, 202, 277–305. [Google Scholar] [CrossRef]
- Mishura, Y.S. Stochastic Calculus for Fractional Brownian Motion and Related Processes, 1st ed.; Lecture Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar] [CrossRef]
- Ascione, G.; Mishura, Y.; Pirozzi, E. Fractional Deterministic and Stochastic Calculus; De Gruyter: Berlin, Germany; Boston, MA, USA, 2024. [Google Scholar] [CrossRef]
- Ullah, R.; Farooq, M.; Faizullah, F.; Alghafli, M.A.; Mlaiki, N. Fractional stochastic functional differential equations with non-Lipschitz condition. AIMS Math. 2025, 10, 7127–7143. [Google Scholar] [CrossRef]
- Bishwal, J.P.N. Parameter Estimation in Stochastic Differential Equations, 1st ed.; Lecture Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar] [CrossRef]
- Bishwal, J.P.N. Parameter Estimation in Stochastic Volatility Models, 1st ed.; Springer: Cham, Switzerland, 2012. [Google Scholar] [CrossRef]
- Rao, B.P. Statistical Inference for Fractional Diffusion Processes, 1st ed.; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2010. [Google Scholar] [CrossRef]
- Risken, H. The Fokker-Planck Equation: Methods of Solution and Applications, 2nd ed.; Springer Series in Synergetics; Springer: Berlin/Heidelberg, Germany, 1996; Volume 18. [Google Scholar] [CrossRef]
- Živorad, T.; Ralf, M.; Stefan, G. Fractional characteristic functions, and a fractional calculus approach for moments of random variables. Fract. Calc. Appl. Anal. 2022, 25, 1307–1323. [Google Scholar] [CrossRef]
- Cottone, G.; Di Paola, M.; Metzler, R. Fractional calculus approach to the statistical characterization of random variables and vectors. Phys. A Stat. Mech. Its Appl. 2010, 389, 909–920. [Google Scholar] [CrossRef]
- Graham, C.; Talay, D. Stochastic Modelling and Applied Probability. In Stochastic Simulation and Monte Carlo Methods: Mathematical Foundations of Stochastic Simulation, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2013; Volume 68. [Google Scholar] [CrossRef]
- Thomopoulos, N.T. Essentials of Monte Carlo Simulation: Statistical Methods for Building Simulation Models, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar] [CrossRef]
- Li, M. PSD and Cross-PSD of Responses of Seven Classes of Fractional Vibrations Driven by fGn, fBm, Fractional OU Process, and von Kármán Process. Symmetry 2024, 16, 635. [Google Scholar] [CrossRef]
- Russo, F.; Vallois, P. Stochastic calculus with respect to continuous finite quadratic variation processes. Stochastics Stoch. Rep. 2000, 70, 1–40. [Google Scholar] [CrossRef]
- Nualart, D.; Răşcanu, A. Differential equations driven by fractional Brownian motion. Collect. Math. 2002, 53, 55–81. [Google Scholar]
- Guerra, J.; Nualart, D. Stochastic Differential Equations Driven by Fractional Brownian Motion and Standard Brownian Motion. Stoch. Anal. Appl. 2008, 26, 1053–1075. [Google Scholar] [CrossRef]
- Merton, R.C. Theory of Rational Option Pricing. Bell J. Econ. Manag. Sci. 1973, 4, 141–183. [Google Scholar] [CrossRef]
- Cheridito, P.; Kawaguchi, H.; Maejima, M. Fractional Ornstein-Uhlenbeck processes. Electron. J. Probab. 2003, 8, 1–14. [Google Scholar] [CrossRef]
- Guidoum, A.C.; Boukhetala, K. Exact higher-order moments for linear non-homogeneous stochastic differential equation. Model Assist. Stat. Appl. 2023, 18, 321–329. [Google Scholar] [CrossRef]
- Decreusefond, L.; Üstünel, A.S. Stochastic Analysis of the Fractional Brownian Motion. Potential Anal. 2001, 10, 177–214. [Google Scholar] [CrossRef]
- Alòs, E.; Mazet, O.; Nualart, D. Stochastic Calculus with Respect to Gaussian Processes. Ann. Probab. 2001, 29, 766–801. [Google Scholar] [CrossRef]
- Bender, C. An Itô formula for generalized functionals of a fractional Brownian motion with arbitrary Hurst parameter. Stoch. Process. Their Appl. 2003, 104, 81–106. [Google Scholar] [CrossRef]
- Olver, F.W.; Lozier, D.W.; Boisvert, R.F.; Clark, C.W. NIST Handbook of Mathematical Functions, 1st ed.; Cambridge University Press: New York, NY, USA, 2010. [Google Scholar]
- Willink, R. Normal moments and Hermite polynomials. Stat. Probab. Lett. 2005, 73, 271–275. [Google Scholar] [CrossRef]
- Winkelbauer, A. Moments and Absolute Moments of the Normal Distribution. arXiv 2014. [Google Scholar] [CrossRef]
- R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2025; Available online: https://www.r-project.org/ (accessed on 15 March 2025).
- Guidoum, A.C.; Boukhetala, K. Performing Parallel Monte Carlo and Moment Equations Methods for Itô and Stratonovich Stochastic Differential Systems: R Package Sim.DiffProc. J. Stat. Softw. 2020, 96, 1–82. [Google Scholar] [CrossRef]
- Brouste, A.; Fukasawa, M.; Hino, H.; Iacus, S.M.; Kamatani, K.; Koike, Y.; Masuda, H.; Nomura, R.; Ogihara, T.; Shimuzu, Y.; et al. The yuima Project: A Computational Framework for Simulation and Inference of Stochastic Differential Equations. J. Stat. Softw. 2014, 57, 1–51. [Google Scholar] [CrossRef]
- Iacus, S.M.; Yoshida, N. Simulation and Inference for Stochastic Processes with yuima: A Comprehensive R Framework for SDEs and Other Stochastic Processes; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar] [CrossRef]
- Burlon, A. On the numerical solution of fractional differential equations under white noise processes. Probabilistic Eng. Mech. 2023, 73, 103465. [Google Scholar] [CrossRef]



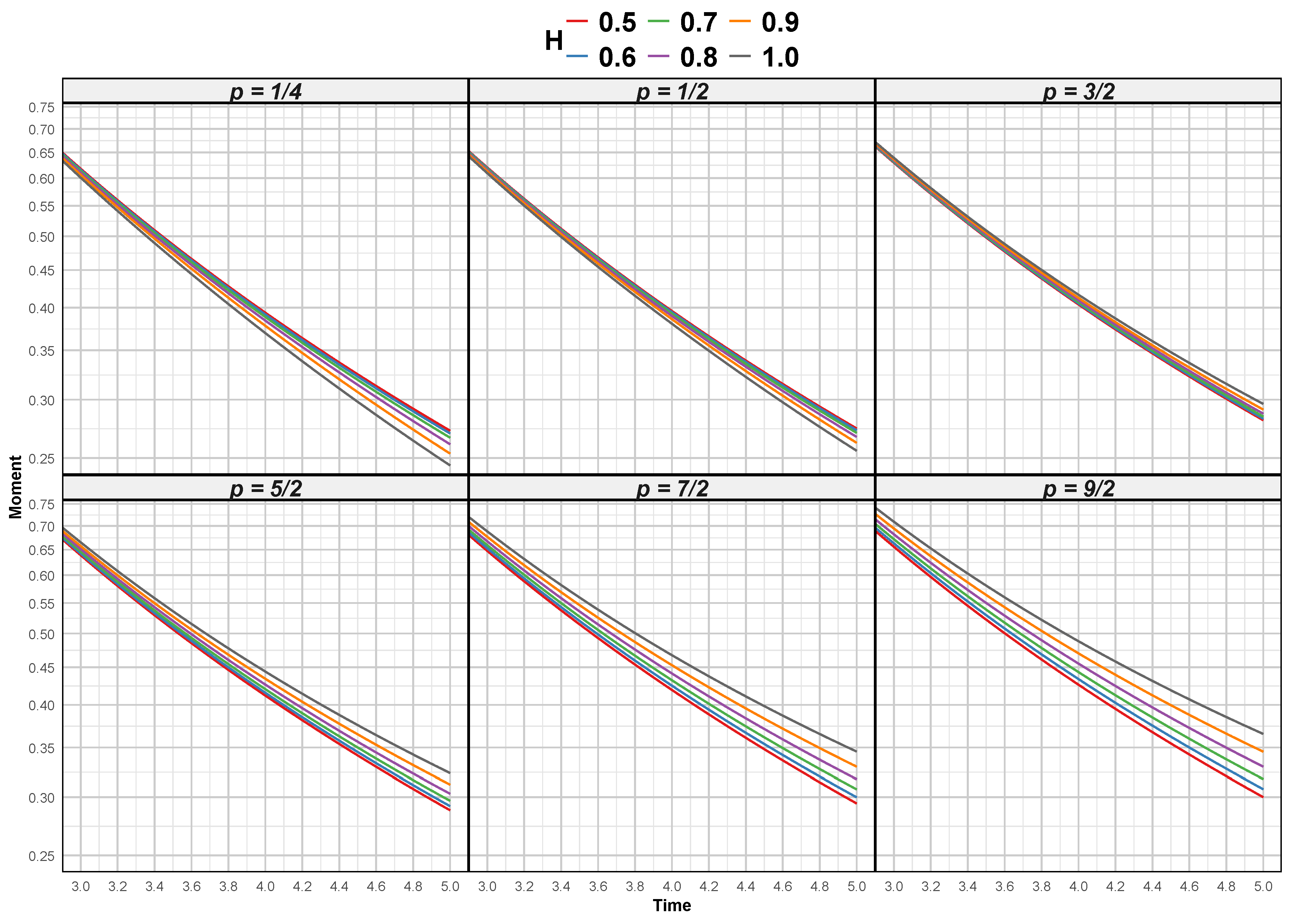
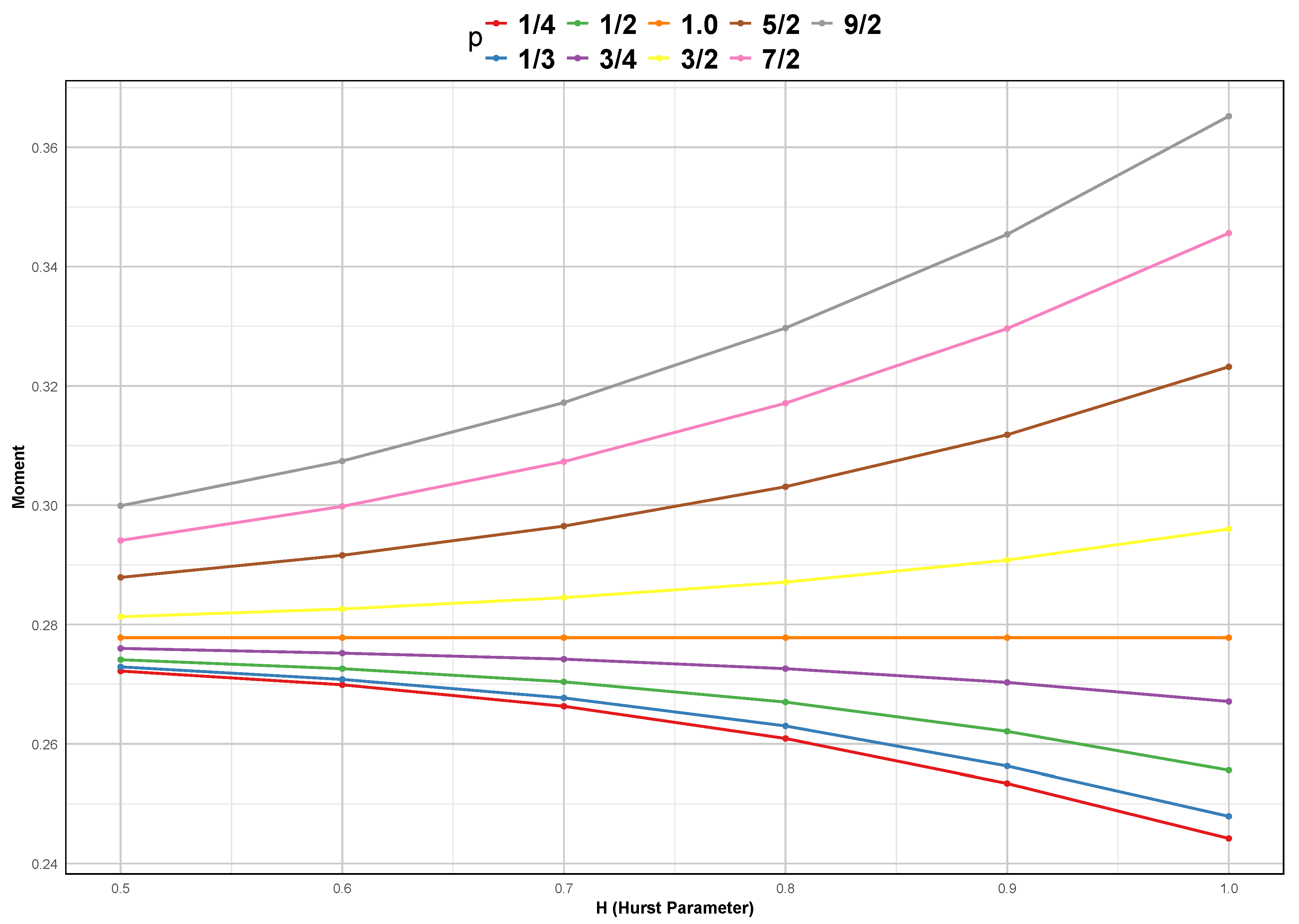
| p | H | Time | ||||||
|---|---|---|---|---|---|---|---|---|
| 0.0 | 1.0 | 2.0 | 3.0 | 4.0 | 5.0 | |||
| 0.5 | 10.0000 | 2.4905 | 1.1026 | 0.6177 | 0.3937 | 0.2722 | ||
| 0.6 | 10.0000 | 2.4905 | 1.1013 | 0.6158 | 0.3915 | 0.2699 | ||
| 0.7 | 10.0000 | 2.4905 | 1.0998 | 0.6134 | 0.3885 | 0.2663 | ||
| 0.8 | 10.0000 | 2.4905 | 1.0980 | 0.6103 | 0.3841 | 0.2609 | ||
| 0.9 | 10.0000 | 2.4905 | 1.0960 | 0.6063 | 0.3779 | 0.2534 | ||
| 1.0 | 10.0000 | 2.4905 | 1.0936 | 0.6008 | 0.3694 | 0.2442 | ||
| 0.5 | 10.0000 | 2.4937 | 1.1055 | 0.6202 | 0.3958 | 0.2741 | ||
| 0.6 | 10.0000 | 2.4937 | 1.1046 | 0.6189 | 0.3944 | 0.2726 | ||
| 0.7 | 10.0000 | 2.4937 | 1.1036 | 0.6174 | 0.3925 | 0.2704 | ||
| 0.8 | 10.0000 | 2.4937 | 1.1024 | 0.6154 | 0.3897 | 0.2670 | ||
| 0.9 | 10.0000 | 2.4937 | 1.1011 | 0.6128 | 0.3858 | 0.2621 | ||
| 1.0 | 10.0000 | 2.4937 | 1.0996 | 0.6093 | 0.3804 | 0.2556 | ||
| 0.5 | 10.0000 | 2.5063 | 1.1167 | 0.6297 | 0.4040 | 0.2813 | ||
| 0.6 | 10.0000 | 2.5063 | 1.1175 | 0.6309 | 0.4053 | 0.2826 | ||
| 0.7 | 10.0000 | 2.5063 | 1.1185 | 0.6323 | 0.4070 | 0.2845 | ||
| 0.8 | 10.0000 | 2.5063 | 1.1196 | 0.6341 | 0.4093 | 0.2871 | ||
| 0.9 | 10.0000 | 2.5063 | 1.1208 | 0.6364 | 0.4124 | 0.2908 | ||
| 1.0 | 10.0000 | 2.5063 | 1.1223 | 0.6393 | 0.4165 | 0.2960 | ||
| 0.5 | 10.0000 | 2.5186 | 1.1276 | 0.6388 | 0.4117 | 0.2879 | ||
| 0.6 | 10.0000 | 2.5186 | 1.1300 | 0.6421 | 0.4153 | 0.2916 | ||
| 0.7 | 10.0000 | 2.5186 | 1.1327 | 0.6462 | 0.4200 | 0.2965 | ||
| 0.8 | 10.0000 | 2.5186 | 1.1359 | 0.6513 | 0.4261 | 0.3031 | ||
| 0.9 | 10.0000 | 2.5186 | 1.1395 | 0.6574 | 0.4339 | 0.3118 | ||
| 1.0 | 10.0000 | 2.5186 | 1.1436 | 0.6650 | 0.4438 | 0.3232 | ||
| 0.5 | 10.0000 | 2.5542 | 1.1758 | 0.6672 | 0.4295 | 0.3036 | ||
| 0.6 | 10.0000 | 2.5542 | 1.1784 | 0.6710 | 0.4336 | 0.3080 | ||
| 0.7 | 10.0000 | 2.5542 | 1.1814 | 0.6753 | 0.4385 | 0.3134 | ||
| 0.8 | 10.0000 | 2.5542 | 1.1850 | 0.6804 | 0.4443 | 0.3199 | ||
| 0.9 | 10.0000 | 2.5542 | 1.1891 | 0.6864 | 0.4511 | 0.3276 | ||
| 1.0 | 10.0000 | 2.5542 | 1.1937 | 0.6933 | 0.4590 | 0.3366 | ||
| 0.5 | 10.0000 | 2.5664 | 1.1862 | 0.6755 | 0.4363 | 0.3094 | ||
| 0.6 | 10.0000 | 2.5664 | 1.1890 | 0.6795 | 0.4407 | 0.3141 | ||
| 0.7 | 10.0000 | 2.5664 | 1.1922 | 0.6839 | 0.4459 | 0.3197 | ||
| 0.8 | 10.0000 | 2.5664 | 1.1960 | 0.6892 | 0.4519 | 0.3265 | ||
| 0.9 | 10.0000 | 2.5664 | 1.2003 | 0.6955 | 0.4589 | 0.3345 | ||
| 1.0 | 10.0000 | 2.5664 | 1.2051 | 0.7027 | 0.4669 | 0.3438 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guidoum, A.C.; Almulhim, F.A.; Bassoudi, M.; Boukhetala, K.; Alamari, M.B. Fractional and Higher Integer-Order Moments for Fractional Stochastic Differential Equations. Symmetry 2025, 17, 665. https://doi.org/10.3390/sym17050665
Guidoum AC, Almulhim FA, Bassoudi M, Boukhetala K, Alamari MB. Fractional and Higher Integer-Order Moments for Fractional Stochastic Differential Equations. Symmetry. 2025; 17(5):665. https://doi.org/10.3390/sym17050665
Chicago/Turabian StyleGuidoum, Arsalane Chouaib, Fatimah A. Almulhim, Mohammed Bassoudi, Kamal Boukhetala, and Mohammed B. Alamari. 2025. "Fractional and Higher Integer-Order Moments for Fractional Stochastic Differential Equations" Symmetry 17, no. 5: 665. https://doi.org/10.3390/sym17050665
APA StyleGuidoum, A. C., Almulhim, F. A., Bassoudi, M., Boukhetala, K., & Alamari, M. B. (2025). Fractional and Higher Integer-Order Moments for Fractional Stochastic Differential Equations. Symmetry, 17(5), 665. https://doi.org/10.3390/sym17050665










