Quantum Steering and Fidelity in a Two-Photon System Subjected to Symmetric and Asymmetric Phasing Interactions
Abstract
1. Introduction
2. Non-Markovian Dynamics in Quantum Systems
3. Quantum Steering and Fidelity
3.1. Quantum Steering
- If then no steering can be observed; this is known as no-way steering.
- If either or then one-way steering occurs, meaning that only one party can steer the other.
- If both and then two-way steering is present. However, this does not imply symmetry in the degree of steerability. The steering can be asymmetric, such that . In this case, mode can steer mode and vice versa, but with possibly different strengths. The bipartite modes described by the covariance matrix thus exhibit directional steerability in both directions.
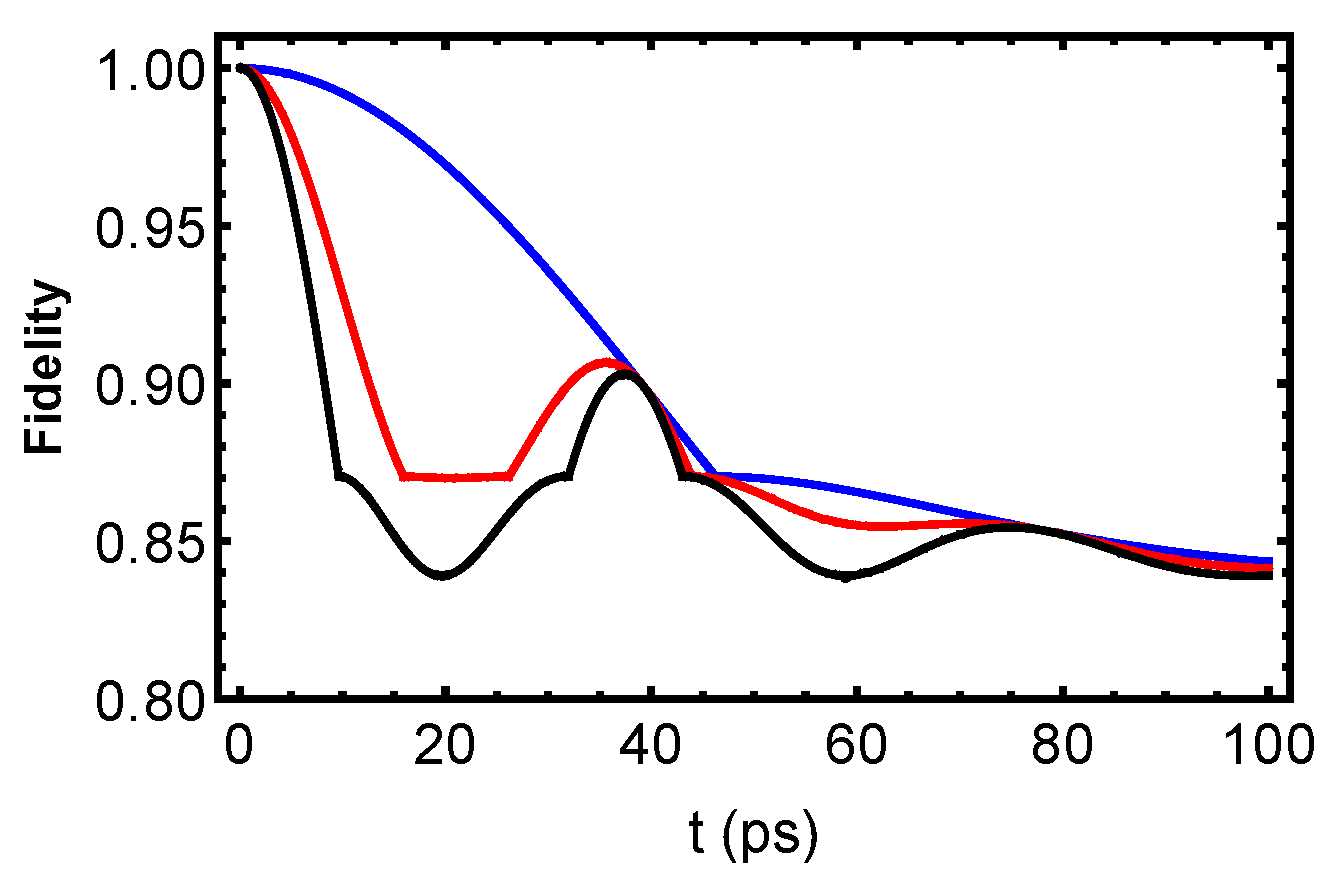
3.2. Fidelity
4. Initial Photon States and Data Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar] [CrossRef]
- Ekert, A.K. Quantum cryptography based on Bell’s theorem. Phys. Rev. Lett. 1991, 67, 661–663. [Google Scholar] [CrossRef] [PubMed]
- Acín, A.; Brunner, N.; Gisin, N.; Massar, S.; Pironio, S.; Scarani, V. Device-independent security of quantum cryptography against collective attacks. Phys. Rev. Lett. 2007, 98, 230501. [Google Scholar] [CrossRef] [PubMed]
- Giovannetti, V.; Lloyd, S.; Maccone, L. Advances in quantum metrology. Nat. Photonics 2011, 5, 222–229. [Google Scholar] [CrossRef]
- Modi, K.; Brodutch, A.; Cable, H.; Paterek, T.; Vedral, V. The classical-quantum boundary for correlations: Discord and related measures. Rev. Mod. Phys. 2012, 84, 1655–1707. [Google Scholar] [CrossRef]
- Horodecki, R.; Horodecki, P.; Horodecki, M.; Horodecki, K. Quantum entanglement. Rev. Mod. Phys. 2009, 81, 865–942. [Google Scholar] [CrossRef]
- Brunner, N.; Cavalcanti, D.; Pironio, S.; Scarani, V.; Wehner, S. Bell nonlocality. Rev. Mod. Phys. 2014, 86, 419–478. [Google Scholar] [CrossRef]
- Schrödinger, E. Discussion of probability relations between separated systems. Math. Proc. Cambridge Philos. Soc. 1935, 31, 555–563. [Google Scholar] [CrossRef]
- Schrödinger, E. Probability relations between separated systems. Math. Proc. Cambridge Philos. Soc. 1936, 32, 446–452. [Google Scholar] [CrossRef]
- Wiseman, H.M.; Jones, S.J.; Doherty, A.C. Steering, Entanglement, Nonlocality, and the Einstein-Podolsky-Rosen Paradox. Phys. Rev. Lett. 2007, 98, 140402. [Google Scholar] [CrossRef]
- Skrzypczyk, P.; Navascués, M.; Cavalcanti, D. Quantifying Einstein-Podolsky-Rosen Steering. Phys. Rev. Lett. 2014, 112, 180404. [Google Scholar] [CrossRef]
- Einstein, A.; Podolsky, B.; Rosen, N. Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 1935, 47, 777–780. [Google Scholar] [CrossRef]
- Reid, M.D.; Drummond, P.; Bowen, W.; Cavalcanti, E.G.; Lam, P.K.; Bachor, H.; Andersen, U.L.; Leuchs, G. Colloquium: The Einstein-Podolsky-Rosen paradox: From concepts to applications. Rev. Mod. Phys. 2009, 81, 1727–1751. [Google Scholar] [CrossRef]
- Bell, J.S. On the Einstein Podolsky Rosen paradox. Physics 1964, 1, 195–200. [Google Scholar] [CrossRef]
- Bell, J.S. Epistemological Letters. Epistemol. Lett. 1976, 9, 11–19. [Google Scholar] [CrossRef]
- Aspect, A.; Grangier, P.; Roger, G. Experimental test of Bell’s inequalities using time-varying analyzers. Phys. Rev. Lett. 1982, 49, 91–94. [Google Scholar] [CrossRef]
- Reid, M.D. Tests of the Einstein-Podolsky-Rosen paradox. Physics 2023, 16, 92. [Google Scholar] [CrossRef]
- Bennet, A.J.; Evans, D.A.; Saunders, D.J.; Branciard, C.; Cavalcanti, E.G.; Wiseman, H.M.; Pryde, G.J. Arbitrarily loss-tolerant Einstein-Podolsky-Rosen steering allowing a demonstration over 1 km of optical fiber with no detection loophole. Phys. Rev. A 2012, 85, QT4A.4. [Google Scholar] [CrossRef]
- Schiavon, M.; Vallone, G.; Villoresi, P. Experimental realization of equiangular three-state quantum key distribution. Sci. Rep. 2016, 6, 020101. [Google Scholar] [CrossRef]
- Wittmann, B.; Ramelow, S.; Steinlechner, F.; Langford, N.K.; Brunner, N.; Wiseman, H.M.; Ursin, R.; Zeilinger, A. Loophole-free Einstein–Podolsky–Rosen experiment via quantum steering. New J. Phys. 2012, 14, 053030. [Google Scholar] [CrossRef]
- Piani, M.; Watrous, J. Necessary and Sufficient Quantum Information Characterization of Einstein-Podolsky-Rosen Steering. Phys. Rev. Lett. 2015, 114, 060404. [Google Scholar] [CrossRef]
- He, Q. Quantum steering and its applications in quantum information. In Proceedings of the Quantum and Nonlinear Optics IX, Online, 5–11 December 2022; p. 503. [Google Scholar] [CrossRef]
- Kocsis, S.; Hall, M.J.W.; Bennet, A.J.; Saunders, D.J.; Pryde, G.J. Experimental measurement-device-independent verification of quantum steering. Nat. Commun. 2015, 6, 5886. [Google Scholar] [CrossRef]
- Saunders, D.J.; Jones, S.J.; Wiseman, H.M.; Pryde, G.J. Experimental EPR-Steering using Bell-local States. Nat. Phys. 2010, 6, 845. [Google Scholar] [CrossRef]
- Eberle, T.; Händchen, V.; Duhme, J.; Franz, T.; Werner, R.F.; Schnabel, R. Strong Einstein-Podolsky-Rosen entanglement from a single squeezed light source. Phys. Rev. A 2011, 83, 052329. [Google Scholar] [CrossRef]
- Händchen, V.; Eberle, T.; Steinlechner, S.; Samblowski, A.; Franz, T.; Werner, R.F.; Schnabel, R. Observation of one-way Einstein–Podolsky–Rosen steering. Nat. Photonics 2012, 6, 596–599. [Google Scholar] [CrossRef]
- Smith, D.H.; Gillett, G.; de Almeida, M.P.; Branciard, C.; Fedrizzi, A.; Weinhold, T.J.; Lita, A.; Calkins, B.; Gerrits, T.; Wiseman, H.M.; et al. Conclusive quantum steering with superconducting transition-edge sensors. Nat. Commun. 2012, 3, 625. [Google Scholar] [CrossRef] [PubMed]
- Steinlechner, S.; Bauchrowitz, J.; Eberle, T.; Schnabel, R. Strong EPR Steering with Unbalanced Homodyne Detection. Phys. Rev. A 2013, 87, 022104. [Google Scholar] [CrossRef]
- Sun, K.; Xu, J.S.; Ye, X.J.; Wu, Y.C.; Chen, J.L.; Li, C.F.; Guo, G.C. Experimental Demonstration of the Einstein-Podolsky-Rosen Steering Game Based on the All-Versus-Nothing Proof. Phys. Rev. Lett. 2014, 113, 140402. [Google Scholar] [CrossRef]
- Cavalcanti, E.G.; Jones, S.J.; Wiseman, H.M.; Reid, M.D. Experimental criteria for steering and the Einstein-Podolsky-Rosen paradox. Phys. Rev. A 2009, 80, 032112. [Google Scholar] [CrossRef]
- Walborn, S.P.; Salles, A.; Gomes, R.M.; Toscano, F.; Ribeiro, P.H.S. Revealing Hidden Einstein-Podolsky-Rosen Nonlocality. Phys. Rev. Lett. 2011, 106, 130402. [Google Scholar] [CrossRef]
- Schneeloch, J.; Broadbent, C.J.; Walborn, S.P.; Cavalcanti, E.G.; Howell, J.C. Einstein-Podolsky-Rosen steering inequalities from entropic uncertainty relations. Phys. Rev. A 2013, 87, 062103. [Google Scholar] [CrossRef]
- Cavalcanti, D.; Skrzypczyk, P.; Aguilar, G.; Nery, R.V.; Ribeiro, P.S.; Walborn, S. Detection of entanglement in asymmetric quantum networks and multipartite quantum steering. Nat. Commun. 2015, 6, 7941. [Google Scholar] [CrossRef]
- Pramanik, T.; Kaplan, M.; Majumdar, A.S. Fine-grained Einstein-Podolsky-Rosen steering inequalities. Phys. Rev. A 2014, 90, 050305(R). [Google Scholar] [CrossRef]
- Olsen, M.K. Pseudo-steady-state non-Gaussian Einstein-Podolsky-Rosen steering of massive particles in pumped and damped Bose-Hubbard dimers. Phys. Rev. A 2013, 95, 052313. [Google Scholar] [CrossRef]
- He, Q.Y.; Reid, M.D. Genuine Multipartite Einstein-Podolsky-Rosen Steering. Phys. Rev. Lett. 2013, 111, 250403. [Google Scholar] [CrossRef] [PubMed]
- Reid, M.D. Monogamy inequalities for the Einstein-Podolsky-Rosen paradox and quantum steering. Phys. Rev. A 2013, 88, 062108. [Google Scholar] [CrossRef]
- Bowles, J.; Vértesi, T.; Quintino, M.T.; Brunner, N. One-way Einstein-Podolsky-Rosen Steering. Phys. Rev. Lett. 2014, 112, 200402. [Google Scholar] [CrossRef]
- Plenio, M.B.; Virmani, S. An introduction to entanglement measures. Quantum Inf. Comput. 2007, 7, 1. [Google Scholar] [CrossRef]
- Xu, J.S.; Li, C.F.; Gong, M.; Zou, X.B.; Shi, C.H.; Chen, G.; Guo, G.C. Experimental Demonstration of Photonic Entanglement Collapse and Revival. Phys. Rev. Lett. 2010, 104, 100502. [Google Scholar] [CrossRef]
- Bylicka, B.; Chruściński, D.; Maniscalco, S. Non-Markovianity and reservoir memory of quantum channels: A quantum information theory perspective. Sci. Rep. 2014, 4, 5720. [Google Scholar] [CrossRef]
- Breuer, H.P.; Laine, E.M.; Piilo, J. Measure for the Degree of Non-Markovian Behavior of Quantum Processes in Open Systems. Phys. Rev. Lett. 2009, 103, 210401. [Google Scholar] [CrossRef]
- Rivas, Á.; Huelga, S.F.; Plenio, M.B. Quantum Non-Markovianity: Characterization, Quantification and Detection. Rep. Progr. Phys. 2014, 77, 094001. [Google Scholar] [CrossRef] [PubMed]
- de Vega, I.; Alonso, D. Dynamics of Non-Markovian Open Quantum Systems. Rev. Mod. Phys. 2017, 89, 015001. [Google Scholar] [CrossRef]
- Li, L.; Hall, M.J.W.; Wiseman, H.M. Concepts of Quantum Non-Markovianity: A Hierarchy. Phys. Rep. 2018, 759, 1–51. [Google Scholar] [CrossRef]
- Deffner, S.; Lutz, E. Quantum Speed Limit for Non-Markovian Dynamics. Phys. Rev. Lett. 2013, 111, 010402. [Google Scholar] [CrossRef]
- Liu, B.H.; Li, L.; Huang, Y.F.; Li, C.F.; Guo, G.C.; Laine, E.M.; Breuer, H.P.; Piilo, J. Experimental control of the transition from Markovian to non-Markovian dynamics of open quantum systems. Nat. Phys. 2011, 7, 931–934. [Google Scholar] [CrossRef]
- Tang, J.S.; Li, C.F.; Li, Y.L.; Zou, X.B.; Guo, G.C.; Breuer, H.P.; Laine, E.M.; Piilo, J. Measuring non-Markovianity of processes with controllable system-environment interaction. Europhys. Lett. 2012, 97, 10002. [Google Scholar] [CrossRef]
- Gu, W.; Li, T.; Tian, Y.; Yi, Z.; Li, G.x. Two-photon dynamics in non-Markovian waveguide QED with a giant atom. Phys. Rev. A 2024, 110, 033707. [Google Scholar] [CrossRef]
- Laine, E.M.; Breuer, H.P.; Piilo, J.; Li, C.F.; Guo, G.C. Erratum: Nonlocal Memory Effects in the Dynamics of Open Quantum Systems. Phys. Rev. Lett. 2013, 111, 229901. [Google Scholar] [CrossRef]
- Wootters, W.K. Optimal Information Transfer and Real-Vector-Space Quantum Theory. In Quantum Theory: Informational Foundations and Foils; Springer: Dordrecht, The Netherlands, 1983; pp. 21–43. [Google Scholar] [CrossRef]
- Kogias, I.; Lee, A.R.; Ragy, S.; Adesso, G. Quantification of Gaussian quantum steering. Phys. Rev. Lett. 2015, 114, 060403. [Google Scholar] [CrossRef]
- Loeve, M.; Renyi, A. Foundations of Probability. Am. Math. Mon. 1971, 78, 1037. [Google Scholar] [CrossRef]
- Wilde, M.M. Quantum Information Theory; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar] [CrossRef]
- Adesso, G.; Girolami, D.; Serafini, A. Measuring Gaussian Quantum Information and Correlations Using the Rényi Entropy of Order 2. Phys. Rev. Lett. 2012, 109, 190502. [Google Scholar] [CrossRef]
- Bougouffa, S.; Al-Hmoud, M.; Hakami, J.W. Probing quantum correlations in a hybrid optomechanical system. Int. J. Theor. Phys. 2022, 61, 190. [Google Scholar] [CrossRef]
- Bennett, C.H.; Wiesner, S.J. Communication via one-and two-particle operators on Einstein-Podolsky-Rosen states. Phys. Rev. lett. 1992, 69, 2881. [Google Scholar] [CrossRef] [PubMed]
- Bouwmeester, D.; Pan, J.W.; Mattle, K.; Eibl, M.; Weinfurter, H.; Zeilinger, A. Experimental quantum teleportation. Nature 1997, 390, 575–579. [Google Scholar] [CrossRef]
- Furusawa, A.; Sørensen, J.L.; Braunstein, S.L.; Fuchs, C.A.; Kimble, H.J.; Polzik, E.S. Unconditional quantum teleportation. Science 1998, 282, 706–709. [Google Scholar] [CrossRef]
- Collins, D.; Gisin, N. A relevant two qubit Bell inequality inequivalent to the CHSH inequality. J. Phys. A 2004, 37, 1775. [Google Scholar] [CrossRef]
- Munro, W.J.; James, D.F.; White, A.G.; Kwiat, P.G. Maximizing the entanglement of two mixed qubits. Phys. Rev. A 2001, 64, 030302. [Google Scholar] [CrossRef]
- Werner, R.F. Quantum states with Einstein-Podolsky-Rosen correlations admitting a hidden-variable model. Phys. Rev. A 1989, 40, 4277. [Google Scholar] [CrossRef]
- Wei, T.C.; Altepeter, J.B.; Branning, D.; Goldbart, P.M.; James, D.; Jeffrey, E.; Kwiat, P.G.; Mukhopadhyay, S.; Peters, N.A. Synthesizing arbitrary two-photon polarization mixed states. Phys. Rev. A—At. Mol. Opt. Phys. 2005, 71, 032329. [Google Scholar] [CrossRef]
- Xu, K.; Liu, L.; Wang, N.N.; Zhang, C.; Huang, Y.F.; Liu, B.H.; Cheng, S.; Li, C.F.; Guo, G.C. Experimental verification of the steering ellipsoid zoo via two-qubit states. Phys. Rev. A 2024, 109, 032415. [Google Scholar] [CrossRef]
- Cavalcanti, D.; Skrzypczyk, P. Quantitative relations between measurement incompatibility, quantum steering, and nonlocality. Phys. Rev. A 2016, 93, 052112. [Google Scholar] [CrossRef]
- Bartkiewicz, K.; Černoch, A.; Lemr, K.; Miranowicz, A.; Nori, F. Experimental temporal quantum steering. Sci. Rep. 2016, 6, 38076. [Google Scholar] [CrossRef] [PubMed]
- Quintino, M.T.; Vértesi, T.; Brunner, N. Joint Measurability, Einstein-Podolsky-Rosen Steering, and Bell Nonlocality. Phys. Rev. Lett. 2014, 113, 160402. [Google Scholar] [CrossRef]
- Li, Z.Z.; Yip, C.T.; Li, B. Spectral signatures of the Markovian to Non-Markovian transition in open quantum systems. arXiv 2024, arXiv:2409.14661. [Google Scholar] [CrossRef]
- Liu, F.; Zhou, X.; Zhou, Z.W. Memory effect and non-Markovian dynamics in an open quantum system. Phys. Rev. A 2019, 99, 052119. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berrada, K.; Bougouffa, S. Quantum Steering and Fidelity in a Two-Photon System Subjected to Symmetric and Asymmetric Phasing Interactions. Symmetry 2025, 17, 647. https://doi.org/10.3390/sym17050647
Berrada K, Bougouffa S. Quantum Steering and Fidelity in a Two-Photon System Subjected to Symmetric and Asymmetric Phasing Interactions. Symmetry. 2025; 17(5):647. https://doi.org/10.3390/sym17050647
Chicago/Turabian StyleBerrada, Kamal, and Smail Bougouffa. 2025. "Quantum Steering and Fidelity in a Two-Photon System Subjected to Symmetric and Asymmetric Phasing Interactions" Symmetry 17, no. 5: 647. https://doi.org/10.3390/sym17050647
APA StyleBerrada, K., & Bougouffa, S. (2025). Quantum Steering and Fidelity in a Two-Photon System Subjected to Symmetric and Asymmetric Phasing Interactions. Symmetry, 17(5), 647. https://doi.org/10.3390/sym17050647







