Explanation of the Mass Pattern of the Low-Lying Scalar Nonet

Abstract

The aim of this work is to propose an explanation of the inverse mass hierarchy of the low-lying nonet of the scalar mesons in the framework of the massless Nambu–Jona-Lasinio $U_R\left(3\right)\times U_L\left(3\right)$ quark model. The proposed explanation is based on symmetry principles. The collective meson states are described via quark–antiquark pairs, whose condensates lead simultaneously to spontaneous breaking of chiral and flavour symmetry. It is shown that, due to flavour symmetry breaking, two iso-doublets of $K_0^{*}\left(700\right)$ mesons play the role of Goldstone bosons. It is also proven that there exists a solution with degenerate masses of the $a_0\left(980\right)$ and $f_0\left(980\right)$ mesons and a zero mass of the $f_0\left(500\right)$ meson.

1. Introduction

Symmetries and symmetry breaking have a fundamental role in Physics. It is known that spontaneous symmetry breaking of the chiral $S{U}_R\left(2\right)\times S{U}_L\left(2\right)$ symmetry leads to massless Goldstone bosons, whose role is played by the pions. In the case of the spontaneous symmetry breaking of the $U_f\left(3\right)$ flavour symmetry, $K_0^{*}\left(700\right)$ mesons play the role of massless Goldstone bosons. Further on in this article we will call these bosons Bogoliubov–Nambu–Goldstone (BNG) bosons to highlight the legacy of Bogoliubov [1,2].

In this work, we will consider simultaneous chiral and flavour spontaneous symmetry breaking and their important role for the explanation of the mass spectrum of the low-lying scalar mesons.

The pions, consisting of quark–antiquark pairs ($q\overline{q}$), have a very small mass in comparison with the other hadronic states. The smallness of the pion mass would have been a problem for their constituent quark models, however, it is well known that the small mass of the pseudoscalar pions is explained by the mechanism of spontaneous breaking of the chiral $S{U}_R\left(2\right)\times S{U}_L\left(2\right)$ symmetry [3]. As a result of that symmetry breaking, the pions play the role of massless BNG bosons [4,5].

There exists another problem connected with the mass of the low-lying scalar mesons, namely the explanation of the inverse hierarchy mass in the low-lying scalar nonet. The constituent quark models, describing the mesons as quark–antiquark pairs, could not explain this problem. Therefore, one of the first hypotheses offering an explanation of the inverse hierarchy was the work by Jaffe [6] that proposed a diquark–antidiquark structure ($qq\overline{q}\overline{q}$) for the scalar mesons. Another explanation, which preserves the quark–antiquark structure of scalar mesons [7], uses the $U_A\left(1\right)$ anomaly term in dynamical chiral symmetry breaking of chiral effective theories. However, while this mechanism explains the difference between the lowest-lying scalar mesons $f_0\left(500\right)$ (or $\sigma$) and $a_0\left(980\right)$, it cannot explain the mass of the $K_0^{*}\left(700\right)$ (or $\kappa$) meson [8].

In this paper, we propose an alternative explanation for the smallness of the mass of the $K_0^{*}\left(700\right)$ meson. It is based on the well-known mechanism of spontaneous symmetry breaking of $U_f\left(3\right)$ flavour symmetry to $S{U}_f\left(2\right)$ isotopic symmetry. As a result of this symmetry breaking, $K_0^{*}\left(700\right)$ mesons with isospin $I=1/2$ play the role of massless BNG bosons. According to us, this is a natural explanation of the small masses of the $K_0^{*}\left(700\right)$ mesons, similar to the explanation for the small masses of pions. Note that $S{U}_f\left(2\right)$ isotopic symmetry cannot be spontaneously broken, as shown in [9], i.e., there exists isotopic symmetry in nature.

In Section 2, we introduce a model of self-interaction of scalar quark currents, analogous to the Nambu–Jona-Lasinio (NJL) model [10,11]. We present a quantisation of this model and derive the effective potential for the scalar mesons nonet. In Section 3, we present the minimisation of this effective potential and we obtain the mass states of the scalar mesons. In the Conclusion, we list the basic results and conclusions of this work.

2. The Model

Let us consider a $U_R\left(3\right)\times U_L\left(3\right)$ chiral self-interaction of the scalar quark currents:

$$\mathcal{L}=i\overline{\Psi }\partial /\Psi +{\displaystyle \frac{G_0}{2}}{\left(\overline{\Psi }\Psi \right)}^2+{\displaystyle \frac{{\tilde{G}}_0}{2}}\sum _{a=1}^8{\left(\overline{\Psi }{\lambda }_a\Psi \right)}^2,$$

(1)

where, $\Psi ={\left(uds\right)}^T$ is the triplet of massless color quarks (here, the colour indices are suppressed), while ${\lambda }_a(1,\dots ,8)$ are the Gell-Mann matrices. Here, $G_0$ and ${\tilde{G}}_0$ are positive constants of the self-interaction of the singlet state quark scalar current and the octet states of the quark scalar currents, respectively, and both have a dimension ${\left[mass\right]}^{-2}$. We consider massless quarks, which will obtain masses due to spontaneous symmetry breaking.

It is obvious that the quantisation of this Lagrangian in perturbation theory on the dimensional constants $G_0$ and ${\tilde{G}}_0$ will result in a nonrenormalisable theory. Therefore, we will use the linearisation method of this Lagrangian developed in [12,13]. The linearisation of the Lagrangian (Equation (1)) leads to the following equivalent on the classical level Lagrangian:

$$\mathcal{L}=i\overline{\Psi }\partial /\Psi +g_0\overline{\Psi }\Psi S_0-{\displaystyle \frac{g_0^2}{2G_0}}{S}_0^2+\sum _{a=1}^8\left(g_a\overline{\Psi }{\lambda }_a\Psi S_a-{\displaystyle \frac{g_a^2}{2{\tilde{G}}_0}}{S}_a^2\right)$$

(2)

where we have introduced the auxiliary fields

$$S_0={\displaystyle \frac{G_0}{g_0}}\overline{\Psi }\Psi \mathrm{and}{S}_a={\displaystyle \frac{{\tilde{G}}_0}{g_a}}\overline{\Psi }{\lambda }_a\Psi ,$$

(3)

which will play the role of collective excitation states of the corresponding currents. Here, $g_0$ and $g_a$ are the dimensionless Yukawa coupling constants.

All the collective modes become dynamical as a result of the self-energy quantum corrections from fermion loops (Figure 1a).

Let us consider the self-energy quantum corrections to the scalar field $S_0$:

$$\begin{array}{ccc}\hfill {\Pi }_{00}\left(q\right)& =& i{g}_0^2{N}_C\mathrm{Tr}\left[\mathbb{1}\right]\int {\displaystyle \frac{{\mathrm{d}}^{4}p}{{\left(2\pi \right)}^4}}\mathrm{Tr}\left[{\left(p/-m_0\right)}^{-1}{\left(p/-q/-m_0\right)}^{-1}\right]\hfill \\ & =& 12i{g}_0^2{N}_C\int {\displaystyle \frac{{\mathrm{d}}^{4}p}{{\left(2\pi \right)}^4}}{\displaystyle \frac{1}{p^2-m_0^2}}-6i{g}_0^2{N}_C\int {\displaystyle \frac{{\mathrm{d}}^{4}p}{{\left(2\pi \right)}^4}}{\displaystyle \frac{1}{{\left(p^2-m_0^2\right)}^2}}{q}^2+\mathrm{finite}\mathrm{terms}\mathcal{O}\left(q^4\right)\hfill \\ & =& 12g_0^2{N}_C{I}_2+6g_0^2{N}_C{I}_0{q}^2+\mathrm{finite}\mathrm{terms}\mathcal{O}\left(q^4\right),\hfill \end{array}$$

(4)

and to the scalar fields $S_a$

$$\begin{array}{ccc}\hfill {\Pi }_{ab}\left(q\right)& =& i{g}_a{g}_b{N}_C\mathrm{Tr}\left[{\lambda }_a{\lambda }_b\right]\int {\displaystyle \frac{{\mathrm{d}}^{4}p}{{\left(2\pi \right)}^4}}\mathrm{Tr}\left[{\left(p/-m_0\right)}^{-1}{\left(p/-q/-m_0\right)}^{-1}\right]\hfill \\ & =& 8i{g}_a^2{N}_C{\delta }_{ab}\int {\displaystyle \frac{{\mathrm{d}}^{4}p}{{\left(2\pi \right)}^4}}{\displaystyle \frac{1}{p^2-m_0^2}}-4i{g}_a^2{N}_C{\delta }_{ab}\int {\displaystyle \frac{{\mathrm{d}}^{4}p}{{\left(2\pi \right)}^4}}{\displaystyle \frac{1}{{\left(p^2-m_0^2\right)}^2}}{q}^2+\mathrm{finite}\mathrm{terms}\mathcal{O}\left(q^4\right)\hfill \\ & =& 8g_a^2{N}_C{I}_2{\delta }_{ab}+4g_a^2{N}_C{I}_0{\delta }_{ab}{q}^2+\mathrm{finite}\mathrm{terms}\mathcal{O}\left(q^4\right),\hfill \end{array}$$

(5)

where p is the internal and q is the external momentum, $N_C$ denotes the number of colours, and

$$I_2\equiv i\int {\displaystyle \frac{{\mathrm{d}}^{4}p}{{\left(2\pi \right)}^4}}{\displaystyle \frac{1}{p^2-m_0^2}}=\int {\displaystyle \frac{{\mathrm{d}}^4{p}_E}{{\left(2\pi \right)}^4}}{\displaystyle \frac{1}{p_E^2+m_0^2}}>0$$

(6)

is the quadratically divergent integral, while

$$I_0\equiv -i\int {\displaystyle \frac{{\mathrm{d}}^{4}p}{{\left(2\pi \right)}^4}}{\displaystyle \frac{1}{{\left(p^2-m_0^2\right)}^2}}=\int {\displaystyle \frac{{\mathrm{d}}^4{p}_E}{{\left(2\pi \right)}^4}}{\displaystyle \frac{1}{{\left(p_E^2+m_0^2\right)}^2}}>0$$

(7)

is a logarithmically divergent integral, which are both positive in Euclidean momentum space.

Here, we have introduced the small current mass $m_0$ for the quark, which will help to avoid the infrared divergences in the denominator and which will be neglected in the numerator. This kind of quark mass introduction explicitly breaks the chiral symmetry, and is called soft symmetry breaking. Such symmetry breaking does not cause extra ultraviolet divergences in the scalar particles masses. Due to the dimensionless of the Yukawa coupling constants $g_0$ and $g_a$ in the four dimensional space, there exist only two types of divergent integrals (6) and (7). The ultraviolet divergences in (6) and (7) are removed using one of the known regularisation methods.

The first terms in the last lines of Equations (4) and (5) represent the corrections to the mass terms of the scalar fields $S_0$: ${\mu }^2=g_0^2/G_0-12g_0^2{N}_C{I}_2$ and $S_a$: ${\tilde{\mu }}^2=g_a^2/{\tilde{G}}_0-8g_a^2{N}_C{I}_2$. The second terms represent the kinetic parts of the scalar fields. For a correct normalisation of the scalar fields wave functions, the following requirements should be fulfilled:

$$6g_0^2{N}_C{I}_0=4g_a^2{N}_C{I}_0=1.$$

(8)

Due to the dynamical origin of the kinetic terms, all the interactions in the NJL model are descried by a single dimensionless coupling constant

$$g\equiv g_0=\sqrt{2/3}{g}_a.$$

(9)

Another essential point of the NJL model is the generation of the self-interactions of the scalar fields; these self-interactions lead to a spontaneous dynamical breaking of the chiral symmetry. Let us denote by □ the integral on the internal momentum p, which comes from the fermionic loop in Figure 1b:

$$\square =\int {\displaystyle \frac{d^{4}p}{{\left(2\pi \right)}^4}}\mathrm{Tr}\left[{\left(p/-m_0\right)}^{-1}{\left(p/-m_0\right)}^{-1}{\left(p/-m_0\right)}^{-1}{\left(p/-m_0\right)}^{-1}\right].$$

(10)

Thus, at a quantum level, due to the radiative corrections (Figure 1b) in the effective Lagrangian, local terms appear for the self-interaction of the scalar mesons with zero external momentum and corresponding symmetry factors:

$${\square }_{0000}\left(0\right)={\displaystyle \frac{i}{4}}{g}_0^4{N}_C\mathrm{Tr}\left[\mathbb{1}\right]\square =-3g_0^4{N}_C{I}_0+\mathcal{O}\left(m_0^2\right)\approx -{\displaystyle \frac{1}{2}}{g}^2;$$

(11)

$${\square }_{00aa}\left(0\right)={\displaystyle \frac{3i}{2}}{g}_0^2{g}_a^2{N}_C\mathrm{Tr}\left[{\lambda }_a^2\right]\square =-12g_0^2{g}_a^2{N}_C{I}_0+\mathcal{O}\left(m_0^2\right)\approx -3g^2;$$

(12)

$$\begin{array}{c}\hfill {\square }_{0aab}\left(0\right)=3i{g}_0{g}_a^2{g}_b{N}_C\mathrm{Tr}\left[{\lambda }_a^2{\lambda }_b\right]\square =-12g_0{g}_a^2{g}_b{N}_C{I}_0{d}^{aab}+\mathcal{O}\left(m_0^2\right)\\ \hfill \approx \left\{\begin{array}{ccc}\hfill -3\sqrt{{\displaystyle \frac{3}{2}}}{g}^2,& \mathrm{for}\hfill & \hfill a=4,5\mathrm{and}b=3;\\ \hfill 3\sqrt{{\displaystyle \frac{3}{2}}}{g}^2,& \mathrm{for}\hfill & \hfill a=6,7\mathrm{and}b=3;\\ \hfill -3\sqrt{2}{g}^2,& \mathrm{for}\hfill & \hfill a=1,2,3\mathrm{and}b=8;\\ \hfill {\displaystyle \frac{3}{\sqrt{2}}}{g}^2,& \mathrm{for}\hfill & \hfill a=4,5,6,7\mathrm{and}b=8;\end{array}\right.\end{array}$$

(13)

$${\square }_{0888}\left(0\right)=i{g}_0{g}_8^3{N}_C\mathrm{Tr}\left[{\lambda }_8^3\right]\square ={\displaystyle \frac{8}{\sqrt{3}}}{g}_0{g}_8^3{N}_C{I}_0+\mathcal{O}\left(m_0^2\right)\approx \sqrt{2}{g}^2;$$

(14)

$${\square }_{0abc}\left(0\right)=3i{g}_0{g}_a{g}_b{g}_c{N}_C\mathrm{Tr}\left[{\lambda }_a\{{\lambda }_b,{\lambda }_c\}\right]\square =-48g_0{g}_a{g}_b{g}_c{N}_C{I}_0{d}^{abc}+\mathcal{O}\left(m_0^2\right)\approx -6\sqrt{6}{g}^2{d}^{abc};$$

(15)

$${\square }_{aaaa}\left(0\right)={\displaystyle \frac{i}{4}}{g}_a^4{N}_C\mathrm{Tr}\left[{\lambda }_a^4\right]\square =-2g_a^4{N}_C{I}_0+\mathcal{O}\left(m_0^2\right)\approx -{\displaystyle \frac{3}{4}}{g}^2;$$

(16)

$${\square }_{aabb}\left(0\right)={\displaystyle \frac{i}{2}}{g}_a^2{g}_b^2{N}_C\mathrm{Tr}\left[{\lambda }_a^2{\lambda }_b^2+{\lambda }_a{\lambda }_b\{{\lambda }_a,{\lambda }_b\}\right]\square =-4g_a^2{g}_b^2{N}_C{I}_0+\mathcal{O}\left(m_0^2\right)\approx -{\displaystyle \frac{3}{2}}{g}^2.$$

(17)

Here, $d^{abc}$ are totally symmetric structure constants of $su\left(3\right)$ algebra, where the indices a, b and c obtain different values non-equal to each others.

The last expressions (11)–(17) were obtained using the normalisation condition (8), the relation between coupling constants (9), and neglecting the small current quark mass $m_0$, which was introduced only to remove infrared divergencies. The normalisation condition (8) requires that the leading term in the effective Lagrangian consists only of the divergent terms of the diagrams with zero external momentum [14], depicted in Figure 1b.

Thus, the effective potential reads:

$$\begin{array}{ccc}\hfill V_{\mathrm{eff}}& =& {\displaystyle \frac{{\mu }^2}{2}}{S}_0^2+{\displaystyle \frac{{\tilde{\mu }}^2}{2}}\sum _{a=1}^8{S}_a^2+{\displaystyle \frac{g^2}{2}}{S}_0^4+3g^2{S}_0^2\sum _{a=1}^8{S}_a^2+{\displaystyle \frac{3g^2}{4}}{\left(\sum _{a=1}^8{S}_a^2\right)}^2\hfill \\ & +& {\displaystyle \frac{3\sqrt{3}}{\sqrt{2}}}{g}^2{S}_0{S}_3\left(S_4^2+S_5^2-S_6^2-S_7^2\right)-\sqrt{2}{g}^2{S}_0{S}_8^3\hfill \\ & +& 3\sqrt{2}{g}^2{S}_0{S}_8\left(S_1^2+S_2^2+S_3^2-{\displaystyle \frac{S_4^2+S_5^2+S_6^2+S_7^2}{2}}\right)\hfill \\ & +& 3\sqrt{6}{g}^2{S}_0\left(S_1{S}_4{S}_6+S_1{S}_5{S}_7-S_2{S}_4{S}_7+S_2{S}_5{S}_6\right).\hfill \end{array}$$

(18)

The advantage of this potential is that it depends only on one dimensionless constant $g^2$ and two dimensional parameters of the mass: ${\mu }^2$ and ${\tilde{\mu }}^2$. This allows exact solutions of spontaneous symmetry breaking and the mass spectrum of the scalar mesons to be found, which will be presented in the next section.

3. Symmetry Breaking and Mass Spectrum of Scalar Mesons

Due to the spontaneous symmetry breaking, the scalar fields obtain non-zero vacuum expectation values. The physical vacuum must conserve the electric charge and the quark flavour. Therefore, only the scalar fields $S_0$, $S_3$ and $S_8$, which interact with the diagonal combinations of quark–antiquark flavours, can have non-zero vacuum expectation values. In order to find the minimum of the effective potential (18), we will differentiate the potential only on these degrees of freedom. The minimisation leads to the following system of equations:

$$\left\{\begin{array}{c}{\displaystyle \frac{\partial V_{\mathrm{eff}}}{\partial S_0}}{{\displaystyle |}}_{\stackrel{S_0=\langle S_0\rangle }{\begin{array}{c}{S}_3=\langle S_3\rangle \\ S_8=\langle S_8\rangle \end{array}}}={\mu }^2\langle S_0\rangle +2g^2{\langle S_0\rangle }^3+6g^2\langle S_0\rangle \left({\langle S_3\rangle }^2+{\langle S_8\rangle }^2\right)\hfill \\ -\sqrt{2}{g}^2{\langle S_8\rangle }^3+3\sqrt{2}{g}^2{\langle S_3\rangle }^2\langle S_8\rangle =0,\hfill \\ {\displaystyle \frac{\partial V_{\mathrm{eff}}}{\partial S_3}}{{\displaystyle |}}_{\stackrel{S_0=\langle S_0\rangle }{\begin{array}{c}{S}_3=\langle S_3\rangle \\ S_8=\langle S_8\rangle \end{array}}}=\left[{\tilde{\mu }}^2+3g^2\left(2{\langle S_0\rangle }^2+{\langle S_3\rangle }^2+{\langle S_8\rangle }^2+2\sqrt{2}\langle S_0\rangle \langle S_8\rangle \right)\right]\langle S_3\rangle =0,\hfill \\ {\displaystyle \frac{\partial V_{\mathrm{eff}}}{\partial S_8}}{{\displaystyle |}}_{\stackrel{S_0=\langle S_0\rangle }{\underset{S_8=\langle S_8\rangle }{S_3=\langle S_3\rangle }}}={\tilde{\mu }}^2\langle S_8\rangle +3g^2\left(2{\langle S_0\rangle }^2+{\langle S_3\rangle }^2+{\langle S_8\rangle }^2-\sqrt{2}\langle S_0\rangle \langle S_8\rangle \right)\langle S_8\rangle \hfill \\ +3\sqrt{2}{g}^2\langle S_0\rangle {\langle S_3\rangle }^2=0.\hfill \end{array}\right.$$

(19)

Note that for $a=1,2,4,5,6,7$, $\langle S_a\rangle =0$ due to neutrality of vacuum expectation values. It has also been shown in [9], that the $S{U}_f\left(2\right)$ group cannot be spontaneously broken and $\langle S_3\rangle =0$ is the right solution of the system (19), while $\langle S_0\rangle \ne 0$ and $\langle S_8\rangle \ne 0$ acquire non-zero vacuum expectation values.

Spontaneous symmetry breaking is possible only at strong coupling constants $G_0$ and ${\tilde{G}}_0$, when the massive parameters have negative values, i.e., ${\mu }^2<0$ and ${\tilde{\mu }}^2<0$. To avoid the irrational coefficients and the dimensional parameters in (19), we introduce the dimensionless variables $x=3\sqrt{2}g\langle S_0\rangle /\sqrt{-{\tilde{\mu }}^2}$, $z=3g\langle S_8\rangle /\sqrt{-{\tilde{\mu }}^2}$ and $r^2={\mu }^2/{\tilde{\mu }}^2$. Then, the constituent quark masses can be obtained from the following relations:

$$\begin{array}{ccc}\hfill m_u=m_d& =& -\sqrt{{\displaystyle \frac{-{\tilde{\mu }}^2}{18}}}(x+z),\hfill \end{array}$$

(20)

$$\begin{array}{ccc}\hfill m_s& =& -\sqrt{{\displaystyle \frac{-{\tilde{\mu }}^2}{18}}}(x-2z).\hfill \end{array}$$

(21)

The first and the third equations of the system (19) in the new dimensionless variables read:

$$\left\{\begin{array}{c}{x}^2+6z^2-2{\displaystyle \frac{z^3}{x}}=9r^2,\hfill \\ x^2-xz+z^2=3.\hfill \end{array}\right.$$

(22)

Before solving this system, let us first find the square of the masses of the scalar meson isotriplet $a_0^{\pm }\left(980\right)=(S_1\mp i{S}_2)/\sqrt{2}$, $a_0^0\left(980\right)=S_3$:

$${\mathcal{M}}_i^2={\displaystyle \frac{{\partial }^2{V}_{\mathrm{eff}}}{\partial S_i^2}}{{\displaystyle |}}_{\underset{S_8=\langle S_8\rangle }{S_0=\langle S_0\rangle }}=9\sqrt{2}{g}^2\langle S_0\rangle \langle S_8\rangle =(-{\tilde{\mu }}^2)xz,\mathrm{where}i=1,2,3.$$

(23)

This result shows that for non negative square masses, the vacuum expectation values of $S_0$ and $S_8$ should have the same signs. We should note that the solutions of the system (22) are invariant with respect to simultaneous sign changing of the vacuum expectation values: $x\to -x$ and $z\to -z$. Therefore, for definiteness, we will search solutions for positive vacuum expectation values.

The solutions of the second equation of the system (22) are:

$$z_{\pm }={\displaystyle \frac{x\pm \sqrt{3(4-x^2)}}{2}}.$$

(24)

Substituting these solutions into the first equation of the system (22), the following expression is obtained:

$$\pm \sqrt{4-x^2}\left(x^2-1\right)=\sqrt{3}ax,\mathrm{where}a=r^2-1.$$

(25)

In order to solve this equation analytically, it is necessary to square its right and left hand sides. Thus, using the substitution $x^2=t$, a cubic equation follows:

$$4-9t+6t^2-t^3=3a^{2}t.$$

(26)

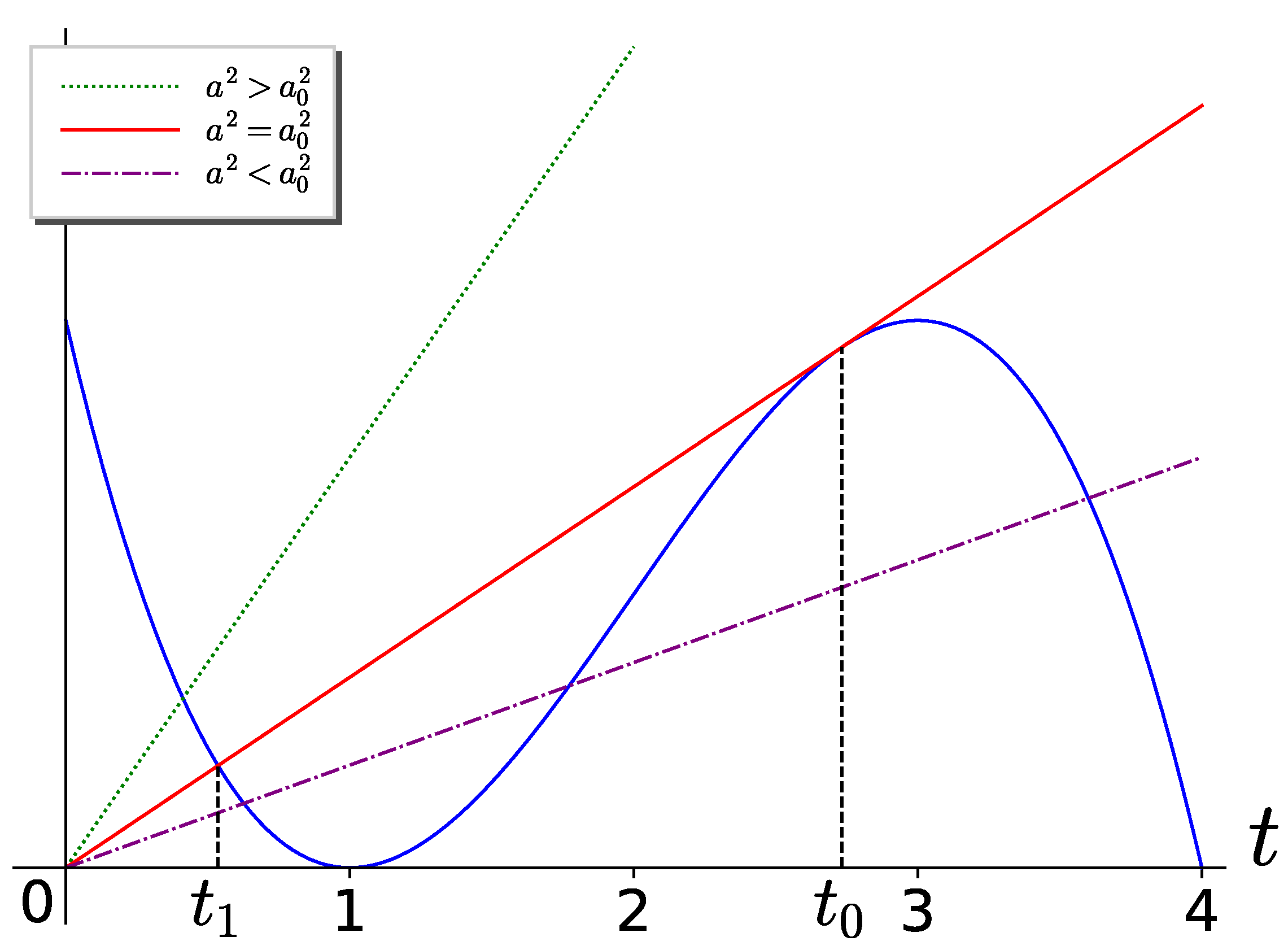
The solutions of this equation are illustrated in Figure 2, where $t_1=2-2\sqrt[3]{6\sqrt{3}-10}$ and $t_0=1+\sqrt{3}$.

It is obvious from Figure 2, that three real roots exist for $0<a^2<a_0^2$, where $a_0^2=2\sqrt{3}-3$. Vice versa, for $a^2>a_0^2$, only one real root exists. For $a^2=0$ and $a^2=a_0^2$, we obtain only two real roots. From Equation (25), it follows that $x^2\le 4$.

Let us describe all the exact solutions of Equation (26), dividing them into three intervals, such that within each interval, one single solution exists. The first case corresponds to the interval $0<x^2\le 1$. This interval consists of two subintervals, namely $0<x^2\le t_1$ and $t_1\le x^2\le 1$. In the first subinterval, the solution with one real root for $a^2\ge a_0^2$ is:

$$x^2=2-\sqrt[3]{3a^2-1+\sqrt{Q}}-\sqrt[3]{3a^2-1-\sqrt{Q}},\mathrm{where}Q=a^2(a^4+6a^2-3).$$

(27)

In the other subinterval, the solution for $0\le a^2\le a_0^2$ reads:

$$x^2=2+2\sqrt{1-a^2}cos\left({\displaystyle \frac{1}{3}}arccos{\displaystyle \frac{1-3a^2}{\sqrt{{(1-a^2)}^3}}}+{\displaystyle \frac{2\pi }{3}}\right).$$

(28)

This solution corresponds to one of the three real roots of Equation (26).

In the second case, corresponding to the interval $1\le x^2\le t_0$, the solution for $0\le a^2\le a_0^2$ reads:

$$x^2=2+2\sqrt{1-a^2}cos\left({\displaystyle \frac{1}{3}}arccos{\displaystyle \frac{1-3a^2}{\sqrt{{(1-a^2)}^3}}}-{\displaystyle \frac{2\pi }{3}}\right).$$

(29)

In the third case, corresponding to the interval $t_0\le x^2\le 4$, the solution for $0\le a^2\le a_0^2$ reads:

$$x^2=2+2\sqrt{1-a^2}cos\left({\displaystyle \frac{1}{3}}arccos{\displaystyle \frac{1-3a^2}{\sqrt{{(1-a^2)}^3}}}\right).$$

(30)

Let us now calculate all the masses of the scalar meson nonet. We have already carried this out for the isotriplet $a_0\left(980\right)$ (23). The squares of the masses of the scalar mesons $\kappa$ with isospin $I=1/2$: ${\kappa }^{\pm }=(S_4\mp i{S}_5)/\sqrt{2}$, ${\kappa }^0=(S_6-i{S}_7)/\sqrt{2}$, ${\overline{\kappa }}^0=(S_6+i{S}_7)/\sqrt{2}$

$${\mathcal{M}}_s^2={\displaystyle \frac{{\partial }^2{V}_{\mathrm{eff}}}{\partial S_s^2}}{{\displaystyle |}}_{\begin{array}{c}{S}_0=\langle S_0\rangle \\ S_8=\langle S_8\rangle \end{array}}={\tilde{\mu }}^2+3g^2\left(2{\langle S_0\rangle }^2-\sqrt{2}\langle S_0\rangle \langle S_8\rangle +{\langle S_8\rangle }^2\right)=0$$

(31)

are equal to zero, where $s=4,5,6,7$ and the last equality follows from the third equation of the system (19) with $\langle S_3\rangle =0$. The fact that the squares of the masses are equal to zero for these states is a direct consequence of the Goldstone theorem [4,5].

Now let us calculate the squares of the masses of the isosinglet states with isospin $I=0$, $S_0$ and $S_8$:

$${\mathcal{M}}_0^2={\displaystyle \frac{{\partial }^2{V}_{\mathrm{eff}}}{\partial S_0^2}}{{\displaystyle |}}_{\begin{array}{c}{S}_0=\langle S_0\rangle \\ S_8=\langle S_8\rangle \end{array}}={\mu }^2+6g^2\left({\langle S_0\rangle }^2+{\langle S_8\rangle }^2\right)=(-{\tilde{\mu }}^2){\displaystyle \frac{2}{9}}\left(x^2+{\displaystyle \frac{z^3}{x}}\right),$$

(32)

$$\begin{array}{c}\hfill {\mathcal{M}}_8^2={\displaystyle \frac{{\partial }^2{V}_{\mathrm{eff}}}{\partial S_8^2}}{{\displaystyle |}}_{\begin{array}{c}{S}_0=\langle S_0\rangle \\ S_8=\langle S_8\rangle \end{array}}={\tilde{\mu }}^2+3g^2\left(2{\langle S_0\rangle }^2-2\sqrt{2}\langle S_0\rangle \langle S_8\rangle +3{\langle S_8\rangle }^2\right)\\ \hfill =(-{\tilde{\mu }}^2){\displaystyle \frac{z}{3}}\left(2z-x\right),\end{array}$$

(33)

$${\mathcal{M}}_{08}^2={\displaystyle \frac{{\partial }^2{V}_{\mathrm{eff}}}{\partial S_0\partial S_8}}{{\displaystyle |}}_{\begin{array}{c}{S}_0=\langle S_0\rangle \\ S_8=\langle S_8\rangle \end{array}}=3g^2\left(4\langle S_0\rangle -2\sqrt{2}\langle S_8\rangle \right)\langle S_8\rangle =(-{\tilde{\mu }}^2){\displaystyle \frac{\sqrt{2}z}{3}}\left(2x-z\right)\ne 0.$$

(34)

From the last equation, it follows that there exists a non-trivial mixing between these states. This can be described by the non-diagonal matrix:

$${\mathcal{M}}_{I=0}^2=(-{\tilde{\mu }}^2)\left(\begin{array}{cc}A& B\\ B& C\end{array}\right),$$

(35)

where $A={\displaystyle \frac{2}{9}}\left(x^2+{\displaystyle \frac{z^3}{x}}\right)$, $B={\displaystyle \frac{\sqrt{2}}{3}}z\left(2x-z\right)$, $C={\displaystyle \frac{1}{3}}z\left(2z-x\right)$. The diagonalisation of this matrix leads to two eigenvalues:

$${\mathcal{M}}_{\sigma }^2=(-{\tilde{\mu }}^2){\displaystyle \frac{A+C-\sqrt{{(A-C)}^2+4B^2}}{2}},$$

(36)

$${\mathcal{M}}_{f_0\left(980\right)}^2=(-{\tilde{\mu }}^2){\displaystyle \frac{A+C+\sqrt{{(A-C)}^2+4B^2}}{2}}.$$

(37)

These correspond to the squares of the masses of the physical states $\sigma$ and $f_0\left(980\right)$.

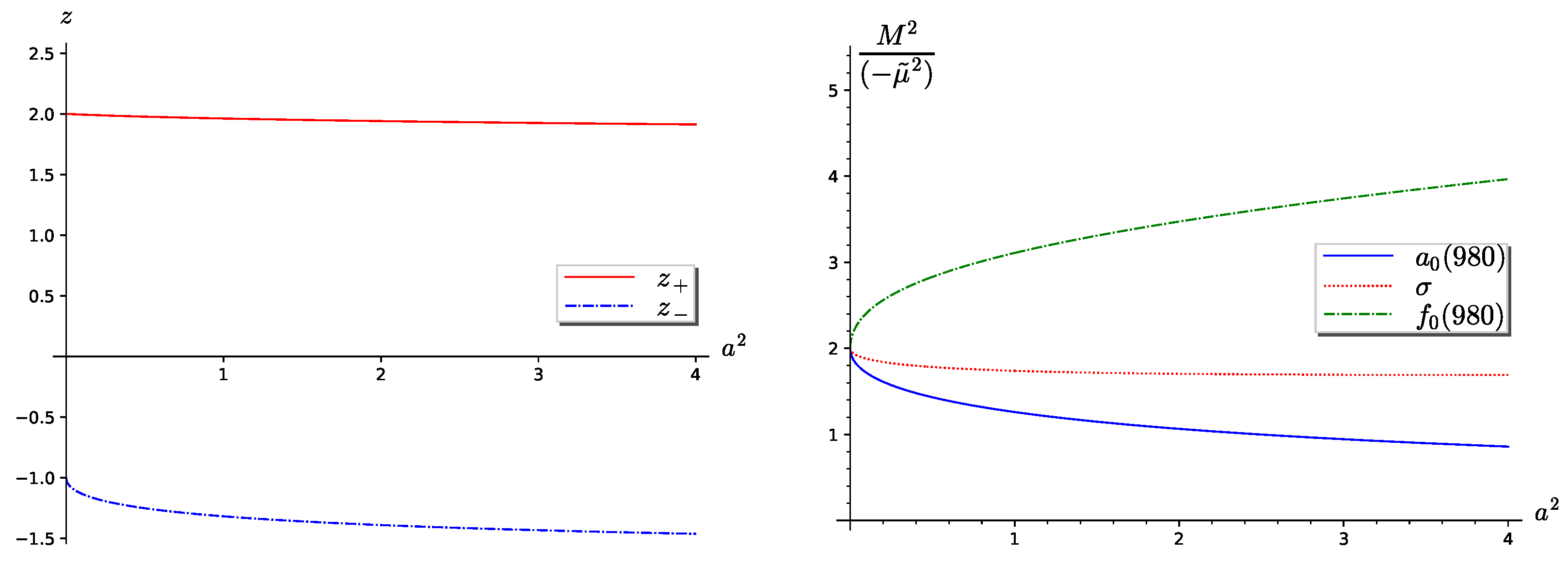
Let us analyse the obtained solutions (27)–(30), keeping in mind that the squares of masses of $\sigma$, $f_0\left(980\right)$ and $a_0\left(980\right)$ mesons should be non-negative. In the first case, the solutions (27) and (28), are illustrated in Figure 3. The left panel of the figure presents the solutions of Equation (24). From the panel, it is obvious that only the solution $z_{+}$ is positive. For positive x, this leads to positive masses of the scalar isotriplet $a_0\left(980\right)$. The right panel presents the reduced squares of the masses of the meson isotriplet $a_0\left(980\right)$ and the two isosinglets, $\sigma$ and $f_0\left(980\right)$. Thus, it is seen from the right panel, that this case does not correspond to physical masses, due to the unphysical pattern ${\mathcal{M}}_{a_0\left(980\right)}<{\mathcal{M}}_{\sigma }<{\mathcal{M}}_{f_0\left(980\right)}$.

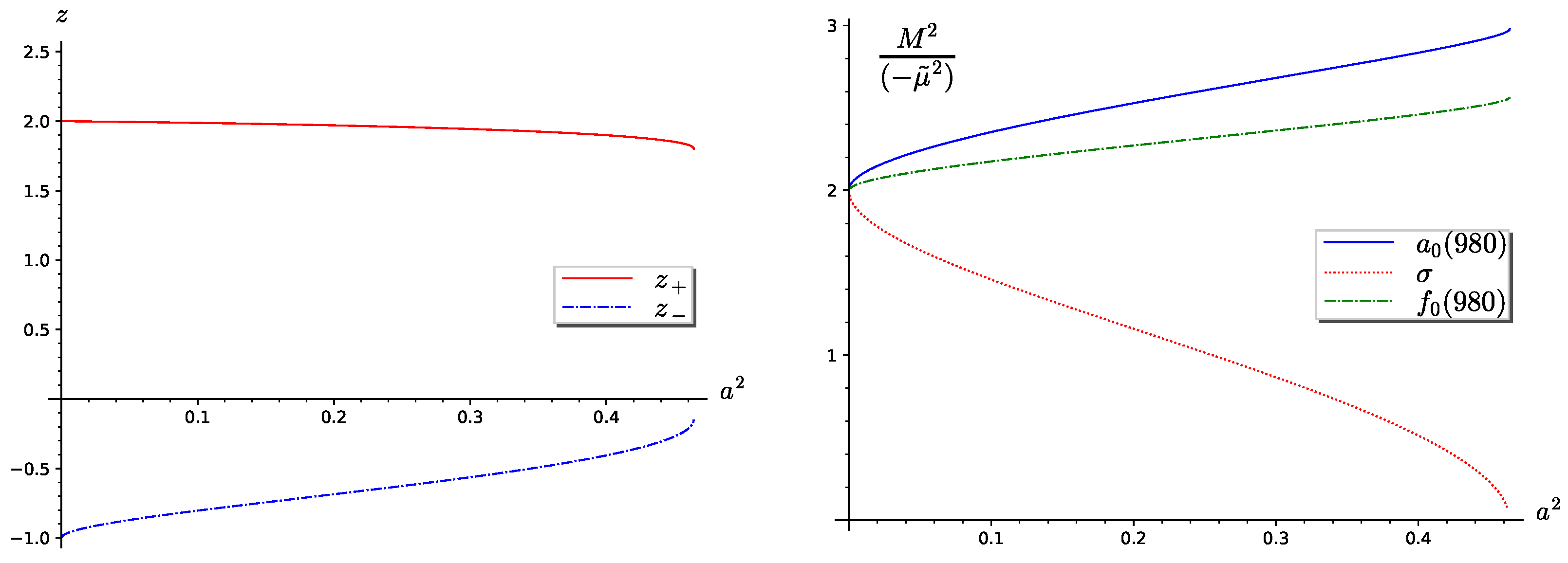
The second case, solution (29), is illustrated in Figure 4. The left panel of the figure presents the solutions of Equation (24). Again, only solution $z_{+}$ is positive. The right panel presents the reduced squares of the masses of the meson isotriplet and the two isosinglets. This result is more attractive from a physical point of view. Namely, for $a^2\to a_0^2$, the masses of the isotriplet $a_0\left(980\right)$ and isosinglet $f_0\left(980\right)$ are almost degenerate and heavy, while the $\sigma$ meson mass tends to zero, and it equals zero for $a^2=a_0^2$. From Equation (25), it follows that for the case $z_{+}>0$, the parameter a is positive: $a>0$. So, for the second case, the mass ratio ${\mu }^2/{\tilde{\mu }}^2=1+a>1$. This mass ratio depends on the initial coupling constants in the Lagrangian (1) and this means that $G_0$ is greater than ${\tilde{G}}_0$.

Let us analyse the mixing at $a=a_0=\sqrt{2\sqrt{3}-3}$. The physical states $\sigma$ and $f_0\left(980\right)$ are defined by the states $S_0$ and $S_8$ from the following relation:

$$\left(\begin{array}{c}\sigma \\ f_0\end{array}\right)=\left(\begin{array}{cc}cos\phi & -sin\phi \\ sin\phi & cos\phi \end{array}\right)\left(\begin{array}{c}{S}_0\\ S_8\end{array}\right),$$

(38)

where the mixing angle $\phi$ is

$$\phi ={\displaystyle \frac{1}{2}}arccos{\displaystyle \frac{C-A}{\sqrt{{(C-A)}^2+4B^2}}}.$$

(39)

The corresponding illustration of this mixing angle is presented in Figure 5.

The formula connecting the pure states $(u\overline{u}+d\overline{d})/\sqrt{2}$ and $s\overline{s}$ to the physical states $\sigma$ and $f_0\left(980\right)$ reads:

$$\left(\begin{array}{c}\sigma \\ f_0\end{array}\right)=\left(\begin{array}{cc}cos\omega & sin\omega \\ -sin\omega & cos\omega \end{array}\right)\left(\begin{array}{c}s\overline{s}\\ {\displaystyle \frac{u\overline{u}+d\overline{d}}{\sqrt{2}}}\end{array}\right),$$

(40)

where the mixing angle $\omega$ is related to the mixing angle $\phi$ by the relation:

$$\omega =arctan\sqrt{2}-\phi .$$

(41)

It is interesting to note that at point $a=a_0$, $\omega ={\omega }_0$, where ${\omega }_0\approx 54.7°-47.5°=7.2°$. This very small angle corresponds to a nearly ideal mixing. However, contrary to the common assumption, that the $f_0\left(980\right)$ meson has an almost pure $s\overline{s}$ state, we obtain that actually, it is the $\sigma$ meson which has an almost pure $s\overline{s}$ state, while the $f_0\left(980\right)$ meson is close to a $(u\overline{u}+d\overline{d})/\sqrt{2}$ state.

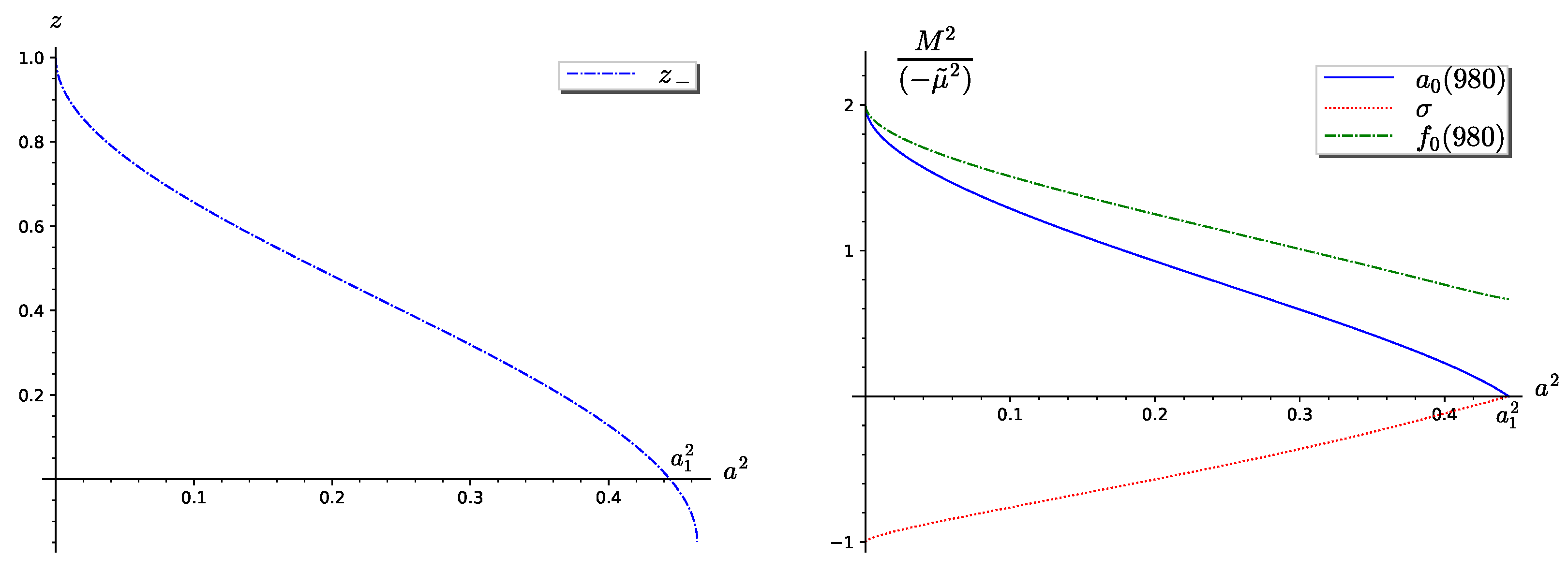
In the third case, solution (30), both the solutions $z_{+}$ and $z_{-}$ can be positive. Therefore, we will consider separately both cases. Figure 6 presents the solution $z_{+}$, while in Figure 7,  $z_{-}$ solution is presented. The solution $z_{-}$ is non-negative for $0\le a^2\le a_1^2={(-2/3)}^2$.

However, as is seen from the right panels of these figures, the square of the mass of the $\sigma$ meson is negative in the whole range of variation of parameter $a^2$, except for point $a^2=a_0^2$ in Figure 6 and point $a^2=a_1^2$ in Figure 7, at which points the mass of the $\sigma$ meson is zero. The first point is included in the second case, as an intermediate point between the second and third cases, and already have been considered as physical solution, while at the second point in the right panel of Figure 7, the mass of the $a_0\left(980\right)$ meson is also zero, which does not correspond to a physical case. Negative values of the mass of the $\sigma$ meson do not correspond to the minimum of the potential.

In conclusion, only the second case, illustrated in Figure 4, corresponds to a physical solution.

4. Conclusions and Discussion

In this article, we propose an explanation for the inverted mass hierarchy of the low-lying nonet of the scalar mesons. This is a new type of explanation. The explanation is provided in the framework of the quark–antiquark NJL model. In particular, the chiral symmetry $U_R\left(3\right)\times U_L\left(3\right)$ is broken to $U_V\left(3\right)$, which in its turn, is broken simultaneously to $SU\left(2\right)$. The latter type of spontaneous symmetry breaking has not been considered before. Remarkably, this type of symmetry breaking leads to the appearance of BNG bosons, which results into zero masses for the $\kappa$ mesons. Moreover, our model suggests also a zero mass for the $\sigma$ meson. We have also determined that the $\sigma$ meson state consists almost completely of a $s\overline{s}$ quark combination. In previous studies, it was assumed that the $\sigma$ meson consists predominantly of light quarks. We have also shown that $f_0\left(980\right)$ consists predominantly of light quarks, contrary to the previous assumptions that it consists mainly of strange quarks. Thus, the experimentally observed decay of $f_0\left(980\right)$ into two pions is nicely described by our model.

Future analysis should consider the realistic case of non-zero current masses. In order to explain the real mass spectrum, it is necessary to introduce explicit breaking of $SU\left(3\right)$ flavour symmetry due to non-zero current quark masses. The method for the introduction of heavy current mass of the strange quark has been developed in the framework of the chiral model $U\left(1\right)$ with spontaneous symmetry breaking [15]. The results obtained in this work confirmed the mass value of the $h_1\left(1415\right)$ meson predicted in [16]. This meson was experimentally discovered 15 years later [17], with the exact predicted mass value. Such an analysis will be provided elsewhere. Here, we want only to note that during the $U_R\left(3\right)\times U_L\left(3\right)$ symmetry breaking, besides pseudoscalar pions, K mesons, which contain a strange quark, also obtain zero mass.

It is known that the account for the current quark masses, in the case of dominant strange quark mass, leads to a real mass of the pseudoscalar K mesons of the order of 500 MeV. We expect that, similarly to the K mesons case, the $\sigma$ and $\kappa$ mesons, which contain strange quarks, will obtain masses of the same order as those of the K mesons.