Baryon Construction with η′ Meson Field
Abstract
1. Introduction
2. Baryon Construction for
2.1. Skyrmion
2.2. Chiral Bag
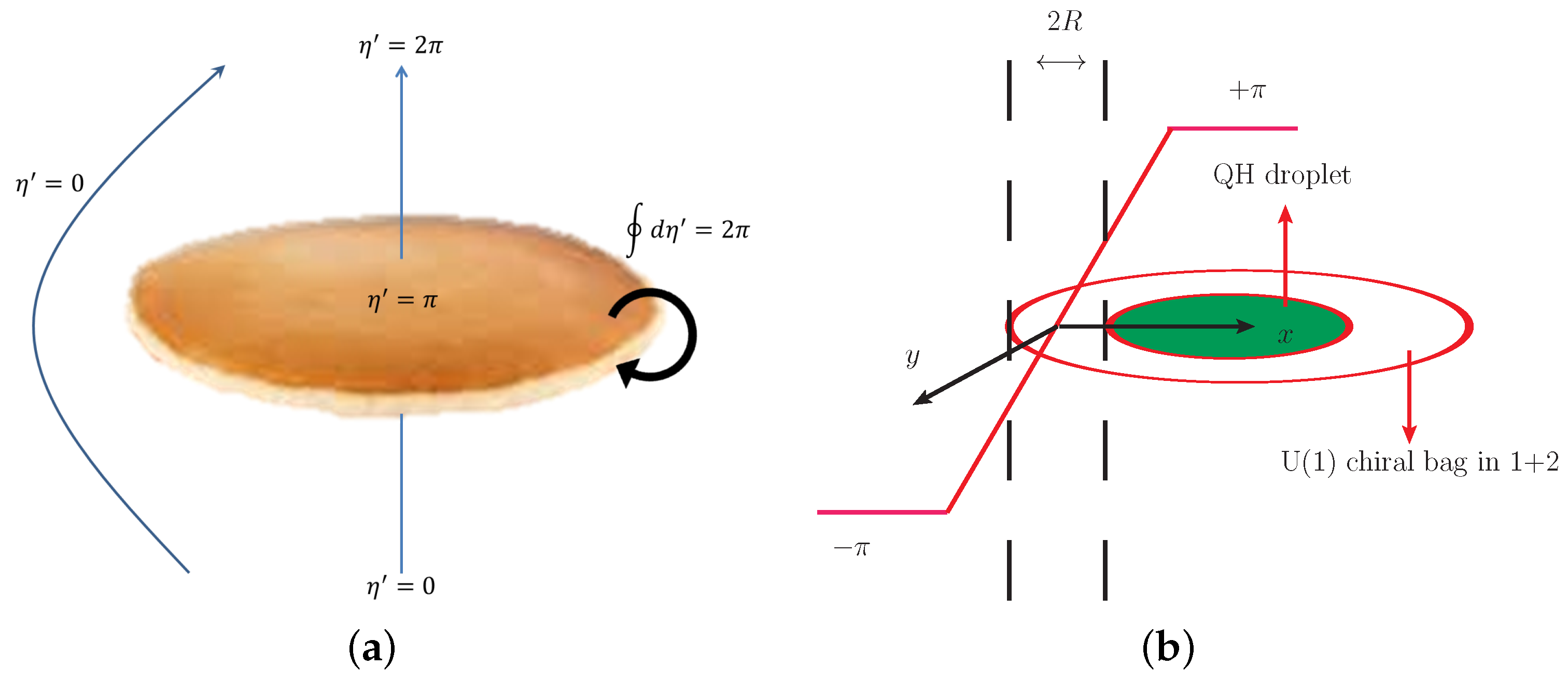
3. Baryons as Quantum Hall Droplets and Quark-Hadron Duality
4. Baryons as Vortices
4.1. Baryons as Vortices for and Particle-Vortex Duality
4.2. Baryons as Vortices for
5. Topological Chiral Bag Model
5.1. Confined Monopoles Inside the Chiral Bag
5.2. Block the Outflow of Color Charge
6. Conclusions and Discussion
Author Contributions
Funding
Conflicts of Interest
References
- Gross, D.J.; Wilczek, F. Ultraviolet Behavior of Nonabelian Gauge Theories. Phys. Rev. Lett. 1973, 30, 1343–1346. [Google Scholar] [CrossRef]
- Politzer, H.D. Reliable Perturbative Results for Strong Interactions? Phys. Rev. Lett. 1973, 30, 1346–1349. [Google Scholar] [CrossRef]
- Marciano, W.J.; Pagels, H. Quantum Chromodynamics: A Review. Phys. Rep. 1978, 36, 137. [Google Scholar] [CrossRef]
- Callan, C.G., Jr.; Coleman, S.R.; Wess, J.; Zumino, B. Structure of phenomenological Lagrangians. 2. Phys. Rev. 1969, 177, 2247–2250. [Google Scholar] [CrossRef]
- Coleman, S.R.; Wess, J.; Zumino, B. Structure of phenomenological Lagrangians. 1. Phys. Rev. 1969, 177, 2239–2247. [Google Scholar] [CrossRef]
- Weinberg, S. Phenomenological Lagrangians. Physica A 1979, 96, 327–340. [Google Scholar] [CrossRef]
- Gasser, J.; Leutwyler, H. Chiral Perturbation Theory to One Loop. Ann. Phys. 1984, 158, 142. [Google Scholar] [CrossRef]
- Gasser, J.; Leutwyler, H. Chiral Perturbation Theory: Expansions in the Mass of the Strange Quark. Nucl. Phys. B 1985, 250, 465–516. [Google Scholar] [CrossRef]
- Weinberg, S. Nuclear forces from chiral Lagrangians. Phys. Lett. B 1990, 251, 288–292. [Google Scholar] [CrossRef]
- Gasser, J.; Sainio, M.E.; Svarc, A. Nucleons with chiral loops. Nucl. Phys. B 1988, 307, 779–853. [Google Scholar] [CrossRef]
- Hatsuda, T.; Kunihiro, T. QCD phenomenology based on a chiral effective Lagrangian. Phys. Rep. 1994, 247, 221–367. [Google Scholar] [CrossRef]
- Bernard, V. Chiral Perturbation Theory and Baryon Properties. Prog. Part. Nucl. Phys. 2008, 60, 82–160. [Google Scholar] [CrossRef]
- Machleidt, R.; Entem, D.R. Chiral effective field theory and nuclear forces. Phys. Rep. 2011, 503, 1–75. [Google Scholar] [CrossRef]
- Skyrme, T.H.R. A Nonlinear field theory. Proc. R. Soc. Lond. A 1961, 260, 127–138. [Google Scholar] [CrossRef]
- Skyrme, T.H.R. A Unified Field Theory of Mesons and Baryons. Nucl. Phys. 1962, 31, 556–569. [Google Scholar] [CrossRef]
- Zahed, I.; Brown, G.E. The Skyrme Model. Phys. Rep. 1986, 142, 1–102. [Google Scholar] [CrossRef]
- Capstick, S.; Isgur, N. Baryons in a relativized quark model with chromodynamics. Phys. Rev. D 1986, 34, 2809–2835. [Google Scholar] [CrossRef]
- Koniuk, R.; Isgur, N. Baryon Decays in a Quark Model with Chromodynamics. Phys. Rev. D 1980, 21, 1868, Erratum in Phys. Rev. D 1981, 23, 818. [Google Scholar] [CrossRef]
- Capstick, S.; Roberts, W. Quark models of baryon masses and decays. Prog. Part. Nucl. Phys. 2000, 45, S241–S331. [Google Scholar] [CrossRef]
- Ma, Y.L.; Harada, M. Lecture notes on the Skyrme model. arXiv 2016, arXiv:1604.04850. [Google Scholar]
- ’t Hooft, G. A Planar Diagram Theory for Strong Interactions. Nucl. Phys. B 1974, 72, 461. [Google Scholar] [CrossRef]
- Witten, E. Baryons in the 1/n Expansion. Nucl. Phys. B 1979, 160, 57–115. [Google Scholar] [CrossRef]
- Adkins, G.S.; Nappi, C.R.; Witten, E. Static Properties of Nucleons in the Skyrme Model. Nucl. Phys. B 1983, 228, 552. [Google Scholar] [CrossRef]
- Witten, E. Global Aspects of Current Algebra. Nucl. Phys. B 1983, 223, 422–432. [Google Scholar] [CrossRef]
- Nadkarni, S.; Nielsen, H.B.; Zahed, I. Bosonization Relations as Bag Boundary Conditions. Nucl. Phys. B 1985, 253, 308–322. [Google Scholar] [CrossRef]
- Nadkarni, S.; Zahed, I. Nonabelian Cheshire Cat Bag Models in (1+1)-dimensions. Nucl. Phys. B 1986, 263, 23–36. [Google Scholar] [CrossRef]
- Rho, M. Cheshire cat hadrons. Phys. Rep. 1994, 240, 1–142. [Google Scholar] [CrossRef]
- Nielsen, H.B.; Rho, M.; Wirzba, A.; Zahed, I. Color anomaly in a hybrid bag model. Phys. Lett. B 1991, 269, 389–393. [Google Scholar] [CrossRef]
- Rho, M.; Goldhaber, A.S.; Brown, G.E. Topological Soliton Bag Model for Baryons. Phys. Rev. Lett. 1983, 51, 747–750. [Google Scholar] [CrossRef]
- Witten, E. Current Algebra Theorems for the U(1) Goldstone Boson. Nucl. Phys. B 1979, 156, 269–283. [Google Scholar] [CrossRef]
- Veneziano, G. U(1) Without Instantons. Nucl. Phys. B 1979, 159, 213–224. [Google Scholar] [CrossRef]
- Vecchia, P.D.; Veneziano, G. Chiral Dynamics in the Large n Limit. Nucl. Phys. B 1980, 171, 253–272. [Google Scholar] [CrossRef]
- Ohta, N. Vacuum Structure and Chiral Charge Quantization in the Large N Limit. Prog. Theor. Phys. 1981, 66, 1408–1421, Erratum in Prog. Theor. Phys. 1982, 67, 993. [Google Scholar] [CrossRef]
- Kawarabayashi, K.; Ohta, N. On the Partial Conservation of the U(1) Current. Prog. Theor. Phys. 1981, 66, 1789. [Google Scholar] [CrossRef]
- Kawarabayashi, K.; Ohta, N. The Problem of η in the Large N Limit: Effective Lagrangian Approach. Nucl. Phys. B 1980, 175, 477–492. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Dichotomy of Baryons as Quantum Hall Droplets and Skyrmions: Topological Structure of Dense Matter. Symmetry 2021, 13, 1888. [Google Scholar] [CrossRef]
- Kapustin, A.; Thorngren, R. Anomalies of discrete symmetries in three dimensions and group cohomology. Phys. Rev. Lett. 2014, 112, 231602. [Google Scholar] [CrossRef]
- Kapustin, A.; Seiberg, N. Coupling a QFT to a TQFT and Duality. J. High Energy Phys. 2014, 2014, 1. [Google Scholar] [CrossRef]
- Gaiotto, D.; Kapustin, A.; Seiberg, N.; Willett, B. Generalized Global Symmetries. J. High Energy Phys. 2015, 2015, 172. [Google Scholar] [CrossRef]
- Gaiotto, D.; Kapustin, A.; Komargodski, Z.; Seiberg, N. Theta, Time Reversal, and Temperature. J. High Energy Phys. 2017, 2017, 91. [Google Scholar] [CrossRef]
- Gaiotto, D.; Komargodski, Z.; Seiberg, N. Time-reversal breaking in QCD4, walls, and dualities in 2 + 1 dimensions. J. High Energy Phys. 2018, 2018, 110. [Google Scholar] [CrossRef]
- Hsin, P.S.; Seiberg, N. Level/rank Duality and Chern-Simons-Matter Theories. J. High Energy Phys. 2016, 2016, 95. [Google Scholar] [CrossRef]
- Komargodski, Z. Baryons as Quantum Hall Droplets. arXiv 2018, arXiv:1812.09253. [Google Scholar]
- Ma, Y.L.; Nowak, M.A.; Rho, M.; Zahed, I. Baryon as a Quantum Hall Droplet and the Cheshire Cat Principle. Phys. Rev. Lett. 2019, 123, 172301. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Towards the hadron–quark continuity via a topology change in compact stars. Prog. Part. Nucl. Phys. 2020, 113, 103791. [Google Scholar] [CrossRef]
- Lin, F.; Ma, Y.L. Baryons as vortexes on the η’ domain wall. J. High Energy Phys. 2024, 2014, 270. [Google Scholar] [CrossRef]
- Zhang, S.C.; Hansson, T.H.; Kivelson, S. An effective field theory model for the fractional quantum hall effect. Phys. Rev. Lett. 1988, 62, 82–85. [Google Scholar] [CrossRef]
- Chodos, A.; Jaffe, R.L.; Johnson, K.; Thorn, C.B.; Weisskopf, V.F. A New Extended Model of Hadrons. Phys. Rev. D 1974, 9, 3471–3495. [Google Scholar] [CrossRef]
- Thomas, A.W. Chiral Symmetry and the Bag Model: A New Starting Point for Nuclear Physics. Adv. Nucl. Phys. 1984, 13, 1–137. [Google Scholar] [CrossRef]
- Chodos, A.; Thorn, C.B. Chiral Hedgehogs in the Bag Theory. Phys. Rev. D 1975, 12, 2733. [Google Scholar] [CrossRef]
- Mulders, P.J. Theoretical Aspects of Hybrid Chiral Bag Models. Phys. Rev. D 1984, 30, 1073. [Google Scholar] [CrossRef]
- Goldman, J.T.; Haymaker, R.W. Dynamically Broken Chiral Symmetry With Bag Confinement. Phys. Rev. D 1981, 24, 724. [Google Scholar] [CrossRef]
- Nadkarni, S.; Nielsen, H.B. Partial Bosonization: The Formalism of Cheshire Cat Bag Models. Nucl. Phys. B 1986, 263, 1–22. [Google Scholar] [CrossRef]
- Perry, R.J.; Rho, M. Removing Bag Dynamics From Chiral Bag Models: An Illustrative Example. Phys. Rev. D 1986, 34, 1169–1175. [Google Scholar] [CrossRef]
- Damgaard, P.H.; Nielsen, H.B.; Sollacher, R. Smooth bosonization: The Cheshire cat revisited. Nucl. Phys. B 1992, 385, 227–250. [Google Scholar] [CrossRef]
- Nielsen, H.B.; Rho, M.; Wirzba, A.; Zahed, I. The tale of the eta-prime from the cheshire cat principle. Phys. Lett. B 1992, 281, 345–350. [Google Scholar] [CrossRef]
- Lin, F.; Ma, Y.L. Confined Monopoles in Chiral Bag. arXiv 2025, arXiv:2501.16653. [Google Scholar]
- Wess, J.; Zumino, B. Consequences of anomalous Ward identities. Phys. Lett. B 1971, 37, 95–97. [Google Scholar] [CrossRef]
- Karasik, A. From Skyrmions to One Flavored Baryons and Beyond. Symmetry 2022, 14, 2347. [Google Scholar] [CrossRef]
- Niemi, A.J.; Semenoff, G.W. Fermion Number Fractionization in Quantum Field Theory. Phys. Rep. 1986, 135, 99. [Google Scholar] [CrossRef]
- Battye, R.; Manton, N.S.; Sutcliffe, P. Skyrmions and the alpha-particle model of nuclei. Proc. R. Soc. Lond. A 2007, 463, 261–279. [Google Scholar] [CrossRef]
- Park, B.Y.; Vento, V. Skyrmion approach to finite density and temperature. arXiv 2009, arXiv:0906.3263. [Google Scholar] [CrossRef]
- Brown, G.E.; Rho, M. The Multifaceted Skyrmion; World Scientific: Singapore, 2010; ISBN 978-981-4280-69-3. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Recent progress on dense nuclear matter in skyrmion approaches. Sci. China Phys. Mech. Astron. 2017, 60, 032001. [Google Scholar] [CrossRef]
- Naya, C.; Sutcliffe, P. Skyrmions and clustering in light nuclei. Phys. Rev. Lett. 2018, 121, 232002. [Google Scholar] [CrossRef]
- Manton, N.S. Skyrmions—A Theory of Nuclei; World Scientific: Singapore, 2022; ISBN 978-1-80061-247-1. [Google Scholar] [CrossRef]
- Wang, J.S.; Ma, Y.L. Vector meson effects on multi-Skyrmion states from the rational map ansatz. Sci. China Phys. Mech. Astron. 2023, 66, 112011. [Google Scholar] [CrossRef]
- Kugler, M.; Shtrikman, S. A New Skyrmion Crystal. Phys. Lett. B 1988, 208, 491–494. [Google Scholar] [CrossRef]
- Kugler, M.; Shtrikman, S. Skyrmion Crystals and Their Symmetries. Phys. Rev. D 1989, 40, 3421. [Google Scholar] [CrossRef]
- Goldhaber, A.S.; Manton, N.S. Maximal Symmetry of the Skyrme Crystal. Phys. Lett. B 1987, 198, 231–234. [Google Scholar] [CrossRef]
- Lee, H.J.; Park, B.Y.; Rho, M.; Vento, V. Sliding vacua in dense skyrmion matter. Nucl. Phys. A 2003, 726, 69–92. [Google Scholar] [CrossRef]
- Lee, H.K.; Park, B.Y.; Rho, M. Half-Skyrmions, Tensor Forces and Symmetry Energy in Cold Dense Matter. Phys. Rev. C 2011, 83, 025206, Erratum in Phys. Rev. C 2011, 84, 059902. [Google Scholar] [CrossRef]
- Holt, J.W.; Brown, G.E.; Kuo, T.T.S.; Holt, J.D.; Machleidt, R. Shell model description of the C-14 dating beta decay with Brown-Rho-scaled NN interactions. Phys. Rev. Lett. 2008, 100, 062501. [Google Scholar] [CrossRef]
- Paeng, W.G.; Kuo, T.T.S.; Lee, H.K.; Rho, M. Scale-Invariant Hidden Local Symmetry, Topology Change and Dense Baryonic Matter. Phys. Rev. C 2016, 93, 055203. [Google Scholar] [CrossRef]
- Paeng, W.G.; Kuo, T.T.S.; Lee, H.K.; Ma, Y.L.; Rho, M. Scale-invariant hidden local symmetry, topology change, and dense baryonic matter. II. Phys. Rev. D 2017, 96, 014031. [Google Scholar] [CrossRef]
- Ma, Y.L.; Lee, H.K.; Paeng, W.G.; Rho, M. Pseudoconformal equation of state in compact-star matter from topology change and hidden symmetries of QCD. Sci. China Phys. Mech. Astron. 2019, 62, 112011. [Google Scholar] [CrossRef]
- Hosaka, A.; Toki, H. Chiral bag model for the nucleon. Phys. Rep. 1996, 277, 65–188. [Google Scholar] [CrossRef]
- Goldstone, J.; Wilczek, F. Fractional Quantum Numbers on Solitons. Phys. Rev. Lett. 1981, 47, 986–989. [Google Scholar] [CrossRef]
- Jaroszewicz, T. On the Vacuum Baryon Number in the Chiral Bag Model. Phys. Lett. B 1984, 143, 217–221. [Google Scholar] [CrossRef]
- Rho, M. Skyrmions and Fractional Quantum Hall Droplets Unified by Hidden Symmetries in Dense Matter. arXiv 2021, arXiv:2109.10059. [Google Scholar]
- Karasik, A. Vector dominance, one flavored baryons, and QCD domain walls from the “hidden” Wess-Zumino term. SciPost Phys. 2021, 10, 138. [Google Scholar] [CrossRef]
- Karasik, A. Skyrmions, Quantum Hall Droplets, and one current to rule them all. SciPost Phys. 2020, 9, 8. [Google Scholar] [CrossRef]
- ’t Hooft, G. Symmetry Breaking Through Bell-Jackiw Anomalies. Phys. Rev. Lett. 1976, 37, 8–11. [Google Scholar] [CrossRef]
- ’t Hooft, G. How Instantons Solve the U(1) Problem. Phys. Rep. 1986, 142, 357–387. [Google Scholar] [CrossRef]
- Naculich, S.G.; Schnitzer, H.J. Level-rank duality of the U(N) WZW model, Chern-Simons theory, and 2-D qYM theory. J. High Energy Phys. 2007, 2007, 23. [Google Scholar] [CrossRef]
- Nakanishi, T.; Tsuchiya, A. Level rank duality of WZW models in conformal field theory. Commun. Math. Phys. 1992, 144, 351–372. [Google Scholar] [CrossRef]
- Aharony, O. Baryons, monopoles and dualities in Chern-Simons-matter theories. J. High Energy Phys. 2016, 2016, 93. [Google Scholar] [CrossRef]
- Naculich, S.G.; Riggs, H.A.; Schnitzer, H.J. Group Level Duality in WZW Models and Chern-Simons Theory. Phys. Lett. B 1990, 246, 417–422. [Google Scholar] [CrossRef]
- Camperi, M.; Levstein, F.; Zemba, G. The Large N Limit of Chern-Simons Gauge Theory. Phys. Lett. B 1990, 247, 549–554. [Google Scholar] [CrossRef]
- Zahed, I. Anomalous Baryon Number in (1+1)-Dimensions. Phys. Rev. D 1984, 30, 2221–2226. [Google Scholar] [CrossRef]
- Callan, C.G., Jr.; Harvey, J.A. Anomalies and Fermion Zero Modes on Strings and Domain Walls. Nucl. Phys. B 1985, 250, 427–436. [Google Scholar] [CrossRef]
- Jatkar, D.P.; Khare, A. Peculiar Charged Vortices in Higgs Models With Pure Chern-Simons Term. Phys. Lett. B 1990, 236, 283–286. [Google Scholar] [CrossRef]
- Wilczek, F. Magnetic Flux, Angular Momentum, and Statistics. Phys. Rev. Lett. 1982, 48, 1144–1146. [Google Scholar] [CrossRef]
- Rao, S. An Anyon primer. arXiv 1992, arXiv:hep-th/9209066. [Google Scholar]
- Weigel, H.; Schwesinger, B.; Holzwarth, G. Exotic Baryon Number B=2 States in the SU(2) Skyrme Model. Phys. Lett. B 1986, 168, 321–325. [Google Scholar] [CrossRef]
- Houghton, C.J.; Manton, N.S.; Sutcliffe, P.M. Rational maps, monopoles and Skyrmions. Nucl. Phys. B 1998, 510, 507–537. [Google Scholar] [CrossRef]
- Peskin, M.E. Mandelstam ’t Hooft Duality in Abelian Lattice Models. Ann. Phys. 1978, 113, 122. [Google Scholar] [CrossRef]
- Tong, D. Lectures on the Quantum Hall Effect. arXiv 2016, arXiv:1606.06687. [Google Scholar]
- Eto, M.; Nishimura, K.; Nitta, M. Domain-wall Skyrmion phase in a rapidly rotating QCD matter. J. High Energy Phys. 2024, 2024, 19. [Google Scholar] [CrossRef]
- Eto, M.; Nishimura, K.; Nitta, M. Non-Abelian chiral soliton lattice in rotating QCD matter: Nambu-Goldstone and excited modes. J. High Energy Phys. 2024, 2024, 35. [Google Scholar] [CrossRef]
- Kudryavtsev, A.E.; Piette, B.; Zakrzewski, W.J. Mesons, baryons and waves in the baby Skyrmion model. Eur. Phys. J. C 1998, 1, 333–341. [Google Scholar] [CrossRef]
- Battye, R.A.; Haberichter, M. Isospinning baby Skyrmion solutions. Phys. Rev. D 2013, 88, 125016. [Google Scholar] [CrossRef]
- Winyard, T. Skyrmion and Baby Skyrmion Formation from Domain Walls. arXiv 2015, arXiv:1507.07482. [Google Scholar]
- Leask, P. Baby skyrmion crystals stabilized by vector mesons. Phys. Lett. B 2024, 855, 138842. [Google Scholar] [CrossRef]
- Bigazzi, F.; Cotrone, A.L.; Olzi, A. Hall Droplet Sheets in Holographic QCD. J. High Energy Phys. 2023, 2023, 194. [Google Scholar] [CrossRef]
- Huang, X.G.; Nishimura, K.; Yamamoto, N. Anomalous effects of dense matter under rotation. J. High Energy Phys. 2018, 2018, 69. [Google Scholar] [CrossRef]
- Huang, X.G.; Nishimura, K.; Yamamoto, N. Anomaly-Induced Effects of Rotating Dense Matter. JPS Conf. Proc. 2019, 26, 031020. [Google Scholar] [CrossRef]
- Nishimura, K.; Yamamoto, N. Topological term, QCD anomaly, and the η′ chiral soliton lattice in rotating baryonic matter. J. High Energy Phys. 2020, 2020, 196. [Google Scholar] [CrossRef]
- Eto, M.; Nishimura, K.; Nitta, M. Phases of rotating baryonic matter: Non-Abelian chiral soliton lattices, antiferro-isospin chains, and ferri/ferromagnetic magnetization. J. High Energy Phys. 2022, 2022, 305. [Google Scholar] [CrossRef]
- Nitta, M. Correspondence between Skyrmions in 2+1 and 3+1 Dimensions. Phys. Rev. D 2013, 87, 025013. [Google Scholar] [CrossRef]
- Eto, M.; Nitta, M. Non-Abelian Sine-Gordon Solitons: Correspondence between SU(N) Skyrmions and CPN-1 Lumps. Phys. Rev. D 2015, 91, 085044. [Google Scholar] [CrossRef]
- Nitta, M. Relations among topological solitons. Phys. Rev. D 2022, 105, 105006. [Google Scholar] [CrossRef]
- Gudnason, S.B.; Nitta, M. Domain wall Skyrmions. Phys. Rev. D 2014, 89, 085022. [Google Scholar] [CrossRef]
- Eto, M.; Nishimura, K.; Nitta, M. How baryons appear in low-energy QCD: Domain-wall Skyrmion phase in strong magnetic fields. arXiv 2023, arXiv:2304.02940. [Google Scholar]
- Eto, M.; Nishimura, K.; Nitta, M. Phase diagram of QCD matter with magnetic field: Domain-wall Skyrmion chain in chiral soliton lattice. J. High Energy Phys. 2023, 2023, 32. [Google Scholar] [CrossRef]
- Qiu, Z.; Nitta, M. Baryonic Vortex Phase and Magnetic Field Generation in QCD with Isospin and Baryon Chemical Potentials. arXiv 2024, arXiv:2403.07433. [Google Scholar]
- Chen, S.; Fukushima, K.; Qiu, Z. Skyrmions in a magnetic field and π0 domain wall formation in dense nuclear matter. Phys. Rev. D 2022, 105, L011502. [Google Scholar] [CrossRef]
- Fukushima, K.; Imaki, S. Anomaly inflow on QCD axial domain-walls and vortices. Phys. Rev. D 2018, 97, 114003. [Google Scholar] [CrossRef]
- Dierigl, M.; Pritzel, A. Topological Model for Domain Walls in (Super-)Yang-Mills Theories. Phys. Rev. D 2014, 90, 105008. [Google Scholar] [CrossRef]
- Banks, T.; Seiberg, N. Symmetries and Strings in Field Theory and Gravity. Phys. Rev. D 2011, 83, 084019. [Google Scholar] [CrossRef]
- Gukov, S.; Kapustin, A. Topological Quantum Field Theory, Nonlocal Operators, and Gapped Phases of Gauge Theories. arXiv 2013, arXiv:1307.4793. [Google Scholar]
- Horowitz, G.T. Exactly Soluble Diffeomorphism Invariant Theories. Commun. Math. Phys. 1989, 125, 417. [Google Scholar] [CrossRef]
- Kitano, R.; Matsudo, R. Vector mesons on the wall. J. High Energy Phys. 2021, 2021, 23. [Google Scholar] [CrossRef]
- Witten, E. Dyons of Charge e theta/2 pi. Phys. Lett. B 1979, 86, 283–287. [Google Scholar] [CrossRef]
- Harada, M.; Yamawaki, K. Hidden local symmetry at one loop. Phys. Lett. B 1992, 297, 151–158. [Google Scholar] [CrossRef]
- Harada, M.; Yamawaki, K. Hidden local symmetry at loop: A New perspective of composite gauge boson and chiral phase transition. Phys. Rep. 2003, 381, 1–233. [Google Scholar] [CrossRef]
- Bando, M.; Kugo, T.; Uehara, S.; Yamawaki, K.; Yanagida, T. Is rho Meson a Dynamical Gauge Boson of Hidden Local Symmetry? Phys. Rev. Lett. 1985, 54, 1215. [Google Scholar] [CrossRef]
- Bando, M.; Kugo, T.; Yamawaki, K. Nonlinear Realization and Hidden Local Symmetries. Phys. Rep. 1988, 164, 217–314. [Google Scholar] [CrossRef]
- Goldstone, J.; Jaffe, R.L. The Baryon Number in Chiral Bag Models. Phys. Rev. Lett. 1983, 51, 1518. [Google Scholar] [CrossRef]
- Dreiner, H.K.; Ellis, J.R.; Flores, R.A. The Spin of the Proton in a Hybrid Chiral Bag Model. Phys. Lett. B 1989, 221, 167–172. [Google Scholar] [CrossRef]
- Crewther, R.J.; Tunstall, L.C. ΔI=1/2 rule for kaon decays derived from QCD infrared fixed point. Phys. Rev. D 2015, 91, 034016. [Google Scholar] [CrossRef]
- Li, Y.L.; Ma, Y.L.; Rho, M. Chiral-scale effective theory including a dilatonic meson. Phys. Rev. D 2017, 95, 114011. [Google Scholar] [CrossRef]
- Harada, M.; Yamawaki, K. Vector manifestation of the chiral symmetry. Phys. Rev. Lett. 2001, 86, 757–760. [Google Scholar] [CrossRef] [PubMed]
- Harada, M.; Yamawaki, K. Fate of vector dominance in the effective field theory. Phys. Rev. Lett. 2001, 87, 152001. [Google Scholar] [CrossRef]
- Georgi, H. Vector Realization of Chiral Symmetry. Nucl. Phys. B 1990, 331, 311–330. [Google Scholar] [CrossRef]
- Ma, Y.L.; Yang, W.C. Topology and Emergent Symmetries in Dense Compact Star Matter. Symmetry 2023, 15, 776. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, F.; Ma, Y.-L. Baryon Construction with η′ Meson Field. Symmetry 2025, 17, 477. https://doi.org/10.3390/sym17040477
Lin F, Ma Y-L. Baryon Construction with η′ Meson Field. Symmetry. 2025; 17(4):477. https://doi.org/10.3390/sym17040477
Chicago/Turabian StyleLin, Fan, and Yong-Liang Ma. 2025. "Baryon Construction with η′ Meson Field" Symmetry 17, no. 4: 477. https://doi.org/10.3390/sym17040477
APA StyleLin, F., & Ma, Y.-L. (2025). Baryon Construction with η′ Meson Field. Symmetry, 17(4), 477. https://doi.org/10.3390/sym17040477