Spectral Polynomials and Spectra of Graphs Beyond Cubic and Icosahedral Symmetries: n-Octahedra, n-Cubes, Symmetric and Semi-Symmetric Graphs, Giant Fullerene Cages and Generalized Petersen Graphs
Abstract
1. Introduction
2. Mathematical and Computational Methods Pertinent to Spectral Polynomials
3. Results and Discussions
- A.
- Spectral polynomials of small graphs with high automorphism groups: Heawood graph, Folkman’s graph, Möbius–Kantor, and Pappus, Desargues–Levi, Coxeter, Meringer, Coxeter–Tutte, and Dyck graphs.
- B.
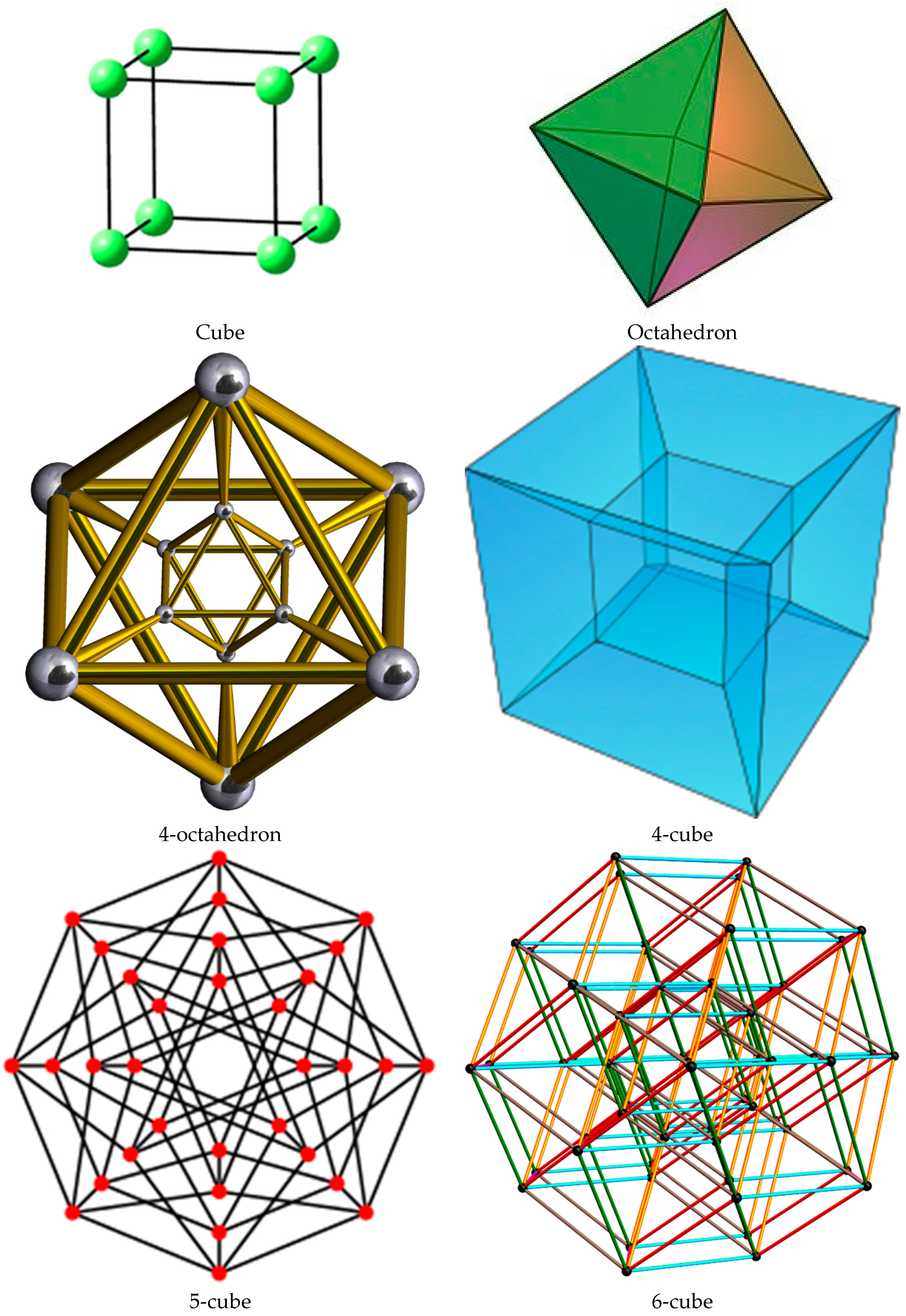
- Spectral Polynomials and Spectra of n-polyhedra: n-octahedra and n-cubes
- C.
- Spectral Polynomials of Supergiant Fullerenes and Generalized Petersen Graphs
4. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Read, R.C.; Corneil, D.G. The graph isomorphism disease. J. Graph. Theory 1977, 1, 339–363. [Google Scholar] [CrossRef]
- Harary, F. The determinant of the adjacency matrix of a graph. Siam. Rev. 1962, 4, 202–210. [Google Scholar] [CrossRef]
- Schwenk, A.J. Computing the characteristic polynomial of a graph. In Graphs and Combinatorics, Proceedings of the Capital Conference on Graph Theory and Combinatorics at the George Washington University, Washington, DC, USA, 18–22 June 1973; Springer: Berlin/Heidelberg, Germany, 2006; pp. 153–172. [Google Scholar]
- Balaban, A.T.; Harary, F. The Characteristic Polynomial Does Not Uniquely Determine the Topology of a Molecule. J. Chem. Doc. 1971, 11, 258–259. [Google Scholar] [CrossRef]
- Godsil, C.; McKay, B. Products of graphs and their spectra. In Combinatorial Mathematics IV, Proceedings of the Fourth Australian Conference Held at the University of Adelaide, Adelaide, SA, Australia, 27–29 August 1975; Springer: Berlin/Heidelberg, Germany, 2006; pp. 61–72. [Google Scholar]
- Randić, M. On the evaluation of the characteristic polynomial via symmetric function theory. J. Math. Chem. 1987, 1, 145–152. [Google Scholar] [CrossRef]
- Balasubramanian, K.; Randić, M. spectral Polynomials of Systems with General Interactions. Int. J. Quant. Chem. 1985, 28, 481–498. [Google Scholar] [CrossRef]
- Balasubramanian, K. Spectra of Chemical Trees. Int. J. Quant. Chem. 1982, 21, 581–590. [Google Scholar] [CrossRef]
- Balasubramanian, K. The Use of Frame’s Method for the Characteristic-Polynomials of Chemical Graphs. Theor. Chim. Acta 1984, 65, 49–58. [Google Scholar]
- Balasubramanian, K. Computer Generation of the Characteristic-Polynomials of Chemical Graphs. J. Comput. Chem. 1984, 5, 387–394. [Google Scholar] [CrossRef]
- Randić, M.; El-Basil, S.; King, R.B. On non-symmetry equivalence. Math. Comput. Model. 1988, 11, 641–646. [Google Scholar] [CrossRef]
- Balasubramanian, K. Topological peripheral shapes and distance-based characterization of fullerenes C20-C720: Existence of isoperipheral fullerenes. Polycycl. Aromat. Compd. 2022, 42, 1649–1667. [Google Scholar] [CrossRef]
- Balasubramanian, K. Matching Polynomial-Based Similarity Matrices and Descriptors for Isomers of Fullerenes. Inorganics 2023, 11, 335. [Google Scholar] [CrossRef]
- Balasubramanian, K. A Topological Analysis of the C60 Buckminsterfullerene and C70 Based on Distance Matrices. Chem. Phys. Lett. 1995, 239, 117–123. [Google Scholar] [CrossRef]
- Zhang, H.X.; Balasubramanian, K. Analytical Expressions for the Moments and Characteristic-Polynomials of Fullerenes Containing Isolated Pentagons. J. Phys. Chem. 1993, 97, 10341–10345. [Google Scholar] [CrossRef]
- Balasubramanian, K. New Insights into Aromaticity through Novel Delta Polynomials and Delta Aromatic Indices. Symmetry 2024, 16, 391. [Google Scholar] [CrossRef]
- Hosoya, H. Aromaticity Index Can Predict and Explain the Stability of Polycyclic Conjugated Hydrocarbons. Monatshefte Für Chem. 2005, 36, 1037–1054. [Google Scholar] [CrossRef]
- Hosoya, H.; Motoyama, A. An effective algorithm for obtaining polynomials for dimer statistics. Application of operator technique on the topological index to two-and three-dimensional rectangular and torus lattices. J. Math. Phys. 1985, 26, 157–167. [Google Scholar] [CrossRef]
- Hosoya, H. On some counting polynomials in chemistry. Discrete Appl. Math. 1988, 19, 239–257. [Google Scholar] [CrossRef]
- Cash, C.G. Relationship between the Hosoya polynomial and the hyper-Wiener index. Appl. Math. Lett. 2002, 15, 893–895. [Google Scholar] [CrossRef]
- Hosoya, H. Topological index. A newly proposed quantity characterizing the topological nature of structural isomers of saturated hydrocarbons. Bull. Chem. Soc. Jpn. 1971, 44, 2332–2339. [Google Scholar] [CrossRef]
- Hosoya, H.; Balasubramanian, K. Computational Algorithms for spectral Polynomials of Graphs from the Characteristic Polynomials of Edge-Weighted Graphs. J. Comput. Chem. 1989, 10, 698–710. [Google Scholar] [CrossRef]
- Hosoya, H.; Balasubramanian, K. Exact Dimer Statistics and Characteristic-Polynomials of Cacti Lattices. Theor. Chim. Acta 1989, 76, 315–329. [Google Scholar] [CrossRef]
- Babić, D.; Ori, O. Matching polynomial and topological resonance energy of C70. Chem. Phys. Lett. 1995, 234, 240–244. [Google Scholar] [CrossRef]
- Liu, J.; Shan, B.Z. The Computation of the Matching Polynomials of Fullerene Graphs. Croat. Chem. Acta 1997, 70, 971–977. [Google Scholar]
- Aihara, J.I.; Babić, D.; Gutman, I. Matching spectra of fullerenes. MATCH (Commun. Math. Comput. Chem.) 1996, 33, 7–16. [Google Scholar]
- Herndon, W.C.; Radhakrishnan, T.P.; Zivković, T.P. Characteristic and Matching polynomials of chemical graphs. Chem. Phys. Lett. 1988, 152, 233–238. [Google Scholar] [CrossRef]
- Liu, Y.; Ma, C.; Yao, H.; Wang, X. Computing the forcing and anti-forcing numbers of perfect Matchings for graphs by integer linear programmings. MATCH Commun. Math. Comput. Chem. 2022, 87, 561–575. [Google Scholar] [CrossRef]
- Salvador, J.M.; Hernandez, A.; Beltran, A.; Duran, R.; Mactutis, A. Fast partial-differential synthesis of the Matching polynomial of C72-100. J. Chem. Inf. Comput. Sci. 1988, 38, 1105–1110. [Google Scholar] [CrossRef]
- Kasteleyn, P.W. Graph Theory and Theoretical Physics; Harary, F., Ed.; Academic Press: London, UK, 1967. [Google Scholar]
- Temperley, H.N.V. Phase Transition and Critical Phenomena; Domb, C., Green, M.S., Eds.; Academic Press: London, UK, 1972; Volume I, p. 227. [Google Scholar]
- Kaatz, F.H.; Bultheel, A. Informational thermodynamic model for nanostructures. J. Math. Chem. 2014, 52, 1563–1575. [Google Scholar] [CrossRef]
- Balasubramanian, K. Matching Polynomials of Fullerene Clusters. Chem. Phys. Lett. 1993, 201, 306–314. [Google Scholar] [CrossRef]
- Balasubramanian, K. Density Functional and Graph Theory Computations of Vibrational, Electronic and Topological Properties of Porous Nanographenes. J. Phys. Org. Chem. 2022, 36, e4435. [Google Scholar] [CrossRef]
- Aihara, J. Graph theory of aromatic stabilization. Bull. Chem. Soc. Jpn. 2016, 89, 1425–1454. [Google Scholar] [CrossRef]
- Aihara, J. Circuit resonance energy: A key quantity that links energetic and magnetic criteria of aromaticity. J. Am. Chem. Soc. 2006, 128, 2873–2879. [Google Scholar] [CrossRef] [PubMed]
- Aihara, J. Topological resonance energy, bond resonance energy, and circuit resonance energy. J. Phys. Org. Chem. 1979, 21, 79–85. [Google Scholar] [CrossRef]
- Dias, J.R. Valence-bond determination of diradical character of polycyclic aromatic hydrocarbons: From acenes to rectangular benzenoids. J. Phys. Chem. A 2013, 117, 4716–4725. [Google Scholar] [CrossRef]
- Dias, J.R. The Formula Periodic Table for Benzenoid Hydrocarbons and the Unifying Theory of a Periodic Table Set. Polycycl. Aromat. Compd. 1994, 4, 87–106. [Google Scholar] [CrossRef]
- Aihara, J.; Makino, M.; Ishida, T.; Dias, J.R. Analytical study of superaromaticity in cycloarenes and related coronoid hydrocarbons. J. Phys. Chem. A 2013, 117, 4688–4697. [Google Scholar] [CrossRef]
- Randić, M. Aromaticity of polycyclic conjugated hydrocarbons. Chem. Rev. 2003, 103, 3449–3606. [Google Scholar] [CrossRef]
- Aihara, J. Aromaticity and superaromaticity in cyclopolyacenes. J. Chem. Soc. Perkin Trans. 1994, 2, 971–974. [Google Scholar] [CrossRef]
- Randić, M.; Balaban, A.T. Local aromaticity and aromatic sextet theory beyond Clar. Int. J. Quant. Chem. 2018, 118, e25657. [Google Scholar] [CrossRef]
- Kroto, H.W.; Heath, J.R.; O’Brien, S.C.; Curl, R.F.; Smalley, R.E. C60: Buckminsterfullerene. Nature 1985, 318, 162–163. [Google Scholar] [CrossRef]
- Kroto, H.W.; Heath, J.R.; O’Brien, S.C.; Curl, R.F.; Smalley, R. E, Long carbon chain molecules in circumstellar shells. Astrophys. J. 1987, 314, 352–355. [Google Scholar] [CrossRef]
- Iijima, S. Helical microtubules of graphitic carbon. Nature 1991, 354, 56–58. [Google Scholar] [CrossRef]
- Slanina, Z.; Lee, S.L.; Yuf, C.H. Computations in treating fullerenes and carbon aggregates. In Reviews in Computational Chemistry; John Wiley & Sons: Hoboken, NJ, USA, 2009; Volume 8. [Google Scholar]
- Ōsawa, E.; Ueno, H.; Yoshida, M.; Slanina, Z.; Zhao, X.; Nishiyama, M.; Saito, H. Combined topological and energy analysis of the annealing process in fullerene formation. Stone–Wales interconversion pathways among IPR isomers of higher fullerenes. J. Chem. Soc. Perkin Trans. 1998, 2, 943–950. [Google Scholar] [CrossRef]
- Balaban, A.T.; Liu, X.; Klein, D.J.; Babić, D.; Schmalz, T.G.; Seitz, W.A.; Randi’c, M. Graph invariants for fullerenes. J. Chem. Inf. Comput. Sci. 1995, 35, 396–404. [Google Scholar] [CrossRef]
- Bača, M.; Horváthová, J.; Mokrišová, M.; Suhányiová, A. On topological indices of fullerenes. Appl. Math. Comput. 2015, 251, 154–161. [Google Scholar] [CrossRef]
- Alizadeh, Y.; Iranmanesh, A.; Mirzaie, S. Computing Schultz polynomial, Schultz index of C60 fullerene by gap program. Dig. J. Nanomater. Biostructures 2009, 4, 7–10. [Google Scholar]
- Ghorbani, M. Connective eccentric index of fullerenes. J. Math. Nanosci. 2011, 1, 43–50. [Google Scholar]
- Balasubramanian, K. Symmetry, Combinatorics, Artificial Intelligence, Music and Spectroscopy. Symmetry 2021, 13, 1850. [Google Scholar] [CrossRef]
- Balasubramanian, K. Computational and Artificial Intelligence Techniques for Drug Discovery & Administration. In Comprehensive Pharmacology; Kenakin, T., Ed.; Elsevier: Amsterdam, The Netherlands, 2022; Volume 2, pp. 553–616. [Google Scholar] [CrossRef]
- Walker, P.D.; Maggiora, G.M.; Johnson, M.A.; Petke, J.D.; Mezey, P.G. Shape group analysis of molecular similarity: Shape similarity of six-membered aromatic ring systems. J. Chem. Inf. Comput. Sci. 1995, 35, 568–578. [Google Scholar] [CrossRef]
- Mezey, P.G. Fuzzy electron density fragments in macromolecular quantum chemistry, combinatorial quantum chemistry, functional group analysis, and shape–activity relations. Acc. Chem. Res. 2014, 47, 2821–2827. [Google Scholar] [CrossRef]
- Harary, F.; Schwenk, A.J. Which graphs have integral spectra. In Graphs and Combinatorics: Proceedings of the Capital Conference on Graph Theory and Combinatorics at the George Washington University, Washington, DC, USA, 18–22 June 1973; Springer: Berlin/Heidelberg, Germany, 1974; pp. 45–51. [Google Scholar]
- Cvetković, D.; Simić, S. Graph spectra in computer science. Linear Algebra Its Appl. 2011, 434, 1545–1562. [Google Scholar] [CrossRef]
- Balasubramanian, K. Enumeration of Internal-Rotation Reactions and Their Reaction Graphs. Theor. Chim. Acta 1979, 53, 129–146. [Google Scholar] [CrossRef]
- Mislow, K. Role of pseudorotation in the stereochemistry of nucleophilic displacement reactions. Acc. Chem. Res. 1970, 3, 321–331. [Google Scholar] [CrossRef]
- Zon, G.; Mislow, K. Studies in phosphorus stereochemistry. Chem. Organophosphorus Compd. I 2006, 61–94. [Google Scholar]
- Randić, M. Symmetry properties of graphs of interest in chemistry. II. Desargues–Levi graph. Int. J. Quantum Chem. 1979, 15, 663–682. [Google Scholar] [CrossRef]
- Balasubramanian, K. Relativity and the Jahn–Teller, Berry pseudorotations of TBP clusters: Group theory, spin–orbit and combinatorial nuclear spin statistics of TBP Desargues–Levi isomerization graph. J. Math. Chem. 2018, 56, 2194–2225. [Google Scholar] [CrossRef]
- Balasubramanian, K. Nonrigid group theory, tunneling splittings, and nuclear spin statistics of water pentamer:(H2O)5. J. Phys. Chem. A. 2004, 108, 5527–5536. [Google Scholar] [CrossRef]
- Harary, F. Graph Theory; Addison-Wesley: Reading, MA, USA, 1969. [Google Scholar]
- Lijnen, E.; Ceulemans, A. Oriented 2-Cell Embeddings of a Graph and Their Symmetry Classification: Generating Algorithms and Case Study of the Möbius-Kantor Graph. J. Chem. Inf. Model. 2004, 44, 1552–1564. [Google Scholar] [CrossRef]
- Herges, R. Topology in chemistry: Designing Möbius molecules. Chem. Rev. 2006, 106, 4820–4842. [Google Scholar] [CrossRef]
- Segawa, Y.; Watanabe, T.; Yamanoue, K.; Kuwayama, M.; Watanabe, K.; Pirillo, J.; Hijikata, Y.; Itami, K. Synthesis of a Möbius carbon nanobelt. Nat. Synth. 2022, 1, 535–541. [Google Scholar] [CrossRef]
- Balasubramanian, K. Relativistic double group spinor representations of nonrigid molecules. J. Chem. Phys. 2004, 120, 5524–5535. [Google Scholar] [CrossRef] [PubMed]
- Balasubramanian, K. Double group of the icosahedral group (Ih) and its application to fullerenes. Chem. Phys. Lett. 1996, 260, 476–484. [Google Scholar] [CrossRef]
- Balasubramanian, K. Relativistic Effects in Chemistry: Part A Theory & Techniques; John Wiley & Sons: New York, NK, USA, 1997; p. 301. [Google Scholar]
- Judd, B.R.; Lister, G.M.S. Complementary groups in the quark model of the atom. J. Phys. A Math. Gen. 1992, 25, 2615. [Google Scholar] [CrossRef]
- Balasubramanian, K. Enumeration of n-Dimensional Hypercubes, Icosahedra, Rubik’s Cube Dice, Colorings, Chirality, and Encryptions Based on Their Symmetries. Symmetry 2024, 16, 1020. [Google Scholar] [CrossRef]
- Balasubramanian, K. Topological Indices, Graph Spectra, Entropies, Laplacians, and Matching Polynomials of n-Dimensional Hypercubes. Symmetry 2023, 15, 557. [Google Scholar] [CrossRef]
- Coxeter, H.S.M. Self-dual configurations and regular graphs. Bull. Amer. Math. Soc. 1950, 56, 413–455. [Google Scholar] [CrossRef]
- Frucht, R.; Graver, J.E.; Watkins, M.E. The groups of the generalized Petersen graphs. In Math. Proc. Camb. Philos. Soc. 1971, 70, 211–218. [Google Scholar] [CrossRef]
- David Eppstein-Own work, Public Domain. Available online: https://commons.wikimedia.org/w/index.php?curid=2798751 (accessed on 24 December 2024).
- David Eppstein-Own work, Public Domain. Available online: https://en.wikipedia.org/wiki/Desargues_graph#/media/File:DesarguesGraph.svg (accessed on 24 December 2024).
- David Eppstein-Own work, Public Domain. Available online: https://commons.wikimedia.org/wiki/File:Folkman_Lombardi.svg (accessed on 24 December 2024).
- David Eppstein-Own work, Public Domain. Available online: https://commons.wikimedia.org/wiki/User:David_Eppstein/Gallery (accessed on 24 December 2024).
- Tesseract. The File is Licensed Under the Creative Commons Attribution-Share Alike 3.0 Unported License. Available online: https://en.wikipedia.org/wiki/File:Hypercube.svg (accessed on 24 December 2024).
- Ruen, T. Open Source, Copy-Right Free, Public Domain Image. Available online: https://commons.wikimedia.org/wiki/File:5-cube_t0.svg (accessed on 24 December 2024).
- Ahmadi, O.; Alon, N.; Blake, I.F.; Shparlinski, I.E. Graphs with integral spectrum. Linear Algebra Appl. 2009, 430, 547–552. [Google Scholar] [CrossRef]
- Ilić, A. Distance spectra and distance energy of integral circulant graphs. Linear Algebra Appl. 2010, 433, 1005–1014. [Google Scholar] [CrossRef]
- Beliaev, S.T. Energy-spectrum of a non-ideal Bose gas. In Basic Notions of Condensed Matter Physics; CRC Press: Boca Raton, FL, USA, 1997; pp. 432–440. [Google Scholar]
- Balasubramanian, K. Orthogonal polynomials through complex matrix graph theory. J. Math. Chem. 2023, 61, 144–165. [Google Scholar] [CrossRef]
- Parisot, S.; Ktena, S.I.; Ferrante, E.; Lee, M.; Guerrero, R.; Glocker, B.; Rueckert, D. Disease prediction using graph convolutional networks: Application to autism spectrum disorder and Alzheimer’s disease. Med. Image Anal. 2018, 48, 117–130. [Google Scholar] [CrossRef] [PubMed]
- Ktena, S.I.; Parisot, S.; Ferrante, E.; Rajchl, M.; Lee, M.; Glocker, B.; Rueckert, D. Metric learning with spectral graph convolutions on brain connectivity networks. NeuroImage 2018, 169, 431–442. [Google Scholar] [CrossRef] [PubMed]
- Alshammari, M.; Stavrakakis, J.; Takatsuka, M. Refining a k-nearest neighbor graph for a computationally efficient spectral clustering. Pattern Recognit. 2021, 114, 107869. [Google Scholar] [CrossRef]
- Balasubramanian, K. Characteristic polynomials, spectral-based Riemann-Zeta functions and entropy indices of n-dimensional hypercubes. J. Math. Chem. 2023, 61, 1570–1591. [Google Scholar] [CrossRef]
- Balasubramanian, K.; Basak, S.C. Characterization of isospectral graphs using graph invariants and derived orthogonal parameters. J. Chem. Inf. Comput. Sci. 1998, 38, 367–373. [Google Scholar] [CrossRef]
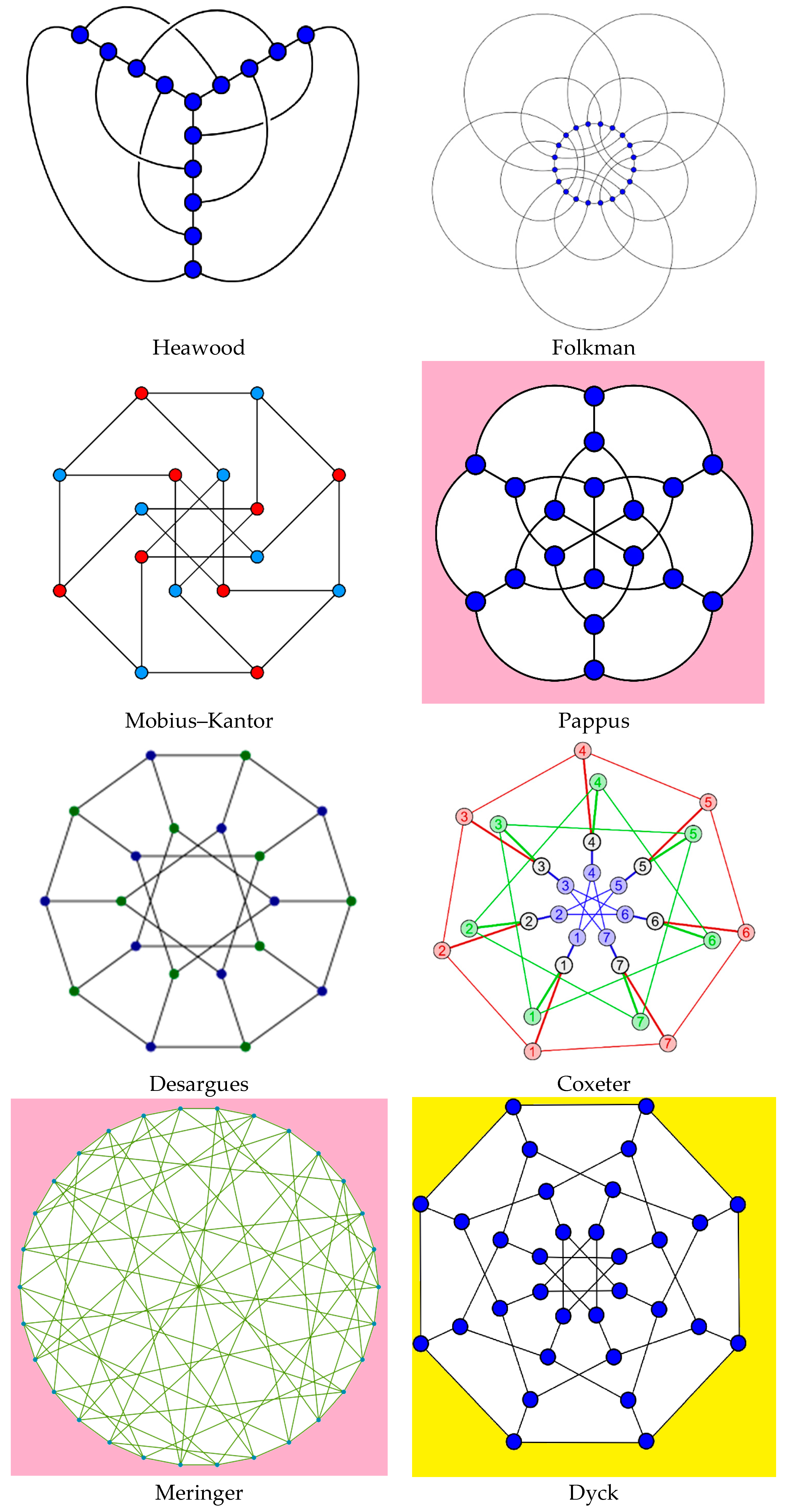


| Heawood | Mobius– Kantor | Pappus | Desargues | Coxeter | Meringer | Dyck | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| k | C14−k | k | C16−k | k | C18−k | k | C20−k | k | C28−k | k | C30−k | k | C32−k |
| 14 | 1 | 16 | 1 | 18 | 1 | 20 | 1 | 28 | 1 | 30 | 1 | 32 | 1 |
| 13 | 0 | 15 | 0 | 17 | 0 | 19 | 0 | 27 | 0 | 29 | 0 | 31 | 0 |
| 12 | −21 | 14 | −24 | 16 | −27 | 18 | −30 | 26 | −42 | 28 | −75 | 30 | −48 |
| 11 | 0 | 13 | −0 | 15 | 0 | 17 | 0 | 25 | −0 | 27 | 0 | 29 | 0 |
| 10 | 168 | 12 | 228 | 14 | 297 | 16 | 375 | 24 | 777 | 26 | 2475 | 28 | 1032 |
| 9 | 0 | 11 | −0 | 13 | 0 | 15 | 0 | 23 | −0 | 25 | −384 | 27 | 0 |
| 8 | −700 | 10 | −1144 | 12 | −1755 | 14 | −2580 | 22 | −8344 | 24 | −48,401 | 26 | −13,168 |
| 7 | 0 | 9 | −0 | 11 | 0 | 13 | 0 | 21 | −48 | 23 | 19,104 | 25 | 0 |
| 6 | 1680 | 8 | 3342 | 10 | 6075 | 12 | 10,815 | 20 | 57,666 | 22 | 628,056 | 24 | 111,372 |
| 5 | 0 | 7 | −0 | 9 | 0 | 11 | 0 | 19 | 1232 | 21 | −430,848 | 23 | 0 |
| 4 | −2352 | 6 | −5832 | 8 | −12,393 | 10 | −28,830 | 18 | −268,716 | 20 | −5,679,240 | 22 | −660,720 |
| 3 | 0 | 5 | −0 | 7 | 0 | 9 | 0 | 17 | −13,104 | 19 | 5,785,632 | 21 | 0 |
| 2 | 1792 | 4 | 5940 | 6 | 13,851 | 8 | 49,545 | 16 | 860,314 | 18 | 36,262,896 | 20 | 2,839,768 |
| 1 | 0 | 3 | −0 | 5 | 0 | 7 | 0 | 15 | 74,256 | 17 | −51,046,080 | 19 | 0 |
| 0 | −576 | 2 | −3240 | 4 | −6561 | 6 | −54,480 | 14 | −1,893,960 | 16 | −160,269,840 | 18 | −9,014,832 |
| Folkman | 1 | −0 | 3 | 0 | 5 | 0 | 13 | −239,568 | 15 | 307,544,960 | 17 | 0 | |
| 20 | 1 | 0 | 729 | 2 | 0 | 4 | 36,960 | 12 | 2,827,965 | 14 | 452,708,352 | 16 | 21,377,718 |
| 19 | 0 | Cox–Tutte | 1 | 0 | 3 | 0 | 11 | 433,776 | 13 | −1,269,818,112 | 15 | 0 | |
| 18 | −40 | 30 | 1 | 0 | 0 | 2 | −14,080 | 10 | −2,790,970 | 12 | −584,794,368 | 14 | −38,077,072 |
| 17 | 0 | 29 | 0 | 1 | 0 | 9 | −396,816 | 11 | 3,482,735,616 | 13 | 0 | ||
| 16 | 600 | 28 | −45 | 0 | 2304 | 8 | 1,772,925 | 10 | −840,302,592 | 12 | 50,932,344 | ||
| 15 | 0 | 27 | 0 | 7 | 118,192 | 9 | −5,777,448,960 | 11 | 0 | ||||
| 14 | −4320 | 26 | 900 | 6 | −719,376 | 8 | 4,925,411,328 | 10 | −50,813,520 | ||||
| 13 | 0 | 25 | 0 | 5 | 44,352 | 7 | 4,173,987,840 | 9 | 0 | ||||
| 12 | 15,120 | 24 | −10,560 | 4 | 170,464 | 6 | −793,758,9248 | 8 | 37,211,500 | ||||
| 11 | 0 | 23 | 0 | 3 | −37,632 | 5 | 1,877,016,576 | 7 | 0 | ||||
| 10 | −20,736 | 22 | 80,640 | 2 | −16,128 | 4 | 3,963,420,672 | 6 | −19,410,000 | ||||
| 9 | 0 | 21 | 0 | 1 | 7168 | 3 | −3,784,048,640 | 5 | 0 | ||||
| 8 | 0 | 20 | −419,328 | 0 | −768 | 2 | 1,374,683,136 | 4 | 6,825,000 | ||||
| 7 | 0 | 19 | 0 | 1 | −188,743,680 | 3 | 0 | ||||||
| 6 | 0 | 18 | 1,505,280 | 0 | 0 | 2 | −1,450,000 | ||||||
| 5 | 0 | 17 | 0 | 1 | 0 | ||||||||
| 4 | 0 | 16 | −3,686,400 | 0 | 140,625 | ||||||||
| 15 | 0 | ||||||||||||
| 14 | 5,898,240 | ||||||||||||
| 13 | 0 | ||||||||||||
| 12 | −5,570,560 | ||||||||||||
| 11 | 0 | ||||||||||||
| 10 | 2,359,296 | ||||||||||||
| Spectra of Heawood graph | |||||||||||||
| −3.0 | −1.414214 | −1.414214 | −1.414214 | −1.414214 | −1.414214 | ||||||||
| −1.414214 | 1.414214 | 1.414214 | 1.414214 | 1.414214 | 1.414214 | ||||||||
| 1.414214 | 3.0 | ||||||||||||
| Spectra of Folkman’s graph | |||||||||||||
| −4.0 | −2.449490 | −2.449490 | −2.449490 | −2.449490 | 0.0 | ||||||||
| 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | ||||||||
| 0.0 | 0.0 | 0.0 | 2.449490 | 2.449490 | 2.449490 | ||||||||
| 2.449490 | 4.0 | ||||||||||||
| Spectra of Mobius–Kantor graph | |||||||||||||
| −3.0 | −1.732051 | −1.732051 | −1.732051 | −1.732051 | −1.0 | ||||||||
| −1.0 | −1.0 | 1.0 | 1.0 | 1.0 | 1.732051 | ||||||||
| 1.732051 | 1.732051 | 1.732051 | 3.0 | ||||||||||
| Spectra of Coxeter–Tutte graph | |||||||||||||
| −3.0 | −2.0 | −2.0 | −2.0 | −2.0 | −2.0 | ||||||||
| −2.0 | −2.0 | −2.0 | −2.0 | −0.0 | −0.0 | ||||||||
| −0.0 | −0.0 | −0.0 | −0.0 | 0.0 | 0.0 | ||||||||
| 0.0 | 0.0 | 2.0 | 2.0 | 2.0 | 2.0 | ||||||||
| 2.0 | 2.0 | 2.0 | 2.0 | 2.0 | 3.0 | ||||||||
| Spectra of Pappus graph | |||||||||||||
| −3.0 | −1.732051 | −1.732051 | −1.732051 | −1.732051 | −1.732051 | ||||||||
| −1.732051 | −0.0 | −0.0 | 0.0 | 0.0 | 1.732051 | ||||||||
| 1.732051 | 1.732051 | 1.732051 | 1.732051 | 1.732051 | 3.0 | ||||||||
| Spectra of Desargues–Levi graph | |||||||||||||
| −3.0 | −2.0 | −2.0 | −2.0 | −2.0 | −1.0 | ||||||||
| −1.0 | −1.0 | −1.0 | −1.0 | 1.0 | 1.0 | ||||||||
| 1.0 | 1.0 | 1.0 | 2.0 | 2.0 | 2.0 | ||||||||
| 2.0 | 3.0 | ||||||||||||
| Spectra of Coxeter graph | |||||||||||||
| −2.414214 | −2.414214 | −2.414214 | −2.414214 | −2.414214 | −2.414214 | ||||||||
| −1.0 | −1.0 | −1.0 | −1.0 | −1.0 | −1.0 | ||||||||
| −1.0 | 0.414214 | 0.414214 | 0.414214 | 0.414214 | 0.414214 | ||||||||
| 0.414214 | 2.0 | 2.0 | 2.0 | 2.0 | 2.0 | ||||||||
| 2.0 | 2.0 | 2.0 | 3.0 | ||||||||||
| Spectra of Meringer graph | |||||||||||||
| −3.0 | −3.0 | −2.732051 | −2.732051 | −2.732051 | −2.732051 | ||||||||
| −2.561553 | −2.561553 | −2.561553 | −2.0 | −2.0 | −2.0 | ||||||||
| 0.0 | 0.732051 | 0.732051 | 0.732051 | 0.732051 | 1.561553 | ||||||||
| 1.561553 | 1.561553 | 2.0 | 2.0 | 2.0 | 2.0 | ||||||||
| 2.0 | 2.0 | 2.0 | 2.0 | 2.0 | 5.0 | ||||||||
| Spectra of Dyck graph | |||||||||||||
| −3.0 | −2.236068 | −2.236068 | −2.236068 | −2.236068 | −2.236068 | ||||||||
| −2.236068 | −1.0 | −1.0 | −1.0 | −1.0 | −1.0 | ||||||||
| −1.0 | −1.0 | −1.0 | −1.0 | 1.0 | 1.0 | ||||||||
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | ||||||||
| 1.0 | 2.236068 | 2.236068 | 2.236068 | 2.236068 | 2.236068 | ||||||||
| 2.236068 | 3.0 | ||||||||||||
| 4-Octa | 5-Octa | 6-Octa | 4-Cube | 5-Cube | 6-Cube | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| k | C12-k | k | Cn-k | k | Cn-k | k | C16-k | k | C32-k | k | C64-k |
| 12 | 1 | 24 | 1 | 48 | 1 | 16 | 1 | 32 | 1 | 64 | 1 |
| 11 | −0 | 23 | 0 | 47 | 0 | 15 | 0 | 31 | 0 | 63 | 0 |
| 10 | −30 | 22 | −72 | 46 | −168 | 14 | −32 | 30 | −80 | 62 | −192 |
| 9 | −32 | 21 | −64 | 45 | −128 | 13 | 0 | 29 | 0 | 61 | 0 |
| 8 | 231 | 20 | 1968 | 44 | 12,420 | 12 | 352 | 28 | 2680 | 60 | 16,896 |
| 7 | 384 | 19 | 3072 | 43 | 17,664 | 11 | 0 | 27 | 0 | 59 | 0 |
| 6 | −388 | 18 | −25,856 | 42 | −532,280 | 10 | −1792 | 26 | −50,160 | 58 | −908,800 |
| 5 | −960 | 17 | −55,296 | 41 | −1,072,512 | 9 | 0 | 25 | 0 | 57 | 0 |
| 4 | 63 | 16 | 169,728 | 40 | 14,672,178 | 8 | 4352 | 24 | 586,140 | 56 | 33,592,320 |
| 3 | 896 | 15 | 475,136 | 39 | 37,926,400 | 7 | 0 | 23 | 0 | 55 | 0 |
| 2 | 258 | 14 | −509,952 | 38 | −272,681,208 | 6 | −4096 | 22 | −4,516,176 | 54 | −909,139,968 |
| 1 | −288 | 13 | −2,113,536 | 37 | −872,304,000 | 5 | 0 | 21 | 0 | 53 | 0 |
| 0 | −135 | 12 | 249,856 | 36 | 3,470,385,364 | 4 | 0 | 20 | 23,674,440 | 52 | 18,737,135,616 |
| 11 | 4,718,592 | 35 | 13,809,256,704 | 3 | 0 | 19 | 0 | 51 | 0 | ||
| 10 | 1,966,080 | 34 | −29,739,824,040 | 2 | 0 | 18 | −86,417,520 | 50 | −301,908,492,288 | ||
| 9 | −4,194,304 | 33 | −155,328,255,104 | 1 | 0 | 17 | 0 | 49 | 0 | ||
| 8 | −3,145,728 | 32 | 157,058,583,615 | 0 | 0 | 16 | 224,447,430 | 48 | 3,873,651,425,280 | ||
| 7 | 0 | 31 | 1,263,649,277,952 | 15 | 0 | 47 | 0 | ||||
| 6 | 0 | 30 | −301,066,597,648 | 14 | −421,986,160 | 46 | −40,094,935,285,760 | ||||
| 5 | 0 | 29 | −7,502,268,038,400 | 13 | 0 | 45 | 0 | ||||
| 4 | 0 | 28 | −2,440,891,321,464 | 12 | 580,113,224 | 44 | 337,843,889,111,040 | ||||
| 3 | 0 | 27 | 32,604,315,138,560 | 11 | 0 | 43 | 0 | ||||
| 2 | 0 | 26 | 25,318,817,961,552 | 10 | −583,700,560 | 42 | −2,331,017,163,571,200 | ||||
| 1 | 0 | 25 | −103,524,258,893,568 | 9 | 0 | 41 | 0 | ||||
| 0 | 0 | 24 | −123,311,080,690,180 | 8 | 425,462,940 | 40 | 13,209,766,838,927,360 | ||||
| 23 | 237,736,933,180,416 | 7 | 0 | 39 | 0 | ||||||
| 22 | 394,216,798,254,480 | 6 | −218,887,920 | 38 | −61,503,043,157,360,640 | ||||||
| 21 | −382,142,821,520,128 | 5 | 0 | 37 | 0 | ||||||
| 20 | −900,143,474,860,728 | 4 | 75,436,920 | 36 | 234,656,483,109,765,120 | ||||||
| 19 | 384,459,573,742,080 | 3 | 0 | 35 | 0 | ||||||
| 18 | 1,518,867,106,899,248 | 2 | −15,641,424 | 34 | −72,938,303,710,769,9712 | ||||||
| 17 | −111,013,896,556,800 | 1 | 0 | 33 | 0 | ||||||
| 16 | −1,919,452,896,925,809 | 0 | 1,476,225 | 32 | 1,828,695,408,465,936,384 | ||||||
| 15 | −355,750,689,224,704 | 31 | 0 | ||||||||
| 14 | 1,815,718,389,293,880 | 30 | −3,641,408,101,162,156,032 | ||||||||
| 13 | 719,896,206,628,224 | 29 | 0 | ||||||||
| 12 | −1,266,265,410,678,540 | 28 | 5,624,722,156,190,433,280 | ||||||||
| 11 | −753,880,362,630,912 | 27 | 0 | ||||||||
| 10 | 626,650,172,863,848 | 26 | −6,496,794,306,202,828,800 | ||||||||
| 9 | 517,774,476,424,320 | 25 | 0 | ||||||||
| 8 | −200,757,797,644,302 | 24 | 5,280,189,088,115,195,904 | ||||||||
| 7 | −241,587,920,079,360 | 23 | 0 | ||||||||
| 6 | 30,076,757,583,336 | 22 | −2,693,152,577,167,556,608 | ||||||||
| 5 | 74,358,227,028,096 | 21 | 0 | ||||||||
| 4 | 3,814,694,126,820 | 20 | 648,518,346,341,351,424 | ||||||||
| 3 | −13,696,722,604,800 | ||||||||||
| 2 | −2,434,531,221,000 | ||||||||||
| 1 | 1,147,912,560,000 | ||||||||||
| 0 | 313,882,340,625 | ||||||||||
| Spectra of 4-Octahedron | |||||||||||
| −3.0 | −3.0 | −1.0 | −1.0 | −1.0 | −1.0 | ||||||
| −1.0 | 1.0 | 1.0 | 1.0 | 3.0 | 5.0 | ||||||
| Spectra of 5-Octahedron | |||||||||||
| −4.0 | −4.0 | −2.0 | −2.0 | −2.0 | −2.0 | ||||||
| −2.0 | −2.0 | −2.0 | 0.0 | 0.0 | 0.0 | ||||||
| 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 2.0 | ||||||
| 2.0 | 2.0 | 2.0 | 4.0 | 4.0 | 6.0 | ||||||
| Spectra of 6-Octahedron | |||||||||||
| −5.0 | −5.0 | −3.0 | −3.0 | −3.0 | −3.0 | ||||||
| −3.0 | −3.0 | −3.0 | −3.0 | −3.0 | −1.0 | ||||||
| −1.0 | −1.0 | −1.0 | −1.0 | −1.0 | −1.0 | ||||||
| −1.0 | −1.0 | −1.0 | −1.0 | −1.0 | −1.0 | ||||||
| −1.0 | −1.0 | 1.0 | 1.0 | 1.0 | 1.0 | ||||||
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | ||||||
| 1.0 | 1.0 | 3.0 | 3.0 | 3.0 | 3.0 | ||||||
| 3.0 | 3.0 | 5.0 | 5.0 | 5.0 | 7.0 | ||||||
| Spectra of 4-Cube | |||||||||||
| −4.0 | −2.0 | −2.0 | −2.0 | −2.0 | −0.0 | ||||||
| 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 2.0 | ||||||
| 2.0 | 2.0 | 2.0 | 4.0 | ||||||||
| Spectra of 5-Cube | |||||||||||
| −5.0 | −3.0 | −3.0 | −3.0 | −3.0 | −3.0 | ||||||
| −1.0 | −1.0 | −1.0 | −1.0 | −1.0 | −1.0 | ||||||
| −1.0 | −1.0 | −1.0 | −1.0 | 1.0 | 1.0 | ||||||
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | ||||||
| 1.0 | 1.0 | 3.0 | 3.0 | 3.0 | 3.0 | ||||||
| 3.0 | 5.0 | ||||||||||
| Spectra of 6-Cube | |||||||||||
| −6.0 | −4.0 | −4.0 | −4.0 | −4.0 | −4.0 | ||||||
| −4.0 | −2.0 | −2.0 | −2.0 | −2.0 | −2.0 | ||||||
| −2.0 | −2.0 | −2.0 | −2.0 | −2.0 | −2.0 | ||||||
| −2.0 | −2.0 | −2.0 | −2.0 | −0.0 | −0.0 | ||||||
| −0.0 | −0.0 | −0.0 | −0.0 | −0.0 | −0.0 | ||||||
| −0.0 | −0.0 | 0.0 | 0.0 | 0.0 | 0.0 | ||||||
| 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | ||||||
| 2.0 | 2.0 | 2.0 | 2.0 | 2.0 | 2.0 | ||||||
| 2.0 | 2.0 | 2.0 | 2.0 | 2.0 | 2.0 | ||||||
| 2.0 | 2.0 | 2.0 | 4.0 | 4.0 | 4.0 | ||||||
| 4.0 | 4.0 | 4.0 | 6.0 | ||||||||
| Supergiant Fullerene C240: Ih Symmetry | Fullerene C80: Ih Symmetry | ||||
|---|---|---|---|---|---|
| k | C240-k | k | C80-k | ||
| 240 | 1 | 80 | 1 | ||
| 239 | 0 | 79 | −0 | ||
| 238 | −360 | 78 | −120 | ||
| 237 | 0 | 77 | −0 | ||
| 236 | 63,900 | 76 | 6,900 | ||
| 235 | −24 | 75 | −24 | ||
| 234 | −7,455,700 | 74 | −253,220 | ||
| 233 | 8,400 | 73 | 2,640 | ||
| 232 | 643,238,070 | 72 | 6,665,250 | ||
| 231 | −1,449,240 | 71 | −138,840 | ||
| 230 | −43,765,604,316 | 70 | −134,074,668 | ||
| 229 | 164,318,400 | 69 | 4,649,280 | ||
| 228 | 2445,983,522,150 | 68 | 2,144,400,610 | ||
| 227 | −13,772,791,440 | 67 | −111,390,840 | ||
| 226 | −115,483,543,870,380 | 66 | −28,014,828,600 | ||
| 225 | 910,181,939,872 | 65 | 2,034,173,640 | ||
| 224 | 4,701,527,059,529,265 | 64 | 304,771,472,295 | ||
| 223 | −49,395,215,227,920 | 63 | −29,454,679,080 | ||
| 222 | −167,648,707,691,087,420 | 62 | −2,800,871,913,120 | ||
| 221 | 2,264,002,673,490,240 | 61 | 347,355,807,000 | ||
| 220 | 5,300,892,235,419,864,426 | 60 | 21,982,913,536,506 | ||
| 219 | −89,455,905,039,333,480 | 59 | −3,400,629,844,080 | ||
| 218 | −150,106,442,753,314,932,360 | 58 | −148,597,115,610,060 | ||
| 217 | 3,095,063,861,360,503,920 | 57 | 28,032,598,630,800 | ||
| 216 | 3,837,990,865,403,514,684,635 | 56 | 870,782,603,143,245 | ||
| 215 | −94,929,696,941,052,350,832 | 55 | −196,675,986,598,104 | ||
| 214 | −89,214,815,606,936,913,307,740 | 54 | −4,446,095,763,848,880 | ||
| 213 | 2,606,866,654,781,819,504,840 | 53 | 1,184,149,280,392,400 | ||
| 212 | 1,896,368,878,405,224,684,770,400 | 52 | 19,855,655,662,646,790 | ||
| 211 | −64,620,553,360,631,460,336,960 | 51 | −6,157,335,886,362,960 | ||
| 210 | −37,044,948,371,954,529,056,063,656 | 50 | −77,775,412,124,784,276 | ||
| 209 | 1,455,895,045,330,922,474,674,320 | 49 | 27,786,279,810,761,760 | ||
| 208 | 667,936,566,937,916,468,417,641,050 | 48 | 267,713,181,302,978,565 | ||
| 207 | −29,986,069,149,330,479,067,914,440 | 47 | −109,222,881,940,156,720 | ||
| 206 | −11,157,980,465,768,328,477,435,561,180 | 46 | −810,610,645,577,880,600 | ||
| 205 | 567,420,196,254,073,164,997,202,232 | 45 | 374,966,869,205,421,336 | ||
| 204 | 173,272,188,234,359,680,778,390,285,010 | 44 | 2,159,504,514,172,334,900 | ||
| 203 | −9,907,490,766,609,936,176,882,434,560 | 43 | −1,126,235,220,601,566,120 | ||
| 202 | −2,508,706,834,146,599,915,120,612,739,600 | 42 | −5,058,329,868,205,795,680 | ||
| 201 | 160,228,001,555,018,310,378,540,341,040 | 41 | 2,962,294,863,144,099,920 | ||
| 200 | 33,954,306,934,029,341,067,084,013,161,996 | 40 | 10,401,040,837,191,228,618 | ||
| 199 | −2,408,107,366,425,017,858,165,622,286,560 | 39 | −6,824,327,055,789,596,280 | ||
| 198 | −430,617,032,155,529,976,402,418,565,888,418 | 38 | −18,723,827,983,032,022,240 | ||
| 197 | 33,733,309,861,467,028,170,339,491,094,360 | 37 | 13,761,319,627,538,589,720 | ||
| 196 | 5,128,190,205,096,393,871,427,396,075,601,794 | 36 | 29,389,984,702,098,348,120 | ||
| 195 | −441,602,915,472,953,441,053,236,800,435,968 | 35 | −24,256,112,112,485,663,584 | ||
| 194 | −57,457,939,438,911,746,894,371,388,726,145,160 | 34 | −39,987,774,847,141,119,240 | ||
| 193 | 5,415,272,424,948,212,577,371,671,947,088,195 | 33 | 37,287,514,249,778,822,040 | ||
| 192 | 606,747,145,859,062,755,972,596,777,547,121,216 | 32 | 46,754,900,712,550,670,195 | ||
| 191 | −62,337,209,301,203,951,387,803,680,398,611,184 | 31 | −49,829,511,883,061,754,120 | ||
| 190 | −6.048221186670528995008469688407721 × 1036 | 30 | −46,365,386,945,168,044,056 | ||
| 189 | 6.74912600115078522823923911813332 × 1035 | 29 | 57,635,855,484,766,164,320 | ||
| 188 | 5.699540039759760361514507167613912 × 1037 | 28 | 38,163,065,106,847,280,460 | ||
| 187 | −6.884583524982830022301898752320899 × 1036 | 27 | −57,367,377,814,733,742,720 | ||
| 186 | −5841734623839887376439698725423304 × 1038 | 26 | −25,028,715,850,369,172,460 | ||
| 185 | 6.627142032028232385508691182230046 × 1037 | 25 | 48,761,446,947,388,280,472 | ||
| 184 | 4.2983064355992869496309485626364736 × 1039 | 24 | 11,836,058,202,682,257,720 | ||
| 183 | −628668554149521028367699179934285 × 1038 | 23 | −35,032,832,823,083,608,120 | ||
| 182 | −3.44790786602597840263375583644471 × 1040 | 22 | −2,546,201,709,135,643,740 | ||
| 181 | 5.1896410873880857187239934664719776 × 1039 | 21 | 20,976,283,634,720,851,080 | ||
| 180 | 2.626884237337310961834946860618086 × 1041 | 20 | −1,729,379,075,500,688,166 | ||
| 179 | −4.232531280244278624723071801119075 × 1040 | 19 | −10,255,337,938,895,367,360 | ||
| 178 | −1.9026703048880961127625771343127042 × 1042 | 18 | 2,388,847,093,968,947,640 | ||
| 177 | 3.274107113301917807776400399677189 × 1041 | 17 | 3,963,782,111,401,409,320 | ||
| 176 | 1.3112983988222523472609017774452832 × 1043 | 16 | −1,568,049,183,041,116,425 | ||
| 175 | −2.4046912924293780571314609164157965 × 1042 | 15 | −1,141,429,955,133,602,688 | ||
| 174 | −8.606042251846876681048515170294774 × 1043 | 14 | 696,942,492,398,230,080 | ||
| 173 | 1.6784484961723877612599983253829556 × 1043 | 13 | 211,387,203,870,296,880 | ||
| 172 | 5.3825845751316303370481341944343536 × 1044 | 12 | −219,949,319,166,357,690 | ||
| 171 | −1.114333623087190923789821800463615 × 1044 | 11 | −9,893,398,173,518,960 | ||
| 170 | −3.2104081045968696155099365191591615 × 1045 | 10 | 47,809,319,577,549,300 | ||
| … | … | 9 | −7,196,177,291,756,040 | ||
| … | … | 8 | −6,355,394,133,712,305 | ||
| 0 | 4.7272376508569497895171096411125664 × 1030 | 7 | 2,265,666,348,699,840 | ||
| 6 | 307,052,294,351,520 | ||||
| 5 | −293,140,993,064,048 | ||||
| 4 | 36,331,138,153,170 | ||||
| 3 | 11,793,686,090,760 | ||||
| 2 | −4,491,814,487,580 | ||||
| 1 | 581,319,267,480 | ||||
| 0 | −28,012,848,759 | ||||
| Spectra of Supergiant Golden Fullerene C240 (Ih) | |||||
| −2.883504 | −2.883504 | −2.883504 | −2.867285 | −2.867285 | −2.867285 |
| −2.867285 | −2.703937 | −2.703937 | −2.703937 | −2.703937 | −2.592337 |
| −2.592337 | −2.592337 | −2.592337 | −2.592337 | −2.444550 | −2.444550 |
| −2.444550 | −2.420027 | −2.420027 | −2.420027 | −2.420027 | −2.420027 |
| −2.306530 | −2.306530 | −2.306530 | −2.149180 | −2.149180 | −2.149180 |
| −2.149180 | −2.149180 | −2.067984 | −2.067984 | −2.067984 | −2.067984 |
| −2.025797 | −2.025797 | −2.025797 | −2.000000 | −1.810855 | −1.810855 |
| −1.810855 | −1.810855 | −1.784087 | −1.784087 | −1.784087 | −1.784087 |
| −1.784087 | −1.740291 | −1.740291 | −1.740291 | −1.687162 | −1.687162 |
| −1.687162 | −1.621105 | −1.621105 | −1.621105 | −1.433222 | −1.433222 |
| −1.433222 | −1.433222 | −1.373319 | −1.373319 | −1.373319 | −1.373319 |
| −1.373319 | −1.356691 | −1.356691 | −1.356691 | −1.356691 | −1.356691 |
| −1.257513 | −1.257513 | −1.257513 | −1.257513 | −1.207321 | −1.207321 |
| −1.207321 | −1.207321 | −1.207321 | −1.062685 | −1.062685 | −1.062685 |
| −1.041813 | −1.041813 | −1.041813 | −1.000000 | −1.000000 | −1.000000 |
| −1.000000 | −1.000000 | −1.000000 | −1.000000 | −1.000000 | −1.000000 |
| −1.000000 | −0.924454 | −0.924454 | −0.924454 | −0.812907 | −0.812907 |
| −0.812907 | −0.812907 | −0.673649 | −0.673649 | −0.673649 | −0.673649 |
| −0.673649 | −0.583190 | −0.583190 | −0.583190 | −0.583190 | −0.583190 |
| −0.120318 | −0.120318 | −0.120318 | −0.059657 | −0.059657 | −0.059657 |
| 0.436773 | 0.436773 | 0.436773 | 0.436773 | 0.436773 | 0.535260 |
| 0.535260 | 0.535260 | 0.535260 | 0.535260 | 0.634142 | 0.634142 |
| 0.634142 | 0.634142 | 0.795960 | 0.795960 | 0.795960 | 0.795960 |
| 0.807945 | 0.807945 | 0.807945 | 0.881840 | 0.881840 | 0.881840 |
| 0.908763 | 0.908763 | 0.908763 | 0.908763 | 0.908763 | 1.000000 |
| 1.000000 | 1.000000 | 1.000000 | 1.000000 | 1.000000 | 1.000000 |
| 1.000000 | 1.000000 | 1.000000 | 1.178291 | 1.178291 | 1.178291 |
| 1.178291 | 1.178291 | 1.187699 | 1.187699 | 1.187699 | 1.187699 |
| 1.239628 | 1.239628 | 1.239628 | 1.298090 | 1.298090 | 1.298090 |
| 1.298090 | 1.298090 | 1.503117 | 1.503117 | 1.503117 | 1.503117 |
| 1.521385 | 1.521385 | 1.521385 | 1.521385 | 1.521385 | 1.556133 |
| 1.556133 | 1.556133 | 1.611066 | 1.611066 | 1.611066 | 1.815577 |
| 1.815577 | 1.815577 | 1.815577 | 1.850203 | 1.850203 | 1.850203 |
| 1.866030 | 1.866030 | 1.866030 | 1.866030 | 1.866030 | 2.000000 |
| 2.128884 | 2.128884 | 2.128884 | 2.128884 | 2.128884 | 2.142063 |
| 2.142063 | 2.142063 | 2.215507 | 2.215507 | 2.215507 | 2.392017 |
| 2.392017 | 2.392017 | 2.392017 | 2.445125 | 2.445125 | 2.445125 |
| 2.445125 | 2.445125 | 2.625191 | 2.625191 | 2.625191 | 2.625191 |
| 2.673879 | 2.673879 | 2.673879 | 2.821200 | 2.821200 | 2.821200 |
| 2.821200 | 2.821200 | 2.939600 | 2.939600 | 2.939600 | 3.000000 |
| Spectra of Icosahedral Fullerene C80 (Ih) | |||||
| −2.699315 | −2.699315 | −2.699315 | −2.651093 | −2.651093 | −2.651093 |
| −2.651093 | −2.198691 | −2.198691 | −2.198691 | −2.198691 | −1.935432 |
| −1.935432 | −1.935432 | −1.935432 | −1.935432 | −1.618034 | −1.618034 |
| −1.618034 | −1.618034 | −1.618034 | −1.618034 | −1.618034 | −1.618034 |
| −1.200081 | −1.200081 | −1.200081 | −1.0 | −1.0 | −1.0 |
| −1.0 | −1.0 | −1.0 | −0.713538 | −0.713538 | −0.713538 |
| −0.713538 | 0.273891 | 0.273891 | 0.273891 | 0.273891 | 0.618034 |
| 0.618034 | 0.618034 | 0.618034 | 0.618034 | 0.618034 | 0.618034 |
| 0.618034 | 1.0 | 1.0 | 1.0 | 1.0 | 1.0 |
| 1.0 | 1.377203 | 1.377203 | 1.377203 | 1.377203 | 1.462598 |
| 1.462598 | 1.462598 | 1.462598 | 1.462598 | 1.912229 | 1.912229 |
| 1.912229 | 1.912229 | 281281 | 281281 | 281281 | 2.472834 |
| 2.472834 | 2.472834 | 2.472834 | 2.472834 | 2.818115 | 2.818115 |
| 2.818115 | 3.0 | ||||
| G(60,3)—All Odd Coefs Are Zero Only Even Coefs Are Shown | Archimedene C120 Ih | ||||
|---|---|---|---|---|---|
| k | Cb-k | Cn-k | |||
| 120 | 1 | 1 | |||
| 118 | −180 | −180 | |||
| 116 | 15,750 | 15,690 | |||
| 114 | −892,860 | −882,460 | |||
| 112 | 36,876,345 | 36,002,715 | |||
| 110 | −1,183,071,096 | −1,135,676,880 | |||
| 108 | 30,697,459,620 | 28,830,540,620 | |||
| 106 | −662,294,994,720 | 605,370,867,120 | |||
| 104 | 12,122,712,447,420 | 10,724,090,893,725 | |||
| 102 | −191,146,403,259,680 | −162,682,223,973,660 | |||
| 100 | 2,627,342,156,012,562 | 2,137,824,997,367,262 | |||
| 98 | −31,781,816,819,028,000 | −24,559,722,045,606,780 | |||
| 96 | 340,965,242,569,443,770 | 248,476,237,317,489,919 | |||
| 94 | −3,264,986,310,221,207,160 | −2,227,172,552,007,275,639 | |||
| 92 | 28,054,594,391,644,991,610 | 17,773,081,766,605,412,040 | |||
| 90 | −217,281,600,535,414,573,160 | −126,785,990,833,562,201,901 | |||
| 88 | 1,522,597,140,765,638,802,780 | 811,214,030,007,340,751,802 | |||
| 86 | −9,684,792,477,464,440,484,880 | −4,668,308,290,447,788,760,611 | |||
| 84 | 56,070,178,428,467,218,064,830 | 24,217,880,944,735,623,869,286 | |||
| 82 | −296,160,109,890,416,361,428,760 | −11,346,917,356,303,897,063,139 | |||
| 80 | 1,430,008,713,073,408,819,374,015 | 480,886,446,025,397,096,756,536 | |||
| 78 | −6,322,658,765,178,971,561,833,460 | −1,845,667,128,265,467,014,180,368 | |||
| 76 | 25,634,415,237,650,811,338,783,790 | 6,421,197,446,225,090,384,763,177 | |||
| 74 | −95,415,256,746,818,787,811,819,500 | −20,264,333,185,513,523,340,033,948 | |||
| 72 | 326,358,788,644,990,105,475,935,560 | 58,037,942,867,756,242,524,535,748 | |||
| 70 | −1,026,550,222,361,385,631,900,698,756 | −150,897,704,088,219,599,214,664,480 | |||
| 68 | 2,971,083,522,023,498,075,620,793,850 | 356,203,198,431,727,918,898,852,579 | |||
| 66 | −7,915,244,885,046,631,268,351,837,700 | −763,386,163,922,575,136,997,166,481 | |||
| 64 | 19,414,214,933,695,045,040,860,422,315 | 1,485,072,345,968,873,883,971,819,917 | |||
| 62 | −43,843,051,820,752,972,910,346,678,720 | −2,621,662,455,033,825,652,650,138,049 | |||
| 60 | 91,149,903,160,281,097,618,182,429,904 | 4,198,049,320,217,010,824,338,368,942 | |||
| 58 | −174,407,398,329,673,957,422,500,193,480 | −6,094,310,658,772,104,625,950,326,473 | |||
| 56 | 306,997,303,344,994,767,604,833,350,865 | 8,015,348,715,315,034,977,772,825,607 | |||
| 54 | −496,821,074,662,257,075,415,252,129,260 | −9,543,437,842,639,635,315,518,318,973 | |||
| 52 | 738,618,693,971,750,466,919,056,091,500 | 1.027739682936630734700449256×1028 | |||
| 50 | −1,007,800,557,988,460,823,587,457,781,068 | −1.000028973818866162960590646×1028 | |||
| 48 | 1,260,540,542,432,930,681,069,386,712,300 | 8,781,884,478,265,548,931,050,238,250 | |||
| 46 | −1,443,340,157,403,216,035,993,067,030,060 | −6,950,813,776,786,441,008,698,586,201 | |||
| 44 | 1,510,466,448,053,717,869,780,001,900,430 | 4,951,169,673,130,384,907,223,135,336 | |||
| 42 | −1,442,026,246,599,052,952,378,971,479,380 | −3,168,616,508,417,229,180,219,494,941 | |||
| 40 | 1,253,196,204,279,457,770,381,146,932,305 | 1,818,395,639,160,030,392,431,910,492 | |||
| 38 | −988,943,111,078,689,399,839,434,418,960 | −933,722,074,986,794,748,257,541,866 | |||
| 36 | 706,626,962,932,350,094,618,120,735,490 | 427,936,739,631,884,433,905,609,166 | |||
| 34 | −455,667,663,241,511,840,138,930,828,880 | −174,559,200,434,963,752,237,231,269 | |||
| 32 | 264,178,558,483,371,293,785,696,421,190 | 63,167,679,119,015,442,272,490,183 | |||
| 30 | −137,097,377,892,211,849,514,626,681,408 | −20,202,702,500,850,294,524,063,193 | |||
| 28 | 63,360,615,577,689,007,821,823,287,330 | 5,685,948,285,290,922,542,550,711 | |||
| 26 | −25,921,535,062,352,622,278,618,137,560 | −1,401,137,114,127,009,299,874,253 | |||
| 24 | 9,321,131,766,342,541,097,932,696,205 | 300,515,209,314,416,465,229,072 | |||
| 22 | −2,921,155,890,212,282,221,257,004,020 | −55,706,952,143,363,900,167,310 | |||
| 20 | 789,674,303,777,263,666,519,980,294 | 8,850,309,146,844,763,155,406 | |||
| 18 | −181,817,382,076,098,654,444,319,620 | -I,192,863,242,058,215,039,057 | |||
| 16 | 35,088,808,404,272,449,157,332,545 | 134,700,818,861,930,470,640 | |||
| 14 | −5,559,522,299,955,307,222,951,320 | −12,545,905,215,362,926,146 | |||
| 12 | 703,240,714,219,584,001,807,760 | 944,752,313,686,677,771 | |||
| 10 | −68,254,034,915,538,778,780,032 | −56,038,624,208,633,088 | |||
| 8 | 4,782,522,709,793,521,720,320 | 2,527,779,421,175,040 | |||
| 6 | −217,682,509,196,494,551,040 | −82,532,952,345,600 | |||
| 4 | 5,118,895,223,567,585,280 | 1,811,566,632,960 | |||
| 2 | −24,777,598,446,305,280 | −23,581,808,640 | |||
| 0 | 33,790,875,992,064 | 136,048,896 | |||
| Spectra of Generalized Petersen Graph G(60,3) | |||||
| −3.000000 | −2.946523 | −2.946523 | −2.801366 | −2.801366 | −2.602781 |
| −2.602781 | −2.391089 | −2.391089 | −2.302776 | −2.302776 | −2.230618 |
| −2.230618 | −2.198492 | −2.198492 | −2.188901 | −2.188901 | −2.000000 |
| −2.000000 | −2.000000 | −2.000000 | −1.924521 | −1.924521 | −1.820104 |
| −1.820104 | −1.657815 | −1.657815 | −1.644748 | −1.644748 | −1.509385 |
| −1.509385 | −1.471949 | −1.471949 | −1.302776 | −1.302776 | −1.286625 |
| −1.286625 | −1.141979 | −1.141979 | −1.000000 | −1.000000 | −1.000000 |
| −1.000000 | −1.000000 | −1.000000 | −1.000000 | −0.944634 | −0.944634 |
| −0.898068 | −0.898068 | −0.877648 | −0.877648 | −0.772963 | −0.772963 |
| −0.474903 | −0.474903 | −0.456850 | −0.456850 | −0.054036 | −0.054036 |
| 0.054036 | 0.054036 | 0.456850 | 0.456850 | 0.474903 | 0.474903 |
| 0.772963 | 0.772963 | 0.877648 | 0.877648 | 0.898068 | 0.898068 |
| 0.944634 | 0.944634 | 1.000000 | 1.000000 | 1.000000 | 1.000000 |
| 1.000000 | 1.000000 | 1.000000 | 1.141979 | 1.141979 | 1.286625 |
| 1.286625 | 1.302776 | 1.302776 | 1.471949 | 1.471949 | 1.509385 |
| 1.509385 | 1.644748 | 1.644748 | 1.657815 | 1.657815 | 1.820104 |
| 1.820104 | 1.924521 | 1.924521 | 2.000000 | 2.000000 | 2.000000 |
| 2.000000 | 2.188901 | 2.188901 | 2.198492 | 2.198492 | 2.230618 |
| 2.230618 | 2.302776 | 2.302776 | 2.391089 | 2.391089 | 2.602781 |
| 2.602781 | 2.801366 | 2.801366 | 2.946523 | 2.946523 | 3.000000 |
| Spectra of Archimedene C120 (Ih) | |||||
| −3.00000 | −2.90210 | −2.90210 | −2.90210 | −2.72140 | −2.72140 |
| −2.72140 | −2.72140 | −2.72140 | −2.54502 | −2.54502 | −2.54502 |
| −2.54502 | −2.17561 | −2.17561 | −2.17561 | −2.15420 | −2.15420 |
| −2.15420 | −2.15420 | −1.88840 | −1.88840 | −1.88840 | −1.88840 |
| −1.88840 | −1.82800 | −1.82800 | −1.82800 | −1.82800 | −1.82800 |
| −1.00000 | −1.00000 | −1.00000 | −1.00000 | −1.00000 | −1.00000 |
| −0.90210 | −0.90210 | −0.90210 | −0.83021 | −0.83021 | −0.83021 |
| −0.83021 | −0.68460 | −0.68460 | −0.68460 | −0.68460 | −0.68460 |
| −0.46641 | −0.46641 | −0.46641 | −0.46641 | −0.46641 | −0.43940 |
| −0.43940 | −0.43940 | −0.43940 | −0.17561 | −0.17561 | −0.17561 |
| 0.17561 | 0.17561 | 0.17561 | 0.43940 | 0.43940 | 0.43940 |
| 0.43940 | 0.46641 | 0.46641 | 0.46641 | 0.46641 | 0.46641 |
| 0.68460 | 0.68460 | 0.68460 | 0.68460 | 0.68460 | 0.83021 |
| 0.83021 | 0.83021 | 0.83021 | 0.90210 | 0.90210 | 0.90210 |
| 1.00000 | 1.00000 | 1.00000 | 1.00000 | 1.00000 | 1.00000 |
| 1.82800 | 1.82800 | 1.82800 | 1.82800 | 1.82800 | 1.88840 |
| 1.88840 | 1.88840 | 1.88840 | 1.88840 | 2.15420 | 2.15420 |
| 2.15420 | 2.15420 | 2.17561 | 2.17561 | 2.17561 | 2.54502 |
| 2.54502 | 2.54502 | 2.54502 | 2.72140 | 2.72140 | 2.72140 |
| 2.72140 | 2.72140 | 2.90210 | 2.90210 | 2.90210 | 3.00000 |
| Spectral Polynomial of Generalized Petersen Graph G(360,19) | |||||
|---|---|---|---|---|---|
| K | Cn-k | ||||
| 720 | 1 | ||||
| 718 | −1080 | ||||
| 716 | 580,500 | ||||
| 714 | −207,046,440 | ||||
| 712 | 55,127,270,610 | ||||
| 710 | −11,687,473,970,856 | ||||
| 708 | 2,055,192,601,955,220 | ||||
| 706 | −308,311,704,981,633,240 | ||||
| 704 | 40,279,248,752,112,449,415 | ||||
| 702 | −4,655,425,300,557,056,838,960 | ||||
| 700 | 481,961,518,974,263,346,274,728 | ||||
| 698 | −45,143,834,567,423,768,973,556,560 | ||||
| 696 | 3,857,580,142,194,956,808,063,882,900 | ||||
| 694 | −3,028,178,938,227,920,486,720,376,156,005 | ||||
| 692 | 21,966,898,016,471,960,685,886,317,562,680 | ||||
| 690 | −1,480,100,147,994,601,999,836,922,582,716,650 | ||||
| 688 | 93,041,387,533,636,155,387,504,144,396,818,912 | ||||
| … | … | ||||
| 11 | 0 | ||||
| …. | |||||
| 0 | 0 | ||||
| Spectra of Generalized Petersen Graph G(360,19) | |||||
| −3.000000 | −2.946841 | −2.946841 | −2.946841 | −2.946841 | −2.902113 |
| −2.902113 | −2.879385 | −2.879385 | −2.809498 | −2.809498 | −2.809498 |
| −2.809498 | −2.767294 | −2.767294 | −2.767294 | −2.767294 | −2.722888 |
| −2.722888 | −2.722888 | −2.722888 | −2.641498 | −2.641498 | −2.641498 |
| −2.641498 | −2.618034 | −2.618034 | −2.598258 | −2.598258 | −2.598258 |
| −2.598258 | −2.533123 | −2.533123 | −2.533123 | −2.533123 | −2.532089 |
| −2.532089 | −2.492879 | −2.492879 | −2.492879 | −2.492879 | −2.449490 |
| −2.449490 | −2.449490 | −2.449490 | −2.422441 | −2.422441 | −2.422441 |
| −2.422441 | −2.383369 | −2.383369 | −2.383369 | −2.383369 | −2.363567 |
| −2.363567 | −2.363567 | −2.363567 | −2.343352 | −2.343352 | −2.343352 |
| −2.343352 | −2.309275 | −2.309275 | −2.309275 | −2.309275 | −2.298068 |
| −2.298068 | −2.298068 | −2.298068 | −2.275827 | −2.275827 | −2.275827 |
| −2.275827 | −2.260958 | −2.260958 | −2.260958 | −2.260958 | −2.247589 |
| −2.247589 | −2.247589 | −2.247589 | −2.236068 | −2.236068 | −2.236068 |
| −2.236068 | −2.236068 | −2.236068 | −2.236068 | −2.236068 | −2.230746 |
| −2.230746 | −2.230746 | −2.230746 | −2.215479 | −2.215479 | −2.215479 |
| −2.215479 | −2.214072 | −2.214072 | −2.214072 | −2.214072 | −2.208933 |
| −2.208933 | −2.208933 | −2.208933 | −2.175571 | −2.175571 | −2.146832 |
| −2.146832 | −2.146832 | −2.146832 | −2.125697 | −2.125697 | −2.125697 |
| −2.125697 | −2.085383 | −2.085383 | −2.085383 | −2.085383 | −2.085285 |
| −2.085285 | −2.085285 | −2.085285 | −2.052962 | −2.052962 | −2.052962 |
| −2.052962 | −2.043421 | −2.043421 | −2.043421 | −2.043421 | −2.016105 |
| −2.016105 | −2.016105 | −2.016105 | −2.014904 | −2.014904 | −2.014904 |
| −2.014904 | −2.001464 | −2.001464 | −2.001464 | −2.001464 | −2.000000 |
| −2.000000 | −2.000000 | −2.000000 | −2.000000 | −2.000000 | −1.940970 |
| −1.940970 | −1.940970 | −1.940970 | −1.924015 | −1.924015 | −1.924015 |
| −1.924015 | −1.810771 | −1.810771 | −1.810771 | −1.810771 | −1.773754 |
| −1.773754 | −1.773754 | −1.773754 | −1.732051 | −1.732051 | −1.732051 |
| −1.732051 | −1.717941 | −1.717941 | −1.717941 | −1.717941 | −1.677111 |
| −1.677111 | −1.677111 | −1.677111 | −1.676487 | −1.676487 | −1.676487 |
| −1.676487 | −1.639862 | −1.639862 | −1.639862 | −1.639862 | −1.628712 |
| −1.628712 | −1.628712 | −1.628712 | −1.618034 | −1.618034 | −1.618034 |
| −1.618034 | −1.618034 | −1.618034 | −1.612687 | −1.612687 | −1.612687 |
| −1.612687 | −1.500960 | −1.500960 | −1.500960 | −1.500960 | −1.365312 |
| −1.365312 | −1.365312 | −1.365312 | −1.350714 | −1.350714 | −1.350714 |
| −1.350714 | −1.347296 | −1.347296 | −1.248834 | −1.248834 | −1.248834 |
| −1.248834 | −1.211574 | −1.211574 | −1.211574 | −1.211574 | −1.179453 |
| −1.179453 | −1.179453 | −1.179453 | −1.134582 | −1.134582 | −1.134582 |
| −1.134582 | −1.133704 | −1.133704 | −1.133704 | −1.133704 | −1.108549 |
| −1.108549 | −1.108549 | −1.108549 | −1.106079 | −1.106079 | −1.106079 |
| −1.106079 | −1.047801 | −1.047801 | −1.047801 | −1.047801 | −1.000000 |
| −1.000000 | −1.000000 | −0.943892 | −0.943892 | −0.943892 | −0.943892 |
| −0.902113 | −0.902113 | −0.879385 | −0.879385 | −0.840363 | −0.840363 |
| −0.840363 | −0.840363 | −0.765305 | −0.765305 | −0.765305 | −0.765305 |
| −0.749080 | −0.749080 | −0.749080 | −0.749080 | −0.742587 | −0.742587 |
| −0.742587 | −0.742587 | −0.705046 | −0.705046 | −0.705046 | −0.705046 |
| −0.698564 | −0.698564 | −0.698564 | −0.698564 | −0.652704 | −0.652704 |
| −0.618034 | −0.618034 | −0.618034 | −0.618034 | −0.618034 | −0.618034 |
| −0.612230 | −0.612230 | −0.612230 | −0.612230 | −0.565291 | −0.565291 |
| −0.565291 | −0.565291 | −0.553028 | −0.553028 | −0.553028 | −0.553028 |
| −0.542273 | −0.542273 | −0.542273 | −0.542273 | −0.532089 | −0.532089 |
| −0.445039 | −0.445039 | −0.445039 | −0.445039 | −0.442652 | −0.442652 |
| −0.442652 | −0.442652 | −0.381966 | −0.381966 | −0.381083 | −0.381083 |
| −0.381083 | −0.381083 | −0.366704 | −0.366704 | −0.366704 | −0.366704 |
| −0.363016 | −0.363016 | −0.363016 | −0.363016 | −0.241427 | −0.241427 |
| −0.241427 | −0.241427 | −0.183172 | −0.183172 | −0.183172 | −0.183172 |
| −0.175571 | −0.175571 | −0.152597 | −0.152597 | −0.152597 | −0.152597 |
| −0.072655 | −0.072655 | −0.072655 | −0.072655 | −0.038239 | −0.038239 |
| −0.038239 | −0.038239 | −0.013907 | −0.013907 | −0.013907 | −0.013907 |
| −0.000000 | −0.000000 | −0.000000 | −0.000000 | −0.000000 | −0.000000 |
| 0.000000 | 0.000000 | 0.000000 | 0.000000 | 0.000000 | 0.000000 |
| 0.013907 | 0.013907 | 0.013907 | 0.013907 | 0.038239 | 0.038239 |
| 0.038239 | 0.038239 | 0.072655 | 0.072655 | 0.072655 | 0.072655 |
| 0.152597 | 0.152597 | 0.152597 | 0.152597 | 0.175571 | 0.175571 |
| 0.183172 | 0.183172 | 0.183172 | 0.183172 | 0.241427 | 0.241427 |
| 0.241427 | 0.241427 | 0.363016 | 0.363016 | 0.363016 | 0.363016 |
| 0.366704 | 0.366704 | 0.366704 | 0.366704 | 0.381083 | 0.381083 |
| 0.381083 | 0.381083 | 0.381966 | 0.381966 | 0.442652 | 0.442652 |
| 0.442652 | 0.442652 | 0.445039 | 0.445039 | 0.445039 | 0.445039 |
| 0.532089 | 0.532089 | 0.542273 | 0.542273 | 0.542273 | 0.542273 |
| 0.553028 | 0.553028 | 0.553028 | 0.553028 | 0.565291 | 0.565291 |
| 0.565291 | 0.565291 | 0.612230 | 0.612230 | 0.612230 | 0.612230 |
| 0.618034 | 0.618034 | 0.618034 | 0.618034 | 0.618034 | 0.618034 |
| 0.652704 | 0.652704 | 0.698564 | 0.698564 | 0.698564 | 0.698564 |
| 0.705046 | 0.705046 | 0.705046 | 0.705046 | 0.742587 | 0.742587 |
| 0.742587 | 0.742587 | 0.749080 | 0.749080 | 0.749080 | 0.749080 |
| 0.765305 | 0.765305 | 0.765305 | 0.765305 | 0.840363 | 0.840363 |
| 0.840363 | 0.840363 | 0.879385 | 0.879385 | 0.902113 | 0.902113 |
| 0.943892 | 0.943892 | 0.943892 | 0.943892 | 1.000000 | 1.000000 |
| 1.000000 | 1.047801 | 1.047801 | 1.047801 | 1.047801 | 1.106079 |
| 1.106079 | 1.106079 | 1.106079 | 1.108549 | 1.108549 | 1.108549 |
| 1.108549 | 1.133704 | 1.133704 | 1.133704 | 1.133704 | 1.134582 |
| 1.134582 | 1.134582 | 1.134582 | 1.179453 | 1.179453 | 1.179453 |
| 1.179453 | 1.211574 | 1.211574 | 1.211574 | 1.211574 | 1.248834 |
| 1.248834 | 1.248834 | 1.248834 | 1.347296 | 1.347296 | 1.350714 |
| 1.350714 | 1.350714 | 1.350714 | 1.365312 | 1.365312 | 1.365312 |
| 1.365312 | 1.500960 | 1.500960 | 1.500960 | 1.500960 | 1.612687 |
| 1.612687 | 1.612687 | 1.612687 | 1.618034 | 1.618034 | 1.618034 |
| 1.618034 | 1.618034 | 1.618034 | 1.628712 | 1.628712 | 1.628712 |
| 1.628712 | 1.639862 | 1.639862 | 1.639862 | 1.639862 | 1.676487 |
| 1.676487 | 1.676487 | 1.676487 | 1.677111 | 1.677111 | 1.677111 |
| 1.677111 | 1.717941 | 1.717941 | 1.717941 | 1.717941 | 1.732051 |
| 1.732051 | 1.732051 | 1.732051 | 1.773754 | 1.773754 | 1.773754 |
| 1.773754 | 1.810771 | 1.810771 | 1.810771 | 1.810771 | 1.924015 |
| 1.924015 | 1.924015 | 1.924015 | 1.940970 | 1.940970 | 1.940970 |
| 1.940970 | 2.000000 | 2.000000 | 2.000000 | 2.000000 | 2.000000 |
| 2.000000 | 2.001464 | 2.001464 | 2.001464 | 2.001464 | 2.014904 |
| 2.014904 | 2.014904 | 2.014904 | 2.016105 | 2.016105 | 2.016105 |
| 2.016105 | 2.043421 | 2.043421 | 2.043421 | 2.043421 | 2.052962 |
| 2.052962 | 2.052962 | 2.052962 | 2.085285 | 2.085285 | 2.085285 |
| 2.085285 | 2.085383 | 2.085383 | 2.085383 | 2.085383 | 2.125697 |
| 2.125697 | 2.125697 | 2.125697 | 2.146832 | 2.146832 | 2.146832 |
| 2.146832 | 2.175571 | 2.175571 | 2.208933 | 2.208933 | 2.208933 |
| 2.208933 | 2.214072 | 2.214072 | 2.214072 | 2.214072 | 2.215479 |
| 2.215479 | 2.215479 | 2.215479 | 2.230746 | 2.230746 | 2.230746 |
| 2.230746 | 2.236068 | 2.236068 | 2.236068 | 2.236068 | 2.236068 |
| 2.236068 | 2.236068 | 2.236068 | 2.247589 | 2.247589 | 2.247589 |
| 2.247589 | 2.260958 | 2.260958 | 2.260958 | 2.260958 | 2.275827 |
| 2.275827 | 2.275827 | 2.275827 | 2.298068 | 2.298068 | 2.298068 |
| 2.298068 | 2.309275 | 2.309275 | 2.309275 | 2.309275 | 2.343352 |
| 2.343352 | 2.343352 | 2.343352 | 2.363567 | 2.363567 | 2.363567 |
| 2.363567 | 2.383369 | 2.383369 | 2.383369 | 2.383369 | 2.422441 |
| 2.422441 | 2.422441 | 2.422441 | 2.449490 | 2.449490 | 2.449490 |
| 2.449490 | 2.492879 | 2.492879 | 2.492879 | 2.492879 | 2.532089 |
| 2.532089 | 2.533123 | 2.533123 | 2.533123 | 2.533123 | 2.598258 |
| 2.598258 | 2.598258 | 2.598258 | 2.618034 | 2.618034 | 2.641498 |
| 2.641498 | 2.641498 | 2.641498 | 2.722888 | 2.722888 | 2.722888 |
| 2.722888 | 2.767294 | 2.767294 | 2.767294 | 2.767294 | 2.809498 |
| 2.809498 | 2.809498 | 2.809498 | 2.879385 | 2.879385 | 2.902113 |
| 2.902113 | 2.946841 | 2.946841 | 2.946841 | 2.946841 | 3.000000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Balasubramanian, K. Spectral Polynomials and Spectra of Graphs Beyond Cubic and Icosahedral Symmetries: n-Octahedra, n-Cubes, Symmetric and Semi-Symmetric Graphs, Giant Fullerene Cages and Generalized Petersen Graphs. Symmetry 2025, 17, 247. https://doi.org/10.3390/sym17020247
Balasubramanian K. Spectral Polynomials and Spectra of Graphs Beyond Cubic and Icosahedral Symmetries: n-Octahedra, n-Cubes, Symmetric and Semi-Symmetric Graphs, Giant Fullerene Cages and Generalized Petersen Graphs. Symmetry. 2025; 17(2):247. https://doi.org/10.3390/sym17020247
Chicago/Turabian StyleBalasubramanian, Krishnan. 2025. "Spectral Polynomials and Spectra of Graphs Beyond Cubic and Icosahedral Symmetries: n-Octahedra, n-Cubes, Symmetric and Semi-Symmetric Graphs, Giant Fullerene Cages and Generalized Petersen Graphs" Symmetry 17, no. 2: 247. https://doi.org/10.3390/sym17020247
APA StyleBalasubramanian, K. (2025). Spectral Polynomials and Spectra of Graphs Beyond Cubic and Icosahedral Symmetries: n-Octahedra, n-Cubes, Symmetric and Semi-Symmetric Graphs, Giant Fullerene Cages and Generalized Petersen Graphs. Symmetry, 17(2), 247. https://doi.org/10.3390/sym17020247





