Investigating the Symmetric Control of a Hydraulic System Based on Status Feedback
Abstract
1. Introduction
2. System Modelling
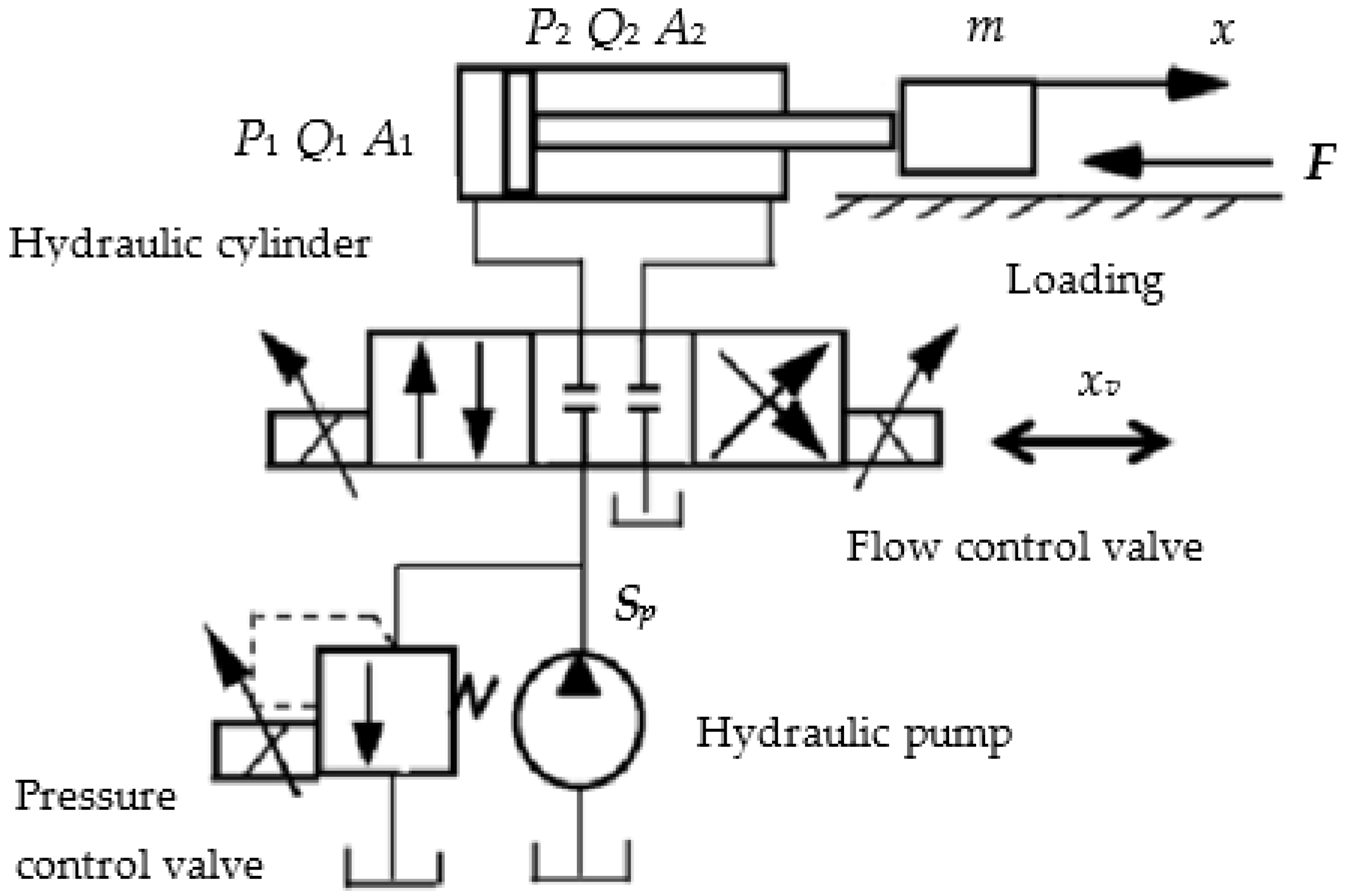
2.1. Basic Description
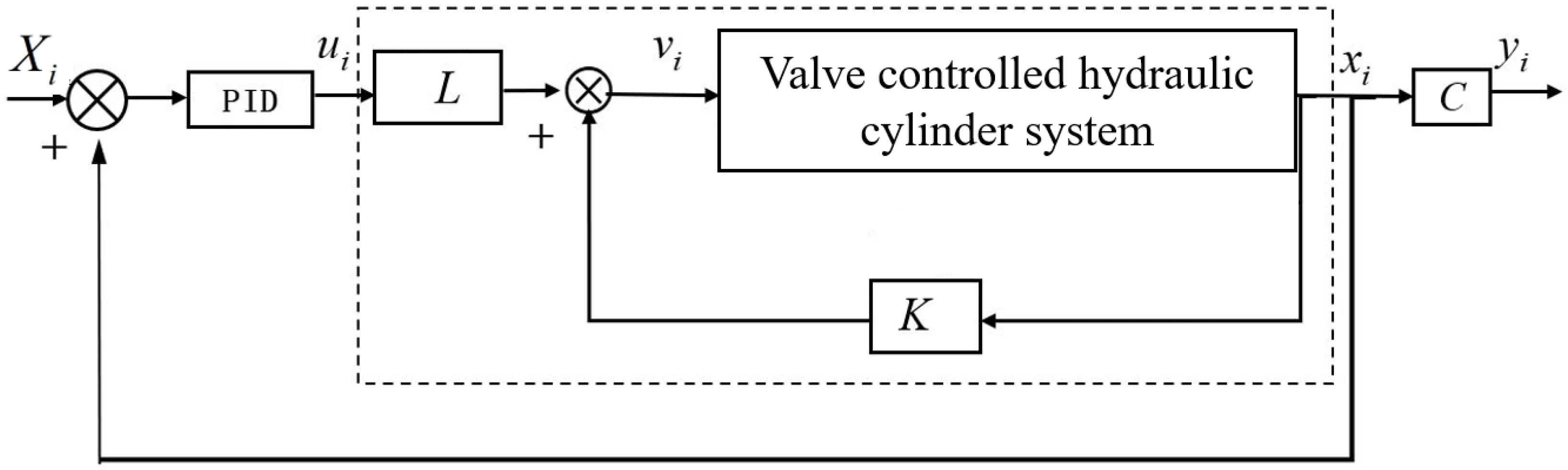
2.2. Mathematical Model of the System State Space
2.3. System Simulation Model
3. Results and Discussion
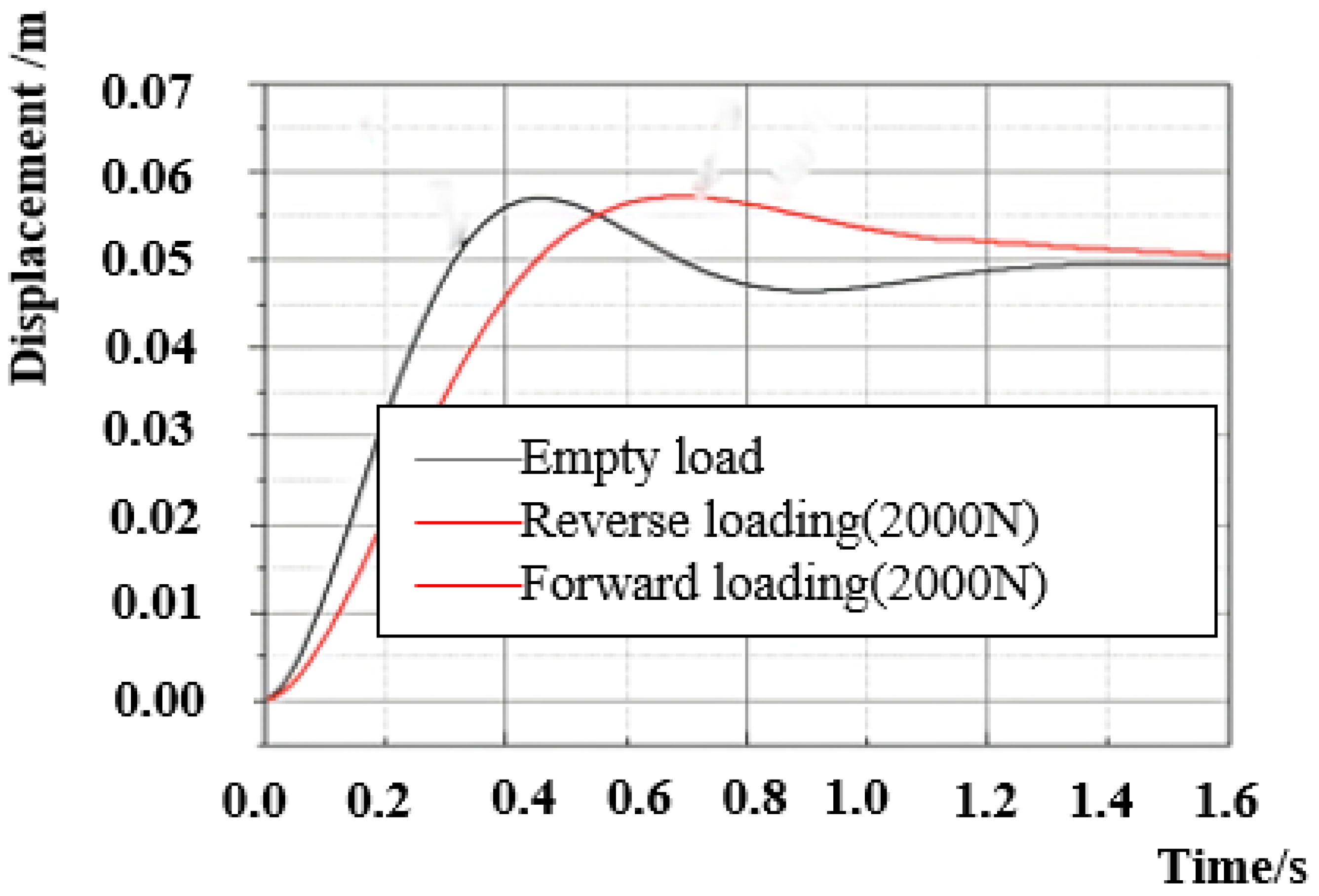
3.1. Influence of Asymmetric Dynamic Characteristics of Systems
3.1.1. Influence of Asymmetric Structure on Dynamic Characteristics
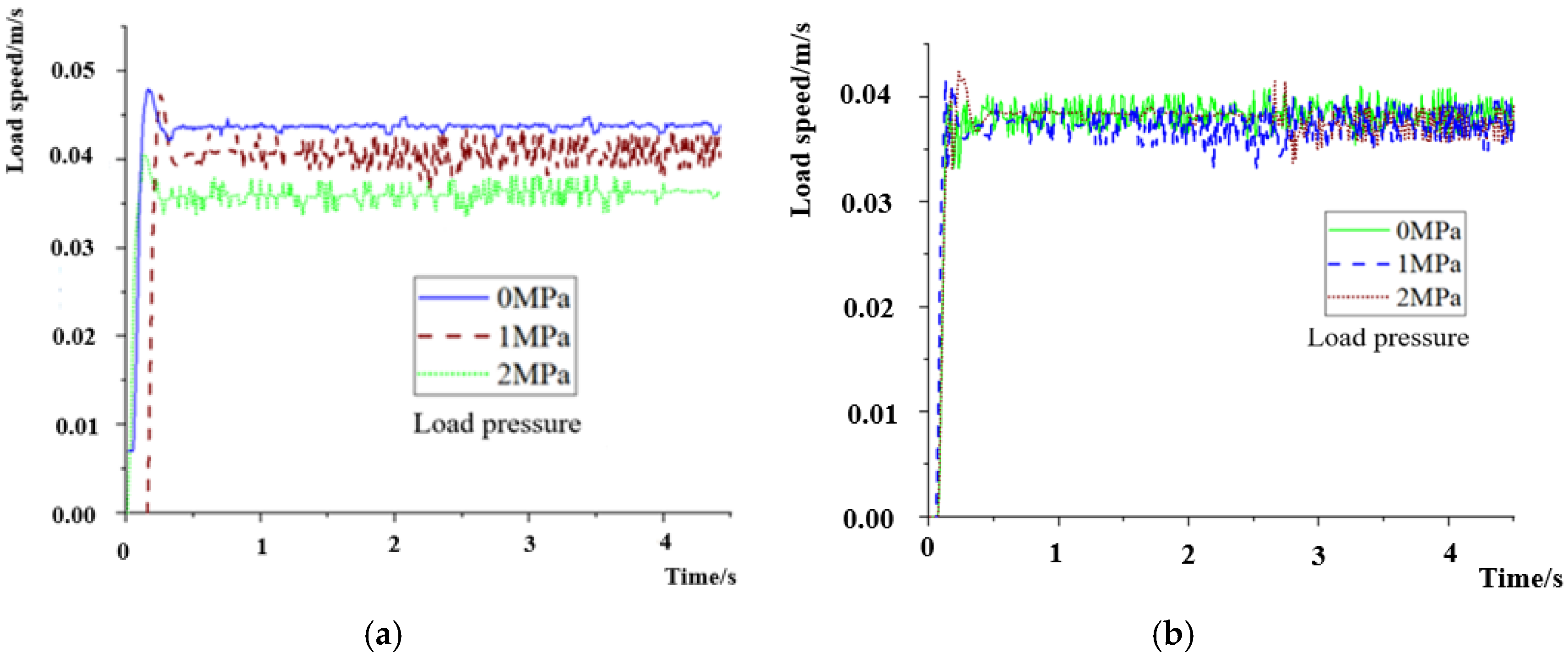
3.1.2. Influence of Asymmetric External Load on Dynamic Characteristics
3.1.3. Asymmetric Dynamic Characteristics of Systems Under Coupling Effects
3.2. Symmetric Controller System Design Based on State Feedback
3.2.1. Symmetric Controller System Design
3.2.2. System Symmetry and Stability Verification
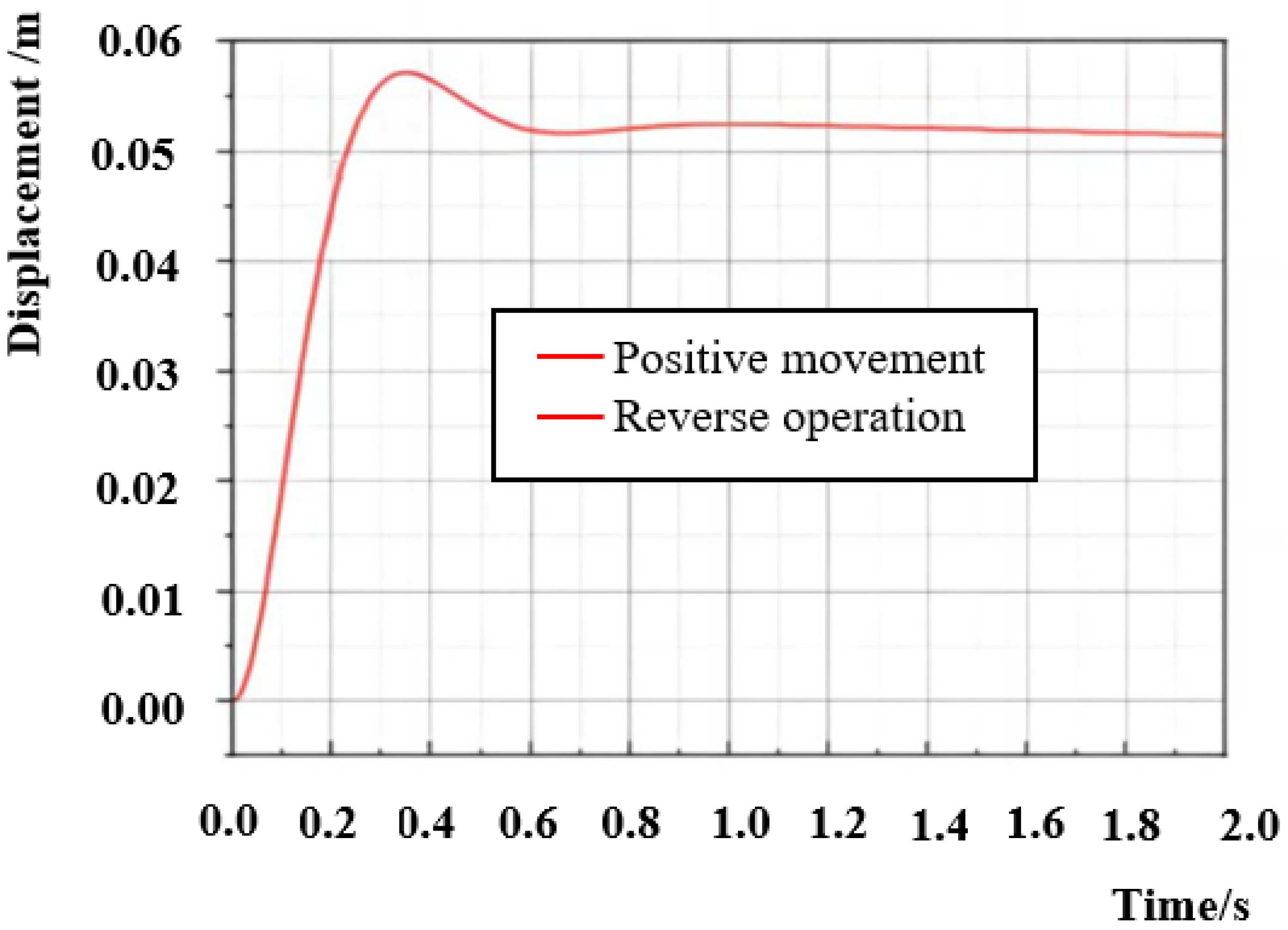
3.2.3. Consistent Responsiveness of System Dynamic Characteristics
3.3. Experimental Verification
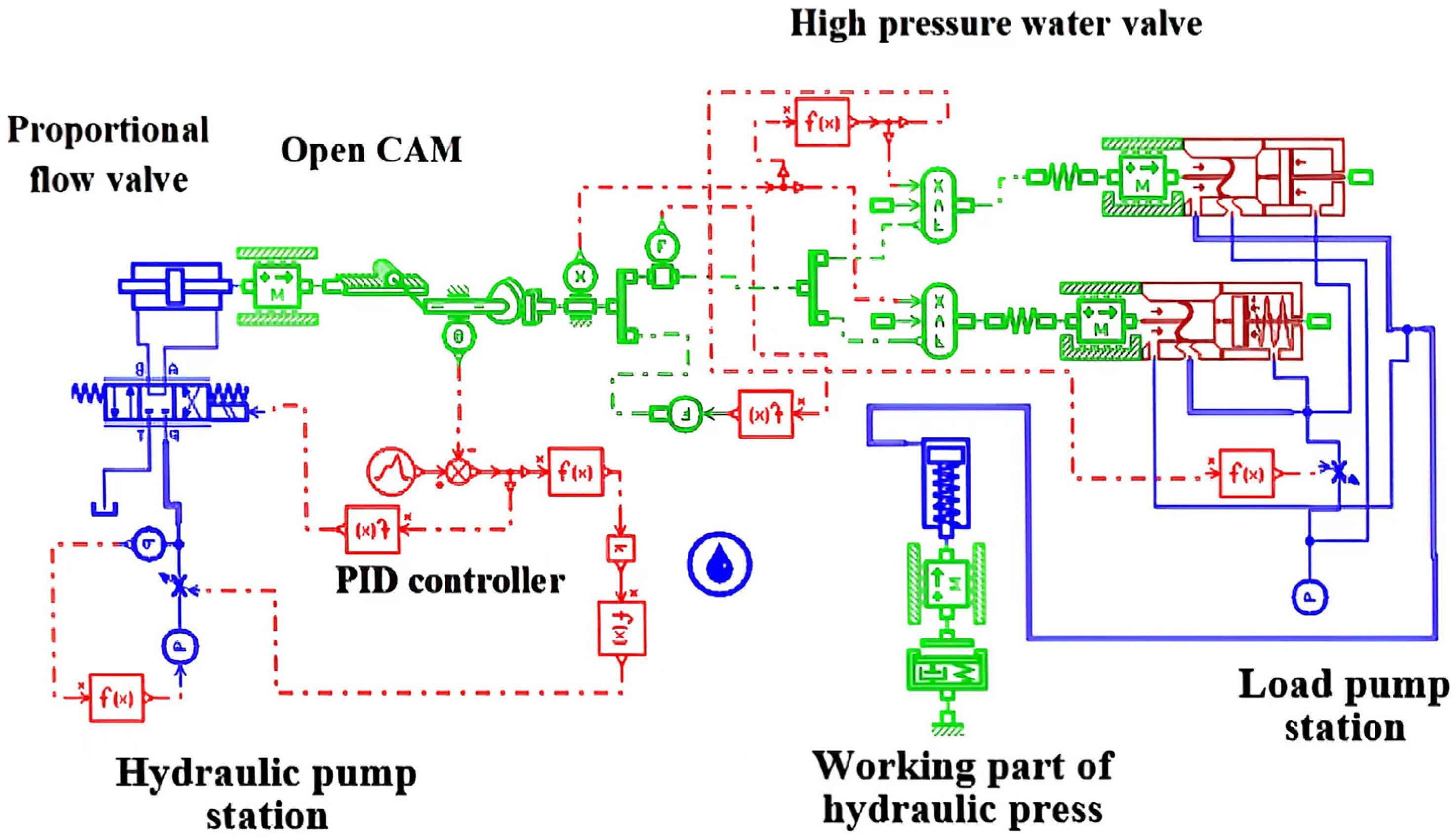
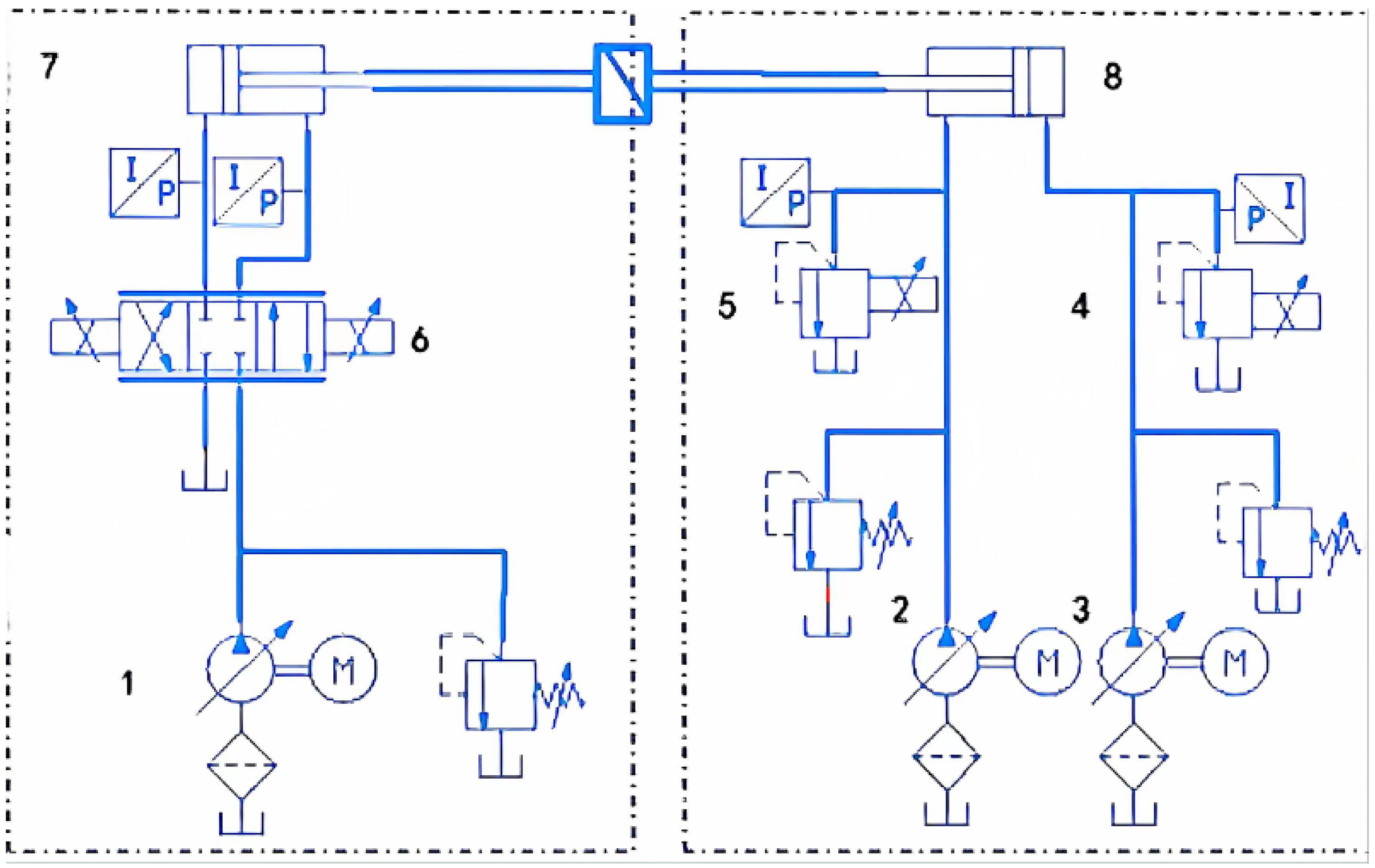
3.3.1. Introduction to Experimental Equipment
3.3.2. Basic Experimental Parameters
3.3.3. Experimental Methods and Steps
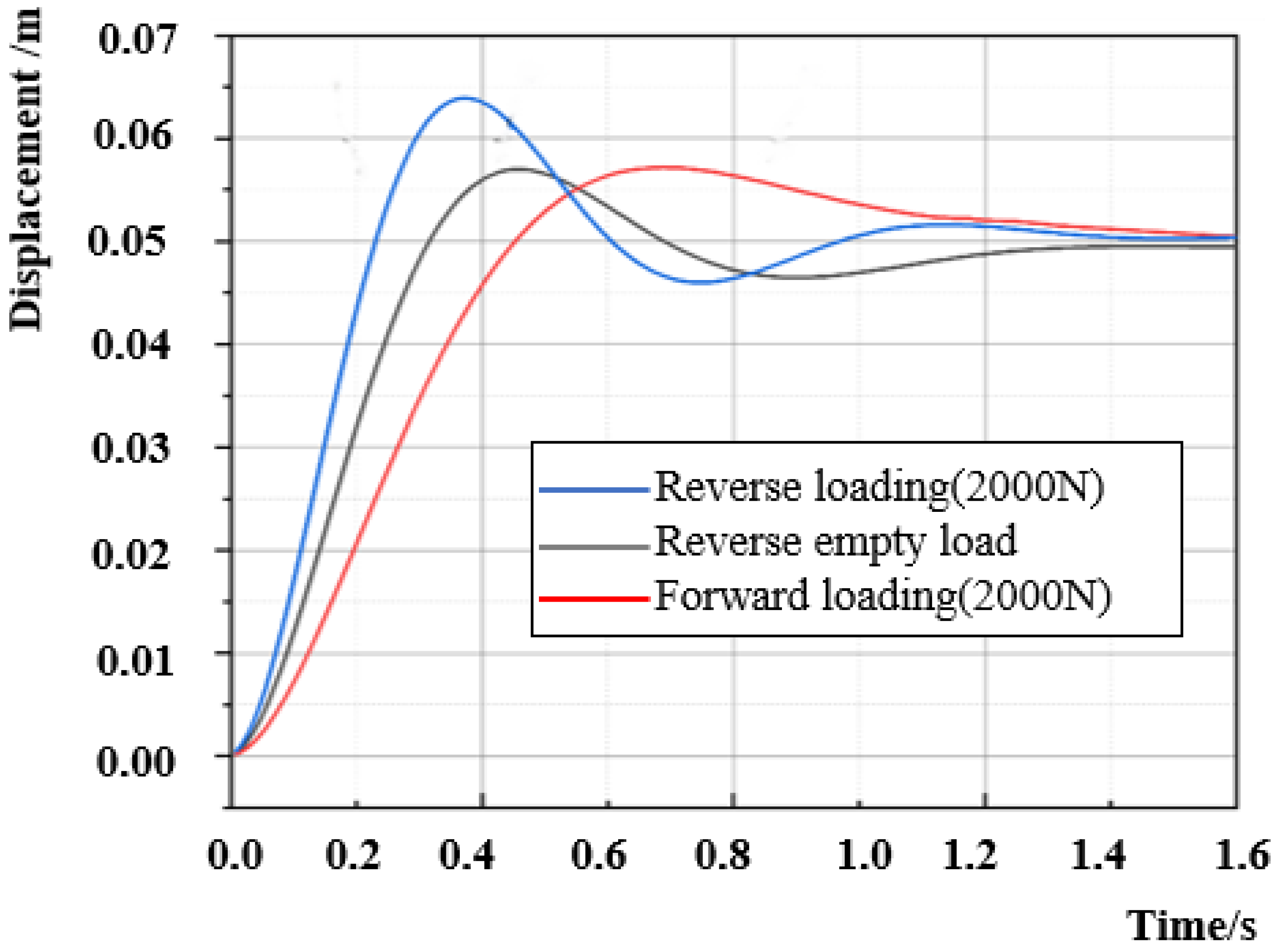
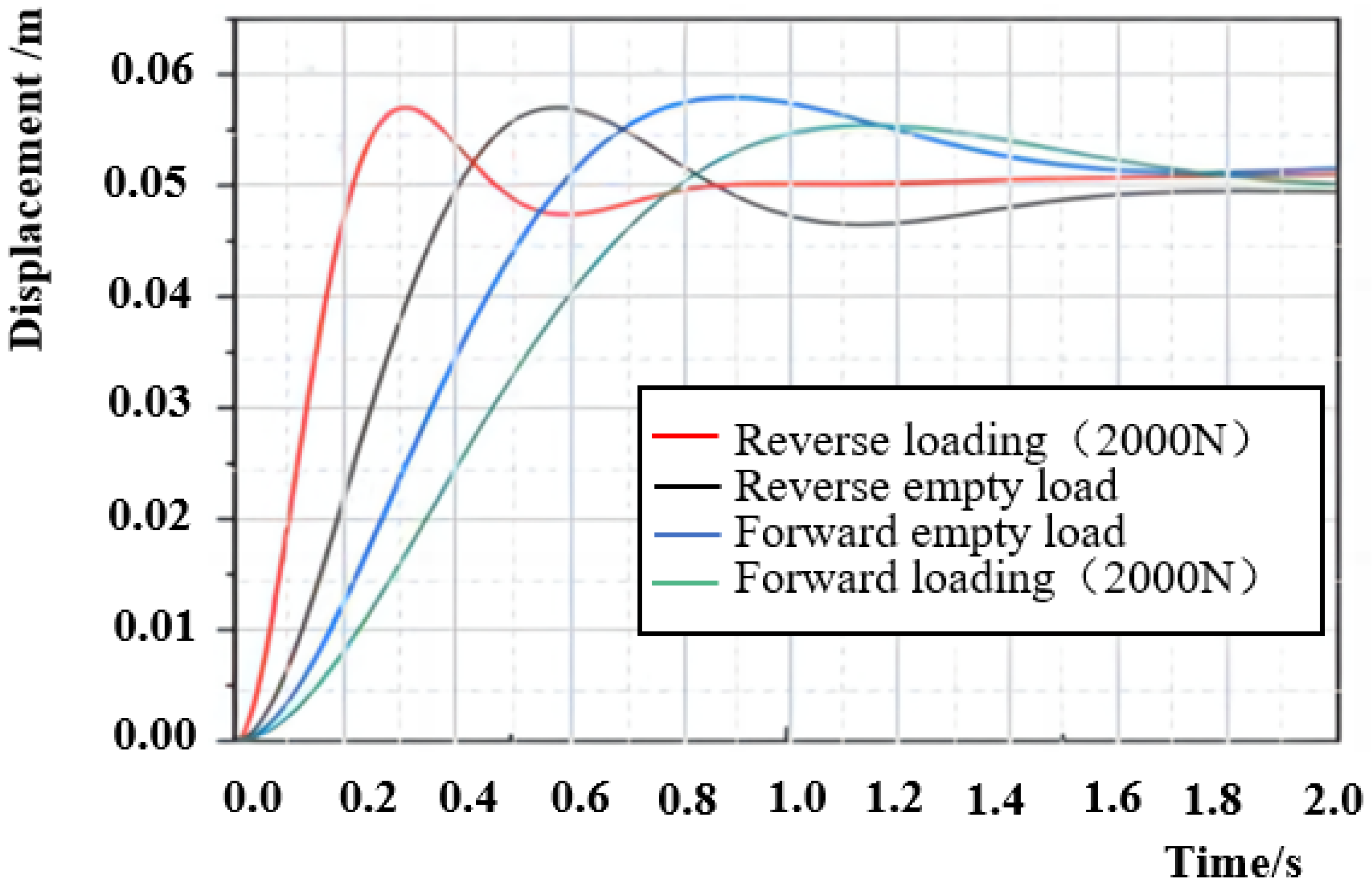
3.3.4. Experimental Results and Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kim, S.W.; Cho, B.; Shin, S.; Oh, J.H.; Hwangbo, J.; Park, H.W. Force Control of a Hydraulic Actuator with a Neural Network Inverse Model. IEEE Robot. Autom. Lett. 2021, 6, 2814–2821. [Google Scholar] [CrossRef]
- Kosek, M.; Downar, D.; Sliwinski, P. Hydraulic valve design methodology for hydro turbine control system. Flow Meas. Instrum. 2024, 100, 102718. [Google Scholar] [CrossRef]
- Zhang, Q.; Song, X.; Song, S.; Stojanovic, V. Finite-Time sliding mode control for singularly perturbed PDE systems. J. Frankl. Inst. 2023, 360, 841–861. [Google Scholar] [CrossRef]
- Coskun, G.; Kolcuoglu, T.; Dogramacı, T.; Turkmen, A.C.; Celik, C.; Soyhan, H.S. Analysis of a priority fow control valve with hydraulic system simulation model. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 1597–1605. [Google Scholar] [CrossRef]
- Djordjevic, V.; Tao, H.; Song, X.; He, S.; Gao, W.; Stojanovic, V. Data-driven control of hydraulic servo actuator: An event-triggered adaptive dynamic programming approach. Math. Biosci. Eng. 2023, 20, 8561–8582. [Google Scholar] [CrossRef]
- Li, X.; Zhu, Z.C.; Rui, G.C.; Cheng, D.; Shen, G.; Tang, Y. Force Loading Tracking Control of an Electro-Hydraulic Actuator Based on a Nonlinear Adaptive Fuzzy Backstepping Control Scheme. Symmetry 2018, 10, 155. [Google Scholar] [CrossRef]
- Fresia, P.; Rundo, M.; Padovani, D.; Altare, G. Combined speed control and centralized power supply for hybrid energy-efficient mobile hydraulics. Autom. Constr. 2022, 140, 104337. [Google Scholar] [CrossRef]
- Schonborn, A.; Chantzidakis, M. Development of a hydraulic control mechanism for cyclic pitch marine current turbines. Renew. Energy 2007, 32, 662–679. [Google Scholar] [CrossRef]
- Lyu, L.; Chen, Z.; Yao, B. Development of Pump and Valves Combined Hydraulic System for Both High Tracking Precision and High Energy Efficiency. IEEE Trans. Ind. Electron. 2019, 66, 7189–7198. [Google Scholar] [CrossRef]
- Zang, W.S.; Zhang, Q.; Su, J.P.; Feng, L. Robust Nonlinear Control Scheme For Electro-Hydraulic Force Tracking Control with Time-Varying Output Constraint. Symmetry 2021, 13, 2074. [Google Scholar] [CrossRef]
- Ko, Y.R.; Kim, T.H. Feedforward Plus Feedback Control of an Electro-Hydraulic Valve System Using a Proportional Control Valve. Actuators 2020, 9, 45. [Google Scholar] [CrossRef]
- Liu, W.; Xu, B.; Yang, H.Y.; Zhao, H.F.; Wu, J.H. Hydraulic operating mechanisms for high voltage circuit breakers Progress evolution and future trends. Sci. China Technol. Sci. 2011, 54, 116–125. [Google Scholar] [CrossRef]
- Zhao, W.S.; Wang, X.; Jiang, Z.; Long, Y.F. A Gain Scheduling Design Method of the Aero-Engine Fuel Servo Constant Pressure Valve with High Accuracy and Fast Response Ability. Symmetry 2023, 15, 45. [Google Scholar] [CrossRef]
- Wei, H.E.; Huang, J.H.; Hao, H.M. Design and Analysis of a Swashplate Control System FOR an Asymmetric Axial Piston Pump. J. Dyn. Syst. Meas. Control 2020, 142, 26–33. [Google Scholar]
- Emelyanov, R.T.; Klimov, A.S.; Kravtsov, K.S.; Olenev, I.B.; Turysheva, E.S. Improving the efficiency of a hydraulic drive with a closed-loop hydraulic circuit. J. Phys. 2020, 1515, 042078. [Google Scholar] [CrossRef]
- Guo, L.; Xu, C.; Yu, T.H.; Wumaier, T. An improved mayfly optimization algorithm based on median position and its application in the optimization of PID parameters of hydro-turbine governor. IEEE Access 2022, 10, 36335–36349. [Google Scholar]
- Kumar, S.; Tewari, V.K.; Bharti, C.K.; Ranjan, A. Modeling, simulation and experimental validation of flow rate of electro-hydraulic hitch control valve of agricultural tractor. Flow Meas. Instrum. 2021, 82, 102070. [Google Scholar] [CrossRef]
- Jensen, K.J.; Ebbesen, M.K.; Hansen, M.R. Novel Concept for Electro-Hydrostatic Actuators for Motion Control of Hydraulic Manipulators. Energies 2021, 14, 6566. [Google Scholar] [CrossRef]
- Zhang, W.; Yuan, Q.H.; Xu, Y.F.; Wang, X.G.; Bai, S.Z.; Zhao, L.; Hua, Y.; Ma, X.X. Research on Control Strategy of Electro-Hydraulic Lifting System Based on AMESim and MATLAB. Symmetry 2023, 15, 435. [Google Scholar] [CrossRef]
- Liu, A.L.; Li, S.L.; Yu, H.L. Research on synchronous control of dual cylinder hydraulic system based on fuzzy theory. Hydraul. Pneum. 2016, 4, 44–47. [Google Scholar]
- Guo, Q.; Yu, T.; Jiang, D. Robust H positional control of 2-DOF robotic arm driven by electro-hydraulic servo system. ISA Trans. 2015, 59, 55–64. [Google Scholar] [CrossRef]
- Wu, W.; Yu, C.Y. Simulation and Experimental Analysis of Hydraulic irectional Control for Displacement Controlled System. IEEE Access 2018, 6, 27993–28000. [Google Scholar] [CrossRef]
- Guo, K.; Wei, J.H.; Fang, J.H. Position tracking control of electro-hydraulic single-rod actuator based on anextended disturbance observer. Mechatronics 2015, 27, 47–56. [Google Scholar] [CrossRef]
- Ding, R.Q.; Zhang, J.H.; Xu, B.; Cheng, M. Programmable hydraulic control technique in construction machinery: Status, challenges and countermeasures. Autom. Constr. 2018, 95, 172–192. [Google Scholar] [CrossRef]
- Liu, M.; Lin, H.; Wang, Y.; Chen, G. Negative imaginariness and positive realness analysis of state-space symmetric systems with interval uncertainty. Proc. Inst. Mech. Eng. 2022, 236, 792–799. [Google Scholar] [CrossRef]
- Kong, X.D.; Cai, B.P.; Liu, Y.H.; Zhu, H.M.; Liu, Y.Q.; Shao, H.D.; Yang, C.; Li, H.J.; Mo, T.Y. Optimal sensor placement methodology of hydraulic control system for fault diagnosis. Mech. Syst. Signal Process. 2022, 174, 109069. [Google Scholar] [CrossRef]
- Deng, W.X.; Yao, J.Y.; Wang, Y.Y.; Yang, X.W.; Chen, J.H. Output feedback backstepping control of hydraulic actuators with valve dynamics compensation. Mech. Syst. Signal Process. 2021, 158, 107769. [Google Scholar] [CrossRef]
- Piller, O.; van Zyl, J.E. Modeling Control Valves in Water Distribution Systems Using a Continuous State Formulation. J. Hydraul. Eng. 2014, 140, 04014052. [Google Scholar] [CrossRef]






| Parameters | Value |
|---|---|
| Type of hydraulic oil | N46 |
| Internal leakage coefficient | 3 × 10−11 m3/sPa |
| Hydraulic oil viscosity, viscosity of oil | 51 |
| Initial volume of the working cylinder | 4.9 × 10−4 m3 |
| The system rated pressure | 6 MPa |
| Maximum system flow | 90 L/min |
| Effective working area of the rodless cavity | 0.0169 m2 |
| Effective working area of the rod cavity | 0.0116 m2 |
| Piston viscous friction coefficient | 80 N/(m/s) |
| Discharge coefficient | 0.61 |
| Proportional valve flow coefficient | 2.8961 m2/s |
| Proportional valve pressure coefficient | 6.66 × 10−12 m3/sPa |
| Effective bulk elastic modulus | 7 × 108 Pa |
| Viscous damping coefficient of the piston and load | 120 |
| Parameters | Symbols and Units | Numerical Value | Parameter | Symbols and Units | Numerical Value |
|---|---|---|---|---|---|
| Hydraulic cylinder volume | 0.00057 | Hydraulic pump pressure | 0–6 (adjustable) | ||
| Rated pressure of the proportional valve | 4 | Rated flow rate of the proportional valve | 14 | ||
| Area of action of the rodless cavity | 0.001963 | Elastic bulk modulus of hydraulic oil | 1. 4 × 103 | ||
| Effective area of the rod cavity | 0.001347 | Load quality | 110 | ||
| Rated current of the proportional valve | 0.4 | Overflow valve pressure | 0–6 (adjustable) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wen, Y.; Teng, S.; Li, Q.; Tan, J.; Song, Y.; Sun, S. Investigating the Symmetric Control of a Hydraulic System Based on Status Feedback. Symmetry 2025, 17, 246. https://doi.org/10.3390/sym17020246
Wen Y, Teng S, Li Q, Tan J, Song Y, Sun S. Investigating the Symmetric Control of a Hydraulic System Based on Status Feedback. Symmetry. 2025; 17(2):246. https://doi.org/10.3390/sym17020246
Chicago/Turabian StyleWen, Yuebing, Shuhua Teng, Qiang Li, Jianping Tan, Yuwei Song, and Shiyuan Sun. 2025. "Investigating the Symmetric Control of a Hydraulic System Based on Status Feedback" Symmetry 17, no. 2: 246. https://doi.org/10.3390/sym17020246
APA StyleWen, Y., Teng, S., Li, Q., Tan, J., Song, Y., & Sun, S. (2025). Investigating the Symmetric Control of a Hydraulic System Based on Status Feedback. Symmetry, 17(2), 246. https://doi.org/10.3390/sym17020246






