The Effect of Repetitive Mechanical Perturbations on Lower Limb Symmetry in Postural Control
Abstract
1. Introduction
2. Materials and Methods
2.1. Participants
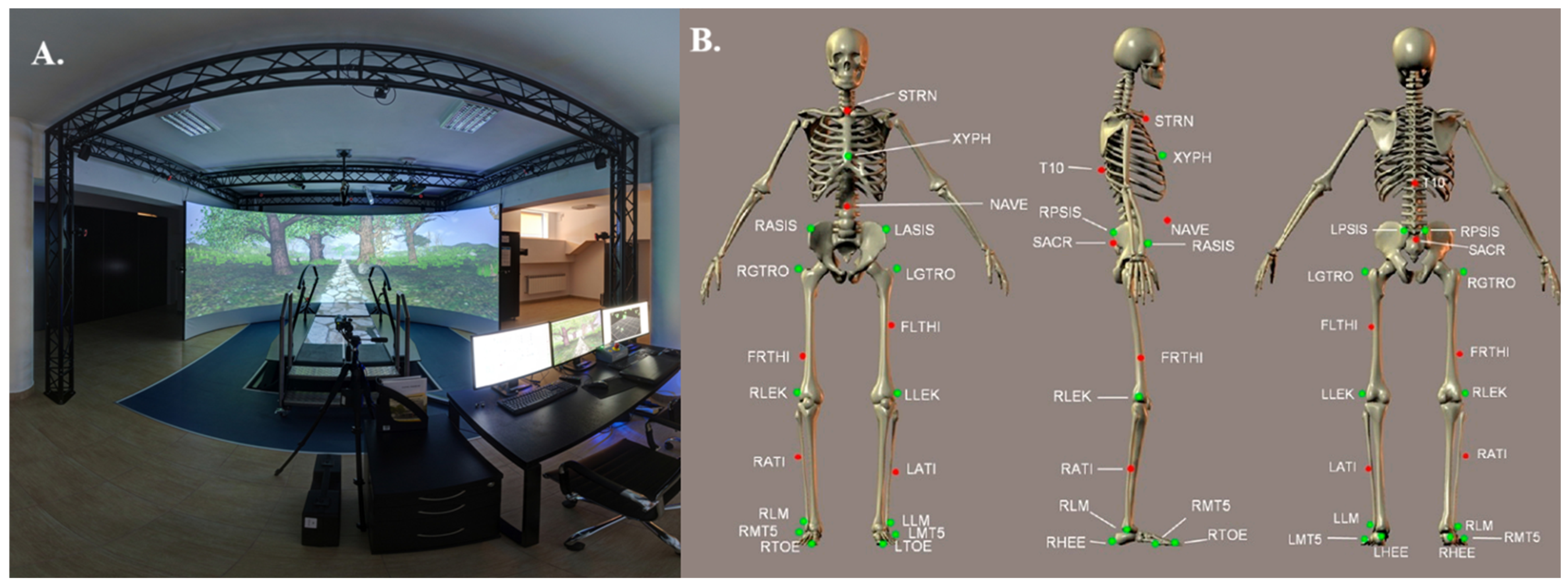
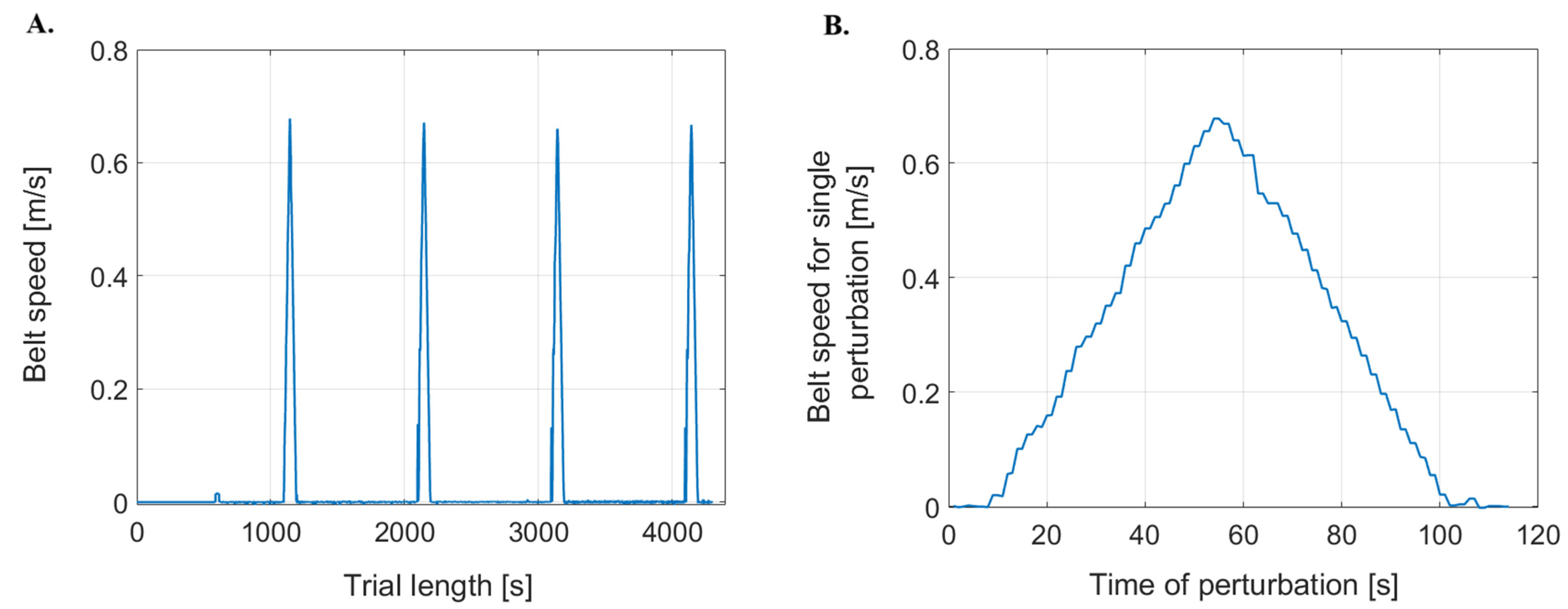
2.2. Measurement Protocol
2.3. Statistical Analysis
3. Results
3.1. Comparison of Kinematic and Kinetic Parameters for Perturbed Dominant and Non-Dominant Lower Limbs
3.2. Comparison of Kinematic and Kinetic Parameters of the Perturbed and Responding Limb
3.2.1. Perturbation of the Non-Dominant (Left) Limb
3.2.2. Perturbation of the Dominant (Right) Limb
4. Discussion
4.1. Limb Dominance and Postural Control
4.2. Joint-Specific Responses
4.3. Implications for Rehabilitation
4.4. Study Limitations and Future Directions
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kędziorek, J.; Błażkiewicz, M. Nonlinear Measures to Evaluate Upright Postural Stability: A Systematic Review. Entropy 2020, 22, 1357. [Google Scholar] [CrossRef] [PubMed]
- Kašček Bučinel, A.; Supej, M.; Petrone, N.; Čuk, I. How does body symmetry influence standing balance? Kinesiology 2019, 51, 52–59. [Google Scholar] [CrossRef]
- Kumar, S.N.; Omar, B.; Joseph, L.H.; Htwe, O.; Jagannathan, K.; Hamdan, N.M.; Rajalakshmi, D. Evaluation of limb load asymmetry using two new mathematical models. Glob. J. Health Sci. 2014, 7, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Blanchet, M.; Guertin, P.; Pilon, F.; Gorce, P.; Prince, F. From Neural Command to Robotic Use: The Role of Symmetry/Asymmetry in Postural and Locomotor Activities. Symmetry 2021, 13, 1773. [Google Scholar] [CrossRef]
- Ogihara, H.; Tsushima, E.; Kamo, T.; Sato, T.; Matsushima, A.; Niioka, Y.; Asahi, R.; Azami, M. Kinematic gait asymmetry assessment using joint angle data in patients with chronic stroke-A normalized cross-correlation approach. Gait Posture 2020, 80, 168–173. [Google Scholar] [CrossRef]
- Keogh, J.A.J.; Waddington, E.E.; Masood, Z.; Mahmood, S.; Palanisamy, A.C.; Ruder, M.C.; Karsan, S.; Bishop, C.; Jordan, M.J.; Heisz, J.J.; et al. Monitoring lower limb biomechanical asymmetry and psychological measures in athletic populations-A scoping review. Scand. J. Med. Sci. Sports 2023, 33, 2125–2148. [Google Scholar] [CrossRef]
- Ciunelis, K.; Borkowski, R.; Błażkiewicz, M. The Impact of Induced Acceleration Perturbations in Selected Phases of the Gait Cycle on Kinematic and Kinetic Parameters. Appl. Sci. 2024, 14, 4849. [Google Scholar] [CrossRef]
- Błażkiewicz, M.; Hadamus, A. Effect of wearing high-heeled shoes on postural control and foot loading symmetry. Acta Bioeng. Biomech. 2023, 25, 49–57. [Google Scholar] [CrossRef]
- Błażkiewicz, M.; Hadamus, A.; Borkowski, R. Recurrence Quantification Analysis as a Form of Postural Control Assessment: A Systematic Review. Appl. Sci. 2023, 13, 5587. [Google Scholar] [CrossRef]
- Kadri, M.A.; Noé, F.; Maitre, J.; Maffulli, N.; Paillard, T. Effects of Limb Dominance on Postural Balance in Sportsmen Practicing Symmetric and Asymmetric Sports: A Pilot Study. Symmetry 2021, 13, 2199. [Google Scholar] [CrossRef]
- Arsenault, D.; Ivanova, T.D.; Garland, S.J. Postural control in response to unilateral and bilateral external perturbations in older adults. Gait Posture 2022, 94, 26–31. [Google Scholar] [CrossRef] [PubMed]
- Prado-Rico, J.M.; Duarte, M. Asymmetry of Body Weight Distribution During Quiet and Relaxed Standing Tasks. Mot. Control 2019, 23, 553–565. [Google Scholar] [CrossRef]
- Nolff, M.R.; Conner, N.O.; Haworth, J.L.; Goble, D.J. Lower Limb Asymmetry Evaluation Using the Balance Tracking System (BTrackS) Single Leg Stance Protocol. J. Mot. Behav. 2023, 55, 493–498. [Google Scholar] [CrossRef] [PubMed]
- Hadamus, A.; Gulatowska, M.; Ferenc, A.; Shahnazaryan, K.; Brzuszkiewicz-Kuźmicka, G.; Błażkiewicz, M. Influence of leg dominance on the symmetry in body balance measurements. Phys. Act. Rev. 2025, 13, 88–96. [Google Scholar] [CrossRef]
- Błażkiewicz, M.; Gulatowska, M.; Hadamus, A.; Kędziorek, J.; Brzuszkiewicz-Kuźmicka, G. Effect of Annoying Sounds on Postural Control. J. Clin. Med. 2024, 13, 2638. [Google Scholar] [CrossRef]
- Zhu, R.T.; Lyu, P.-Z.; Li, S.; Tong, C.Y.; Ling, Y.T.; Ma, C.Z. How Does Lower Limb Respond to Unexpected Balance Perturbations? New Insights from Synchronized Human Kinetics, Kinematics, Muscle Electromyography (EMG) and Mechanomyography (MMG) Data. Biosensors 2022, 12, 430. [Google Scholar] [CrossRef]
- Promsri, A.; Haid, T.; Federolf, P. How does lower limb dominance influence postural control movements during single leg stance? Hum. Mov. Sci. 2018, 58, 165–174. [Google Scholar] [CrossRef]
- Barut, C.; Ozer, C.M.; Sevinc, O.; Gumus, M.; Yunten, Z. Relationships between hand and foot preferences. Int. J. Neurosci. 2007, 117, 177–185. [Google Scholar] [CrossRef]
- Feldhege, F.; Richter, K.; Bruhn, S.; Fischer, D.C.; Mittlmeier, T. MATLAB-based tools for automated processing of motion tracking data provided by the GRAIL. Gait Posture 2021, 90, 422–426. [Google Scholar] [CrossRef]
- Blazkiewicz, M.; Wiszomirska, I.; Wit, A. Comparison of four methods of calculating the symmetry of spatial-temporal parameters of gait. Acta Bioeng. Biomech. 2014, 16, 29–35. [Google Scholar]
- Rosenthal, R. Parametric measures of effect size. In The Handbook of Research Synthesis; Russell Sage Foundation: New York, NY, USA, 1994; pp. 231–244. [Google Scholar]
- Rosnow, R.L. Effect sizes for experimenting psychologists. Can. J. Exp. Psychol. 2003, 57, 221–237. [Google Scholar] [CrossRef] [PubMed]
- Prajzner, A. Selected Indicators of Effect Size in Psychological Research. Ann. Univ. Mariae Curie-Skłodowska Sect. J. Paedagog.-Psychol. 2023, 35, 139–157. [Google Scholar] [CrossRef]
- Gribble, P.A.; Hertel, J.; Plisky, P. Using the Star Excursion Balance Test to assess dynamic postural-control deficits and outcomes in lower extremity injury: A literature and systematic review. J. Athl. Train. 2012, 47, 339–357. [Google Scholar] [CrossRef]
- Promsri, A.; Bangkomdet, K.; Jindatham, I.; Jenchang, T. Leg Dominance—Surface Stability Interaction: Effects on Postural Control Assessed by Smartphone-Based Accelerometry. Sports 2023, 11, 75. [Google Scholar] [CrossRef]
- Promsri, A.; Haid, T.; Werner, I.; Federolf, P. Leg Dominance Effects on Postural Control When Performing Challenging Balance Exercises. Brain Sci. 2020, 10, 128. [Google Scholar] [CrossRef]
- Promsri, A.; Longo, A.; Haid, T.; Doix, A.M.; Federolf, P. Leg Dominance as a Risk Factor for Lower-Limb Injuries in Downhill Skiers-A Pilot Study into Possible Mechanisms. Int. J. Environ. Res. Public Health 2019, 16, 3399. [Google Scholar] [CrossRef]
- Blazkiewicz, M.; Kedziorek, J.; Hadamus, A. The Impact of Visual Input and Support Area Manipulation on Postural Control in Subjects after Osteoporotic Vertebral Fracture. Entropy 2021, 23, 375. [Google Scholar] [CrossRef]
- Błażkiewicz, M.; Lann Vel Lace, K.; Hadamus, A. Gait Symmetry Analysis Based on Dynamic Time Warping. Symmetry 2021, 13, 836. [Google Scholar] [CrossRef]
- Kędziorek, J.; Błażkiewicz, M. The impact of external perturbations on postural control. Acta Bioeng. Biomech. 2024, 26, 3–11. [Google Scholar] [CrossRef]
- Macmillan, C.; Olivier, B.; Benjamin-Damons, N.; Wood, W.A.; Obiora, O.L. Altered sagittal plane mechanics is associated with Functional Movement Screen deep squat score. S. Afr. J. Physiother. 2023, 79, a1865. [Google Scholar] [CrossRef]
- Escamilla-Nunez, R.; Sivasambu, H.; Andrysek, J. Exploration of Vibrotactile Biofeedback Strategies to Induce Stance Time Asymmetries. Can. Prosthet. Orthot. J. 2022, 5, 36744. [Google Scholar] [CrossRef] [PubMed]
- Matla, J.; Filar-Mierzwa, K.; Ścisłowska-Czarnecka, A.; Jankowicz-Szymańska, A.; Bac, A. The Influence of the Physiotherapeutic Program on Selected Static and Dynamic Foot Indicators and the Balance of Elderly Women Depending on the Ground Stability. Int. J. Environ. Res. Public Health 2021, 18, 4660. [Google Scholar] [CrossRef] [PubMed]
- Kim, H.; Kim, H.; Shin, W.S. Effects of Vibrotactile Biofeedback Providing Real-Time Pressure Information on Static Balance Ability and Weight Distribution Symmetry Index in Patients with Chronic Stroke. Brain Sci. 2022, 12, 358. [Google Scholar] [CrossRef] [PubMed]
- Queen, R.M.; Peebles, A.T.; Miller, T.K.; Savla, J.; Ollendick, T.; Messier, S.P.; Williams, D.B., 3rd. Reduction of Risk Factors for ACL Re-injuries using an Innovative Biofeedback Approach: Rationale and Design. Contemp. Clin. Trials Commun. 2021, 22, 100769. [Google Scholar] [CrossRef]
- Nawasreh, Z.; Logerstedt, D.; Failla, M.; Snyder-Mackler, L. No difference between mechanical perturbation training with compliant surface and manual perturbation training on knee functional performance after ACL rupture. J. Orthop. Res. Off. Publ. Orthop. Res. Soc. 2018, 36, 1391–1397. [Google Scholar] [CrossRef]
- Choi, J.M.; Cho, E.Y.; Lee, B.H. Effects of Dynamic Stretching Combined with Manual Therapy on Pain, ROM, Function, and Quality of Life of Adhesive Capsulitis. Healthcare 2023, 12, 45. [Google Scholar] [CrossRef]


| Parameter | Perturbed—Non-Dominant (Left) Lower Limb | Perturbed—Dominant (Right) Lower Limb | SI | p-Value/Effect Size |
|---|---|---|---|---|
| Angle values [deg] | ||||
| Hip | 36.26 (32.24; 42.98) | 30.75 (27.72; 34.98) | 0.15 (0.05; 0.25) | 0.0016 */0.77 |
| Knee | 70.17 (66.09; 76.15) | 61.68 (59.83; 66.81) | 0.08 (0.07; 0.18) | 0.0001 */0.85 |
| Ankle | 72.48 (65.82; 78.16) | 72.29 (66.79; 74.62) | 0.03 (−0.04; 0.09) | 0.2637/0.34 |
| Torque values [Nm/kg] | ||||
| Hip | 0.88 (0.68; 1.09) | 0.82 (0.63; 1.12) | 0.03 (−0.17; 0.38) | 0.4840/0.11 |
| Knee | 0.37 (0.27; 0.44) | 0.30 (0.25; 0.38) | 0.19 (−0.09; 0.44) | 0.0224 */0.33 |
| Ankle | 1.02 (0.89; 1.24) | 0.88 (0.77; 0.97) | 0.23 (−0.01; 0.32) | 0.0005 */0.56 |
| GRF [N/kg] | ||||
| ML | 1.02 (0.90; 1.39) | 0.05 (0.03; 0.16) | 1.84 (1.50; 1.90) | 0.0001 */0.87 |
| AP | 0.79 (0.54; 1.33) | 0.72 (0.49; 0.99) | 0.14 (−0.17; 0.40) | 0.0755/0.24 |
| PD | 9.96 (8.69; 10.71) | 9.57 (8.64; 10.62) | 0.04 (−0.06; 0.13) | 0.5601/0.32 |
| Parameter | Perturbed—Non-Dominant (Left) Lower Limb | Reactive—Dominant (Right) Lower Limb | SI | p-Value/Effect Size |
|---|---|---|---|---|
| Angle values [deg] | ||||
| Hip | 36.25 (32.24; 42.98) | 29.16 (20.28; 34.73) | 0.24 (0.17; 0.42) | 0.0001 */0.87 |
| Knee | 70.17 (66.09; 76.15) | 30.73 (21.82; 41.40) | 0.74 (0.57; 1.25) | 0.0001 */0.87 |
| Ankle | 72.48 (65.60; 78.16) | 95.60 (91.15; 100.54) | −0.28 (−0.38; −0.22) | 0.0001 */0.87 |
| Torque values [Nm/kg] | ||||
| Hip | 0.88 (0.68; 1.10) | 1.05 (0.93; 1.32) | −0.26 (−0.38; 0.00) | 0.0001 */0.65 |
| Knee | 0.37 (0.27; 0.44) | 0.09 (0.04; 0.14) | 1.23 (0.94; 1.56) | 0.0001 */0.87 |
| Ankle | 1.02 (0.89; 1.12) | 0.40 (0.31; 0.51) | 0.87 (0.75; 1.04) | 0.0001 */0.87 |
| GRF [N/kg] | ||||
| ML | 1.02 (0.90; 1.39) | 0.18 (0.13; 0.33;) | 1.49 (0.91; 1.57) | 0.0001 */0.87 |
| AP | 0.79 (0.54; 1.33) | 0.65 (0.52; 1.13;) | 0.05 (−0.10; 0.52) | 0.0679/0.87 |
| PD | 9.96 (8.68; 10.71) | 11.74 (11.15; 12.21;) | −0.20 (−0.25; −0.10) | 0.0001 */0.24 |
| Parameter | Reactive—Non-Dominant (Left) Lower Limb | Perturbed Dominant (Right) Lower Limb | SI | p-Value/Effect Size |
|---|---|---|---|---|
| Angle values [deg] | ||||
| Hip | 25.83 (22.18; 34.25) | 30.75 (27.72; 34.98) | −0.13 (−0.22; −0.02) | 0.0004 */0.50 |
| Knee | 25.96 (20.73; 33.36) | 61.86 (59.83; 66.81) | −0.83 (−0.99; −0.65) | 0.0001 */0.87 |
| Ankle | 95.66 (93.74; 98.13) | 72.29 (66.79; 74.62) | 0.27 (0.26; 0.33) | 0.0001 */0.87 |
| Torque values [Nm/kg] | ||||
| Hip | 0.92 (0.84; 1.07) | 0.82 (0.63; 1.12) | 0.11 (−0.03; 0.28) | 0.0178 */0.35 |
| Knee | 0.00 (0.07; 0.08) | 0.30 (0.25; 0.38) | −2.00 (−3.30; −1.34) | 0.0001 */0.87 |
| Ankle | 0.60 (0.43; 0.75) | 0.88 (0.77; 0.97) | −0.32 (−0.75; −0.03) | 0.0001 */0.54 |
| GRF [N/kg] | ||||
| ML | 1.10 (1.00; 1.44) | 0.05 (0.03; 0.16) | 1.80 (1.62; 1.89) | 0.0001 */0.87 |
| AP | 0.64 (0.49; 0.82) | 0.72 (0.49; 0.99) | −0.01 (−0.45; 0.33) | 0.4003/0.16 |
| PD | 11.37 (10.81; 12.48) | 9.57 (8.64; 10.62) | 0.23 (0.11; 0.30) | 0.0001 */0.86 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gulatowska, M.; Błażkiewicz, M. The Effect of Repetitive Mechanical Perturbations on Lower Limb Symmetry in Postural Control. Symmetry 2025, 17, 245. https://doi.org/10.3390/sym17020245
Gulatowska M, Błażkiewicz M. The Effect of Repetitive Mechanical Perturbations on Lower Limb Symmetry in Postural Control. Symmetry. 2025; 17(2):245. https://doi.org/10.3390/sym17020245
Chicago/Turabian StyleGulatowska, Michalina, and Michalina Błażkiewicz. 2025. "The Effect of Repetitive Mechanical Perturbations on Lower Limb Symmetry in Postural Control" Symmetry 17, no. 2: 245. https://doi.org/10.3390/sym17020245
APA StyleGulatowska, M., & Błażkiewicz, M. (2025). The Effect of Repetitive Mechanical Perturbations on Lower Limb Symmetry in Postural Control. Symmetry, 17(2), 245. https://doi.org/10.3390/sym17020245








