A Brief Survey on the Riemann Hypothesis and Some Attempts to Prove It

Abstract

This paper presents a brief survey on the Riemann Hypothesis, a central conjecture in number theory with profound implications, and describes various recent attempts aimed at proving it.

1. Introduction

The Riemann zeta function is usually defined as the Dirichlet series

$$\zeta \left(s\right):={\displaystyle \sum _{n=1}^{\infty }}{\displaystyle \frac{1}{n^s}},s=\sigma +it,$$

(1)

[1] (Ch. 25), [2] (Ch. I, sec. 1.1, (1.1.1), p. 1), [3] (Ch.1, sec. 1.2, (1), p. 6), which converges for all $s\in \mathbf{C}$ such that $\sigma =$ Re$\left\{s\right\}>1$, and as its meromorphic continuation in the complex plane, where it has only a simple pole at $s=1$. It can also be defined by the Euler product formula,

$$\zeta \left(s\right)=\prod _p{\left(1-{\displaystyle \frac{1}{p^s}}\right)}^{-1},\mathrm{Res}>1,$$

where p runs through all primes, $p=2,3,5,7,\dots$, [2] (Ch. I, sec. 1.1, (1.1.2), p. 1), [3] (sec. 2.1, (1), p. 6).

It is known that $\zeta \left(s\right)$ vanishes at the infinitely many negative even integers $s_n:=-2n$, $n\in \mathbf{N}$, called the trivial zeros, as well as at infinitely (countably) many zeros, called nontrivial zeros, located on the “critical line”, Re$\left\{s\right\}=1/2$, a result due to Hardy [3] (Ch. 11, sec. 11.1, p. 226)) [4]. It is not known to date whether other nontrivial zeros exist in $\mathbf{C}$, which should necessarily belong to the “critical strip”, $\Sigma :=\left\{\right(\sigma ,t)\in \mathbf{C}:0<\sigma <1,-\infty <t<+\infty \}$. That no such zeros exist is what is called the Riemann Hypothesis (RH, for short).

The importance of the RH rests on its relation with the distribution of primes [3] (Sec. 1.11, p. 22), [5], but also on many equivalent formulations, some quite technical. For some extensive lists, see [6,7,8], for example. There is, in addition, a large number of results whose validity would immediately follow from the validity of the RH.

Indeed, the RH implies, for instance, some strong bound on the growth of the primes counting function, namely sharp estimates on the remainder term in the formulation of the prime number theorem, for which, in 1899, de la Vallée Poussin [9] proved the form

$$\pi \left(x\right)=\mathrm{Li}\left(x\right)+\mathcal{O}\left({xe}^{-a\sqrt{logx}}\right),\mathrm{as}x\to +\infty ,$$

(2)

for some positive constant a, where Li$\left(x\right):=\mathrm{li}\left(x\right)-\mathrm{li}\left(2\right)={\int }_2^x{\displaystyle \frac{dt}{logt}}$ is the logarithmic integral. The error term has been ameliorated in the following years. However, it is interesting that some refinements hold assuming the validity of the RH: in 1901, it was shown by Helge von Koch [10] that the RH is equivalent to the more precise estimate

$$\pi \left(x\right)=\mathrm{Li}\left(x\right)+\mathcal{O}\left(\sqrt{x}logx\right).$$

(3)

A bound for the constant implied by the $\mathcal{O}$ symbol was obtained much later by Schoenfeld [11], but again under the validity of the RH.

Many results have been established over the time, the most pertaining to pure mathematics, under the condition that the RH is true. As usual, in coping with difficult unsolved mathematical problems, several collateral results are obtained. Establishing a number of new results may be even more important than achieving the final goal of proving the RH.

To study the zeros of the zeta function, the so-called (Riemann’s) xi function,

(4)

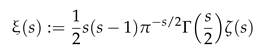
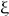[2] (Ch. II, (2.1.12), p. 16) has also been widely used. For instance, Hardy proved that $\left(s\right)$ has infinitely (countably) many zeros on the critical line Re$\left\{s\right\}=1/2$ [3] (Ch. 11, sec. 11.1, p. 226)) [4].

Numerous properties of general results about the zeta function can be found in a number of books; see [2,3,12], e.g., Several surveys, recording advances and possible paths to prove the RH have appeared regularly in the literature over the time; see, e.g., [12,13,14,15,16]. In particular, it should be emphasized that Titchmarsh’s work, dating back to 1951 [2], is mostly useful for researchers who wish to conduct research on the RH. N. Kurokawa [17], in 2000, provided a significant body of knowledge on the RH for allied zeta-functions, but this work remains relatively unknown, partly due to its being written in Japanese. This list is not intended to be exhaustive.

The literature concerning the RH is vast and constantly growing, with several significant contributions appearing in qualified periodicals in the first half of 2024 alone. Fortunately, unlike in the case of Fermat’s Last Theorem, where any amateur, regardless of his or her incompetence or lack of mathematical background, could naively attempt to tackle the problem, addressing the RH requires a solid understanding of basic mathematics, specifically, knowledge that goes beyond that of a typical sophomore.

It is claimed that some implications of the RH exist also for other fields, such as cryptography and physics. The difficult task of factoring large composite numbers, crucial for some encryption systems, could potentially benefit from a deeper description of prime number distribution. Yet, it is believed that the truth or falsity of the RH has no relevance for the development of factorization methods.

The zeta function and the distribution of primes play also a role in various models met in different branches of physics, such as classical mechanics, statistical physics, and quantum field theory (in particular, in string theory). These relations might even suggest a path towards proving the RH. Mathematics is traditionally seen as supplying the theoretical tools with which physical theories are analyzed. Here this relationship might be reversed, with quantum physics potentially offering new insights into number theory. One proposed strategy for proving the RH is to exploit the Hilbert–Pólya conjecture (see Section 2.2). This requires determining a self-adjoint operator on a Hilbert space, whose eigenvalues would be the ordinates of the zeros of the zeta function. Since this operator is self-adjoint, its eigenvalues must be real. In quantum mechanics, systems are governed by self-adjoint operators, that is, their Hamiltonians. This analogy suggests that perhaps a quantum mechanical system could be found, whose energy levels correspond to the zeros of the zeta function.

The following set of attempts to prove the RH is far from being exhaustive. Typically, they are (recent or less recent) failures.

2. About Some Attempts to Prove the RH

Many attempts aiming either to prove or to disprove the correctness of the RH have been made since the 1859 work of Bernhard Riemann [18], resorting to a variety of methods, some of which somewhat sophisticated.

2.1. Zero-Free Regions

The interesting idea of identifying “zero-free” regions inside the critical strip should be mentioned. Over the years, there has been some interest in determining regions of the shape $\sigma \ge 1-1/f\left(\right|t\left|\right)$, for some function $f\left(t\right)$ tending to infinity as $t\to \pm \infty$, since this behavior has some relation to the distribution of the prime numbers.

The first of such regions was constructed in 1899 by de la Vallée–Poussin [3] (sec. 5.2, p. 79) [19], but one of the best results has been considered for long time that achieved by Vinogradov and Korobov (see the review paper [19] for references and other cases). For this reason, sometimes these regions are called “Korobov–Vinogradov regions.”

In 2015, Mossinghoff and Trudgian have obtained the largest known zero-free region [20], then further improved in [21]. In [20] the authors showed that no zeros exist in the domain $\sigma \ge 1-1/(R_{0}logt)$, where $R_0=5.573412$, which result improved the previously best-known value $R_0=5.68371$, obtained by Jang and Kwon in 2014 [22].

Very recently, in [21] Mossinghoff, Trudgian, and Yang obtained ameliorated explicit zero-free regions. For example, no zeros of the zeta function exist in the Korobov–Vinogradov region

$$\sigma \ge 1-{\displaystyle \frac{1}{55.241{(log|t\left|\right)}^{2/3}{(loglog|t\left|\right)}^{1/3}}}$$

(5)

for $\left|t\right|\ge 3$, and in the classical zero-free region

$$\sigma \ge 1-{\displaystyle \frac{1}{5.558691log\left|t\right|}}$$

(6)

for $\left|t\right|\ge 2$. In addition, the authors also obtained the intermediate zero-free region

$$\sigma >1-{\displaystyle \frac{0.05035}{h\left(t\right)}}+{\displaystyle \frac{0.0349}{h^2\left(t\right)}}$$

(7)

for $\left|t\right|\ge e^{1000}$, where $h\left(t\right)={\displaystyle \frac{27}{164}}log\left|t\right|+7.096$.

Earlier, in 2006, Freitas [23] established a Li-type criterion (see Section 2.3) to obtain a necessary and sufficient condition for the existence of zero-free strips, contained inside the critical strip, $0<$ Re$\left\{s\right\}<1$. A numerical rigorous result due to Platt and Trudgian belongs to this kind of approach, but this will be reported later, in more detail.

2.2. The Hilbert–Pólya Conjecture

Among the most intriguing ideas proposed to prove the RH, one should perhaps recall the Hilbert–Pólya conjecture, according to which the RH is true because non-trivial zeros of the zeta function correspond (in a certain canonical way) to the eigenvalues of some positive operator. This conjecture has been often regarded as a most promising approach. However, very little is known about its origins; some people attributed its formulation to Hilbert and Pólya, independently, some time in the 1910s.

Andrew Odlyzko had a correspondence with George Pólya between 1981–1982 [24,25], where he asked for a reference, time, and reasoning about this issue. George Pólya replied that he had spent two years in Göttingen, ending around the beginning of 1914, when he tried to learn analytic number theory from Landau. The latter asked him one day: “You know some physics. Do you know a physical reason that the Riemann hypothesis should be true”. This would be the case, he answered, if the nontrivial zeros of the Xi-function were so connected with the physical problem that the Riemann hypothesis would be equivalent to the fact that all the eigenvalues of the physical problem are real. Pólya added that he never published this remark. Incidentally, the aforementioned Xi-function is defined as $\Xi \left(z\right)=$$\left({\displaystyle \frac{1}{2}}+zi\right)$.

That is it. It may be too little, too vague, to base some investigations on this, but several researchers, mostly physicists, took it seriously, even though still unsuccessfully.

There are also some theorems by Pólya’s [26,27], [3] (Ch. 12, sec. 12.5, p. 270), that perhaps could be related to the aforementioned plan.

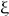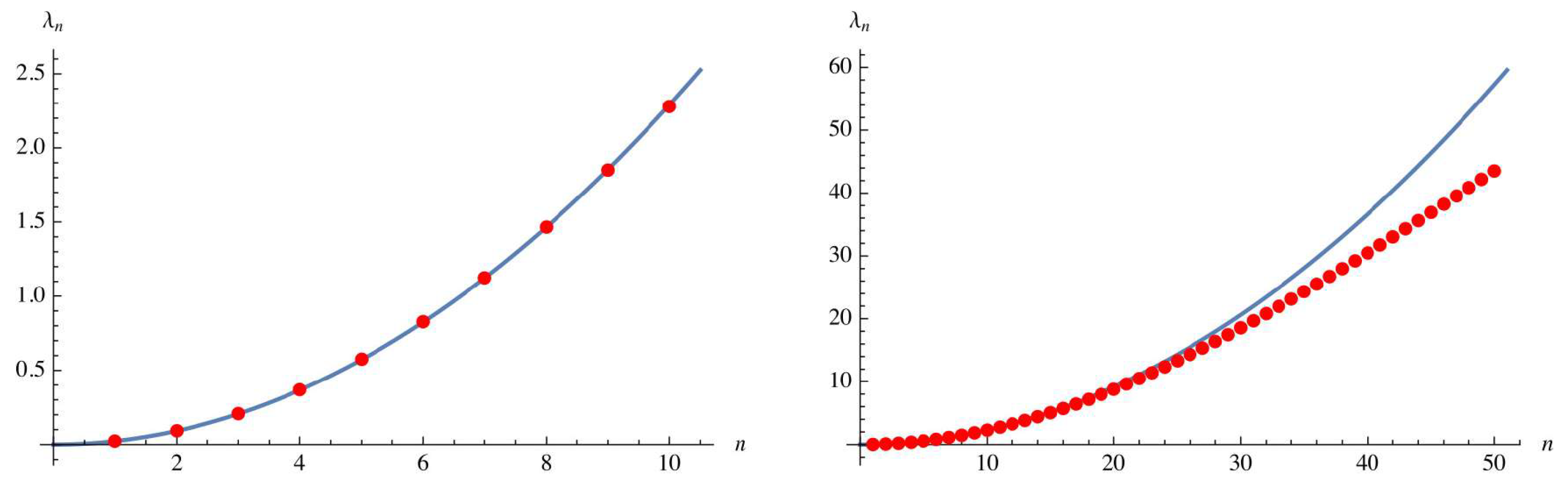
2.3. The Keiper–Li’s Criterion

The Li’s, or better, Keiper–Li’s criterion, concerns the positivity of a certain numerical sequence [28,29,30,31,32]. In 1997, Xian-Jin Li established a necessary and sufficient condition for the validity of the RH, since then known as the “Li’s criterion” [32]; see also [33]. This has the form of a set of inequalities, namely,

$${\lambda }_n:=\sum _{\rho }\left[1-{\left(1-{\displaystyle \frac{1}{\rho }}\right)}^n\right]\ge 0\mathrm{for}n=1,2,\dots ,$$

(8)

the sum being extended to all nontrivial zeros, $\rho$, of the Riemann zeta function, and understood as in (13) below (Section 3). In [28], the conditions in (8) are formulated with the ${\lambda }_n$’s strictly positive.

In some sense, there are two ways to evaluate the ${\lambda }_n$’s. One is to look at their definition,

(9)

which comes from the representation

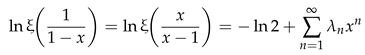
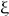
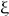of the xi function (4) [33] (sec. 4, eq. (20), p. 767) [34] (sec. 4.2). Here, the symmetry relation $\left(s\right)=$$(1-s)$ has been used. Other equivalent formulations for the ${\lambda }_n$’s exist; see, e.g., [35], and also [30,36,37]. In several papers [30,35,36], M. Coffey derived an alternative representation of Li’s ${\lambda }_n$ coefficients and then used it to tabulate the first 25 values of ${\lambda }_n$ (for $n=0,\dots ,25$) in [35], and 101 values (for $n=0,\dots ,100$) in [36]. These alternative coefficients involve other well-known constants, such as the Stieltjes constants and Bernoulli numbers.

In Figure 1, the behavior of ${\lambda }_n$ is shown as a function of n, for n up to about 50. Up to $n\approx 10$, these values are very well fit by a parabola with ${\lambda }_n\sim 0.023\times n^2$, then a departure from this behavior is clear [38].

The first 3300 Keiper–Li coefficients were evaluated by K. Maślanka in [39]. A computation for up to n = 100,000, made in [34], shows an agreement on the first two decimals with a certain “Keiper’s conjecture” (hence, with the RH), providing

$${\lambda }_{100,000}=4.62580782406902231409416038...$$

Keiper’s asymptotic approximation yields in fact ${\lambda }_{100,000}\approx 4.626132$ [40] (pp. 63–65).

Another way to evaluate the ${\lambda }_n$’s is using the successively obtained Formula (8); see [32]. Using the latter, however, the validity or not of the RH cannot be established since it involves all nontrivial zeros, without knowing whether any of them exist or not off the critical line. In [41], Voros established an alternative asymptotic behavior, basing on (8).

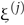In order to prove (or disprove) the RH via Li’s criterion, one should instead use (9), e.g., which does not involve explicitly the nontrivial zeros themselves. Trying to make explicit the Li’s representation of the parameters ${\lambda }_n$, one could use the integral representation of $\left(1\right)$, given in [35] (eq. (9), p. 527), and consider resorting to the celebrated Faa’ di Bruno formula, which yields the n-th derivative of a given composite function. However, this formula uses sums over set partitions and hence is extremely involved, to the point that to follow this approach seems to be a hopeless task.

2.4. Horizontal Monotonicity

In [42], the so-called “horizontal behavior” of $\left|\zeta \right(s\left)\right|$, mentioned by Saidak and Zvengrowski [43], and earlier by Spira [44] was emphasized. It was observed that, at the beginning of the article on the Riemann zeta function, in the Wolfram MathWorld [45], a plot shows horizontal “ridges” of $\left|\zeta \right(\sigma +it\left)\right|$, for $0<\sigma <1$ and $1<t<100$. If indeed such ridges would decrease strictly monotonically for $0<\sigma <1/2$, then the RH would be proved to be true (cf. [43,46]). This behavior is easy to prove outside the critical strip, but nobody has been able to prove it, so far, inside the critical strip.

2.5. Hyperbolicity of Jensen Polynomials

In 1927, G. Pólya proved that the RH is equivalent to the so-called hyperbolicity of Jensen polynomials for the Riemann zeta function at its point of symmetry. This is called the Pólya–Jensen criterion. Advances in this direction are based in the newly discovered fact that these polynomials can be well approximated in terms of Hermite polynomials [47]. This result was initially praised by E. Bombieri [14] as a breakthrough, but this approach was soon considered actually not so useful, hence a not too promising route, by D.W. Farmer [48].

2.6. Basing on New Bounds for Large Values of Dirichlet Polynomials

Very recently, in [49], L. Guth and J. Maynard have posted a significant new result on the arXiv. Largely using Fourier analysis, the authors’ advance towards the Riemann hypothesis rests on improving a result deemed insurmountable for more than 50 years, that is, making the first substantial improvement to a classical 1940 bound of Ingham regarding the zeros of the Riemann zeta function. Field medalist Terence Tao considered this work a remarkable breakthrough. Yet, he still judged it very far from fully proving the RH.

3. Numerical Approach

A continuous progress has been made over the years in computing numerically more and more zeros on the critical line, and exploring the possible existence of zeros off it; see, e.g., [50,51]. Often, computations are based on the Riemann–Siegel formula [52]. Of course, no numerical approach can provide acceptable proofs of the RH, but the numerical path may be useful to formulate conjectures and develop insight. For instance, simulations and visualizations suggest the possible “horizontal monotonicity” of the modulus of $\zeta \left(s\right)$, even though, unfortunately, nobody has been able to prove that this occurs inside the critical strip [42].

Relevant computations of the sum of the series

$$\sum _k{\displaystyle \frac{1}{t_k^2}}\mathrm{and}\sum _k{\displaystyle \frac{1}{1/4+t_k^2}},$$

$1/2+i{t}_k$ being the nontrivial zeros on the critical line, and estimates (with bounds) for some more general sums were carried out in [53,54]. Some attention has also been devoted to computing the sums ${\sigma }_k:={\sum }_{\rho }{\rho }^{-k}$, extended over all nontrivial zeros, for fixed k’s, $k\in \mathbf{N}$. The ${\sigma }_k$’s are also related to the Li’s coefficients, ${\lambda }_n$, defined below; see [33] (eq. (27), p. 768), e.g.

Here are a few examples. In 1982, R.P. Brent et al. have tested the RH computationally, and confirmed its validity for the first 200,000,001 zeros [55]. Such zeros are all located in the critical strip (and lie on the critical line), up to the ordinate t = 81,702,130.19. In 2002, S. Wedeniwski has shown that the first ${10}^{12}$ nontrivial zeros lie on the critical line [56]. In [57], it is recalled that in 2004 X. Gourdon [58] used a fast method, developed by Odlyzko and Schoenhage, to verify that the first ${10}^{13}$ nontrivial zeros of the zeta function lie on the critical line, which fact validates the RH up to about the ordinate $2.4\times {10}^{12}$, in the critical strip; see [59]. Actually, A.M. Odlyzko in [51,60] showed the heights of the zeros of the Riemann zeta function numbered ${10}^{22}+1$ up to ${10}^{22}+{10}^4$.

More precisely, in 1988, Odlyzko and Schoenhage developed a fast algorithm for evaluating the Riemann zeta function at many points, using the Fast Fourier Transform [61]. This algorithm reduces the number of operations required to evaluate a finite Dirichlet series of length N at $\mathcal{O}\left(N\right)$ equally spaced values from $\mathcal{O}\left(N^2\right)$ to $\mathcal{O}\left(N^{1+\epsilon }\right)$. Such a method would enable testing the RH for the first n zeros in $\mathcal{O}\left(n^{1+\epsilon }\right)$ operations, as opposed to $\mathcal{O}\left(n^{3/2}\right)$ operations required by earlier methods, provided that no multiple zeros or closely spaced zeros of the zeta function occur.

Later, in 2001 Odlyzko computed the first 10 billion zeros of the Riemann zeta function near height ${10}^{22}$, thus verifying the RH for these zeros and providing further evidence for other conjectures related to the distribution of zeros of the Riemann zeta function [60].

In his 2004 paper, Gourdon presented an optimization of the Odlyzko–Schoenhage algorithm [58]. This efficiently computes the Riemann zeta function at large heights on the critical line and facilitates the computation of zeros of the zeta function through its implementation. He was able to compute two billion zeros from the 1024-th zero of the Riemann Zeta function.

More recently, in 2021, Platt and Trudgian established a remarkable result, verifying that the RH is true up to the height $3\times {10}^{12}$, in a rigorous way, using interval arithmetic [62]. In this way, numerical results can provide proofs.

As it is well known, in order to prove the RH, it suffices to show that no zero of the zeta function exists in the semi-infinite open critical upper half-strip, $H:=\left\{\right(\sigma ,t)\in \mathbf{C}:1/2<\sigma <1,t>0\}$. In fact, if a nontrivial zero, say $\rho$, exists in $\Sigma$, then also its conjugate, $\overline{\rho }$, would be a zero (since $\zeta \left(s\right)$ is meromorphic and real on the real line), as well as its symmetric one with respect to the critical line Re$\left\{s\right\}=1/2$, that is $1-\rho$, and thus $1-\overline{\rho }$ as well. This follows from the functional equation obeyed by the zeta function,

$$\zeta \left(s\right)=2^s{\pi }^{s-1}sin\left({\displaystyle \frac{\pi }{2}}s\right)\Gamma (1-s)\zeta (1-s)$$

(10)

[2] (Ch. II, (2.1.1), p. 13), [3] (eq. (4), p. 13), also written, changing s into $1-s$, $\zeta (1-s)=2^{1-s}{\pi }^{-s}cos\left({\displaystyle \frac{\pi }{2}}s\right)\Gamma \left(s\right)\zeta \left(s\right)$ [2] (Ch. II, (2.1.8), p. 16).

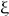It follows that nontrivial zeros would come in groups of four if they exist in the critical strip, off the critical line, and in pairs if they are on the critical line. Therefore, if $s_0:={\sigma }_0+i{t}_0$ with $1/2<{\sigma }_0<1$ and $t_0>0$ is a zero, the Weierstrass factorization of the entire function would contain terms like

$${\left\{(s-s_0)(s-\overline{s_0})[s-(1-s_0)][s-(1-\overline{s_0})]\right\}}^{m_0},$$

(11)

where $m_0\in \mathbf{N}$ would be the multiplicity of $s_0$.

On the other hand, it is common knowledge too that no zeros exist on the intersection of the critical strip and the real line, as well as on the lines Re$\left\{s\right\}=0$, Re$\left\{s\right\}=1$. One can thus confine the discussion to the set H, but this region can be restricted further. In fact, it is also known that no nontrivial zero exists in H with an ordinate less than some value. For instance, in [63] (Ex. 10.2.1, (c), p. 353) it is recorded that, if ${\rho }_0={\sigma }_0+i{t}_0$ is a nontrivial zero with ${\sigma }_0\ne 1/2$, then $|t_0|>9.{2518}^{+}$.

However, as mentioned above, in a recent paper, Platt and Trudgian have shown numerically, yet rigorously, using interval arithmetic, that the RH is true up to about the height $3\times {10}^{12}$ (cf. [58,59]), that is, all zeros existing in the critical strip, with imaginary part $0<t\le 3\times {10}^{12}$, have real part Re$\left\{s\right\}=1/2$ (and are all simple), [62]. Consequently, any investigation aimed at ruling out the existence of nontrivial zeros can be confined just to the set $H_{t^{\ast }}:=H\cap \{t>t^{\ast }\}$, for some $t^{\ast }$, that can be taken equal to $3\times {10}^{12}$. Considering the shape of the known zero-free regions, we can state that any hypothetical nontrivial zero should be located in such set $H_{t^{\ast }}$ and very close to and at the right of the vertical line Re$s=1/2$, or very close to and at the left of the vertical line Re$s=1$ (or symmetrically, of course, both with respect the line Re$s=1/2$ and to the real line).

That no nontrivial zeros exist off the critical line, below some value of t can also be shown aby pplying the well-known equation for the sum of the inverse nontrivial zeta’s zeros,

$$\sum _{\rho }{\displaystyle \frac{1}{\rho }}=1+{\displaystyle \frac{\gamma }{2}}-{\displaystyle \frac{1}{2}}ln\left(4\pi \right)=0.{0230957}^{+},$$

(12)

where the sum is extended over all nontrivial zeros of the zeta function, and $\gamma =0.{5772156}^{+}$ is the Euler–Mascheroni constant. This equation was already known to Riemann [3] (Ch. 3, sec. 3.8, p. 67, eq. (4)), and appears also in Li’s criterion [28,31,32]. The sum on the left-hand side of (12) does not converge absolutely, but each term $1/\rho$ is intended to be grouped with its conjugate [64] (Ch. 12, p. 81), [65] (p. 214), and the sum is understood as

$$\sum _{\rho }:=\underset{T\to +\infty }{lim}\sum _{\left|\mathrm{Im}\right\{\rho \left\}\right|\le T}$$

(13)

termed “*-convergence” by Bombieri and Lagarias [who also used the unusual notation $x^{+}$ to denote chopping off further decimals of x] [28] (p. 275). As already recalled, each nontrivial zero is necessarily accompanied by its complex conjugate as well as by its symmetric one with respect to the critical line (if it lies off this line), and thus by the conjugate of the latter too. Therefore, as we stated above, if a nontrivial zero, say ${\rho }_0={\sigma }_0+i{t}_0$, exists in the critical strip, off the critical line, with $1/2<{\sigma }_0<1$, $t_0>0$, then also ${\overline{\rho }}_0$, $1-{\rho }_0$, and $1-{\overline{\rho }}_0$ will contribute to the sum on the left-hand side of (12) with the amount

$${\displaystyle \frac{1}{{\rho }_0}}+{\displaystyle \frac{1}{{\overline{\rho }}_0}}+{\displaystyle \frac{1}{1-{\rho }_0}}+{\displaystyle \frac{1}{1-{\overline{\rho }}_0}}$$

$$={\displaystyle \frac{2\mathrm{Re}\left\{{\rho }_0\right\}}{|{\rho }_0{|}^2}}+{\displaystyle \frac{2(1-\mathrm{Re}\left\{{\rho }_0\right\})}{|1-{\rho }_0{|}^2}}=2\left({\displaystyle \frac{{\sigma }_0}{{\sigma }_0^2+t_0^2}}+{\displaystyle \frac{1-{\sigma }_0}{{(1-{\sigma }_0)}^2+t_0^2}}\right)\ge {\displaystyle \frac{2}{1+t_0^2}}.$$

(14)

For the last inequality, the result of [63] (Ex. 10.2, 8. (a), p. 355) was used. This estimate is useful to rule out that some ${\rho }_0$ might be a nontrivial zero.

In fact, if we define the following sums of the inverses of zeros:

• $S_{\mathrm{all}}$: when extended to all nontrivial zeros (including both those on the critical line and those off the critical line, if any exist), see Equation (12);
• $S_{\mathrm{on}}$: when extended only to all nontrivial zeros located on the critical line;
• $S_{\mathrm{off}}$: when extended only to all nontrivial zeros off the critical line;
• $S_{\mathrm{on},200}$: when extended only to the first 200 nontrivial zeros located on the critical line (note that each zero with $t>0$ is included along with its conjugate),

then we have

$$S_{\mathrm{all}}=S_{\mathrm{on}}+S_{\mathrm{off}}\ge S_{\mathrm{on},200}+{\displaystyle \frac{2}{1+t_0^2}}$$

(15)

since $S_{\mathrm{on},200}$ does not include all nontrivial zeros lying on the critical line, and ${\displaystyle \frac{2}{1+t_0^2}}$ represents the contribution of a single nontrivial zero located off the critical line (along with its complex conjugate, its symmetrical counterpart, and the conjugate of the symmetrical counterpart). Since

$$S_{\mathrm{all}}=1+{\displaystyle \frac{\gamma }{2}}-{\displaystyle \frac{1}{2}}ln\left(4\pi \right)\approx 0.0230957$$

(16)

by (12), and

$$S_{\mathrm{on},200}\approx 0.021,$$

(17)

as reported in [66] (p. 249), it follows that

$${\displaystyle \frac{2}{1+t_0^2}}\le S_{\mathrm{all}}-S_{\mathrm{on},200}\approx 0.0230957-0.021=0.0020957,$$

(18)

which implies the approximate bound

$$t_0\ge 30.918.$$

(19)

That is, if a nontrivial zero like ${\sigma }_0+i{t}_0$ exists off the critical line, then, necessarily, $t_0$ must be larger than roughly $30.918$. No such zero can exist below this ordinate.

Of course, significantly larger lower bounds are known to exist: recall for instance, the various results on zero-free regions or the Platt and Trudgian bound of $3\times {10}^{12}$.

4. Summary

It has long been known that all nontrivial zeros of the Riemann zeta function must lie within the open critical strip $\Sigma :=\left\{\right(\sigma ,t)\in \mathbf{C}:0<\sigma <1,-\infty <t<+\infty \}$. Although the existence of infinitely (countably) many zeros on the critical line, Re$\left\{s\right\}=1/2$, has been known since 1914, no proof of the existence or nonexistence of other nontrivial zeros within $\Sigma$ has been found to date. The Riemann Hypothesis (RH) states that no such other nontrivial zeros exist. This short survey describes a few more or less recent attempts to prove the RH.