Charging a Dimerized Quantum XY Chain
Abstract
1. Introduction
2. Model
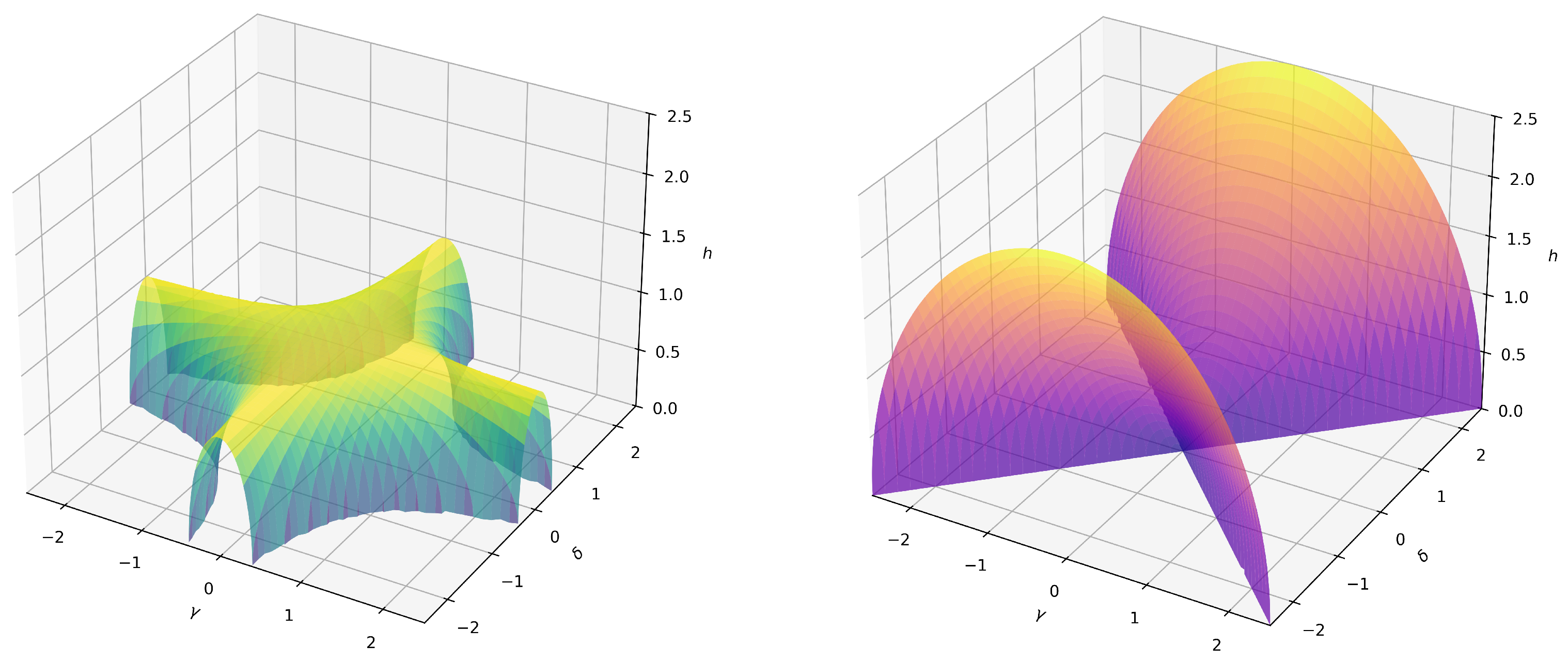
3. Study of QPT Effects
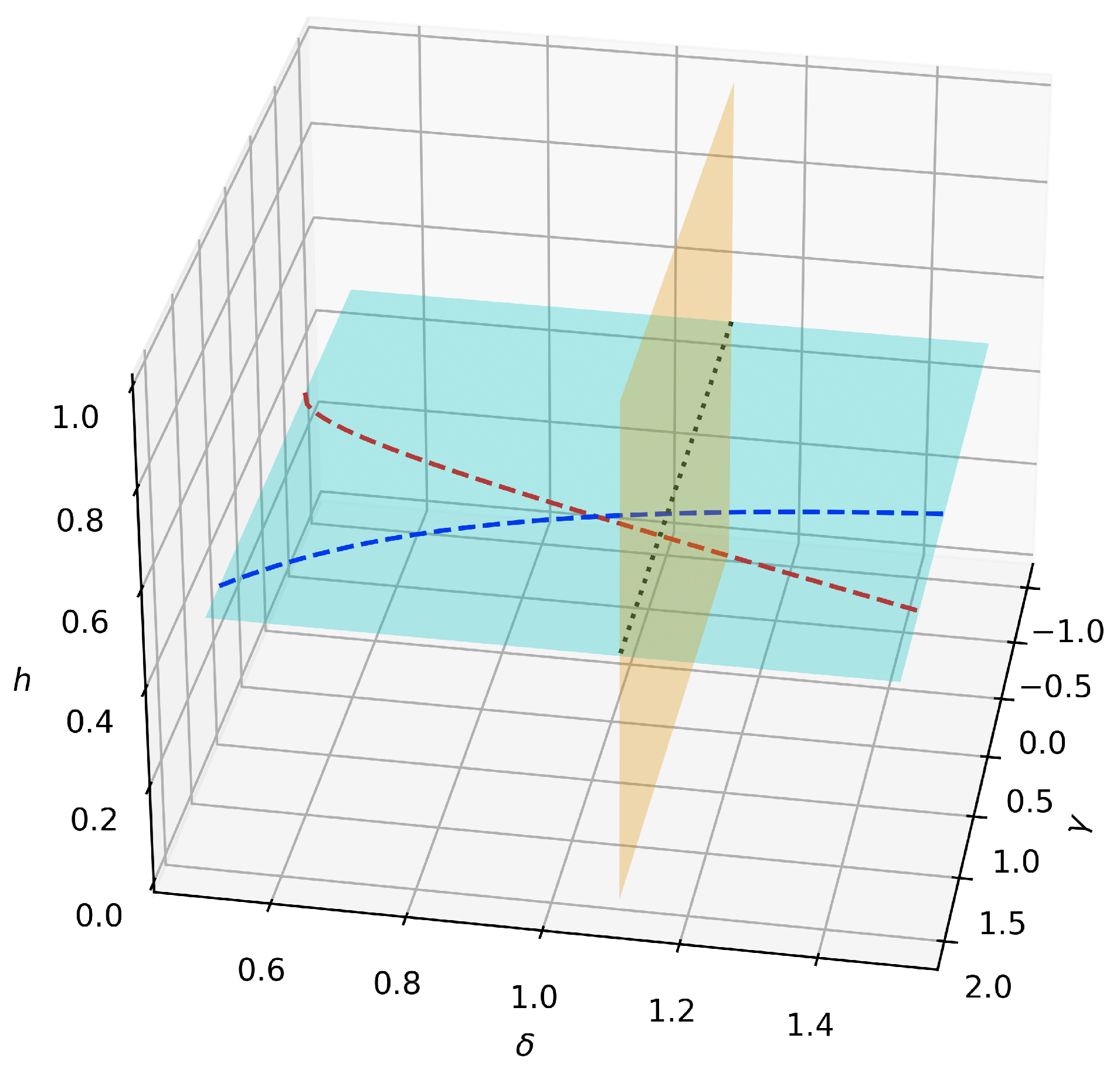
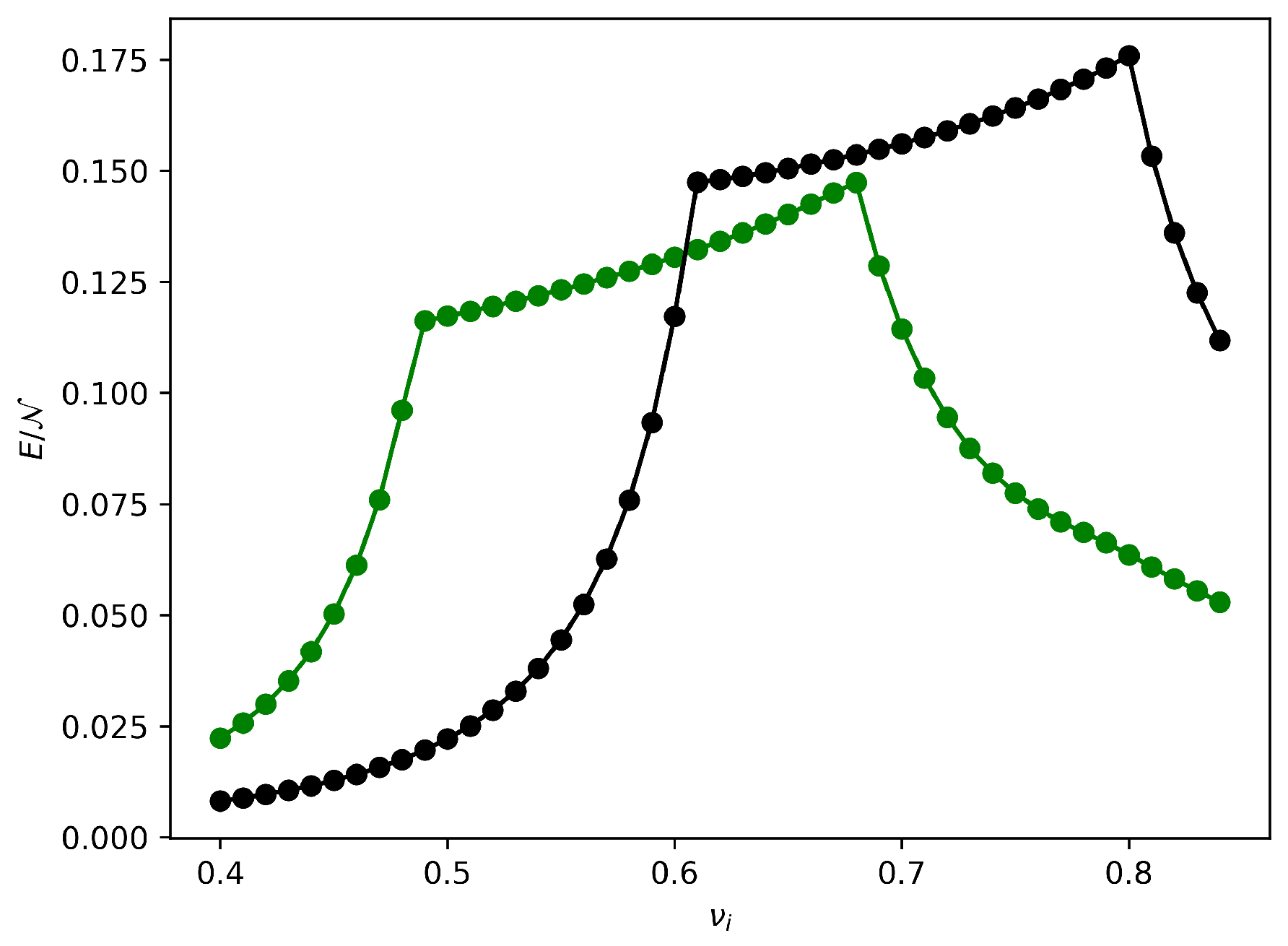
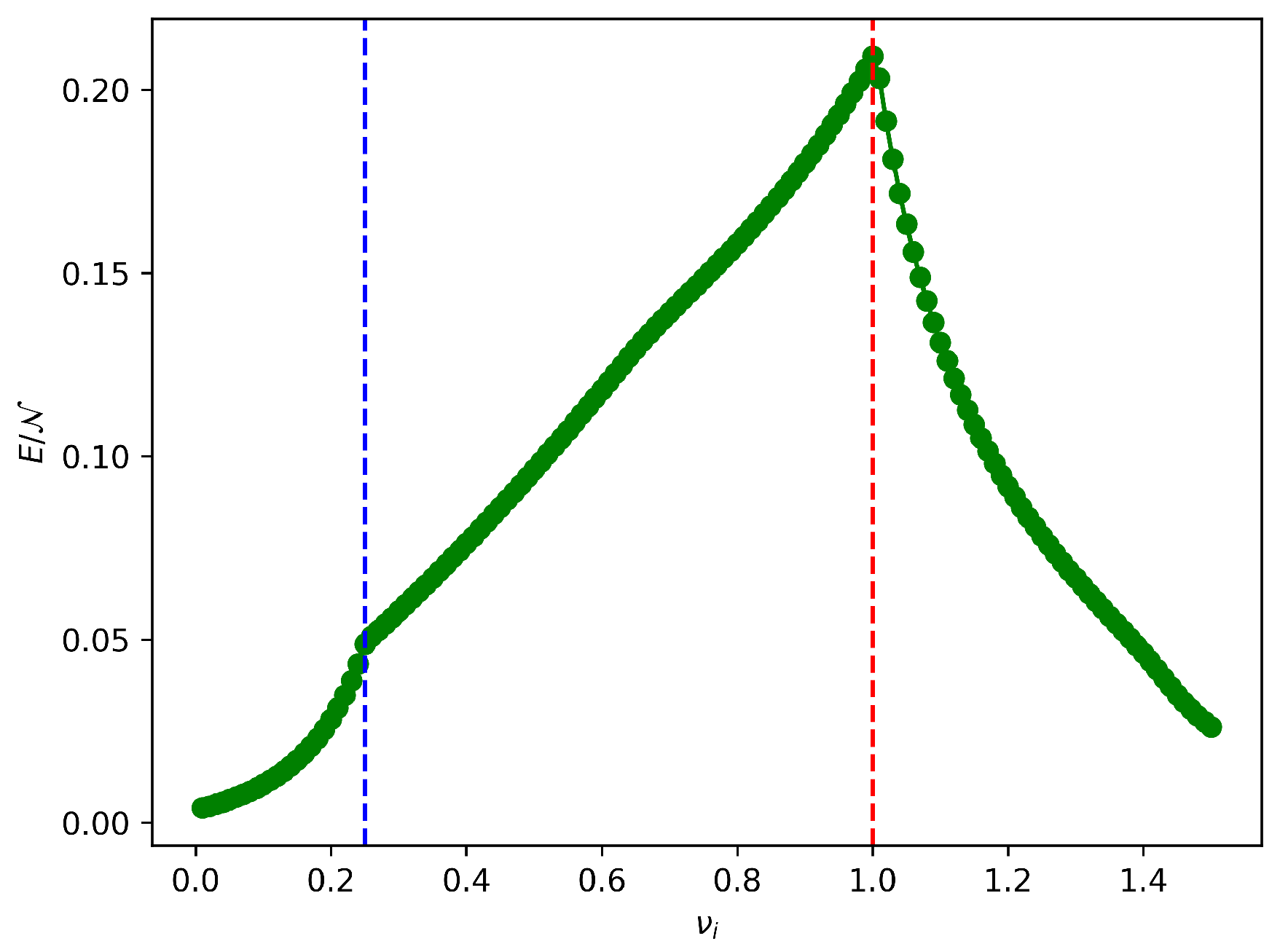
3.1. Quench of at Given h and
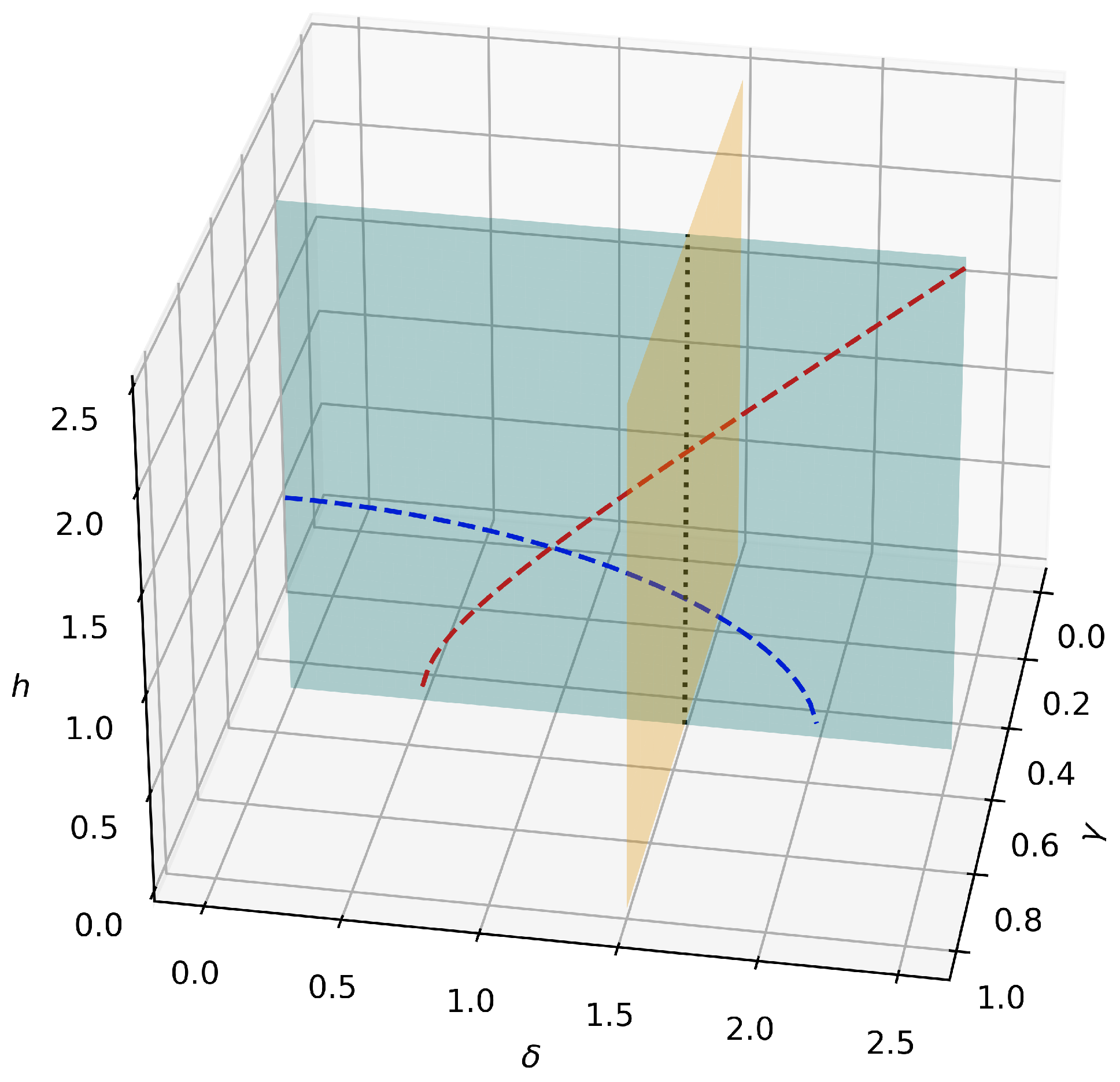
3.2. Quench of h at Given and
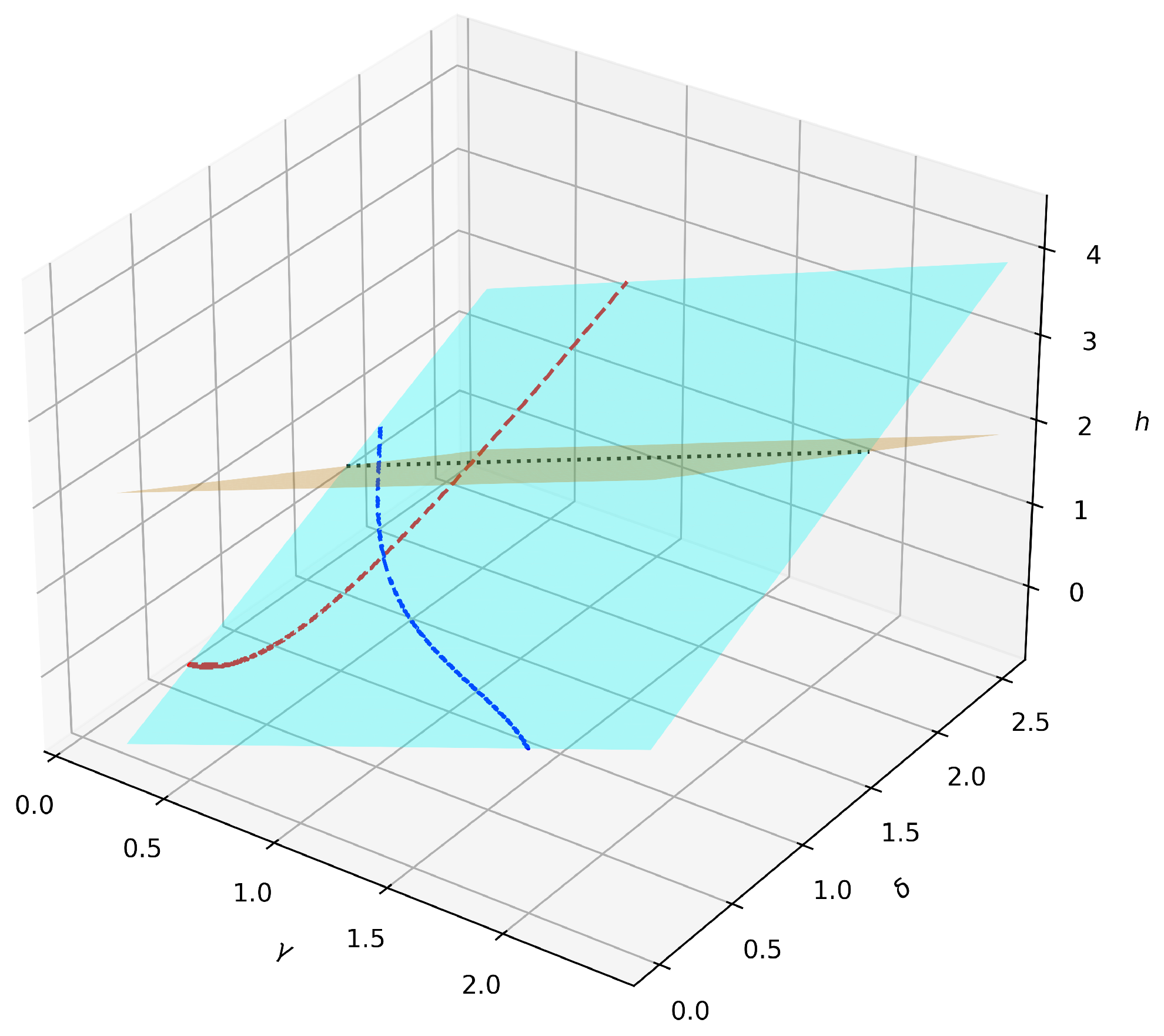
3.3. Quench of h and at Given
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- De las Cuevas, G.; Cubitt, T.S. Simple universal models capture all classical spin physics. Science 2016, 351, 1180–1183. [Google Scholar] [CrossRef]
- Auerbach, A. Interacting Electrons and Quantum Magnetism; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Wysin, G.M. Magnetism theory: Spin models. In Magnetic Excitations and Geometric Confinement; 2053–2563; IOP Publishing: Bristol, UK, 2015; pp. 1–47. [Google Scholar] [CrossRef]
- Cialone, M.; Celegato, F.; Coïsson, M.; Barrera, G.; Fiore, G.; Shvab, R.; Klement, U.; Rizzi, P.; Tiberto, P. Tailoring magnetic properties of multicomponent layered structure via current annealing in FePd thin films. Sci. Rep. 2017, 7, 16691. [Google Scholar] [CrossRef] [PubMed]
- Cialone, M.; Fernandez-Barcia, M.; Celegato, F.; Coisson, M.; Barrera, G.; Uhlemann, M.; Gebert, A.; Sort, J.; Pellicer, E.; Rizzi, P.; et al. A comparative study of the influence of the deposition technique (electrodeposition versus sputtering) on the properties of nanostructured Fe70Pd30 films. Sci. Technol. Adv. Mater. 2020, 21, 424–434. [Google Scholar] [CrossRef] [PubMed]
- Manousakis, E. The spin-½ Heisenberg antiferromagnet on a square lattice and its application to the cuprous oxides. Rev. Mod. Phys. 1991, 63, 1–62. [Google Scholar] [CrossRef]
- Bramwell, S.; Harris, M. Frustration in Ising-type spin models on the pyrochlore lattice. J. Phys. Condens. Matter 1998, 10, L215. [Google Scholar] [CrossRef]
- Mydosh, J.A. Spin glasses: Redux: An updated experimental/materials survey. Rep. Prog. Phys. 2015, 78, 052501. [Google Scholar] [CrossRef]
- Pan, F.; Chico, J.; Delin, A.; Bergman, A.; Bergqvist, L. Extended spin model in atomistic simulations of alloys. Phys. Rev. B 2017, 95, 184432. [Google Scholar] [CrossRef]
- Cavaliere, F.; Razzoli, L.; Carrega, M.; Benenti, G.; Sassetti, M. Hybrid quantum thermal machines with dynamical couplings. iScience 2023, 26, 106235. [Google Scholar] [CrossRef]
- Eckhardt, C.J.; Passetti, G.; Othman, M.; Karrasch, C.; Cavaliere, F.; Sentef, M.A.; Kennes, D.M. Quantum Floquet engineering with an exactly solvable tight-binding chain in a cavity. Commun. Phys. 2022, 5, 122. [Google Scholar] [CrossRef]
- Feng, X.Y.; Zhang, G.M.; Xiang, T. Topological Characterization of Quantum Phase Transitions in a Spin-1/2 Model. Phys. Rev. Lett. 2007, 98, 087204. [Google Scholar] [CrossRef]
- Duivenvoorden, K.; Quella, T. Topological phases of spin chains. Phys. Rev. B 2013, 87, 125145. [Google Scholar] [CrossRef]
- Dubinkin, O.; Hughes, T.L. Higher-order bosonic topological phases in spin models. Phys. Rev. B 2019, 99, 235132. [Google Scholar] [CrossRef]
- Balents, L. Spin liquids in frustrated magnets. Nature 2010, 464, 199–208. [Google Scholar] [CrossRef] [PubMed]
- Diep, H.T. Frustrated Spin Systems; World Scientific: Singapore, 2013. [Google Scholar]
- Sacco Shaikh, D.; Catalano, A.G.; Cavaliere, F.; Franchini, F.; Sassetti, M.; Traverso Ziani, N. Phase diagram of the topologically frustrated XY chain. Eur. Phys. J. Plus 2024, 139, 1–14. [Google Scholar] [CrossRef]
- Marić, V.; Giampaolo, S.M.; Franchini, F. Quantum phase transition induced by topological frustration. Commun. Phys. 2020, 3, 220. [Google Scholar] [CrossRef]
- Lacroix, C.; Mendels, P.; Mila, F. Introduction to Frustrated Magnetism: Materials, Experiments, Theory; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2011; Volume 164. [Google Scholar]
- Mitra, A. Quantum quench dynamics. Annu. Rev. Condens. Matter Phys. 2018, 9, 245–259. [Google Scholar] [CrossRef]
- Essler, F.H.; Fagotti, M. Quench dynamics and relaxation in isolated integrable quantum spin chains. J. Stat. Mech. Theory Exp. 2016, 2016, 064002. [Google Scholar] [CrossRef]
- Porta, S.; Gambetta, F.M.; Traverso Ziani, N.; Kennes, D.M.; Sassetti, M.; Cavaliere, F. Nonmonotonic response and light-cone freezing in fermionic systems under quantum quenches from gapless to gapped or partially gapped states. Phys. Rev. B 2018, 97, 035433. [Google Scholar] [CrossRef]
- Porta, S.; Cavaliere, F.; Sassetti, M.; Traverso Ziani, N. Topological classification of dynamical quantum phase transitions in the xy chain. Sci. Rep. 2020, 10, 12766. [Google Scholar] [CrossRef]
- Faure, Q.; Takayoshi, S.; Simonet, V.; Grenier, B.; Månsson, M.; White, J.S.; Tucker, G.S.; Rüegg, C.; Lejay, P.; Giamarchi, T.; et al. Tomonaga-Luttinger Liquid Spin Dynamics in the Quasi-One-Dimensional Ising-Like Antiferromagnet BaCo2V2O8. Phys. Rev. Lett. 2019, 123, 027204. [Google Scholar] [CrossRef]
- Faure, Q.; Takayoshi, S.; Petit, S.; Simonet, V.; Raymond, S.; Regnault, L.P.; Boehm, M.; White, J.S.; Månsson, M.; Rüegg, C.; et al. Topological quantum phase transition in the Ising-like antiferromagnetic spin chain BaCo2V2O8. Nat. Phys. 2018, 14, 716–722. [Google Scholar] [CrossRef]
- Kinoshita, T.; Wenger, T.; Weiss, D.S. A quantum Newton’s cradle. Nature 2006, 440, 900–903. [Google Scholar] [CrossRef]
- Holstein, T.; Primakoff, H. Field Dependence of the Intrinsic Domain Magnetization of a Ferromagnet. Phys. Rev. 1940, 58, 1098–1113. [Google Scholar] [CrossRef]
- Popov, V.N.; Fedotov, S. The functional-integration method and diagram technique for spin systems. Zh. Eksp. Teor. Fiz 1988, 94, 183–194. [Google Scholar]
- Traverso, S.; Fleckenstein, C.; Sassetti, M.; Ziani, N.T. An exact local mapping from clock-spins to fermions. SciPost Phys. Core 2023, 6, 055. [Google Scholar] [CrossRef]
- Traverso Ziani, N.; Fleckenstein, C.; Dolcetto, G.; Trauzettel, B. Fractional charge oscillations in quantum dots with quantum spin Hall effect. Phys. Rev. B 2017, 95, 205418. [Google Scholar] [CrossRef]
- Rodriguez, R.; Parmentier, F.; Ferraro, D.; Roulleau, P.; Gennser, U.; Cavanna, A.; Sassetti, M.; Portier, F.; Mailly, D.; Roche, P. Relaxation and revival of quasiparticles injected in an interacting quantum Hall liquid. Nat. Commun. 2020, 11, 2426. [Google Scholar] [CrossRef] [PubMed]
- Gambetta, F.M.; Ziani, N.T.; Barbarino, S.; Cavaliere, F.; Sassetti, M. Anomalous Friedel oscillations in a quasihelical quantum dot. Phys. Rev. B 2015, 91, 235421. [Google Scholar] [CrossRef]
- Ziani, N.T.; Cavaliere, F.; Sassetti, M. Theory of the STM detection of Wigner molecules in spin-incoherent CNTs. Europhys. Lett. 2013, 102, 47006. [Google Scholar] [CrossRef]
- Ziani, N.T.; Cavaliere, F.; Becerra, K.G.; Sassetti, M. A Short Review of One-Dimensional Wigner Crystallization. Crystals 2021, 11, 20. [Google Scholar] [CrossRef]
- Bloch, I.; Dalibard, J.; Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 2008, 80, 885–964. [Google Scholar] [CrossRef]
- Cazalilla, M.A.; Citro, R.; Giamarchi, T.; Orignac, E.; Rigol, M. One dimensional bosons: From condensed matter systems to ultracold gases. Rev. Mod. Phys. 2011, 83, 1405–1466. [Google Scholar] [CrossRef]
- Trotzky, S.; Chen, Y.A.; Flesch, A.; McCulloch, I.P.; Schollwöck, U.; Eisert, J.; Bloch, I. Probing the relaxation towards equilibrium in an isolated strongly correlated one-dimensional Bose gas. Nat. Phys. 2012, 8, 325–330. [Google Scholar] [CrossRef]
- Imambekov, A.; Glazman, L.I. Universal Theory of Nonlinear Luttinger Liquids. Science 2009, 323, 228–231. [Google Scholar] [CrossRef] [PubMed]
- Imambekov, A.; Schmidt, T.L.; Glazman, L.I. One-dimensional quantum liquids: Beyond the Luttinger liquid paradigm. Rev. Mod. Phys. 2012, 84, 1253–1306. [Google Scholar] [CrossRef]
- Wu, C.; Bernevig, B.A.; Zhang, S.C. Helical Liquid and the Edge of Quantum Spin Hall Systems. Phys. Rev. Lett. 2006, 96, 106401. [Google Scholar] [CrossRef]
- Fiete, G.A.; Le Hur, K.; Balents, L. Coulomb drag between two spin-incoherent Luttinger liquids. Phys. Rev. B 2006, 73, 165104. [Google Scholar] [CrossRef]
- Fiete, G.A. Colloquium: The spin-incoherent Luttinger liquid. Rev. Mod. Phys. 2007, 79, 801–820. [Google Scholar] [CrossRef]
- Matveev, K.A.; Furusaki, A.; Glazman, L.I. Bosonization of strongly interacting one-dimensional electrons. Phys. Rev. B 2007, 76, 155440. [Google Scholar] [CrossRef]
- Voit, J. One-dimensional Fermi liquids. Rep. Prog. Phys. 1995, 58, 977. [Google Scholar] [CrossRef]
- Haldane, F.D.M. Effective Harmonic-Fluid Approach to Low-Energy Properties of One-Dimensional Quantum Fluids. Phys. Rev. Lett. 1981, 47, 1840–1843. [Google Scholar] [CrossRef]
- Haldane, F.D.M. ’Luttinger liquid theory’ of one-dimensional quantum fluids. I. Properties of the Luttinger model and their extension to the general 1D interacting spinless Fermi gas. J. Phys. Solid State Phys. 1981, 14, 2585. [Google Scholar] [CrossRef]
- Giamarchi, T. Quantum Physics in One Dimension; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Bethe, H. Zur theorie der metalle: I. Eigenwerte und eigenfunktionen der linearen atomkette. Z. Phys. 1931, 71, 205–226. [Google Scholar] [CrossRef]
- Jordan, P.; Wigner, E. Über das Paulische Äquivalenzverbot. Z. Phys. 1928, 47, 631–651. [Google Scholar] [CrossRef]
- Franchini, F. An Introduction to Integrable Techniques for One-Dimensional Quantum Systems; Springer: Berlin/Heidelberg, Germany, 2017; Volume 940. [Google Scholar]
- Bayat, A.; Bose, S.; Johannesson, H. Entanglement in Spin Chains: From Theory to Quantum Technology Applications; Springer: Berlin/Heidelberg, Germany, 2022. [Google Scholar]
- Le, T.P.; Levinsen, J.; Modi, K.; Parish, M.M.; Pollock, F.A. Spin-chain model of a many-body quantum battery. Phys. Rev. A 2018, 97, 022106. [Google Scholar] [CrossRef]
- Liu, J.X.; Shi, H.L.; Shi, Y.H.; Wang, X.H.; Yang, W.L. Entanglement and work extraction in the central-spin quantum battery. Phys. Rev. B 2021, 104, 245418. [Google Scholar] [CrossRef]
- Zhao, F.; Dou, F.Q.; Zhao, Q. Quantum battery of interacting spins with environmental noise. Phys. Rev. A 2021, 103, 033715. [Google Scholar] [CrossRef]
- Catalano, A.; Giampaolo, S.; Morsch, O.; Giovannetti, V.; Franchini, F. Frustrating Quantum Batteries. PRX Quantum 2024, 5, 030319. [Google Scholar] [CrossRef]
- Grazi, R.; Sacco Shaikh, D.; Sassetti, M.; Traverso Ziani, N.; Ferraro, D. Controlling Energy Storage Crossing Quantum Phase Transitions in an Integrable Spin Quantum Battery. Phys. Rev. Lett. 2024, 133, 197001. [Google Scholar] [CrossRef] [PubMed]
- Ali, A.; Elghaayda, S.; Al-Kuwari, S.; Hussain, M.I.; Rahim, M.T.; Kuniyil, H.; Seuda, C.; Allati, A.E.; Mansour, M.; Haddadi, S. Super-Extensive Scaling in 1D Spin-1/2XY − Γ(γ) Chain Quantum Battery. arXiv 2024, arXiv:2411.14074. [Google Scholar]
- Alicki, R.; Fannes, M. Entanglement boost for extractable work from ensembles of quantum batteries. Phys. Rev. E 2013, 87, 042123. [Google Scholar] [CrossRef] [PubMed]
- Bhattacharjee, S.; Dutta, A. Quantum thermal machines and batteries. Eur. Phys. J. 2021, 94, 239. [Google Scholar] [CrossRef]
- Campaioli, F.; Gherardini, S.; Quach, J.Q.; Polini, M.; Andolina, G.M. Colloquium: Quantum batteries. Rev. Mod. Phys. 2024, 96, 031001. [Google Scholar] [CrossRef]
- Quach, J.; Cerullo, G.; Virgili, T. Quantum batteries: The future of energy storage? Joule 2023, 7, 2195–2200. [Google Scholar] [CrossRef]
- Benenti, G.; Casati, G.; Saito, K.; Whitney, R.S. Fundamental aspects of steady-state conversion of heat to work at the nanoscale. Phys. Rep. 2017, 694, 1–124. [Google Scholar] [CrossRef]
- Esposito, M.; Harbola, U.; Mukamel, S. Nonequilibrium fluctuations, fluctuation theorems, and counting statistics in quantum systems. Rev. Mod. Phys. 2009, 81, 1665–1702. [Google Scholar] [CrossRef]
- Campisi, M.; Fazio, R. Dissipation, correlation and lags in heat engines. J. Phys. Math. Theor. 2016, 49, 345002. [Google Scholar] [CrossRef]
- Vinjanampathy, S.; Anders, J. Quantum thermodynamics. Contemp. Phys. 2016, 57, 545–579. [Google Scholar] [CrossRef]
- Potts, P.P. Quantum Thermodynamics. arXiv 2024, arXiv:2406.19206. [Google Scholar]
- Hu, C.K.; Qiu, J.; Souza, P.J.P.; Yuan, J.; Zhou, Y.; Zhang, L.; Chu, J.; Pan, X.; Hu, L.; Li, J.; et al. Optimal charging of a superconducting quantum battery. Quantum Sci. Technol. 2022, 7, 045018. [Google Scholar] [CrossRef]
- Gemme, G.; Andolina, G.M.; Pellegrino, F.M.D.; Sassetti, M.; Ferraro, D. Off-Resonant Dicke Quantum Battery: Charging by Virtual Photons. Batteries 2023, 9, 197. [Google Scholar] [CrossRef]
- Dou, F.Q.; Yang, F.M. Superconducting transmon qubit-resonator quantum battery. Phys. Rev. A 2023, 107, 023725. [Google Scholar] [CrossRef]
- Razzoli, L.; Gemme, G.; Khomchenko, I.; Sassetti, M.; Ouerdane, H.; Ferraro, D.; Benenti, G. Cyclic solid-state quantum battery: Thermodynamic characterization and quantum hardware simulation. Quantum Sci. Technol. 2025, 10, 015064. [Google Scholar] [CrossRef]
- Cavaliere, F.; Gemme, G.; Benenti, G.; Ferraro, D.; Sassetti, M. Dynamical blockade of a reservoir for optimal performances of a quantum battery. arXiv 2024, arXiv:2407.16471. [Google Scholar]
- Chiribella, G.; Yang, Y.; Renner, R. Fundamental Energy Requirement of Reversible Quantum Operations. Phys. Rev. X 2021, 11, 021014. [Google Scholar] [CrossRef]
- Menta, R.; Cioni, F.; Aiudi, R.; Polini, M.; Giovannetti, V. Globally driven superconducting quantum computing architecture. arXiv 2024, arXiv:2407.01182. [Google Scholar]
- Elyasi, S.N.; Rossi, M.A.C.; Genoni, M.G. Experimental simulation of daemonic work extraction in open quantum batteries on a digital quantum computer. arXiv 2024, arXiv:2410.16567. [Google Scholar]
- Binder, F.C.; Vinjanampathy, S.; Modi, K.; Goold, J. Quantacell: Powerful charging of quantum batteries. New J. Phys. 2015, 17, 075015. [Google Scholar] [CrossRef]
- Andolina, G.M.; Farina, D.; Mari, A.; Pellegrini, V.; Giovannetti, V.; Polini, M. Charger-mediated energy transfer in exactly solvable models for quantum batteries. Phys. Rev. B 2018, 98, 205423. [Google Scholar] [CrossRef]
- Crescente, A.; Ferraro, D.; Carrega, M.; Sassetti, M. Enhancing coherent energy transfer between quantum devices via a mediator. Phys. Rev. Res. 2022, 4, 033216. [Google Scholar] [CrossRef]
- Perk, J.; Capel, H.; Zuilhof, M.; Siskens, T. On a soluble model of an antiferromagnetic chain with alternating interactions and magnetic moments. Phys. Stat. Mech. Its Appl. 1975, 81, 319–348. [Google Scholar] [CrossRef]
- Wakatsuki, R.; Ezawa, M.; Tanaka, Y.; Nagaosa, N. Fermion fractionalization to Majorana fermions in a dimerized Kitaev superconductor. Phys. Rev. B 2014, 90, 014505. [Google Scholar] [CrossRef]
- Ziani, N.T.; Fleckenstein, C.; Vigliotti, L.; Trauzettel, B.; Sassetti, M. From fractional solitons to Majorana fermions in a paradigmatic model of topological superconductivity. Phys. Rev. B 2020, 101, 195303. [Google Scholar] [CrossRef]
- Jackiw, R.; Rebbi, C. Solitons with fermion number ½. Phys. Rev. D 1976, 13, 3398–3409. [Google Scholar] [CrossRef]
- Kivelson, S.; Schrieffer, J.R. Fractional charge, a sharp quantum observable. Phys. Rev. B 1982, 25, 6447–6451. [Google Scholar] [CrossRef]
- Goldstone, J.; Wilczek, F. Fractional Quantum Numbers on Solitons. Phys. Rev. Lett. 1981, 47, 986–989. [Google Scholar] [CrossRef]
- Heeger, A.J.; Kivelson, S.; Schrieffer, J.R.; Su, W.P. Solitons in conducting polymers. Rev. Mod. Phys. 1988, 60, 781–850. [Google Scholar] [CrossRef]
- Qi, X.L.; Hughes, T.L.; Zhang, S.C. Fractional charge and quantized current in the quantum spin Hall state. Nat. Phys. 2008, 4, 273–276. [Google Scholar] [CrossRef]
- Fleckenstein, C.; Ziani, N.T.; Calzona, A.; Sassetti, M.; Trauzettel, B. Formation and detection of Majorana modes in quantum spin Hall trenches. Phys. Rev. B 2021, 103, 125303. [Google Scholar] [CrossRef]
- Traverso, S.; Sassetti, M.; Traverso Ziani, N. Emerging topological bound states in Haldane model zigzag nanoribbons. Npj Quantum Mater. 2024, 9, 9. [Google Scholar] [CrossRef]
- Traverso, S.; Traverso Ziani, N.; Sassetti, M. Effects of the Vertices on the Topological Bound States in a Quasicrystalline Topological Insulator. Symmetry 2022, 14, 1736. [Google Scholar] [CrossRef]
- Traverso, S.; Sassetti, M.; Ziani, N.T. Role of the edges in a quasicrystalline Haldane model. Phys. Rev. B 2022, 106, 125428. [Google Scholar] [CrossRef]
- Kitaev, A.Y. Unpaired Majorana fermions in quantum wires. Physics-Uspekhi 2001, 44, 131. [Google Scholar] [CrossRef]
- Deng, M.T.; Vaitiekėnas, S.; Hansen, E.B.; Danon, J.; Leijnse, M.; Flensberg, K.; Nygård, J.; Krogstrup, P.; Marcus, C.M. Majorana bound state in a coupled quantum-dot hybrid-nanowire system. Science 2016, 354, 1557–1562. [Google Scholar] [CrossRef]
- Peñaranda, F.; Aguado, R.; San-Jose, P.; Prada, E. Quantifying wave-function overlaps in inhomogeneous Majorana nanowires. Phys. Rev. B 2018, 98, 235406. [Google Scholar] [CrossRef]
- Fleckenstein, C.; Domínguez, F.; Traverso Ziani, N.; Trauzettel, B. Decaying spectral oscillations in a Majorana wire with finite coherence length. Phys. Rev. B 2018, 97, 155425. [Google Scholar] [CrossRef]
- Roy, D.; Bondyopadhaya, N.; Tewari, S. Topologically trivial zero-bias conductance peak in semiconductor Majorana wires from boundary effects. Phys. Rev. B 2013, 88, 020502. [Google Scholar] [CrossRef]
- Flensberg, K. Tunneling characteristics of a chain of Majorana bound states. Phys. Rev. B 2010, 82, 180516. [Google Scholar] [CrossRef]
- Law, K.T.; Lee, P.A.; Ng, T.K. Majorana Fermion Induced Resonant Andreev Reflection. Phys. Rev. Lett. 2009, 103, 237001. [Google Scholar] [CrossRef] [PubMed]
- Prada, E.; San-Jose, P.; de Moor, M.W.A.; Geresdi, A.; Lee, E.J.H.; Klinovaja, J.; Loss, D.; Nygård, J.; Aguado, R.; Kouwenhoven, L.P. From Andreev to Majorana bound states in hybrid superconductor–semiconductor nanowires. Nat. Rev. Phys. 2020, 2, 575–594. [Google Scholar] [CrossRef]
- Ivanov, D.A. Non-Abelian Statistics of Half-Quantum Vortices in p-Wave Superconductors. Phys. Rev. Lett. 2001, 86, 268–271. [Google Scholar] [CrossRef]
- Marra, P. Majorana nanowires for topological quantum computation. J. Appl. Phys. 2022, 132, 231101. [Google Scholar] [CrossRef]
- Leijnse, M.; Flensberg, K. Introduction to topological superconductivity and Majorana fermions. Semicond. Sci. Technol. 2012, 27, 124003. [Google Scholar] [CrossRef]
- Nayak, C.; Simon, S.H.; Stern, A.; Freedman, M.; Das Sarma, S. Non-Abelian anyons and topological quantum computation. Rev. Mod. Phys. 2008, 80, 1083–1159. [Google Scholar] [CrossRef]
- Kitaev, A. Fault-tolerant quantum computation by anyons. Ann. Phys. 2003, 303, 2–30. [Google Scholar] [CrossRef]
- Oreg, Y.; Refael, G.; von Oppen, F. Helical Liquids and Majorana Bound States in Quantum Wires. Phys. Rev. Lett. 2010, 105, 177002. [Google Scholar] [CrossRef] [PubMed]
- Lutchyn, R.M.; Sau, J.D.; Das Sarma, S. Majorana Fermions and a Topological Phase Transition in Semiconductor-Superconductor Heterostructures. Phys. Rev. Lett. 2010, 105, 077001. [Google Scholar] [CrossRef] [PubMed]
- Smacchia, P.; Amico, L.; Facchi, P.; Fazio, R.; Florio, G.; Pascazio, S.; Vedral, V. Statistical mechanics of the cluster Ising model. Phys. Rev. A 2011, 84, 022304. [Google Scholar] [CrossRef]
- Ding, C. Phase transitions of a cluster Ising model. Phys. Rev. E 2019, 100, 042131. [Google Scholar] [CrossRef] [PubMed]
- Susskind, L. Lattice fermions. Phys. Rev. D 1977, 16, 3031–3039. [Google Scholar] [CrossRef]
- Shamir, Y. Chiral fermions from lattice boundaries. Nucl. Phys. 1993, 406, 90–106. [Google Scholar] [CrossRef]
- Neyenhuis, B.; Zhang, J.; Hess, P.W.; Smith, J.; Lee, A.C.; Richerme, P.; Gong, Z.X.; Gorshkov, A.V.; Monroe, C. Observation of prethermalization in long-range interacting spin chains. Sci. Adv. 2017, 3, e1700672. [Google Scholar] [CrossRef]
- Kim, M.S.; de Oliveira, F.A.M.; Knight, P.L. Properties of squeezed number states and squeezed thermal states. Phys. Rev. A 1989, 40, 2494–2503. [Google Scholar] [CrossRef] [PubMed]
- Puskarov, T.; Schuricht, D. Time evolution during and after finite-time quantum quenches in the transverse-field Ising chain. SciPost Phys. 2016, 1, 003. [Google Scholar] [CrossRef]
- Jeske, J.; Vogt, N.; Cole, J.H. Excitation and state transfer through spin chains in the presence of spatially correlated noise. Phys. Rev. A 2013, 88, 062333. [Google Scholar] [CrossRef]
- Aftergood, J.; Takei, S. Noise in tunneling spin current across coupled quantum spin chains. Phys. Rev. B 2018, 97, 014427. [Google Scholar] [CrossRef]
- Singh, M.; Gangadharaiah, S. Driven quantum spin chain in the presence of noise: Anti-Kibble-Zurek behavior. Phys. Rev. B 2021, 104, 064313. [Google Scholar] [CrossRef]
- Werner, P.; Troyer, M.; Sachdev, S. Quantum Spin Chains with Site Dissipation. J. Phys. Soc. Jpn. 2005, 74, 67–70. [Google Scholar] [CrossRef]
- Chen, C.; An, J.H.; Luo, H.G.; Sun, C.P.; Oh, C.H. Floquet control of quantum dissipation in spin chains. Phys. Rev. A 2015, 91, 052122. [Google Scholar] [CrossRef]
- Schwager, H.; Cirac, J.I.; Giedke, G. Dissipative spin chains: Implementation with cold atoms and steady-state properties. Phys. Rev. A 2013, 87, 022110. [Google Scholar] [CrossRef]
- Shibata, N.; Katsura, H. Dissipative spin chain as a non-Hermitian Kitaev ladder. Phys. Rev. B 2019, 99, 174303. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grazi, R.; Cavaliere, F.; Traverso Ziani, N.; Ferraro, D. Charging a Dimerized Quantum XY Chain. Symmetry 2025, 17, 220. https://doi.org/10.3390/sym17020220
Grazi R, Cavaliere F, Traverso Ziani N, Ferraro D. Charging a Dimerized Quantum XY Chain. Symmetry. 2025; 17(2):220. https://doi.org/10.3390/sym17020220
Chicago/Turabian StyleGrazi, Riccardo, Fabio Cavaliere, Niccolò Traverso Ziani, and Dario Ferraro. 2025. "Charging a Dimerized Quantum XY Chain" Symmetry 17, no. 2: 220. https://doi.org/10.3390/sym17020220
APA StyleGrazi, R., Cavaliere, F., Traverso Ziani, N., & Ferraro, D. (2025). Charging a Dimerized Quantum XY Chain. Symmetry, 17(2), 220. https://doi.org/10.3390/sym17020220







