Coverings of Graphoids: Existence Theorem and Decomposition Theorems
Abstract
1. Introduction
2. Preliminaries
2.1. Graphoids
2.2. Coverings of Graphoids
- (1)
- The mapping ℘ is an epimorphism. It maps the set of vertices of onto the set of vertices of X, and it maps the set of darts of onto the set of darts of X.
- (2)
- For each vertex in , the set of darts with initial vertex is mapped bijectively onto the set of darts with initial vertex , and the set of darts with terminal vertex is mapped bijectively onto the set of darts with terminal vertex .
2.3. Isomorphism and Equivalence of Covering Projections
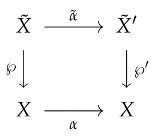 This is denoted as . If in the above diagram, and are isomorphisms, then is an isomorphism of covering projections. This is a standard concept that formalises the intuitive notion of “coverings that are structurally the same”. In particular, and are equivalent whenever there exists an isomorphism of the form . Observe that the set of self-equivalences forms a group, called the group of covering transformations.
This is denoted as . If in the above diagram, and are isomorphisms, then is an isomorphism of covering projections. This is a standard concept that formalises the intuitive notion of “coverings that are structurally the same”. In particular, and are equivalent whenever there exists an isomorphism of the form . Observe that the set of self-equivalences forms a group, called the group of covering transformations.- (i)
- The connected components of are in a one-to-one correspondence with the orbits of the action of on the fibre through unique walk lifting. In particular, is connected if and only if the action of is transitive.
- (ii)
- Let . Then, the induced monoid homomorphism (denoted by the same letter for simplicity) is a monomorphism, and the stabiliser of the action of , which consists of all those closed walks at that lift as closed walks at , is equal to .
2.4. Combinatorialisation in Terms of Voltage Actions
 Furthermore, if the projection is homogeneous, then we can assume that holds whenever a dart x has an inverse.
Furthermore, if the projection is homogeneous, then we can assume that holds whenever a dart x has an inverse.- (i)
- The connected components of the derived graphoid are in bijective correspondence with the orbits of in its action on F. In particular, the covering is connected if and only if acts transitively.
- (ii)
- Closed walks at the vertex are in bijective correspondence with closed walks at whose voltages belong to the stabiliser .

- (i)
- Suppose that projects to an isomorphism . Then, α lifts to an isomorphism of covering projections if and only if is admissible for the action of local groups.
- (ii)
- Suppose that the local groups act faithfully. Then, α lifts to an isomorphism of covering projections if and only if α projects to an admissible isomorphism .
2.5. T-Reduced Voltages
3. Regular Coverings
3.1. The Concept
- 1.
- acts transitively with all stabilisers being equal.
- 2.
- The lifts of any closed walk are either all closed or all open.
- 3.
- The image of the representation homomorphism is a regular subgroup of .
- 4.
- The group acts transitively with a normal stabiliser, denoted as , or, in the case of homogeneous coverings, acts transitively with a normal stabiliser, denoted as .
- 5.
- The covering can be reconstructed in terms of regular voltages.
- 6.
- acts transitively (and hence regularly) on each vertex fibre.
- 7.
- The homomorphism is a 1-fold covering (see the diagram below).

3.2. Lifting Automorphism Groups along Regular Coverings
- (i)
- is transitive if and only if G is transitive.
- (ii)
- is semiregular if and only if G is semiregular.
- (iii)
- In particular, is regular if and only if G is regular.
4. Existence Theorem
5. Decomposition Theorems
5.1. Decomposition of Coverings and the Universal Covering
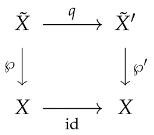
5.2. Decomposing Regular Coverings
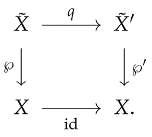
- (i)
- Suppose that q and are regular coverings. Then, ℘ is regular if and only if lifts along q. In this case, is the lift of along q.
- (ii)
- Suppose that ℘ is regular. Then, q is necessarily regular. In contrast, is regular if and only if it is inverse-consistent and projects along q. In this case, projects along q onto .
- (i)
- There exists an epimorphism such that ;
- (ii)
- There is a normal subgroup such that is equivalent to the regular derived covering , where the voltage function is defined by ;
- (iii)
- There is a 1-fold covering , where is a normal subgroup.
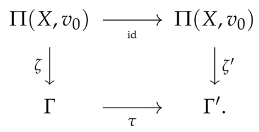 is commutative, which establishes equivalence with (i).
is commutative, which establishes equivalence with (i).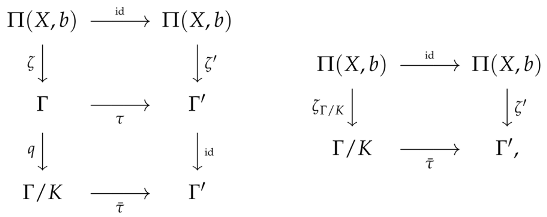 are commutative. The derived covering is then equivalent to by condition (7) of Corollary 6. This establishes equivalence between (i) and (ii).
are commutative. The derived covering is then equivalent to by condition (7) of Corollary 6. This establishes equivalence between (i) and (ii).5.3. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Malnič, A.; Zgrablić, B. Covers of general digraphs. Ars Math. Contemp. 2023. submitted for publication. [Google Scholar]
- Biggs, N.L. Algebraic Graph Theory; Cambridge University Press: Cambridge, UK, 1974. [Google Scholar]
- Djoković, D.Ž. Automorphisms of graphs and coverings. J. Comb. Theory Ser. B 1974, 16, 243–247. [Google Scholar] [CrossRef][Green Version]
- Gross, J.L. Voltage graphs. Discret. Math. 1974, 9, 239–246. [Google Scholar] [CrossRef]
- Ezell, C.L. Observations on the construction of covers using permutation voltage assignments. Discret. Math. 1979, 28, 7–20. [Google Scholar] [CrossRef][Green Version]
- Biggs, N.L. Homological coverings of graphs. J. Lond. Math. Soc. 1984, 30, 1–14. [Google Scholar] [CrossRef]
- Škoviera, M. A contribution to the theory of voltage graphs. Discret. Math. 1986, 61, 281–292. [Google Scholar] [CrossRef]
- Gross, J.L.; Tucker, T.W. Topological Graph Theory; Wiley–Interscience: New York, NY, USA, 1987. [Google Scholar]
- Archdeacon, D.; Gvozdjak, P.; Širáň, J. Constructing and forbidding automorphisms in lifted maps. Math. Slovaca 1997, 47, 113–129. [Google Scholar]
- Brodnik, A.; Malnič, A.; Požar, R. The simultaneous conjugacy problem in the symmetric group. Math. Comp. 2021, 90, 2977–2995. [Google Scholar] [CrossRef]
- Conder, M.D.E.; Ma, J. Arc-transitive abelian regular covers of cubic graphs. J. Algebra 2013, 387, 215–242. [Google Scholar] [CrossRef]
- Du, S.F.; Marušič, D.; Waller, A.O. On 2-arc-transitive covers of complete graphs. J. Comb. Theory Ser. B 1998, 74, 276–290. [Google Scholar] [CrossRef]
- Jones, G.A. Elementary abelian regular coverings of Platonic maps. Case I: Ordinary representations. J. Algebr. Comb. 2015, 41, 461–491. [Google Scholar] [CrossRef]
- Feng, Y.Q.; Wang, K. s-Regular cyclic coverings of the three-dimensional hypercube Q3. Eur. J. Comb. 2003, 24, 719–731. [Google Scholar] [CrossRef][Green Version]
- Feng, Y.Q.; Kwak, J.H. s-Regular dihedral coverings of the complete graph of order 4. Chin. Ann. Math. B 2004, 25, 57–64. [Google Scholar] [CrossRef]
- Gramlich, R.; Hofmann, G.W.; Neeb, K.H. Semi-edges, reflections and Coxeter groups. Trans. Am. Math. Soc. 2007, 359, 3647–3668. [Google Scholar] [CrossRef][Green Version]
- Hofmeister, M. Enumeration of concrete regular covering projections. SIAM J. Discret. Math. 1995, 8, 51–61. [Google Scholar] [CrossRef]
- Kuzman, B.; Malnič, A.; Potočnik, P. Tetravalent vertex-and edge-transitive graphs over doubled cycles. J. Comb. Theory Ser. B 2018, 131, 109–137. [Google Scholar] [CrossRef]
- Li, C.H.; Zhu, Y.Z. Covers and pseudocovers of symmetric graphs. arXiv 2022, arXiv:2210.02679v1. [Google Scholar]
- Malnič, A.; Nedela, R.; Škoviera, M. Lifting graph automorphisms by voltage assignments. Eur. J. Comb. 2000, 21, 927–947. [Google Scholar] [CrossRef]
- Malnič, A.; Marušič, D.; Potočnik, P. Elementary abelian covers of graphs. J. Algebr. Comb. 2004, 20, 71–97. [Google Scholar] [CrossRef]
- Potočnik, P.; Požar, R. Smallest tetravalent half-arc-transitive graphs with the vertex-stabiliser isomorphic to the dihedral group of order 8. J. Comb. Theory Ser. A 2017, 145, 172–183. [Google Scholar] [CrossRef]
- Požar, R. Sectional split extensions arising from lifts of groups. Ars Math. Contemp. 2013, 6, 393–408. [Google Scholar] [CrossRef]
- Požar, R. Testing whether the lifted group splits. Ars Math. Contemp. 2016, 11, 147–156. [Google Scholar] [CrossRef][Green Version]
- Požar, R. Computing stable epimorphisms onto finite groups. J. Symb. Comput. 2019, 92, 22–30. [Google Scholar] [CrossRef]
- Požar, R. Fast computation of the centralizer of a permutation group in the symmetric group. J. Symb. Comput. 2024, 123, 102287. [Google Scholar] [CrossRef]
- Sato, I. Isomorphism of some graph coverings. Discret. Math. 1994, 128, 317–326. [Google Scholar] [CrossRef]
- Venkatesh, A. Graph Coverings and Group Liftings; preprint; Department of Mathematics, University of Western Australia: Perth, Australia, 1998. [Google Scholar]
- Xu, W.; Du, S.F.; Kwak, J.H.; Xu, M.Y. 2-Arc-transitive metacyclic covers of complete graphs. J. Comb. Theory Ser. B 2015, 111, 54–74. [Google Scholar] [CrossRef]
- Dörfler, W.; Harary, F.; Malle, G. Covers of digraphs. Math. Slovaca 1980, 30, 269–280. [Google Scholar]
- Fiala, J.; Seifrtová, M. A novel approach to covers of multigraphs with semiedges. Discuss. Math. Graph Theory 2024. in print. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Malnič, A.; Zgrablić, B. Coverings of Graphoids: Existence Theorem and Decomposition Theorems. Symmetry 2024, 16, 375. https://doi.org/10.3390/sym16030375
Malnič A, Zgrablić B. Coverings of Graphoids: Existence Theorem and Decomposition Theorems. Symmetry. 2024; 16(3):375. https://doi.org/10.3390/sym16030375
Chicago/Turabian StyleMalnič, Aleksander, and Boris Zgrablić. 2024. "Coverings of Graphoids: Existence Theorem and Decomposition Theorems" Symmetry 16, no. 3: 375. https://doi.org/10.3390/sym16030375
APA StyleMalnič, A., & Zgrablić, B. (2024). Coverings of Graphoids: Existence Theorem and Decomposition Theorems. Symmetry, 16(3), 375. https://doi.org/10.3390/sym16030375





