Exploring a Graph Complement in Quadratic Congruence
Abstract
1. Introduction
2. Results
- We call the subgraph a unit residue graph modulo if its vertex set is and ;
- We call the subgraph a quadratic residue graph modulo if its vertex set is reduced residue system mod such that two distinct vertices and are adjacent provided that . i.e., and ;
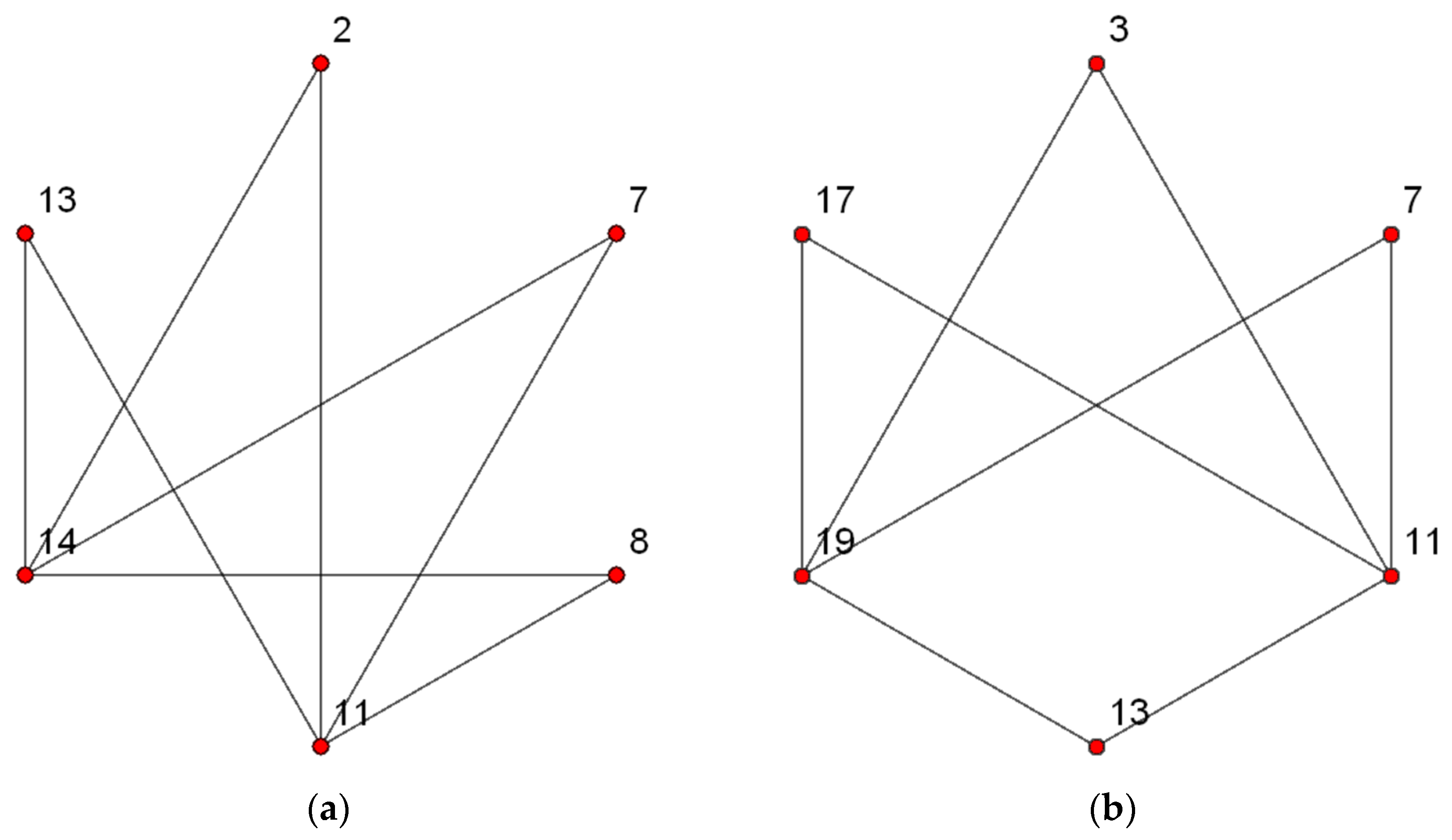
3. Computer Calculations for Some Integers
- The graph is regular but not complete.
- The graph is regular for values of and is not complete. However, for values of , it is complete. Notably, it is complete for certain composite values of such as
- The graph is not regular, thus it is not complete for any induced value of .
- In general, the variation of degrees of vertices in is not influenced by idempotent and nilpotent properties.
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
List of Symbols
| Symbol | Description |
| The ring of integers modulo . | |
| The group of units modulo . | |
| The group of quadratic residues modulo . | |
| The undirected graph of the ring . | |
| The undirected graph of the ring . | |
| The undirected graph of the ring . | |
| The undirected graph of the ring . | |
| The set of vertices in the undirected graph . | |
| The set of vertices in the undirected graph . | |
| The set of vertices in the undirected graph . | |
| The Euler totient function. | |
| . | |
| . | |
| . | |
| . | |
| The set of edges in the undirected graph . | |
| The set of edges in the undirected graph . | |
| The set of edges in the undirected graph . | |
| The degree of a vertex . | |
| The number roots of a quadratic residue. |
References
- Castillo, J.H.; Jhony, F.; Caranguay, M. The set of k-units modulo n. Involv. A J. Math. 2022, 15, 367–378. [Google Scholar] [CrossRef]
- Mateen, M.H.; Mahmood, M.K.; Ali, S.; Alam, M.D.A. On symmetry of complete graphs over quadratic and cubic residues. J. Chem. 2021, 2021, 4473637. [Google Scholar] [CrossRef]
- Ali, B.; Jahani-Nezhad, R. Unit and unitary Cayley graphs for the ring of Gaussian integers modulo n. Quasigroups Relat. Syst. 2017, 25, 189–200. [Google Scholar]
- Somer, L.; Michal, K. Structure of digraphs associated with quadratic congruences with composite moduli. Discret. Math. 2006, 306, 2174–2185. [Google Scholar] [CrossRef]
- Mateen, M.; Mahmmod, M.; Alghazzawi, D.; Liu, J.-B. Structures of power digraphs over the congruence equation xp ≡ y (mod m) and enumerations. AIMS Math. 2021, 6, 4581–4596. [Google Scholar] [CrossRef]
- Deng, G.; Pingzhi, Y. On the symmetric digraphs from powers modulo n. Discret. Math. 2012, 312, 720–728. [Google Scholar] [CrossRef]
- Fabrykowski, J. On quadratic residues and nonresidues in difference sets modulo m. Proc. Am. Math. Soc. 1994, 122, 325–331. [Google Scholar] [CrossRef][Green Version]
- Daoub, H. An Exposition of Graphs Related to Finite Commutative Rings. In Proceedings of the 1st International Conference of the Faculties of Sciences, Faculty of Science, Zawia, Libya, 20 December 2021; pp. 341–347. [Google Scholar]
- Rogers, T.D. The graph of the square mapping on the prime fields. Discret. Math. 1996, 148, 317–324. [Google Scholar] [CrossRef]
- Rezaei, M.; Rehman, S.; Khan, Z.; Baig, A.; Farahani, M. Quadratic residues graph. Int. J. Pure Appl. Math 2017, 113, 465–470. [Google Scholar] [CrossRef]
- Pirzada, S.; Altaf, A. Co-unit graphs associated to ring of integers modulo n. Acta Univ. Sapientiae Math. 2022, 14, 308–316. [Google Scholar] [CrossRef]
- Daoub, H.; Shafah, O. Graphs Related to Finite Abelian Groups. J. Fac. Educ. 2021, 24, 13–23. [Google Scholar]
- Dudley, U. Elementary Number Theory; Courier Corporation: Chelmsford, MA, USA, 2012. [Google Scholar]
- Jones, G.A.; Jones, J.M. Elementary Number Theory; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
| ϕ(n) | Idempotents | Nilpotents | |||||||
|---|---|---|---|---|---|---|---|---|---|
| 3 | 2 | {0,1} | {0} | 3 | 2 | 1 | {1,2} | {} | {} |
| 4 | 2 | {0,1} | {0,2} | 4 | 2 | 1 | {2} | {} | {} |
| 5 | 4 | {0,1} | {0} | 5 | 4 | 2 | {3,4} | {2} | {} |
| 6 | 2 | {0,1,3,4} | {0} | 6 | 2 | 1 | {4,5} | {} | {} |
| 7 | 6 | {0,1} | {0} | 7 | 6 | 3 | {5,6} | {4} | {2} |
| 8 | 4 | {0,1} | {0,4} | 8 | 4 | 1 | {4,6} | {} | {} |
| 9 | 6 | {0,1} | {0,3,6} | 9 | 6 | 3 | {6,7} | {4} | {2} |
| 10 | 4 | {0,1,5,6} | {0} | 10 | 4 | 2 | {8,9} | {2} | {} |
| 11 | 10 | {0,1} | {0} | 11 | 10 | 5 | {9,10} | {8} | {4} |
| 12 | 4 | {0,1,4,9} | {0,6} | 12 | 4 | 1 | {8,10} | {} | {} |
| 13 | 12 | {0,1} | {0} | 13 | 12 | 6 | {11,12} | {10} | {4} |
| 14 | 6 | {0,1,7,8} | {0} | 14 | 6 | 3 | {12,13} | {4} | {2} |
| 15 | 8 | {0,1,6,10} | {0} | 15 | 8 | 2 | {11,13,14} | {4} | {} |
| 16 | 8 | {0,1} | {0,4,8,12} | 16 | 8 | 2 | {12} | {4} | {} |
| 17 | 16 | {0,1} | {0} | 17 | 16 | 8 | {15,16} | {14} | {6} |
| 18 | 6 | {0,1,9,10} | {0,6,12} | 18 | 6 | 3 | {15,16} | {4} | {2} |
| 19 | 18 | {0,1} | {0} | 19 | 18 | 9 | {17,18} | {16} | {8} |
| 20 | 8 | {0,1,5,16} | {0,10} | 20 | 8 | 2 | {16,18} | {4} | {} |
| 21 | 12 | {0,1,7,15} | {0} | 21 | 12 | 3 | {17,19,20} | {8} | {2} |
| 22 | 10 | {0,1,11,12} | {0} | 22 | 10 | 5 | {20,21} | {8} | {4} |
| 23 | 22 | {0,1} | {0} | 23 | 22 | 11 | {21,22} | {20} | {10} |
| 24 | 8 | {0,1,9,16} | {0,12} | 24 | 8 | 1 | {16,20,22} | {} | {} |
| 25 | 20 | {0,1} | {0,5,10,15,20} | 25 | 20 | 10 | {20,23} | {18} | {8} |
| 26 | 12 | {0,1,13,14} | {0} | 26 | 12 | 6 | {24,25} | {10} | {4} |
| 27 | 18 | {0,1} | {0,9,18} | 27 | 18 | 9 | {21,24,25} | {16} | {8} |
| 28 | 12 | {0,1,8,21} | {0,14} | 28 | 12 | 3 | {24,26} | {8} | {2} |
| 29 | 28 | {0,1} | {0} | 29 | 28 | 14 | {27,28} | {26} | {12} |
| 30 | 8 | {0,1,6,10,15,16,21,25} | {0} | 30 | 8 | 2 | {26,28,29} | {4} | {} |
| 31 | 30 | {0,1} | {0} | 31 | 30 | 15 | {29,30} | {28} | {14} |
| 32 | 16 | {0,1} | {0,8,16,24} | 32 | 16 | 4 | {24,28} | {12} | {2} |
| 33 | 20 | {0,1,12,22} | {0} | 33 | 20 | 5 | {29,31,32} | {16} | {4} |
| 34 | 16 | {0,1,17,18} | {0} | 34 | 16 | 8 | {32,33} | {14} | {6} |
| 35 | 24 | {0,1,15,21} | {0} | 35 | 24 | 6 | {31,33,34} | {20} | {4} |
| 36 | 12 | {0,1,9,28} | {0,6,12,18,24,30} | 36 | 12 | 3 | {30,32} | {8} | {2} |
| 37 | 36 | {0,1} | {0} | 37 | 36 | 18 | {35,36} | {34} | {16} |
| 38 | 18 | {0,1,19,20} | {0} | 38 | 18 | 9 | {36,37} | {16} | {8} |
| 39 | 24 | {0,1,13,27} | {0} | 39 | 24 | 6 | {35,37,38} | {20} | {4} |
| 40 | 16 | {0,1,16,25} | {0,20} | 40 | 16 | 2 | {32,36,38} | {8} | {} |
| 41 | 40 | {0,1} | {0} | 41 | 40 | 20 | {39,40} | {38} | {18} |
| 42 | 12 | {0,1,7,15,21,22,28,36} | {0} | 42 | 12 | 3 | {38,40,41} | {8} | {2} |
| 43 | 42 | {0,1} | {0} | 43 | 42 | 21 | {41,42} | {40} | {20} |
| 44 | 20 | {0,1,12,33} | {0,22} | 44 | 20 | 5 | {40,42} | {16} | {4} |
| 45 | 24 | {0,1,10,36} | {0,15,30} | 45 | 24 | 6 | {39,41,42,43} | {20} | {4} |
| 46 | 22 | {0,1,23,24} | {0} | 46 | 22 | 11 | {44,45} | {20} | {10} |
| ϕ(n) | Idempotents | Nilpotents | |||||||
|---|---|---|---|---|---|---|---|---|---|
| 47 | 46 | {0,1} | {0} | 47 | 46 | 23 | {45,46} | {44} | {22} |
| 48 | 16 | {0,1,16,33} | {0,12,24,36} | 48 | 16 | 2 | {40,44} | {8} | {} |
| 49 | 42 | {0,1} | {0,7,14,21,28,35,42} | 49 | 42 | 21 | {42,47} | {40} | {20} |
| 50 | 20 | {0,1,25,26} | {0,10,20,30,40} | 50 | 20 | 10 | {45,48} | {18} | {8} |
| 51 | 32 | {0,1,18,34} | {0} | 51 | 32 | 8 | {47,49,50} | {28} | {6} |
| 52 | 24 | {0,1,13,40} | {0,26} | 52 | 24 | 6 | {48,50} | {20} | {4} |
| 53 | 52 | {0,1} | {0} | 53 | 52 | 26 | {51,52} | {50} | {24} |
| 54 | 18 | {0,1,27,28} | {0,18,36} | 54 | 18 | 9 | {48,51,52} | {16} | {8} |
| 55 | 40 | {0,1,11,45} | {0} | 55 | 40 | 10 | {51,53,54} | {36} | {8} |
| 56 | 24 | {0,1,8,49} | {0,28} | 56 | 24 | 3 | {48,52,54} | {16} | {2} |
| 57 | 36 | {0,1,19,39} | {0} | 57 | 36 | 9 | {53,55,56} | {32} | {8} |
| 58 | 28 | {0,1,29,30} | {0} | 58 | 28 | 14 | {56,57} | {26} | {12} |
| 59 | 58 | {0,1} | {0} | 59 | 58 | 29 | {57,58} | {56} | {28} |
| 60 | 16 | {0,1,16,21,25,36,40,45} | {0,30} | 60 | 16 | 2 | {52,56,58} | {8} | {} |
| 61 | 60 | {0,1} | {0} | 61 | 60 | 30 | {59,60} | {58} | {28} |
| 62 | 30 | {0,1,31,32} | {0} | 62 | 30 | 15 | {60,61} | {28} | {14} |
| 63 | 36 | {0,1,28,36} | {0,21,42} | 63 | 36 | 9 | {57,59,60,61} | {32} | {8} |
| 64 | 32 | {0,1} | {0,8,16,24,32,40,48,56} | 64 | 32 | 8 | {56,60} | {28} | {6} |
| 65 | 48 | {0,1,26,40} | {0} | 65 | 48 | 12 | {61,63,64} | {44} | {8} |
| 66 | 20 | {0,1,12,22,33,34,45,55} | {0} | 66 | 20 | 5 | {62,64,65} | {16} | {4} |
| 67 | 66 | {0,1} | {0} | 67 | 66 | 33 | {65,66} | {64} | {32} |
| 68 | 32 | {0,1,17,52} | {0,34} | 68 | 32 | 8 | {64,66} | {28} | {6} |
| 69 | 44 | {0,1,24,46} | {0} | 69 | 44 | 11 | {65,67,68} | {40} | {10} |
| 70 | 24 | {0,1,15,21,35,36,50,56} | {0} | 70 | 24 | 6 | {66,68,69} | {20} | {4} |
| 71 | 70 | {0,1} | {0} | 71 | 70 | 35 | {69,70} | {68} | {34} |
| 72 | 24 | {0,1,9,64} | {0,12,24,36,48,60} | 72 | 24 | 3 | {60,64,66,68} | {16} | {2} |
| 73 | 72 | {0,1} | {0} | 73 | 72 | 36 | {71,72} | {70} | {34} |
| 74 | 36 | {0,1,37,38} | {0} | 74 | 36 | 18 | {72,73} | {34} | {16} |
| 75 | 40 | {0,1,25,51} | {0,15,30,45,60} | 75 | 40 | 10 | {65,70,71,73} | {36} | {8} |
| 76 | 36 | {0,1,20,57} | {0,38} | 76 | 36 | 9 | {72,74} | {32} | {8} |
| 77 | 60 | {0,1,22,56} | {0} | 77 | 60 | 15 | {73,75,76} | {56} | {14} |
| 78 | 24 | {0,1,13,27,39,40,52,66} | {0} | 78 | 24 | 6 | {74,76,77} | {20} | {4} |
| 79 | 78 | {0,1} | {0} | 79 | 78 | 39 | {77,78} | {76} | {38} |
| 80 | 32 | {0,1,16,65} | {0,20,40,60} | 80 | 32 | 4 | {72,76} | {24} | {} |
| 81 | 54 | {0,1} | {0,9,18,27,36,45,54,63,72} | 81 | 54 | 27 | {72,75,79} | {52} | {26} |
| 82 | 40 | {0,1,41,42} | {0} | 82 | 40 | 20 | {80,81} | {38} | {18} |
| 83 | 82 | {0,1} | {0} | 83 | 82 | 41 | {81,82} | {80} | {40} |
| 84 | 24 | {0,1,21,28,36,49,57,64} | {0,42} | 84 | 24 | 3 | {76,80,82} | {16} | {2} |
| 85 | 64 | {0,1,35,51} | {0} | 85 | 64 | 16 | {81,83,84} | {60} | {12} |
| ϕ(n) | Idempotents | Nilpotents | |||||||
|---|---|---|---|---|---|---|---|---|---|
| 86 | 42 | {0,1,43,44} | {0} | 86 | 42 | 21 | {84,85} | {40} | {20} |
| 87 | 56 | {0,1,30,58} | {0} | 87 | 56 | 14 | {83,85,86} | {52} | {12} |
| 88 | 40 | {0,1,33,56} | {0,44} | 88 | 40 | 5 | {80,84,86} | {32} | {4} |
| 89 | 88 | {0,1} | {0} | 89 | 88 | 44 | {87,88} | {86} | {42} |
| 90 | 24 | {0,1,10,36,45,46,55,81} | {0,30,60} | 90 | 24 | 6 | {84,86,87,88} | {20} | {4} |
| 91 | 72 | {0,1,14,78} | {0} | 91 | 72 | 18 | {87,89,90} | {68} | {16} |
| 92 | 44 | {0,1,24,69} | {0,46} | 92 | 44 | 11 | {88,90} | {40} | {10} |
| 93 | 60 | {0,1,31,63} | {0} | 93 | 60 | 15 | {89,91,92} | {56} | {14} |
| 94 | 46 | {0,1,47,48} | {0} | 94 | 46 | 23 | {92,93} | {44} | {22} |
| 95 | 72 | {0,1,20,76} | {0} | 95 | 72 | 18 | {91,93,94} | {68} | {16} |
| 96 | 32 | {0,1,33,64} | {0,24,48,72} | 96 | 32 | 4 | {80,88,92} | {24} | {2} |
| 97 | 96 | {0,1} | {0} | 97 | 96 | 48 | {95,96} | {94} | {46} |
| 98 | 42 | {0,1,49,50} | {0,14,28,42,56,70,84} | 98 | 42 | 21 | {91,96} | {40} | {20} |
| 99 | 60 | {0,1,45,55} | {0,33,66} | 99 | 60 | 15 | {93,95,96,97} | {56} | {14} |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Daoub, H.; Shafah, O.; Almutlg, A.A. Exploring a Graph Complement in Quadratic Congruence. Symmetry 2024, 16, 213. https://doi.org/10.3390/sym16020213
Daoub H, Shafah O, Almutlg AA. Exploring a Graph Complement in Quadratic Congruence. Symmetry. 2024; 16(2):213. https://doi.org/10.3390/sym16020213
Chicago/Turabian StyleDaoub, Hamza, Osama Shafah, and Ahmad A. Almutlg. 2024. "Exploring a Graph Complement in Quadratic Congruence" Symmetry 16, no. 2: 213. https://doi.org/10.3390/sym16020213
APA StyleDaoub, H., Shafah, O., & Almutlg, A. A. (2024). Exploring a Graph Complement in Quadratic Congruence. Symmetry, 16(2), 213. https://doi.org/10.3390/sym16020213








