Symmetric Properties of λ-Szász Operators Coupled with Generalized Beta Functions and Approximation Theory
Abstract
1. Introduction
2. Some Estimates and Approximation Results
3. Graphical and Numerical Analysis
4. Local Approximation
5. Bivariate Extension of Generalized Beta Type -Szász–Mirakjan Operators
6. Bivariate Graphical Analysis
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
References
- Szász, O. Generalization of S. Bernstein’s polynomials to the infinite interval. Res. Nat. Bur. Stand. 1950, 45, 239–245. [Google Scholar] [CrossRef]
- Bernšteın, S. Demonstration du théoreme de Weierstrass fondée sur le calcul des probabilities. Commum. Soc. Math. Kharkov. 1912, 13, 1–2. [Google Scholar]
- Izadbakhsh, A.; Kalat, A.A.; Khorashadizadeh, S. Observer-based adaptive control for HIV infection therapy using the Baskakov operator. Biomed. Signal Process. Control 2021, 65, 102343. [Google Scholar] [CrossRef]
- Uyan, H.; Aslan, A.O.; Karateke, S.; Buyukyazıcı, I. Interpolation for neural network operators activated with a generalized logistic-type function. J. Inequal. Appl. 2024, 125, 31. [Google Scholar] [CrossRef]
- Zhang, Q.; Mu, M.; Wang, X. A Modified Robotic Manipulator Controller Based on Bernstein-Kantorovich-Stancu Operator. Micromachines 2022, 14, 44. [Google Scholar] [CrossRef] [PubMed]
- Khan, K.; Lobiyal, D.K. Bezier curves based on Lupas (p,q)-analogue of Bernstein functions in CAGD. Comput. Appl. Math. 2017, 317, 458–477. [Google Scholar] [CrossRef]
- Rao, N.; Farid, M.; Ali, R. A Study of Szász–Durremeyer-Type Operators Involving Adjoint Bernoulli Polynomials. Mathematics 2024, 12, 3645. [Google Scholar] [CrossRef]
- Braha, N.L.; Mansour, T.; Mursaleen, M. Some Approximation Properties of Parametric Baskakov–Schurer–Szász Operators Through a Power Series Summability Method. Complex Anal. Oper. Theory 2024, 18, 71. [Google Scholar] [CrossRef]
- Özger, F.; Demiric, K. Approximation by Kantorovich Variant of λ—Schurer Operators and Related Numerical Results. In Topics in Contemporary Mathematics, Analysis and Applications; CRC Press: Boca Raton, FL, USA, 2020; pp. 77–94. [Google Scholar]
- Ansari, K.J.; Özger, F.; Ödemiş, Ö.Z. Numerical and theoretical approximation results for Schurer–Stancu operators with shape parameter λ. Comput. Appl. Math. 2022, 41, 181. [Google Scholar] [CrossRef]
- Khan, A.; Iliyas, M.; Khan, K.; Mursaleen, M. Approximation of conic sections by weighted Lupaş post-quantum Bézier curves. Demonstr. Math. 2022, 55, 328–342. [Google Scholar] [CrossRef]
- Acar, T.; Mursaleen, M.; Mohiuddine, S.A. Stancu type (p, q)-Szász-Mirakyan-Baskakov operators. Commun. Fac. Sci. Univ. Ank. Ser. Math. Stat. 2018, 67, 116–128. [Google Scholar]
- Alotaibi, A. Approximation of GBS type q-Jakimovski-Leviatan-Beta integral operators in Bögel space. Mathematics 2022, 10, 675. [Google Scholar] [CrossRef]
- Mohiuddine, S.A.; Singh, K.K.; Alotaibi, A. On the order of approximation by modified summation-integral-type operators based on two parameters. Demonstr. Math. 2023, 8, 20220182. [Google Scholar] [CrossRef]
- Nasiruzzaman, M.; Rao, N.; Kumar, M.; Kumar, R. Approximation on bivariate parametric extension of Baskakov-Durrmeyer-opeator. Filomat 2021, 35, 2783–2800. [Google Scholar] [CrossRef]
- Çiçek, H.; İzgi, A. Approximation by modified bivariate Bernstein-Durrmeyer and GBS bivariate Bernstein-Durrmeyer operators on a triangular region. Fund. J. Math. Appl. 2022, 5, 135–144. [Google Scholar] [CrossRef]
- Cai, Q.-B.; Aslan, R.; Özger, F.; Srivastava, H.M. Approximation by a new Stancu variant of generalized (λ, μ)-Bernstein operators. Alex. Eng. J. 2024, 107, 205–214. [Google Scholar] [CrossRef]
- Aslan, R. Rate of approximation of blending type modified univariate and bivariate λ-Schurer-Kantorovich operators. Kuwait J. Sci. 2024, 51, 100168. [Google Scholar] [CrossRef]
- Rao, N.; Ayman-Mursaleen, M.; Aslan, R. A note on a general sequence of λ-Szász Kantorovich type operators. Comput. Appl. Math. 2024, 43, 428. [Google Scholar] [CrossRef]
- Izgi, A.; Serenbay, S.K. Approximation by complex Chlodowsky-Szász-Durrmeyer operators in compact disks. Creat. Math. Inform. 2020, 29, 37–44. [Google Scholar] [CrossRef]
- Qi, Q.; Guo, D.; Yang, G. Approximation properties of λ-Szász-Mirakian operators. Int. J. Eng. Res. 2019, 12, 662–669. [Google Scholar]
- Păltănea, R. A class of Durrmeyer type operators preserving linear functions. Ann. Tiberiu Popoviciu Sem. Funct. Equat. Approxim. Convex. 2007, 5, 109–117. [Google Scholar]
- Rao, N.; Raiz, M.; Mursaleen, M.A.; Mishra, V.N. Approximation properties of Generalized beta-type Szász–Mirakjan operators. Iran. J. Sci. 2023, 47, 1771–1781. [Google Scholar] [CrossRef]
- DeVore, R.A.; Lorentz, G.G. Constructive Approximation. In Grundlehren der Mathematischen Wissenschaften; Springer: Berlin/Heidelberg, Germany, 1993; p. 303. [Google Scholar]
- Altomare, F.; Campiti, M. Korovkin-Type Approximation Theory and Its Applications; Appendix A by Michael Pannenberg and Appendix B by Ferdinand Beckhoff; de Gruyter Studies in Mathematics; Walter de Gruyter and Co.: Berlin, Germany, 1994. [Google Scholar]
- Özarslan, M.A.; Aktuglu, H. Local approximation for certain King type operators. Filomat 2013, 27, 173–181. [Google Scholar] [CrossRef]
- Lenze, B. On Lipschitz type maximal functions and their smoothness spaces. Nederl. Akad. Indag. Math. 1988, 50, 53–63. [Google Scholar] [CrossRef]
- Volkov, V.I. On the convergence of sequences of linear positive operators in the space of continuous functions of two variables. Dokl. Akad. Nauk SSSR (NS) 1957, 115, 17–19. (In Russian) [Google Scholar]
- Stancu, F. Apoximarea Funcțiilor de Două și mai Multe Variabile Prin Șiruri de Operatori Liniari și Pozitivi. Ph.D. Thesis, Cluj-Napoca, Romania, 1984. (In Romanian). [Google Scholar]
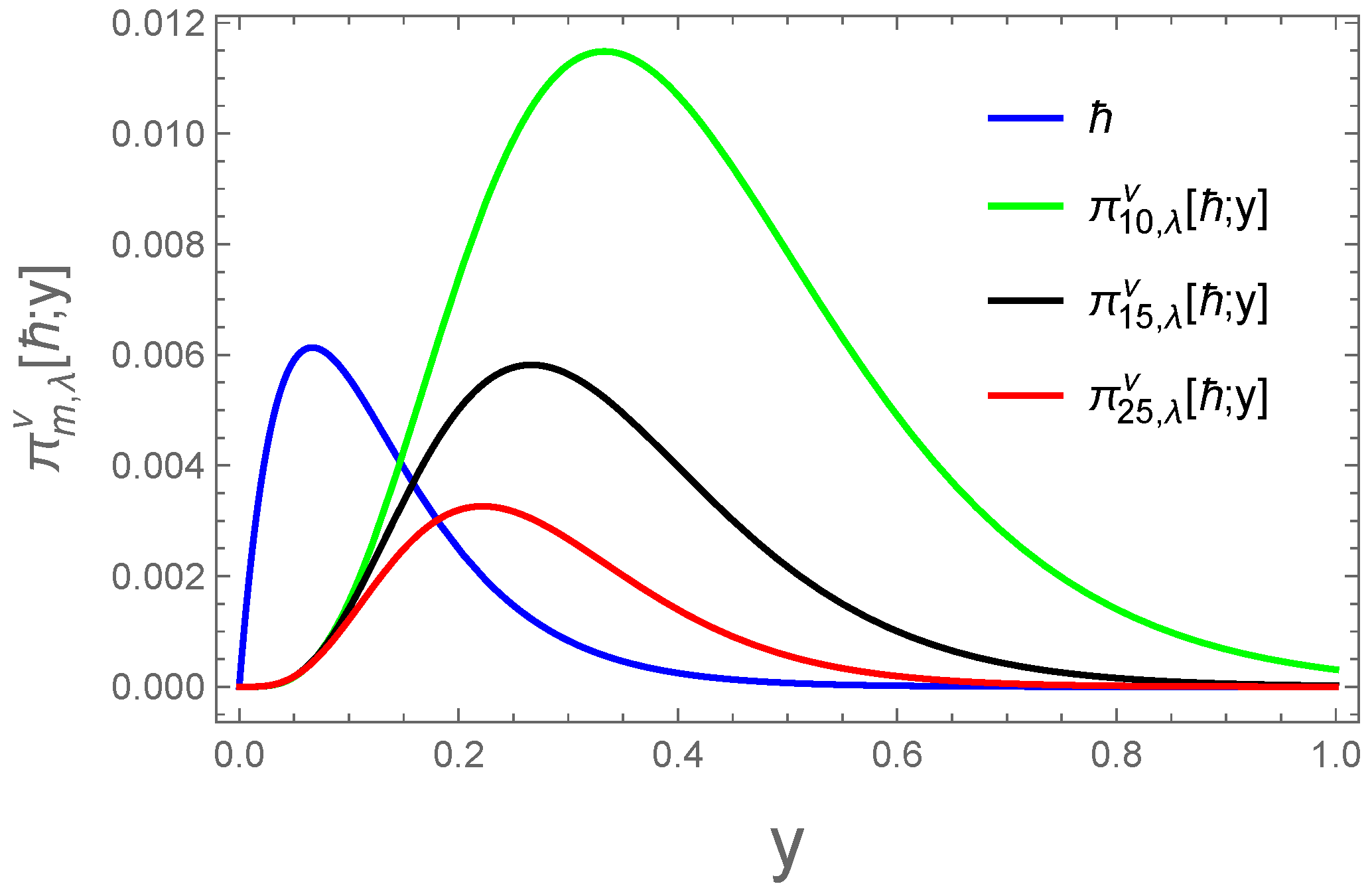


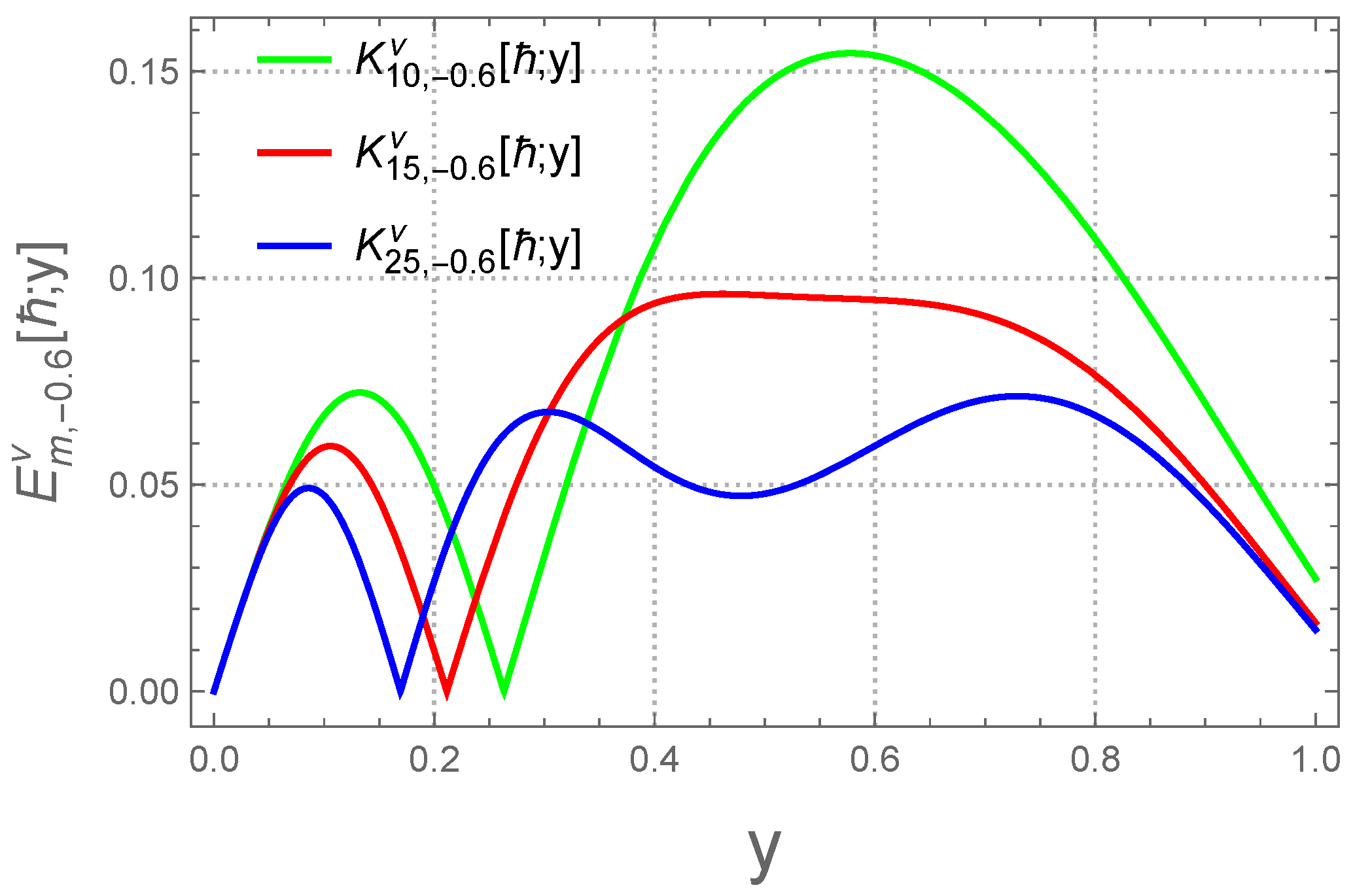

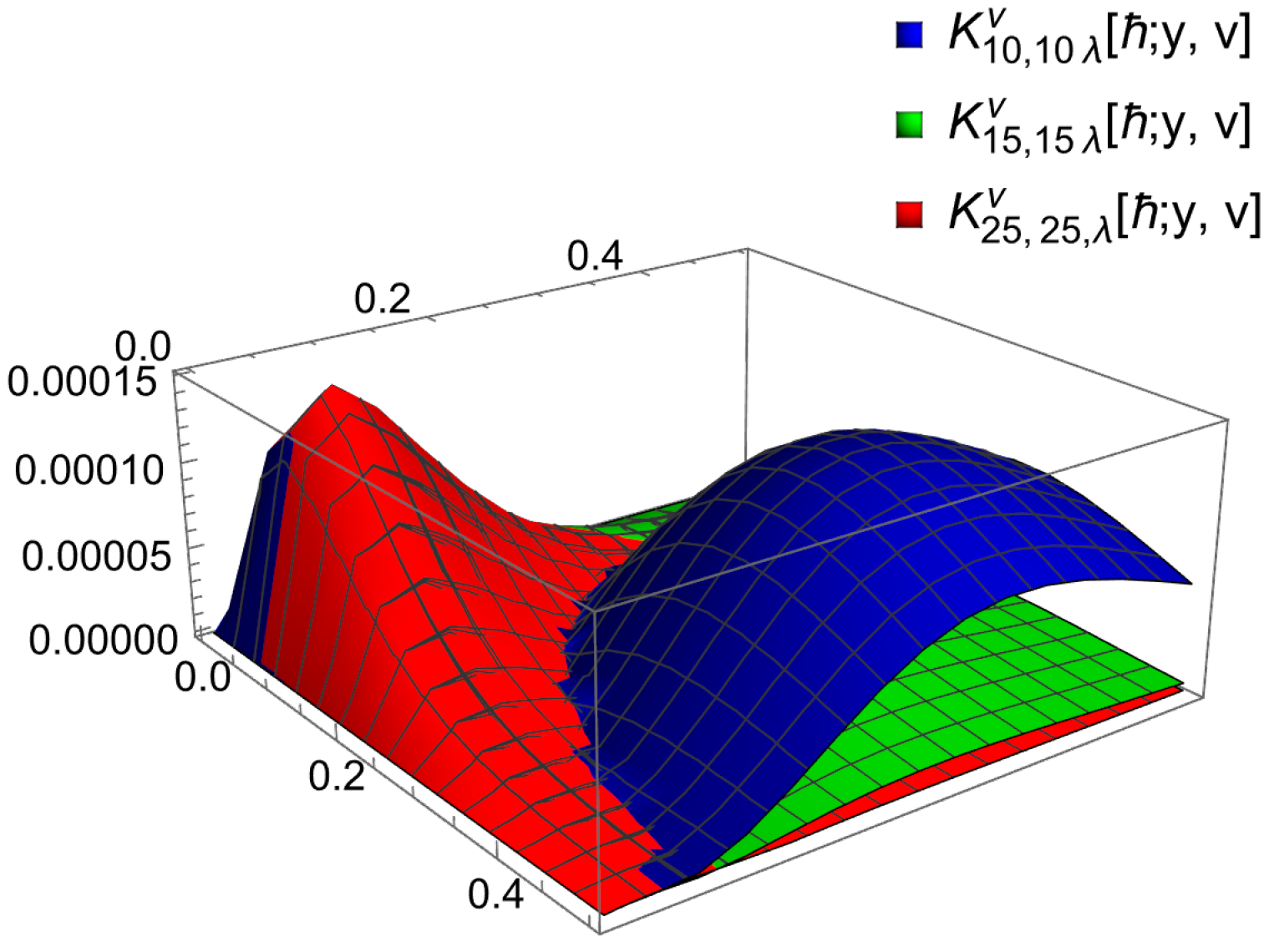
| y | |||
|---|---|---|---|
| 0.1 | 0.00855716 | 0.00868693 | 0.00888307 |
| 0.2 | 0.00331262 | 0.000933993 | 0.000881886 |
| 0.3 | 0.010011 | 0.00441485 | 0.0014342 |
| 0.4 | 0.0103584 | 0.00364727 | 0.00106068 |
| 0.5 | 0.00776931 | 0.00208241 | 0.000478021 |
| 0.6 | 0.00488011 | 0.000981387 | 0.000172209 |
| 0.7 | 0.00272745 | 0.00040907 | 0.000054154 |
| 0.8 | 0.00140172 | 0.000156346 | 0.0000155106 |
| 0.9 | 0.000676062 | 0.0000559933 | 4.14678 × |
| y | |||
|---|---|---|---|
| 0.1 | 0.0668425 | 0.05911693 | 0.0473892 |
| 0.2 | 0.0496822 | 0.00973307 | 0.0267445 |
| 0.3 | 0.032826 | 0.0654061 | 0.0.0675773 |
| 0.4 | 0.107994 | 0.0938944 | 0.0542833 |
| 0.5 | 0.146655 | 0.0958518 | 0.0478268 |
| 0.6 | 0.153884 | 0.0947532 | 0.000172209 |
| 0.7 | 0.13949 | 0.0908188 | 0.0706674 |
| 0.8 | 0.109585 | 0.0765646 | 0.0667936 |
| 0.9 | 0.0696738 | 0.0499619 | 0.0457327 |
| 0.1 0.1 | 0.000124468 | 2.3644 × | 1.46835 × |
| 0.2 0.2 | 0.000054595 | 0.0000251022 | 0.0000102038 |
| 0.3 0.3 | 0.000126461 | 0.0000319147 | 7.12176 × |
| 0.4 0.4 | 0.000114291 | 0.0000158371 | 1.94043 × |
| 0.5 0.5 | 0.0000616724 | 4.69277 × | 3.15716 × |
| 0.6 0.6 | 0.0000240142 | 1.00341 × | 3.70668 × |
| 0.7 0.7 | 7.46513 × | 1.71277 × | 3.47392 × |
| 0.8 0.8 | 1.96793 × | 2.47905 × | 2.76057 × |
| 0.9 0.9 | 4.574 × | 3.1634 × | 1.9339 × |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rao, N.; Farid, M.; Raiz, M. Symmetric Properties of λ-Szász Operators Coupled with Generalized Beta Functions and Approximation Theory. Symmetry 2024, 16, 1703. https://doi.org/10.3390/sym16121703
Rao N, Farid M, Raiz M. Symmetric Properties of λ-Szász Operators Coupled with Generalized Beta Functions and Approximation Theory. Symmetry. 2024; 16(12):1703. https://doi.org/10.3390/sym16121703
Chicago/Turabian StyleRao, Nadeem, Mohammad Farid, and Mohd Raiz. 2024. "Symmetric Properties of λ-Szász Operators Coupled with Generalized Beta Functions and Approximation Theory" Symmetry 16, no. 12: 1703. https://doi.org/10.3390/sym16121703
APA StyleRao, N., Farid, M., & Raiz, M. (2024). Symmetric Properties of λ-Szász Operators Coupled with Generalized Beta Functions and Approximation Theory. Symmetry, 16(12), 1703. https://doi.org/10.3390/sym16121703







