Robustness of the Proxy-SU(3) Symmetry in Atomic Nuclei and the Role of the Next-Highest-Weight Irreducible Representation
Abstract
:1. Introduction
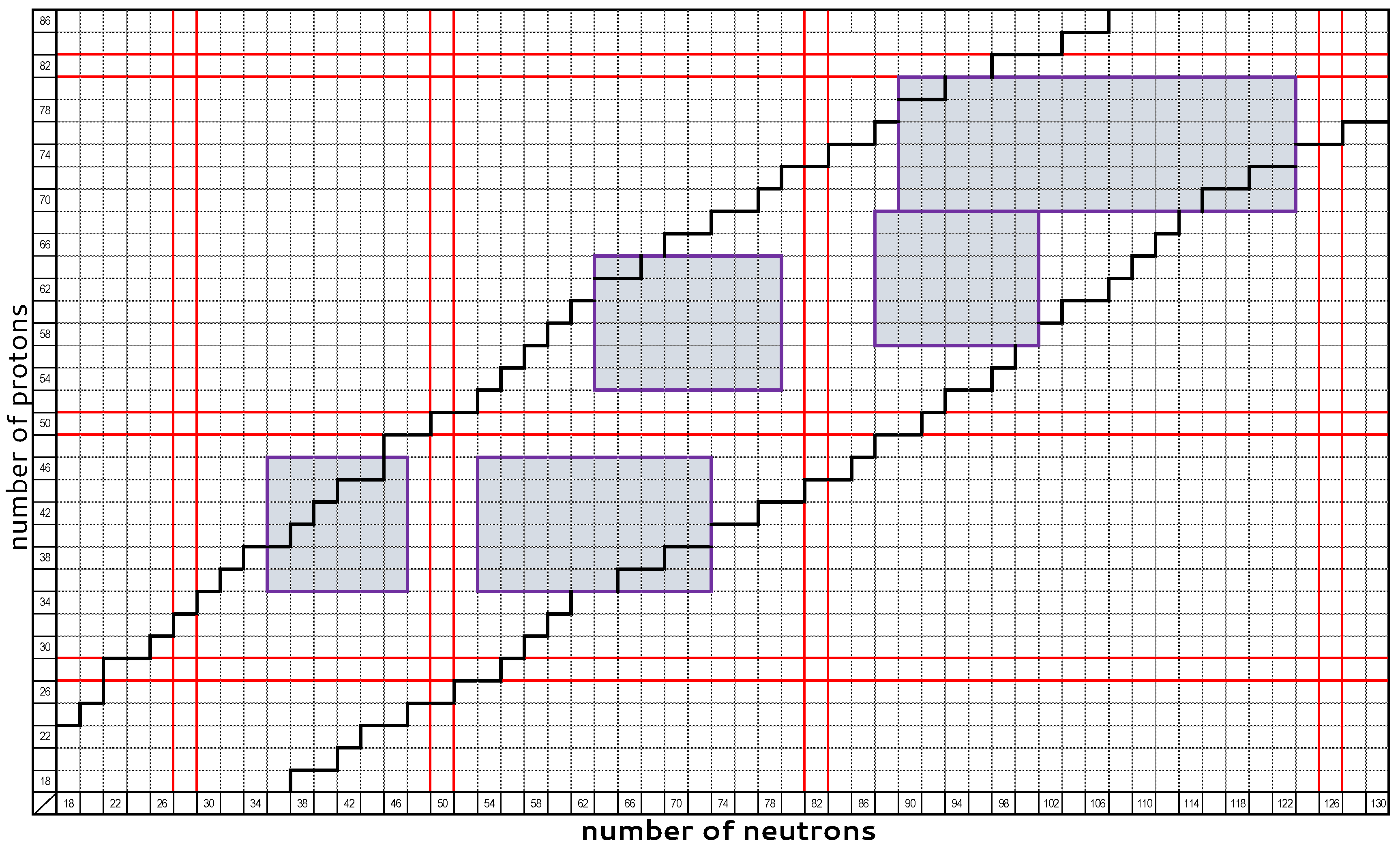
2. Highest Weight Irreducible Representations
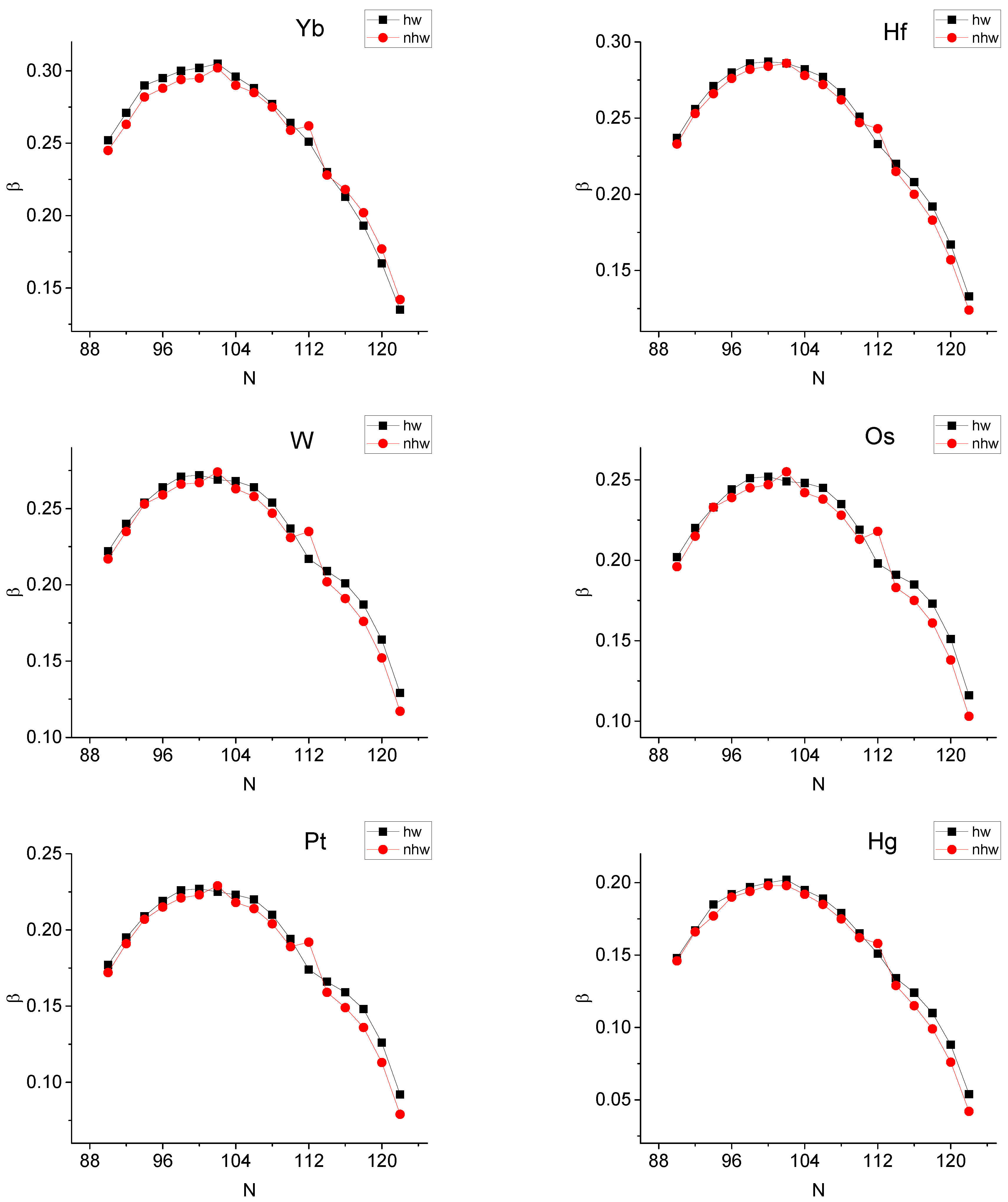
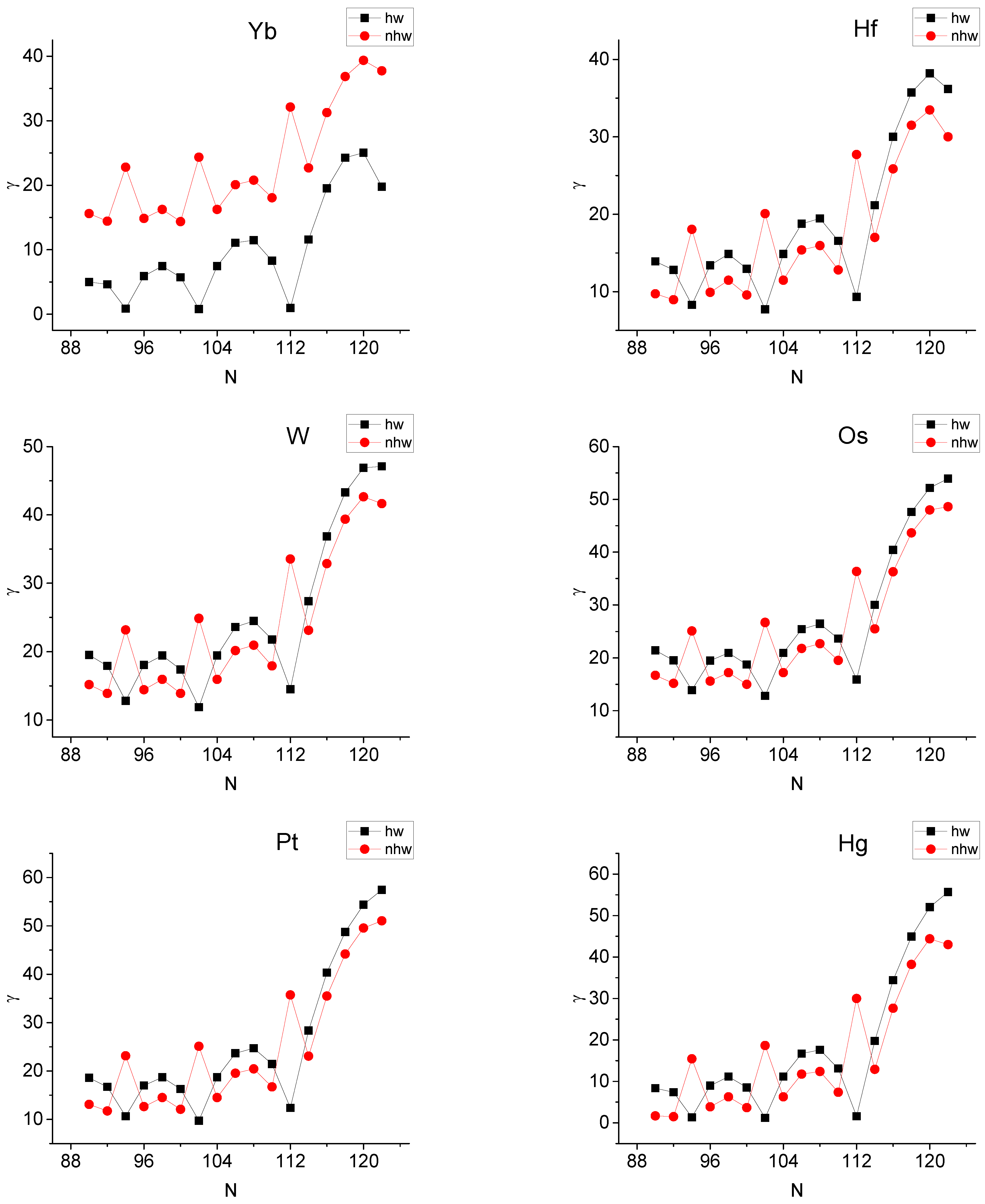
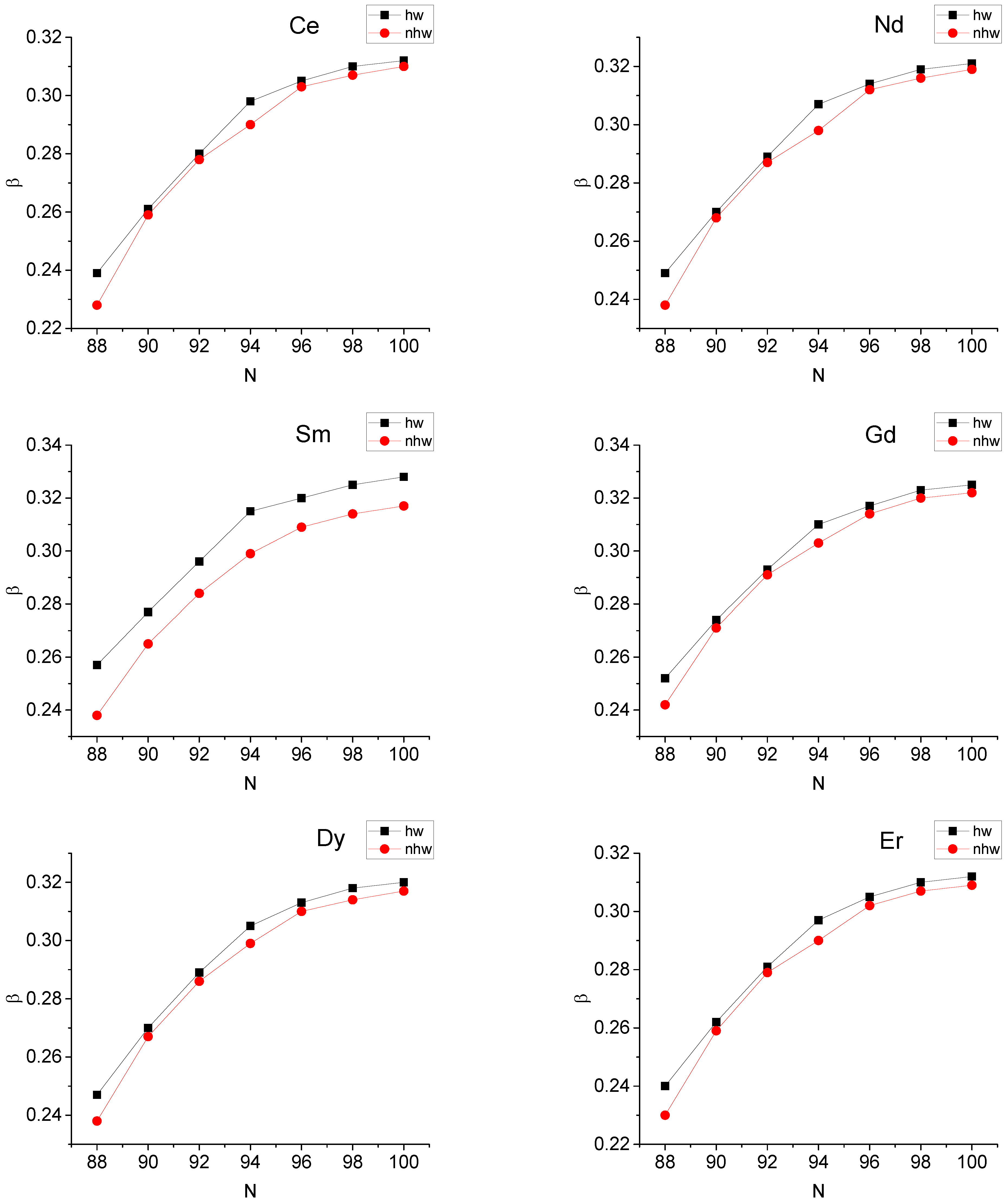
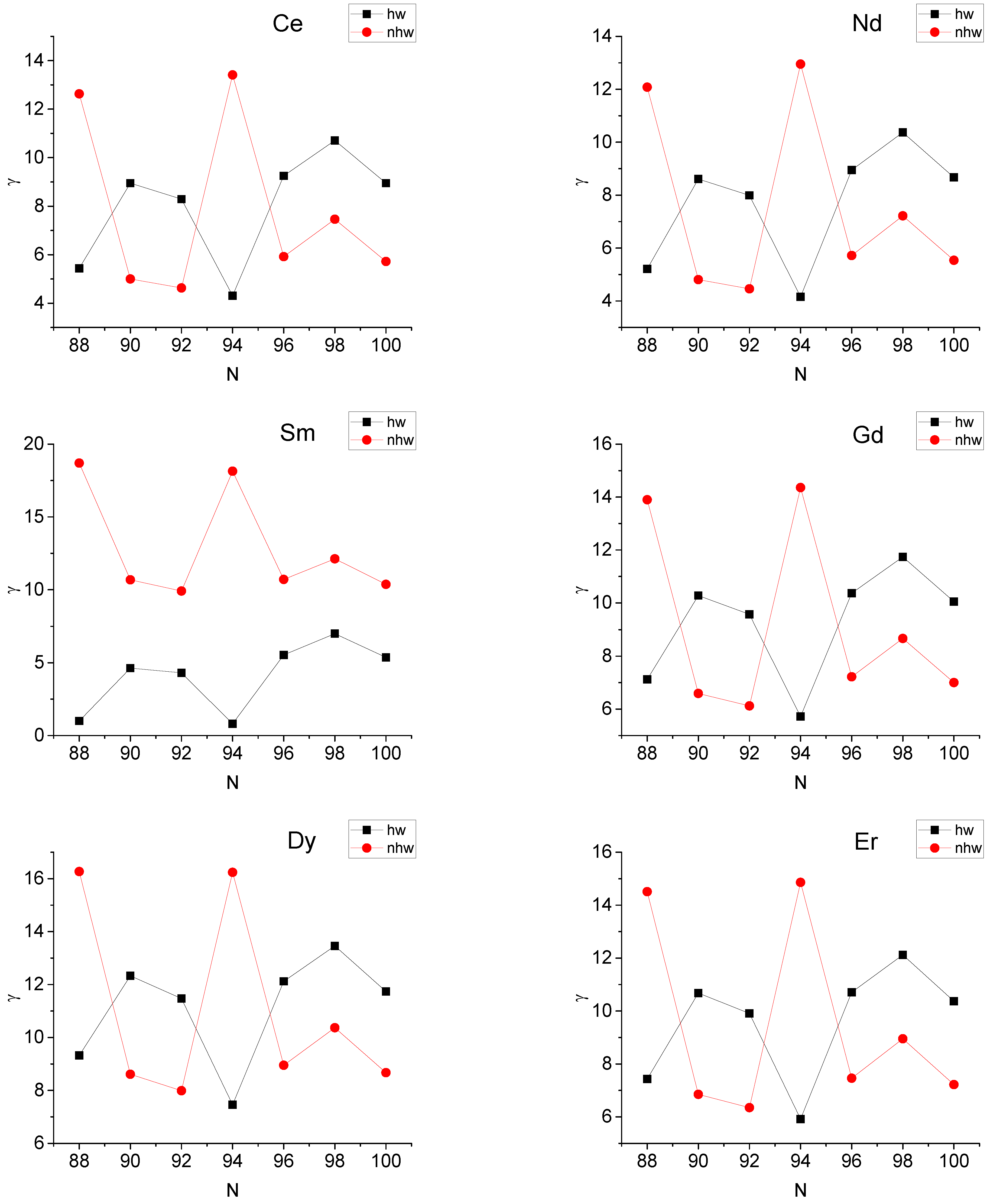
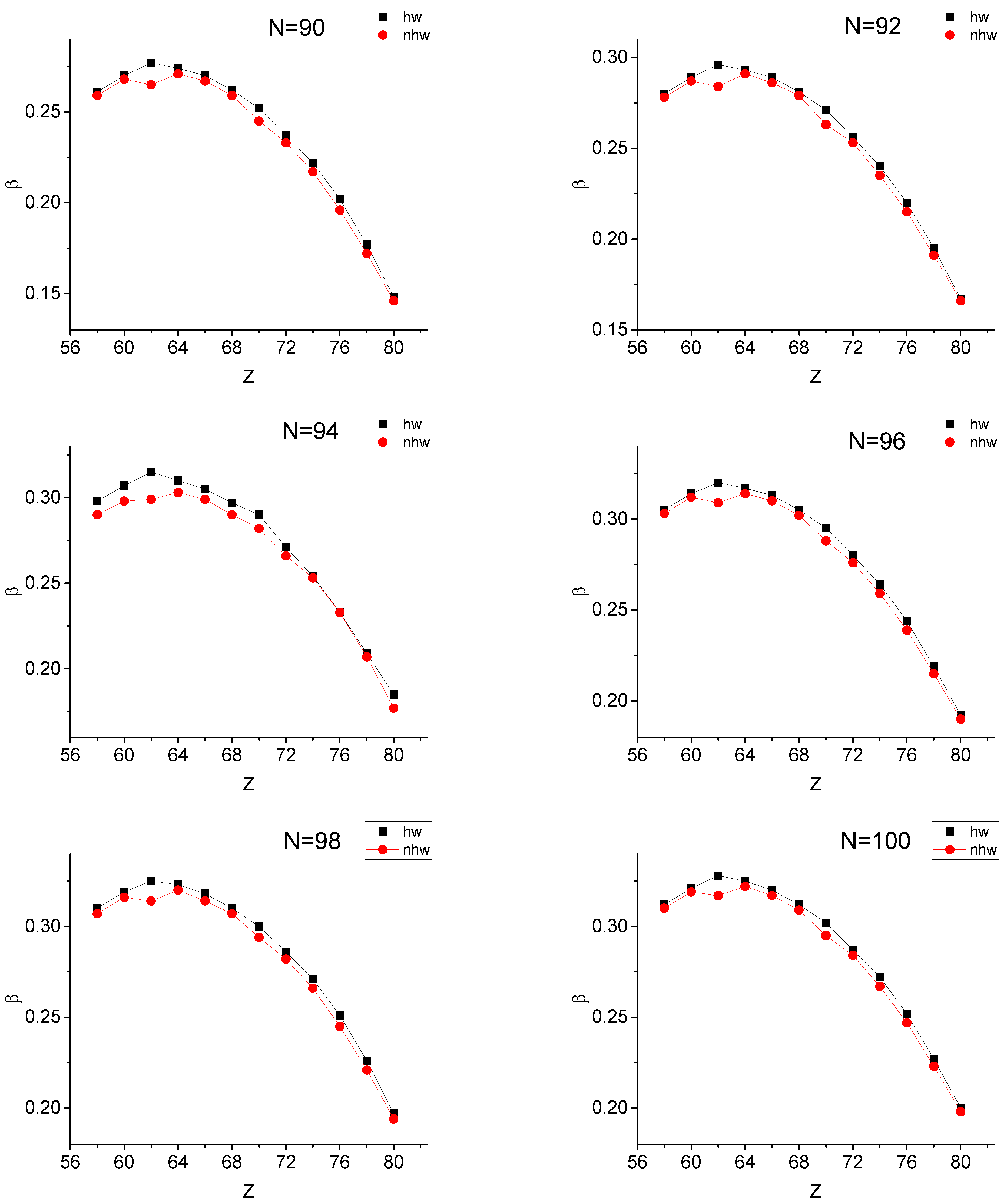
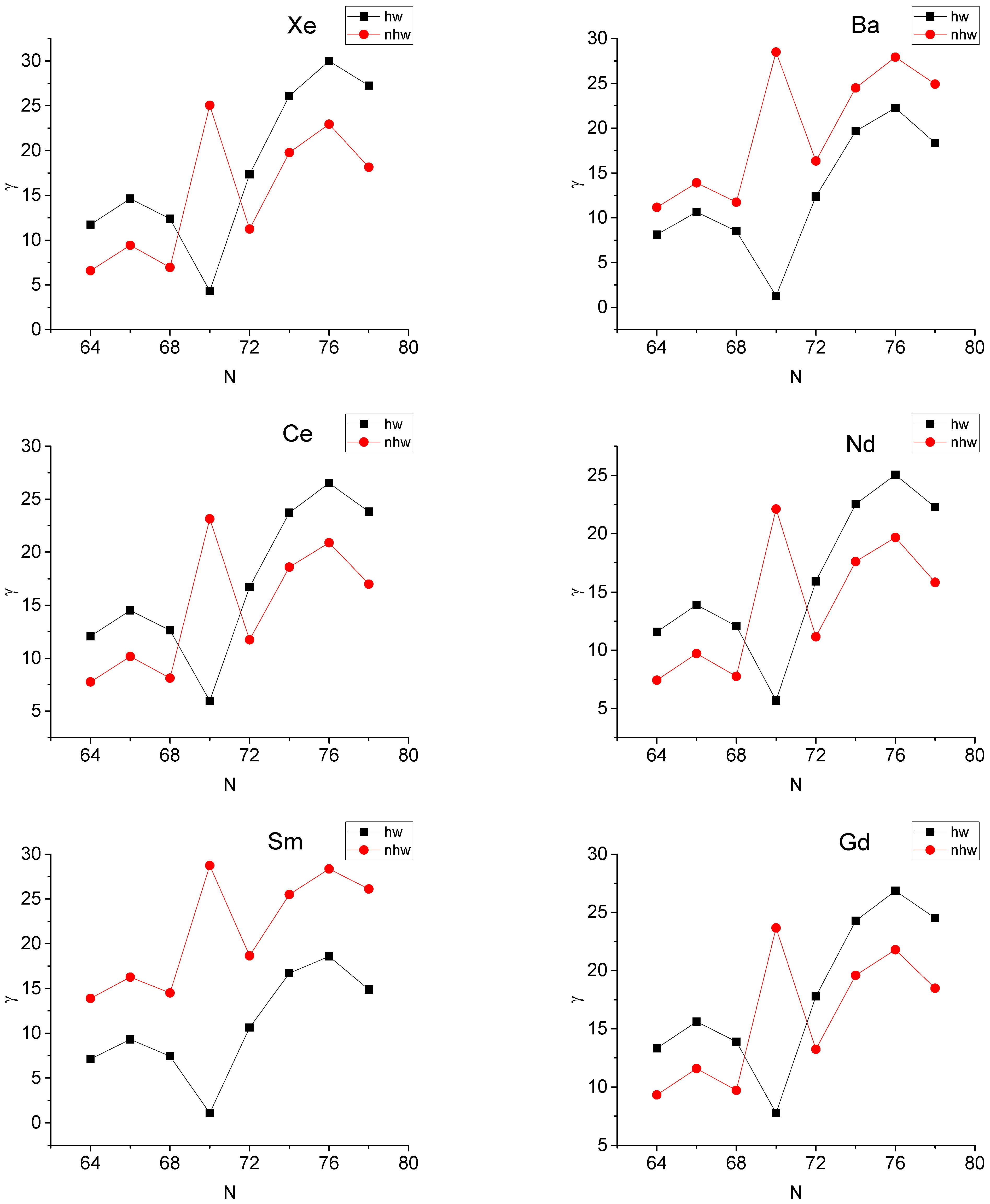
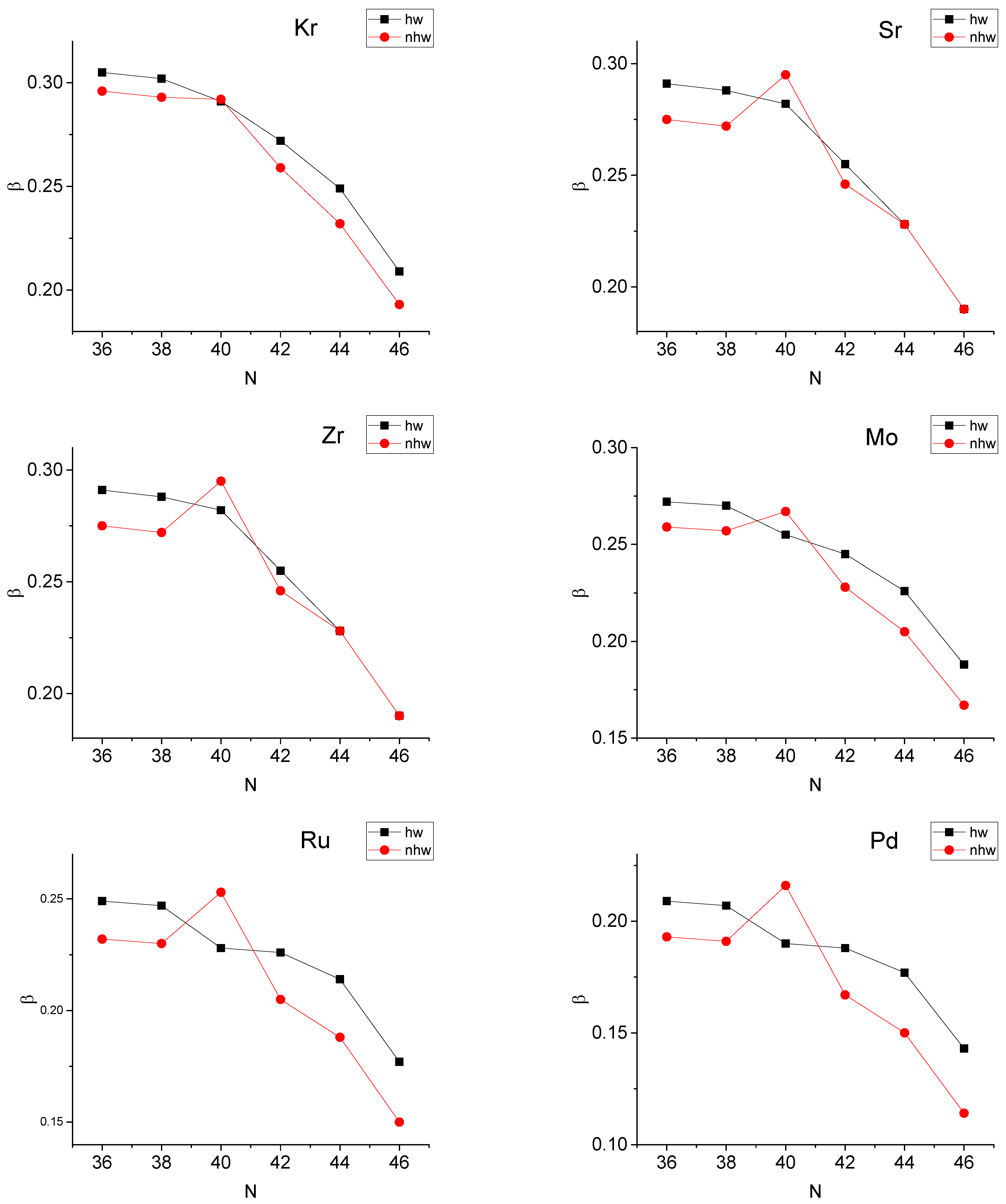
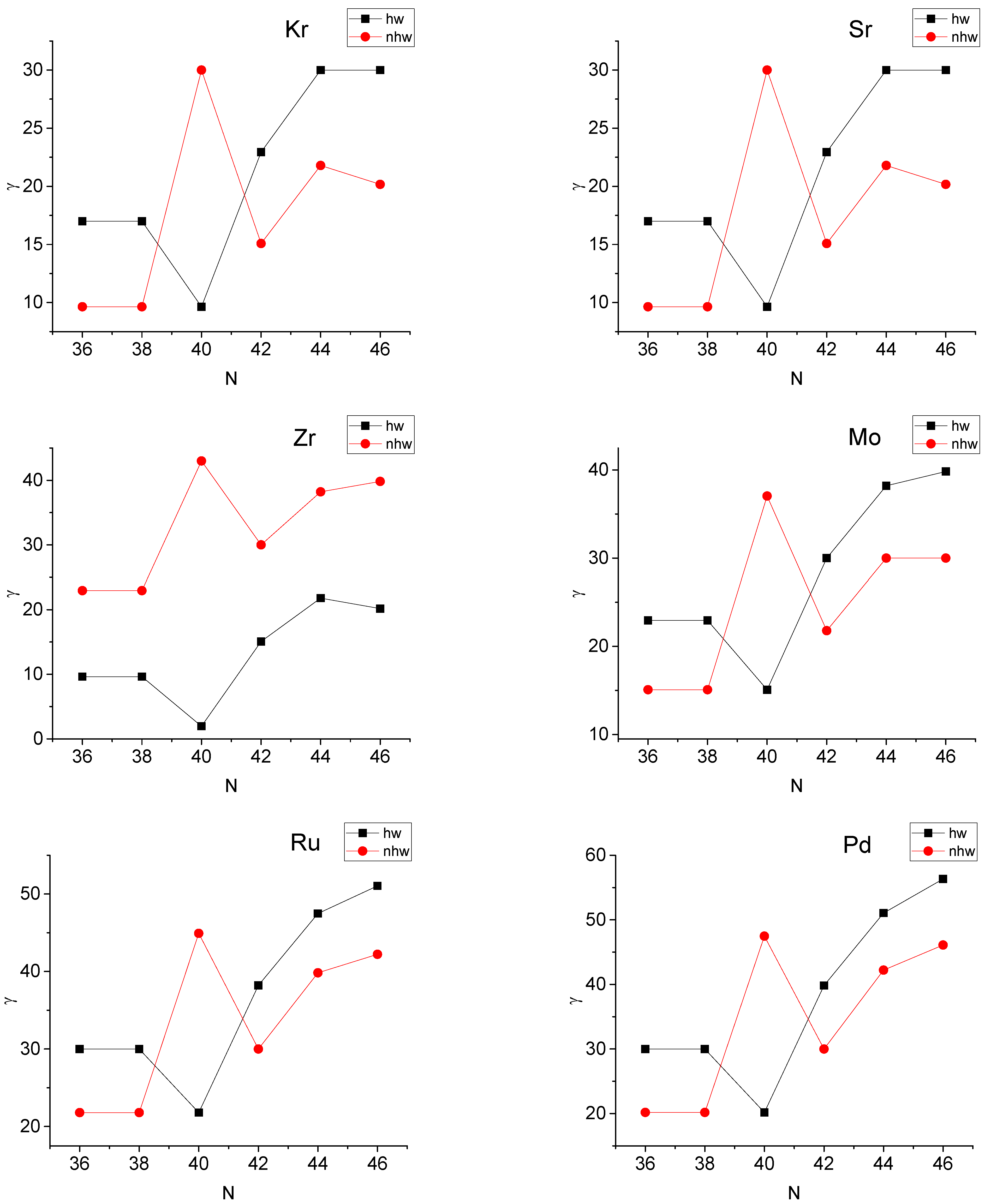
3. Parameter-Free Predictions for the and Collective Variables from the hw Irreps
4. Next-Highest-Weight Irreducible Representations
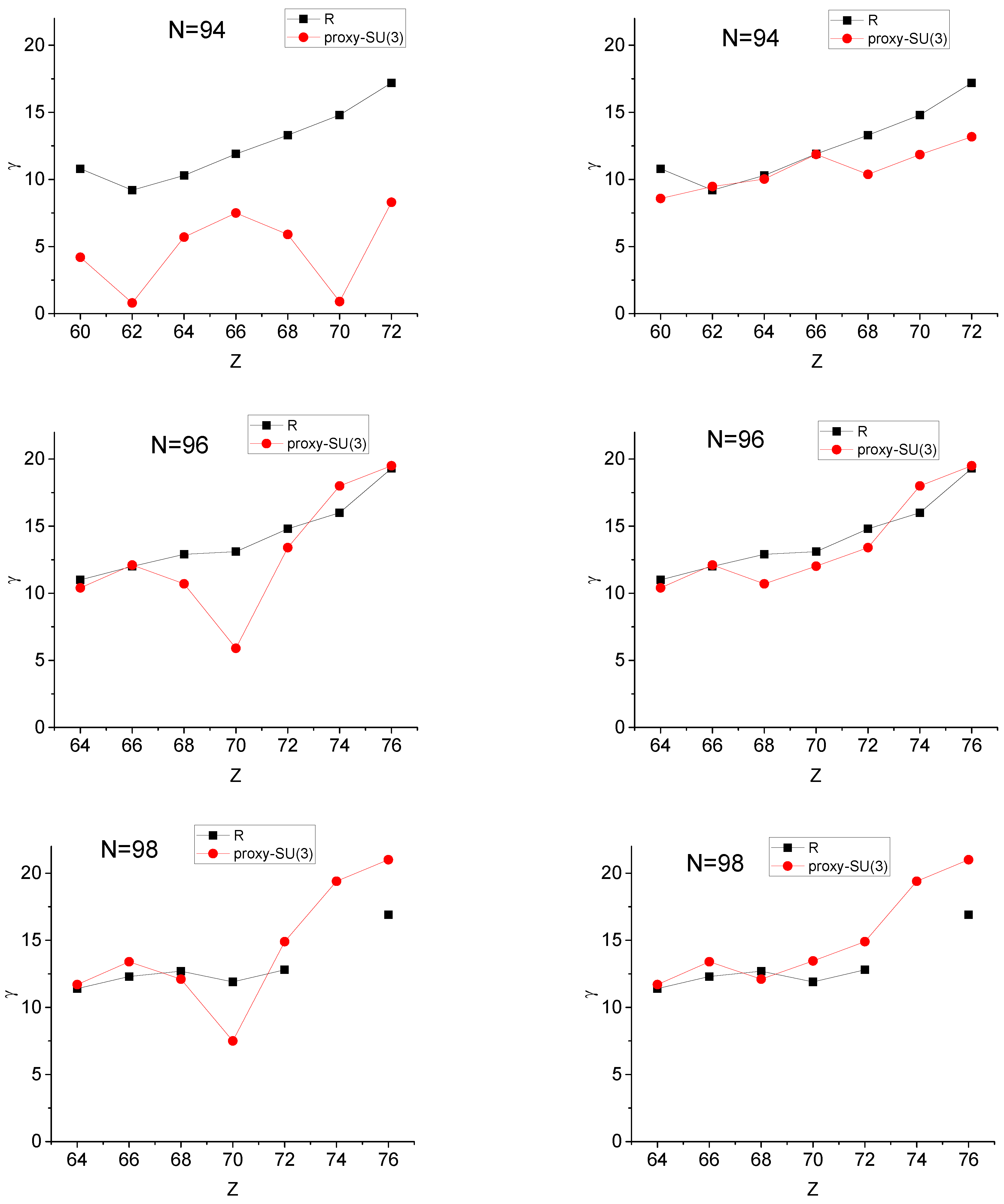
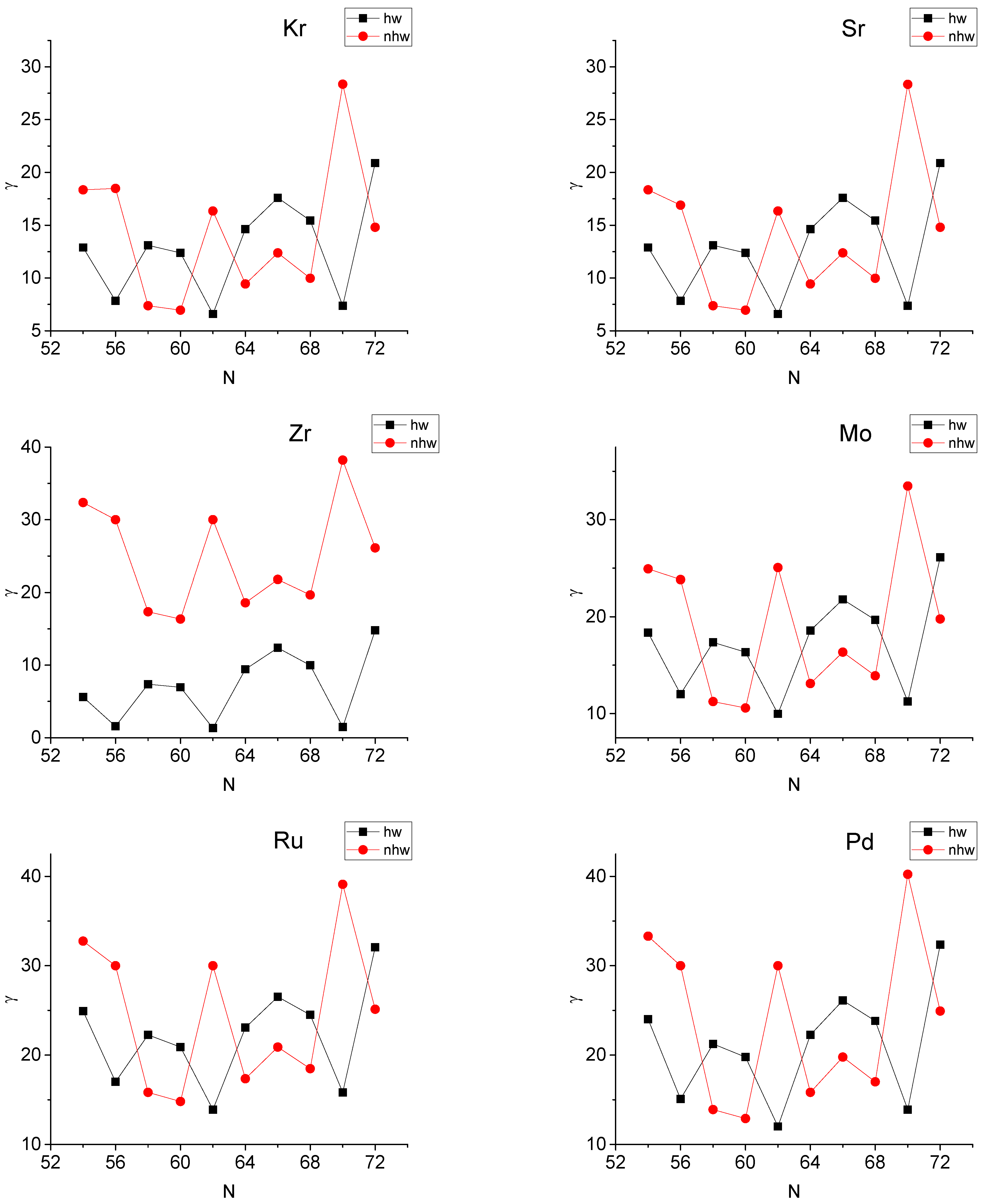
5. Parameter-Free Predictions for the and Collective Variables from the nhw Irreps
6. Combining the hw and nhw Predictions
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kota, V.K.B. SU(3) Symmetry in Atomic Nuclei; Springer Nature: Singapore, 2020. [Google Scholar]
- Mayer, M.G. On Closed Shells in Nuclei. Phys. Rev. 1948, 74, 235. [Google Scholar] [CrossRef]
- Mayer, M.G. On Closed Shells in Nuclei. II. Phys. Rev. 1949, 75, 1969. [Google Scholar] [CrossRef]
- Haxel, O.; Jensen, J.H.D.; Suess, H.E. On the “Magic Numbers” in Nuclear Structure. Phys. Rev. 1949, 75, 1766. [Google Scholar] [CrossRef]
- Mayer, M.G.; Jensen, J.H.D. Elementary Theory of Nuclear Shell Structure; Wiley: New York, NY, USA, 1955. [Google Scholar]
- Heyde, K.L.G. The Nuclear Shell Model; Springer: Berlin, Germany, 1990. [Google Scholar]
- Talmi, I. Simple Models of Complex Nuclei: The Shell Model and the Interacting Boson Model; Harwood: Chur, Switzerland, 1993. [Google Scholar]
- Wybourne, B.G. Classical Groups for Physicists; Wiley: New York, NY, USA, 1974. [Google Scholar]
- Moshinsky, M.; Smirnov, Y.F. The Harmonic Oscillator in Modern Physics; Harwood: Amsterdam, The Netherlands, 1996. [Google Scholar]
- Iachello, F. Lie Algebras and Applications; Springer: Berlin, Germany, 2006. [Google Scholar]
- Bonatsos, D.; Klein, A. Exact boson mappings for nuclear neutron (proton) shell-model algebras having SU(3) subalgebras. Ann. Phys. 1986, 169, 61. [Google Scholar] [CrossRef]
- Elliott, J.P. Collective motion in the nuclear shell model. I. Classification schemes for states of mixed configurations. Proc. Roy. Soc. A Ser. A 1958, 245, 128. [Google Scholar]
- Elliott, J.P. Collective motion in the nuclear shell model II. The introduction of intrinsic wave-functions. Proc. Roy. Soc. A Ser. A 1958, 245, 562. [Google Scholar]
- Elliott, J.P.; Harvey, M. Collective motion in the nuclear shell model III. The calculation of spectra. Proc. Roy. Soc. A Ser. A 1963, 272, 557. [Google Scholar]
- Elliott, J.P.; Wilsdon, C.E. Collective motion in the nuclear shell model IV. Odd-mass nuclei in the sd shell. Proc. Roy. Soc. A Ser. A 1968, 302, 509. [Google Scholar]
- Harvey, M. The nuclear SU3 model. In Advances in Nuclear Physics; Baranger, M., Vogt, E., Eds.; Plenum: New York, NY, USA, 1968; Volume 1, p. 67. [Google Scholar]
- Bohr, A. The coupling of nuclear surface oscillations to the motion of individual nucleons. Dan. Mat. Fys. Medd. 1952, 26, 14. [Google Scholar]
- Bohr, A.; Mottelson, B.R. Collective and individual-particle aspects of nuclear structure. Dan. Mat. Fys. Medd. 1953, 27, 16. [Google Scholar]
- Bohr, A.; Mottelson, B.R. Nuclear Structure Vol. I: Single-Particle Motion; World Scientific: Singapore, 1998. [Google Scholar]
- Bohr, A.; Mottelson, B.R. Nuclear Structure Vol. II: Nuclear Deformations; World Scientific: Singapore, 1998. [Google Scholar]
- Arima, A.; Harvey, M.; Shimizu, K. Pseudo LS coupling and pseudo SU3 coupling schemes. Phys. Lett. B 1969, 30, 517. [Google Scholar] [CrossRef]
- Hecht, K.T.; Adler, A. Generalized seniority for favored J≠0 pairs in mixed configurations. Nucl. Phys. A 1969, 137, 129. [Google Scholar] [CrossRef]
- Ratna Raju, R.D.; Draayer, J.P.; Hecht, K.T. Search for a coupling scheme in heavy deformed nuclei: The pseudo SU(3) model. Nucl. Phys. A 1973, 202, 433. [Google Scholar] [CrossRef]
- Draayer, J.P.; Weeks, K.J.; Hecht, K.T. Strength of the Qπ·Qν interaction and the strong-coupled pseudo-SU(3) limit. Nucl. Phys. A 1982, 381, 1. [Google Scholar] [CrossRef]
- Draayer, J.P.; Weeks, K.J. Shell-Model Description of the Low-Energy Structure of Strongly Deformed Nuclei. Phys. Rev. Lett. 1983, 51, 1422. [Google Scholar] [CrossRef]
- Draayer, J.P.; Weeks, K.J. Towards a shell model description of the low-energy structure of deformed nuclei I. Even-even systems. Ann. Phys. 1984, 156, 41. [Google Scholar] [CrossRef]
- Ginocchio, J.N. Pseudospin as a Relativistic Symmetry. Phys. Rev. Lett. 1997, 78, 436. [Google Scholar] [CrossRef]
- Bahri, C.; Draayer, J.P.; Moszkowski, S.A. Pseudospin symmetry in nuclear physics. Phys. Rev. Lett. 1992, 68, 2133. [Google Scholar] [CrossRef]
- Zuker, A.P.; Retamosa, J.; Poves, A.; Caurier, E. Spherical shell model description of rotational motion. Phys. Rev. C 1995, 52, R1741(R). [Google Scholar] [CrossRef]
- Zuker, A.P.; Poves, A.; Nowacki, F.; Lenzi, S.M. Nilsson-SU3 self-consistency in heavy N=Z nuclei. Phys. Rev. C 2015, 92, 024320. [Google Scholar] [CrossRef]
- Bonatsos, D.; Assimakis, I.E.; Minkov, N.; Martinou, A.; Cakirli, R.B.; Casten, R.F.; Blaum, K. Proxy-SU(3) symmetry in heavy deformed nuclei. Phys. Rev. C 2017, 95, 064325. [Google Scholar] [CrossRef]
- Bonatsos, D.; Assimakis, I.E.; Minkov, N.; Martinou, A.; Sarantopoulou, S.; Cakirli, R.B.; Casten, R.F.; Blaum, K. Analytic predictions for nuclear shapes, prolate dominance, and the prolate-oblate shape transition in the proxy-SU(3) model. Phys. Rev. C 2017, 95, 064326. [Google Scholar] [CrossRef]
- Bonatsos, D.; Martinou, A.; Peroulis, S.K.; Mertzimekis, T.J.; Minkov, N. The Proxy-SU(3) Symmetry in Atomic Nuclei. Symmetry 2023, 15, 169. [Google Scholar] [CrossRef]
- Rosensteel, G.; Rowe, D.J. Nuclear Sp(3,R) Model. Phys. Rev. Lett. 1977, 38, 10. [Google Scholar] [CrossRef]
- Rosensteel, G.; Rowe, D.J. On the algebraic formulation of collective models III. The symplectic shell model of collective motion. Ann. Phys. 1980, 126, 343. [Google Scholar] [CrossRef]
- Rowe, D.J. Microscopic theory of the nuclear collective model. Rep. Prog. Phys. 1985, 48, 1419. [Google Scholar] [CrossRef]
- Dytrych, T.; Sviratcheva, K.D.; Draayer, J.P.; Bahri, C.; Vary, J.P. Ab initio symplectic no-core shell model. J. Phys. G: Nucl. Part. Phys. 2008, 35, 123101. [Google Scholar] [CrossRef]
- Launey, K.D.; Draayer, J.P.; Dytrych, T.; Sun, G.-H.; Dong, S.-H. Approximate symmetries in atomic nuclei from a large-scale shell-model perspective. Int. J. Mod. Phys. E 2015, 24, 1530005. [Google Scholar] [CrossRef]
- Launey, K.D.; Dytrych, T.; Draayer, J.P. Symmetry-guided large-scale shell-model theory. Prog. Part. Nucl. Phys. 2016, 89, 101. [Google Scholar] [CrossRef]
- Launey, K.D.; Dytrych, T.; Sargsyan, G.H.; Baker, R.B.; Draayer, J.P. Emergent symplectic symmetry in atomic nuclei: Ab initio symmetry-adapted no-core shell model. Eur. Phys. J. Spec. Top. 2020, 229, 2429. [Google Scholar] [CrossRef]
- Launey, K.D.; Mercenne, A.; Dytrych, T. Nuclear Dynamics and Reactions in the Ab Initio Symmetry-Adapted Framework. Annu. Rev. Nucl. Part. Sci. 2021, 71, 253. [Google Scholar] [CrossRef]
- Arima, A.; Iachello, F. Collective Nuclear States as Representations of a SU(6) Group. Phys. Rev. Lett. 1975, 35, 1069. [Google Scholar] [CrossRef]
- Iachello, F.; Arima, A. The Interacting Boson Model; Cambridge U. Press: Cambridge, UK, 1987. [Google Scholar]
- Iachello, F.; Van Isacker, P. The Interacting Boson-Fermion Model; Cambridge U. Press: Cambridge, UK, 1991. [Google Scholar]
- Algebraic Approaches to Nuclear Structure: Interacting Boson and Fermion Models; Casten, R.F. (Ed.) Harwood: Chur, Switzerland, 1993. [Google Scholar]
- Frank, A.; Van Isacker, P. Symmetry Methods in Molecules and Nuclei; Mexico, D.F., Ed.; SyG Editores: Coyoacan, Mexico, 2005. [Google Scholar]
- Arima, A.; Iachello, F. Interacting boson model of collective nuclear states II. The rotational limit. Ann. Phys. 1978, 111, 201. [Google Scholar] [CrossRef]
- Casten, R.F.; Warner, D.D. The interacting boson approximation. Rev. Mod. Phys. 1988, 60, 389. [Google Scholar] [CrossRef]
- Casten, R.F. Nuclear Structure from a Simple Perspective; Oxford University Press: Oxford, UK, 1990. [Google Scholar]
- Minkov, N.; Drenska, S.B.; Raychev, P.P.; Roussev, R.P.; Bonatsos, D. Broken SU(3) symmetry in deformed even-even nuclei. Phys. Rev. C 1997, 55, 2345. [Google Scholar] [CrossRef]
- Martinou, A.; Bonatsos, D.; Minkov, N.; Assimakis, I.E.; Peroulis, S.K.; Sarantopoulou, S.; Cseh, J. Proxy-SU(3) symmetry in the shell model basis. Eur. Phys. J. A 2020, 56, 239. [Google Scholar] [CrossRef]
- Martinou, A.; Bonatsos, D.; Karakatsanis, K.E.; Sarantopoulou, S.; Assimakis, I.E.; Peroulis, S.K.; Minkov, N. Why nuclear forces favor the highest weight irreducible representations of the fermionic SU(3) symmetry. Eur. Phys. J. A 2021, 57, 83. [Google Scholar] [CrossRef]
- Ring, P.; Schuck, P. The Nuclear Many-Body Problem; Springer: Berlin, Germany, 1980. [Google Scholar]
- Bonatsos, D. Prolate over oblate dominance in deformed nuclei as a consequence of the SU(3) symmetry and the Pauli principle. Eur. Phys. J. A 2017, 53, 148. [Google Scholar] [CrossRef]
- Martinou, A.; Bonatsos, D.; Metzimekis, T.J.; Karakatsanis, K.E.; Assimakis, I.E.; Peroulis, S.K.; Sarantopoulou, S.; Minkov, N. The islands of shape coexistence within the Elliott and the proxy-SU(3) Models. Eur. Phys. J. A 2021, 57, 84. [Google Scholar] [CrossRef]
- Martinou, A.; Bonatsos, D.; Peroulis, S.K.; Karakatsanis, K.E.; Mertzimekis, T.J.; Minkov, N. Islands of Shape Coexistence: Theoretical Predictions and Experimental Evidence. Symmetry 2023, 15, 29. [Google Scholar] [CrossRef]
- Bonatsos, D.; Andriana Martinou, A.; Peroulis, S.K.; Mertzimekis, T.J.; Minkov, N. Shape Coexistence in Even–Even Nuclei: A Theoretical Overview. Atoms 2023, 11, 117. [Google Scholar] [CrossRef]
- Martinou, A.; Bonatsos, D.; Assimakis, I.E.; Minkov, N.; Sarantopoulou, S.; Cakirli, R.B.; Casten, R.F.; Blaum, K. Parameter Free Predictions within the Proxy-SU(3) Model. Bulg. J. Phys. 2017, 44, 407. [Google Scholar]
- Draayer, J.P.; Leschber, Y.; Park, S.C.; R. Lopez, R. Representations of U(3) in U(N). Comput. Phys. Commun. 1989, 56, 279. [Google Scholar] [CrossRef]
- Langr, D.; Dytrych, T.; Draayer, J.P.; Launey, K.D.; Tvrdík, P. Efficient algorithm for representations of U(3) in U(N). Comput. Phys. Commun. 2019, 244, 442. [Google Scholar] [CrossRef]
- Kota, V.K.B. Simple formula for leading SU(3) irreducible representation for nucleons in an oscillator shell. arXiv 2018, arXiv:1812.01810. [Google Scholar]
- Bonatsos, D.; Martinou, A.; Peroulis, S.K.; Petrellis, D.; Vasileiou, P.; Mertzimekis, T.J.; Minkov, N. Preponderance of triaxial shapes in atomic nuclei predicted by the proxy-SU(3) symmetry. J. Phys. G: Nucl. Part. Phys. 2024. in print. [Google Scholar] [CrossRef]
- ENSDF Database. Available online: https://www.nndc.bnl.gov/ensdf (accessed on 1 July 2024).
- Börner, H.G.; Jolie, J.; Robinson, S.J.; Krusche, B.; Piepenbring, R.; Casten, R.F.; Aprahamian, A.; Draayer, J.P. Evidence for the existence of two-phonon collective excitations in deformed nuclei. Phys. Rev. Lett. 1991, 66, 691. [Google Scholar] [CrossRef] [PubMed]
- Wu, X.; Aprahamian, A.; Fischer, S.M.; Reviol, W.; Liu, G.; Saladin, J.X. Multiphonon vibrational states in deformed nuclei. Phys. Rev. C 1994, 49, 1837. [Google Scholar] [CrossRef]
- Burke, D.G. Hexadecapole-Phonon versus Double-γ-Phonon Interpretation for Kπ=4+ Bands in Deformed Even-Even Nuclei. Phys. Rev. Lett. 1994, 73, 1899. [Google Scholar] [CrossRef]
- Garrett, P.E.; Kulp, W.D.; Wood, J.L.; Bandyopadhyay, D.; Christen, S.; Choudry, S.; Dewald, A.; Fitzler, A.; Fransen, C.; Jessen, K.; et al. Octupole and hexadecapole bands in 152Sm. J. Phys. G: Nucl. Part. Phys. 2005, 31, S1855. [Google Scholar] [CrossRef]
- Jolos, R.V.; von Brentano, P. Mass coefficient and Grodzins relation for the ground-state band and γ band. Phys. Rev. C 2006, 74, 064307. [Google Scholar] [CrossRef]
- Jolos, R.V.; von Brentano, P. Bohr Hamiltonian with different mass coefficients for the ground- and γ bands from experimental data. Phys. Rev. C 2007, 76, 024309. [Google Scholar] [CrossRef]
- Bonatsos, D.; Assimakis, I.E.; Martinou, A.; Sarantopoulou, S.; Peroulis, S.K.; Minkov, N. Energy differences of ground state and γ1 bands as a hallmark of collective behavior. Nucl. Phys. A 2021, 1009, 122158. [Google Scholar] [CrossRef]
- Castaños, O.; Draayer, J.P.; Leschber, Y. Shape variables and the shell model. Z. Phys. A 1988, 329, 33. [Google Scholar]
- Draayer, J.P.; Park, S.C.; Castaños, O. Shell-Model Interpretation of the Collective-Model Potential-Energy Surface. Phys. Rev. Lett. 1989, 62, 20. [Google Scholar] [CrossRef] [PubMed]
- De Vries, H.; De Jager, C.W.; De Vries, C. Nuclear charge-density-distribution parameters from elastic electron scattering. At. Data Nucl. Data Tables 1987, 36, 495. [Google Scholar] [CrossRef]
- Stone, J.R.; Stone, N.J.; Moszkowski, S.A. Incompressibility in finite nuclei and nuclear matter. Phys. Rev. C 2014, 89, 044316. [Google Scholar] [CrossRef]
- Pritychenko, B.; Birch, M.; Singh, B.; Horoi, M. Tables of E2 transition probabilities from the first 2+ states in even-even nuclei. At. Data Nucl. Data Tables 2016, 107, 1. [Google Scholar] [CrossRef]
- Davydov, A.S.; Filippov, G.F. Rotational states in even atomic nuclei. Nucl. Phys. 1958, 8, 237. [Google Scholar] [CrossRef]
- Davydov, A.S.; Rostovsky, V.S. Relative transition probabilities between rotational levels of non-axial nuclei. Nucl. Phys. 1959, 12, 58. [Google Scholar] [CrossRef]
- Martinou, A.; Peroulis, S.; Bonatsos, D.; Assimakis, I.E.; Sarantopoulou, S.; Minkov, N.; Cakirli, R.B.; Casten, R.F.; Blaum, K. Parameter-independent predictions for nuclear shapes and B(E2) transition rates in the Proxy-SU(3) Model. HNPS Adv. Nucl. Phys. 2017, 25, 21. [Google Scholar] [CrossRef]
- Minkov, N.; Drenska, S.B.; Raychev, P.P.; Roussev, R.P.; Bonatsos, D. Ground-γ band coupling in heavy deformed nuclei and SU(3) contraction limit. Phys. Rev. C 1999, 60, 034305. [Google Scholar] [CrossRef]
- Minkov, N.; Drenska, S.B.; Raychev, P.P.; Roussev, R.P.; Dennis Bonatsos, D. Ground-γ band mixing and odd-even staggering in heavy deformed nuclei. Phys. Rev. C 2000, 61, 064301. [Google Scholar] [CrossRef]
- Kota, V.K.B.; Sahu, R. Proxy-SU(4) symmetry in A = 60–90 region. Phys. Scr. 2024, 99, 065306. [Google Scholar] [CrossRef]
- Sarantopoulou, S.; Bonatsos, D.; Assimakis, I.E.; Minkov, N.; Martinou, A.; Cakirli, R.B.; Casten, R.F.; Blaum, K. Proxy-SU(3) Symmetry in Heavy Nuclei: Prolate Dominance and Prolate-Oblate Shape Transition. Bulg. J. Phys. 2017, 44, 417. [Google Scholar]
- Bhatt, K.H.; Kahane, S.; Raman, S. Collective properties of nucleons in the abnormal-parity states. Phys. Rev. C 2000, 61, 034317. [Google Scholar] [CrossRef]
- Bhatt, K.H.; Nestor, C.W., Jr.; Raman, S. Do nucleons in abnormal-parity states contribute to deformation? Phys. Rev. C 1992, 46, 164. [Google Scholar] [CrossRef] [PubMed]
- Bhatt, K.H.; Raman, S.; Nestor, C.W., Jr. Symplectic pseudo-SU(3) model and B(E2;01+→21+) value of 238U. Phys. Rev. C 1994, 49, 808. [Google Scholar] [CrossRef]
- Kahane, S.; Raman, S.; Bhatt, K.H. Angular-momentum structure of the yrast bands of deformed nuclei. Phys. Rev. C 1997, 55, 2885. [Google Scholar] [CrossRef]
- Raman, S.; Nestor, C.W., Jr.; Bhatt, K.H. Systematics of B(E2;01+→21+) values for even-even nuclei. Phys. Rev. C 1988, 37, 805. [Google Scholar] [CrossRef]
- Alex, A.; Kalus, M.; Huckleberry, A.; von Delft, J. A numerical algorithm for the explicit calculation of SU(N) and SL(N,C) Clebsch–Gordan coefficients. J. Math. Phys. 2011, 52, 023507. [Google Scholar] [CrossRef]
- SU(N) Clebsch–Gordan Coefficients. 2010. Available online: http://homepages.physik.uni-muenchen.de/~vondelft/Papers/ClebschGordan/ (accessed on 1 July 2024).
- Dytrych, T.; Langr, D.; Draayer, J.P.; Launey, K.D.; Gazda, D. SU3lib: A C++ library for accurate computation of Wigner and Racah coefficients of SU(3). Comput. Phys. Commun. 2021, 269, 108137. [Google Scholar] [CrossRef]
- Bahri, C.; Rowe, D.J.; Draayer, J.P. Programs for generating Clebsch–Gordan coefficients of SU(3) in SU(2) and SO(3) bases. Comput. Phys. Commun. 2004, 159, 121. [Google Scholar] [CrossRef]
- Brink, D.M.; Broglia, R.A. Nuclear Superfluidity: Pairing in Finite Systems; Cambridge U. Press: Cambridge, UK, 2005. [Google Scholar]
- Rakavy, G. The classification of states of surface vibrations. Nucl. Phys. 1957, 4, 289. [Google Scholar] [CrossRef]
- Troltenier, D.; Bahri, C.; Draayer, J.P. Generalized pseudo-SU(3) model and pairing. Nucl. Phys. A 1995, 586, 53. [Google Scholar] [CrossRef]
- Troltenier, D.; Bahri, C.; Draayer, J.P. Effects of pairing in the pseudo-SU(3) model. Nucl. Phys. A 1995, 589, 75. [Google Scholar] [CrossRef]
- Bahri, C. Pairing and Quadrupole Interactions in the Pseudo-SU(3) Shell Model. Ph.D. Thesis, Louisiana State University, Baton Rouge, LA, USA, 1994. [Google Scholar]
- Bahri, C.; Escher, J.; Draayer, J.P. Monopole-pairing and deformation in atomic nuclei. Nucl. Phys. A 1995, 592, 171. [Google Scholar] [CrossRef]
- Escher, J.; Bahri, C.; Troltenier, D.; Draayer, J.P. Pairing-plus-quadrupole model and nuclear deformation: A look at the spin-orbit interaction. Nucl. Phys. A 1998, 633, 662. [Google Scholar] [CrossRef]
- Bonatsos, D.; Martinou, A.; Sarantopoulou, S.; Assimakis, I.E.; Peroulis, S.; Minkov, N. Parameter-free predictions for the collective deformation variables β and γ within the pseudo-SU(3) scheme. Eur. Phys. J. Spec. Top. 2020, 229, 2367. [Google Scholar] [CrossRef]
- Castaños, O.; Moshinsky, M.; Quesne, C. Transformation from U(3) to pseudo U(3) basis. In Group Theory and Special Symmetries in Nuclear Physics (AnnArbor, 1991); Draayer, J.P., Jänecke, J., Eds.; World Scientific: Singapore, 1992; p. 80. [Google Scholar]
- Castaños, O.; Moshinsky, M.; Quesne, C. Transformation to pseudo-SU(3) in heavy deformed nuclei. Phys. Lett. B 1992, 277, 238. [Google Scholar] [CrossRef]
- Castaños, O.; Velázquez, A.V.; Hess, P.O.; Hirsch, J.G. Transformation to pseudo-spin-symmetry of a deformed Nilsson hamiltonian. Phys. Lett. B 1994, 321, 303. [Google Scholar] [CrossRef]



| M | U(6) | U(6) | U(10) | U(10) | U(15) | U(15) | U(21) | U(21) | U(28) | U(28) |
|---|---|---|---|---|---|---|---|---|---|---|
| 8–20 | 8–20 | 28–50 | 28–50 | 50–82 | 50–82 | 82–126 | 82–126 | 126–128 | 126–184 | |
| hw | nhw | hw | nhw | hw | nhw | hw | nhw | hw | nhw | |
| 2 | 4,0 | 0,2 | 6,0 | 2,2 | 8,0 | 4,2 | 10,0 | 6,2 | 12,0 | 8,2 |
| 4 | 4,2 | 0,4 | 8,2 | 4,4 | 12,2 | 8,4 | 16,2 | 12,4 | 20,2 | 16,4 |
| 6 | 6,0 | 0,6 | 12.0 | 6,6 | 18,0 | 12,6 | 24,0 | 18,6 | 30,0 | 24,6 |
| 8 | 2,4 | 4,0 | 10,4 | 12,0 | 18,4 | 20,0 | 26,4 | 28,0 | 34,4 | 36,0 |
| 10 | 0,4 | 2,0 | 10,4 | 12,0 | 20,4 | 22,0 | 30,4 | 32,0 | 40,4 | 42,0 |
| 12 | 0,0 | 12,0 | 4,10 | 24,0 | 16,10 | 36,0 | 28,10 | 48,0 | 40,10 | |
| 14 | 6,6 | 8,2 | 20,6 | 22,2 | 34,6 | 36,2 | 48,6 | 50,2 | ||
| 16 | 2,8 | 4,4 | 18,8 | 20,4 | 34,8 | 36,4 | 50,8 | 52,4 | ||
| 18 | 0,6 | 2,2 | 18,6 | 20,2 | 36,6 | 38,2 | 54,6 | 56,2 | ||
| 20 | 0,0 | 20,0 | 10,14 | 40,0 | 30,14 | 60,0 | 50,14 | |||
| 22 | 12,8 | 14,4 | 34,8 | 36,4 | 56,8 | 58,4 | ||||
| 24 | 6,12 | 8,8 | 30,12 | 32,8 | 54,12 | 56,8 | ||||
| 26 | 2,12 | 4,8 | 28,12 | 30,8 | 54,12 | 56,8 | ||||
| 28 | 0,8 | 2,4 | 28,8 | 30,4 | 56,8 | 58,4 | ||||
| 30 | 0,0 | 30,0 | 18,18 | 60,0 | 48,18 | |||||
| 32 | 20,10 | 22,6 | 52,10 | 54,6 | ||||||
| 34 | 12,16 | 14,12 | 46,16 | 48,12 | ||||||
| 36 | 6,18 | 8,14 | 42,18 | 44,14 | ||||||
| 38 | 2,16 | 4,12 | 40,16 | 42,12 | ||||||
| 40 | 0,10 | 2,6 | 40,10 | 42,6 | ||||||
| 42 | 0,0 | 42,0 | 28,22 | |||||||
| 44 | 30,12 | 32,8 | ||||||||
| 46 | 20,20 | 22,16 | ||||||||
| 48 | 12,24 | 14,20 | ||||||||
| 50 | 6,24 | 8,20 | ||||||||
| 52 | 2,20 | 4,16 | ||||||||
| 54 | 0,12 | 2,8 | ||||||||
| 56 | 0,0 |
| Nucleus | hw | nhw | ||||
|---|---|---|---|---|---|---|
| 46, 4 | 38, 14 | 0.252 | 0.245 | 5.00 | 15.61 | |
| 50, 4 | 42, 14 | 0.271 | 0.263 | 4.63 | 14.43 | |
| 56, 0 | 38, 24 | 0.290 | 0.282 | 0.86 | 22.80 | |
| 54, 6 | 46, 16 | 0.295 | 0.288 | 5.92 | 14.86 | |
| 54, 8 | 46, 18 | 0.300 | 0.294 | 7.46 | 16.24 | |
| 56, 6 | 48, 16 | 0.302 | 0.295 | 5.72 | 14.36 | |
| 60, 0 | 40, 28 | 0.305 | 0.302 | 0.81 | 24.35 | |
| 54, 8 | 46, 18 | 0.296 | 0.290 | 7.46 | 16.24 | |
| 50, 12 | 42, 22 | 0.288 | 0.285 | 11.08 | 20.08 | |
| 48, 12 | 40, 22 | 0.277 | 0.275 | 11.47 | 20.78 | |
| 48, 8 | 40, 18 | 0.264 | 0.259 | 8.29 | 18.05 | |
| 50, 0 | 28, 32 | 0.251 | 0.262 | 0.96 | 32.13 | |
| 40, 10 | 32, 20 | 0.230 | 0.228 | 11.58 | 22.69 | |
| 32, 16 | 24, 26 | 0.213 | 0.218 | 19.53 | 31.27 | |
| 26, 18 | 18, 28 | 0.193 | 0.202 | 24.27 | 36.86 | |
| 22, 16 | 14, 26 | 0.167 | 0.177 | 25.05 | 39.37 | |
| 20, 10 | 12, 20 | 0.135 | 0.142 | 19.77 | 37.74 | |
| 38, 12 | 40, 8 | 0.237 | 0.233 | 13.90 | 9.72 | |
| 42, 12 | 44, 8 | 0.256 | 0.253 | 12.81 | 8.95 | |
| 48, 8 | 40, 18 | 0.271 | 0.266 | 8.29 | 18.05 | |
| 46, 14 | 48, 10 | 0.280 | 0.276 | 13.41 | 9.91 | |
| 46, 16 | 48, 12 | 0.286 | 0.282 | 14.86 | 11.47 | |
| 48, 14 | 50, 10 | 0.287 | 0.284 | 12.95 | 9.57 | |
| 52, 8 | 42, 22 | 0.286 | 0.286 | 7.72 | 20.08 | |
| 46, 16 | 48, 12 | 0.282 | 0.278 | 14.86 | 11.47 | |
| 42, 20 | 44, 16 | 0.277 | 0.272 | 18.77 | 15.39 | |
| 40, 20 | 42, 16 | 0.267 | 0.262 | 19.45 | 15.95 | |
| 40, 16 | 42, 12 | 0.251 | 0.247 | 16.56 | 12.81 | |
| 42, 8 | 30, 26 | 0.233 | 0.243 | 9.32 | 27.72 | |
| 32, 18 | 34, 14 | 0.220 | 0.215 | 21.16 | 17.00 | |
| 24, 24 | 26, 20 | 0.208 | 0.200 | 30.00 | 25.87 | |
| 18, 26 | 20, 22 | 0.192 | 0.183 | 35.73 | 31.50 | |
| 14, 24 | 16, 20 | 0.167 | 0.157 | 38.21 | 33.48 | |
| 12, 18 | 14, 14 | 0.133 | 0.124 | 36.18 | 30.00 | |
| 32, 16 | 34, 12 | 0.222 | 0.217 | 19.53 | 15.18 | |
| 36, 16 | 38, 12 | 0.240 | 0.235 | 17.93 | 13.90 | |
| 42, 12 | 34, 22 | 0.254 | 0.253 | 12.81 | 23.19 | |
| 40, 18 | 42, 14 | 0.264 | 0.259 | 18.05 | 14.43 | |
| 40, 20 | 42, 16 | 0.271 | 0.266 | 19.45 | 15.95 | |
| 42, 18 | 44, 14 | 0.272 | 0.267 | 17.40 | 13.90 | |
| 46, 12 | 36, 26 | 0.269 | 0.274 | 11.88 | 24.85 | |
| 40, 20 | 42, 16 | 0.268 | 0.263 | 19.45 | 15.95 | |
| 36, 24 | 38, 20 | 0.264 | 0.258 | 23.62 | 20.17 | |
| 34, 24 | 36, 20 | 0.254 | 0.247 | 24.50 | 20.95 | |
| 34, 20 | 36, 16 | 0.237 | 0.231 | 21.79 | 17.93 | |
| 36, 12 | 24, 30 | 0.217 | 0.235 | 14.51 | 33.54 | |
| 26, 22 | 28, 18 | 0.209 | 0.202 | 27.36 | 23.14 | |
| 18, 28 | 20, 24 | 0.201 | 0.191 | 36.86 | 32.87 | |
| 12, 30 | 14, 26 | 0.187 | 0.176 | 43.29 | 39.37 | |
| 8, 28 | 10, 24 | 0.164 | 0.152 | 46.90 | 42.65 | |
| 6, 22 | 8, 18 | 0.129 | 0.117 | 47.11 | 41.65 | |
| 28, 16 | 30, 12 | 0.202 | 0.196 | 21.43 | 16.71 | |
| 32, 16 | 34, 12 | 0.220 | 0.215 | 19.53 | 15.18 | |
| 38, 12 | 30, 22 | 0.233 | 0.233 | 13.90 | 25.11 | |
| 36, 18 | 38, 14 | 0.244 | 0.239 | 19.49 | 15.61 | |
| 36, 20 | 38, 16 | 0.251 | 0.245 | 20.95 | 17.22 | |
| 38, 18 | 40, 14 | 0.252 | 0.247 | 18.74 | 14.99 | |
| 42, 12 | 32, 26 | 0.249 | 0.255 | 12.81 | 26.70 | |
| 36, 20 | 38, 16 | 0.248 | 0.242 | 20.95 | 17.22 | |
| 32, 24 | 34, 20 | 0.245 | 0.238 | 25.45 | 21.79 | |
| 30, 24 | 32, 20 | 0.235 | 0.228 | 26.46 | 22.69 | |
| 30, 20 | 32, 16 | 0.219 | 0.213 | 23.66 | 19.53 | |
| 32, 12 | 20, 30 | 0.198 | 0.218 | 15.91 | 36.34 | |
| 22, 22 | 24, 18 | 0.191 | 0.183 | 30.00 | 25.50 | |
| 14, 28 | 16, 24 | 0.185 | 0.175 | 40.41 | 36.28 | |
| 8, 30 | 10, 26 | 0.173 | 0.161 | 47.62 | 43.66 | |
| 4, 28 | 6, 24 | 0.151 | 0.138 | 51.17 | 47.99 | |
| 2, 22 | 4, 18 | 0.116 | 0.103 | 53.95 | 48.61 | |
| 26, 12 | 28, 8 | 0.177 | 0.172 | 18.58 | 13.10 | |
| 30, 12 | 32, 8 | 0.195 | 0.191 | 16.71 | 11.74 | |
| 36, 8 | 28, 18 | 0.209 | 0.207 | 10.64 | 23.14 | |
| 34, 14 | 36, 10 | 0.219 | 0.215 | 17.00 | 12.63 | |
| 34, 16 | 36, 12 | 0.226 | 0.221 | 18.70 | 14.51 | |
| 36, 14 | 38, 10 | 0.227 | 0.223 | 16.27 | 12.08 | |
| 40, 8 | 30, 22 | 0.225 | 0.229 | 9.72 | 25.11 | |
| 34, 16 | 36, 12 | 0.223 | 0.218 | 18.70 | 14.51 | |
| 30, 20 | 32, 16 | 0.220 | 0.214 | 23.66 | 19.53 | |
| 28, 20 | 30, 16 | 0.210 | 0.204 | 24.72 | 20.44 | |
| 28, 16 | 30, 12 | 0.194 | 0.189 | 21.43 | 16.71 | |
| 30, 8 | 18, 26 | 0.174 | 0.192 | 12.38 | 35.73 | |
| 20, 18 | 22, 14 | 0.166 | 0.159 | 28.35 | 23.07 | |
| 12, 24 | 14, 20 | 0.159 | 0.149 | 40.33 | 35.50 | |
| 6, 26 | 8, 22 | 0.148 | 0.136 | 48.76 | 44.18 | |
| 2, 24 | 4, 20 | 0.126 | 0.113 | 54.40 | 49.56 | |
| 0, 18 | 2, 14 | 0.092 | 0.079 | 57.46 | 51.05 | |
| 26, 4 | 28, 0 | 0.148 | 0.146 | 8.35 | 1.68 | |
| 30, 4 | 32, 0 | 0.167 | 0.166 | 7.37 | 1.48 | |
| 36, 0 | 28, 10 | 0.185 | 0.177 | 1.32 | 15.44 | |
| 34, 6 | 36, 2 | 0.192 | 0.190 | 8.95 | 3.86 | |
| 34, 8 | 36, 4 | 0.197 | 0.194 | 11.16 | 6.26 | |
| 36, 6 | 38, 2 | 0.200 | 0.198 | 8.51 | 3.67 | |
| 40, 0 | 30, 14 | 0.202 | 0.198 | 1.20 | 18.65 | |
| 34, 8 | 36, 4 | 0.195 | 0.192 | 11.16 | 6.26 | |
| 30, 12 | 32, 8 | 0.189 | 0.185 | 16.71 | 11.74 | |
| 28, 12 | 30, 8 | 0.179 | 0.175 | 17.60 | 12.38 | |
| 28, 8 | 30, 4 | 0.165 | 0.162 | 13.10 | 7.37 | |
| 30, 0 | 18, 18 | 0.151 | 0.158 | 1.57 | 30.00 | |
| 20, 10 | 22, 6 | 0.134 | 0.129 | 19.77 | 12.89 | |
| 12, 16 | 14, 12 | 0.124 | 0.115 | 34.40 | 27.64 | |
| 6, 18 | 8, 14 | 0.110 | 0.099 | 44.92 | 38.21 | |
| 2, 16 | 4, 12 | 0.088 | 0.076 | 52.01 | 44.39 | |
| 0, 10 | 2, 6 | 0.054 | 0.042 | 55.69 | 43.00 |
| Nucleus | hw | nhw | ||||
|---|---|---|---|---|---|---|
| 42, 4 | 36, 10 | 0.239 | 0.228 | 5.44 | 12.63 | |
| 44, 8 | 46, 4 | 0.261 | 0.259 | 8.95 | 5.00 | |
| 48, 8 | 50, 4 | 0.280 | 0.278 | 8.29 | 4.63 | |
| 54, 4 | 46, 14 | 0.298 | 0.290 | 4.31 | 13.41 | |
| 52, 10 | 54, 6 | 0.305 | 0.303 | 9.25 | 5.92 | |
| 52, 12 | 54, 8 | 0.310 | 0.307 | 10.71 | 7.46 | |
| 54, 10 | 56, 6 | 0.312 | 0.310 | 8.95 | 5.72 | |
| 44, 4 | 38, 10 | 0.249 | 0.238 | 5.21 | 12.08 | |
| 46, 8 | 48, 4 | 0.270 | 0.268 | 8.61 | 4.81 | |
| 50, 8 | 52, 4 | 0.289 | 0.287 | 7.99 | 4.46 | |
| 56, 4 | 48, 14 | 0.307 | 0.298 | 4.16 | 12.95 | |
| 54, 10 | 56, 6 | 0.314 | 0.312 | 8.95 | 5.72 | |
| 54, 12 | 56, 8 | 0.319 | 0.316 | 10.37 | 7.22 | |
| 56, 10 | 58, 6 | 0.321 | 0.319 | 8.67 | 5.54 | |
| 48, 0 | 34, 16 | 0.257 | 0.238 | 1.00 | 18.70 | |
| 50, 4 | 44, 10 | 0.277 | 0.265 | 4.63 | 10.68 | |
| 54, 4 | 48, 10 | 0.296 | 0.284 | 4.31 | 9.91 | |
| 60, 0 | 44, 20 | 0.315 | 0.299 | 0.81 | 18.14 | |
| 58, 6 | 52, 12 | 0.320 | 0.309 | 5.54 | 10.71 | |
| 58, 8 | 52, 14 | 0.325 | 0.314 | 7.00 | 12.12 | |
| 60, 6 | 54, 12 | 0.328 | 0.317 | 5.37 | 10.37 | |
| 44, 6 | 38, 12 | 0.252 | 0.242 | 7.12 | 13.90 | |
| 46, 10 | 48, 6 | 0.274 | 0.271 | 10.28 | 6.59 | |
| 50, 10 | 52, 6 | 0.293 | 0.291 | 9.57 | 6.12 | |
| 56, 6 | 48, 16 | 0.310 | 0.303 | 5.72 | 14.36 | |
| 54, 12 | 56, 8 | 0.317 | 0.314 | 10.37 | 7.22 | |
| 54, 14 | 56, 10 | 0.323 | 0.320 | 11.74 | 8.67 | |
| 56, 12 | 58, 8 | 0.325 | 0.322 | 10.05 | 7.00 | |
| 42, 8 | 36, 14 | 0.247 | 0.238 | 9.32 | 16.27 | |
| 44, 12 | 46, 8 | 0.270 | 0.267 | 12.33 | 8.61 | |
| 48, 12 | 50, 8 | 0.289 | 0.286 | 11.47 | 7.99 | |
| 54, 8 | 46, 18 | 0.305 | 0.299 | 7.46 | 16.24 | |
| 52, 14 | 54, 10 | 0.313 | 0.310 | 12.12 | 8.95 | |
| 52, 16 | 54, 12 | 0.318 | 0.314 | 13.46 | 10.37 | |
| 54, 14 | 56, 10 | 0.320 | 0.317 | 11.74 | 8.67 | |
| 42, 6 | 36, 12 | 0.240 | 0.230 | 7.43 | 14.51 | |
| 44, 10 | 46, 6 | 0.262 | 0.259 | 10.68 | 6.85 | |
| 48, 10 | 50, 6 | 0.281 | 0.279 | 9.91 | 6.35 | |
| 54, 6 | 46, 16 | 0.297 | 0.290 | 5.92 | 14.86 | |
| 52, 12 | 54, 8 | 0.305 | 0.302 | 10.71 | 7.46 | |
| 52, 14 | 54, 10 | 0.310 | 0.307 | 12.12 | 8.95 | |
| 54, 12 | 56, 8 | 0.312 | 0.309 | 10.37 | 7.22 |
| Nucleus | hw | nhw | ||||
|---|---|---|---|---|---|---|
| 32, 8 | 34, 4 | 0.256 | 0.252 | 11.74 | 6.59 | |
| 30, 10 | 32, 6 | 0.250 | 0.245 | 14.63 | 9.43 | |
| 30, 8 | 32, 4 | 0.240 | 0.236 | 12.38 | 6.95 | |
| 32, 2 | 22, 16 | 0.227 | 0.228 | 4.31 | 25.05 | |
| 24, 10 | 26, 6 | 0.209 | 0.203 | 17.35 | 11.24 | |
| 18, 14 | 20, 10 | 0.192 | 0.183 | 26.11 | 19.77 | |
| 14, 14 | 16, 10 | 0.168 | 0.158 | 30.00 | 22.95 | |
| 12, 10 | 14, 6 | 0.134 | 0.117 | 27.25 | 18.14 | |
| 38, 6 | 34, 8 | 0.285 | 0.267 | 8.12 | 11.16 | |
| 36, 8 | 32, 10 | 0.279 | 0.262 | 10.64 | 13.90 | |
| 36, 6 | 32, 8 | 0.269 | 0.252 | 8.51 | 11.74 | |
| 38, 0 | 22, 20 | 0.258 | 0.249 | 1.26 | 28.50 | |
| 30, 8 | 26, 10 | 0.236 | 0.220 | 12.38 | 16.34 | |
| 24, 12 | 20, 14 | 0.216 | 0.202 | 19.67 | 24.50 | |
| 20, 12 | 16, 14 | 0.191 | 0.178 | 22.26 | 27.93 | |
| 18, 8 | 14, 10 | 0.158 | 0.144 | 18.35 | 24.92 | |
| 38, 10 | 40, 6 | 0.300 | 0.296 | 12.08 | 7.76 | |
| 36, 12 | 38, 8 | 0.295 | 0.290 | 14.51 | 10.16 | |
| 36, 10 | 38, 6 | 0.284 | 0.276 | 12.63 | 8.12 | |
| 38, 4 | 28, 18 | 0.271 | 0.272 | 5.96 | 23.14 | |
| 30, 12 | 32, 8 | 0.253 | 0.247 | 16.71 | 11.74 | |
| 24, 16 | 26, 12 | 0.235 | 0.227 | 23.72 | 18.58 | |
| 20, 16 | 22, 12 | 0.211 | 0.202 | 26.52 | 20.89 | |
| 18, 12 | 20, 8 | 0.177 | 0.169 | 23.82 | 17.00 | |
| 40, 10 | 42, 6 | 0.312 | 0.308 | 11.58 | 7.43 | |
| 38, 12 | 40, 8 | 0.306 | 0.301 | 13.90 | 9.72 | |
| 38, 10 | 40, 6 | 0.296 | 0.292 | 12.08 | 7.76 | |
| 40, 4 | 30, 18 | 0.282 | 0.282 | 5.68 | 22.11 | |
| 32, 12 | 34, 8 | 0.264 | 0.259 | 15.91 | 11.16 | |
| 26, 16 | 28, 12 | 0.246 | 0.238 | 22.52 | 17.60 | |
| 22, 16 | 24, 12 | 0.221 | 0.213 | 25.05 | 19.67 | |
| 20, 12 | 22, 8 | 0.188 | 0.174 | 22.26 | 15.82 | |
| 44, 6 | 38, 12 | 0.319 | 0.306 | 7.12 | 13.90 | |
| 42, 8 | 36, 14 | 0.313 | 0.301 | 9.32 | 16.27 | |
| 42, 6 | 36, 12 | 0.303 | 0.290 | 7.43 | 14.51 | |
| 44, 0 | 26, 24 | 0.293 | 0.290 | 1.09 | 28.73 | |
| 36, 8 | 30, 14 | 0.270 | 0.260 | 10.64 | 18.65 | |
| 30, 12 | 24, 18 | 0.249 | 0.243 | 16.71 | 25.50 | |
| 26, 12 | 20, 18 | 0.224 | 0.220 | 18.58 | 28.35 | |
| 24, 8 | 18, 14 | 0.192 | 0.186 | 14.80 | 26.11 | |
| 40, 12 | 42, 8 | 0.317 | 0.313 | 13.33 | 9.32 | |
| 38, 14 | 40, 10 | 0.312 | 0.307 | 15.61 | 11.58 | |
| 38, 12 | 40, 8 | 0.301 | 0.296 | 13.90 | 9.72 | |
| 40, 6 | 30, 20 | 0.287 | 0.290 | 7.76 | 23.66 | |
| 32, 14 | 34, 10 | 0.271 | 0.265 | 17.78 | 13.24 | |
| 26, 18 | 28, 14 | 0.254 | 0.246 | 24.27 | 19.59 | |
| 22, 18 | 24, 14 | 0.230 | 0.221 | 26.85 | 21.79 | |
| 20, 14 | 22, 10 | 0.196 | 0.188 | 24.50 | 18.48 |
| Nucleus | hw | nhw | ||||
|---|---|---|---|---|---|---|
| 22, 6 | 18, 8 | 0.235 | 0.214 | 12.89 | 18.35 | |
| 28, 4 | 22, 10 | 0.273 | 0.258 | 7.83 | 18.48 | |
| 28, 8 | 30, 4 | 0.293 | 0.288 | 13.10 | 7.37 | |
| 30, 8 | 32, 4 | 0.308 | 0.303 | 12.38 | 6.95 | |
| 34, 4 | 26, 10 | 0.318 | 0.285 | 6.59 | 16.34 | |
| 30, 10 | 32, 6 | 0.315 | 0.309 | 14.63 | 9.43 | |
| 28, 12 | 30, 8 | 0.309 | 0.302 | 17.60 | 12.38 | |
| 28, 10 | 30, 6 | 0.295 | 0.289 | 15.44 | 9.97 | |
| 30, 4 | 20, 18 | 0.277 | 0.284 | 7.37 | 28.35 | |
| 22, 12 | 24, 8 | 0.257 | 0.248 | 20.89 | 14.80 | |
| 22, 6 | 18, 8 | 0.234 | 0.213 | 12.89 | 18.35 | |
| 28, 4 | 22, 10 | 0.271 | 0.256 | 7.83 | 18.48 | |
| 28, 8 | 30, 4 | 0.291 | 0.286 | 13.10 | 7.37 | |
| 30, 8 | 32, 4 | 0.306 | 0.301 | 12.38 | 6.95 | |
| 34, 4 | 26, 10 | 0.315 | 0.283 | 6.59 | 16.34 | |
| 30, 10 | 32, 6 | 0.313 | 0.307 | 14.63 | 9.43 | |
| 28, 12 | 30, 8 | 0.307 | 0.300 | 17.60 | 12.38 | |
| 28, 10 | 30, 6 | 0.293 | 0.287 | 15.44 | 9.97 | |
| 30, 4 | 20, 18 | 0.275 | 0.282 | 7.37 | 28.35 | |
| 22, 12 | 24, 8 | 0.256 | 0.247 | 20.89 | 14.80 | |
| 24, 2 | 12, 14 | 0.227 | 0.207 | 5.60 | 32.36 | |
| 30, 0 | 16, 16 | 0.267 | 0.249 | 1.57 | 30.00 | |
| 30, 4 | 24, 10 | 0.284 | 0.269 | 7.37 | 17.35 | |
| 32, 4 | 26, 10 | 0.299 | 0.283 | 6.95 | 16.34 | |
| 36, 0 | 20, 20 | 0.311 | 0.302 | 1.32 | 30.00 | |
| 32, 6 | 26, 12 | 0.305 | 0.291 | 9.43 | 18.58 | |
| 30, 8 | 24, 14 | 0.298 | 0.287 | 12.38 | 21.79 | |
| 30, 6 | 24, 12 | 0.285 | 0.272 | 9.97 | 19.67 | |
| 32, 0 | 14, 24 | 0.271 | 0.283 | 1.48 | 38.21 | |
| 24, 8 | 18, 14 | 0.246 | 0.238 | 14.80 | 26.11 | |
| 18, 8 | 14, 10 | 0.210 | 0.192 | 18.35 | 24.92 | |
| 24, 6 | 18, 12 | 0.245 | 0.234 | 12.01 | 23.82 | |
| 24, 10 | 26, 6 | 0.267 | 0.260 | 17.35 | 11.24 | |
| 26, 10 | 28, 6 | 0.281 | 0.274 | 16.34 | 10.57 | |
| 30, 6 | 22, 16 | 0.289 | 0.287 | 9.97 | 25.05 | |
| 26, 12 | 28, 8 | 0.290 | 0.282 | 18.58 | 13.10 | |
| 24, 14 | 26, 10 | 0.285 | 0.276 | 21.79 | 16.34 | |
| 24, 12 | 26, 8 | 0.271 | 0.263 | 19.67 | 13.90 | |
| 26, 6 | 16, 20 | 0.250 | 0.265 | 11.24 | 33.48 | |
| 18, 14 | 20, 10 | 0.236 | 0.225 | 26.11 | 19.77 | |
| 14, 10 | 10, 12 | 0.190 | 0.175 | 24.92 | 32.75 | |
| 20, 8 | 14, 14 | 0.223 | 0.212 | 17.00 | 30.00 | |
| 20, 12 | 22, 8 | 0.247 | 0.229 | 22.26 | 15.82 | |
| 22, 12 | 24, 8 | 0.260 | 0.243 | 20.89 | 14.80 | |
| 26, 8 | 18, 18 | 0.266 | 0.270 | 13.90 | 30.00 | |
| 22, 14 | 24, 10 | 0.270 | 0.260 | 23.07 | 17.35 | |
| 20, 16 | 22, 12 | 0.267 | 0.256 | 26.52 | 20.89 | |
| 20, 14 | 22, 10 | 0.252 | 0.242 | 24.50 | 18.48 | |
| 22, 8 | 12, 22 | 0.229 | 0.253 | 15.82 | 39.11 | |
| 14, 16 | 16, 12 | 0.221 | 0.208 | 32.07 | 25.13 | |
| 12, 8 | 8, 10 | 0.160 | 0.145 | 24.01 | 33.30 | |
| 18, 6 | 12, 12 | 0.193 | 0.186 | 15.08 | 30.00 | |
| 18, 10 | 20, 6 | 0.217 | 0.208 | 21.25 | 13.90 | |
| 20, 10 | 22, 6 | 0.231 | 0.230 | 19.77 | 12.89 | |
| 24, 6 | 16, 16 | 0.237 | 0.239 | 12.01 | 30.00 | |
| 20, 12 | 22, 8 | 0.241 | 0.232 | 22.26 | 15.82 | |
| 18, 14 | 20, 10 | 0.237 | 0.226 | 26.11 | 19.77 | |
| 18, 12 | 20, 8 | 0.223 | 0.213 | 23.82 | 17.00 | |
| 20, 6 | 10, 20 | 0.201 | 0.224 | 13.90 | 40.23 | |
| 12, 14 | 14, 10 | 0.192 | 0.179 | 32.36 | 24.92 |
| Nucleus | hw | nhw | ||||
|---|---|---|---|---|---|---|
| 20, 8 | 22, 4 | 0.305 | 0.296 | 17.00 | 9.64 | |
| 20, 8 | 22, 4 | 0.302 | 0.293 | 17.00 | 9.64 | |
| 22, 4 | 14, 14 | 0.291 | 0.292 | 9.64 | 30.00 | |
| 16, 10 | 18, 6 | 0.272 | 0.259 | 22.95 | 15.08 | |
| 12, 12 | 14, 8 | 0.249 | 0.232 | 30.00 | 21.79 | |
| 10, 10 | 12, 6 | 0.209 | 0.193 | 30.00 | 20.17 | |
| 20, 8 | 22, 4 | 0.302 | 0.293 | 17.00 | 9.64 | |
| 20, 8 | 22, 4 | 0.300 | 0.291 | 17.00 | 9.64 | |
| 22, 4 | 14, 14 | 0.288 | 0.289 | 9.64 | 30.00 | |
| 16, 10 | 18, 6 | 0.270 | 0.257 | 22.95 | 15.08 | |
| 12, 12 | 14, 8 | 0.247 | 0.230 | 30.00 | 21.79 | |
| 10, 10 | 12, 6 | 0.207 | 0.191 | 30.00 | 20.17 | |
| 22, 4 | 16, 10 | 0.291 | 0.275 | 9.64 | 22.95 | |
| 22, 4 | 16, 10 | 0.288 | 0.272 | 9.64 | 22.95 | |
| 24, 0 | 8, 20 | 0.282 | 0.295 | 1.95 | 43.00 | |
| 18, 6 | 18, 12 | 0.255 | 0.246 | 15.08 | 30.00 | |
| 14, 8 | 14, 14 | 0.228 | 0.228 | 21.79 | 38.21 | |
| 12, 6 | 12, 12 | 0.190 | 0.190 | 20.17 | 39.83 | |
| 16, 10 | 18, 6 | 0.272 | 0.259 | 22.95 | 15.08 | |
| 16, 10 | 18, 6 | 0.270 | 0.257 | 22.95 | 15.08 | |
| 18, 6 | 10, 16 | 0.255 | 0.267 | 15.08 | 37.05 | |
| 12, 12 | 14, 8 | 0.245 | 0.228 | 30.00 | 21.79 | |
| 8, 14 | 10, 10 | 0.226 | 0.205 | 38.21 | 30.00 | |
| 6, 12 | 8, 8 | 0.188 | 0.167 | 39.83 | 30.00 | |
| 12, 12 | 14, 8 | 0.249 | 0.232 | 30.00 | 21.79 | |
| 12, 12 | 14, 8 | 0.247 | 0.230 | 30.00 | 21.79 | |
| 14, 8 | 6, 18 | 0.228 | 0.253 | 21.79 | 44.92 | |
| 8, 14 | 10, 10 | 0.226 | 0.205 | 38.21 | 30.00 | |
| 4, 16 | 6, 12 | 0.214 | 0.188 | 47.48 | 39.83 | |
| 2, 14 | 4, 10 | 0.177 | 0.150 | 51.05 | 42.22 | |
| 10, 10 | 12, 6 | 0.209 | 0.193 | 30.00 | 20.17 | |
| 10, 10 | 12, 8 | 0.207 | 0.191 | 30.00 | 20.17 | |
| 12, 6 | 4, 16 | 0.190 | 0.216 | 20.17 | 47.48 | |
| 6, 12 | 8, 8 | 0.188 | 0.167 | 39.83 | 30.00 | |
| 2, 14 | 4, 10 | 0.177 | 0.150 | 51.05 | 42.22 | |
| 0, 12 | 2, 8 | 0.143 | 0.114 | 56.33 | 46.10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bonatsos, D.; Martinou, A.; Peroulis, S.K.; Petrellis, D.; Vasileiou, P.; Mertzimekis, T.J.; Minkov, N. Robustness of the Proxy-SU(3) Symmetry in Atomic Nuclei and the Role of the Next-Highest-Weight Irreducible Representation. Symmetry 2024, 16, 1625. https://doi.org/10.3390/sym16121625
Bonatsos D, Martinou A, Peroulis SK, Petrellis D, Vasileiou P, Mertzimekis TJ, Minkov N. Robustness of the Proxy-SU(3) Symmetry in Atomic Nuclei and the Role of the Next-Highest-Weight Irreducible Representation. Symmetry. 2024; 16(12):1625. https://doi.org/10.3390/sym16121625
Chicago/Turabian StyleBonatsos, Dennis, Andriana Martinou, Spyridon K. Peroulis, Dimitrios Petrellis, Polytimos Vasileiou, Theodoros J. Mertzimekis, and Nikolay Minkov. 2024. "Robustness of the Proxy-SU(3) Symmetry in Atomic Nuclei and the Role of the Next-Highest-Weight Irreducible Representation" Symmetry 16, no. 12: 1625. https://doi.org/10.3390/sym16121625
APA StyleBonatsos, D., Martinou, A., Peroulis, S. K., Petrellis, D., Vasileiou, P., Mertzimekis, T. J., & Minkov, N. (2024). Robustness of the Proxy-SU(3) Symmetry in Atomic Nuclei and the Role of the Next-Highest-Weight Irreducible Representation. Symmetry, 16(12), 1625. https://doi.org/10.3390/sym16121625







