Photon Acceleration by Superluminal Ionization Fronts
Abstract
1. Introduction
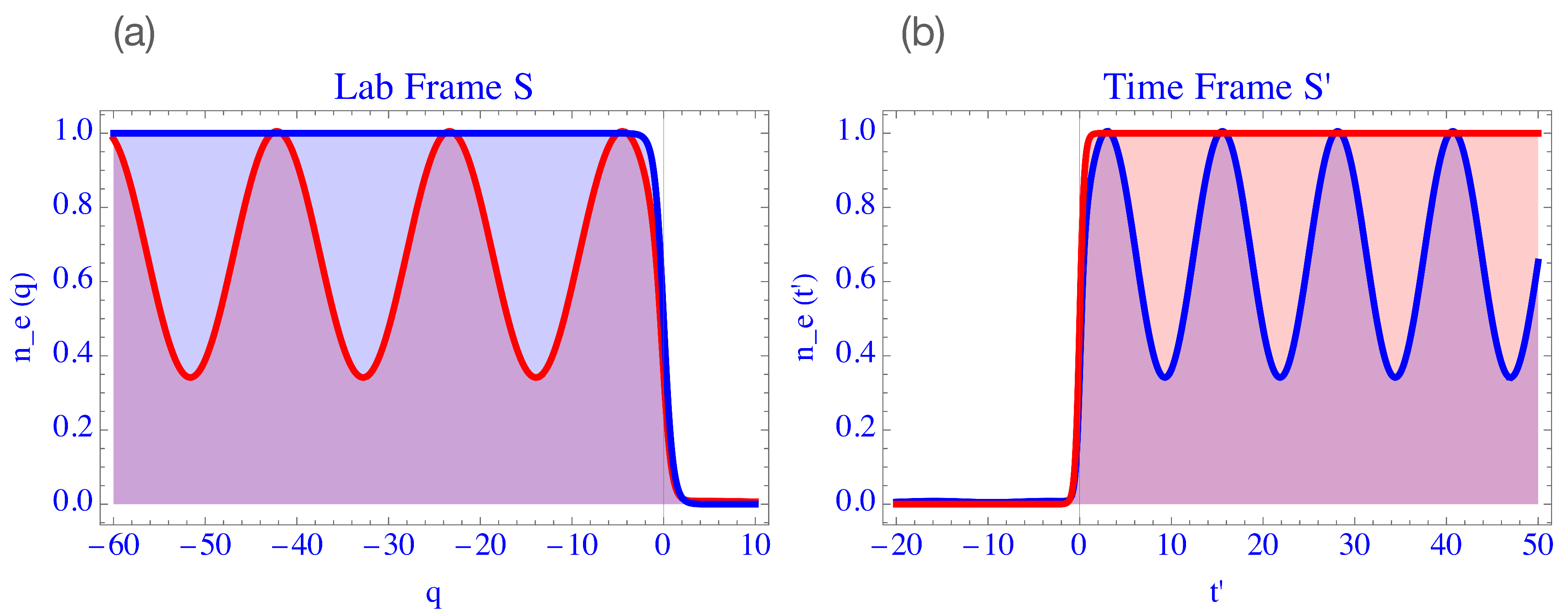
2. Time Frame
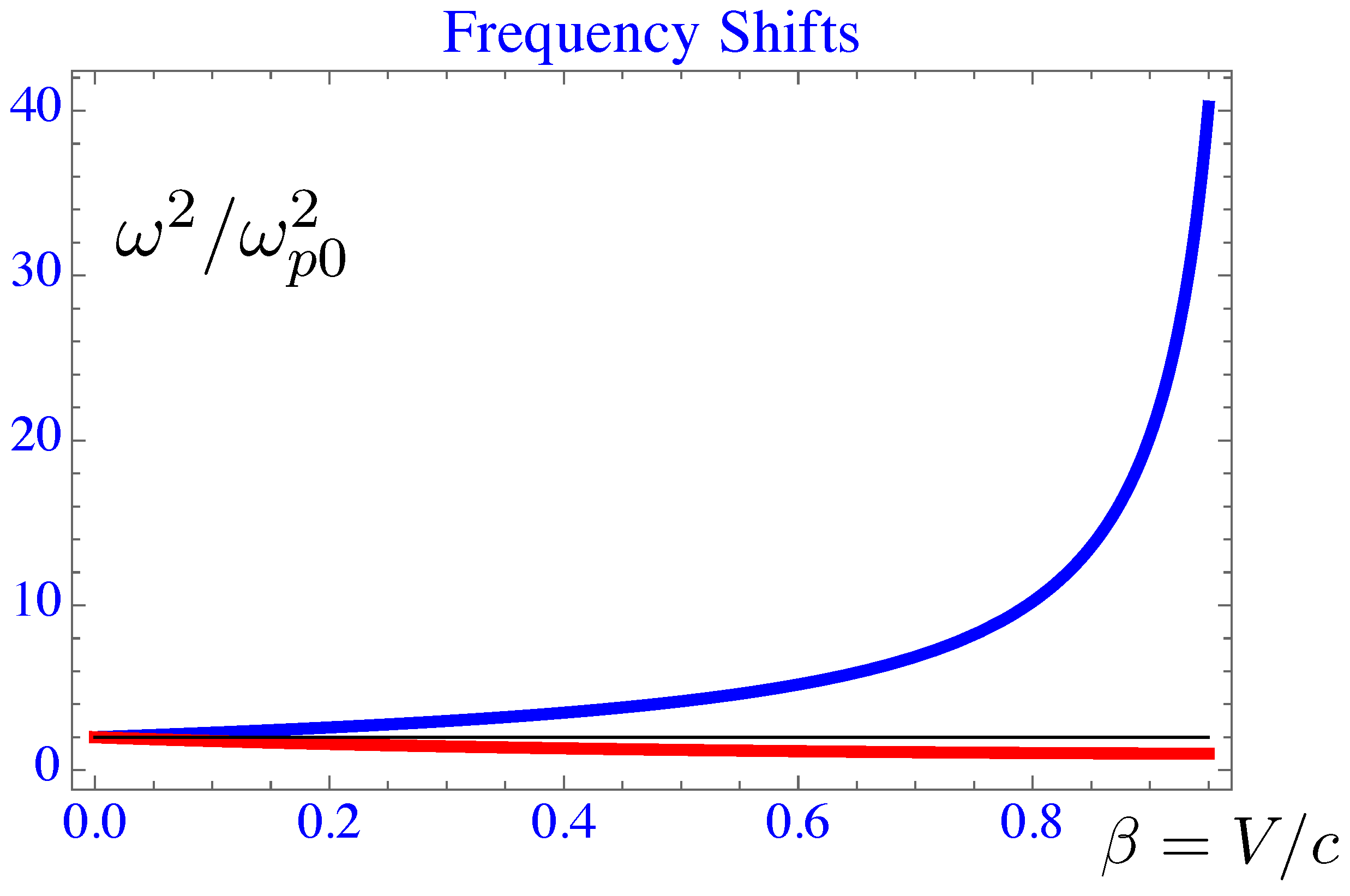
3. Frequency Shifts
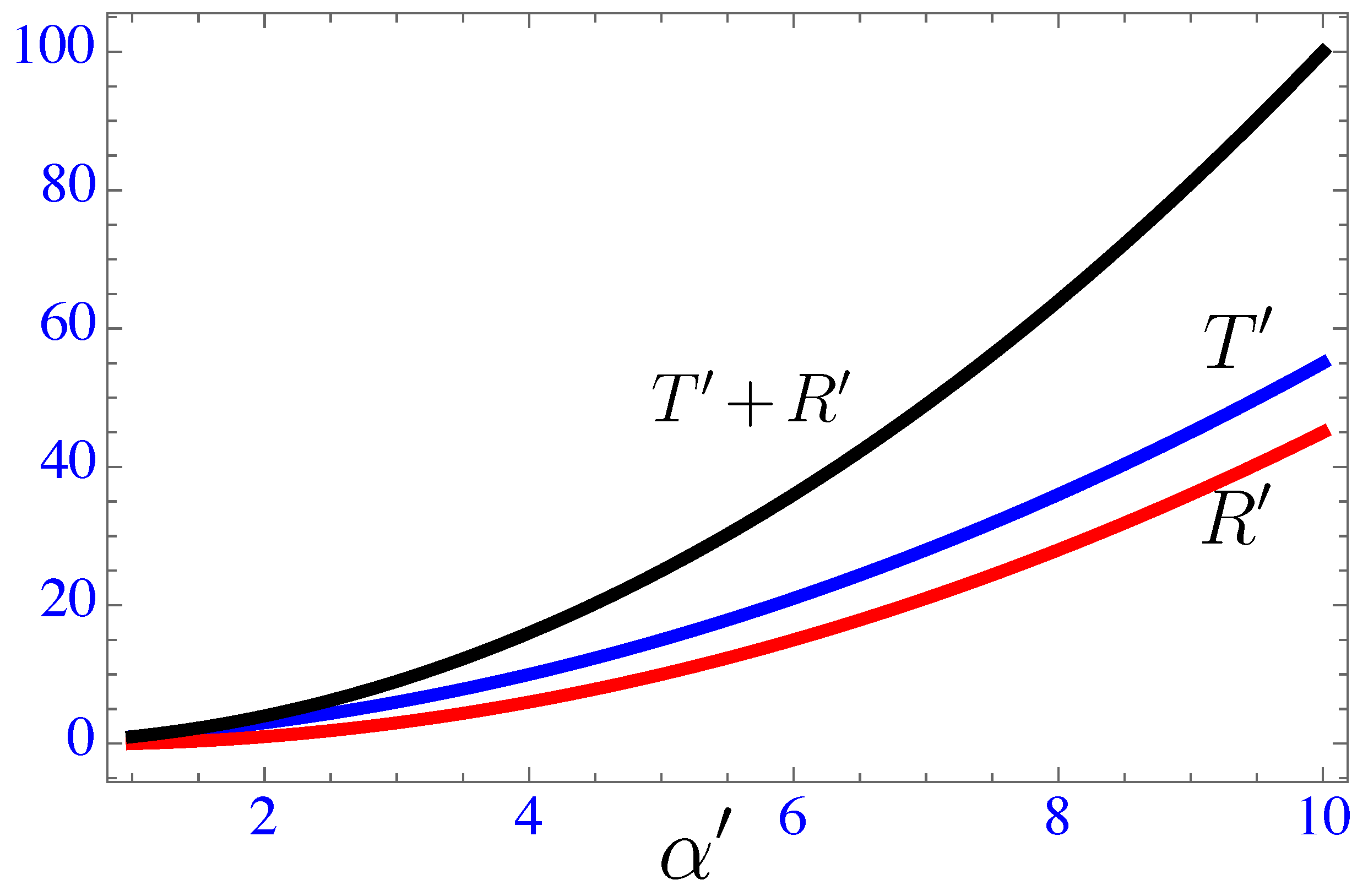
4. Field Transformations
5. Modulated Fronts
6. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Semenova, V.I. Reflection of Electromagnetic Waves from an Ionization Front. Radiophys. Quantum Electron. 1967, 10, 599–604. [Google Scholar] [CrossRef]
- Lampe, M.; Ott, E.; Walker, J.H. Interaction of Electromagnetic Waves with a Moving Ionization Front. Phys. Fluids 1978, 21, 42. [Google Scholar]
- Mendonça, J.T. Nonlinear Interaction of Wavepackets. J. Plasma Phys. 1979, 22, 15. [Google Scholar] [CrossRef]
- Wilks, S.C.; Dawson, J.M.; Mori, W.B.; Katsouleas, T.; Jones, M.E. Photon Accelerator. Phys. Rev. Lett. 1989, 62, 2600. [Google Scholar] [CrossRef] [PubMed]
- Mendonça, J.T. Theory of Photon Acceleration; Institute of Physics Publishing: Bristol, UK, 2001. [Google Scholar]
- Boyd, R.W. Nonlinear Optics; Academic Press: San Diego, CA, USA, 1992. [Google Scholar]
- Silva, L.O.; Mendonça, J.T. Photon Kinetic Theory of Self-Phase Modulation. Opt. Commun. 2001, 196, 285. [Google Scholar] [CrossRef]
- Alfano, R.R. (Ed.) Ths Supercontinuum Laser Source, 4th ed.; Springer Nature: Cham, Switzerland, 2022. [Google Scholar]
- Silva, L.O.; Mendonça, J.T. Photon Acceleration in Superluminous and Accelerated Ionization Fronts. IEEE Trans. Plasma Sci. 1996, 24, 316. [Google Scholar]
- Savage, R.L., Jr.; Joshi, C.; Mori, W.B. Frequency Upconversion of Electromagnetic Radiation upon Transmission into an Ionization Front. Phys. Rev. Lett. 1992, 68, 946. [Google Scholar] [CrossRef]
- Dias, J.M.; Stenz, C.; Lopes, N.; Badiche, X.; Blasco, F.; Santos, A.D.; Silva, L.O.e.; Mysyrowicz, A.; Antonetti, A.; Mendonça, J.T. Experimental Evidence of Photon Acceleration of Ultrashort Laser Pulses in Relativistic Ionization Fronts. Phys. Rev. Lett. 1997, 78, 4773. [Google Scholar]
- Dias, J.M.; Lopes, N.C.; Silva, L.O.; Figueira, G.; Mendonça, J.T.; Stenz, C.; Blasco, F.; Santos, A.D.; Mysyrowicz, A. Photon Acceleration of Ultrashort Laser Pulses by Relativistic Ionization Fronts. Phys. Rev. E 2002, 66, 056406. [Google Scholar] [CrossRef]
- Lopes, N.C.; Figueira, G.; Dias, J.M.; Silva, L.O.; Mendonça, J.T.; Balcou, P.; Rey, G.; Stenz, C. Laser Pulse Frequency Up-shifts by Relativistic Ionization Fronts. EuroPhys. Lett. 2004, 66, 371. [Google Scholar]
- Sainte-Marie, A.; Gobert, O.; Quéré, F. Controlling the Velocity of Ultrashort Light Pulses in Vacuum through Spatio-temporal Couplings. Optica 2017, 4, 1298. [Google Scholar] [CrossRef]
- Froula, D.H.; Turnbull, D.; Davies, A.S.; Kessler, T.J.; Haberberger, D.; Palastro, J.P.; Bahk, S.; Begishev, I.A.; Boni, R.; Bucht, S.; et al. Spatiotemporal Control of Laser Intensity. Nat. Photon. 2018, 12, 262. [Google Scholar]
- Franke, P.; Turnbull, D.; Katz, J.; Palastro, J.P.; Begishev, I.A.; Bromage, J.; Shaw, J.L.; Boni, R.; Froula, D.H. Measurement and Control of Large Diameter Ionization Waves of Arbitrary Velocity. Opt. Express 2019, 27, 31978. [Google Scholar] [PubMed]
- Li, Z.; Liu, Y.; Leng, Y.; Li, R. Investigating Group-Velocity-Tunable Propagation-Invariant Optical Wave-Packets. Sci. Rep. 2022, 12, 16102. [Google Scholar] [CrossRef] [PubMed]
- Agarwal, K.; Bhatt, R.N.; Sondhi, S.L. Fast Preparation of Critical Ground States using Superluminal Fronts. Phys. Rev. Lett. 2018, 120, 210604. [Google Scholar] [CrossRef]
- Kondakci, H.E.; Abouraddy, A.F. Optical Space-time Wave Packets of Arbitrary Group Velocity in Free Space. Nat. Commun. 2019, 10, 929. [Google Scholar] [CrossRef] [PubMed]
- Yessenov, M.; Free, J.; Chen, Z.; Johnson, E.G.; Lavery, M.P.L.; Alonso, M.A.; Abouraddy, A.F. Space-time Wave Packets Localized in All Dimensions. Nat. Commun. 2022, 13, 4573. [Google Scholar]
- Mendonça, J.T.; Shukla, P.K. Time Refraction and Time Reflection: Two Basic Concepts. Phys. Scr. 2002, 65, 160. [Google Scholar] [CrossRef]
- Plansinis, B.W.; Donaldson, W.R.; Agrawal, G.P. What is the Temporal Analog of Reflection and Refraction of Optical Beams. Phys. Rev. Lett. 2015, 115, 183901. [Google Scholar] [CrossRef]
- Malaca, B.; Pardal, M.; Ramsey, D.; Pierce, J.R.; Weichman, K.; Andriyash, I.A.; Mori, W.B.; Palastro, J.P.; Fonseca, R.A.; Vieira, J. Coherence and Superradiance from a Plasma-Based Quasiparticle Accelerator. Nat. Photon. 2023, 18, 39–45. [Google Scholar] [CrossRef]
- Mendonça, J.T.; Guerreiro, A.; Martins, A.M. Quantum Theory of Time Refraction. Phys. Rev. A 2000, 62, 033805. [Google Scholar] [CrossRef]
- Mendonça, J.T.; Martins, A.M.; Guerreiro, A. Temporal Beam Splitter and Temporal Interference. Phys. Rev. A 2003, 68, 043801. [Google Scholar] [CrossRef]
- Mendonça, J.T. The Quantum Nature of Light; Institute of Physics Publishing: Bristol, UK, 2022. [Google Scholar]
- Mendonça, J.T. Temporal Klein Model for Particle-Pair Creation. Symmetry 2021, 13, 1361. [Google Scholar] [CrossRef]
- Mendonça, J.T. Particle-pair Creation by High-harmonic Laser Fields. Phys. Src. 2023, 98, 125606. [Google Scholar]
- Mendonça, J.T.; Guerreiro, A. Time Refraction and the Quantum Properties of Vacuum. Phys. Rev. A 2005, 72, 063805. [Google Scholar] [CrossRef]
- Ginzburg, V.L. Propagation of Electromagnetic Waves in Plasmas; Gordon and Breach: New York, NY, YSA, 1961. [Google Scholar]
- Kline, M.; Kay, I.W. Electromagnetic Theory and Geometric Optics; John Wiley & Sons: New York, NY, USA, 1965. [Google Scholar]
- Shcherbakov, M.R.; Lemasters, R.; Fan, Z.; Song, J.; Lian, T.; Harutyunyan, H.; Shvets, G. Time-Variant Metasurfaces Enable Tunable Spectral Bands of Negative Extinction. Optica 2019, 6, 1441. [Google Scholar] [CrossRef]
- Liu, C.; Alam, M.Z.; Pang, K.; Manukyan, K.; Reshef, O.; Zhou, Y.; Choudhary, S.; Patrow, J.; Pennathurs, A.; Song, H.; et al. Photon Acceleration Using a Time-Varying Epsilon-Near-Zero Metasurface. ACS Photonics 2021, 8, 716. [Google Scholar]
- Yuan, L.; Fan, S. Temporal Modulation Brings Metamaterials into New Era. Light Sci. Appl. 2022, 11, 173. [Google Scholar]
- Castaldi, G.; Rizza, C.; Engheta, N.; Galdi, V. Multiple Actions of Time-resolved Short-pulsed Metamaterials. Appl. Phys. Lett. 2023, 122, 021701. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mendonça, J.T. Photon Acceleration by Superluminal Ionization Fronts. Symmetry 2024, 16, 112. https://doi.org/10.3390/sym16010112
Mendonça JT. Photon Acceleration by Superluminal Ionization Fronts. Symmetry. 2024; 16(1):112. https://doi.org/10.3390/sym16010112
Chicago/Turabian StyleMendonça, José Tito. 2024. "Photon Acceleration by Superluminal Ionization Fronts" Symmetry 16, no. 1: 112. https://doi.org/10.3390/sym16010112
APA StyleMendonça, J. T. (2024). Photon Acceleration by Superluminal Ionization Fronts. Symmetry, 16(1), 112. https://doi.org/10.3390/sym16010112





