New Three Wave and Periodic Solutions for the Nonlinear (2+1)-Dimensional Burgers Equations
Abstract
:1. Introduction
2. Hirota Bilinear Scheme and Its Application
2.1. The Bilinear Form Polynomials
2.2. New Three-Wave Solutions
2.3. New Periodic Wave Solutions
3. Analytical Solitons
3.1. EShGEE Scheme
3.2. Application of EShGEE Scheme
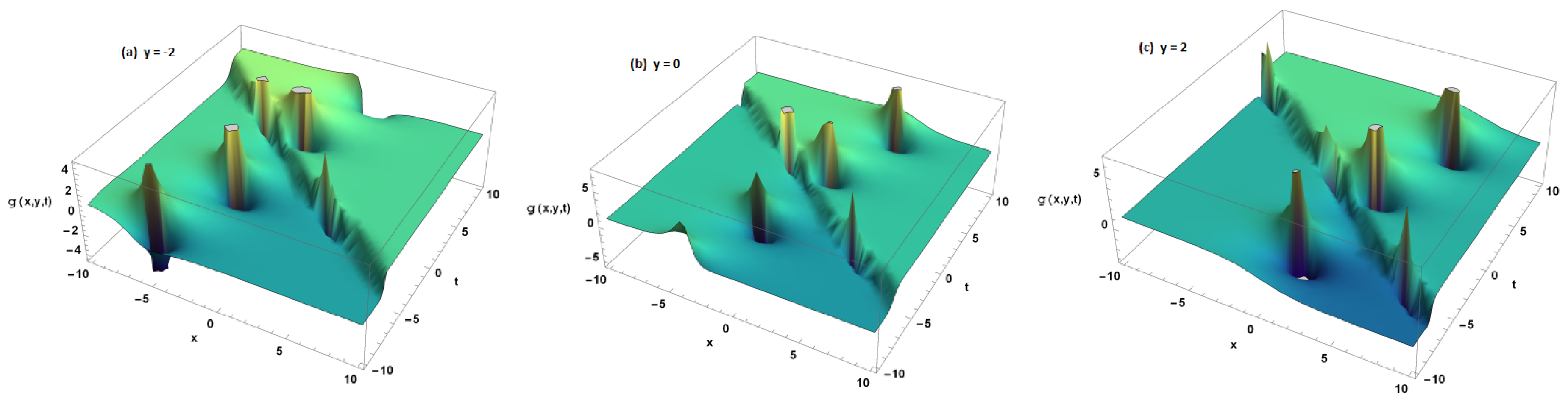
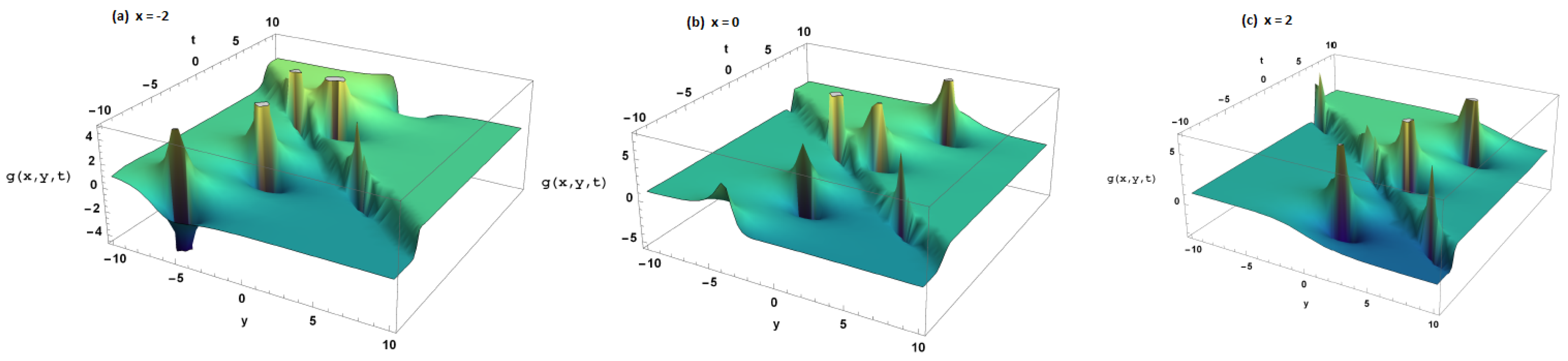
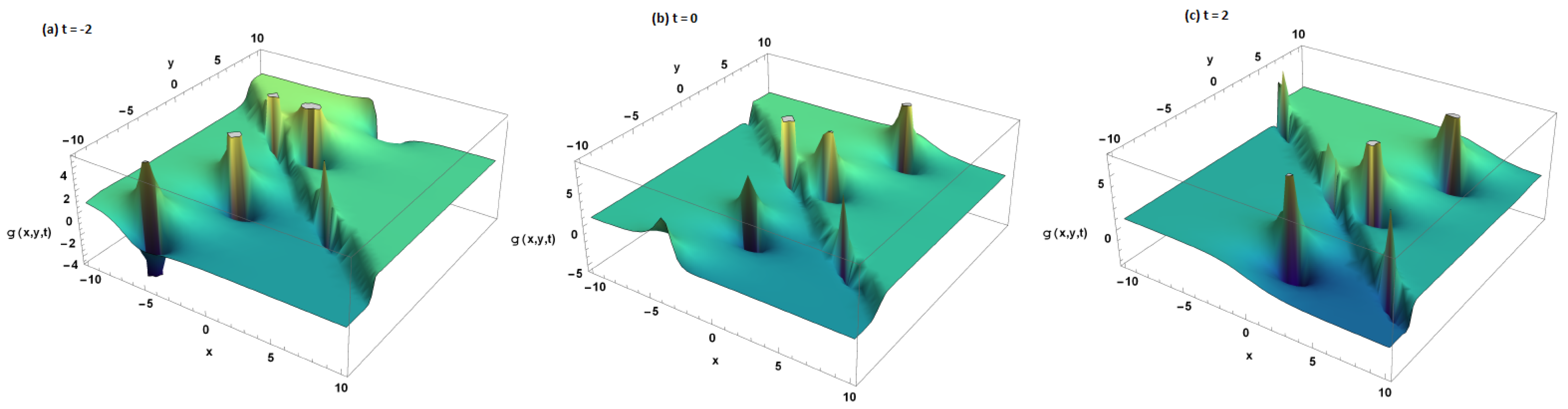
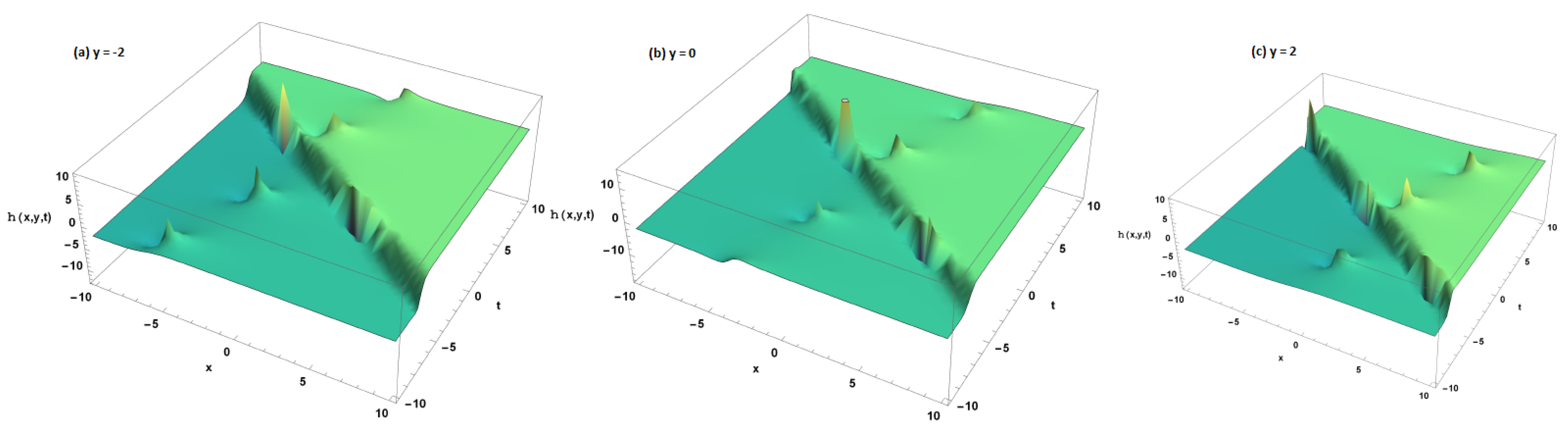
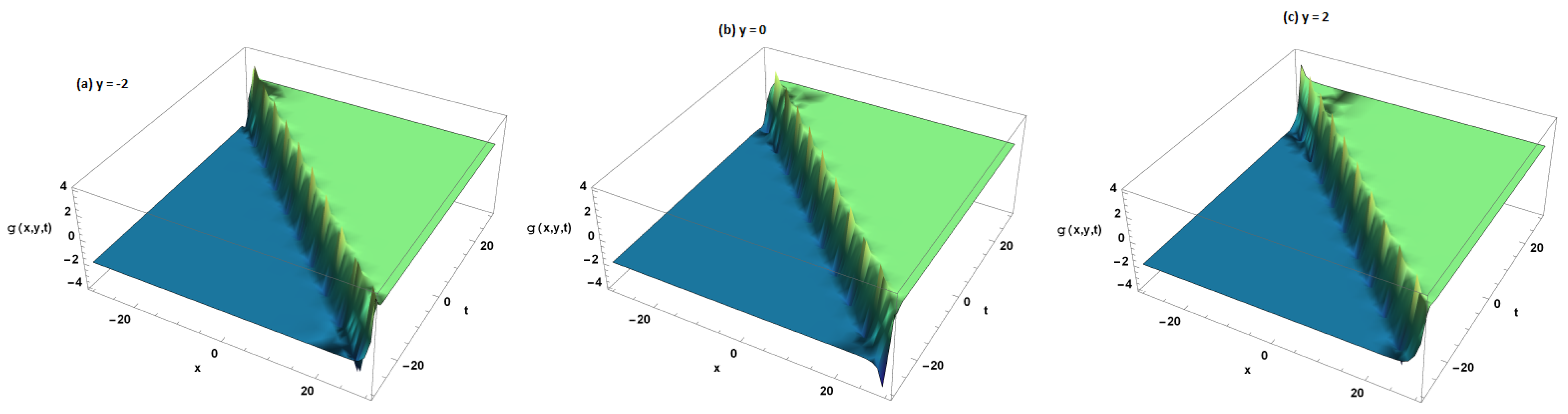
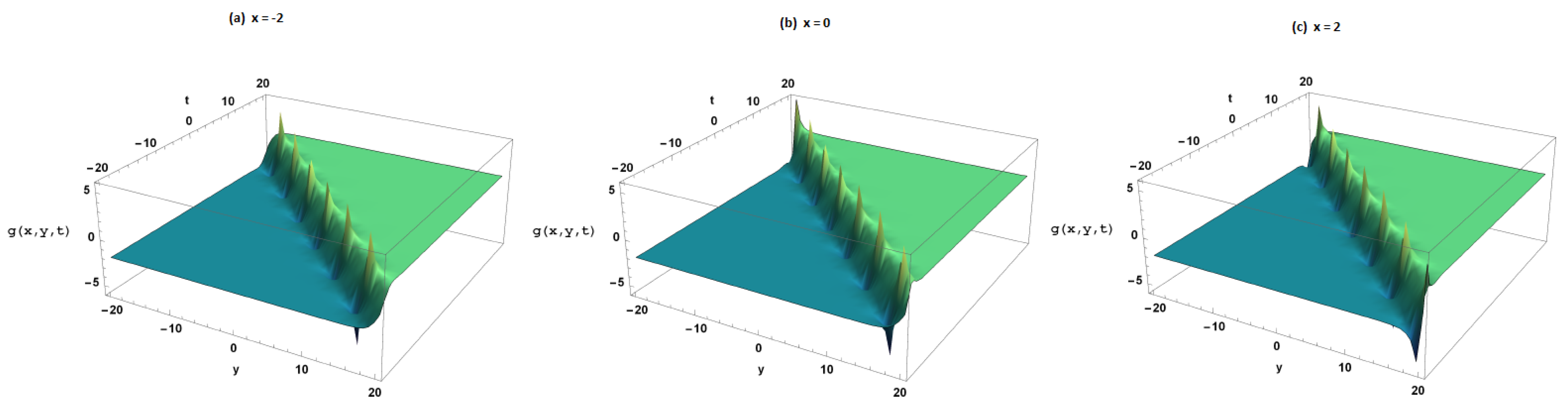
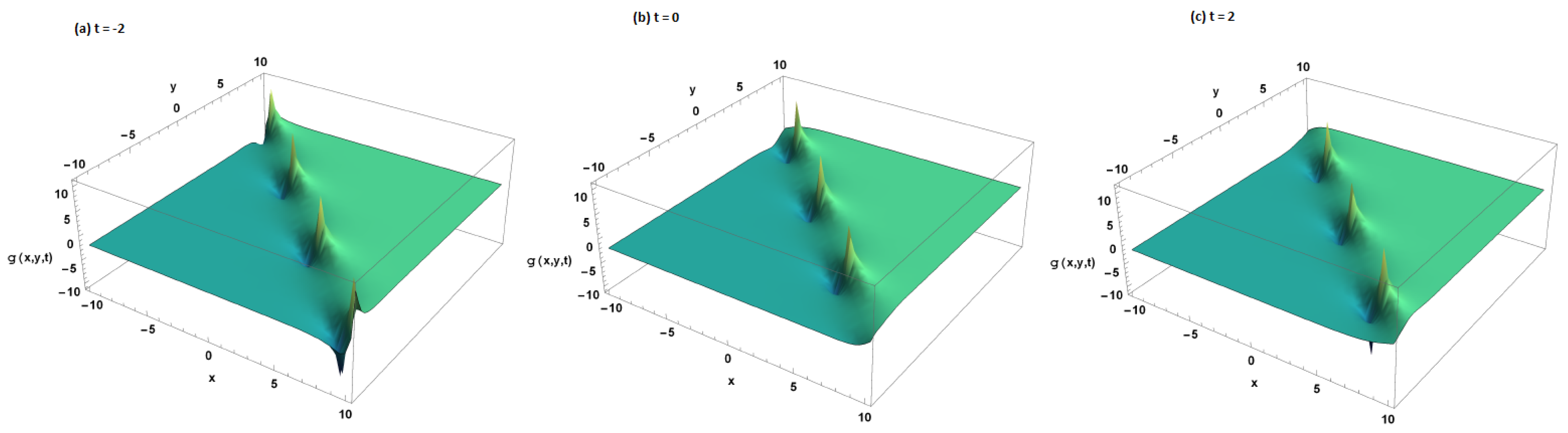
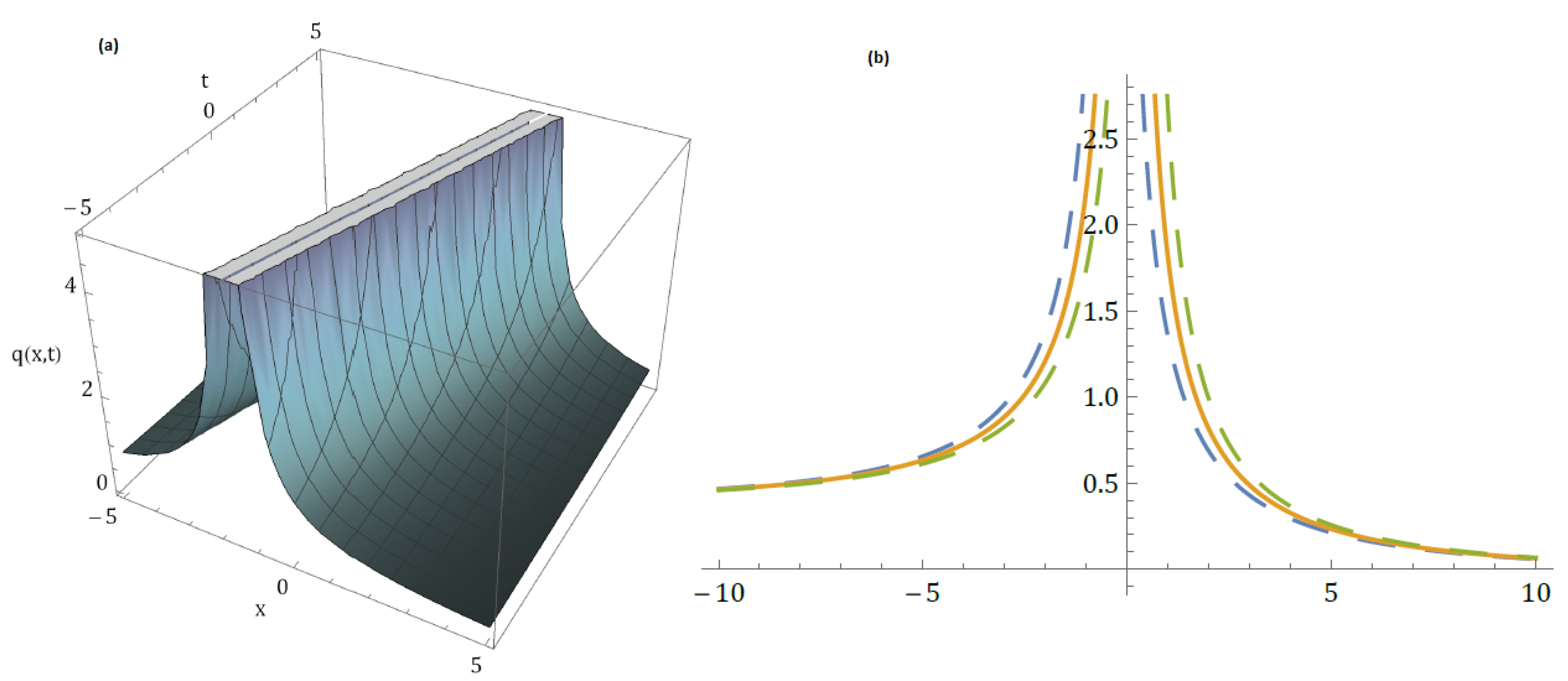
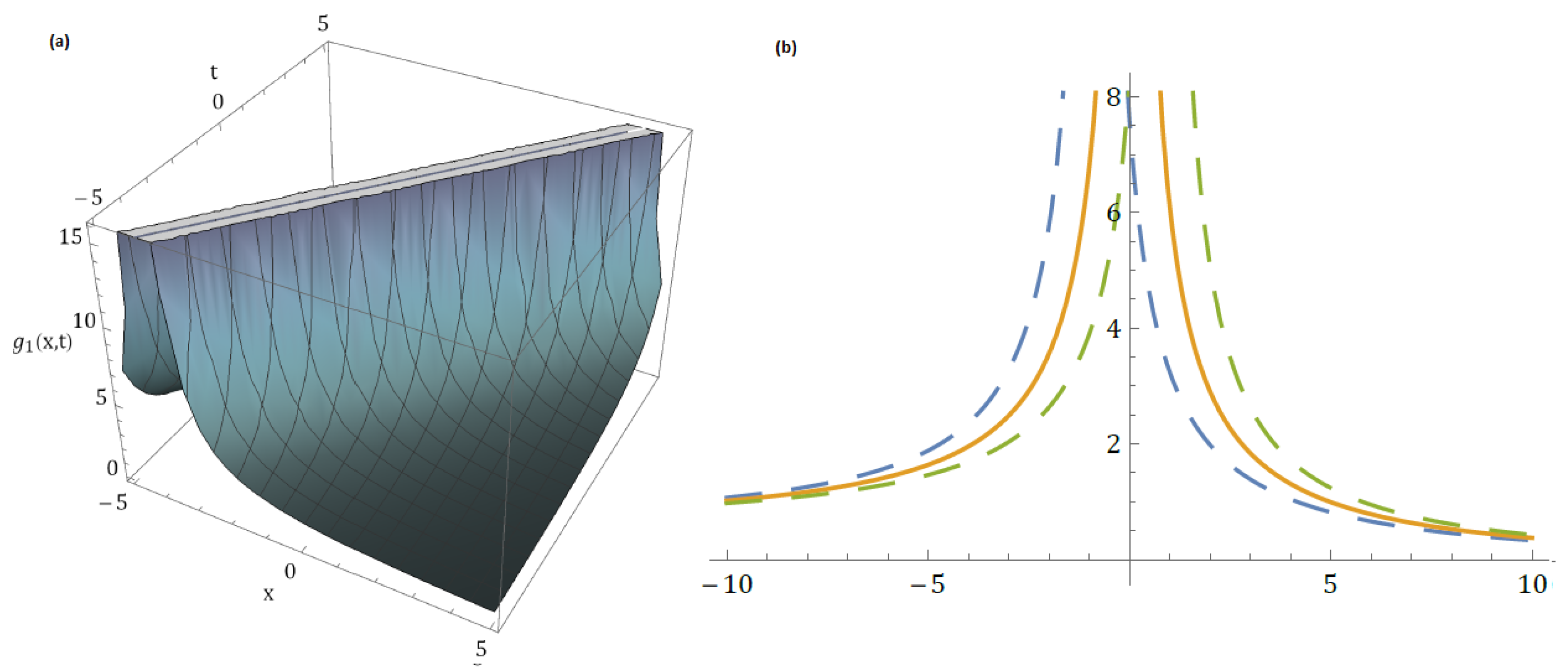
4. Graphical Representation of Solutions
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sandeep, M.; Kumar, S.; Biswas, A.; Yıldırım, Y.; Moraru, L.; Moldovanu, S.; Iticescu, C.; Alotaibi, A. Highly dispersive optical solitons in the absence of self-phase modulation by lie symmetry. Symmetry 2023, 15, 886. [Google Scholar]
- Sachin, K.; Biswas, A.; Yıldırım, Y.; Moraru, L.; Moldovanu, S.; Alshehri, H.M.; Maturi, D.A.; Al-Bogami, D.H. Cubic–quartic optical soliton perturbation with differential group delay for the Lakshmanan–Porsezian–Daniel model by Lie symmetry. Symmetry 2022, 14, 224. [Google Scholar]
- Asghari, Y.; Eslami, M.; Rezazadeh, H. Exact solutions to the conformable time-fractional discretized mKdv lattice system using the fractional transformation method. Opt. Quantum Electron. 2023, 55, 318. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Method for finding highly dispersive optical solitons of nonlinear differential equations. Optik 2020, 206, 163550. [Google Scholar] [CrossRef]
- Iqbal, M.A.; Wang, Y.; Miah, M.M.; Osman, M.S. Study on date–Jimbo–Kashiwara–Miwa equation with conformable derivative dependent on time parameter to find the exact dynamic wave solutions. Fractal Fract. 2021, 6, 4. [Google Scholar] [CrossRef]
- Wu, G.; Guo, Y. New Complex Wave Solutions and Diverse Wave Structures of the (2+1)-Dimensional Asymmetric Nizhnik–Novikov–Veselov Equation. Fractal Fract. 2023, 7, 170. [Google Scholar] [CrossRef]
- Almusawa, H.; Jhangeer, A. A study of the soliton solutions with an intrinsic fractional discrete nonlinear electrical transmission line. Fractal Fract. 2022, 6, 334. [Google Scholar] [CrossRef]
- Areshi, M.; Seadawy, A.R.; Ali, A.; Alharbi, A.F.; Aljohani, A.F. Analytical Solutions of the Predator–Prey Model with Fractional Derivative Order via Applications of Three Modified Mathematical Methods. Fractal Fract. 2023, 7, 128. [Google Scholar] [CrossRef]
- Abdelwahed, H.G.; Alsarhana, A.F.; El-Shewy, E.K.; Abdelrahman, M.A.E. The Stochastic Structural Modulations in Collapsing Maccari’s Model Solitons. Fractal Fract. 2023, 7, 290. [Google Scholar] [CrossRef]
- Zafar, A.; Raheel, M.; Rezazadeh, H.; Inc, M.; Akinlar, M.A. New chirp-free and chirped form optical solitons to the non-linear Schrödinger equation. Opt. Quantum Electron. 2021, 53, 604. [Google Scholar] [CrossRef]
- Rahman, Z.; Abdeljabbar, A.; Ali, M.Z. Novel precise solitary wave solutions of two time fractional nonlinear evolution models via the MSE scheme. Fractal Fract. 2022, 6, 444. [Google Scholar] [CrossRef]
- Shqair, M.; Alabedalhadi, M.; Al-Omari, S.; Al-Smadi, M. Abundant exact travelling wave solutions for a fractional massive Thirring model using extended Jacobi elliptic function method. Fractal Fract. 2022, 6, 252. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Al-Askar, F.M.; Cesarano, C.; El-Morshedy, M. On the Dynamics of Solitary Waves to a (3+1)-Dimensional Stochastic Boiti–Leon–Manna–Pempinelli Model in Incompressible Fluid. Mathematics 2023, 11, 2390. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Cesarano, C. The soliton solutions for the (4+1)-dimensional stochastic Fokas equation. Math. Methods Appl. Sci. 2023, 46, 7589–7597. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Albosaily, S.; Iqbal, N.; El-Morshedy, M. The effect of multiplicative noise on the exact solutions of the stochastic Burgers’ equation. Waves Random Complex Media 2021, 1–13. [Google Scholar] [CrossRef]
- Liu, J.; Du, J.; Zeng, Z.; Nie, B. New three-wave solutions for the (3+1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Nonlinear Dyn. 2017, 88, 655–661. [Google Scholar] [CrossRef]
- Ilhan, O.A.; Manafian, J. Periodic type and periodic cross-kink wave solutions to the (2+1)-dimensional breaking soliton equation arising in fluid dynamics. Mod. Phys. Lett. B 2019, 33, 1950277. [Google Scholar] [CrossRef]
- Ali, K.K.; Raheel, M.; Inc, M. Some new types of optical solitons to the time-fractional new hamiltonian amplitude equation via extended Sinh-Gorden equation expansion method. Mod. Phys. Lett. B 2022, 36, 2250089. [Google Scholar] [CrossRef]
- Zafar, A.; Bekir, A.; Raheel, M.; Razzaq, W. Optical soliton solutions to Biswas–Arshed model with truncated M-fractional derivative. Optik 2020, 222, 165355. [Google Scholar] [CrossRef]
- Wang, Q.; Chen, Y.; Zhang, H.Q. A new Riccati equation rational expansion method and its application to (2+1)-dimensional Burgers equation. Chaos Solitons Fractals 2005, 25, 1019–1028. [Google Scholar] [CrossRef]
- Wang, H. Lump and interaction solutions to the (2+1)-dimensional Burgers equation. Appl. Math. Lett. 2018, 85, 27–34. [Google Scholar] [CrossRef]
- Wang, D.-S.; Li, H.-B.; Wang, J. The novel solutions of auxiliary equation and their application to the (2+1)-dimensional Burgers equations. Chaos Solitons Fractals 2008, 38, 374–382. [Google Scholar] [CrossRef]
- Li, Z.; Manafian, J.; Ibrahimov, N.; Hajar, A.; Nisar, K.S.; Jamshed, W. Variety interaction between k-lump and k-kink solutions for the generalized Burger equation with variable coefficients by bilinear analysis. Results Phys. 2021, 28, 104490. [Google Scholar] [CrossRef]
- Yang, X.L.; Tang, J.S. Travelling wave solutions for Konopelchenko-Dubrovsky equation using an extended sinh-Gordon equation expansion method. Commun. Theor. Phys. 2008, 50, 10471051. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Razzaq, W.; Zafar, A.; Alsharidi, A.K.; Alomair, M.A. New Three Wave and Periodic Solutions for the Nonlinear (2+1)-Dimensional Burgers Equations. Symmetry 2023, 15, 1573. https://doi.org/10.3390/sym15081573
Razzaq W, Zafar A, Alsharidi AK, Alomair MA. New Three Wave and Periodic Solutions for the Nonlinear (2+1)-Dimensional Burgers Equations. Symmetry. 2023; 15(8):1573. https://doi.org/10.3390/sym15081573
Chicago/Turabian StyleRazzaq, Waseem, Asim Zafar, Abdulaziz Khalid Alsharidi, and Mohammed Ahmed Alomair. 2023. "New Three Wave and Periodic Solutions for the Nonlinear (2+1)-Dimensional Burgers Equations" Symmetry 15, no. 8: 1573. https://doi.org/10.3390/sym15081573
APA StyleRazzaq, W., Zafar, A., Alsharidi, A. K., & Alomair, M. A. (2023). New Three Wave and Periodic Solutions for the Nonlinear (2+1)-Dimensional Burgers Equations. Symmetry, 15(8), 1573. https://doi.org/10.3390/sym15081573







