Polarization of Gravitational Waves in Modified Gravity
Abstract
1. Introduction
2. Basic Outline of the Modified Gravity
3. Scalar Field
3.1. Case-I
3.2. Case-II
4. The Friedmann-Lemaître-Robertson-Walker Universe
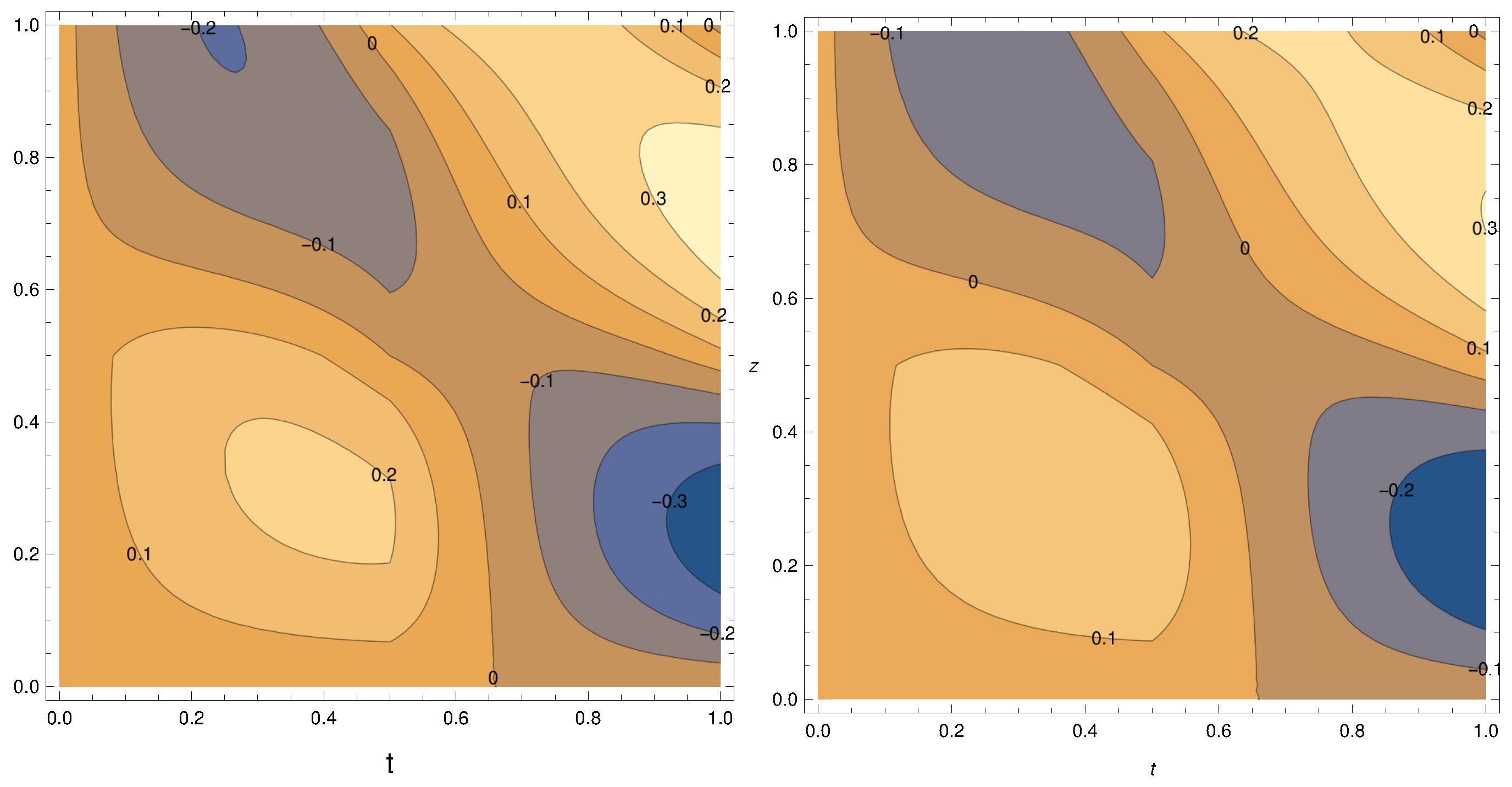
5. Polarization Modes of the Modified Gravity
Newman-Penrose Formalism
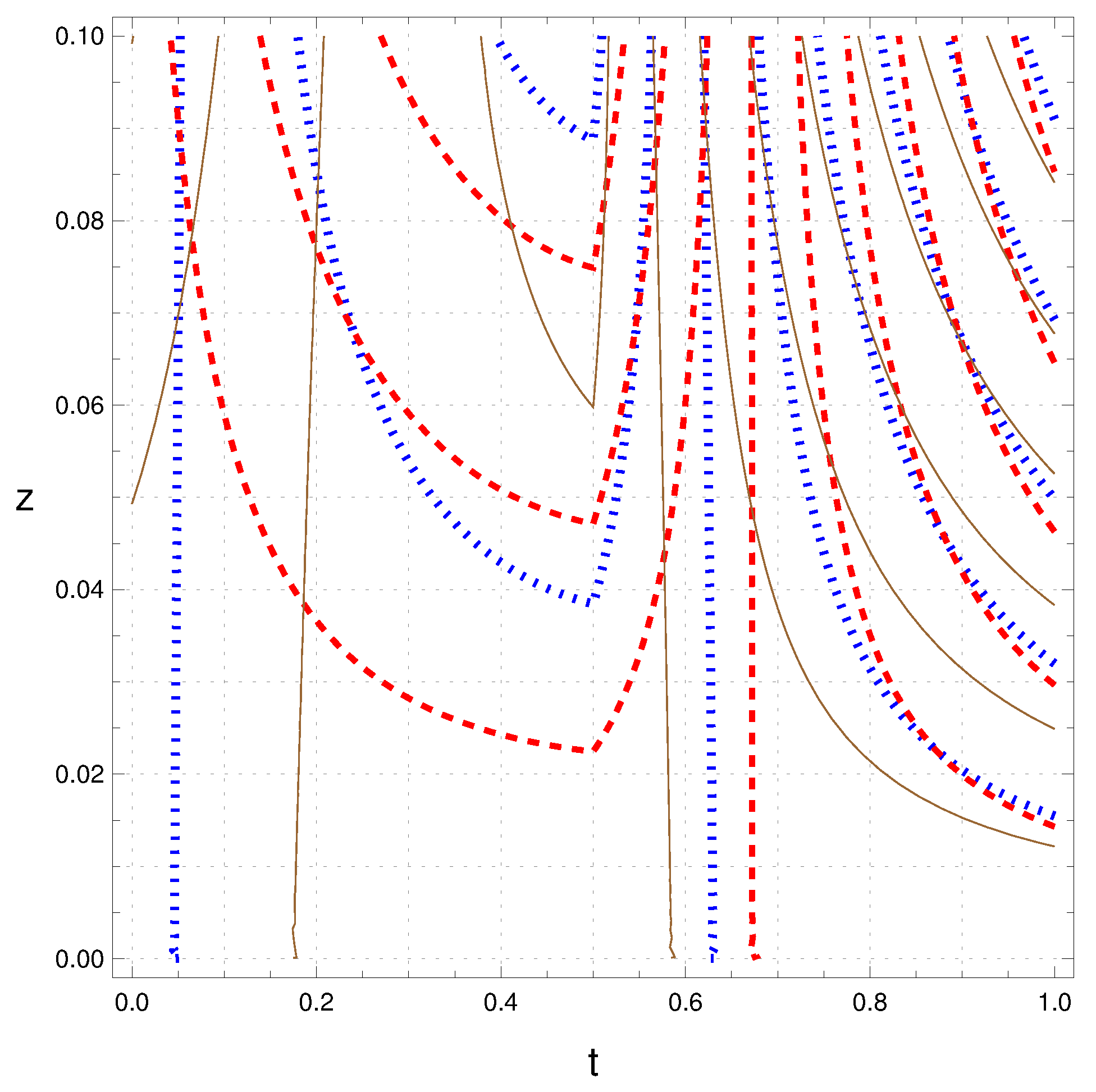
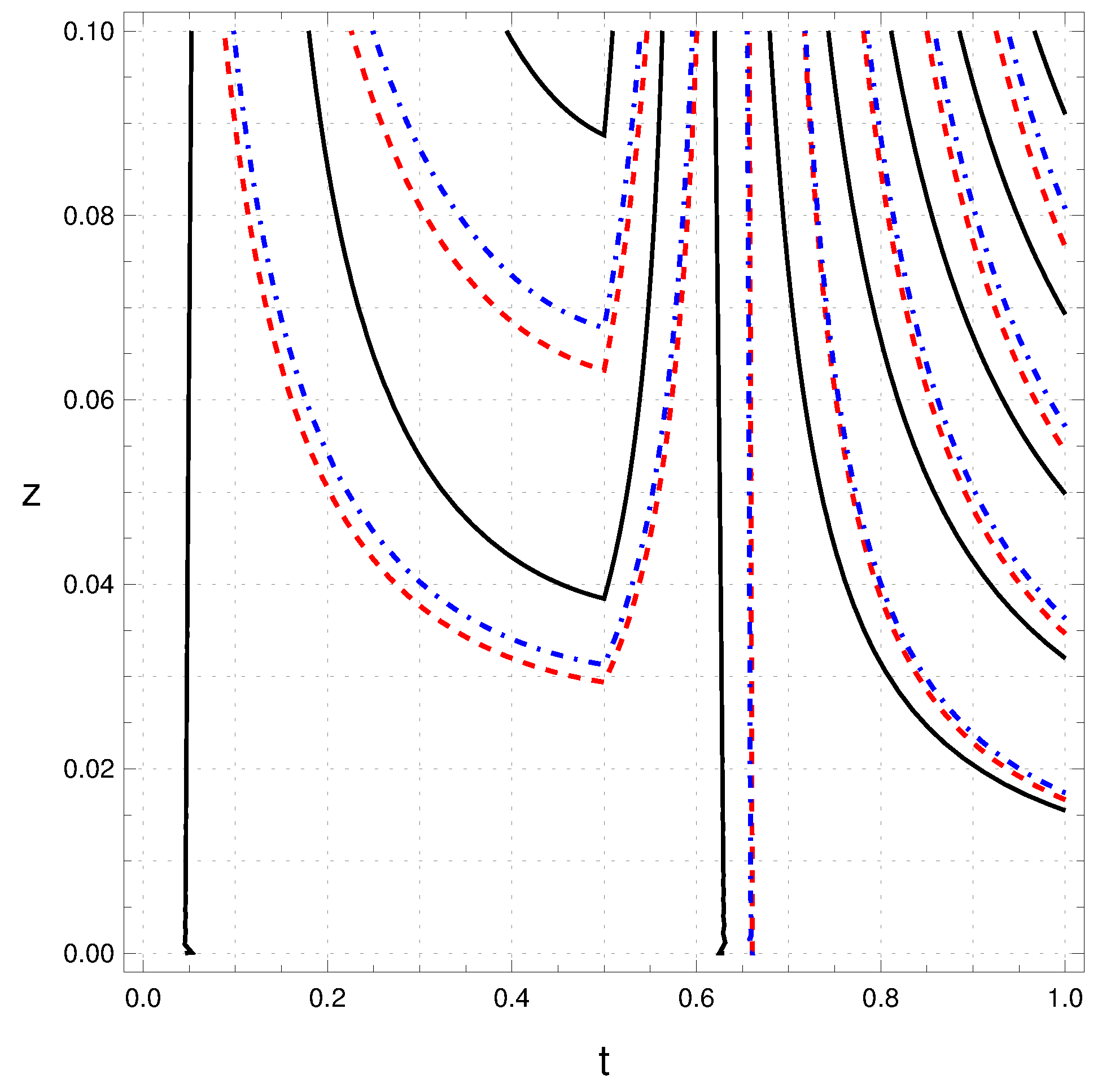
6. Detection of GWs’ Polarization Modes
7. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ade, P.A.; Aghanim, N.; Arnaud, M.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Banday, A.J.; Barreiro, R.B.; Bartlett, J.G.; Bartolo, N.; et al. Planck 2015 results-XIII. Cosmological parameters. Astron. Astrophys. 2016, 594, A13. [Google Scholar]
- Padmanabhan, T. Cosmological constant—The weight of the vacuum. Phys. Rep. 2003, 380, 235. [Google Scholar] [CrossRef]
- Clifton, T.; Ferreira, P.G.; Padilla, A.; Skordis, C. Modified gravity and cosmology. Phys. Rep. 2012, 513, 1. [Google Scholar] [CrossRef]
- Kehagias, A. A conical tear drop as a vacuum-energy drain for the solution of the cosmological constant problem. Phys. Lett. B 2004, 600, 133. [Google Scholar] [CrossRef]
- Harko, T.; Lobo, F.S.N.; Nojiri, S.; Odintsov, S.D. f(R,T) gravity. Phys. Rev. D 2011, 84, 024020. [Google Scholar] [CrossRef]
- Deser, S.; van Nieuwenhuizen, P. One-loop divergences of quantized Einstein-Maxwell fields. Phys. Rev. D 1974, 10, 401. [Google Scholar] [CrossRef]
- Stelle, K.S. Renormalization of higher-derivative quantum gravity. Phys. Rev. D 1977, 16, 953. [Google Scholar] [CrossRef]
- Ostrogradsky, M. Mémoires sur les équations différentielles, relatives au problème des isopérimètres. Mem. Acad. St. Petersbourg 1850, 6, 385. [Google Scholar]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef]
- Abbott, B.P. Multi-messenger Observations of a Binary Neutron Star Merger. Astrophys. J. Lett. 2017, 848, L12. [Google Scholar] [CrossRef]
- Hagihara, Y.; Era, N.; Iikawa, D.; Asada, H. Probing gravitational wave polarizations with Advanced LIGO, Advanced Virgo and KAGRA. Phys. Rev. D 2018, 98, 064035. [Google Scholar] [CrossRef]
- Fesik, L. Polarization states of gravitational waves detected by LIGO-Virgo antennas. arXiv 2017, arXiv:1706.09505. [Google Scholar]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170814: A Three-Detector Observation of Gravitational Waves from a Binary Black Hole Coalescence. Phys. Rev. Lett. 2017, 119, 141101. [Google Scholar] [CrossRef]
- Baker, T.; Bellini, E.; Ferreira, P.G.; Lagos, M.; Noller, J.; Sawicki, I. Strong Constraints on Cosmological Gravity from GW170817 and GRB 170817A. Phys. Rev. Lett. 2017, 119, 251301. [Google Scholar] [CrossRef] [PubMed]
- Will, C.M. The Confrontation between General Relativity and Experiment. Living Rev. Relativ. 2017, 17, 4. [Google Scholar] [CrossRef]
- Chatziioannou, K.; Yunes, N.; Cornish, N. Model-independent test of general relativity: An extended post-Einsteinian framework with complete polarization content. Phys. Rev. D 2012, 86, 022004. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. Tests of general relativity with the binary black hole signals from the LIGO-Virgo catalog GWTC-1. Phys. Rev. D 2019, 100, 104036. [Google Scholar] [CrossRef]
- Hou, S.; Gong, Y.; Liu, Y. Polarizations of gravitational waves in Horndeski theory. Eur. Phys. J. C 2018, 78, 378. [Google Scholar] [CrossRef]
- Alves, M.E.S.; Miranda, O.D.; de Araujo, J.C.N. Probing the f(R) formalism through gravitational wave polarizations. Phys. Lett. B 2009, 679, 401. [Google Scholar] [CrossRef]
- Chowdhury, S.R.; Khlopov, M. Gravitational waves in the extended theory of gravity. Int. J. Mod. Phys. D 2021, 30, 2140011. [Google Scholar] [CrossRef]
- Gogoi, D.J.; Dev Goswami, U. A new f(R) gravity model and properties of gravitational waves in it. Eur. Phys. J. C 2020, 80, 1101. [Google Scholar] [CrossRef]
- Oikonomou, V.K. Amplification of the primordial gravitational waves energy spectrum by a kinetic scalar in f(R) gravity. Astropart. Phys. 2023, 144, 102777. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K.; Myrzakulov, R. Spectrum of Primordial Gravitational Waves in Modified Gravities: A Short Overview. Symmetry 2022, 14, 729. [Google Scholar] [CrossRef]
- Kausar, H.R.; Philippoz, L.; Jetzer, P. Gravitational wave polarization modes in f(R) theories. Phys. Rev. D 2016, 93, 124071. [Google Scholar] [CrossRef]
- Hölscher, P. Gravitational waves and degrees of freedom in higher derivative gravity. Phys. Rev. D 2019, 99, 064039. [Google Scholar] [CrossRef]
- Roshan, M.; Shojai, F. Energy-momentum squared gravity. Phys. Rev. D 2016, 94, 044002. [Google Scholar] [CrossRef]
- Alves, M.E.S.; Moraes, P.H.R.S.; de Araujo, J.C.N.; Malheiro, M. Gravitational waves in f(R,T) and f(R,Tϕ) theories of gravity. Phys. Rev. D 2016, 94, 024032. [Google Scholar] [CrossRef]
- Jenet, F.A.; Hobbs, G.B.; Lee, K.J.; Manchester, R.N. Detecting the Stochastic Gravitational Wave Background Using Pulsar Timing. Astrophys. J. 2017, 625, L123. [Google Scholar] [CrossRef]
- Lee, K.J.; Jenet, F.A.; Price, R.H. Pulsar Timing as a Probe of Non-Einsteinian Polarizations of Gravitational Waves. Astrophys. J. 2008, 685, 1304. [Google Scholar] [CrossRef]
- Lee, K.J.; Wex, N.; Kramer, M.; Stappers, B.W.; Bassa, C.G.; Janssen, G.H.; Karuppusamy, R.; Smits, R. Gravitational wave astronomy of single sources with a pulsar timing array. Mon. Not. R. Astron. Soc. 2011, 414, 3251. [Google Scholar] [CrossRef]
- Burke-Spolaor, S.; Taylor, S.R.; Charisi, M.; Dolch, T.; Hazboun, J.S.; Holgado, A.M.; Kelley, L.Z.; Lazio, T.J.W.; Madison, D.R.; McMann, N.; et al. The astrophysics of nanohertz gravitational waves. Astron. Astrophys. Rev. 2019, 27, 5. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. First Search for Nontensorial Gravitational Waves from Known Pulsars. Phys. Rev. Lett. 2018, 120, 031104. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. Search for Tensor, Vector, and Scalar Polarizations in the Stochastic Gravitational-Wave Background. Phys. Rev. Lett. 2018, 120, 201102. [Google Scholar] [CrossRef]
- Aso, Y.; Michimura, Y.; Somiya, K.; Ando, M.; Miyakawa, O.; Sekiguchi, T.; Tatsumi, D.; Yamamoto, H.; Kagra Collaboration. Interferometer design of the KAGRA gravitational wave detector. Phys. Rev. D 2013, 88, 043007. [Google Scholar] [CrossRef]
- Iyer, B.; Souradeep, T.; Unnikrishnan, C.S.; Dhurandhar, S.; Raja, S.; Sengupta, A. LIGO-India: A Critical Element of the International Network of Gravitational Wave Detectors; Technical Report No. LIGO-M1100296; Indian Initiative in Gravitational-wave Observations: Dughala, India, 2011. [Google Scholar]
- Moraes, P.H.R.S.; Santos, J.R.L. A complete cosmological scenario from f(R,Tϕ) gravity theory. Eur. Phys. J. C 2016, 76, 60. [Google Scholar] [CrossRef]
- Newman, E.; Penrose, R. An Approach to Gravitational Radiation by a Method of Spin Coefficients. J. Math. Phys. 1962, 4, 566, Erratum in J. Math. Phys. 1963, 3, 998. [Google Scholar] [CrossRef]
- Eardley, D.M.; Lee, D.L.; Lightman, A.P. Gravitational-Wave Observations as a Tool for Testing Relativistic Gravity. Phys. Rev. D 1973, 8, 3308. [Google Scholar] [CrossRef]
- Eardley, D.M.; Lee, D.L.; Lightman, A.P.; Wagoner, R.V.; Will, C.M. Gravitational-wave observations as a tool for testing relativistic gravity. Phys. Rev. Lett. 1973, 30, 884–886. [Google Scholar] [CrossRef]
- Lee, K.; Jenet, F.A.; Price, R.H.; Wex, N.; Kramer, M. Detecting massive gravitons using Pulsar timing arrays. Astrophys. J. 2010, 722, 1589. [Google Scholar] [CrossRef]
- Lee, K.J. Pulsar timing arrays and gravity tests in the radiative regime. Class. Quantum Gravit. 2013, 30, 224016. [Google Scholar] [CrossRef]
- Manchester, R.N.; Hobbs, G.; Bailes, M.; Coles, W.A.; Van Straten, W.; Keith, M.J.; Shannon, R.M.; Bhat, N.D.; Brown, A.; Burke-Spolaor, S.G.; et al. The Parkes Pulsar Timing Array Project. Publ. Astron. Soc. Aust. 2013, 30, E017. [Google Scholar] [CrossRef]
- Tiburzi, C.; Hobbs, G.; Kerr, M.; Coles, W.A.; Dai, S.; Manchester, R.N.; Possenti, A.; Shannon, R.M.; You, X.P. A study of spatial correlations in pulsar timing array data. Mon. Not. R. Astron. Soc. 2015, 455, 4339. [Google Scholar] [CrossRef]
- Arzoumanian, Z.; Brazier, A.; Burke-Spolaor, S.; Chamberlin, S.; Chatterjee, S.; Christy, B.; Cordes, J.M.; Cornish, N.; Crowter, K.; Demorest, P.B.; et al. The Nanograv Nine-Year Data Set: Observations, Arrival Time Measurements, and Analysis of 37 Millisecond Pulsars. Astrophys. J. 2015, 813, 65. [Google Scholar]
- Arzoumanian, Z.; Brazier, A.; Burke-Spolaor, S.; Chamberlin, S.; Chatterjee, S.; Christy, B.; Cordes, J.M.; Cornish, N.J.; Crawford, F.; Cromartie, H.T.; et al. The NANOGrav 11-year Data Set: High-precision Timing of 45 Millisecond Pulsars. Astrophys. J. 2018, 235, 37. [Google Scholar] [CrossRef]
- Verbiest, J.P.W.; Lentati, L.; Hobbs, G.; van Haasteren, R.; Demorest, P.B.; Janssen, G.H.; Wang, J.B.; Desvignes, G.; Caballero, R.N.; Keith, M.J.; et al. The International Pulsar Timing Array: First data release. Mon. Not. R. Astron. Soc. 2016, 458, 1267. [Google Scholar] [CrossRef]
- Perera, B.B.P.; DeCesar, M.E.; Demorest, P.B.; Kerr, M.; Lentati, L.; Nice, D.J.; Osłowski, S.; Ransom, S.M.; Keith, M.J.; Arzoumanian, Z.; et al. The International Pulsar Timing Array: Second data release. Mon. Not. R. Astron. Soc. 2019, 490, 4666. [Google Scholar] [CrossRef]
- Corda, C. Interferometric detection of gravitational waves: The definitive test for General Relativity. Int. J. Mod. Phys. D 2009, 18, 2275. [Google Scholar] [CrossRef]
- Joachim, N.; Philippe, J.; Mauro, S. On gravitational waves in spacetimes with a nonvanishing cosmological constant. Phys. Rev. D 2009, 79, 024014. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khlopov, M.; Chowdhury, S.R. Polarization of Gravitational Waves in Modified Gravity. Symmetry 2023, 15, 832. https://doi.org/10.3390/sym15040832
Khlopov M, Chowdhury SR. Polarization of Gravitational Waves in Modified Gravity. Symmetry. 2023; 15(4):832. https://doi.org/10.3390/sym15040832
Chicago/Turabian StyleKhlopov, Maxim, and Sourav Roy Chowdhury. 2023. "Polarization of Gravitational Waves in Modified Gravity" Symmetry 15, no. 4: 832. https://doi.org/10.3390/sym15040832
APA StyleKhlopov, M., & Chowdhury, S. R. (2023). Polarization of Gravitational Waves in Modified Gravity. Symmetry, 15(4), 832. https://doi.org/10.3390/sym15040832






