Classification of Blood Rheological Models through an Idealized Symmetrical Bifurcation
Abstract
1. Introduction
2. Materials and Methods
2.1. Governing Equations and Simulation Setup
2.2. Non-Newtonian Blood Rheological Models
2.3. Grid Generation and Mesh Convergence
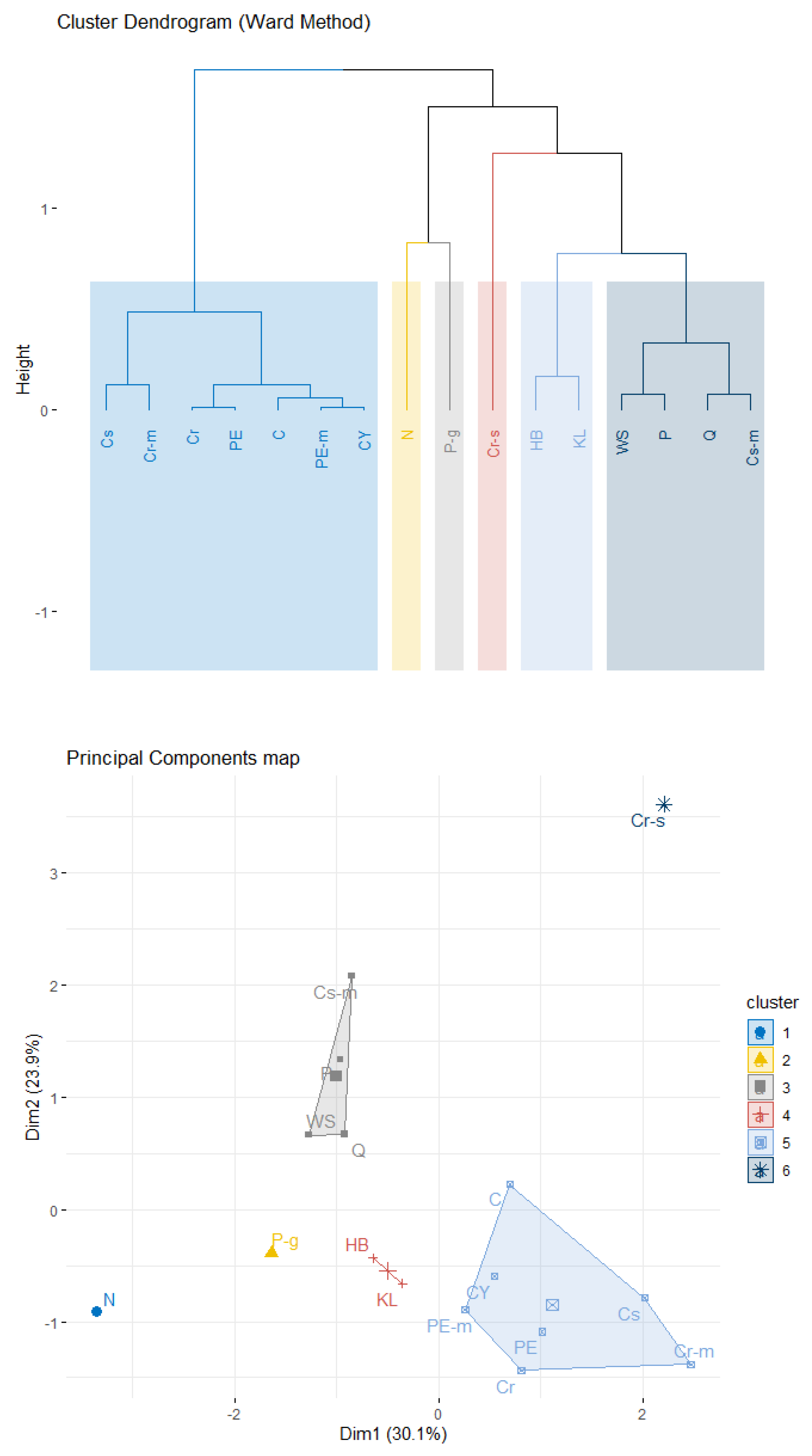
2.4. Statistical Analysis
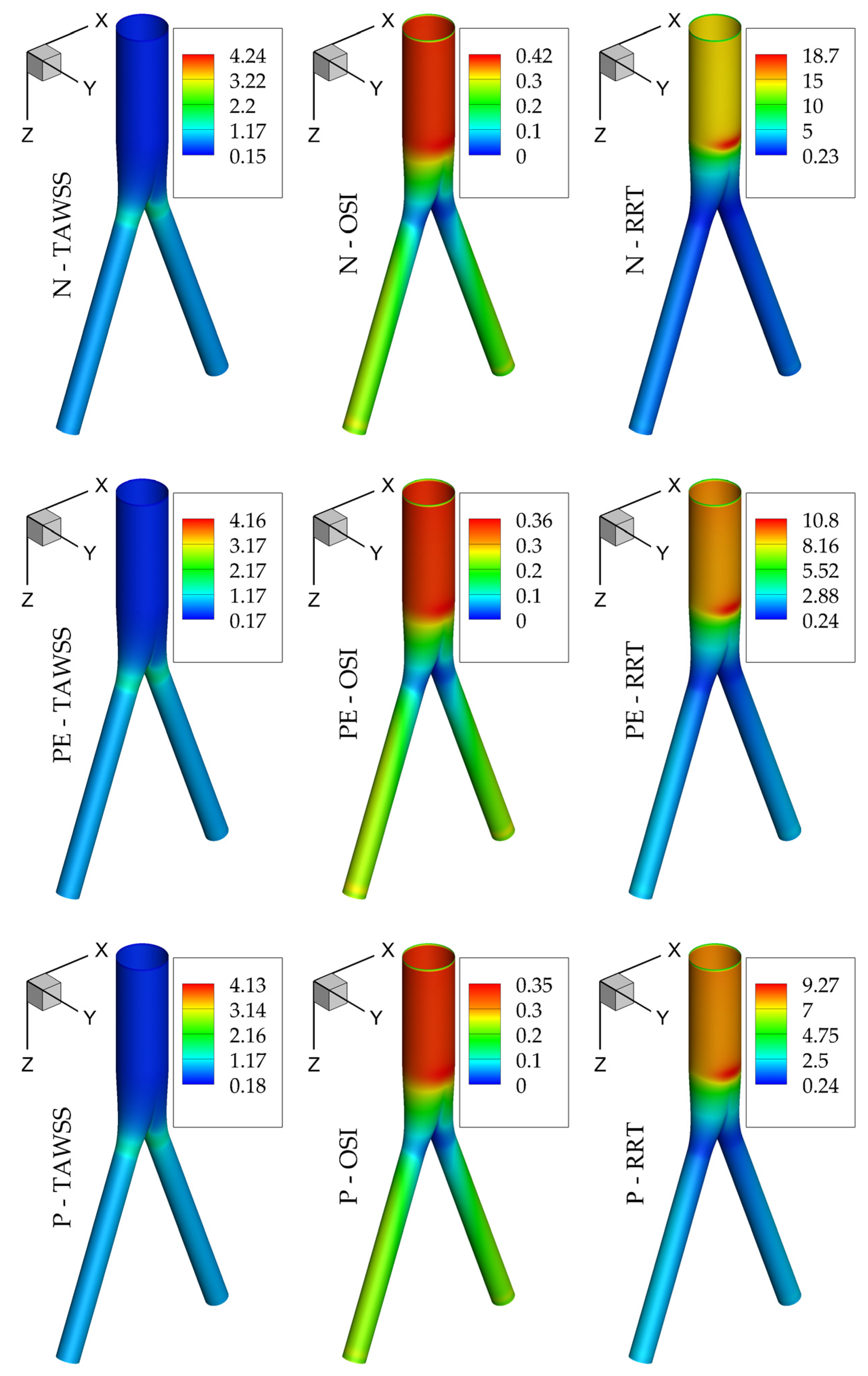
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| U | fluid velocity |
| p | fluid pressure |
| ρ | fluid density |
| τ | stress tensor |
| D | rate-of-deformation tensor |
| shear rate | |
| μ | dynamic viscosity |
| WSS | wall shear stress |
| TAWSS | time evarage wall shear stress |
| OSI | oscillatory shear index |
| RRT | relative residence time |
| IL | local non-Newtonian importance factor |
| IG | global non-Newtonian importance factor |
| NNEF | non-Newtonian effect factor |
| C | Carreau |
| CY | Carreau–Yasuda |
| Cs | Casson |
| Cs-m | Casson modified |
| Cr | Cross |
| Cr-m | Cross modified |
| Cr-s | Cross simplified |
| HB | Herschel–Bulkley |
| KL | Kuang–Luo |
| N | Newtonian |
| PE | Powell–Eyring |
| PE-m | Powell–Eyring modified |
| P | power-law |
| P-g | power-law generalized |
| Q | Quemada |
| WS | Walburn–Schneck |
| VIF | variance-inflation filtering |
| PCA | principal components analysis |
| IGPS | global non-Newtonian importance factor at peak systole |
| IGLD | global non-Newtonian importance factor at late diastole |
| ILPSmax | maximum of local non-Newtonian importance factor at peak systole |
| ILEDdec | decile of local non-Newtonian importance factor at early diastole |
| ILEDmax | maximum of local non-Newtonian importance factor at early diastole |
| ILLDmax | maximum of local non-Newtonian importance factor at late diastole |
| NNEFPSmin | minimum of non-Newtonian effect factor at peak systole |
| NNEFLDmin | minimum of non-Newtonian effect factor at late diastole |
References
- Kontopodis, N.; Metaxa, E.; Papaharilaou, Y.; Tavlas, E.; Tsetis, D.; Ioannou, C.V. Advancements in identifying biomechanical determinants for abdominal aortic aneurysm rupture. Vascular 2015, 23, 65–77. [Google Scholar] [CrossRef] [PubMed]
- Tzirakis, K.; Kamarianakis, Y.; Metaxa, E.; Kontopodis, N.; Ioannou, C.V.; Papaharilaou, Y. A robust approach for exploring hemodynamics and thrombus growth associations in abdominal aortic aneurysms. Med. Biol. Eng. Comput. 2017, 55, 1493–1506. [Google Scholar] [CrossRef] [PubMed]
- Georgakarakos, E.; Argyriou, C.; Schoretsanitis, N.; Ioannou, C.V.; Kontopodis, N.; Morgan, R.; Tsetis, D. Geometrical factors influencing the hemodynamic behavior of the AAA stent grafts: Essentials for the clinician. Cardiovasc. Interv. Radiol. 2014, 37, 1420–1429. [Google Scholar] [CrossRef] [PubMed]
- Jozwik, K.; Obidowski, D. Numerical simulations of the blood flow through vertebral arteries. J. Biomech. 2010, 43, 177–185. [Google Scholar] [CrossRef] [PubMed]
- Shahcheraghi, N.; Dwyer, H.A.; Cheer, A.Y.; Barakat, A.I.; Rutaganira, T. Unsteady and three-dimensional simulation of blood flow in the human aortic arch. J. Biomech. Eng. 2002, 124, 378–387. [Google Scholar] [CrossRef] [PubMed]
- Karimi, S.; Dabagh, M.; Vasava, P.; Dadvar, M.; Dabir, B.; Jalali, P. Effect of rheological models on the hemodynamics within human aorta: CFD study on CT image-based geometry. J. Non-Newton. Fluid Mech. 2014, 207, 42–52. [Google Scholar] [CrossRef]
- Chen, J.; Lu, X.-Y. Numerical investigation of the non-Newtonian pulsatile blood flow in a bifurcation model with a non-planar branch. J. Biomech. 2006, 39, 818–832. [Google Scholar] [CrossRef]
- Kontopodis, N.; Tzirakis, K.; Stylianou, F.; Vavourakis, V.; Patou, G.M.; Ioannou, C.V. Should the Proximal Part of a Bifurcated Aortic Graft be Kept as Short as Possible? A computational study elucidates on aortic graft hemodynamics for various main body lengths. Ann. Vasc. Surg. 2021, 84, 344–353. [Google Scholar] [CrossRef]
- Morbiducci, U.; Gallo, D.; Massai, D.; Ponzini, R.; Deriu, M.A.; Antiga, L.; Redaelli, A.; Montevecchi, F.M. On the importance of blood rheology for bulk flow in hemodynamic models of the carotid bifurcation. J. Biomech. 2011, 44, 2427–2438. [Google Scholar] [CrossRef]
- Gijsen, F.J.; van de Vosse, F.N.; Janssen, J.D. The influence of the non-Newtonian properties of blood on the flow in large arteries: Steady flow in a carotid bifurcation model. J. Biomech. 1999, 32, 601–608. [Google Scholar] [CrossRef]
- Georgakarakos, E.; Xenakis, A.; Manopoulos, C.; Georgiadis, G.S.; Tsangaris, S.; Lazarides, M. Geometric factors affecting the displacement forces in an aortic endograft with crossed limbs: A computational study. J. Endovasc. Ther. 2013, 20, 191–199. [Google Scholar] [CrossRef] [PubMed]
- Ma, J.; Turan, A. Pulsatile non-Newtonian haemodynamics in a 3D bifurcating abdominal aortic aneurysm model. Comput. Methods Biomech. Biomed. Eng. 2011, 14, 683–694. [Google Scholar] [CrossRef] [PubMed]
- Arzani, A. Accounting for residence-time in blood rheology models: Do we really need non-Newtonian blood flow modelling in large arteries? J. R. Soc. Interface 2018, 15, 20180486. [Google Scholar] [CrossRef] [PubMed]
- Biasetti, J.; Hussain, F.; Gasser, T.C. Blood flow and coherent vortices in the normal and aneurysmatic aortas: A fluid dynamical approach to intra-luminal thrombus formation. J. R. Soc. Interface 2011, 8, 1449–1461. [Google Scholar] [CrossRef] [PubMed]
- Rabby, M.G.; Razzak, A.; Molla, M.M. Pulsatile non-Newtonian blood flow through a model of arterial stenosis. Procedia Eng. 2013, 56, 225–231. [Google Scholar] [CrossRef]
- Tzirakis, K.; Papaharilaou, Y.; Giordano, D.; Ekaterinaris, J. Numerical investigation of biomagnetic fluids in circular ducts. Int. J. Numer. Methods Biomed. Eng. 2014, 30, 297–317. [Google Scholar] [CrossRef]
- Oshima, M.; Torii, R.; Kobayashi, T.; Taniguchi, N.; Takagi, K. Finite element simulation of blood flow in the cerebral artery. Comput. Methods Appl. Mech. Eng. 2001, 191, 661–671. [Google Scholar] [CrossRef]
- Valen-Sendstad, K.; Steinman, D.A. Mind the gap: Impact of computational fluid dynamics solution strategy on prediction of intracranial aneurysm hemodynamics and rupture status indicators. AJNR Am. J. Neuroradiol. 2014, 35, 536–543. [Google Scholar] [CrossRef]
- Khan, M.O.; Steinman, D.A.; Valen-Sendstad, K. Non-Newtonian versus numerical rheology: Practical impact of shear-thinning on the prediction of stable and unstable flows in intracranial aneurysms. Int. J. Numer. Methods Biomed. Eng. 2017, 33, e2836. [Google Scholar] [CrossRef]
- Morales, H.G.; Larrabide, I.; Geers, A.J.; Aguilar, M.L.; Frangi, A.F. Newtonian and non-Newtonian blood flow in coiled cerebral aneurysms. J. Biomech. 2013, 46, 2158–2164. [Google Scholar] [CrossRef]
- Berger, S.A.; Jou, L.-D. Flows in stenotic vessels. Annu. Rev. Fluid Mech. 2000, 32, 347–382. [Google Scholar] [CrossRef]
- Misra, J.C.; Patra, M.K.; Misra, S.C. A non-newtonian fluid model for blood flow through arteries under stenotic conditions. J. Biomech. 1993, 26, 1129–1141. [Google Scholar] [CrossRef] [PubMed]
- Tu, C.; Deville, M. Pulsatile flow of non-Newtonian fluids through arterial stenoses. J. Biomech. 1996, 29, 899–908. [Google Scholar] [CrossRef] [PubMed]
- Leondes, C.T. Biomechanical Systems: Techniques and Applications, Volume IV: Biofluid Methods in Vascular and Pulmonary Systems; CRC Press: Boca Raton, FL, USA, 2000; Volume 4. [Google Scholar]
- Cho, Y.I.; Kensey, K.R. Effects of the non-Newtonian viscosity of blood on flows in a diseased arterial vessel. Part 1: Steady flows. Biorheology 1991, 28, 241–262. [Google Scholar] [CrossRef] [PubMed]
- Yilmaz, F.; Gundogdu, M.Y. A critical review on blood flow in large arteries; relevance to blood rheology, viscosity models, and physiologic conditions. Korea-Aust. Rheol. J. 2008, 20, 197–211. [Google Scholar]
- Ashraf, F.; Ambreen, T.; Park, C.W.; Kim, D.I. Comparative evaluation of ballet-type and conventional stent graft configurations for endovascular aneurysm repair: A CFD analysis. Clin. Hemorheol. Microcirc. 2021, 78, 1–27. [Google Scholar] [CrossRef]
- Soares, A.A.; Gonzaga, S.; Oliveira, C.; Simões, A.; Rouboa, A.I. Computational fluid dynamics in abdominal aorta bifurcation: Non-Newtonian versus Newtonian blood flow in a real case study. Comput. Methods Biomech. Biomed. Eng. 2017, 20, 822–831. [Google Scholar] [CrossRef]
- Weddell, J.C.; Kwack, J.; Imoukhuede, P.I.; Masud, A. Hemodynamic Analysis in an Idealized Artery Tree: Differences in Wall Shear Stress between Newtonian and Non-Newtonian Blood Models. PLoS ONE 2015, 10, e0124575. [Google Scholar] [CrossRef]
- Fisher, C.; Rossmann, J.S. Effect of non-newtonian behavior on hemodynamics of cerebral aneurysms. J. Biomech. Eng. 2009, 131, 091004. [Google Scholar] [CrossRef]
- Abbasian, M.; Shams, M.; Valizadeh, Z.; Moshfegh, A.; Javadzadegan, A.; Cheng, S. Effects of different non-Newtonian models on unsteady blood flow hemodynamics in patient-specific arterial models with in-vivo validation. Comput. Methods Programs Biomed. 2020, 186, 105185. [Google Scholar] [CrossRef]
- Leuprecht, A.; Perktold, K. Computer simulation of non-newtonian effects on blood flow in large arteries. Comput. Methods Biomech. Biomed. Engin. 2001, 4, 149–163. [Google Scholar] [CrossRef]
- O’Callaghan, S.; Walsh, M.; McGloughlin, T. Numerical modelling of Newtonian and non-Newtonian representation of blood in a distal end-to-side vascular bypass graft anastomosis. Med. Eng. Phys. 2006, 28, 70–74. [Google Scholar] [CrossRef] [PubMed]
- Iasiello, M.; Vafai, K.; Andreozzi, A.; Bianco, N. Analysis of non-Newtonian effects on Low-Density Lipoprotein accumulation in an artery. J. Biomech. 2016, 49, 1437–1446. [Google Scholar] [CrossRef]
- Krivovichev, G.V. Comparison of Non-Newtonian Models of One-Dimensional Hemodynamics. Mathematics 2021, 9, 2459. [Google Scholar] [CrossRef]
- Fung, Y.C. Biomechanics: Mechanical Properties of Living Tissues; Springer: Berlin/Heidelberg, Germany, 1993. [Google Scholar]
- González, H.A.; Moraga, N.O. On predicting unsteady non-Newtonian blood flow. Appl. Math. Comput. 2005, 170, 909–923. [Google Scholar] [CrossRef]
- Molla, M.M.; Paul, M.C. LES of non-Newtonian physiological blood flow in a model of arterial stenosis. Med. Eng. Phys. 2012, 34, 1079–1087. [Google Scholar] [CrossRef] [PubMed]
- Luo, X.Y.; Kuang, Z.B. A study on the constitutive equation of blood. J. Biomech. 1992, 25, 929–934. [Google Scholar] [CrossRef] [PubMed]
- Quemada, D. Rheology of concentrated disperse systems III. General features of the proposed non-newtonian model. Comparison with experimental data. Rheol. Acta 1978, 17, 643–653. [Google Scholar] [CrossRef]
- Skiadopoulos, A.; Neofytou, P.; Housiadas, C. Comparison of blood rheological models in patient specific cardiovascular system simulations. J. Hydrodyn. B 2017, 29, 293–304. [Google Scholar] [CrossRef]
- Soulis, J.V.; Giannoglou, G.D.; Chatzizisis, Y.S.; Seralidou, K.V.; Parcharidis, G.E.; Louridas, G.E. Non-Newtonian models for molecular viscosity and wall shear stress in a 3D reconstructed human left coronary artery. Med. Eng. Phys. 2008, 30, 9–19. [Google Scholar] [CrossRef]
- Walburn, F.J.; Schneck, D.J. A constitutive equation for whole human blood. Biorheology 1976, 13, 201–210. [Google Scholar] [CrossRef] [PubMed]
- Cokelet, G.R.; Meiselman, H.J. Macro- and micro-rheological properties of blood. In Handbook of Hemorheology and Hemodynamics; IOS Press: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Bird, R.B.; Curtiss, C.F.; Armstrong, R.C.; Hassager, O. Dynamics of polymeric liquids. In Kinetic Theory; Wiley-lnterscience: New York, NY, USA, 1987. [Google Scholar]
- Husain, I.; Labropulu, F.; Langdon, C.; Schwark, J. A comparison of Newtonian and non-Newtonian models for pulsatile blood flow simulations. J. Mech. Behav. Biomed. Mater. 2013, 21, 147–153. [Google Scholar] [CrossRef]
- Valant, A.Z.; Ziberna, L.; Papaharilaou, Y.; Anayiotos, A.; Georgiou, G.C. The infuence of temperature on rheological properties of blood mixtures with different volume expanders-implications in numerical arterial hemodynamics simulations. Rheol. Acta 2011, 50, 389–402. [Google Scholar] [CrossRef]
- Powell, R.E.; Eyring, H. Mechanisms for the Relaxation Theory of Viscosity. Nature 1944, 154, 427–428. [Google Scholar] [CrossRef]
- He, X.; Ku, D.N. Pulsatile flow in the human left coronary artery bifurcation: Average conditions. J. Biomech. Eng. 1996, 118, 74–82. [Google Scholar] [CrossRef]
- Himburg, H.A.; Grzybowski, D.M.; Hazel, A.L.; LaMack, J.A.; Li, X.M.; Friedman, M.H. Spatial comparison between wall shear stress measures and porcine arterial endothelial permeability. Am. J. Physiol. Heart Circ. Physiol. 2004, 286, H1916–H1922. [Google Scholar] [CrossRef]
- Malek, A.M.; Alper, S.L.; Izumo, S. Hemodynamic shear stress and its role in atherosclerosis. JAMA 1999, 282, 2035–2042. [Google Scholar] [CrossRef]
- Morbiducci, U.; Gallo, D.; Ponzini, R.; Massai, D.; Antiga, L.; Montevecchi, F.M.; Redaelli, A. Quantitative analysis of bulk flow in imagebased hemodynamic models of the carotid bifurcation: The influence of outflow conditions as test case. Ann. Biomed. Eng. 2010, 38, 3688–3705. [Google Scholar] [CrossRef]
- Johnston, B.M.; Johnston, P.R.; Corney, S.; Kilpatrick, D. Non-Newtonian blood flow in human right coronary arteries: Steady state simulations. J. Biomech. 2004, 37, 709–720. [Google Scholar] [CrossRef]
- Ardakani, S.S.J.; Jafarnejad, M.; Firoozabadi, B.; Saidi, M.S. Investigation of wall shear stress related factors in realistic carotid bifurcation geometries and different flow conditions. Trans. B Mech. Eng. 2010, 17, 358–366. [Google Scholar]
- Olufsen, M.S.; Peskin, C.S.; Kim, W.Y.; Pedersen, E.M.; Nadim, A.; Larsen, J. Numerical simulation and experimental validation of blood flow in arteries with structured-tree outflow conditions. Ann. Biomed. Eng. 2000, 28, 1281–1299. [Google Scholar] [CrossRef] [PubMed]
- Souza, M.S.; Souza, A.; Carvalho, V.; Teixeira, S.; Fernandes, C.S.; Lima, R.; Ribeiro, J. Fluid Flow and Structural Numerical Analysis of a Cerebral Aneurysm Model. Fluids 2022, 7, 100. [Google Scholar] [CrossRef]
- Kontopodis, N.; Galanakis, N.; Tzartzalou, I.; Tavlas, E.; Georgakarakos, E.; Dimopoulos, I.; Tsetis, D.; Ioannou, C.V. An update on the improvement of patient eligibility with the use of new generation endografts for the treatment of abdominal aortic aneurysms. Expert Rev. Med. Devices 2020, 17, 1231–1238. [Google Scholar] [CrossRef] [PubMed]
- De Santis, G.; Mortier, P.; De Beule, M.; Segers, P.; Verdonck, P.; Verhegghe, B. Patient-specific computational fluid dynamics: Structured mesh generation from coronary angiography. Med. Biol. Eng. Comput. 2010, 48, 371–380. [Google Scholar] [CrossRef]
- Hastie, T.; Tibshirani, R.; Friedman, J.H. The Elements of Statistical Learning: Data Mining, Inference, and Prediction; Springer: New York, NY, USA, 2009; Volume 2, pp. 1–758. [Google Scholar]
- Kutner, M.H.; Nachtsheim, C.J.; Neter, J. Applied Linear Regression Models, 4th ed.; McGraw-Hill/Irwin: New York, NY, USA, 2004. [Google Scholar]
- Jolliffe, I.T. Principal Component Analysis; Springer: New York, NY, USA, 2002. [Google Scholar]
- Husson, F.; Le, S.; Pagès, J. Exploratory Multivariate Analysis by Example Using R; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Giordani, P.; Ferraro, M.B.; Martella, F. An Introduction to Clustering with R; Springer: New York, NY, USA, 2020. [Google Scholar]
- Reeps, C.; Gee, M.; Maier, A.; Gurdan, M.; Eckstein, H.; Wall, W.A. The impact of model assumptions on results of computa-tional mechanics in abdominal aortic aneurysm. J. Vasc. Surg. 2010, 51, 679–688. [Google Scholar] [CrossRef]

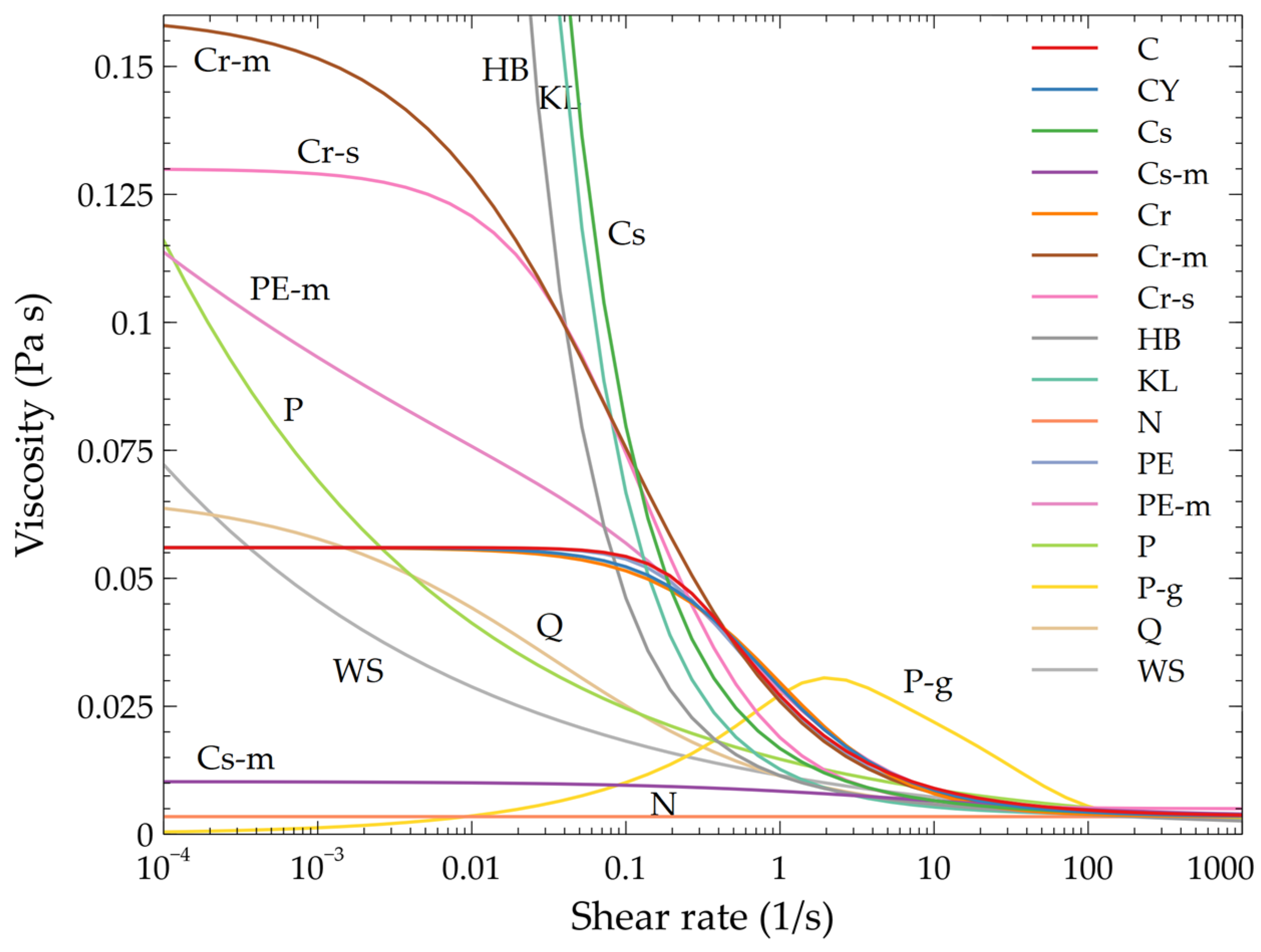
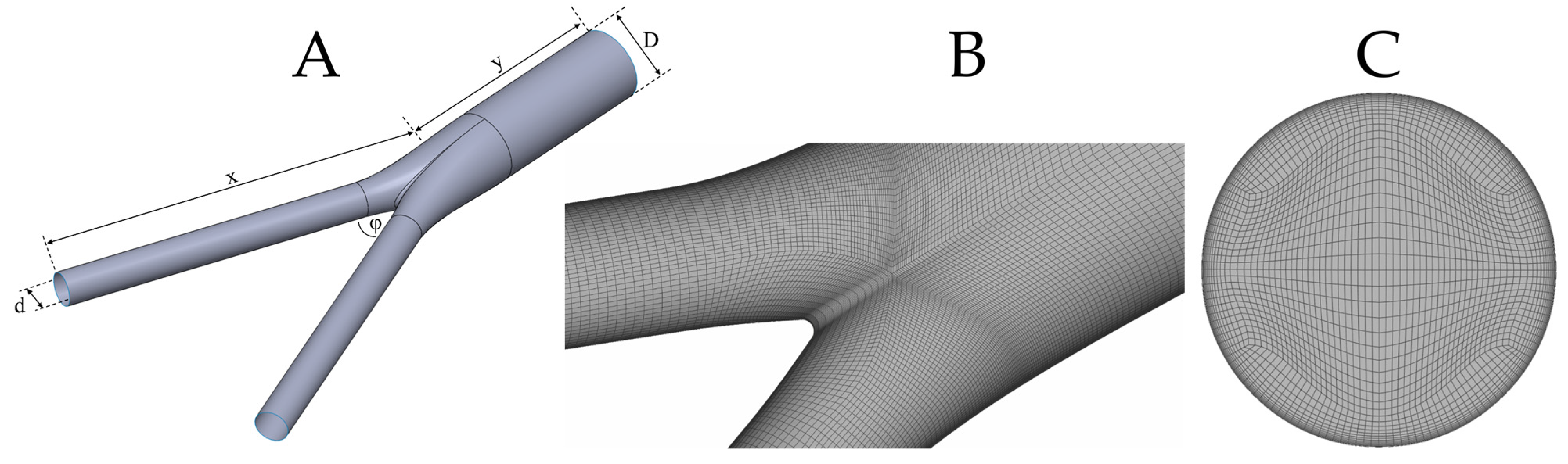
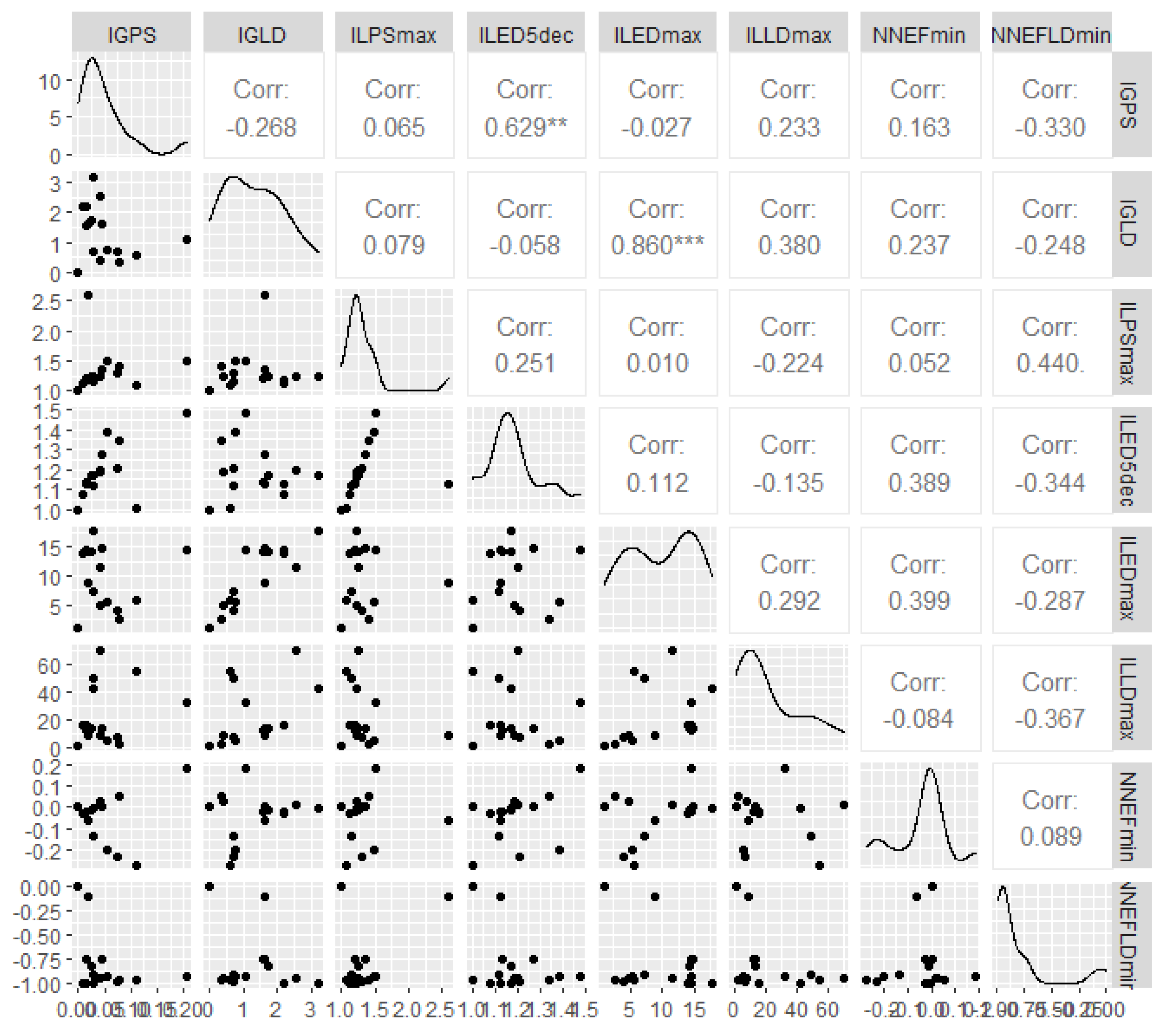



| # | Name (Abbreviation) | Equation | Parameter Values | References |
|---|---|---|---|---|
| 1 | Carreau (C) | [5,25,27,28] | ||
| 2 | Carreau–Yasuda (CY) | [13,25,29,30] | ||
| 3 | Casson (Cs) | [25,36] | ||
| 4 | Casson modified (Cs-m) | [37,38] | ||
| 5 | Cross (Cr) | [15,25,31,32] | ||
| 6 | Cross modified (Cr-m) | [25,31,33,34] | ||
| 7 | Cross simplified (Cr-s) | [25,31] | ||
| 8 | Herschel–Bulkley (HB) | [2,47] | ||
| 9 | Kuang–Luo (KL) | [6,31,39] | ||
| 10 | Newtonian (N) | [42,56] | ||
| 11 | Powell–Eyring (PE) | [25,48] | ||
| 12 | Powell–Eyring modified (PE-m) | [25] | ||
| 13 | Power-law (P) | [38,39,43,45,46] | ||
| 14 | Power-law generalized (P-g) | [5,6] | ||
| 15 | Quemada (Q) | [31,40,41] | ||
| 16 | Walburn–Schneck (WS) | [31,42,43] |
| Mesh | # of Elements | # of Nodes | BL Levels | BL Min (mm) |
|---|---|---|---|---|
| Coarse | 282450 | 299194 | 5 | 0.2688 |
| Medium | 660000 | 683789 | 10 | 0.0770 |
| Fine | 1229952 | 1263617 | 14 | 0.0338 |
| Extra fine | 2464640 | 2515452 | 19 | 0.0129 |
| Mesh | TAWSS Result (Pa)/Error (%) | OSI Result/Error (%) | Outlet Velocity Result (m/s)/Error (%) | Volume Pressure Integral Result (Pa·m3)/Error (%) | ||||
|---|---|---|---|---|---|---|---|---|
| Coarse | 0.7905 | 6.65% | 0.2117 | 5.96% | 0.0548 | 2.81% | 1.4225 | −6.44% |
| Medium | 0.7623 | 2.85% | 0.2043 | 2.25% | 0.0541 | 1.50% | 1.4578 | −4.12% |
| Fine | 0.7433 | 0.28% | 0.2010 | 0.60% | 0.0535 | 0.38% | 1.5312 | −0.70% |
| Extra fine | 0.7412 | - | 0.1998 | - | 0.0533 | - | 1.5205 | - |
| Cluster | Variable | Cluster Mean | p-Value |
|---|---|---|---|
| CL1 | IGLD | 0.914 | 0.001 |
| CL2 | ILLDmax | 1.424 | 0.031 |
| CL3 | IGLD | −0.864 | 0.024 |
| N | NNEFLDmin | 2.640 | 0.006 |
| P-g | ILPSmax | 3.473 | <0.001 |
| Cr-s | IGPS | 3.085 | 0.001 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tzirakis, K.; Kamarianakis, Y.; Kontopodis, N.; Ioannou, C.V. Classification of Blood Rheological Models through an Idealized Symmetrical Bifurcation. Symmetry 2023, 15, 630. https://doi.org/10.3390/sym15030630
Tzirakis K, Kamarianakis Y, Kontopodis N, Ioannou CV. Classification of Blood Rheological Models through an Idealized Symmetrical Bifurcation. Symmetry. 2023; 15(3):630. https://doi.org/10.3390/sym15030630
Chicago/Turabian StyleTzirakis, Konstantinos, Yiannis Kamarianakis, Nikolaos Kontopodis, and Christos V. Ioannou. 2023. "Classification of Blood Rheological Models through an Idealized Symmetrical Bifurcation" Symmetry 15, no. 3: 630. https://doi.org/10.3390/sym15030630
APA StyleTzirakis, K., Kamarianakis, Y., Kontopodis, N., & Ioannou, C. V. (2023). Classification of Blood Rheological Models through an Idealized Symmetrical Bifurcation. Symmetry, 15(3), 630. https://doi.org/10.3390/sym15030630











