Series Solution of the Time-Dependent Schrödinger–Newton Equations in the Presence of Dark Energy via the Adomian Decomposition Method
Abstract
1. Introduction
2. The Adomian Decomposition Method
3. The Adomian Decomposition Method for the Time-Dependent Schrödinger–Newton- System
3.1. The S-N- System
3.2. The Adomian Decomposition Method for the S-N- System
3.3. Testing the Adomian Decomposition Method
4. Series Solution of the Time-Dependent Schrödinger–Newton- System
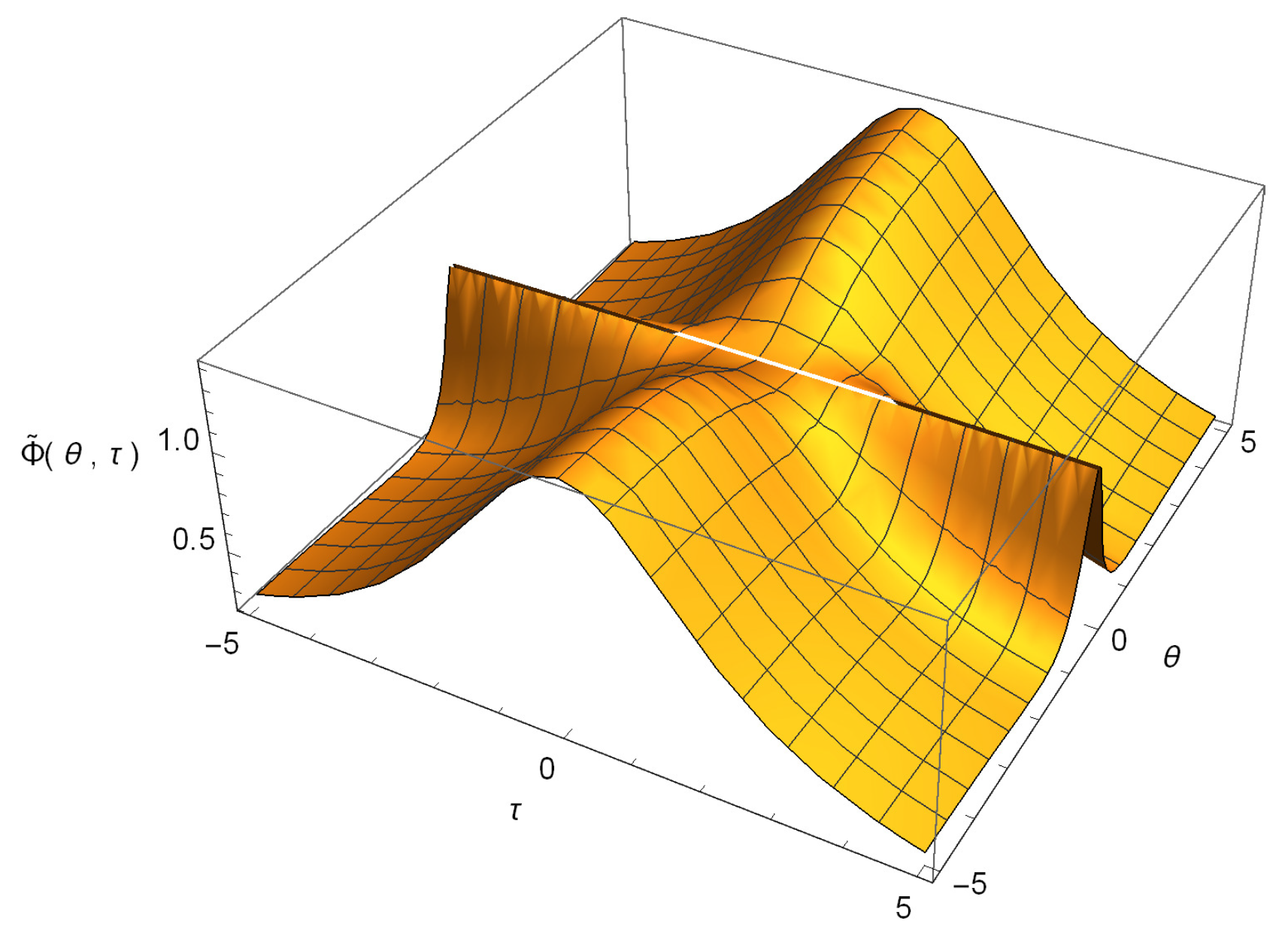
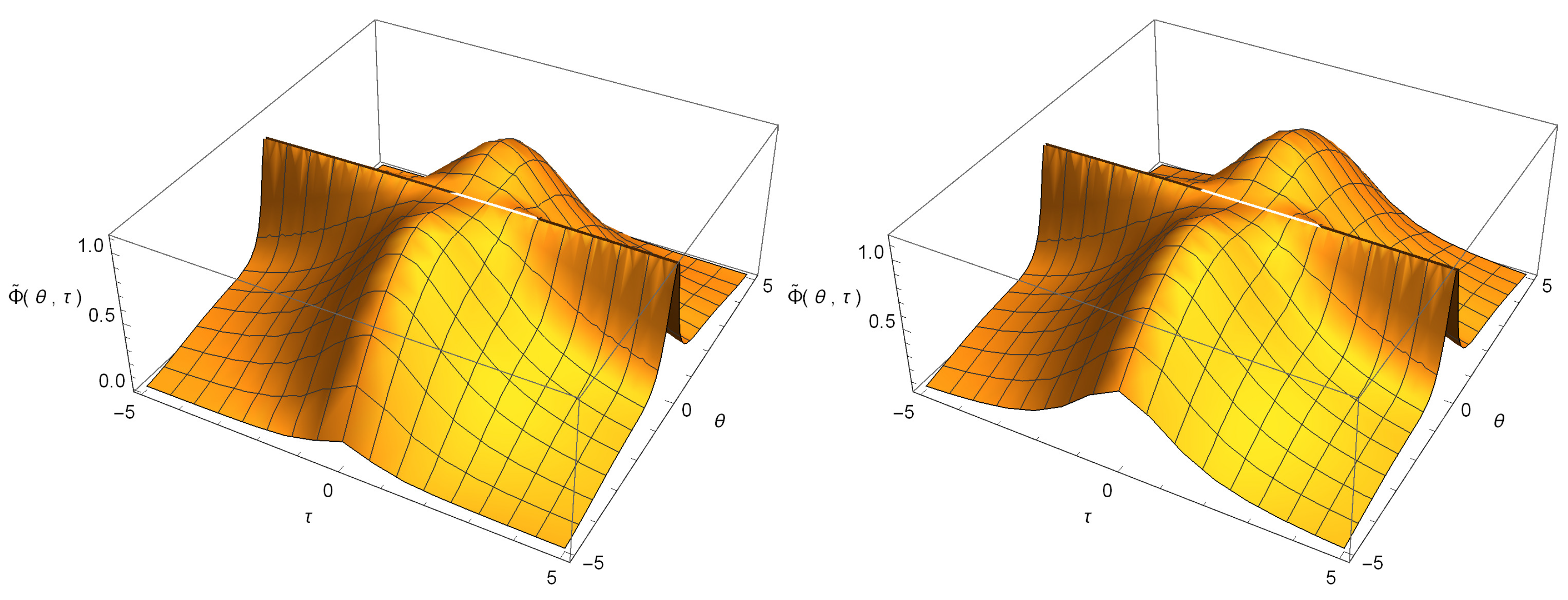
4.1. Semi-Analytical Solutions
The Padé Approximants
4.2. The Dark Energy Dominated Regime
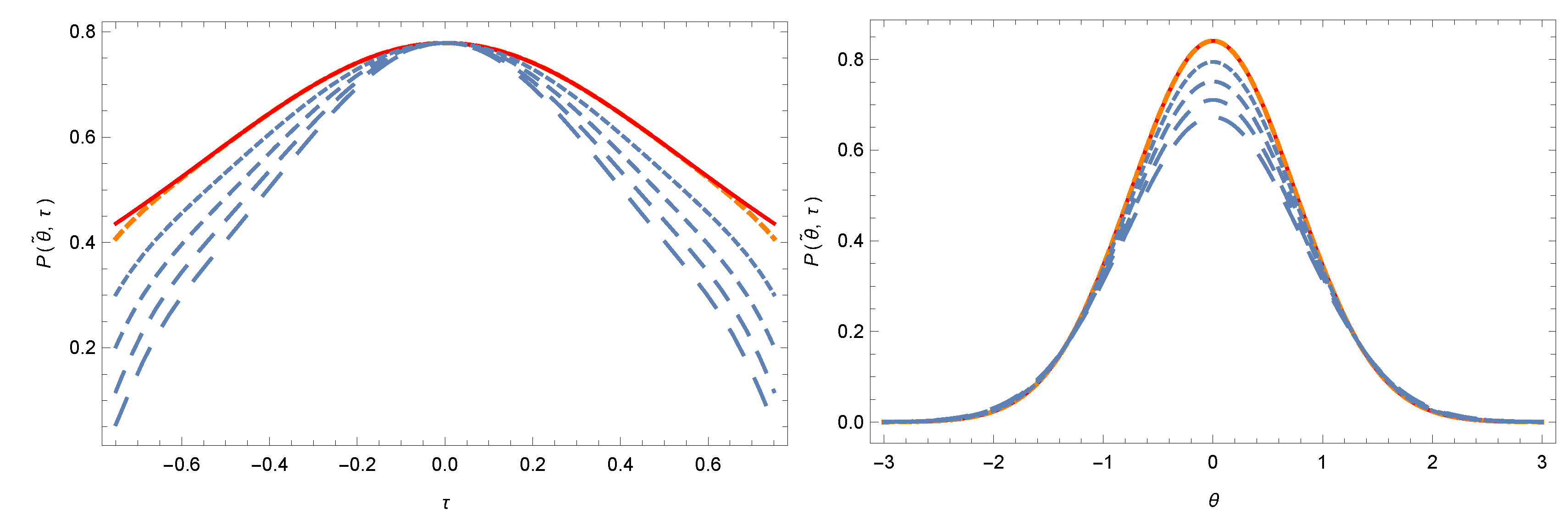
4.3. Numerical Analysis
5. Physical Implications
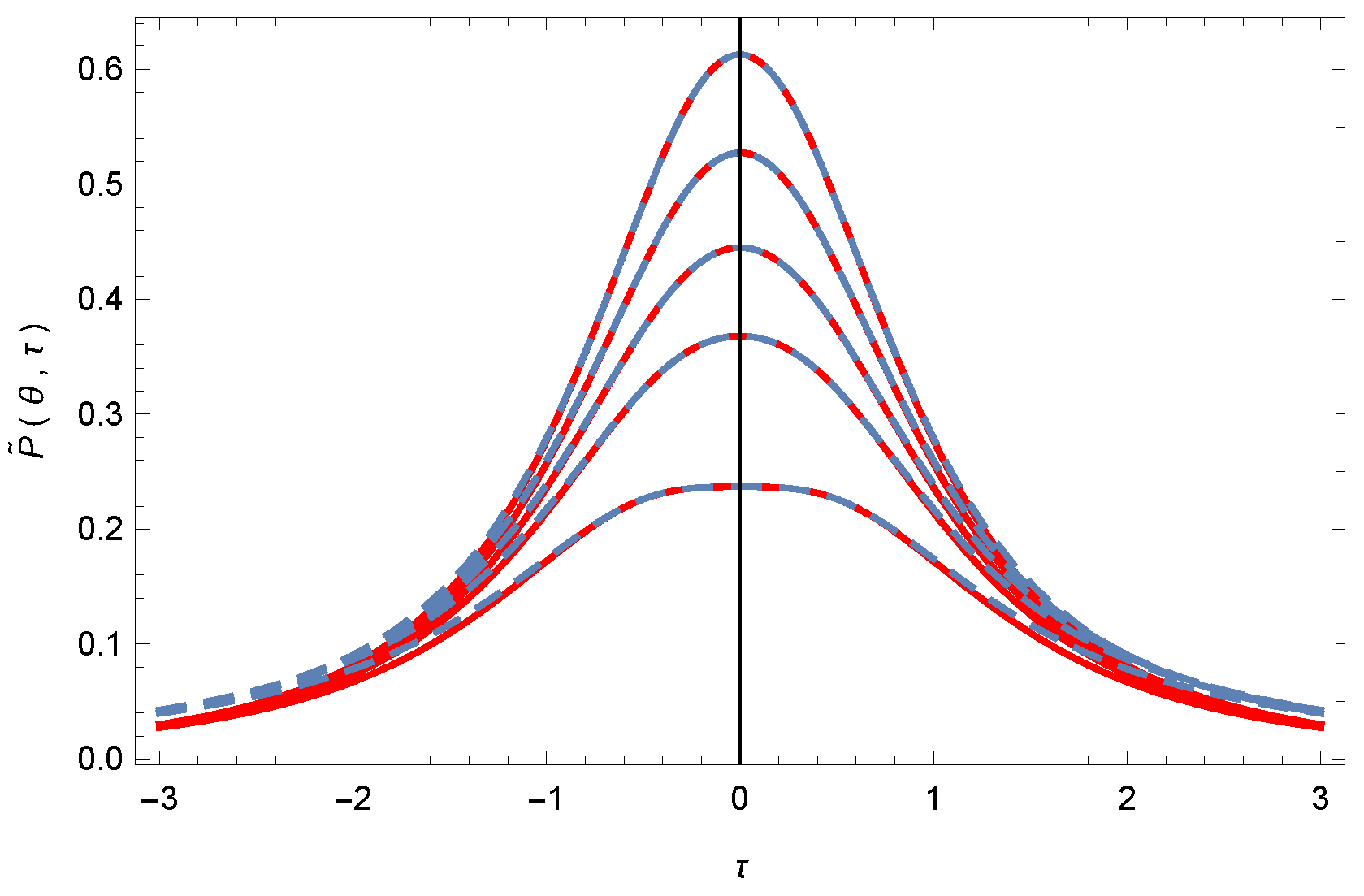
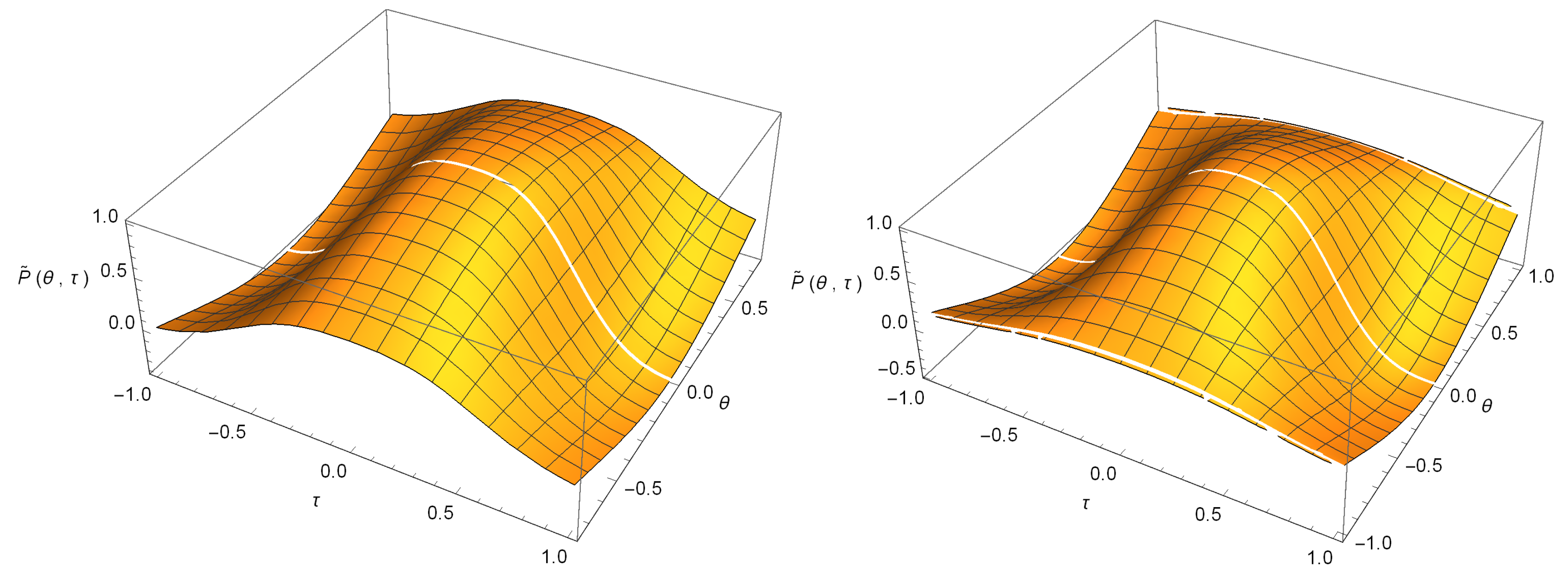
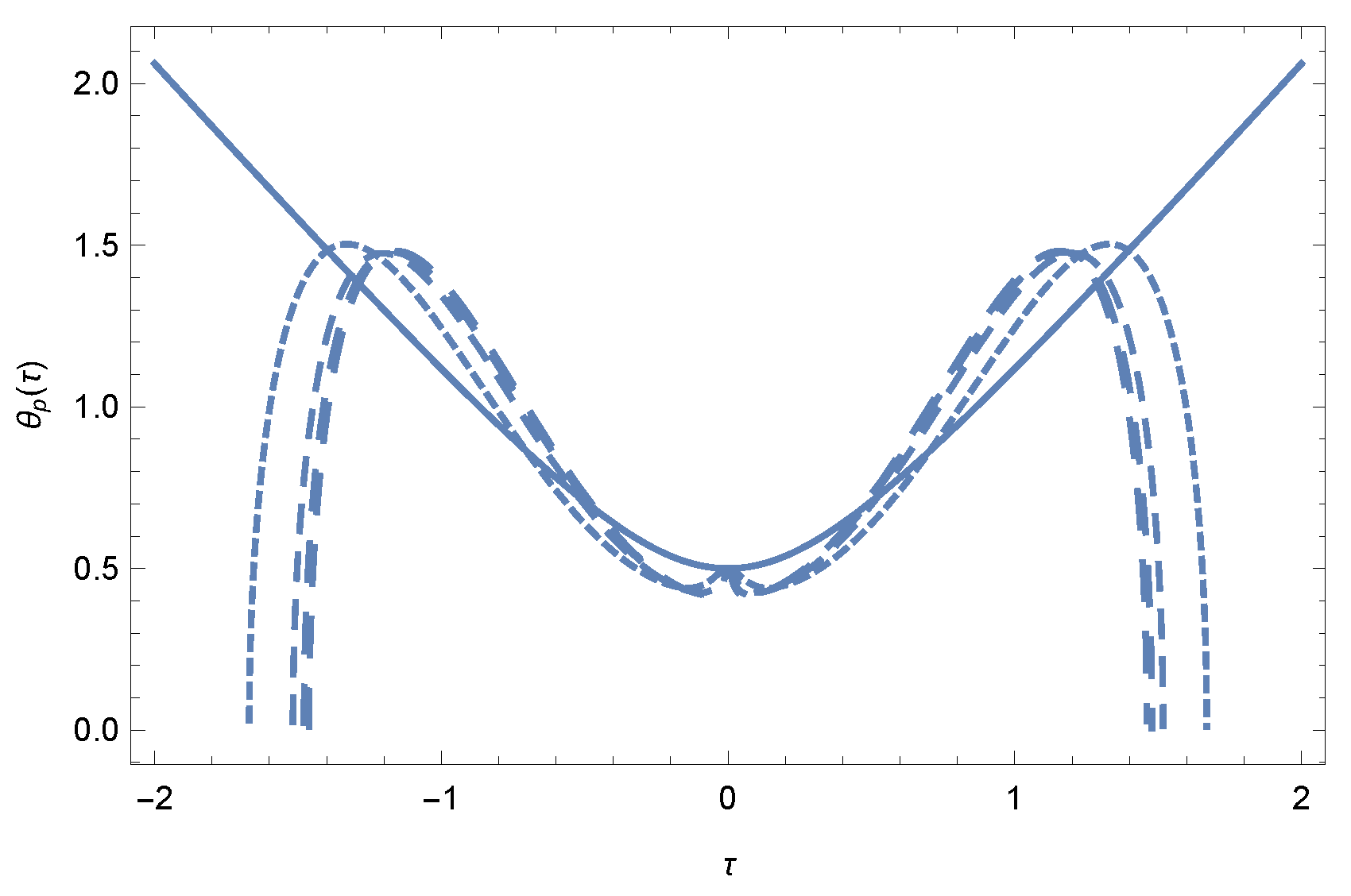
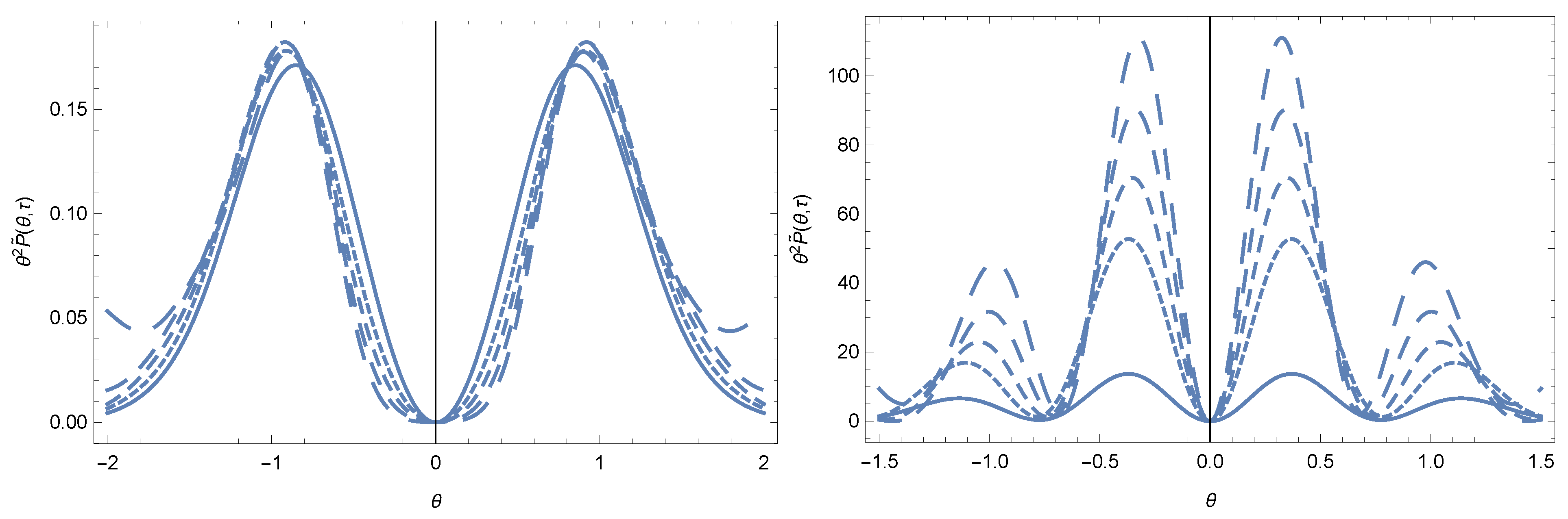
5.1. The Peak Radial Probability
Qualitative Estimations
5.2. Peak Probability in the ADM Approach
6. Discussion and Final Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bronstein, M. Über den spontanen Zerfall der Photonen. Phys. Z. Sowjetunion 1936, 9, 140, Republished as Bronstein, M. Quantum theory of weak gravitational fields. Gen. Relativ. Gravit. 2012, 44, 267. [Google Scholar]
- Bronstein, M.P. Quantization of gravitational waves. Zh. Eksp. Teor. Fiz. 1936, 6, 195. [Google Scholar]
- Tawfik, A.N.; Diab, A.M. Review on Generalized Uncertainty Principle. Rept. Prog. Phys. 2015, 78, 126001. [Google Scholar] [CrossRef]
- Hossenfelder, S. Minimal Length Scale Scenarios for Quantum Gravity. Living Rev. Rel. 2013, 16, 2. [Google Scholar] [CrossRef]
- Adler, R.J.; Santiago, D.I. On gravity and the uncertainty principle. Mod. Phys. Lett. A 1999, 14, 1371. [Google Scholar] [CrossRef]
- Scardigli, F. Generalized uncertainty principle in quantum gravity from micro-black hole Gedanken experiment. Phys. Lett. B 1999, 452, 39. [Google Scholar] [CrossRef]
- Bolen, B.; Cavaglia, M. (Anti-)de Sitter black hole thermodynamics and the generalized uncertainty principle. Gen. Rel. Grav. 2005, 37, 1255. [Google Scholar] [CrossRef]
- Park, M. The Generalized Uncertainty Principle in (A)dS Space and the Modification of Hawking Temperature from the Minimal Length. Phys. Lett. B 2008, 659, 698. [Google Scholar] [CrossRef]
- Bambi, C.; Urban, F.R. Natural Extension of the Generalized Uncertainty Principle. Class. Quant. Grav. 2008, 25, 095006. [Google Scholar] [CrossRef]
- Lake, M.J.; Miller, M.; Ganardi, R.F.; Liu, Z.; Liang, S.D.; Paterek, T. Generalized uncertainty relations from superpositions of geometries. Class. Quant. Grav. 2019, 36, 155012. [Google Scholar] [CrossRef]
- Lake, M.J.; Miller, M.; Liang, S.-D. Generalized uncertainty relations for angular momentum and spin in quantum geometry. Universe 2020, 6, 56. [Google Scholar] [CrossRef]
- Lake, M.J. A New Approach to Generalized Uncertainty Relations. arXiv 2021, arXiv:2008.13183v1. [Google Scholar]
- Rovelli, C. Quantum Gravity, Cambridge Monographs on Mathematical Physics; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Mukhanov, V.; Winitzki, S. Introduction to Quantum Effects in Gravity; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Rovelli, C.; Vidatto, F. Covariant Loop Quantum Gravity: An Elementary Introduction to Quantum Gravity and Spinfoam Theory; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
- Becker, K.; Becker, M.; Schwarz, J.H. String Theory and M-Theory: A Modern Introduction; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Modesto, L.; Rachwal, L. Nonlocal quantum gravity: A review. Int. J. Mod. Phys. 2017, 26, 1730020. [Google Scholar] [CrossRef]
- Gubitosi, G.; Ripken, C.; Saueressig, F. Scales and hierachies in asymptotically safe quantum gravity: A review. Found. Phys. 2019, 49, 972. [Google Scholar] [CrossRef]
- Loll, R. Quantum gravity from causal dynamical triangulations: A review. Class. Quantum Gravity 2020, 37, 013002. [Google Scholar] [CrossRef]
- Steinhaus, S. Coarse graining spin foam quantum gravity—A review. Front. Phys. 2020, 8, 295. [Google Scholar] [CrossRef]
- Carney, D.; Stamp, P.C.E.; Taylor, J.M. Tabletop experiments for quantum gravity: A user’s manual. Class. Quantum Gravity 2019, 36, 034001. [Google Scholar] [CrossRef]
- Kaltenbaek, R.; Aspelmeyer, M.; Vedral, V. Macroscopic quantum resonators (MAQRO): 2015 update. EPJ Quantum Technol. 2016, 3, 5. [Google Scholar] [CrossRef]
- Zee, A. Quantum Field Theory in a Nutshell; Princeton University Press: Princeton, NJ, USA; Oxford, UK, 2003. [Google Scholar]
- Dzhunushaliev, V.; Folomeev, V.; Kleihaus, B.; Kunz, J. Modified gravity from the quantum part of the metric. Eur. Phys. J. 2014, 74, 2743. [Google Scholar] [CrossRef]
- Harko, T.; Lobo, F.S.; Nojiri, S.; Odintsov, S.D. f(R,T) gravity. Phys. Rev. 2011, 84, 024020. [Google Scholar] [CrossRef]
- Harko, T.; Lobo, F.S.N. Extensions of f(R) Gravity Curvature-Matter Couplings and Hybrid Metric-Palatini Theory; Cambridge University Press: Cambridge, UK, 2018. [Google Scholar]
- Liu, X.; Harko, T.; Liang, S.-D. Cosmological implications of modified gravity induced by quantum metric fluctuations. Eur. Phys. J. 2016, 76, 420. [Google Scholar] [CrossRef]
- Møller, C. Les théories relativistes de la gravitation. Colloq. Int. CNRS 1962, 91, 1. [Google Scholar]
- Rosenfeld, L. On quantization of fields. Nucl. Phys. 1963, 40, 353. [Google Scholar] [CrossRef]
- Carlip, S. Is quantum gravity necessary? Class. Quant. Grav. 2008, 25, 154010. [Google Scholar] [CrossRef]
- Kibble, T.W.B.; Randjbar-Daemi, S. Non-linear coupling of quantum theory and classical gravity. J. Phys. A Math. Gen. 1980, 13, 141. [Google Scholar] [CrossRef]
- Diósi, L.; Lukács, B. In favor of a Newtonian quantum gravity. Ann. Phys. 1987, 499, 488. [Google Scholar] [CrossRef]
- Penrose, R. Quantum computation, entanglement and state reduction. Phil. Trans. R. Soc. Lond. 1998, A356, 1927. [Google Scholar] [CrossRef]
- Moroz, I.M.; Penrose, R.; Tod, K.P. Spherically-symmetric solutions of the Schrödinger-Newton equations. Class. Quantum Grav. 1998, 15, 2733. [Google Scholar] [CrossRef]
- Tod, K.P.; Moroz, I.M. An analytical approach to the Schrödinger-Newton equations. Nonlinearity 1999, 12, 201. [Google Scholar] [CrossRef]
- Lan, D. A quantum potential approach for the gravitational Schrödinger equation and its reduction into the Lane-Emden equation. Mod. Phys. Lett. 1999, 14, 2667. [Google Scholar] [CrossRef]
- Kumar, D.; Soni, V. Single particle Schrödinger equation with gravitational self-interaction. Phys. Lett. 2000, 271, 157. [Google Scholar] [CrossRef]
- Tod, K.P. The ground state energy of the Schrödinger–Newton equation. Phys. Lett. 2001, 280, 173. [Google Scholar] [CrossRef]
- Harrison, R.; Moroz, I.; Tod, K.P. A numerical study of the Schrödinger—Newton equations. Nonlinearity 2003, 16, 101. [Google Scholar] [CrossRef]
- Wei, J.; Winter, M. Strongly interacting bumps for the Schrödinger—Newton equations. J. Math. Phys. 2009, 50, 012905. [Google Scholar] [CrossRef]
- Giulini, D.; Grossardt, A. Gravitationally induced inhibitions of dispersion according to the Schrödinger—Newton equation. Class. Quantum Grav. 2011, 28, 195026. [Google Scholar] [CrossRef]
- Manfredi, G.; Hervieux, P.-A.; Haas, F. Variational approach to the time-dependent Schrödinger–Newton equations. Class. Quantum Grav. 2013, 30, 075006. [Google Scholar] [CrossRef]
- Duval, C.; Lazzarini, S. On the Schrödinger–Newton equation and its symmetries: A geometric view. Class. Quantum Gravity 2015, 32, 175006. [Google Scholar] [CrossRef]
- Diósi, L. Gravity-related wave function collapse: Is superfluid He exceptional? Found. Phys. 2014, 44, 483. [Google Scholar] [CrossRef]
- Grossardt, A.; Hiesmayr, B.C. Newtonian self-gravitation in the neutral meson system. Phys. Rev. 2015, 91, 064056. [Google Scholar] [CrossRef]
- Bera, S.; Mohan, R.; Singh, T.P. Stochastic modification of the Schrödinger-Newton equation. Phys. Rev. 2015, 92, 025054. [Google Scholar] [CrossRef]
- Bera, S.; Donadi, S.; Lochan, K.; Singh, T.P. A comparison between models of gravity induced decoherence. Found. Phys. 2015, 45, 1537. [Google Scholar] [CrossRef]
- Prosperi, G.M. Introduction of a Classical Level in Quantum Theory: Continuous Monitoring. Found. Phys. 2016, 46, 1426. [Google Scholar] [CrossRef]
- Bera, S.; Giri, P.; Singh, T.P. Spacetime Fluctuations and a Stochastic Schrödinger–Newton Equation. Found. Phys. 2017, 47, 897. [Google Scholar] [CrossRef]
- Tilloy, A.; Diósi, L. Principle of least decoherence for Newtonian semiclassical gravity. Phys. Rev. 2017, 96, 104045. [Google Scholar] [CrossRef]
- Howl, R.; Penrose, R.; Fuentes, I. Exploring the unification of quantum theory and general relativity with a Bose–Einstein condensate. New J. Phys. 2019, 21, 043047. [Google Scholar] [CrossRef]
- Diósi, L. Planck length challenges non-relativistic quantum mechanics of large masses. J. Phys. Conf. Ser. 2019, 1275, 011001. [Google Scholar] [CrossRef]
- Lotte, B.K.; Mishra, S. Effect of post-Newtonian-like self-energy, quantum gravity and exchange correlations on Schwarzschild black hole: Application of uncertainty principle. Mod. Phys. Lett. 2020, 35, 2050081. [Google Scholar] [CrossRef]
- Kelvin; Onggadinata, K.; Lake, M.J.; Paterek, T. Dark energy effects in the Schrödinger-Newton approach. Phys. Rev. 2020, 101, 063028. [Google Scholar] [CrossRef]
- Amendola, L.; Tsujikawa, S. Dark Energy, Theory and Observations; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Harko, T.; Lobo, F.S.N. Beyond Einstein’s General Relativity: Hybrid metric-Palatini gravity and curvature-matter couplings. Int. J. Mod. Phys. 2020, 29, 2030008. [Google Scholar] [CrossRef]
- Brizuela, D.; Duran-Cabac, A. Relativistic effects on the Schrödinger—Newton equation. Phys. Rev. D 2022, 106, 124038. [Google Scholar] [CrossRef]
- Bhattacharya, S.; Dialektopoulos, K.F.; Romano, A.E.; Skordis, C.; Tomaras, T.N. The maximum sizes of large scale structures in alternative theories of gravity. J. Cosmol. Astropart. Phys. 2017, 2017, 018. [Google Scholar] [CrossRef]
- Adomian, G. Convergent series solution of nonlinear equations. J. Comput. Appl. Math. 1984, 11, 2. [Google Scholar] [CrossRef]
- Adomian, G. A review of the decomposition method in applied mathematics. J. Math. Anal. Appl. 1988, 135, 501. [Google Scholar] [CrossRef]
- Adomian, G. Solving Frontier Problems of Physics: The Decomposition Method; Kluwer: Dordrecht, The Netherlands, 1994. [Google Scholar]
- Adomian, G.; Rach, R. Modified adomian polynomials. Math. Comput. Model. 1996, 24, 39. [Google Scholar] [CrossRef]
- Warwaz, A.-M. The modified decomposition method and Padé approximants for solving the Thomas–Fermi equation. Appl. Math. Comput. 1999, 105, 11. [Google Scholar]
- Warwaz, A.-M. A reliable modification of Adomian decomposition method. Appl. Math. Comput. 1999, 102, 77. [Google Scholar]
- Azreg-Ainou, M. Developed Adomian method for quadratic Kaluza–Klein relativity. Class. Quant. Grav. 2010, 27, 015012. [Google Scholar] [CrossRef]
- Wazwaz, A.-M.; Rach, R.; Duan, J.-S. A study on the systems of the Volterra integral forms of the Lane–Emden equations by the Adomian decomposition method. Math. Meth. Appl. Sci. 2014, 37, 10. [Google Scholar] [CrossRef]
- Ghasemi, H.; Ghovatmand, M.; Zarrinkamar, S.; Hassanabadi, H. Interaction of nanoparticles for the peristaltic flow in an asymmetric channel with the induced magnetic field. Eur. Phys. J. Plus 2014, 129, 32. [Google Scholar] [CrossRef]
- Rach, R.; Wazwaz, A.-M.; Duan, J.-S. The Volterra integral form of the Lane–Emden equation: New derivations and solution by the Adomian decomposition method. J. Appl. Math. Comput. 2015, 47, 365. [Google Scholar] [CrossRef]
- Battista, E.; Di Grezia, E.; Esposito, G. Bicharacteristics and Fourier integral operators in Kasner spacetime. Int. J. Geom. Methods Modern Phys. 2015, 12, 1550060. [Google Scholar]
- Duan, J.-S.; Rach, R.; Wazwaz, A.-M. Higher order numeric solutions of the Lane–Emden-type equations derived from the multi-stage modified Adomian decomposition method. Int. J. Comput. Math. 2017, 94, 197. [Google Scholar] [CrossRef]
- Mak, M.K.; Leung, C.S.; Harko, T. Computation of the general relativistic perihelion precession and of light deflection via the Laplace-Adomian Decomposition Method. Adv. High Energy Phys. 2018, 7093592. [Google Scholar] [CrossRef]
- Mak, M.K.; Leung, C.S.; Harko, T. Solving the nonlinear biharmonic equation by the Laplace-Adomian and Adomian Decomposition Methods. Surv. Math. Its Appl. 2018, 13, 183. [Google Scholar]
- Harko, T.; Mak, M.K.; Leung, C.S. Vortex solutions in atomic Bose-Einstein condensates via the Adomian decomposition method. Rom. Rep. Phys. 2020, 72, 116. [Google Scholar]
- Harko, T.; Mak, M.K. Series solution of the Susceptible-Infected-Recovered (SIR) epidemic model with vital dynamics via the Adomian and Laplace-Adomian Decomposition Methods. arXiv 2020, arXiv:2009.00434. [Google Scholar]
- Alam, M.N.; Tunç, C. Constructions of the optical solitons and other solitons to the conformable fractional Zakharov–Kuznetsov equation with power law nonlinearity. J. Taibah Univ. Sci. 2020, 14, 94. [Google Scholar] [CrossRef]
- Baker, G.A., Jr.; Graves-Morris, P. Padé Approximants; Cambridge University Press: Cambridge, UK; New York, NY, USA; Melbourn, Australia, 1996. [Google Scholar]
- Hobson, M.P.; Efstathiou, G.P.; Lasenby, A.N. General Relativity: An Introduction for Physicists; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Grossardt, A.; Bateman, J.; Ulbricht, H.; Bassi, A. Opto-mechanical test of the Schrödinger–Newton equation. Phys. Rev. 2016, 93, 096003. [Google Scholar]
- Karolyhazy, F. Gravitation and quantum mechanics of macroscopic objects. Nuovo Cim. 1966, 42, 390. [Google Scholar] [CrossRef]
- Diosi, L. Gravitation and quantum-mechanical localization of macro-objects. Phys. Lett. 1984, 105, 199. [Google Scholar] [CrossRef]
- Penrose, R. On Gravity’s Role in Quantum State Reduction. Gen. Rel. Grav. 1996, 28, 581. [Google Scholar] [CrossRef]
- Minar, J.; Sekatski, P.; Sangouard, N. Bounding quantum gravity inspired decoherence using atom interferometry. Phys. Rev. 2016, 94, 062111. [Google Scholar] [CrossRef]
- Bose, S.; Jacobs, K.; Knight, P.L. A scheme to probe the decoherence of a macroscopic object. Phys. Rev. 1999, 59, 3204. [Google Scholar] [CrossRef]
- van Meter, J. Test Schrödinger-Newton ‘collapse’ of the wave function. Class. Quant. Grav. 2011, 28, 215013. [Google Scholar] [CrossRef]
- Burikham, P.; Cheamsawat, K.; Harko, T.; Lake, M.J. The minimum mass of a spherically symmetric object in D-dimensions and its implications for the mass hierarchy problem. Eur. Phys. J. C 2015, 75, 442. [Google Scholar] [CrossRef]
- Lake, M.J. Is there a connection between “dark” and “light” physics? J. Phys. Conf. Ser. 2017, 883, 012001. [Google Scholar] [CrossRef]
- Lake, M.J. Minimum length uncertainty relations in the presence of dark energy. Galaxies 2019, 7, 11. [Google Scholar] [CrossRef]
- Lake, M.J. Why space must be quantised on a different scale to matter. arXiv 2020, arXiv:2005.12724v2. [Google Scholar]
- Hashiba, J. Dark Energy from Eternal Pair-production of Fermions. arXiv 2018, arXiv:1808.06517. [Google Scholar]
- Lake, M.J.; Jain, S.; Paterek, T. A simple model of a zero-energy expanding Universe. 2023; manuscript in preparation. [Google Scholar]
- Perivolaropoulos, L. Submillimeter spatial oscillations of Newton’s constant: Theoretical models and laboratory tests. Phys. Rev. D 2017, 95, 084050. [Google Scholar] [CrossRef]
- Antoniou, I.; Perivolaropoulos, L. Constraints on spatially oscillating sub-mm forces from the Stanford Optically Levitated Microsphere Experiment data. Phys. Rev. D 2017, 96, 104002. [Google Scholar] [CrossRef]
- Perivolaropoulos, L.; Kazantzidis, L. Hints of Modified Gravity in Cosmos and in the Lab? Int. J. Mod. Phys. D 2019, 28, 1942001. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Harko, T.; Lake, M.J.; Mak, M.K. Series Solution of the Time-Dependent Schrödinger–Newton Equations in the Presence of Dark Energy via the Adomian Decomposition Method. Symmetry 2023, 15, 372. https://doi.org/10.3390/sym15020372
Harko T, Lake MJ, Mak MK. Series Solution of the Time-Dependent Schrödinger–Newton Equations in the Presence of Dark Energy via the Adomian Decomposition Method. Symmetry. 2023; 15(2):372. https://doi.org/10.3390/sym15020372
Chicago/Turabian StyleHarko, Tiberiu, Matthew J. Lake, and Man Kwong Mak. 2023. "Series Solution of the Time-Dependent Schrödinger–Newton Equations in the Presence of Dark Energy via the Adomian Decomposition Method" Symmetry 15, no. 2: 372. https://doi.org/10.3390/sym15020372
APA StyleHarko, T., Lake, M. J., & Mak, M. K. (2023). Series Solution of the Time-Dependent Schrödinger–Newton Equations in the Presence of Dark Energy via the Adomian Decomposition Method. Symmetry, 15(2), 372. https://doi.org/10.3390/sym15020372





