Entropy Generation for MHD Peristaltic Transport of Non-Newtonian Fluid in a Horizontal Symmetric Divergent Channel
Abstract
1. Introduction
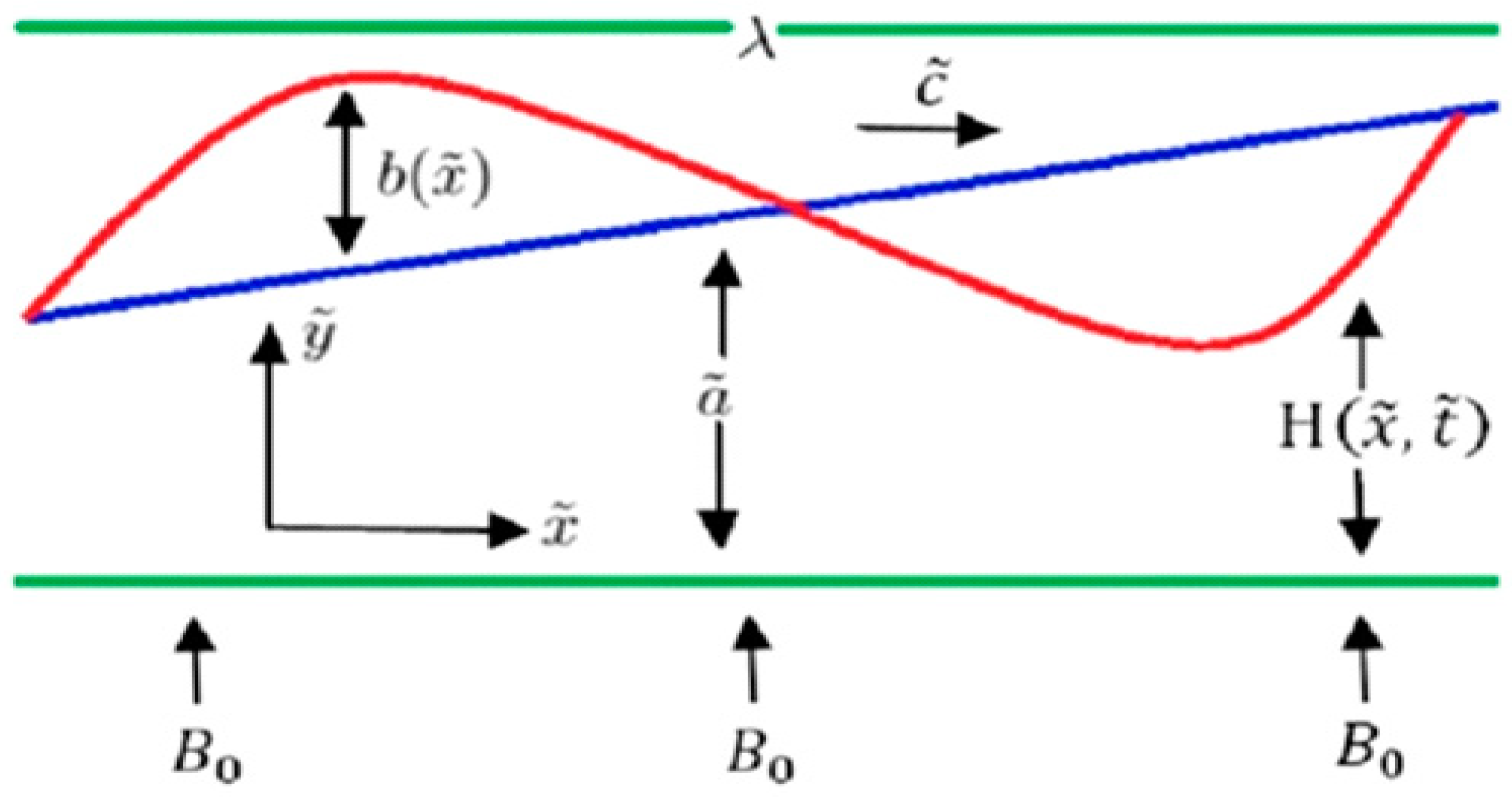
2. Problem Formulation
3. Entropy Generation Analysis
4. Solution of the Problem
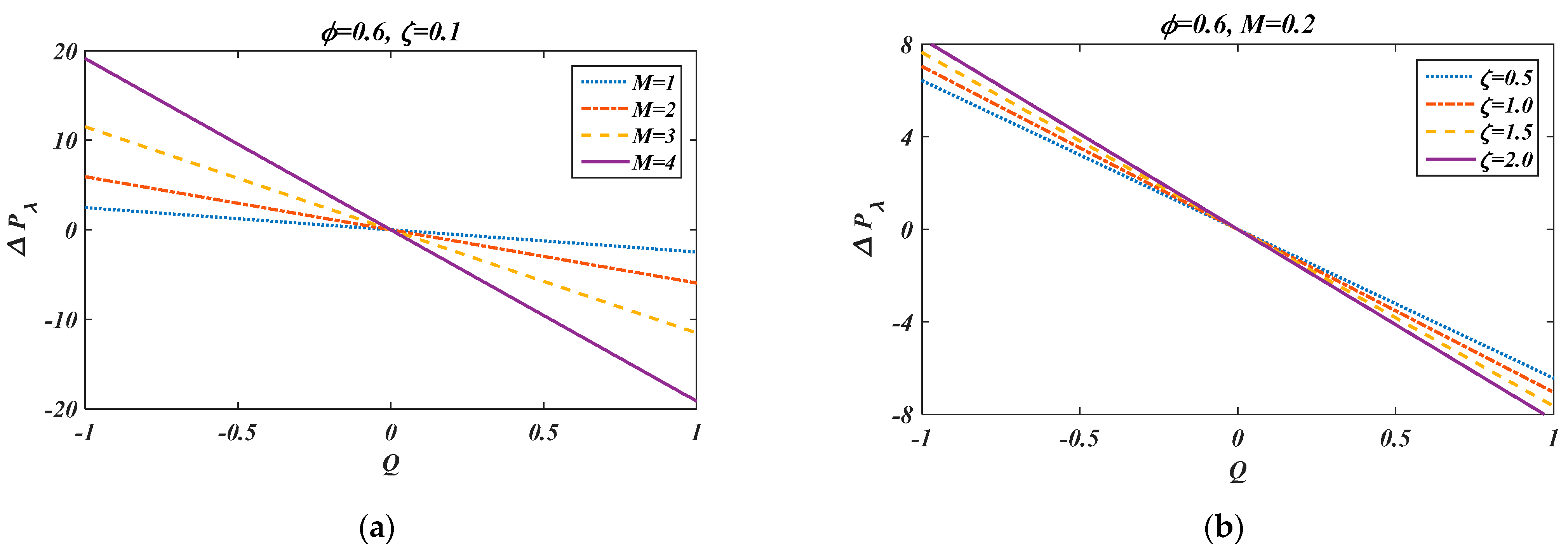
5. Results and Discussion
Validation
6. Closing Remarks
- The velocity profile shows symmetric and the opposite behavior with the enhancement in the Hartmann number and Ree–Eyring parameter.
- When the Hartmann number is allowed to elevate, it has been investigated that a pressure rise causes an upsurge in the retrograde region but displays the reverse action in the co-pumping region. Almost the same trend is manifested in the case of the Ree–Eyring parameter.
- The amplification in the case of entropy generation is analogous to variations in all parameters, while the Hartmann number shows a slight deviation in the vicinity of boundaries.
- Temperature dispersion arguments with the rising values of the Brinkmann number and Ree–Eyring parameter.
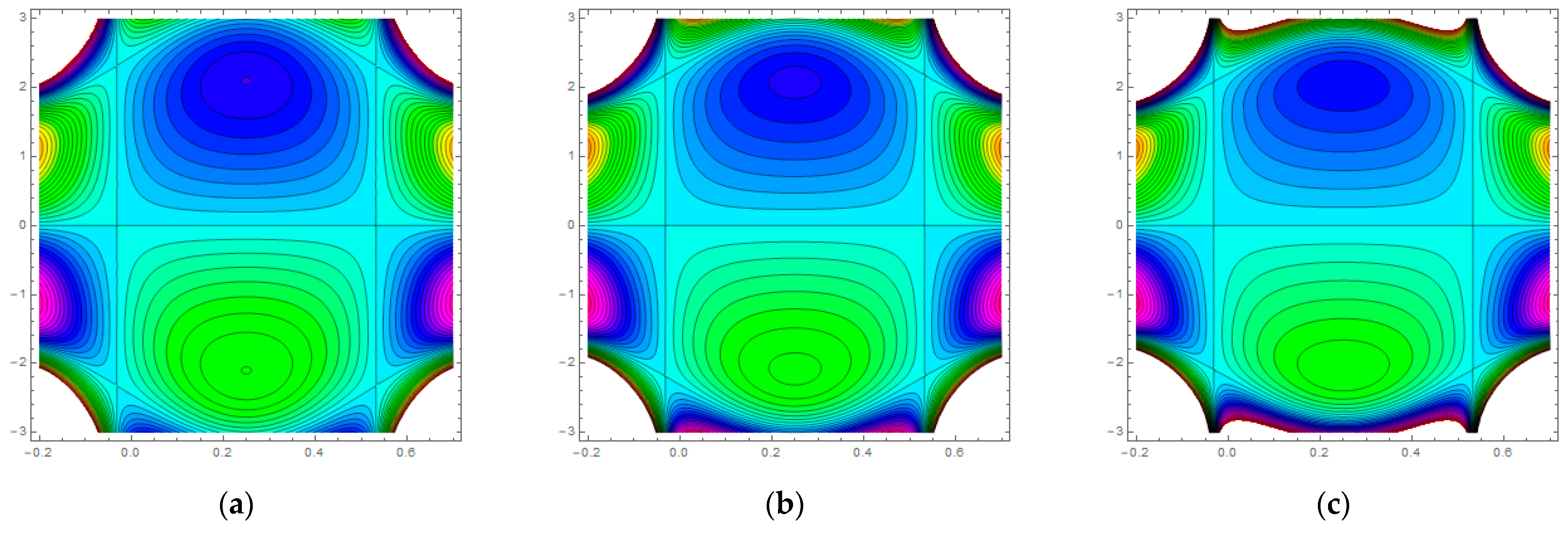
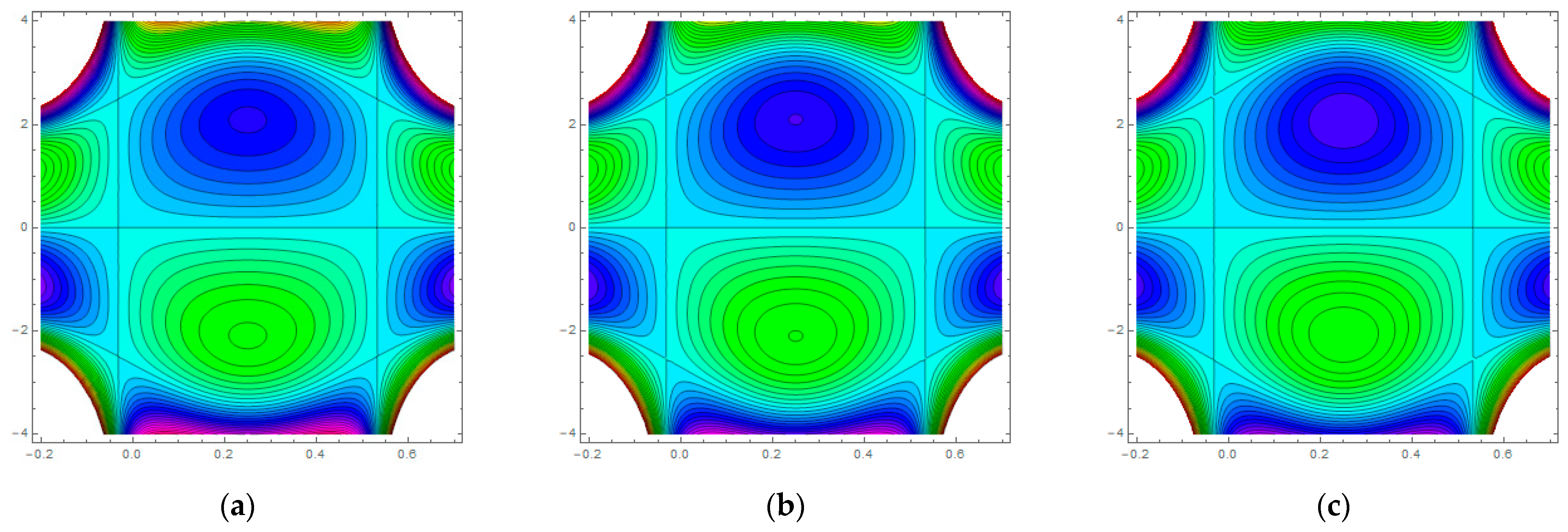
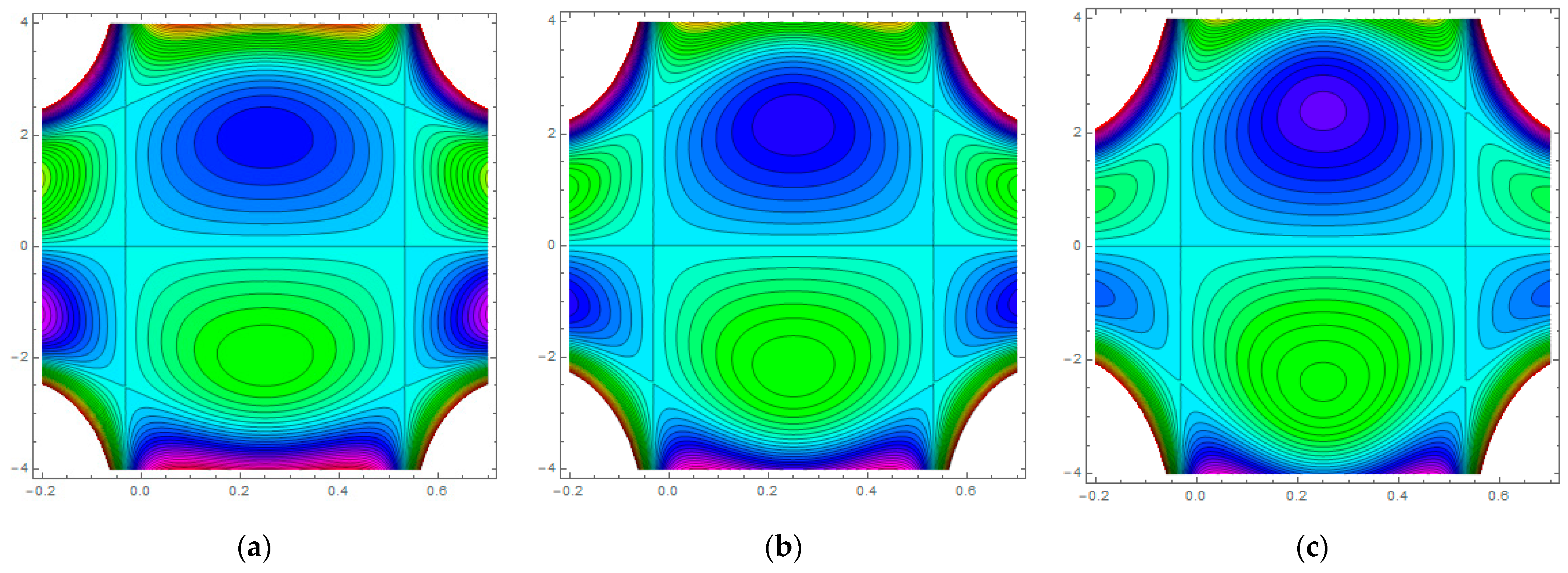
- With elevating values, both the Hartmann number and Ree–Eyring parameter convey an opposite impact on the formation of streamlined trajectories.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Johnson, K.L. Non-Newtonian Effects in Elastohydrodynamic Lubrication. In Tribology Series; Elsevier: Amsterdam, The Netherlands, 1993; Volume 25, pp. 15–26. [Google Scholar]
- Nouri, J.M.; Umur, H.; Whitelaw, J.H. Flow of Newtonian and non-Newtonian fluids in concentric and eccentric annuli. J. Fluid Mech. 1993, 253, 617–641. [Google Scholar] [CrossRef]
- Chhabra, R.P.; Richardson, J.F. Non-Newtonian Flow and Applied Rheology: Engineering Applications; Butterworth-Heinemann: Boston, MA, USA, 2011. [Google Scholar]
- Molla, M.; Yao, L. Mixed convection of non-Newtonian fluids along a heated vertical flat plate. Int. J. Heat Mass Transf. 2009, 52, 3266–3271. [Google Scholar] [CrossRef]
- Sochi, T. Flow of non-Newtonian fluids in porous media. J. Polym. Sci. Part B Polym. Phys. 2010, 48, 2437–2767. [Google Scholar] [CrossRef]
- Ryltseva, K.; Borzenko, E.; Shrager, G. Non-Newtonian fluid flow through a sudden pipe contraction under non-isothermal conditions. J. Non Newton. Fluid Mech. 2020, 286, 104445. [Google Scholar] [CrossRef]
- Latham, T.W. Fluid Motions in a Peristaltic Pump. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1966. [Google Scholar]
- Nadeem, S.; Akram, S. Peristaltic flow of a Williamson fluid in an asymmetric channel. Int. J. Nonlinear Sci. Numer. Simul. 2010, 15, 1705–1716. [Google Scholar] [CrossRef]
- Akram, S.; Sohail, N.; Anwar, H. Partial slip consequences on peristaltic transport of Williamson fluid in an asymmetric channel. Walailak J. Sci. Technol. (WJST) 2015, 12, 885–908. [Google Scholar]
- Akram, S.; Sohail, N. Analytical analysis of peristaltic flow of a 6 constant Jeffreys model of fluid in an inclined planar channel. Walailak J. Sci. Technol. (WJST) 2014, 11, 129–148. [Google Scholar]
- Pandey, S.K.; Tripathi, D. Peristaltic transport of a Casson fluid in a finite channel: Application to flows of concentrated fluids in oesophagus. Int. J. Biomath. 2010, 3, 453–472. [Google Scholar] [CrossRef]
- Ellahi, R.; Bhatti, M.M.; Vafai, K. Effects of heat and mass transfer on peristaltic flow in a non-uniform rectangular duct. Int. J. Heat Mass Transf. 2014, 71, 706–719. [Google Scholar] [CrossRef]
- Riaz, A.; Nadeem, S.; Ellahi, R.; Zeeshan, A. Exact Solution for Peristaltic Flow of Jeffrey Fluid Model in a Three Dimensional Rectangular Duct having Slip at the Walls. Appl. Bionics Biomech. 2014, 11, 81–90. [Google Scholar] [CrossRef]
- Abbas, M.A.; Bai, Y.; Bhatti, M.M.; Rashidi, M.M. Three dimensional peristaltic flow of hyperbolic tangent fluid in non-uniform channel having flexible walls. Alex. Eng. J. 2016, 55, 653–662. [Google Scholar] [CrossRef]
- Imran, M.; Shaheen, A.; Sherif, E.-S.M.; Rahimi-Gorji, M.; Seikh, A.H. Analysis of peristaltic flow of Jeffrey six constant nano fluid in a vertical non-uniform tube. Chin. J. Phys. 2020, 66, 60–73. [Google Scholar] [CrossRef]
- Basha, H.; Sivaraj, R. Entropy generation of peristaltic Eyring–Powell nanofluid flow in a vertical divergent channel for biomedical applications. Proc. Inst. Mech. Eng. Part E J. Process. Mech. Eng. 2021, 235, 1575–1586. [Google Scholar] [CrossRef]
- Ebaid, A. A new numerical solution for the MHD peristaltic flow of a bio-fluid with variable viscosity in a circular cylindrical tube via Adomian decomposition method. Phys. Lett. A 2008, 372, 5321–5328. [Google Scholar] [CrossRef]
- Akbar, N.S. Influence of magnetic field on peristaltic flow of a Casson fluid in an asymmetric channel: Application in crude oil refinement. J. Magn. Magn. Mater. 2015, 378, 463–468. [Google Scholar] [CrossRef]
- Sinha, A.; Shit, G.C.; Ranjit, N.K. Peristaltic transport of MHD flow and heat transfer in an asymmetric channel: Effects of variable viscosity, velocity-slip and temperature jump. Alex. Eng. J. 2015, 54, 691–704. [Google Scholar] [CrossRef]
- Bhatti, M.M.; Ellahi, R.; Zeeshan, A. Study of variable magnetic field on the peristaltic flow of Jeffrey fluid in a non-uniform rectangular duct having compliant walls. J. Mol. Liq. 2016, 222, 101–108. [Google Scholar] [CrossRef]
- Abbas, M.A.; Bai, Y.Q.; Rashidi, M.M.; Bhatti, M.M. Application of Drug Delivery in Magnetohydrodynamics Peristaltic Blood Flow of Nanofluid in a Non-Uniform Channel. J. Mech. Med. Biol. 2016, 16, 1650052. [Google Scholar] [CrossRef]
- Abd-Alla, A.M.; Thabet, E.N.; Bayones, F.S. Numerical solution for MHD peristaltic transport in an inclined nanofluid symmetric channel with porous medium. Sci. Rep. 2022, 12, 3348. [Google Scholar] [CrossRef]
- Bejan, A. A Study of Entropy Generation in Fundamental Convective Heat Transfer. J. Heat Transf. 1979, 101, 718–725. [Google Scholar] [CrossRef]
- Akbar, N.S. Entropy Generation Analysis for a CNT Suspension Nanofluid in Plumb Ducts with Peristalsis. Entropy 2015, 17, 1411–1424. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Bhatti, M.M.; Abbas, M.A.; Ali, M.E.-S. Entropy Generation on MHD Blood Flow of Nanofluid Due to Peristaltic Waves. Entropy 2016, 18, 117. [Google Scholar] [CrossRef]
- Asha, S.; Deepa, C. Entropy generation for peristaltic blood flow of a magneto-micropolar fluid with thermal radiation in a tapered asymmetric channel. Results Eng. 2019, 3, 100024. [Google Scholar] [CrossRef]
- Bibi, A.; Xu, H. Entropy Generation Analysis of Peristaltic Flow and Heat Transfer of a Jeffery Nanofluid in a Horizontal Channel under Magnetic Environment. Math. Probl. Eng. 2019, 2019, 1–13. [Google Scholar] [CrossRef]
- Bhatti, M.M.; Abbas, M.A.; Rashidi, M.M. Combine effects of Magnetohydrodynamics (MHD) and partial slip on peristaltic Blood flow of Ree–Eyring fluid with wall properties. Eng. Sci. Technol. Int. J. 2016, 19, 1497–1502. [Google Scholar] [CrossRef]
- Hayat, T.; Zahir, H.; Alsaedi, A.; Ahmad, B. Heat transfer analysis on peristaltic transport of Ree-Eyring fluid in rotating frame. Chin. J. Phys. 2017, 55, 1894–1907. [Google Scholar] [CrossRef]
- Ijaz, N.; Zeeshan, A.; Bhatti, M. Peristaltic propulsion of particulate non-Newtonian Ree-Eyring fluid in a duct through constant magnetic field. Alex. Eng. J. 2018, 57, 1055–1060. [Google Scholar] [CrossRef]
- Hayat, T.; Akram, J.; Alsaedi, A.; Zahir, H. Endoscopy and homogeneous-heterogeneous reactions in MHD radiative peristaltic activity of Ree-Eyring fluid. Results Phys. 2018, 8, 481–488. [Google Scholar] [CrossRef]
- Tanveer, A.; Malik, M. Slip and porosity effects on peristalsis of MHD Ree-Eyring nanofluid in curved geometry. Ain Shams Eng. J. 2021, 12, 955–968. [Google Scholar] [CrossRef]
- Rajashekhar, C.; Mebarek-Oudina, F.; Vaidya, H.; Prasad, K.V.; Manjunatha, G.; Balachandra, H. Mass and heat transport impact on the peristaltic flow of a Ree–Eyring liquid through variable properties for hemodynamic flow. Heat Transf. 2021, 50, 5106–5122. [Google Scholar] [CrossRef]
- Balachandra, H.; Rajashekhar, C.; Mebarek-Oudina, F.; Manjunatha, G.; Vaidya, H.; Prasad, K.V. Slip Effects on a Ree-Eyring Liquid Peristaltic Flow Towards an Inclined Channel and Variable Liquid Properties. J. Nanofluids 2021, 10, 246–258. [Google Scholar] [CrossRef]
- Bhatti, M.M.; Abbas, M.A.; Rashidi, M.M. Entropy generation for peristaltic blood flow with Casson model and consideration of magnetohydrodynamics effects. Walailak J. Sci. Technol. (WJST) 2017, 14, 451–461. [Google Scholar]


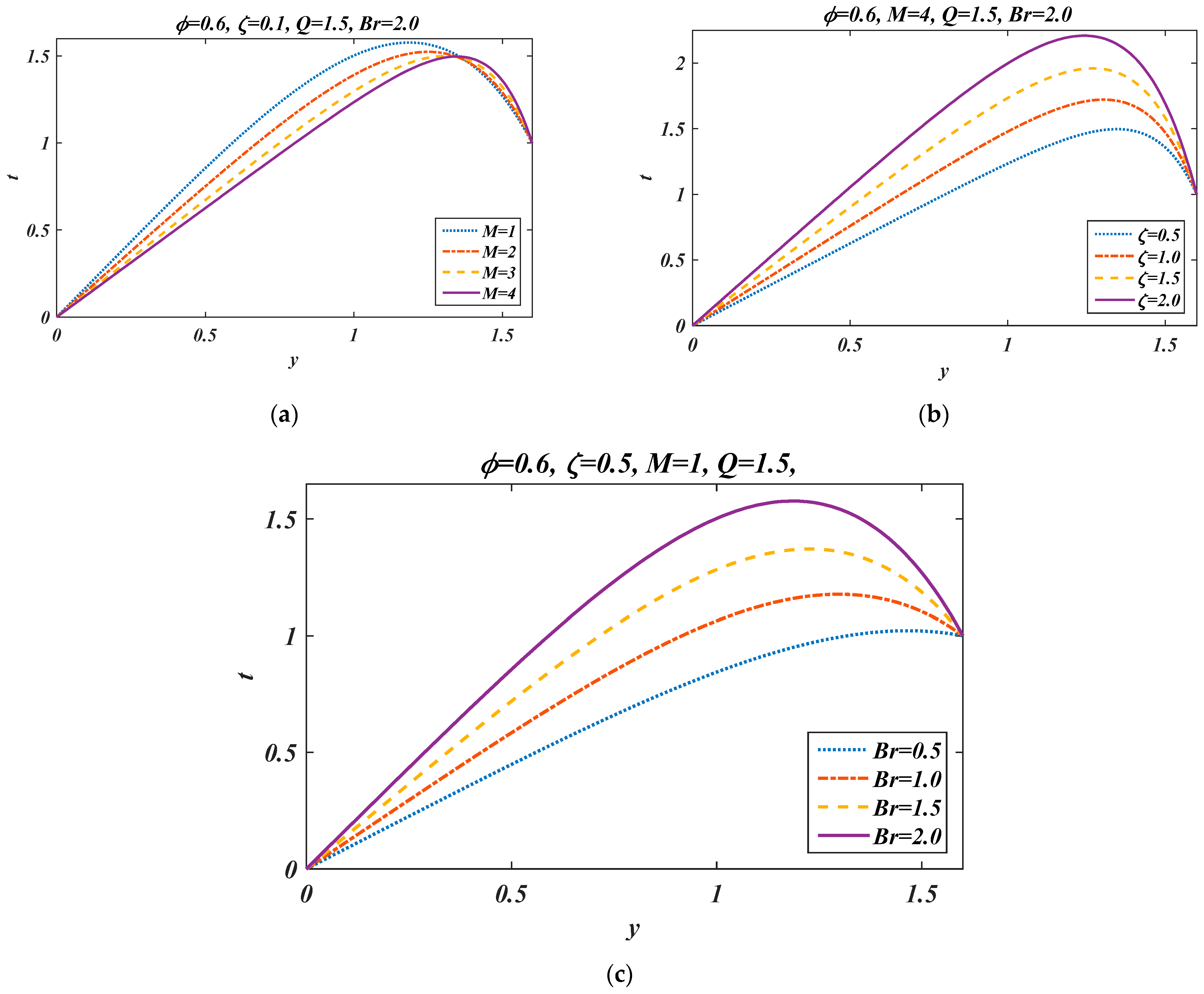
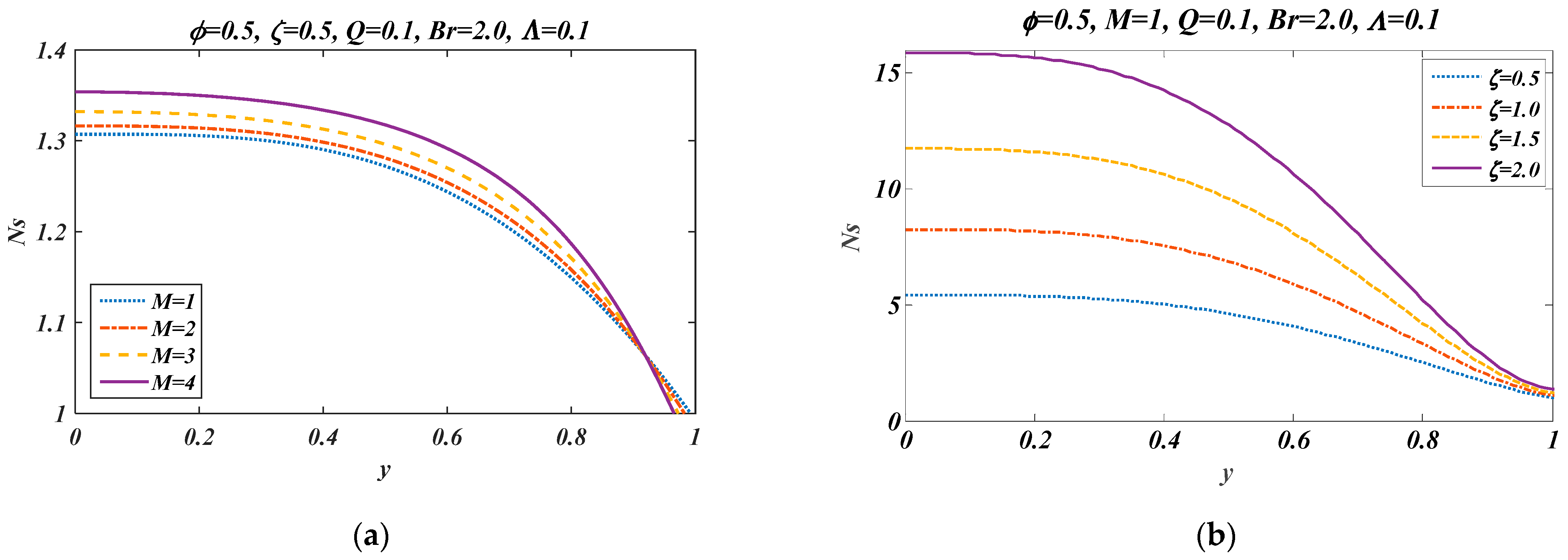

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abuasbeh, K.; Ahmed, B.; Niazi, A.U.K.; Awadalla, M. Entropy Generation for MHD Peristaltic Transport of Non-Newtonian Fluid in a Horizontal Symmetric Divergent Channel. Symmetry 2023, 15, 359. https://doi.org/10.3390/sym15020359
Abuasbeh K, Ahmed B, Niazi AUK, Awadalla M. Entropy Generation for MHD Peristaltic Transport of Non-Newtonian Fluid in a Horizontal Symmetric Divergent Channel. Symmetry. 2023; 15(2):359. https://doi.org/10.3390/sym15020359
Chicago/Turabian StyleAbuasbeh, Kinda, Bilal Ahmed, Azmat Ullah Khan Niazi, and Muath Awadalla. 2023. "Entropy Generation for MHD Peristaltic Transport of Non-Newtonian Fluid in a Horizontal Symmetric Divergent Channel" Symmetry 15, no. 2: 359. https://doi.org/10.3390/sym15020359
APA StyleAbuasbeh, K., Ahmed, B., Niazi, A. U. K., & Awadalla, M. (2023). Entropy Generation for MHD Peristaltic Transport of Non-Newtonian Fluid in a Horizontal Symmetric Divergent Channel. Symmetry, 15(2), 359. https://doi.org/10.3390/sym15020359







