Waiting for a Mathematical Theory of Living Systems from a Critical Review to Research Perspectives
Abstract
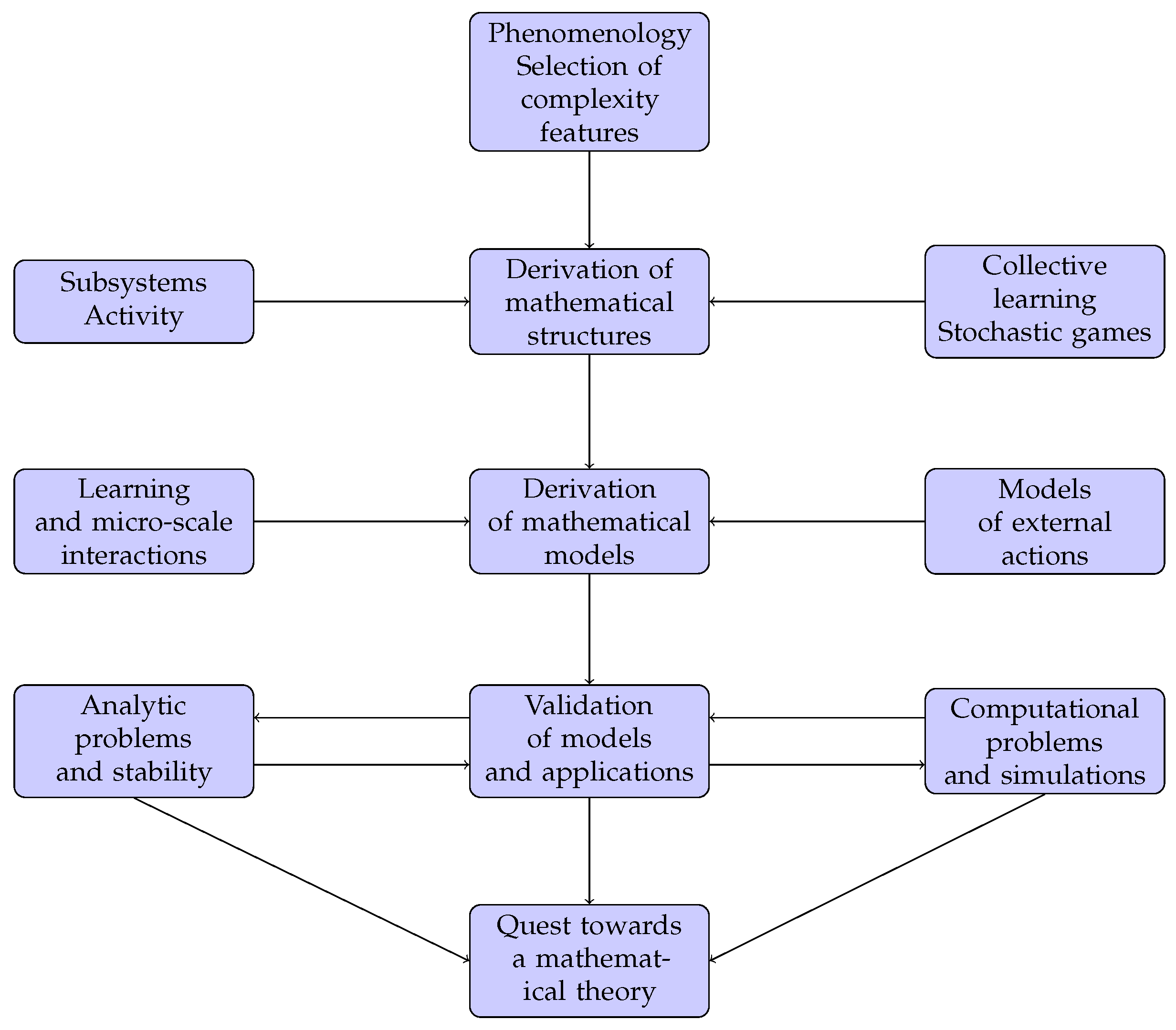
1. Motivations and Plan of the Paper
What is life and how science can contribute to a mathematical theory of living systems?
2. From a Philosophical-Mathematical Excursus towards a Mathematics of Life
2.1. A Philosophical–Mathematical Excursus
We are surrounded by complex systems. Familiar examples include power grids, transportation systems, financial markets, the Internet, and structures underlying everything from the environment to the cells in our bodies. Mathematical models can guide us in understanding these systems […].
Living matter, while not eluding the “laws of physics” as established up to date, is likely to involve “other laws of physic” hitherto unknown, which however, once they have been revealed, will form just as integral a part of science as the former.
Although living systems obey the laws of physics and chemistry, the notion of function or purpose differentiates biology from other natural sciences. Organisms exist to reproduce, whereas, outside religious belief, rocks and stars have no purpose. Selection for function has produced the living cell, with a unique set of properties that distinguish it from inanimate systems of interacting molecules.
2.2. On a Strategy towards a Mathematics of Living Systems
- Expression of functions: Living entities, which may be defined micro-systems to account that their representation is at the lower scale with respect to that of the global system. An alternative definition is active particles, shortly a-particles, see [18]. These have the ability to express a function called activity. These entities are able to develop specific strategies and organization abilities that depend on the state of the surrounding entities and environment.
- Heterogeneity: The ability to express the activity is not the same for all micro-systems as the expression of heterogeneous behaviors is a common feature of a great part of living systems.
- Nonlinearity of interactions: Interactions are nonlinearly additive, nonlocal, as they may involve entities that are not immediate neighbors and, in some cases, asymmetric. Active particles are sensitive not only to a selection of other individual entities, but to the overall system as a whole.
- Learning ability: Living systems receive inputs from the environments and have the ability to learn from past experience. Accordingly, the rules of interaction and activity they develop evolves in time.
- Functional subsystems: Active particles can aggregate into groups, called functional subsystems, shortly FSs, where they pursue the same objectives, share the same activity and interaction rules.
- Output of interactions: Interactions modify the activity and generate proliferative and destructive events.
- Darwinian mutations and selection: All living systems are evolutionary, as interactions can generate, by birth of aggregations, new entities that are increasingly fitted to the environment, that, in turn, generate new entities again more fitted to the environment.
- The overall system of a-particles is subdivided into functional subsystems accounting for the activity variables rather than physical properties of the matter of the system.
- The state of each FS is described by the one particle distribution function over the micro-state of the a-particles belonging to it: with for all , where t is time, and model the localization and velocity of the a-particle, and is the activity shared by all a-particles of the FS.
- Derivation of a general mathematical structure with the aim of offering the conceptual framework toward the derivation of specific models. This structure is required to express the dynamics of the complexity features of living systems.
- Derivation of specific models corresponding to well-defined classes of systems by implementing the said structure with suitable models of individual-based, micro-scale, interactions.
- The phenomenological interpretation of living systems can take advantage of specific measurement devices, but it is always somehow conditioned by individual sensitivity within the framework of the sensitive world by Immanuel Kant [23,24] or even within the framework of the artificial world by Herbert Simon [25].
- Generally, the derivation of models is developed by a hierarchy in which each micro-system firstly learn from the other micro-systems and subsequently, modifies the mechanical state.
- The application of models generates challenging analytic and computational problems which require new inventions to tackle them in real applications by the critical analysis developed in the next section after a review of specific classes of models.
3. From Active Particles Methods to Selected Applications
3.1. Mathematical Models in Space Homogeneity
- Interaction rate for conservative dynamics:, which models the frequency of the interactions between a candidate i-micro-system with state and a field k-micro-system with state , where the label i denotes the FS.
- Interaction rate for non-conservative dynamics:, is analogous to , but corresponding to proliferative and destructive interactions.
- Transition probability density:, which denotes the probability density that a candidate i-micro-system, with state , ends up into the state of the test micro-system of the same FS after an interaction with a field k-micro-system.
- Proliferative term:, which models the proliferative events for a candidate h-particle, with state , into the same FS after interaction with a field k-particle with state .
- Destructive term:, which models the rate of destruction for a test i-particle in its own functional subsystem after an interaction with a field k-particle with state .
- Modeling the competition between a virus and the immune system, accounting for the progression of the virus and the activation of the immune system has been proposed in [32].
- Somehow inspired to the above literature is the study developed in [33] to model the in-host dynamics in the upper respiratory tracts, where the virus proliferates, although contrasted by the immune system attempting to reduce the invasion ability of the virus. Further developments have been presented in [34] to consider the role of mutations and onset of new variants.
3.2. Mathematical Models with Space Dynamics
3.3. A Forward Look to Perspectives in Modeling
- Vector activity variable and related hierarchy of the development of the dynamics. Indeed, more that one variable might be necessary to characterize micro-systems. The difficulty in identifying the correlations between them suggests to understand whether the decision-making process, as often happens in living systems, follows a sequence linked to the components of the activity.
- Correlation of the role of the activity to the dynamics of the mechanical variables. This means selecting the velocity directions and then, adapting the speed to the local flow conditions. This selection is activity-driven. As an example, increasing stress conditions increases the tendency towards the main (overcrowded) stream rather than the seeking less crowded areas.
- Including mutations and selection also in the study of social systems. This type of dynamics is typical of biological systems. However, a dynamic across functional subsystems can be observed also in social systems. For instance in opinion dynamics micro-systems can move from a group of interest to another one.
- Exploring new mathematical structures. The reasoning on the possible developments of the mathematical structures reviewed in this section is definitely a strategic objective. However, a global vision should also consider other frameworks such as those mentioned in Section 2.2, i.e., behavioral swarms [19] and Boltzmann and Fokker-Plank methods [5] and even possible interactions between different frameworks.
- Exploring multi scale methods. The Hilbert’s sixth problem concerns the search of a unified mathematical approach to physical theories. A conceivable preliminary step would be the derivation of models at all scales by the same principles and, subsequently, the passage to mathematical (hence rigorous) methods from the low to the higher scale. This passage is depicted in Figure 2.
4. On a Forward Look to a Mathematical Theory of Living Systems
After selecting of a specific class of case studies, databases can be organized to collect data on a selected number of interactions which are specific to the case studies. The selection should correspond to the causality action characterizing each interaction. Therefore, selecting in the database the dynamics close, with a suitable metric, to those treated by a model would lead to the calibration of the model.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hilbert, D. Mathematical problems. Bull. Am. Math. Soc. 1902, 8, 437–479. [Google Scholar] [CrossRef]
- Ball, P. Why Society Is a Complex Matter; Springer: Heidelberg, Germany, 2012. [Google Scholar]
- Bellomo, N.; Bellouquid, A.; Gibelli, L.; Outada, N. A Quest towards a Mathematical Theory of Living Systems; Modelling and Simulations in Science, Engineering and Technology; Birkhäuser: New York, NY, USA, 2017. [Google Scholar]
- Helbing, D. Quantitative Sociodynamics. Stochastic Methods and Models of Social Interaction Processes; Springer: Heidelberg, Germany; New York, NY, USA, 2010. [Google Scholar]
- Pareschi, L.; Toscani, G. Interacting Multiagent Systems: Kinetic Equations and Monte Carlo Methods; Oxford University Press: Oxford, UK, 2013. [Google Scholar]
- Bellouquid, A.; Delitala, M. Modelling Complex Biological Systems—A Kinetic Theory Approach; Modeling and Simulation in Science, Engineering and Technology; Birkhäuser: New York, NY, USA, 2006. [Google Scholar]
- Schrödinger, E. What Is Life? The Physical Aspect of the Living Cell; Cambridge University Press: Cambridge, UK, 1944. [Google Scholar]
- Prigogine, I.; Herman, R. Kinetic Theory of Vehicular Traffic; Elsevier: New York, NY, USA, 1971. [Google Scholar]
- Paveri Fontana, S. On Boltzmann like treatments for traffic flow. Transp. Res. 1975, 9, 225–235. [Google Scholar]
- Jager, E.; Segel, L.A. On the distribution of dominance in a population of interacting anonymous organisms. SIAM J. Appl. Math. 1992, 52, 1442–1468. [Google Scholar] [CrossRef]
- Bellomo, N.; Forni, G. Dynamics of tumor interactions with the host immune system. Math. Comput. Model. 1994, 20, 107–122. [Google Scholar] [CrossRef]
- Kwon, H.R.; Silva, E.A. Mapping the Landscape of Behavioral Theories: Systematic Literature Review. J. Plan. Lit. 2019, 35, 161–179. [Google Scholar] [CrossRef]
- Aristov, V.V. Biological systems as nonequilibrium structures described by kinetic methods. Res. Phys. 2019, 13, 102232. [Google Scholar] [CrossRef]
- Mayr, E. Toward a New Philosophy of Biology; Harvard University Press: Cambridge, MA, USA, 1989. [Google Scholar]
- May, R.M. Uses and abuses of mathematics in biology. Science 2004, 303, 338–342. [Google Scholar] [CrossRef] [PubMed]
- Herrero, M.A. On the role of mathematics in biology. J. Math. Biol. 2007, 54, 887–889. [Google Scholar] [CrossRef] [PubMed]
- Hartwell, H.L.; Hopfield, J.J.; Leibler, S.; Murray, A.W. From molecular to modular cell biology. Nature 1999, 402, c47–c52. [Google Scholar] [CrossRef] [PubMed]
- Bellomo, N.; Burini, D.; Dosi, G.; Gibelli, L.; Knopoff, D.A.; Outada, N.; Terna, P.; Virgillito, M.E. What is life? A perspective of the mathematical kinetic theory of active particles. Math. Models Methods Appl. Sci. 2021, 31, 1821–1866. [Google Scholar] [CrossRef]
- Bellomo, N.; Ha, S.-Y.; Outada, N. Towards a Mathematical Theory of Behavioral Swarms. ESAIM Control Theory Var. Calc. 2020, 26, 125. [Google Scholar] [CrossRef]
- Cucker, F.; Smale, S. Emergent behavior in flocks. IEEE Trans. Autom. Control 2007, 52, 853–862. [Google Scholar] [CrossRef]
- Burini, D.; De Lillo, S.; Gibelli, L. Collective learning modeling based on the kinetic theory of active particles. Phys. Life Rev. 2016, 16, 126–139. [Google Scholar] [CrossRef]
- Burini, D.; De Lillo, S. On the complex interaction between collective learning and social dynamics. Symmetry 2019, 11, 967. [Google Scholar] [CrossRef]
- Kant, I. De Mundi Sensibilis Atque Intellegibilis Forma and Pricipi; Felix Meiner Verlag: Hamburg, Germany, 1958. [Google Scholar]
- Kant, I. Critique of the Power of Judgement; English Translation; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Simon, H.A. Science of the Artificial, 3rd ed.; MIT Press: Boston, MA, USA, 2019. [Google Scholar]
- Bertotti, M.L.; Delitala, M. Cluster formation in opinion dynamics: A qualitative analysis. Zeitschrift fur Angewandte Mathematik und Physik 2010, 61, 583–602. [Google Scholar] [CrossRef]
- Dolfin, M.; Lachowicz, M. Modeling opinion dynamics: How the network enhances consensus. Netw. Heterog. Media 2015, 10, 421–441. [Google Scholar] [CrossRef]
- Lachowicz, M.; Leszczyński, H.; Puźniakowska-Galuch, E. Diffusive and anti-diffusive behavior for kinetic models of opinion dynamics. Symmetry 2019, 11, 1024. [Google Scholar] [CrossRef]
- Bellouquid, A.; De Angelis, E.; Knopoff, D.A. From the modeling of the immune hallmarks of cancer to a black swan in biology. Math. Models Methods Appl. Sci. 2013, 23, 949–978. [Google Scholar] [CrossRef]
- Hanahan, D.; Weinberg, R.A. Hallmarks of cancer: The next generation. Cell 2011, 144, 646–674. [Google Scholar] [CrossRef]
- Weinberg, R.A. The Biology of Cancer; Garland Sciences—Taylor and Francis: New York, NY, USA, 2007. [Google Scholar]
- De Lillo, S.; Delitala, M.; Salvatori, M. Modelling epidemics and virus mutations by methods of the mathematical kinetic theory for active particles. Math. Models Methods Appl. Sci. 2009, 19, 1405–1425. [Google Scholar] [CrossRef]
- Bellomo, N.; Bingham, R.; Chaplain, M.A.J.; Dosi, G.; Forni, G.; Knopoff, D.A.; Lowengrub, J.; Twarock, R.; Virgillito, M.E. A multi-scale model of virus pandemic: Heterogeneous interactive entities in a globally connected world. Math. Models Methods Appl. Sci. 2020, 30, 1591–1651. [Google Scholar] [CrossRef]
- Bellomo, N.; Burini, D.; Outada, N. Multiscale models of Covid-19 with mutations and variants. Netw. Heterog. Media 2022, 17, 293–310. [Google Scholar] [CrossRef]
- Dolfin, M.; Leonida, L.; Outada, N. Modelling human behaviour in economics and social science. Phys. Life Rev. 2017, 22–23, 1–21. [Google Scholar] [CrossRef] [PubMed]
- Ajmone Marsan, G.; Bellomo, N.; Gibelli, L. Stochastic evolutionary differential games toward a systems theory of behavioral social dynamics. Math. Models Methods Appl. Sci. 2016, 26, 1051–1093. [Google Scholar] [CrossRef]
- Bonacich, P.; Lu, P. Introduction to Mathematical Sociology; University Press: Princeton, NJ, USA, 2012. [Google Scholar]
- Dolfin, M.; Knopoff, D.; Leonida, L.; Patti, D. Escaping the trap of “blocking”: A kinetic model linking economic development and political competition. Kinet. Relat. Models 2017, 10, 423–443. [Google Scholar] [CrossRef]
- Dolfin, M.; Lachowicz, M. Modeling altruism and selfishness in welfare dynamics: The role of nonlinear interactions. Math. Models Methods Appl. Sci. 2014, 24, 2361–2381. [Google Scholar] [CrossRef]
- Bellomo, N.; Ha, S.-Y. A quest toward a mathematical theory of the dynamics of swarms. Math. Models Methods Appl. Sci. 2017, 27, 745–770. [Google Scholar] [CrossRef]
- Coscia, V.; Delitala, M.; Frasca, P. On the mathematical theory of vehicular traffic flow models II. Discrete velocity kinetic models. Int. J. Non-Linear Mech. 2007, 42, 411–421. [Google Scholar] [CrossRef]
- Delitala, M.; Tosin, A. Mathematical modelling of vehicular traffic: A discrete kinetic theory approach. Math. Models Methods Appl. Sci. 2007, 17, 901–932. [Google Scholar] [CrossRef]
- Burini, D.; De Lillo, S.; Fioriti, G. Influence of drivers ability in a discrete vehicular traffic model. Int. J. Mod. Phys. C 2017, 28, 1750030. [Google Scholar] [CrossRef]
- Fermo, L.; Tosin, A. A fully-discrete-state kinetic theory approach to modeling vehicular traffic. SIAM J. Appl. Math. 2013, 73, 1533–1556. [Google Scholar] [CrossRef]
- Calvo, J.; Nieto, J.; Zagour, M. Kinetic Model for Vehicular Traffic with Continuum Velocity and Mean Field Interactions. Symmetry 2019, 11, 1093. [Google Scholar] [CrossRef]
- Ronchi, E. Disaster management: Design buildings for rapid evacuation. Nature 2015, 528, 333. [Google Scholar] [CrossRef] [PubMed]
- Ronchi, E.; Kuligowski, E.D.; Nilsson, D.; Peacock, R.D.; Reneke, P.A. Assessing the verification and validation of building fire evacuation models. Fire Technol. 2016, 52, 197–219. [Google Scholar] [CrossRef]
- Ronchi, F.; Nieto Uriz, F.; Criel, X.; Reilly, P. Modelling large-scale evacuation of music festival. Fire Saf. 2016, 5, 11–19. [Google Scholar] [CrossRef]
- Bellomo, N.; Gibelli, L. Toward a mathematical theory of behavioral-social dynamics for pedestrian crowds. Math. Models Methods Appl. Sci. 2015, 25, 2417–2437. [Google Scholar] [CrossRef]
- Bellomo, N.; Gibelli, L.; Outada, N. On the interplay between behavioral dynamics and social interactions in human crowds. Kinet. Relat. Models 2019, 12, 397–409. [Google Scholar] [CrossRef]
- Aylaj, B.; Bellomo, N.; Gibelli, L.; Reali, A. On a unified multiscale vision of behavioral crowds. Math. Models Methods Appl. Sci. 2020, 30, 1–22. [Google Scholar] [CrossRef]
- Elaiw, A.; Al-Turki, Y. Particle methods simulations by kinetic theory models of human crowds accounting for stress conditions. Symmetry 2019, 12, 14. [Google Scholar] [CrossRef]
- Aristov, V.V. Direct Methods for Solving the Boltzmann Equation and Study of Nonequilibrium Flows; Springer: New York, NY, USA, 2001. [Google Scholar]
- Elaiw, A.; Al-Turki, Y.; Alghamdi, M. A critical analysis of behavioural crowd dynamics: From a modelling strategy to kinetic theory methods. Symmetry 2019, 11, 851. [Google Scholar] [CrossRef]
- Bellomo, N.; Gibelli, L.; Quaini, A.; Reali, A. Towards a mathematical theory of behavioral human crowds. Math. Models Methods Appl. Sci. 2022, 32, 321–358. [Google Scholar] [CrossRef]
- Knopoff, D.; Nieto, J.; Urrutia, L. Numerical simulation of a multiscale cell motility model based on the kinetic theory of active particles. Symmetry 2019, 11, 1003. [Google Scholar] [CrossRef]
- Kim, D.; Quaini, A. A kinetic theory approach to model pedestrian dynamics in bounded domains with obstacles. Kinet. Relat. Models 2019, 12, 1273–1296. [Google Scholar] [CrossRef]
- Kim, D.; Quaini, A. Coupling kinetic theory approaches for pedestrian dynamics and disease contagion in a confined environment. Math. Models Methods Appl. Sci. 2020, 30, 1893–1915. [Google Scholar] [CrossRef]
- Saint-Raymond, L. Hydrodynamic Limits of the Boltzmann Equation; Lecture Notes in Mathematics n.1971; Springer: Berlin, Germany, 2009. [Google Scholar]
- Burini, D.; Chouhad, N. A Multiscale view of nonlinear diffusion in biology: From cells to tissues. Math. Models Methods Appl. Sci. 2019, 29, 791–823. [Google Scholar] [CrossRef]
- Burini, D.; Chouhad, N. Virus Models in Complex Frameworks towards Modeling Space Patterns of SARS-CoV-2 Epidemics. Math. Models Methods Appl. Sci. 2022, 32, 2017–2036. [Google Scholar] [CrossRef]
- Othmer, H.G.; Dunbar, S.R.; Alt, W. Models of dispersal in biological systems. J. Math. Biol. 1988, 26, 263–298. [Google Scholar] [CrossRef]
- Othmer, H.G.; Hillen, T. The diffusion limit of transport equations II: Chemotaxis equations. SIAM J. Appl. Math. 2002, 26, 1222–1250. [Google Scholar] [CrossRef]
- Bellomo, N.; Bellouquid, A.; Chouhad, N. From a multiscale derivation of nonlinear cross-diffusion models to Keller-Segel models in a Navier-Stokes fluid. Math. Models Methods Appl. Sci. 2016, 26, 2041–2069. [Google Scholar] [CrossRef]
- Bellouquid, A.; Chouhad, N. Kinetic models of chemotaxis towards the diffusive limit: Asymptotic analysis. Math. Methods Appl. Sci. 2016, 39, 3136–3151. [Google Scholar] [CrossRef]
- Nieto, J.; Urrutia, L. A multiscale model of cell mobility: From a kinetic to a hydrodynamic description. J. Math. Anal. Appl. 2016, 433, 1055–1071. [Google Scholar] [CrossRef]
- Lachowicz, M. From microscopic to macroscopic description for generalized kinetic models. Math. Models Methods Appl. Sci. 2002, 12, 985–1005. [Google Scholar] [CrossRef]
- Banasiak, J.; Lachowicz, M. Methods of Small Parameter in Mathematical Biology; Modeling and Simulation in Science, Engineering and Technology; Birkhäuser: New York, NY, USA, 2014. [Google Scholar]
- Taleb, N.N. The Black Swan: The Impact of the Highly Improbable; Random House: New York, NY, USA, 2007. [Google Scholar]
- Corbetta, A.; Bruno, L.; Mountean, A.; Toschi, F. High statistics measurements of pedestrian dynamics, models via probabilistic method. Transp. Res. Proc. 2016, 2, 96–104. [Google Scholar]
- Corbetta, A.; Mountean, A.; Vafayi, K. Parameter estimation of social forces in pedestrian dynamics models via probabilistic method. Math. Biosci. Eng. 2015, 12, 337–356. [Google Scholar] [CrossRef]
- Sabeur, Z.; Arbab-Zavar, B. Crowd behaviours understanding using computer vision. In Crowd Dynamics, Volume 3; Modelling and Simulations in Science, Engineering and Technology; Birkhäuser: New York, NY, USA, 2021; pp. 49–72. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Burini, D.; Chouhad, N.; Bellomo, N. Waiting for a Mathematical Theory of Living Systems from a Critical Review to Research Perspectives. Symmetry 2023, 15, 351. https://doi.org/10.3390/sym15020351
Burini D, Chouhad N, Bellomo N. Waiting for a Mathematical Theory of Living Systems from a Critical Review to Research Perspectives. Symmetry. 2023; 15(2):351. https://doi.org/10.3390/sym15020351
Chicago/Turabian StyleBurini, Diletta, Nadia Chouhad, and Nicola Bellomo. 2023. "Waiting for a Mathematical Theory of Living Systems from a Critical Review to Research Perspectives" Symmetry 15, no. 2: 351. https://doi.org/10.3390/sym15020351
APA StyleBurini, D., Chouhad, N., & Bellomo, N. (2023). Waiting for a Mathematical Theory of Living Systems from a Critical Review to Research Perspectives. Symmetry, 15(2), 351. https://doi.org/10.3390/sym15020351





