Single-Photon Superradiance and Subradiance as Collective Emission from Symmetric and Anti-Symmetric States
Abstract
:1. Introduction
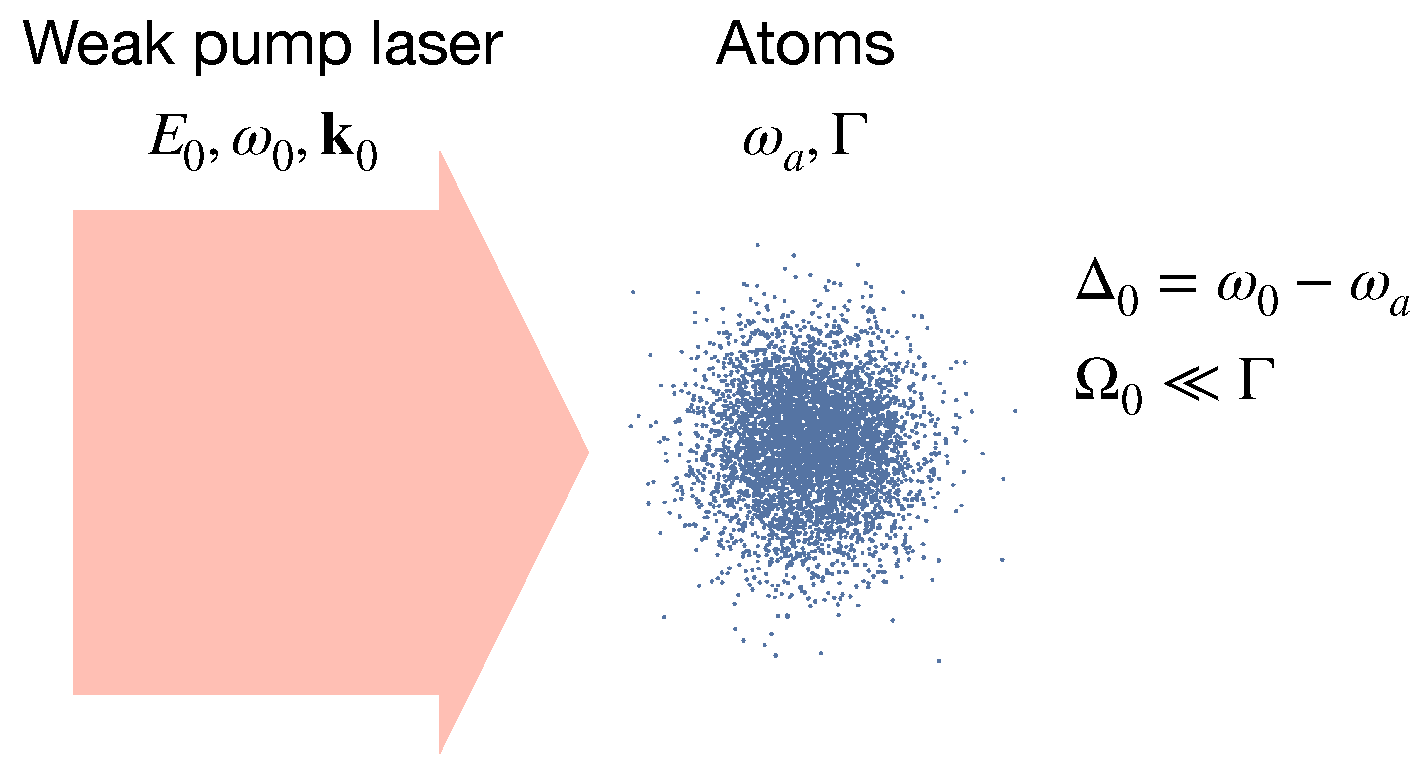
2. The Model
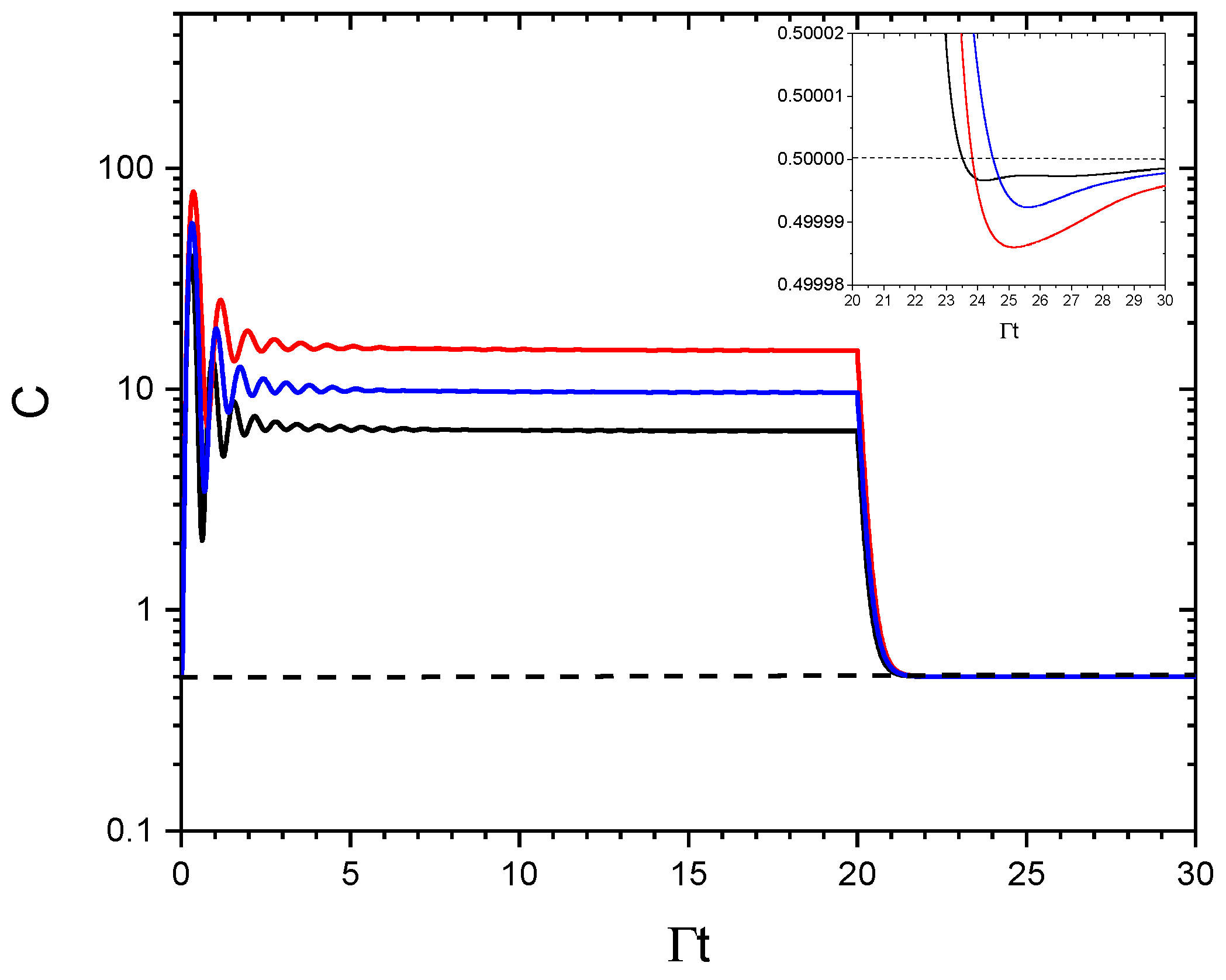
3. Entanglement Properties of the Superradiant and Subradiant Collective States
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Calculation of the First and Second Moments of
Appendix B. Considerations on Equations (34b) and (34c)
References
- Lehmberg, R.H. Radiation from an N-Atom System. I. General Formalism. Phys. Rev. A 1970, 2, 883–888. [Google Scholar] [CrossRef]
- van der Mark, M.B.; van Albada, M.P.; Lagendijk, A. Light scattering in strongly scattering media: Multiple scattering and weak localization. Phys. Rev. B 1988, 37, 3575–3592. [Google Scholar] [CrossRef] [PubMed]
- Labeyrie, G.; Vaujour, E.; Mueller, C.A.; Delande, D.; Miniatura, C.; Wilkowski, D.; Kaiser, R. Slow diffusion of light in a cold atomic cloud. Phys. Rev. Lett. 2003, 91, 223904. [Google Scholar] [CrossRef] [PubMed]
- Guerin, W.; Rouabah, M.; Kaiser, R. Light interacting with atomic ensembles: Collective, cooperative and mesoscopic effects. J. Mod. Opt. 2017, 64, 895–907. [Google Scholar] [CrossRef]
- Dicke, R.H. Coherence in Spontaneous Radiation Processes. Phys. Rev. 1954, 93, 99–100. [Google Scholar] [CrossRef]
- Nourmandipour, A.; Vafafard, A.; Mortezapour, A.; Franzosi, R. Entanglement protection of classically driven qubits in a lossy cavity. Sci. Rep. 2021, 11, 16259. [Google Scholar] [CrossRef]
- Taghipour, J.; Mojaveri, B.; Dehghani, A. Witnessing entanglement between two two-level atoms coupled to a leaky cavity via two-photon relaxation. Eur. Phys. J. Plus 2022, 137, 772. [Google Scholar] [CrossRef]
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Gross, M.; Haroche, S. Superradiance: An essay on the theory of collective spontaneous emission. Phys. Rep. 1982, 93, 301–396. [Google Scholar] [CrossRef]
- Lodahl, P.; Floris van Driel, A.; Nikolaev, I.S.; Irman, A.; Overgaag, K.; Vanmaekelbergh, D.; Vos, W.L. Controlling the dynamics of spontaneous emission from quantum dots by photonic crystals. Nature 2004, 430, 654–657. [Google Scholar] [CrossRef]
- Brandes, T. Coherent and collective quantum optical effects in mesoscopic systems. Phys. Rep. 2005, 408, 315–474. [Google Scholar] [CrossRef]
- Karasik, R.I.; Marzlin, K.P.; Sanders, B.C.; Whaley, K.B. Multiparticle decoherence-free subspaces in extended systems. Phys. Rev. A 2007, 76, 012331. [Google Scholar] [CrossRef]
- Pedersen, L.H.; Mølmer, K. Few qubit atom-light interfaces with collective encoding. Phys. Rev. A 2009, 79, 012320. [Google Scholar] [CrossRef]
- Tong, D.; Farooqi, S.; Stanojevic, J.; Krishnan, S.; Zhang, Y.; Côté, R.; Eyler, E.; Gould, P. Local blockade of Rydberg excitation in an ultracold gas. Phys. Rev. Lett. 2004, 93, 063001. [Google Scholar] [CrossRef] [PubMed]
- Urban, E.; Johnson, T.A.; Henage, T.; Isenhower, L.; Yavuz, D.; Walker, T.; Saffman, M. Observation of Rydberg blockade between two atoms. Nat. Phys. 2009, 5, 110–114. [Google Scholar] [CrossRef]
- Gaëtan, A.; Miroshnychenko, Y.; Wilk, T.; Chotia, A.; Viteau, M.; Comparat, D.; Pillet, P.; Browaeys, A.; Grangier, P. Observation of collective excitation of two individual atoms in the Rydberg blockade regime. Nat. Phys. 2009, 5, 115–118. [Google Scholar] [CrossRef]
- Scully, M.; Fry, E.; Ooi, C.; Wodkiewicz, K. Directed Spontaneous Emission from an Extended Ensemble of N Atoms: Timing Is Everything. Phys. Rev. Lett. 2006, 96, 010501. [Google Scholar] [CrossRef]
- Svidzinsky, A.A.; Chang, J.T.; Scully, M.O. Dynamical Evolution of Correlated Spontaneous Emission of a Single Photon from a Uniformly Excited Cloud of N Atoms. Phys. Rev. Lett. 2008, 100, 160504. [Google Scholar] [CrossRef]
- Svidzinsky, A.A.; Chang, J.T.; Scully, M.O. Cooperative spontaneous emission of N atoms: Many-body eigenstates, the effect of virtual Lamb shift processes, and analogy with radiation of N classical oscillators. Phys. Rev. A 2010, 81, 053821. [Google Scholar] [CrossRef]
- Courteille, P.W.; Bux, S.; Lucioni, E.; Lauber, K.; Bienaimé, T.; Kaiser, R.; Piovella, N. Modification of radiation pressure due to cooperative scattering of light. Eur. Phys. J. D 2010, 58, 69–73. [Google Scholar] [CrossRef]
- Bienaimé, T.; Bux, S.; Lucioni, E.; Courteille, P.; Piovella, N.; Kaiser, R. Observation of a Cooperative Radiation Force in the Presence of Disorder. Phys. Rev. Lett. 2010, 104, 183602. [Google Scholar] [CrossRef]
- Bienaimé, T.; Bachelard, R.; Piovella, N.; Kaiser, R. Cooperativity in light scattering by cold atoms. Fortschritte Der Phys. 2013, 61, 377–392. [Google Scholar] [CrossRef]
- Bienaimé, T.; Piovella, N.; Kaiser, R. Controlled Dicke Subradiance from a Large Cloud of Two-Level Systems. Phys. Rev. Lett. 2012, 108, 123602. [Google Scholar] [CrossRef] [PubMed]
- Guerin, W.; Araújo, M.O.; Kaiser, R. Subradiance in a Large Cloud of Cold Atoms. Phys. Rev. Lett. 2016, 116, 083601. [Google Scholar] [CrossRef]
- Scully, M.O. Single photon subradiance: Quantum control of spontaneous emission and ultrafast readout. Phys. Rev. Lett. 2015, 115, 243602. [Google Scholar] [CrossRef] [PubMed]
- Svidzinsky, A.A.; Zhang, X.; Scully, M.O. Quantum versus semiclassical description of light interaction with atomic ensembles: Revision of the Maxwell-Bloch equations and single-photon superradiance. Phys. Rev. A 2015, 92, 013801. [Google Scholar] [CrossRef]
- Cai, H.; Wang, D.W.; Svidzinsky, A.A.; Zhu, S.Y.; Scully, M.O. Symmetry-protected single-photon subradiance. Phys. Rev. A 2016, 93, 053804. [Google Scholar] [CrossRef]
- Gegg, M.; Carmele, A.; Knorr, A.; Richter, M. Superradiant to subradiant phase transition in the open system Dicke model: Dark state cascades. New J. Phys. 2018, 20, 013006. [Google Scholar] [CrossRef]
- Tóth, G.; Knapp, C.; Gühne, O.; Briegel, H.J. Optimal spin squeezing inequalities detect bound entanglement in spin models. Phys. Rev. Lett. 2007, 99, 250405. [Google Scholar] [CrossRef]
- Akkermans, E.; Gero, A.; Kaiser, R. Photon localization and Dicke superradiance in atomic gases. Phys. Rev. Lett. 2008, 101, 103602. [Google Scholar] [CrossRef]
- Bellando, L.; Gero, A.; Akkermans, E.; Kaiser, R. Cooperative effects and disorder: A scaling analysis of the spectrum of the effective atomic Hamiltonian. Phys. Rev. A 2014, 90, 063822. [Google Scholar] [CrossRef]
- Ellinger, K.; Cooper, J.; Zoller, P. Light-pressure force in N-atom systems. Phys. Rev. A 1994, 49, 3909. [Google Scholar] [CrossRef] [PubMed]
- Bienaimé, T.; Petruzzo, M.; Bigerni, D.; Piovella, N.; Kaiser, R. Atom and photon measurement in cooperative scattering by cold atoms. J. Mod. Opt. 2011, 58, 1942–1950. [Google Scholar] [CrossRef]
- Scully, M.O.; Svidzinsky, A.A. The Lamb Shift—Yesterday, Today, and Tomorrow. Science 2010, 328, 1239–1241. [Google Scholar] [CrossRef] [PubMed]
- Kitagawa, M.; Ueda, M. Squeezed spin states. Phys. Rev. A 1993, 47, 5138–5143. [Google Scholar] [CrossRef] [PubMed]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Piovella, N.; Olivares, S. Single-Photon Superradiance and Subradiance as Collective Emission from Symmetric and Anti-Symmetric States. Symmetry 2023, 15, 1817. https://doi.org/10.3390/sym15101817
Piovella N, Olivares S. Single-Photon Superradiance and Subradiance as Collective Emission from Symmetric and Anti-Symmetric States. Symmetry. 2023; 15(10):1817. https://doi.org/10.3390/sym15101817
Chicago/Turabian StylePiovella, Nicola, and Stefano Olivares. 2023. "Single-Photon Superradiance and Subradiance as Collective Emission from Symmetric and Anti-Symmetric States" Symmetry 15, no. 10: 1817. https://doi.org/10.3390/sym15101817
APA StylePiovella, N., & Olivares, S. (2023). Single-Photon Superradiance and Subradiance as Collective Emission from Symmetric and Anti-Symmetric States. Symmetry, 15(10), 1817. https://doi.org/10.3390/sym15101817







