Islands of Shape Coexistence: Theoretical Predictions and Experimental Evidence
Abstract
1. Introduction
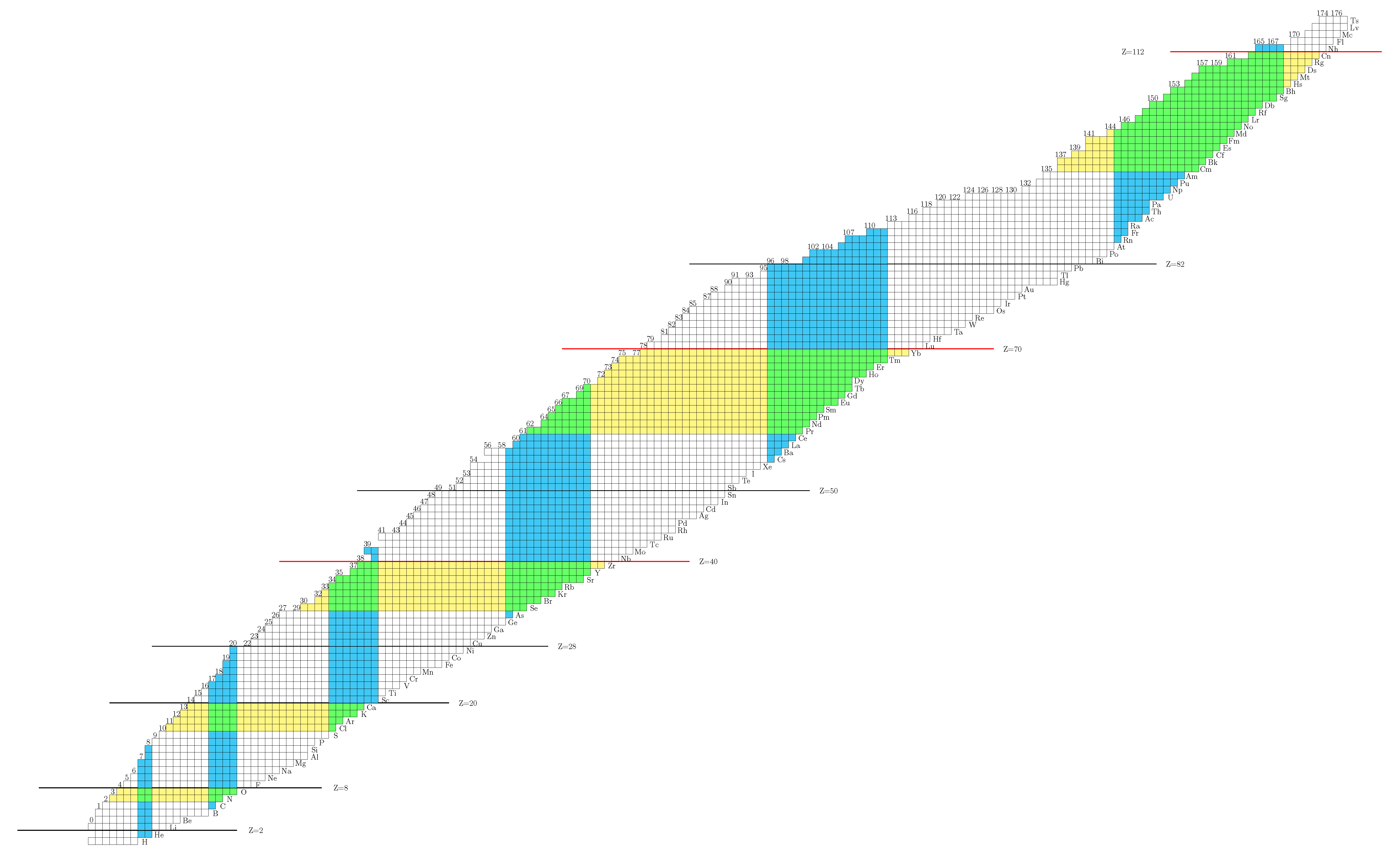
2. Harmonic Oscillator (HO) and Spin-Orbit (SO) Magic Numbers
3. The Dual-Shell Mechanism
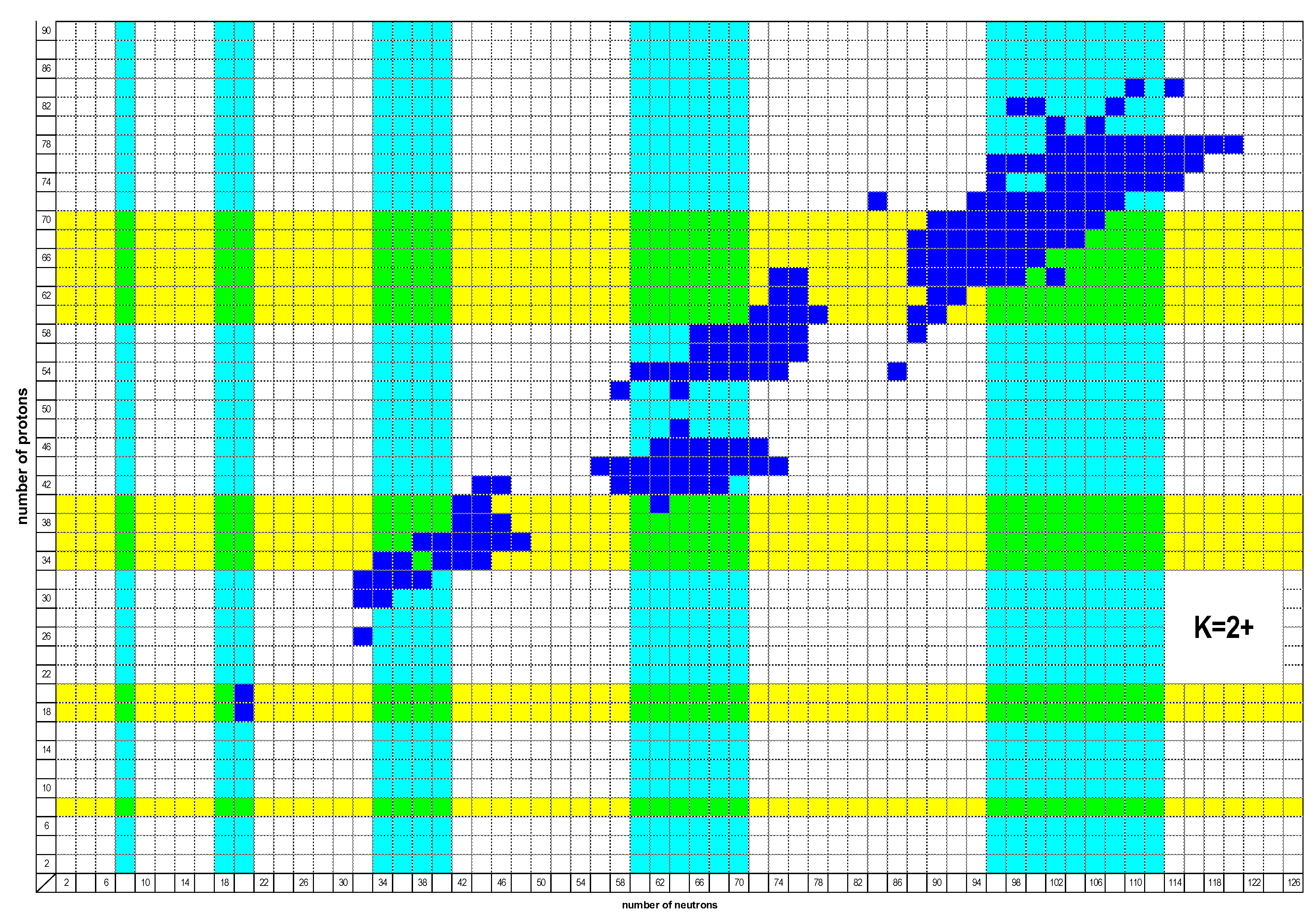
4. Experimental Evidence for the Shape Coexistence (SC) Magic Numbers
5. Islands of Shape Coexistence in Covariant Density Functional Theory
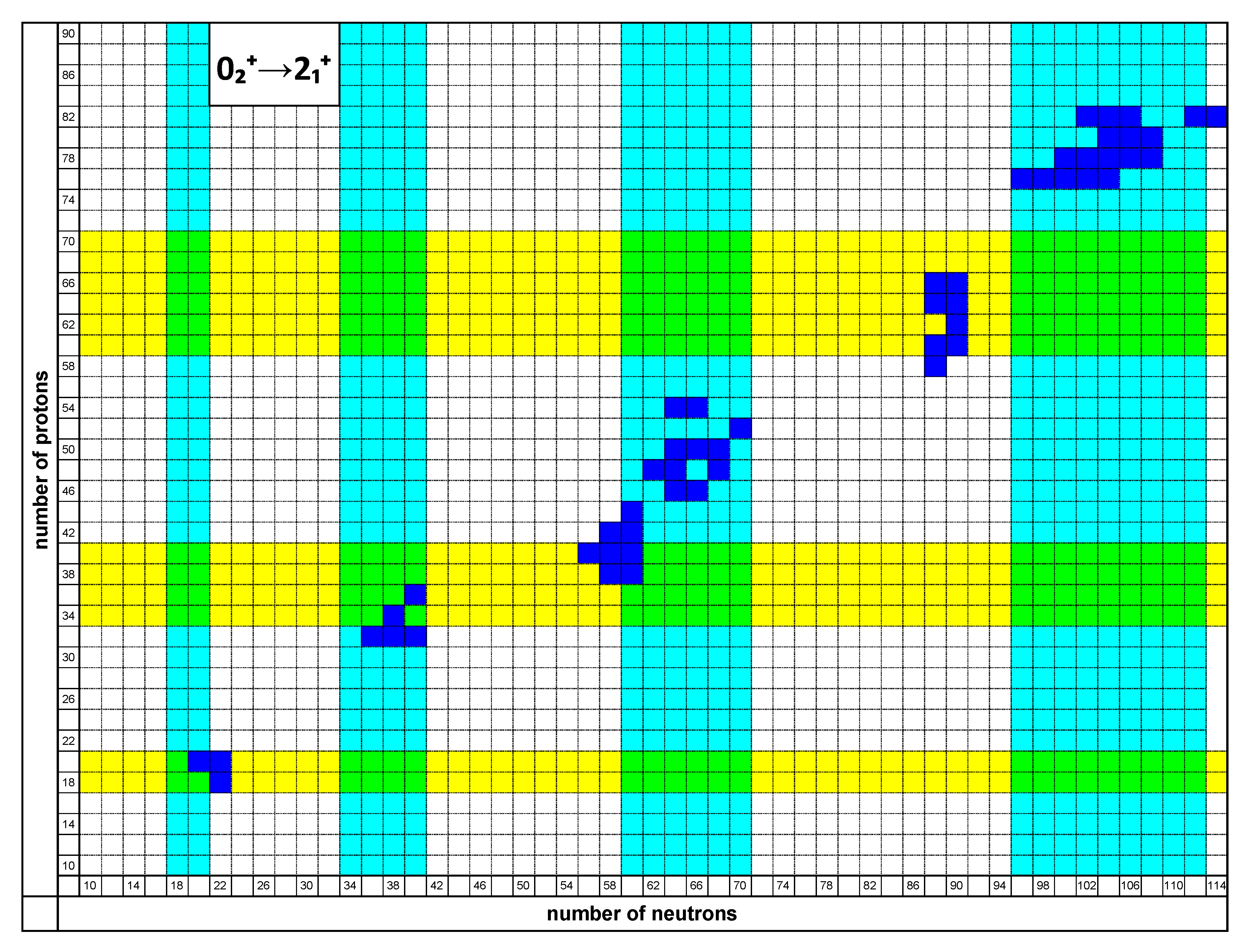
6. Islands of and Bands
7. Energy Differences of and
8. A Mechanism for Multiple Shape Coexistence
9. Discussion
10. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Morinaga, H. Interpretation of some of the excited states of 4n self-conjugate nuclei. Phys. Rev. 1956, 101, 254. [Google Scholar] [CrossRef]
- Heyde, K.; Van Isacker, P.; Waroquier, M.; Wood, J.L.; Meyer, R.A. Coexistence in odd-mass nuclei. Phys. Rep. 1983, 102, 291. [Google Scholar] [CrossRef]
- Wood, J.L.; Heyde, K.; Nazarewicz, W.; Huyse, M.; Van Duppen, P. Coexistence in even-mass nuclei. Phys. Rep. 1992, 215, 101. [Google Scholar] [CrossRef]
- Heyde, K.; Wood, J.L. Shape coexistence in atomic nuclei. Rev. Mod. Phys. 2011, 83, 1467. [Google Scholar] [CrossRef]
- Garrett, P.E.; Zielińska, M.; Clément, E. An experimental view on shape coexistence in nuclei. Prog. Part. Nucl. Phys. 2022, 124, 103931. [Google Scholar] [CrossRef]
- Bonatsos, D.; Assimakis, I.E.; Minkov, N.; Martinou, A.; Cakirli, R.B.; Casten, R.F.; Blaum, K. Proxy-SU(3) symmetry in heavy deformed nuclei. Phys. Rev. C 2017, 95, 064325. [Google Scholar] [CrossRef]
- Bonatsos, D.; Assimakis, I.E.; Minkov, N.; Martinou, A.; Sarantopoulou, S.; Cakirli, R.B.; Casten, R.F.; Blaum, K. Analytic predictions for nuclear shapes, prolate dominance, and the prolate-oblate shape transition in the proxy-SU(3) model. Phys. Rev. C 2017, 95, 064326. [Google Scholar] [CrossRef]
- Martinou, A.; Bonatsos, D.; Metzimekis, T.J.; Karakatsanis, K.E.; Assimakis, I.E.; Peroulis, S.K.; Sarantopoulou, S.; Minkov, N. The islands of shape coexistence within the Elliott and the proxy-SU(3) models. Eur. Phys. J. A 2021, 57, 84. [Google Scholar] [CrossRef]
- Martinou, A. A mechanism for shape coexistence. EPJ Web Conf. 2021, 252, 02005. [Google Scholar] [CrossRef]
- Bonatsos, D.; Karakatsanis, K.E.; Martinou, A.; Mertzimekis, T.J.; Minkov, N. Microscopic origin of shape coexistence in the N=90, Z=64 region. Phys. Lett. B 2022, 829, 137099. [Google Scholar] [CrossRef]
- Bonatsos, D.; Karakatsanis, K.E.; Martinou, A.; Mertzimekis, T.J.; Minkov, N. Islands of shape coexistence from single-particle spectra in covariant density functional theory. Phys. Rev. C 2022, 106, 044323. [Google Scholar] [CrossRef]
- ENSDF Database. Available online: https://www.nndc.bnl.gov/ensdf (accessed on 30 December 2020).
- Garrett, P.E.; Rodríguez, T.R.; Diaz Varela, A.; Green, K.L.; Bangay, J.; Finlay, A.; Austin, R.A.E.; Ball, G.C.; Bandyopadhyay, D.S.; Bildstein, V.; et al. Multiple Shape Coexistence in 110,112Cd. Phys. Rev. Lett. 2019, 123, 142502. [Google Scholar] [CrossRef] [PubMed]
- Garrett, P.E.; Rodríguez, T.R.; Diaz Varela, A.; Green, K.L.; Bangay, J.; Finlay, A.; Austin, R.A.E.; Ball, G.C.; Bandyopadhyay, D.S.; Bildstein, V.; et al. Shape coexistence and multiparticle-multihole structures in 110,112Cd. Phys. Rev. C 2020, 101, 044302. [Google Scholar] [CrossRef]
- Mayer, M.G. On closed shells in nuclei. II. Phys. Rev. 1949, 75, 1969. [Google Scholar] [CrossRef]
- Mayer, M.G.; Jensen, J.H.D. Elementary Theory of Nuclear Shell Structure; Wiley: New York, NY, USA, 1955. [Google Scholar]
- Wybourne, B.G. Classical Groups for Physicists; Wiley: New York, NY, USA, 1974. [Google Scholar]
- Moshinsky, M.; Smirnov, Y.F. The Harmonic Oscillator in Modern Physics; Harwood: Amsterdam, The Netherlands, 1996. [Google Scholar]
- Iachello, F. Lie Algebras and Applications; Springer: Berlin, Germany, 2006. [Google Scholar]
- Sorlin, O.; Porquet, M.-G. Nuclear magic numbers: New features far from stability. Prog. Part. Nucl. Phys. 2008, 61, 602. [Google Scholar] [CrossRef]
- Martinou, A.; Bonatsos, D.; Minkov, N.; Assimakis, I.E.; Peroulis, S.K.; Sarantopoulou, S. Proxy-SU(3) symmetry in the shell model basis. J. Cseh. Eur. Phys. J. A 2020, 56, 239. [Google Scholar] [CrossRef]
- Nilsson, S.G. Binding states of individual nucleons in strongly deformed nuclei. Mat. Fys. Medd. K. Dan. Vidensk. Selsk. 1955, 29, 16. [Google Scholar]
- Nilsson, S.G.; Ragnarsson, I. Shapes and Shells in Nuclear Structure; Cambridge University Press: Cambridge, MA, USA, 1995. [Google Scholar]
- Lederer, C.M.; Shirley, V.S. (Eds.) Table of Isotopes, 7th ed.; Wiley: New York, NY, USA, 1978. [Google Scholar]
- Otsuka, T.; Gade, A.; Sorlin, O.; Suzuki, T.; Utsuno, Y. Evolution of shell structure in exotic nuclei. Rev. Mod. Phys. 2020, 92, 015002. [Google Scholar] [CrossRef]
- Elliott, J.P. Collective motion in the nuclear shell model. I. Classification schemes for states of mixed configurations. Proc. Roy. Soc. Lond. Ser. A 1958, 245, 128. [Google Scholar]
- Elliott, J.P. Collective motion in the nuclear shell model II. The introduction of intrinsic wave-functions. Proc. Roy. Soc. Lond. Ser. A 1958, 245, 562. [Google Scholar]
- Elliott, J.P.; Harvey, M. Collective motion in the nuclear shell model III. The calculation of spectra. Proc. Roy. Soc. Lond. Ser. A 1963, 272, 557. [Google Scholar]
- Elliott, J.P.; Wilsdon, C.E. Collective motion in the nuclear shell model IV. Odd-mass nuclei in the sd shell. Proc. Roy. Soc. Lond. Ser. A 1968, 302, 509. [Google Scholar]
- Bonatsos, D.; Sobhani, H.; Hassanabadi, H. Shell model structure of proxy-SU(3) pairs of orbitals. Eur. Phys. J. Plus 2020, 135, 710. [Google Scholar] [CrossRef]
- Kota, V.K.B. SU(3) Symmetry in Atomic Nuclei; Springer: Singapore, 2020. [Google Scholar]
- Martinou, A.; Bonatsos, D.; Karakatsanis, K.E.; Sarantopoulou, S.; Assimakis, I.E.; Peroulis, S.K.; Minkov, N. Why nuclear forces favor the highest weight irreducible representations of the fermionic SU(3) symmetry. Eur. Phys. J. A 2021, 57, 83. [Google Scholar] [CrossRef]
- Castaños, O.; Draayer, J.P.; Leschber, Y. Shape variables and the shell model. Z. Phys. A 1988, 329, 33. [Google Scholar]
- Cakirli, R.B.; Casten, R.F. Direct empirical correlation between proton-neutron interaction strengths and the growth of collectivity in nuclei. Phys. Rev. Lett. 2006, 96, 132501. [Google Scholar] [CrossRef]
- Cakirli, R.B.; Blaum, K.; Casten, R.F. Indication of a mini-valence Wigner-like energy in heavy nuclei. Phys. Rev. C 2010, 82, 061304(R). [Google Scholar] [CrossRef]
- Bonatsos, D.; Karampagia, S.; Cakirli, R.B.; Casten, R.F.; Blaum, K.; Amon Susam, L. Emergent collectivity in nuclei and enhanced proton-neutron interactions. Phys. Rev. C 2013, 88, 054309. [Google Scholar] [CrossRef]
- Sheline, R.K. Vibrational states in deformed even-even nuclei. Rev. Mod. Phys. 1960, 32, 1. [Google Scholar] [CrossRef]
- Zamfir, N.V.; Bucurescu, D.; Casten, R.F.; Ivasvu, M. A simple phenomenology for states. Phys. Lett. B 1990, 241, 463. [Google Scholar] [CrossRef]
- Ring, P. Relativistic mean field theory in finite nuclei. Prog. Part. Nucl. Phys. 1996, 37, 193. [Google Scholar] [CrossRef]
- Bender, M.; Heenen, P.-H.; Reinhard, P.-G. Self-consistent mean-field models for nuclear structure. Rev. Mod. Phys. 2003, 75, 121. [Google Scholar] [CrossRef]
- Vretenar, D.; Afanasjev, A.V.; Lalazissis, G.A.; Ring, P. Relativistic Hartree–Bogoliubov theory: Static and dynamic aspects of exotic nuclear structure. Phys. Rep. 2005, 409, 101. [Google Scholar] [CrossRef]
- Meng, J.; Toki, H.; Zhou, S.G.; Zhang, S.Q.; Long, W.H.; Geng, L.S. Relativistic continuum Hartree Bogoliubov theory for ground-state properties of exotic nuclei. Prog. Part. Nucl. Phys. 2006, 57, 470. [Google Scholar] [CrossRef]
- Nikšić, T.; Vretenar, D.; Ring, P. Relativistic nuclear energy density functionals: Mean-field and beyond. Prog. Part. Nucl. Phys. 2011, 66, 519. [Google Scholar] [CrossRef]
- Meng, J.; Zhou, S.G. Halos in medium-heavy and heavy nuclei with covariant density functional theory in continuum. J. Phys. G Nucl. Part. Phys. 2015, 42, 093101. [Google Scholar] [CrossRef]
- Liang, H.; Meng, J.; Zhou, S.-G. Hidden pseudospin and spin symmetries and their origins in atomic nuclei. Phys. Rep. 2015, 570, 1. [Google Scholar] [CrossRef]
- Lalazissis, G.A.; Nikšić, T.; Paar, N.; Vretenar, D.; Ring, P. New relativistic mean-field interaction with density-dependent meson-nucleon couplings. Phys. Rev. C 2005, 71, 024312. [Google Scholar] [CrossRef]
- Nikšić, T.; Paar, N.; Vretenar, D.; Ring, P. DIRHB—A relativistic self-consistent mean-field framework for atomic nuclei. Comp. Phys. Commun. 2014, 185, 1808. [Google Scholar] [CrossRef]
- Tian, Y.; Ma, Z.Y.; Ring, P. A finite range pairing force for density functional theory in superfluid nuclei. Phys. Lett. B 2009, 676, 44. [Google Scholar] [CrossRef]
- Tian, Y.; Ma, Z.-Y.; Ring, P. Axially deformed relativistic Hartree Bogoliubov theory with a separable pairing force. Phys. Rev. C 2009, 80, 024313. [Google Scholar] [CrossRef]
- Garrett, P.E. Characterization of the β vibration and states in deformed nuclei. J. Phys. G: Nucl. Part. Phys. 2001, 27, R1. [Google Scholar] [CrossRef]
- Sharpey-Schafer, J.F.; Mullins, S.M.; Bark, R.A.; Kau, J.; Komati, F.; Lawrie, E.A.; Lawrie, J.J.; Madiba, T.E.; Maine, P.; Minkova, A.; et al. Congruent band structures in 154Gd: Configuration-dependent pairing, a double vacuum and lack of β-vibrations. Eur. Phys. J. A 2011, 47, 5. [Google Scholar] [CrossRef]
- Sharpey-Schafer, J.F.; Madiba, T.E.; Bvumbi, S.P.; Lawrie, E.A.; Lawrie, J.J.; Minkova, A.; Mullins, S.M.; Papka, P.; Roux, D.G.; Timár, G. Blocking of coupling to the excitation in 154Gd by the [505]11/2- neutron in 155Gd. J. Eur. Phys. J. A 2011, 47, 6. [Google Scholar] [CrossRef]
- Sharpey-Schafer, J.F.; Bark, R.A.; Bvumbi, S.P.; Dinoko, T.R.S.; Majola, S.N.T. “Stiff” deformed nuclei, configuration dependent pairing and the β and γ degrees of freedom. Eur. Phys. J. A 2019, 55, 15. [Google Scholar] [CrossRef]
- Garrett, P.E.; Green, K.L.; Austin, R.A.E.; Ball, G.C.; Bandyopadhyay, D.S.; Colosimo, S.; Cross, D.S.; Demand, G.A.; Grinyer, G.F.; Hackman, G.; et al. Using β-decay to map the E2 strength in the Cd isotopes and the downfall of vibrational motion. Acta Phys. Pol. B 2011, 42, 799. [Google Scholar] [CrossRef]
- McCutchan, E.A.; Bonatsos, D.; Zamfir, N.V.; Casten, R.F. Staggering in γ-band energies and the transition between different structural symmetries in nuclei. Phys. Rev. C 2007, 76, 024306. [Google Scholar] [CrossRef]
- Draayer, J.P.; Weeks, K.J. Towards a shell model description of the low-energy structure of deformed nuclei I. Even-even systems. Ann. Phys. (N. Y.) 1984, 156, 41. [Google Scholar] [CrossRef]


| nuc. | L | nuc. | L | nuc. | L | nuc. | L | nuc. | L | nuc. | L | nuc. | L |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ar | 16 | Zr | 12 | Xe | 4 | Gd | 2 | Yb | 10 | W | 12 | Pt | 6 |
| Ar | 12 | Mo | 2 | Xe | 4 | Dy | 12 | Yb | 4 | W | 22 | Pt | 8 |
| Ca | 8 | Mo | 2 | Ce | 4 | Dy | 10 | Yb | 18 | W | 4 | Pt | 6 |
| Ca | 12 | Ru | 4 | Ce | 2 | Dy | 8 | Yb | 14 | W | 4 | Pt | 6 |
| Ti | 4 | Pd | 6 | Ce | 6 | Dy | 4 | Yb | 4 | W | 4 | Pt | 2 |
| Ge | 10 | Pd | 4 | Nd | 8 | Dy | 28 | Yb | 2 | Os | 8 | Hg | 20 |
| Ge | 8 | Cd | 6 | Nd | 6 | Dy | 2 | Yb | 4 | Os | 6 | Hg | 28 |
| Ge | 6 | Cd | 8 | Nd | 4 | Er | 22 | Hf | 4 | Os | 6 | Hg | 22 |
| Ge | 6 | Cd | 2 | Sm | 16 | Er | 4 | Hf | 4 | Os | 6 | Hg | 6 |
| Se | 2 | Sn | 30 | Sm | 6 | Er | 2 | Hf | 4 | Os | 6 | Pb | 8 |
| Kr | 2 | Sn | 14 | Gd | 10 | Er | 2 | Hf | 26 | Os | 6 | Pb | 14 |
| Sr | 18 | Sn | 12 | Gd | 10 | Er | 10 | Hf | 10 | Os | 10 | Pb | 14 |
| Sr | 6 | Te | 18 | Gd | 14 | Er | 12 | Hf | 6 | Os | 2 | Pb | 6 |
| Zr | 6 | Xe | 4 | Gd | 6 | Er | 6 | Hf | 14 | Os | 2 | Pb | 8 |
| Zr | 12 | Xe | 4 | Gd | 4 | Er | 22 | W | 6 | Pt | 8 |
| nuc. | nuc. | nuc. | nuc. | nuc. | nuc. | nuc. | ||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ar | 8 | Zr | 6 | 21 | Pd | 8 | 13 | Ce | 8 | 7 | Dy | 8 | 7 | Hf | 2 | 5 | Os | 6 | 7 | |
| Ca | 13 | Mo | 2 | 5 | Pd | 4 | 5 | Nd | 4 | 3 | Dy | 22 | 25 | Hf | 6 | 5 | Os | 10 | 5 | |
| Fe | 4 | 3 | Mo | 2 | 5 | Cd | 10 | 11 | Nd | 44 | 3 | Dy | 18 | 17 | Hf | 4 | 3 | Os | 10 | 7 |
| Zn | 6 | 7 | Zr | 4 | 3 | Te | 6 | Nd | 4 | 5 | Dy | 14 | 9 | Hf | 6 | 5 | Pt | 4 | 9 | |
| Zn | 8 | Mo | 4 | 3 | Te | 6 | 5 | Nd | 6 | 5 | Dy | 4 | 5 | Hf | 4 | 5 | Pt | 6 | 7 | |
| Ge | 8 | Mo | 6 | Xe | 20 | Sm | 14 | Er | 14 | 15 | Hf | 8 | 7 | Pt | 6 | 7 | ||||
| Ge | 10 | Mo | 18 | 17 | Xe | 6 | Sm | 8 | 7 | Er | 10 | 7 | Hf | 14 | 15 | Pt | 10 | 9 | ||
| Ge | 4 | 7 | Mo | 18 | 17 | Xe | 14 | 11 | Gd | 22 | Er | 2 | 13 | Hf | 20 | 15 | Pt | 24 | 3 | |
| Ge | 8 | 5 | Mo | 12 | 11 | Xe | 28 | 5 | Gd | 8 | 7 | Er | 12 | 11 | W | 4 | 3 | Pt | 6 | 5 |
| Se | 14 | Mo | 4 | 5 | Xe | 14 | 15 | Xe | 13 | Er | 18 | 21 | W | 4 | 5 | Pt | 8 | 7 | ||
| Se | 14 | Ru | 8 | 9 | Xe | 10 | 21 | Ce | 4 | 5 | Er | 14 | 13 | W | 4 | 5 | Pt | 4 | 5 | |
| Se | 6 | 19 | Ru | 10 | 11 | Xe | 8 | 7 | Nd | 6 | 3 | Er | 12 | 7 | W | 6 | 7 | Pt | 8 | 5 |
| Se | 8 | 9 | Ru | 8 | 7 | Xe | 6 | 5 | Nd | 4 | 3 | Er | 18 | 19 | W | 8 | 5 | Pt | 8 | |
| Se | 10 | 9 | Ru | 8 | 7 | Ba | 4 | 11 | Sm | 12 | 11 | Er | 2 | 5 | W | 12 | 5 | Hg | 20 | |
| Kr | 7 | Ru | 10 | 13 | Ba | 10 | 11 | Sm | 6 | 7 | Yb | 2 | 3 | W | 12 | 5 | Hg | 2 | 7 | |
| Kr | 8 | 9 | Ru | 14 | 15 | Ba | 16 | 9 | Gd | 8 | 13 | Yb | 2 | 3 | W | 4 | Pb | 8 | ||
| Kr | 10 | 9 | Ru | 16 | 19 | Ba | 14 | 9 | Gd | 6 | 7 | Yb | 4 | 13 | Os | 6 | 5 | Pb | 12 | |
| Kr | 6 | 7 | Ru | 6 | 9 | Ba | 10 | 5 | Gd | 12 | 15 | Yb | 12 | 13 | Os | 4 | 5 | Pb | 8 | |
| Kr | 4 | 5 | Ru | 6 | 5 | Ba | 12 | 7 | Gd | 6 | 5 | Yb | 6 | 29 | Os | 4 | 5 | Po | 6 | |
| Kr | 10 | Ru | 4 | 3 | Ce | 32 | Gd | 12 | 7 | Yb | 16 | 17 | Os | 4 | 5 | Po | 4 | |||
| Sr | 10 | 21 | Pd | 10 | 7 | Ce | 4 | 3 | Gd | 4 | 3 | Yb | 4 | 5 | Os | 6 | 9 | |||
| Sr | 20 | 9 | Pd | 8 | 5 | Ce | 18 | 9 | Gd | 4 | 5 | Yb | 4 | 5 | Os | 6 | 7 | |||
| Sr | 4 | 9 | Pd | 10 | 13 | Ce | 26 | Dy | 6 | 7 | Yb | 4 | 5 | Os | 6 | 5 | ||||
| Zr | 13 | Pd | 10 | 13 | Ce | 14 | 5 | Dy | 12 | 15 | Hf | 6 | Os | 12 | 13 |
| nuc. | nuc. | nuc. | nuc. | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ar | 1970.4 | 4329.1 | Sn | 1229.7 | 1758.3 | Er | 344.5 | 930.1 | 930.5 | W | 111.2 | 1002.5 | 903.3 | ||
| Ar | 1460.8 | 2120.9 | Te | 564.1 | 1357.4 | Er | 192.2 | 806.4 | 820.1 | W | 122.6 | 883.6 | 738.0 | ||
| Ca | 5248.8 | 3352.6 | Xe | 337.3 | 830.4 | 928.1 | Er | 125.8 | 893.6 | 854.4 | Os | 227.8 | 758.3 | 918.8 | |
| Ca | 1524.7 | 1837.3 | Xe | 322.6 | 908.7 | 876.1 | Er | 102.0 | 1087.2 | 900.7 | Os | 158.6 | 545.3 | 846.2 | |
| Ti | 1083.1 | 1904.3 | Xe | 354.0 | 1268.9 | 846.5 | Er | 91.4 | 1246.0 | 860.3 | Os | 135.1 | 601.2 | 863.6 | |
| Ge | 1015.8 | 1754.5 | 1777.4 | Xe | 388.6 | 1313.9 | 879.9 | Er | 80.6 | 1460.0 | 785.9 | Os | 132.2 | 650.5 | 864.4 |
| Ge | 1039.5 | 1215.6 | 1707.7 | Ce | 325.3 | 1158.4 | 822.2 | Er | 79.8 | 1217.2 | 821.2 | Os | 132.1 | 736.4 | 870.4 |
| Ge | 834.0 | 691.4 | Ce | 409.2 | 1533.5 | 965.7 | Er | 78.6 | 890.9 | 934.0 | Os | 119.8 | 1042.0 | 942.9 | |
| Ge | 1348.3 | 2333.6 | Ce | 258.5 | 1043.2 | 1381.9 | Yb | 102.4 | 1043.0 | 932.4 | Os | 137.2 | 1061.0 | 767.5 | |
| Se | 862.1 | 937.2 | Nd | 301.7 | 916.9 | 1248.9 | Yb | 87.7 | 1155.2 | 984.0 | Os | 186.7 | 911.8 | 558.0 | |
| Kr | 424.0 | 769.9 | 1221.7 | Nd | 130.2 | 675.9 | 1062.1 | Yb | 84.3 | 1069.4 | 1145.7 | Os | 205.8 | 956.5 | 489.1 |
| Sr | 814.9 | 1229.3 | Nd | 72.4 | 1139.0 | Yb | 78.7 | 1042.9 | 1465.9 | Pt | 170.3 | 421.0 | |||
| Sr | 144.2 | 215.6 | Sm | 121.8 | 684.8 | 1085.8 | Yb | 76.5 | 1487.1 | 1634.0 | Pt | 153.2 | 478.1 | 677.5 | |
| Zr | 1750.5 | 1581.6 | Sm | 82.0 | 1099.3 | 1440.0 | Yb | 82.1 | 1139.0 | 1260.9 | Pt | 155.0 | 499.7 | 667.8 | |
| Zr | 1222.9 | 854.0 | Gd | 344.3 | 615.4 | 1109.2 | Yb | 84.0 | 1315.0 | Pt | 163.0 | 491.8 | 648.7 | ||
| Zr | 212.5 | 331.1 | Gd | 123.1 | 680.7 | 996.3 | Hf | 124.1 | 942.1 | 875.9 | Pt | 191.5 | 471.5 | 607.2 | |
| Mo | 535.6 | 695.1 | 1063.8 | Gd | 89.0 | 1049.5 | 1154.2 | Hf | 100.8 | 879.6 | 961.3 | Pt | 355.7 | 1135.3 | 688.7 |
| Mo | 296.6 | 698.3 | 847.9 | Gd | 79.5 | 1196.2 | 1187.1 | Hf | 95.2 | 871.3 | 1075.3 | Hg | 366.8 | 375.1 | |
| Ru | 358.0 | 988.3 | 893.1 | Gd | 75.3 | 1325.7 | 988.4 | Hf | 91.0 | 828.1 | 1226.8 | Hg | 405.3 | 523.0 | 1096.6 |
| Pd | 373.8 | 946.7 | 931.2 | Gd | 71.6 | 1427.0 | 864.0 | Hf | 88.3 | 1149.9 | 1341.3 | Hg | 412.8 | 824.5 | |
| Pd | 348.7 | 1125.5 | 813.6 | Dy | 334.3 | 660.6 | 1027.0 | Hf | 93.2 | 1199.4 | 1174.6 | Hg | 416.3 | 1278.6 | |
| Cd | 657.8 | 1473.1 | Dy | 137.8 | 675.6 | 890.5 | Hf | 93.3 | 1101.9 | 1199.7 | Pb | 570.0 | |||
| Cd | 617.5 | 1224.3 | 1312.4 | Dy | 98.9 | 990.5 | 946.3 | W | 156.7 | 937.1 | Pb | 655.0 | |||
| Cd | 513.5 | 1282.6 | Dy | 86.8 | 1279.9 | 966.2 | W | 108.3 | 843.3 | 1040.2 | Pb | 723.6 | 591.0 | ||
| Sn | 1299.9 | 1953.3 | Dy | 80.7 | 1400.3 | 888.2 | W | 105.9 | 997.0 | 1110.4 | Pb | 965.1 | 930.7 | ||
| Sn | 1293.6 | 1756.9 | Dy | 76.6 | 1149.0 | 857.2 | W | 100.1 | 1135.8 | 1221.4 | Pb | 1049.2 | 1142.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martinou, A.; Bonatsos, D.; Peroulis, S.K.; Karakatsanis, K.E.; Mertzimekis, T.J.; Minkov, N. Islands of Shape Coexistence: Theoretical Predictions and Experimental Evidence. Symmetry 2023, 15, 29. https://doi.org/10.3390/sym15010029
Martinou A, Bonatsos D, Peroulis SK, Karakatsanis KE, Mertzimekis TJ, Minkov N. Islands of Shape Coexistence: Theoretical Predictions and Experimental Evidence. Symmetry. 2023; 15(1):29. https://doi.org/10.3390/sym15010029
Chicago/Turabian StyleMartinou, Andriana, Dennis Bonatsos, Spyridon Kosmas Peroulis, Konstantinos Eleftherios Karakatsanis, Theodoros John Mertzimekis, and Nikolay Minkov. 2023. "Islands of Shape Coexistence: Theoretical Predictions and Experimental Evidence" Symmetry 15, no. 1: 29. https://doi.org/10.3390/sym15010029
APA StyleMartinou, A., Bonatsos, D., Peroulis, S. K., Karakatsanis, K. E., Mertzimekis, T. J., & Minkov, N. (2023). Islands of Shape Coexistence: Theoretical Predictions and Experimental Evidence. Symmetry, 15(1), 29. https://doi.org/10.3390/sym15010029








