Soliton Waves with the (3+1)-Dimensional Kadomtsev–Petviashvili–Boussinesq Equation in Water Wave Dynamics
Abstract
1. Introduction
2. Mathematical Analysis and Obtaining a Nonlinear Ordinary Differential Form
3. Quick View of the Methods and Implementation of the KP-B Equation
3.1. Modified Extended tanh Function Method
3.2. Kudryashov Method
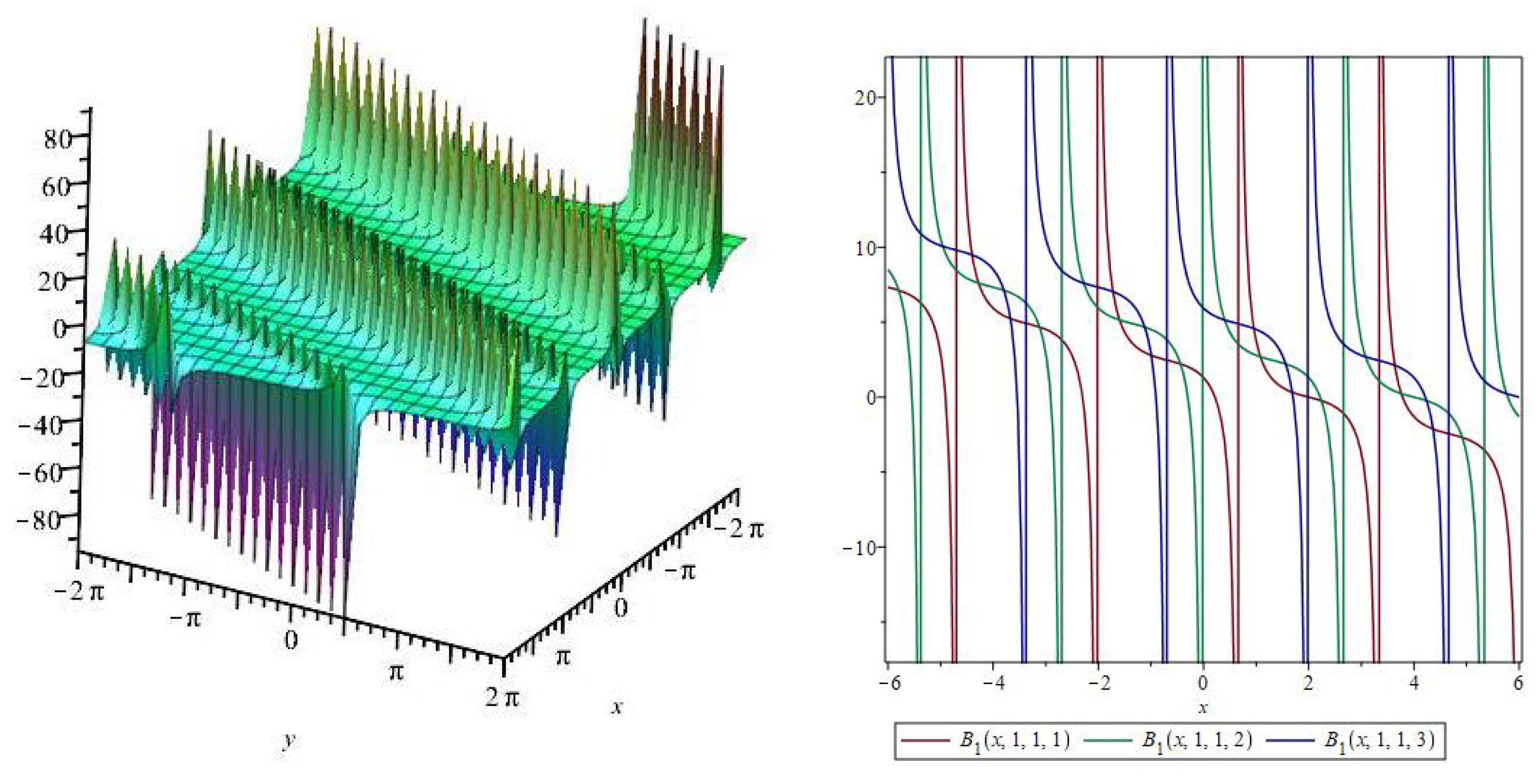
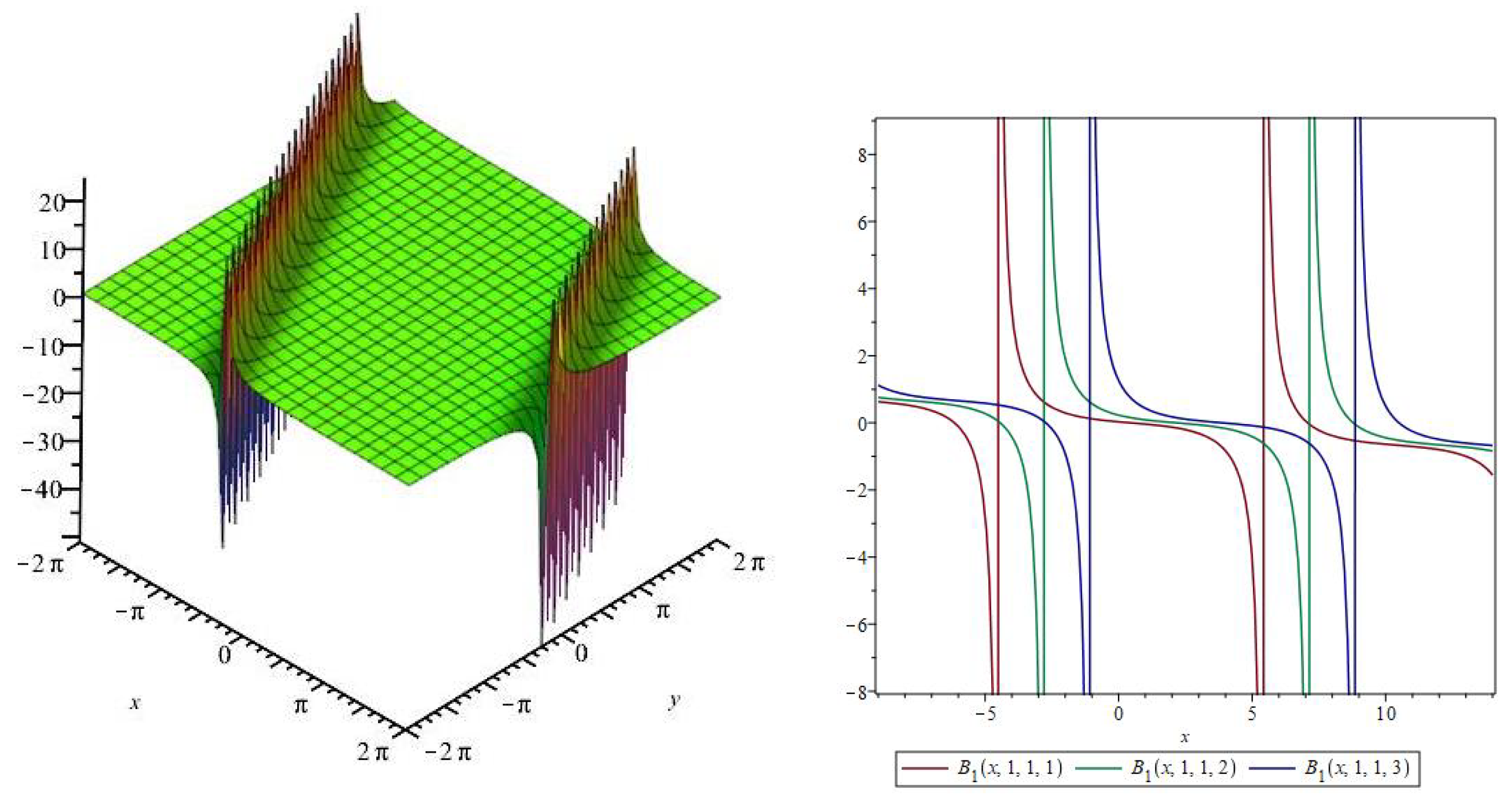
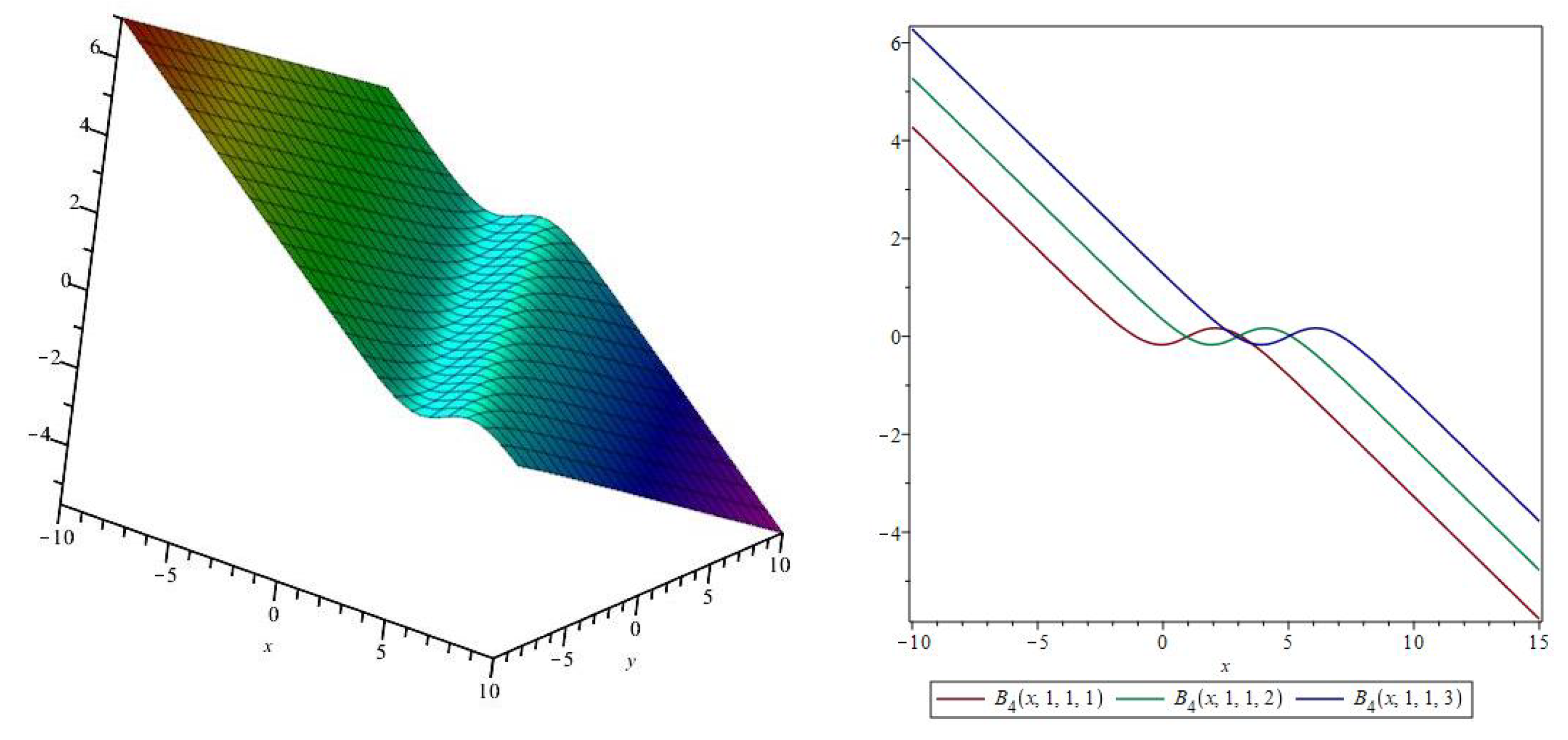
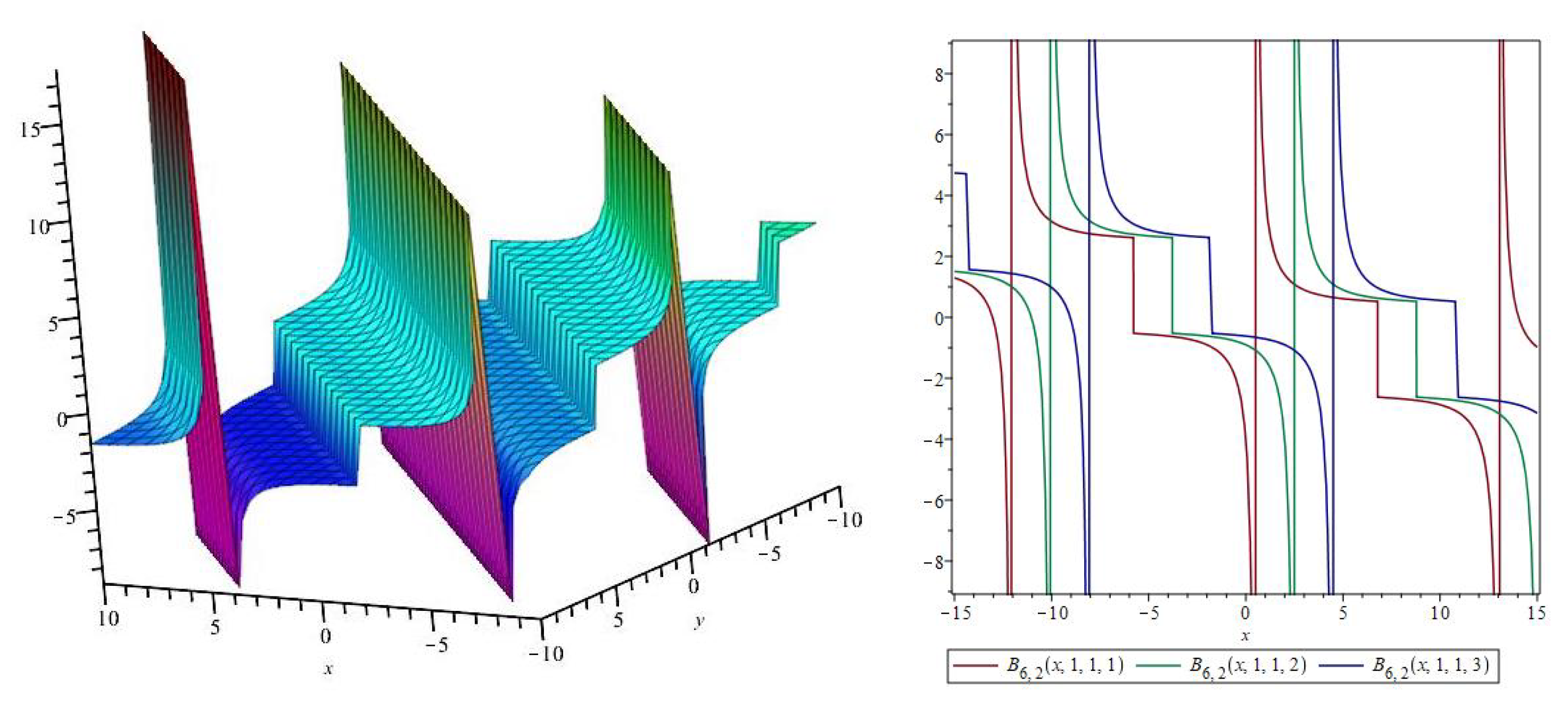
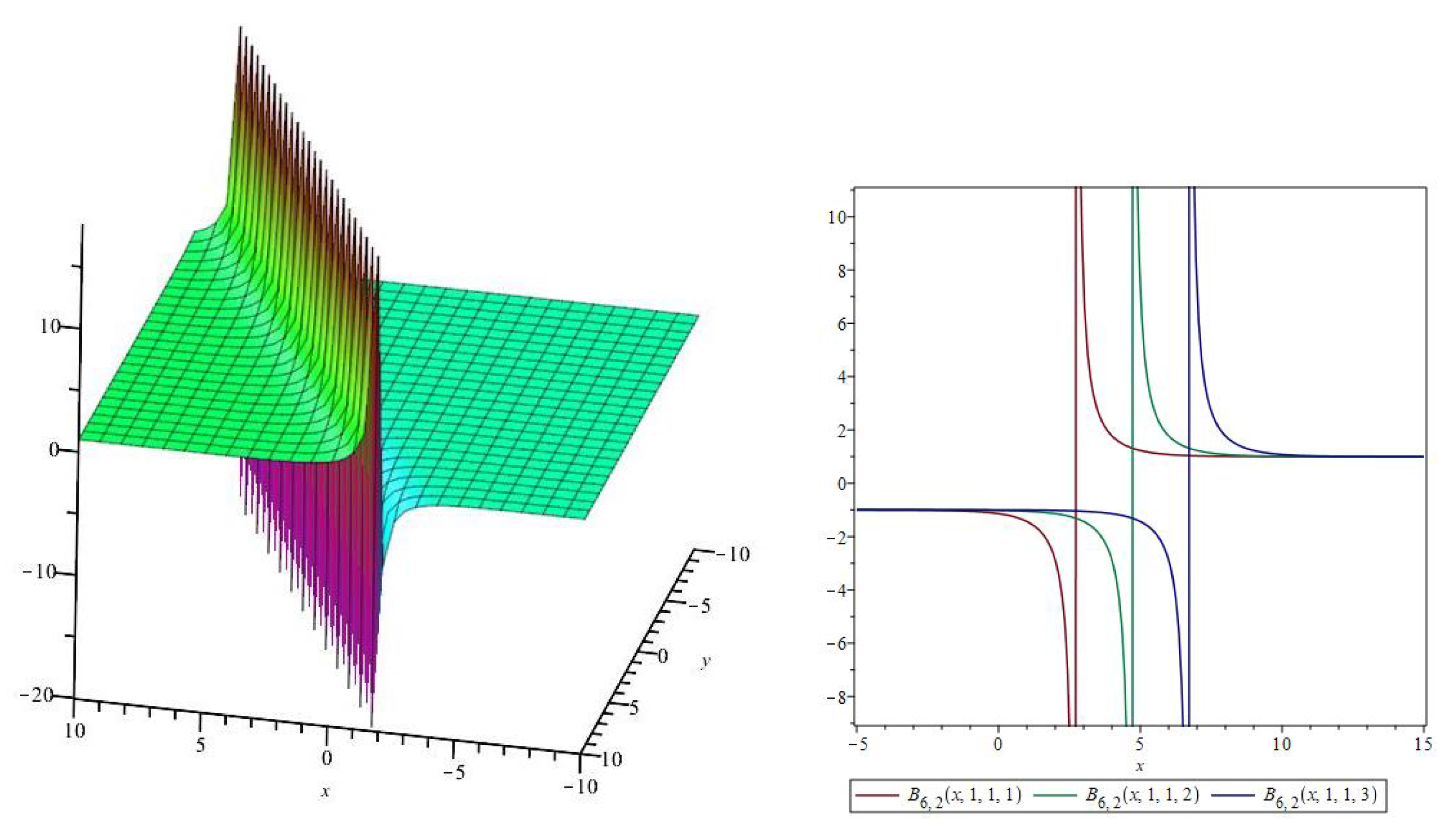
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Wazwaz, A.M. Partial Differential Equations and Solitary Waves Theory; Springer: Berlin/Heidelberg, Germnay, 2009. [Google Scholar] [CrossRef]
- Moroşanu, G. Nonlinear Evolution Equations and Applications; Mathematics and its Applications (East European Series); D. Reidel Publishing Co.: Dordrecht, The Netherlands, 1988; Volume 26, p. xii+340. [Google Scholar]
- Ablowitz, M.J.; Barone, V.; Lillo, S.D.; Sommacal, M. Traveling Waves in Elastic Rods with Arbitrary Curvature and Torsion. J. Nonlinear Sci. 2012, 22, 1013–1040. [Google Scholar] [CrossRef]
- Paul, G.C.; Eti, F.Z.; Kumar, D. Dynamical analysis of lump, lump-triangular periodic, predictable rogue and breather wave solutions to the (3+1)-dimensional gKP–Boussinesq equation. Results Phys. 2020, 19, 103525. [Google Scholar] [CrossRef]
- Heimburg, T.; Jackson, A.D. On soliton propagation in biomembranes and nerves. Proc. Natl. Acad. Sci. USA 2005, 102, 9790–9795. [Google Scholar] [CrossRef] [PubMed]
- Wang, K.J. A new fractional nonlinear singular heat conduction model for the human head considering the effect of febrifuge. Eur. Phys. J. Plus 2020, 135, 871. [Google Scholar] [CrossRef]
- Cinar, M.; Onder, I.; Secer, A.; Yusuf, A.; Sulaiman, T.A.; Bayram, M.; Aydin, H. The analytical solutions of Zoomeron equation via extended rational sin-cos and sinh-cosh methods. Phys. Scr. 2021, 96, 094002. [Google Scholar] [CrossRef]
- Yusuf, A.; Sulaiman, T.A.; Khalil, E.; Bayram, M.; Ahmad, H. Construction of multi-wave complexiton solutions of the Kadomtsev-Petviashvili equation via two efficient analyzing techniques. Results Phys. 2021, 21, 103775. [Google Scholar] [CrossRef]
- Zahran, E.H.M. Traveling Wave Solutions of Nonlinear Evolution Equations via Modified exp-φξ-Expansion Method. J. Comput. Theor. Nanosci. 2015, 12, 5716–5724. [Google Scholar] [CrossRef]
- Wang, K.J.; Wang, K.L. Variational principles for fractal whitham–broer–kaup equations in shallow water. Fractals 2021, 29, 2150028. [Google Scholar] [CrossRef]
- Asjad, M.I.; Ullah, N.; Rehman, H.U.; Inc, M. Construction of optical solitons of magneto-optic waveguides with anti-cubic law nonlinearity. Opt. Quantum Electron. 2021, 53. [Google Scholar] [CrossRef]
- Al-Smadi, M.; Arqub, O.A.; Hadid, S. Approximate solutions of nonlinear fractional Kundu-Eckhaus and coupled fractional massive Thirring equations emerging in quantum field theory using conformable residual power series method. Phys. Scr. 2020, 95, 105205. [Google Scholar] [CrossRef]
- Baleanu, D.; Inc, M.; Yusuf, A.; Aliyu, A.I. Lie symmetry analysis, exact solutions and conservation laws for the time fractional Caudrey–Dodd–Gibbon–Sawada–Kotera equation. Commun. Nonlinear Sci. Numer. Simul. 2018, 59, 222–234. [Google Scholar] [CrossRef]
- Yépez-Martínez, H.; Rezazadeh, H.; Souleymanou, A.; Mukam, S.P.T.; Eslami, M.; Kuetche, V.K.; Bekir, A. The extended modified method applied to optical solitons solutions in birefringent fibers with weak nonlocal nonlinearity and four wave mixing. Chin. J. Phys. 2019, 58, 137–150. [Google Scholar] [CrossRef]
- Akbar, M.A.; Ali, N.H.M. The improved F-expansion method with Riccati equation and its applications in mathematical physics. Cogent Math. 2017, 4, 1282577. [Google Scholar] [CrossRef]
- Zedan, H. Applications of the New Compound Riccati Equations Rational Expansion Method and Fan’s Subequation Method for the Davey-Stewartson Equations. Bound. Value Probl. 2010, 2010, 915721. [Google Scholar] [CrossRef]
- Kudryashov, N.A. One method for finding exact solutions of nonlinear differential equations. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 2248–2253. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Logistic function as solution of many nonlinear differential equations. Appl. Math. Model. 2015, 39, 5733–5742. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Method for finding highly dispersive optical solitons of nonlinear differential equations. Optik 2020, 206, 163550. [Google Scholar] [CrossRef]
- Al-Nowehy, A.G. Generalized Kudryashov method and general Expa-function method for solving a higher order nonlinear Schrödinger equation. J. Space Explor. 2017, 6, 1–26. [Google Scholar]
- Zayed, E.M.; Alngar, M.E.; El-Horbaty, M.; Biswas, A.; Alshomrani, A.S.; Khan, S.; Ekici, M.; Triki, H. Optical solitons in fiber Bragg gratings having Kerr law of refractive index with extended Kudryashov’s method and new extended auxiliary equation approach. Chin. J. Phys. 2020, 66, 187–205. [Google Scholar] [CrossRef]
- Ghanbari, B.; Baleanu, D. New Solutions of Gardner’s Equation Using Two Analytical Methods. Front. Phys. 2019, 7. [Google Scholar] [CrossRef]
- Kaewta, S.; Sirisubtawee, S.; Khansai, N. Explicit Exact Solutions of the (2+1)-Dimensional Integro-Differential Jaulent–Miodek Evolution Equation Using the Reliable Methods. Int. J. Math. Math. Sci. 2020, 2020, 1–19. [Google Scholar] [CrossRef]
- Biswas, A.; Ekici, M.; Sonmezoglu, A.; Belic, M.R. Highly dispersive optical solitons with kerr law nonlinearity by extended Jacobi’s elliptic function expansion. Optik 2019, 183, 395–400. [Google Scholar] [CrossRef]
- Baskonus, H.M.; Ercan, M. Extraction Complex Properties of the Nonlinear Modified Alpha Equation. Fractal Fract. 2021, 5, 6. [Google Scholar] [CrossRef]
- Bulut, H.; Isik, H.A.; Sulaiman, T.A. On Some Complex Aspects of the (2+1)-dimensional Broer-Kaup-Kupershmidt System. ITM Web Conf. 2017, 13, 01019. [Google Scholar] [CrossRef]
- Ilie, M.; Biazar, J.; Ayati, Z. The first integral method for solving some conformable fractional differential equations. Opt. Quantum Electron. 2018, 50, 55. [Google Scholar] [CrossRef]
- Inc, M.; Aliyu, A.I.; Yusuf, A.; Baleanu, D.; Nuray, E. Complexiton and solitary wave solutions of the coupled nonlinear Maccari’s system using two integration schemes. Mod. Phys. Lett. B 2018, 32, 1850014. [Google Scholar] [CrossRef]
- Kundu, P.R.; Fahim, M.R.A.; Islam, M.E.; Akbar, M.A. The sine-Gordon expansion method for higher-dimensional NLEEs and parametric analysis. Heliyon 2021, 7, e06459. [Google Scholar] [CrossRef]
- Cattani, C.; Sulaiman, T.A.; Baskonus, H.M.; Bulut, H. Solitons in an inhomogeneous Murnaghan’s rod. Eur. Phys. J. Plus 2018, 133, 228. [Google Scholar] [CrossRef]
- Ozisik, M. On the optical soliton solution of the (1+1)- dimensional perturbed NLSE in optical nano-fibers. Optik 2022, 250, 168233. [Google Scholar] [CrossRef]
- Filiz, A.; Ekici, M.; Sonmezoglu, A. F-Expansion Method and New Exact Solutions of the Schrödinger-KdV Equation. Sci. World J. 2014, 2014, 1–14. [Google Scholar] [CrossRef]
- Li, L.; Duan, C.; Yu, F. An improved Hirota bilinear method and new application for a nonlocal integrable complex modified Korteweg-de Vries (MKdV) equation. Phys. Lett. A 2019, 383, 1578–1582. [Google Scholar] [CrossRef]
- Liang, Z.; Tang, X.; Lou, S. New nonlocal symmetries and conservation laws of the (1+1)-dimensional Sine-Gordon equation. J. Phys. Conf. Ser. 2014, 490, 012032. [Google Scholar] [CrossRef]
- Rasheed, N.M.; Al-Amr, M.O.; Az-Zo’bi, E.A.; Tashtoush, M.A.; Akinyemi, L. Stable Optical Solitons for the Higher-Order Non-Kerr NLSE via the Modified Simple Equation Method. Mathematics 2021, 9, 1986. [Google Scholar] [CrossRef]
- Jawad, A.J.M.; Petković, M.D.; Biswas, A. Modified simple equation method for nonlinear evolution equations. Appl. Math. Comput. 2010, 217, 869–877. [Google Scholar] [CrossRef]
- Triki, H.; Hayat, T.; Aldossary, O.; Biswas, A. 1-soliton solution of the three component system of Wu-Zhang equations. Hacet. J. Math. Stat. 2012, 41, 537–543. [Google Scholar]
- Ghanbari, B.; Inc, M.; Yusuf, A.; Baleanu, D.; Bayram, M. Families of exact solutions of Biswas-Milovic equation by an exponential rational function method. Tbil. Math. J. 2020, 13, 39–65. [Google Scholar] [CrossRef]
- Tripathy, A.; Sahoo, S. A novel analytical method for solving (2+1)- dimensional extended Calogero-Bogoyavlenskii-Schiff equation in plasma physics. J. Ocean Eng. Sci. 2021, 6, 405–409. [Google Scholar] [CrossRef]
- Gepreel, K.A.; Nofal, T.A.; Al-Asmari, A.A. Abundant travelling wave solutions for nonlinear Kawahara partial differential equation using extended trial equation method. Int. J. Comput. Math. 2018, 96, 1357–1376. [Google Scholar] [CrossRef]
- Zhu, S.D. The generalizing Riccati equation mapping method in non-linear evolution equation: Application to (2+1)-dimensional Boiti–Leon–Pempinelle equation. Chaos Solitons Fractals 2008, 37, 1335–1342. [Google Scholar] [CrossRef]
- Khalique, C.M.; Moleleki, L.D. A (3+1)-dimensional generalized BKP-Boussinesq equation: Lie group approach. Results Phys. 2019, 13, 102239. [Google Scholar] [CrossRef]
- Alotaibi, H. Explore Optical Solitary Wave Solutions of the kp Equation by Recent Approaches. Crystals 2022, 12, 159. [Google Scholar] [CrossRef]
- Alotaibi, H. Traveling Wave Solutions to the Nonlinear Evolution Equation Using Expansion Method and Addendum to Kudryashov’s Method. Symmetry 2021, 13, 2126. [Google Scholar] [CrossRef]
- Wang, K.J. Diverse soliton solutions to the Fokas system via the Cole-Hopf transformation. Optik 2023, 272, 170250. [Google Scholar] [CrossRef]
- Wang, K.J. A fast insight into the optical solitons of the generalized third-order nonlinear Schrödinger’s equation. Results Phys. 2022, 40, 105872. [Google Scholar] [CrossRef]
- Wazwaz, A.M.; El-Tantawy, S.A. Solving the (3+1)-dimensional KP-Boussinesq and BKP-Boussinesq equations by the simplified Hirota’s method. Nonlinear Dyn. 2017, 88, 3017–3021. [Google Scholar] [CrossRef]
- Yu, J.P.; Sun, Y.L. A direct Bäcklund transformation for a (3+1)-dimensional Kadomtsev–Petviashvili–Boussinesq-like equation. Nonlinear Dyn. 2017, 90, 2263–2268. [Google Scholar] [CrossRef]
- Wang, L.; Zhou, Y.; Liu, Q.; Zhang, Q. Traveling waves of the (3+1)-Dimensıonal Kadomtsev-Petviashvili-Boussinesq Equation. J. Appl. Anal. Comput. 2020, 10, 267–281. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Multiple-soliton solutions for a (3+1)-dimensional generalized KP equation. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 491–495. [Google Scholar] [CrossRef]
- Liu, J.G.; Tian, Y.; Zeng, Z.F. New exact periodic solitary-wave solutions for the new (3+1)-dimensional generalized Kadomtsev-Petviashvili equation in multi-temperature electron plasmas. AIP Adv. 2017, 7, 105013. [Google Scholar] [CrossRef]
- Baronio, F.; Onorato, M.; Chen, S.; Trillo, S.; Kodama, Y.; Wabnitz, S. Optical-fluid dark line and X solitary waves in Kerr media. Opt. Data Process. Storage 2017, 3, 1–7. [Google Scholar] [CrossRef]
- Klein, C.; Sparber, C.; Markowich, P. Numerical Study of Oscillatory Regimes in the Kadomtsev–Petviashvili Equation. J. Nonlinear Sci. 2007, 17, 429–470. [Google Scholar] [CrossRef]
- Seadawy, A.; El-Rashidy, K. Dispersive solitary wave solutions of Kadomtsev-Petviashvili and modified Kadomtsev-Petviashvili dynamical equations in unmagnetized dust plasma. Results Phys. 2018, 8, 1216–1222. [Google Scholar] [CrossRef]
- Seadawy, A.R. Ion acoustic solitary wave solutions of two-dimensional nonlinear Kadomtsev-Petviashvili-Burgers equation in quantum plasma. Math. Methods Appl. Sci. 2016, 40, 1598–1607. [Google Scholar] [CrossRef]
- Treumann, R.A.; Pottelette, R. Plasma Soliton Turbulence and Statistical Mechanics. In Proceedings of the Plasma Turbulence and Energetic Particles in Astrophysics, Cracow, Poland, 5–10 September 1999; pp. 167–181. [Google Scholar]
- Qin, Y.; Liu, Y. Multiwave interaction solutions for a (3+1)-dimensional generalized Kadomtsev-Petviashvili equation. Chin. J. Phys. 2021, 71, 561–573. [Google Scholar] [CrossRef]
- Li, Y.; Mei, C.C. Modified Kadomtsev–Petviashvili equation for tsunami over irregular seabed. Nat. Hazards 2016, 84, 513–528. [Google Scholar] [CrossRef]
- Xu, B.; Zhang, Y.; Zhang, S. Line Soliton Interactions for Shallow Ocean Waves and Novel Solutions with Peakon, Ring, Conical, Columnar, and Lump Structures Based on Fractional KP Equation. Adv. Math. Phys. 2021, 2021, 1–15. [Google Scholar] [CrossRef]
- Irwaq, I.A.; Alquran, M.; Jaradat, I.; Baleanu, D. New dual-mode Kadomtsev–Petviashvili model with strong–weak surface tension: Analysis and application. Adv. Differ. Equ. 2018, 2018, 433. [Google Scholar] [CrossRef]
- Wu, P.X.; Zhang, Y.F.; Yin, Q.Q.; Wang, Y. Integrability and lump-type solutions to the 3-D Kadomtsev-Petviashvili-Boussinesq-like equation. Therm. Sci. 2019, 23, 2373–2380. [Google Scholar] [CrossRef]
- Gao, B.; Zhang, Y. Exact Solutions and Conservation Laws of the (3+1)-Dimensional B-Type Kadomstev–Petviashvili (BKP)-Boussinesq Equation. Symmetry 2020, 12, 97. [Google Scholar] [CrossRef]
- Zhou, Q.; Pan, A.; Mirhosseini-Alizamini, S.M.; Mirzazadeh, M.; Liu, W.; Biswas, A. Group Analysis and Exact Soliton Solutions to a New (3+1)-Dimensional Generalized Kadomtsev-Petviashvili Equation in Fluid Mechanics. Acta Phys. Pol. A 2018, 134, 564–569. [Google Scholar] [CrossRef]
- Li, L.; Xie, Y.; Mei, L. Multiple-order rogue waves for the generalized (2+1)-dimensional Kadomtsev–Petviashvili equation. Appl. Math. Lett. 2021, 117, 107079. [Google Scholar] [CrossRef]
- Li, L.; Xie, Y. Rogue wave solutions of the generalized (3+1)-dimensional Kadomtsev–Petviashvili equation. Chaos Solitons Fractals 2021, 147, 110935. [Google Scholar] [CrossRef]
- Wang, Y.H. Nonautonomous lump solutions for a variable–coefficient Kadomtsev–Petviashvili equation. Appl. Math. Lett. 2021, 119, 107201. [Google Scholar] [CrossRef]
- Lu, D.; Tariq, K.; Osman, M.; Baleanu, D.; Younis, M.; Khater, M. New analytical wave structures for the (3+1)-dimensional Kadomtsev-Petviashvili and the generalized Boussinesq models and their applications. Results Phys. 2019, 14, 102491. [Google Scholar] [CrossRef]
- Shen, Y.; Tian, B. Bilinear auto-Bäcklund transformations and soliton solutions of a (3+1)-dimensional generalized nonlinear evolution equation for the shallow water waves. Appl. Math. Lett. 2021, 122, 107301. [Google Scholar] [CrossRef]
- Ma, Y.L.; Wazwaz, A.M.; Li, B.Q. A new (3+1)-dimensional Kadomtsev–Petviashvili equation and its integrability, multiple-solitons, breathers and lump waves. Math. Comput. Simul. 2021, 187, 505–519. [Google Scholar] [CrossRef]
- Singh, S.; Sakkaravarthi, K.; Murugesan, K. Localized nonlinear waves on spatio-temporally controllable backgrounds for a (3+1)-dimensional Kadomtsev-Petviashvili-Boussinesq model in water waves. Chaos Solitons Fractals 2022, 155, 111652. [Google Scholar] [CrossRef]
- Ozisik, M.; Secer, A.; Bayram, M.; Aydin, H. An encyclopedia of Kudryashov’s integrability approaches applicable to optoelectronic devices. Optik 2022, 265, 169499. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ozisik, M.; Secer, A.; Bayram, M. Soliton Waves with the (3+1)-Dimensional Kadomtsev–Petviashvili–Boussinesq Equation in Water Wave Dynamics. Symmetry 2023, 15, 165. https://doi.org/10.3390/sym15010165
Ozisik M, Secer A, Bayram M. Soliton Waves with the (3+1)-Dimensional Kadomtsev–Petviashvili–Boussinesq Equation in Water Wave Dynamics. Symmetry. 2023; 15(1):165. https://doi.org/10.3390/sym15010165
Chicago/Turabian StyleOzisik, Muslum, Aydin Secer, and Mustafa Bayram. 2023. "Soliton Waves with the (3+1)-Dimensional Kadomtsev–Petviashvili–Boussinesq Equation in Water Wave Dynamics" Symmetry 15, no. 1: 165. https://doi.org/10.3390/sym15010165
APA StyleOzisik, M., Secer, A., & Bayram, M. (2023). Soliton Waves with the (3+1)-Dimensional Kadomtsev–Petviashvili–Boussinesq Equation in Water Wave Dynamics. Symmetry, 15(1), 165. https://doi.org/10.3390/sym15010165




