Thermally Radiative Darcy–Forchheimer Flow of Cu/Ag Nanoliquid in Water Past a Heated Stretchy Sheet with Magnetic and Viscous Dissipation Impacts
Abstract
:1. Introduction
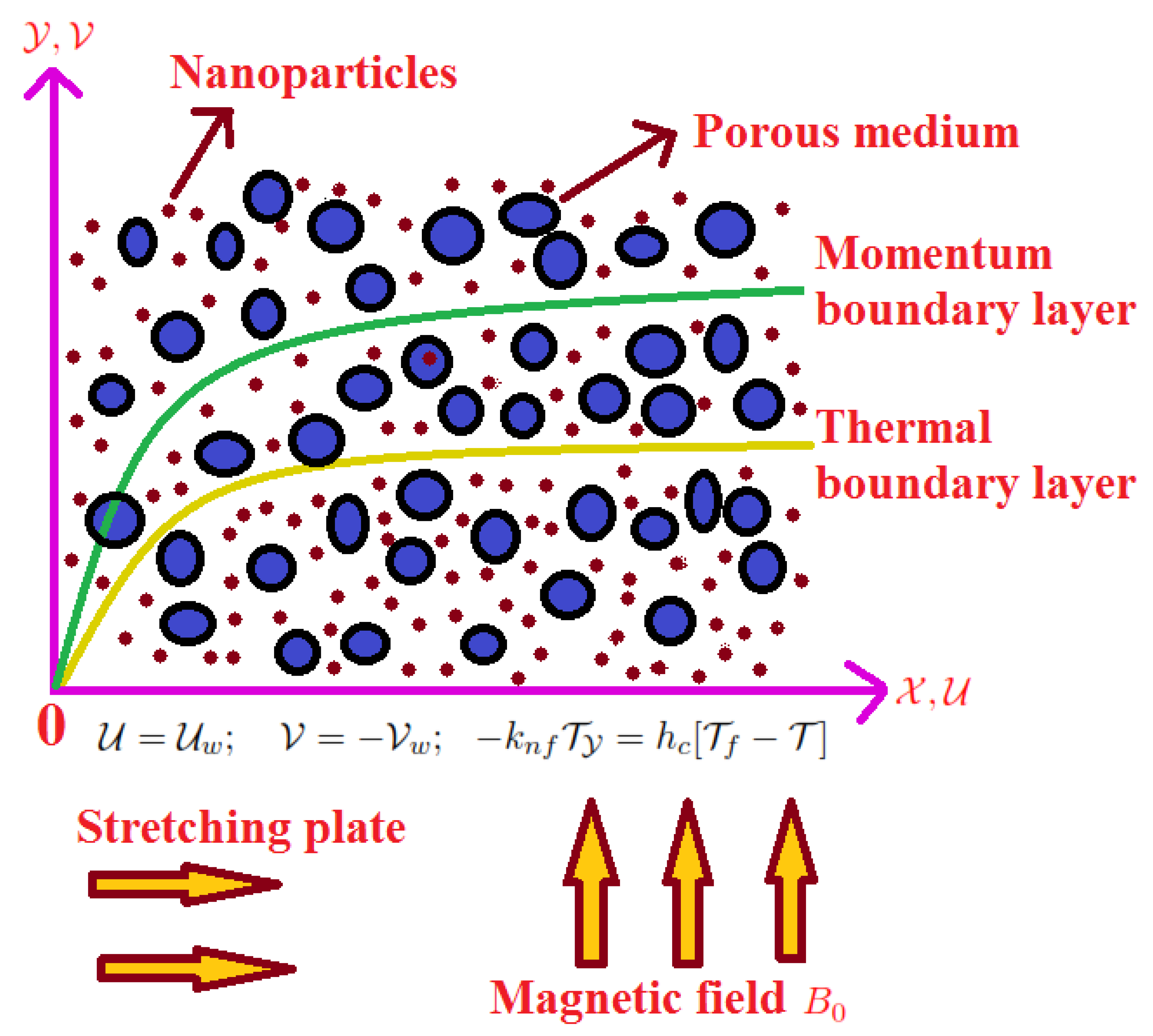
2. Mathematical Formulation
3. Numerical Solution
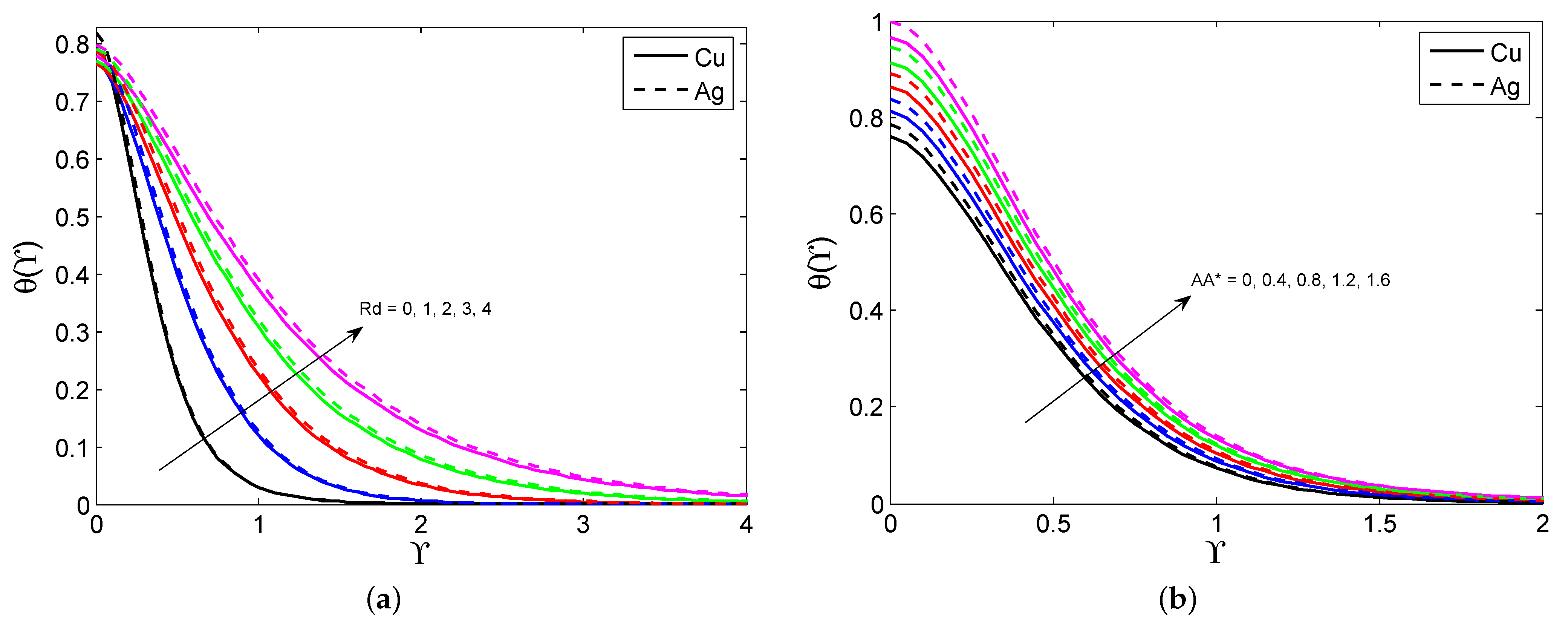
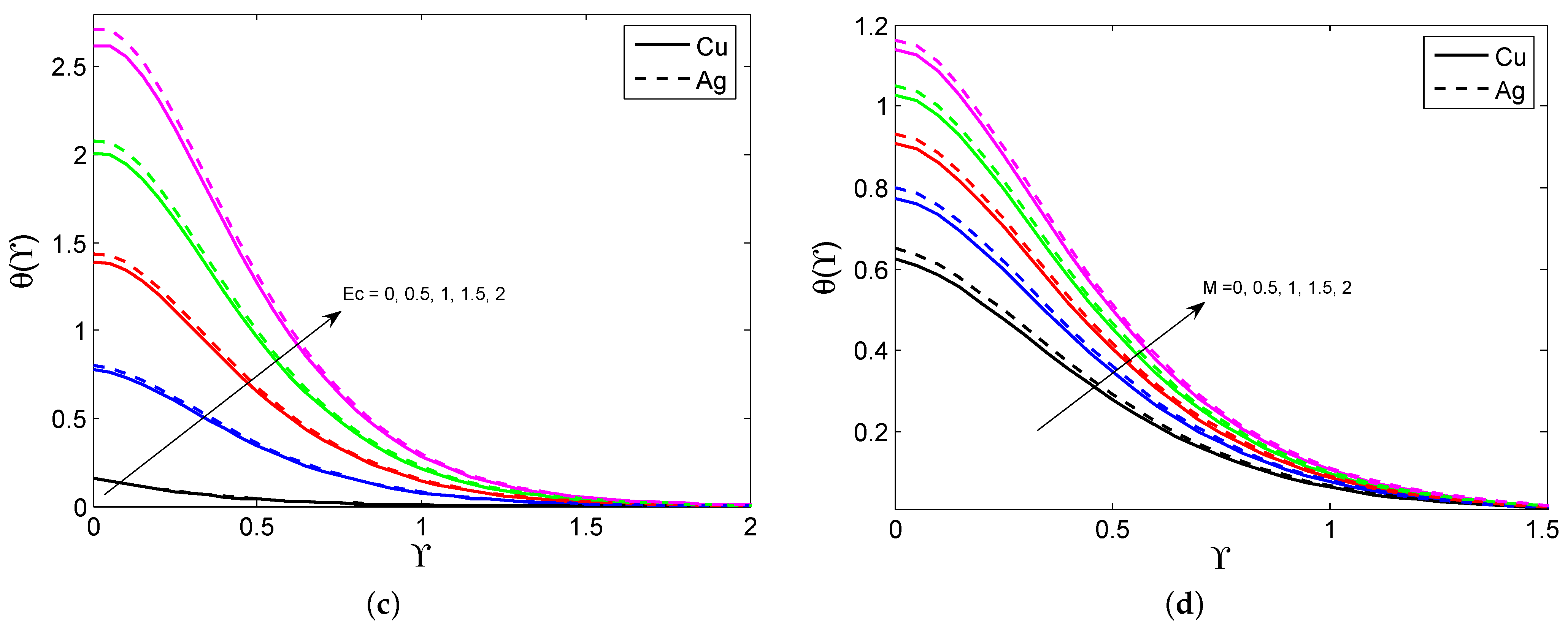
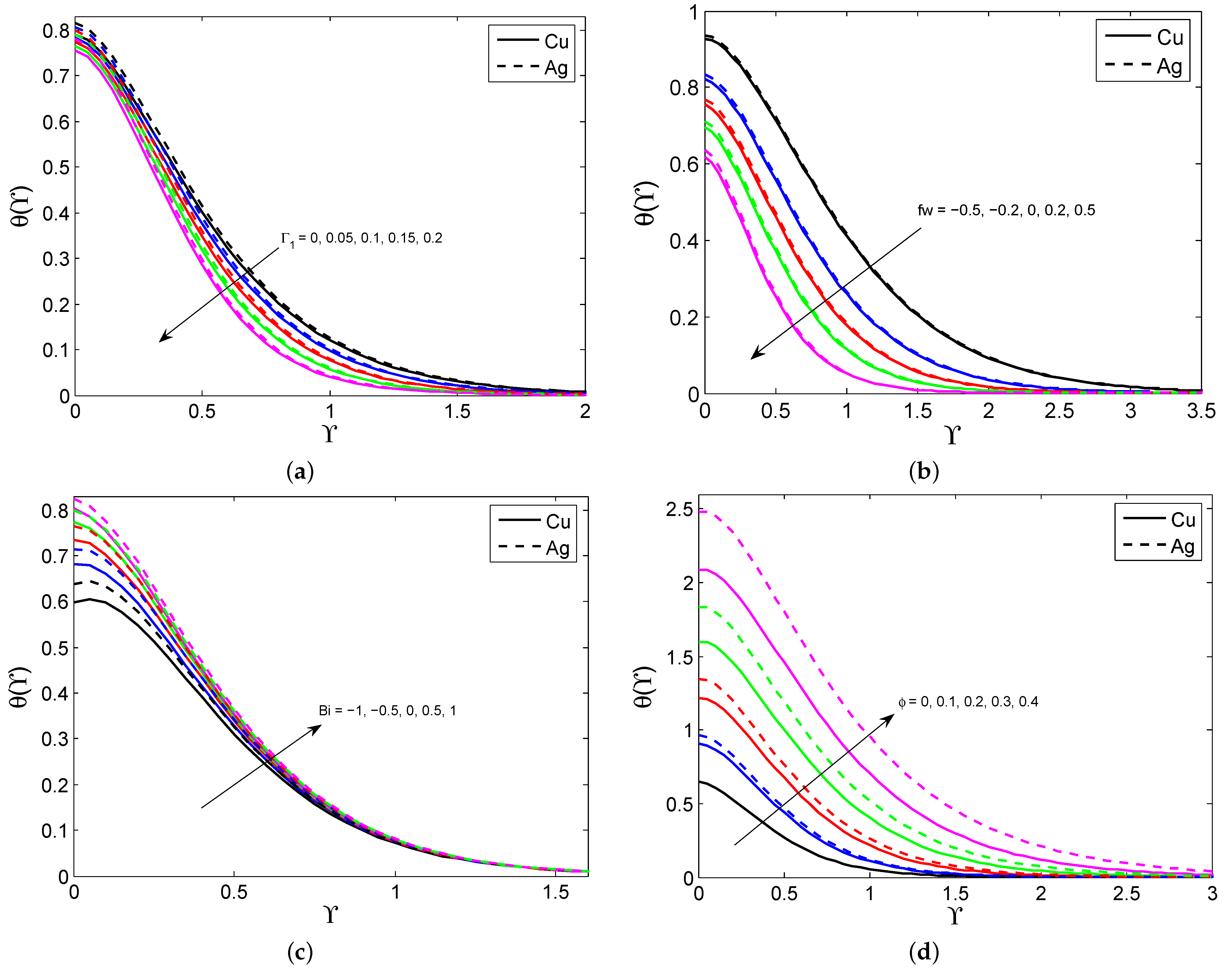
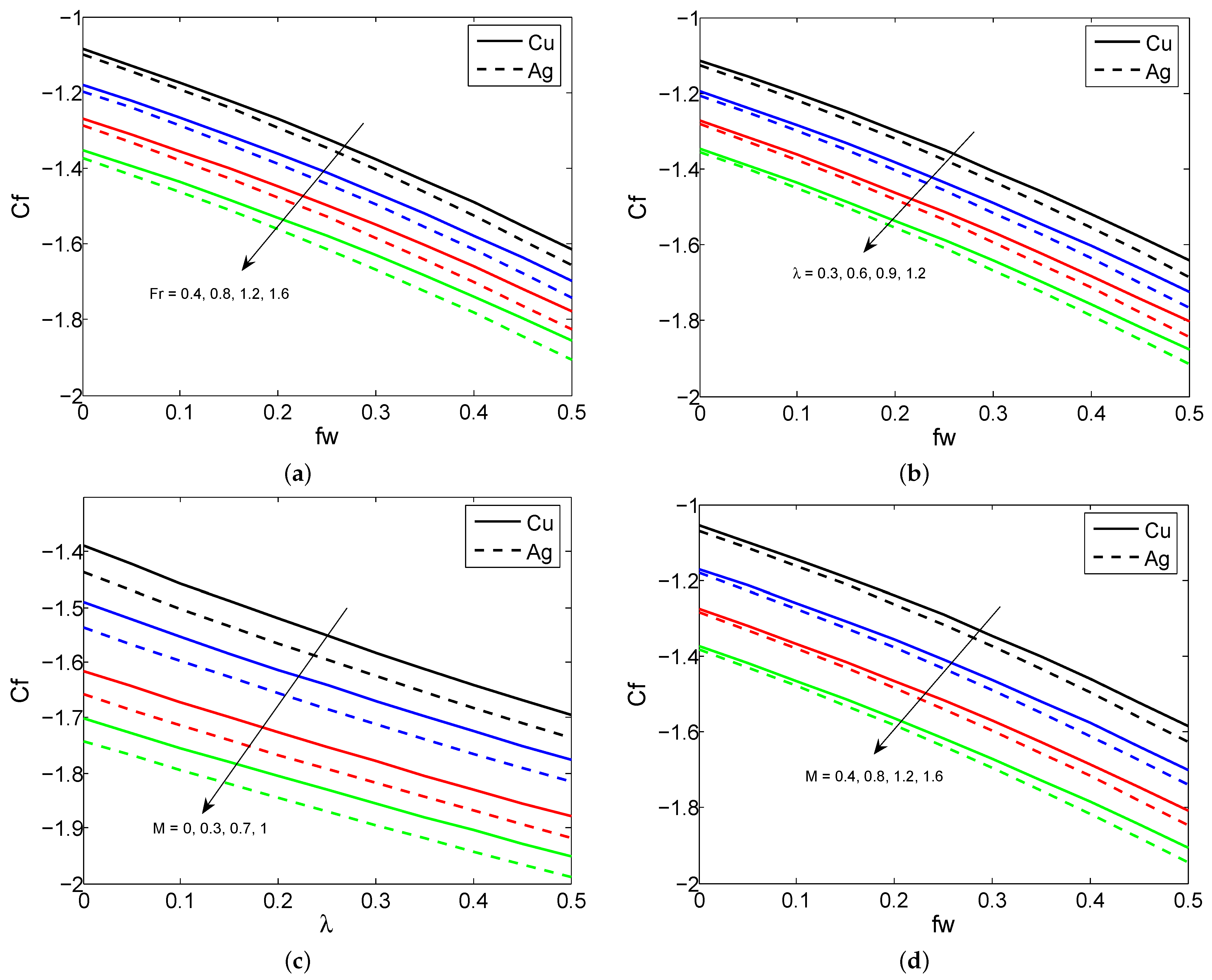
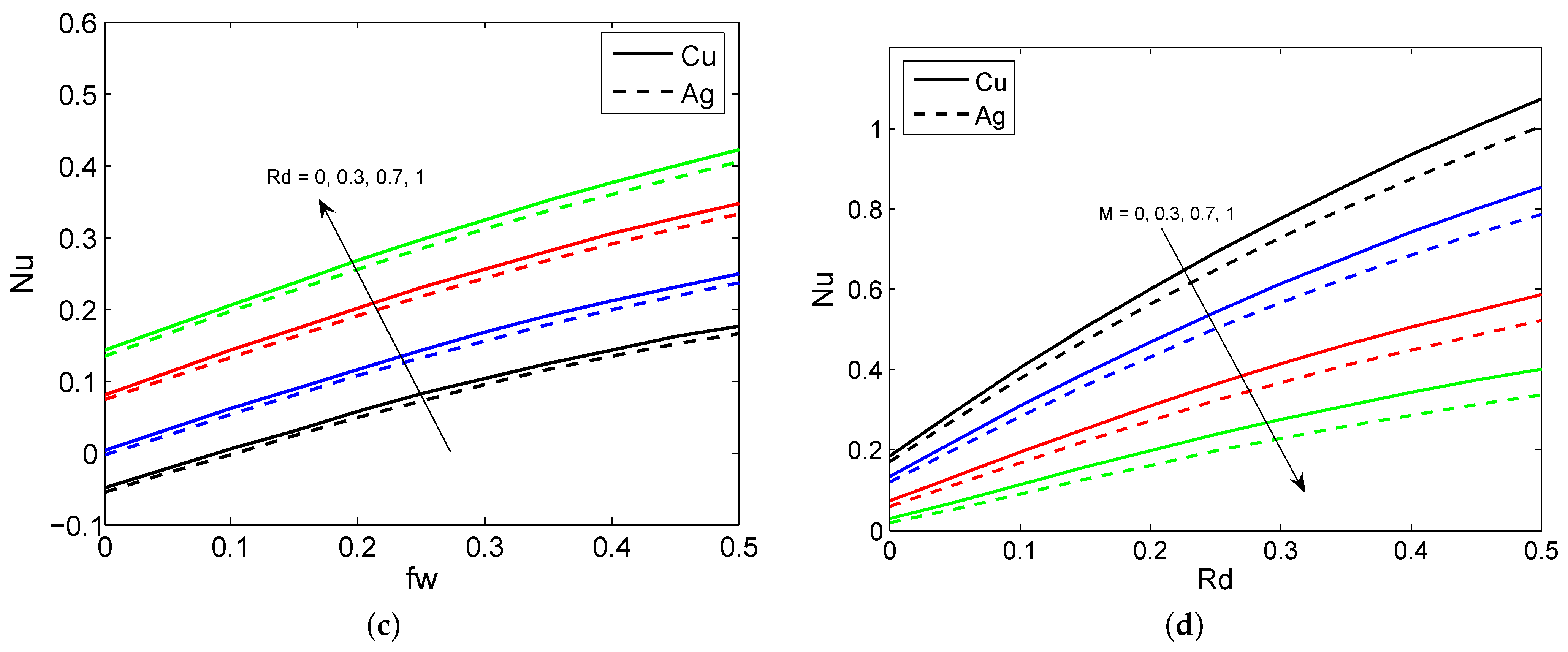
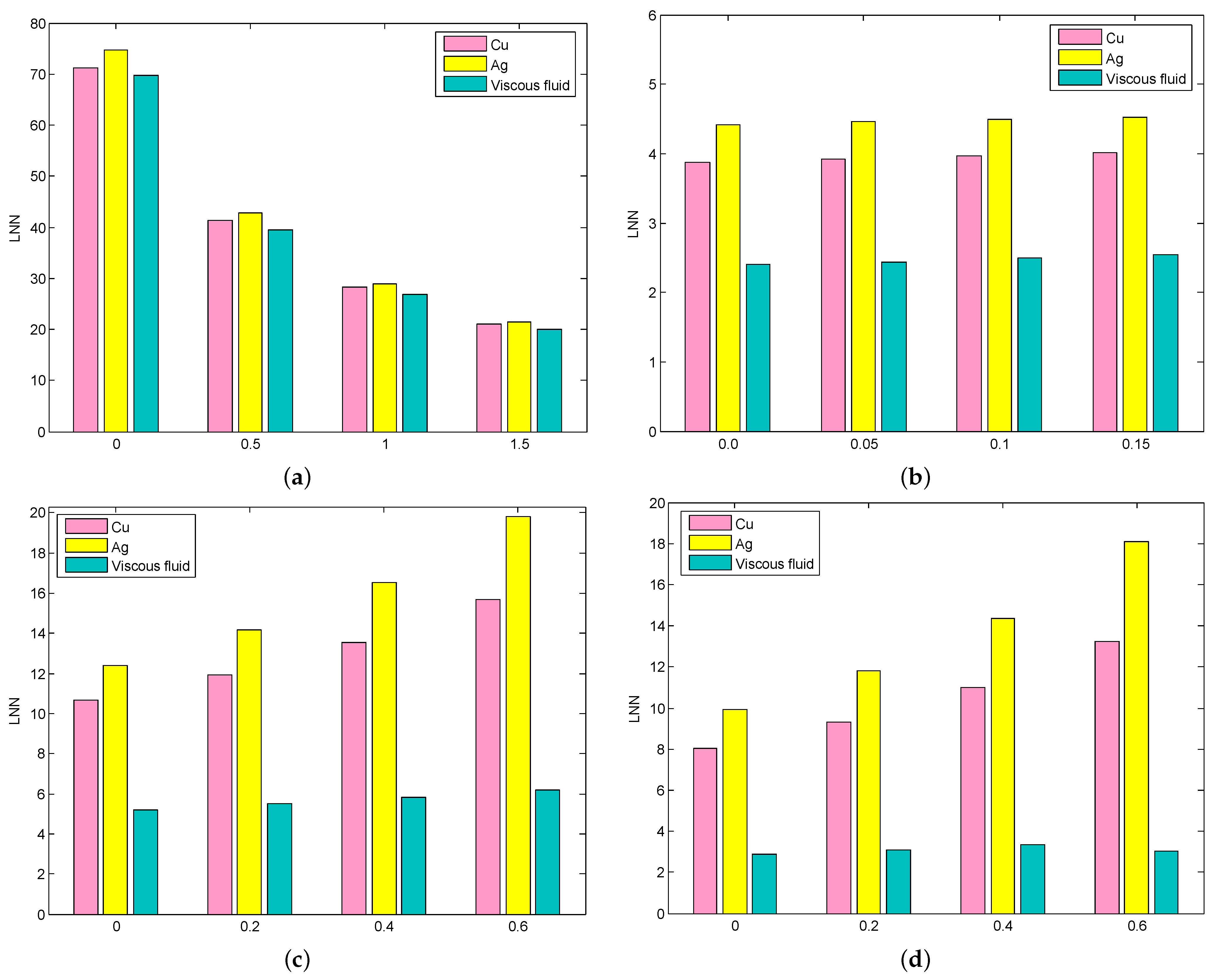
4. Results and Discussion
5. Conclusions
- The nanoliquid speed reduces when strengthening the porosity parameter, Forchheimer number, magnetic parameter, and injection/suction parameter.
- The nanoliquid warmth rises when enhancing the radiation, heat sink/source, viscous dissipation, and magnetic field parameter.
- The nanoliquid temperature declines when raising the heat relaxation time and injection/suction parameter, and it exalts when strengthening the Biot number and nanoparticle volume fraction.
- The skin friction depresses for the higher quantity of the Forchheimer number, porosity, and magnetic field parameter.
- The Nusselt number slumps when mounting the values of the Eckert number and magnetic field parameter. It strengthens the radiation and injection/suction parameters.
- In the future, we will expand this flow model to include the Riga plate and the convective heating scenario.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbols | Description |
| axes coordinates | |
| ambient temperature | |
| Biot number | |
| density | |
| dynamic viscosity | |
| dimensionless variable | |
| dimensionless temperature | |
| factors of velocity | |
| heat transfer coefficient | |
| kinematic viscosity | |
| liquid temperature | |
| nanoparticle volume fraction | |
| Nusselt number | |
| permeability of the porous medium | |
| surface stretching velocities | |
| specific heat capacity | |
| subscript represents nanoliquid and base liquid | |
| space-dependent coefficient | |
| skin friction coefficient | |
| suction/injection parameter | |
| Stefen–Boltzmann constant | |
| temperature of the hot liquid | |
| thermal conductivity | |
| temperature-dependent coefficient | |
| Eckert number | |
| Forchheimer number | |
| heat relaxation time parameter | |
| local porosity parameter | |
| magnetic field parameter | |
| Prandtl number | |
| radiation parameter | |
| Abbreviations | Expansions |
| BLT | boundary layer thickness |
| CCHF | Cattaneo–Christov heat flux |
| DF | Darcy–Forchheimer |
| EG | entropy generation |
| HTG | heat transfer gradient |
| LNN | local Nusselt number |
| MBL | momentum boundary layer |
| MHD | magnetohydrodynamics |
| NPVF | nanoparticle volume fraction |
| ODE | ordinary differential equations |
| PDE | partial differential equations |
| SS | stretching sheet/surface |
| SFC | skin friction coefficient |
| TBL | thermal boundary layer |
| WB | water based |
References
- Choi, S.U.S.; Eastman, J.A. Enhancing thermal conductivity of fluids with nanoparticle, Developments and Applications of Non-Newtonian Flows. In Proceedings of the ASME International Mechanical Engineering Congress and Exhibition, San Francisco, CA, USA, 12–17 November 1995; Volume 231, pp. 99–105. [Google Scholar]
- Govindaraju, M.; Ganesh, N.V.; Ganga, B.; Hakeem, A.A. Entropy generation analysis of magneto hydrodynamic flow of a nanofluid over a stretching sheet. J. Egypt. Math. Soc. 2015, 23, 429–434. [Google Scholar] [CrossRef]
- Malvandi, A.; Hedayati, F.; Ganji, D.D. Nanofluid flow on the stagnation point of a permeable non-linearly stretching/shrinking sheet. Alex. Eng. J. 2018, 57, 2199–2208. [Google Scholar] [CrossRef]
- Mahabaleshwar, U.S.; Sneha, K.N.; Huang, H.N. An effect of MHD and radiation on CNTs-Water based nanofluids due to a stretching sheet in a Newtonian fluid. Case Stud. Therm. Eng. 2021, 28, 101462. [Google Scholar] [CrossRef]
- Sandeep, N.; Sulochana, C. MHD flow over a permeable stretching/shrinking sheet of a nanofluid with suction/injection. Alex. Eng. J. 2016, 55, 819–827. [Google Scholar]
- Iqbal, Z.; Mehmood, R.; Ahmad, B.; Maraj, E.N. Combined impact of viscosity variation and Lorentz force on slip flow of radiative nanofluid towards a vertical stretching surface with convective heat and mass transfer. Alex. Eng. J. 2018, 57, 3189–3197. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Transpiration effects on hybrid nanofluid flow and heat transfer over a stretching/shrinking sheet with uniform shear flow. Alex. Eng. J. 2020, 59, 91–99. [Google Scholar] [CrossRef]
- Waqas, H.; Bukhari, F.F.; Farooq, U.; Alqarni, M.S.; Muhammad, T. Numerical computation of melting heat transfer in nonlinear radiative flow of hybrid nanofluids due to permeable stretching curved surface. Case Stud. Therm. Eng. 2021, 27, 101348. [Google Scholar] [CrossRef]
- Rehman, S.U.; Mariam, A.; Ullah, A.; Asjad, M.I.; Bajuri, M.Y.; Pansera, B.A.; Ahmadian, A. Numerical computation of buoyancy and radiation effects on MHD micropolar nanofluid flow over a stretching/shrinking sheet with heat source. Case Stud. Therm. Eng. 2021, 25, 100867. [Google Scholar] [CrossRef]
- Kakar, N.; Khalid, A.; Al-Johani, A.S.; Alshammari, N.; Khan, I. Melting heat transfer of a magnetized water-based hybrid nanofluid flow past over a stretching/shrinking wedge. Case Stud. Therm. Eng. 2022, 30, 101674. [Google Scholar] [CrossRef]
- Eswaramoorthi, S.; Divya, S.; Faisal, M.; Namgyel, N. Entropy and heat transfer analysis for MHD flow of-water-based nanofluid on a heated 3D plate with nonlinear radiation. Math. Probl. Eng. 2022, 2022, 7319988. [Google Scholar] [CrossRef]
- Hosseinzadeh, K.; Afsharpanah, F.; Zamani, S.; Gholinia, M.; Ganji, D.D. A numerical investigation on ethylene glycol-titanium dioxide nanofluid convective flow over a stretching sheet in presence of heat generation/absorption. Case Stud. Therm. Eng. 2018, 12, 228–236. [Google Scholar] [CrossRef]
- Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. Stability analysis of MHD hybrid nanofluid flow over a stretching/shrinking sheet with quadratic velocity. Alex. Eng. J. 2021, 60, 915–926. [Google Scholar] [CrossRef]
- Sandeep, N.; Sulochana, C. MHD flow of dusty nanofluid over a stretching surface with volume fraction of dust particles. Ain Shams Eng. J. 2016, 7, 709–716. [Google Scholar]
- Das, K.; Sarkar, A.; Kundu, P.K. Cu-water nanofluid flow induced by a vertical stretching sheet in presence of a magnetic field with convective heat transfer. Propuls. Power Res. 2017, 6, 206–213. [Google Scholar] [CrossRef]
- Ali, F.; Loganathan, K.; Eswaramoorthi, S.; Prabu, K.; Zaib, A.; Chaudhary, D.K. Heat Transfer Analysis on Carboxymethyl Cellulose Water-Based Cross Hybrid Nanofluid Flow with Entropy Generation. J. Nanomater. 2022, 2022, 5252918. [Google Scholar] [CrossRef]
- El-Zahar, E.R.; Mahdy, A.E.N.; Rashad, A.M.; Saad, W.; Seddek, L.F. Unsteady MHD mixed convection flow of Non-Newtonian Casson hybrid nanofluid in the stagnation zone of sphere spinning impulsively. Fluids 2021, 6, 197. [Google Scholar] [CrossRef]
- Forchheimer, P.Z. Wasserbewegungdurch Boden. Z. VDI 1901, 45, 1781–1788. [Google Scholar]
- Ullah, M.Z.; Serra-Capizzano, S.; Baleanu, D. A numerical simulation for Darcy-Forchheimer flow of nanofluid by a rotating disk with partial slip effects. Front. Phys. 2020, 7, 219. [Google Scholar] [CrossRef] [Green Version]
- Sarkar, A.; Kundu, P.K. Darcy-Forchheimer flow of Cu-water nanofluid over a vertical sheet owing to solar radiation. J. Phys. 2021, 95, 1–7. [Google Scholar] [CrossRef]
- Khan, S.A.; Hayat, T.; Alsaedi, A.; Alhodaly, M.S. Thermal analysis for radiative flow of Darcy-Forchheimer nanomaterials subject to entropy generation. J. Comput. Des. Eng. 2022, 9, 1756–1764. [Google Scholar] [CrossRef]
- Sureshkumar Raju, S.; Ganesh Kumar, K.; Rahimi-Gorji, M.; Khan, I. Darcy-Forchheimer flow and heat transfer augmentation of a viscoelastic fluid over an incessant moving needle in the presence of viscous dissipation. Microsyst. Technol. 2019, 25, 3399–3405. [Google Scholar] [CrossRef]
- Hayat, T.; Haider, F.; Alsaedi, A. Darcy-Forchheimer flow with nonlinear mixed convection. J. Appl. Math. Mech. 2020, 41, 1685–1696. [Google Scholar] [CrossRef]
- Khan, M.I.; Hayat, T.; Alsaedi, A. Numerical analysis for Darcy-Forchheimer flow in presence of homogeneous-heterogeneous reactions. Results Phys. 2017, 7, 2644–2650. [Google Scholar] [CrossRef]
- Nayak, M.K.; Shaw, S.; Khan, M.I.; Pandey, V.S.; Nazeer, M. Flow and thermal analysis on Darcy-Forchheimer flow of copper-water nanofluid due to a rotating disk: A static and dynamic approach. J. Mater. Res. Technol. 2020, 9, 7387–7408. [Google Scholar] [CrossRef]
- Mahdy, A.; El-Zahar, E.R.; Rashad, A.M.; Saad, W.; Al-Juaydi, H.S. The magneto-natural convection flow of a micropolar hybrid nanofluid over a vertical plate saturated in a porous medium. Fluids 2021, 6, 202. [Google Scholar] [CrossRef]
- Jakeer, S.; Reddy, P.B.; Rashad, A.M.; Nabwey, H.A. Impact of heated obstacle position on magneto-hybrid nanofluid flow in a lid-driven porous cavity with Cattaneo-Christov heat flux pattern. Alex. Eng. J. 2021, 60, 821–835. [Google Scholar] [CrossRef]
- El-Zahar, E.R.; Rashad, A.M.; Al-Juaydi, H.S. Studying massive suction impact on magneto-flow of a hybridized Casson nanofluid on a porous continuous moving or fixed surface. Symmetry 2022, 14, 627. [Google Scholar] [CrossRef]
- Pal, D.; Mandal, G. Double diffusive magnetohydrodynamic heat and mass transfer of nanofluids over a nonlinear stretching/shrinking sheet with viscous-Ohmic dissipation and thermal radiation. Propuls. Power Res. 2017, 6, 58–69. [Google Scholar] [CrossRef]
- Das, S.; Jana, R.N.; Makinde, O.D. Magnetohydrodynamic mixed convective slip flow over an inclined porous plate with viscous dissipation and Joule heating. Alex. Eng. J. 2015, 54, 251–261. [Google Scholar] [CrossRef]
- Bhukta, D.; Dash, G.C.; Mishra, S.R.; Baag, S. Dissipation effect on MHD mixed convection flow over a stretching sheet through porous medium with non-uniform heat source/sink. Ain Shams Eng. J. 2017, 8, 353–361. [Google Scholar] [CrossRef] [Green Version]
- Salahuddin, T.; Awais, M.; Salleh, Z. A flow study of Carreau fluid near the boundary layer region of paraboloid surface with viscous dissipation and variable fluid properties. J. Mater. Res. Technol. 2021, 14, 901–909. [Google Scholar] [CrossRef]
- Gopal, D.; Saleem, S.; Jagadha, S.; Ahmad, F.; Othman Almatroud, A.; Kishan, N. Numerical analysis of higher order chemical reaction on electrically MHD nanofluid under influence of viscous dissipation. Alex. Eng. J. 2021, 60, 1861–1871. [Google Scholar] [CrossRef]
- Hussain, A.; Malik, M.Y.; Salahuddin, T.; Rubab, A.; Khan, M. Effects of viscous dissipation on MHD tangent hyperbolic fluid over a nonlinear stretching sheet with convective boundary conditions. Results Phys. 2017, 7, 3502–3509. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Salah, F. Double stratification effects on unsteady electrical MHD mixed convection flow of nanofluid with viscous dissipation and Joule heating. J. Appl. Res. Technol. 2017, 15, 464–476. [Google Scholar] [CrossRef]
- Hakeem, A.A.; Ganesh, N.V.; Ganga, B. Effect of heat radiation in a Walter’s liquid B fluid over a stretching sheet with non-uniform heat source/sink and elastic deformation. J. King Saud Univ. Eng. Sci. 2014, 26, 168–175. [Google Scholar]
- Sandeep, N.; Sulochana, C. Momentum and heat transfer behaviour of Jeffrey, Maxwell and Oldroyd-B nanofluids past a stretching surface with non-uniform heat source/sink. Ain Shams Eng. J. 2018, 9, 517–524. [Google Scholar] [CrossRef] [Green Version]
- Manvi, B.; Tawade, J.; Biradar, M.; Noeiaghdam, S.; Fernandez-Gamiz, U.; Govindan, V. The effects of MHD radiating and non-uniform heat source/sink with heating on the momentum and heat transfer of Eyring-Powell fluid over a stretching. Results Eng. 2022, 14, 100435. [Google Scholar] [CrossRef]
- Pal, D.; Chatterjee, S. Effects of radiation on Darcy-Forchheimer convective flow over a stretching sheet in a micropolar fluid with non-uniform heat source/sink. J. Appl. Fluid Mech. 2015, 8, 207–212. [Google Scholar] [CrossRef]
- Mabood, F.; Ibrahim, S.M.; Rashidi, M.M.; Shadloo, M.S.; Lorenzini, G. Non-uniform heat source/sink and Soret effects on MHD non-Darcian convective flow past a stretching sheet in a micropolar fluid with radiation. Int. J. Heat Mass Transf. 2016, 93, 674–682. [Google Scholar] [CrossRef]
- Ramandevi, B.; Ramana Reddy, J.V.; Sugunamma, V.; Sandeep, N. Combined influence of viscous dissipation and non-uniform heat source/sink on MHD non-Newtonian fluid flow with Cattaneo-Christov heat flux. Alex. Eng. J. 2018, 57, 1009–1018. [Google Scholar] [CrossRef]
- Song, Y.Q.; Hamid, A.; Sun, T.C.; Khan, M.I.; Qayyum, S.; Kumar, R.N.; Prasannakumara, B.C.; Khan, S.U.; Chinram, R. Unsteady mixed convection flow of magneto-Williamson nanofluid due to stretched cylinder with significant non-uniform heat source/sink features. Alex. Eng. J. 2022, 61, 195–206. [Google Scholar] [CrossRef]
- Mumraiz, S.; Ali, A.; Awais, M.; Shutaywi, M.; Shah, Z. Entropy generation in electrical magnetohydrodynamic flow of Al2O3-Cu/H2O hybrid nanofluid with non-uniform heat flux. J. Therm. Anal. Calorim. 2021, 143, 2135–2148. [Google Scholar] [CrossRef]
- Hamad, M.A.A. Analytical solution of natural convection flow of a nanofluid over a linearly stretching sheet in the presence of magnetic field. Int. Commun. Heat Mass Transf. 2011, 38, 487–492. [Google Scholar] [CrossRef]
- Kameswaran, P.K.; Narayana, M.; Sibanda, P.; Murthy, P.V.S.N. Hydromagnetic nanofluid flow due to a stretching or shrinking sheet with viscous dissipation and chemical reaction effects. Int. J. Heat Mass Transf. 2012, 55, 7587–7595. [Google Scholar] [CrossRef]
- Shankar, B.; Gorfie, E.H. Magnetohydrodynamic nanofluid flow over a stretching sheet with thermal radiation, viscous dissipation, chemical reaction and ohmic effects. J. Nanofluids 2014, 3, 227–237. [Google Scholar] [CrossRef]
- Eswaramoorthi, S.; Loganathan, K.; Faisal, M.; Botmart, T.; Shah, N.A. Analytical and numerical investigation of Darcy-Forchheimer flow of a nonlinear-radiative non-Newtonian fluid over a Riga plate with entropy optimization. Ain Shams Eng. J. 2022, 101887. [Google Scholar] [CrossRef]
- Eswaramoorthi, S.; Alessa, N.; Sangeethavaanee, M.; Kayikci, S.; Namgyel, N. Mixed Convection and Thermally Radiative Flow of MHD Williamson Nanofluid with Arrhenius Activation Energy and Cattaneo–Christov Heat-Mass Flux. J. Math. 2021, 2021, 2490524. [Google Scholar] [CrossRef]
- Rahim, T.; Hasnain, J.; Abid, N.; Abbas, Z. Entropy generation for mixed convection flow in vertical annulus with two regions hydromagnetic viscous and Cu-Ag water hybrid nanofluid through porous zone: A comparative numerical study. Propuls. Power Res. 2022, 11, 401–415. [Google Scholar] [CrossRef]
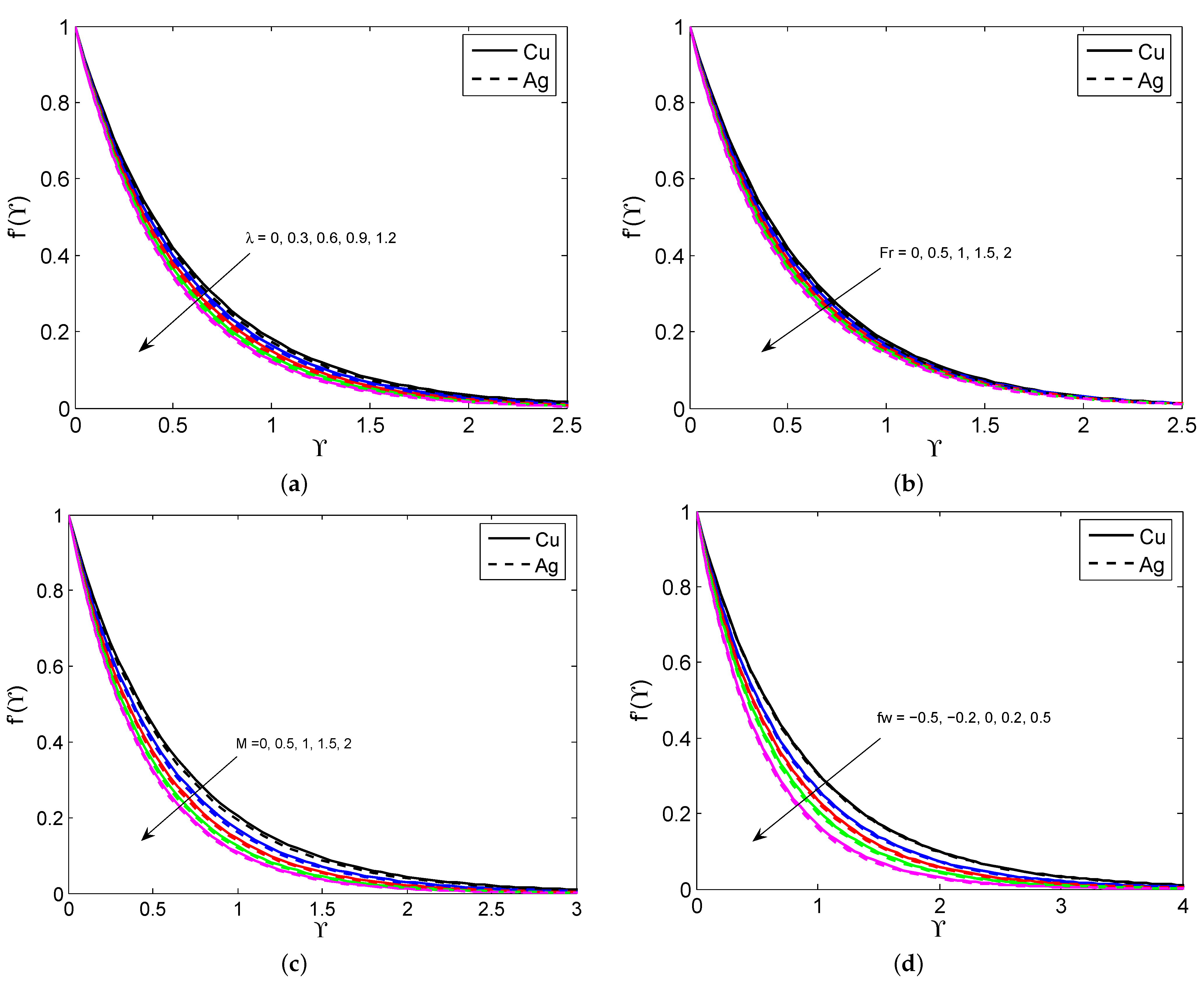

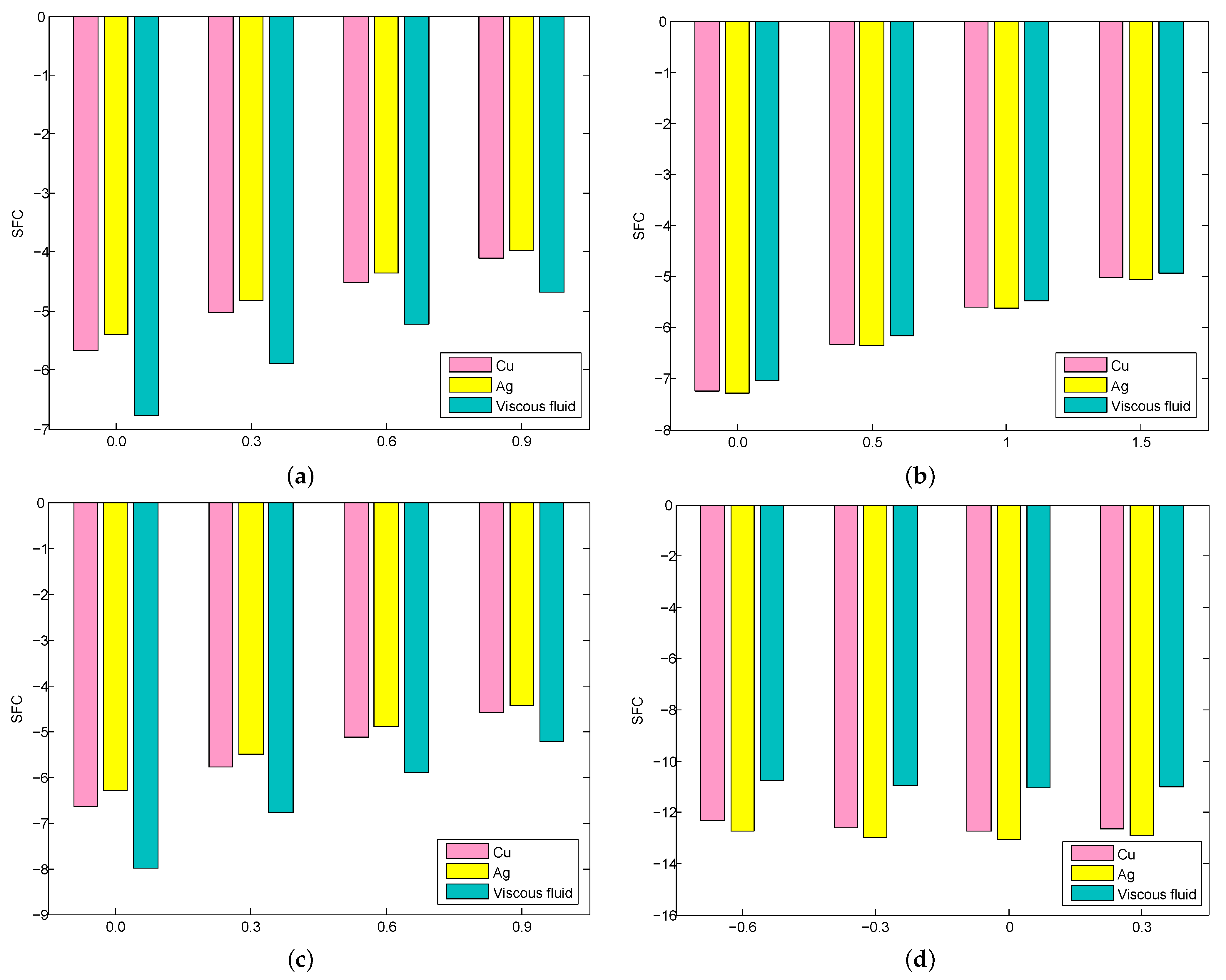
| Base Liquid and Nanoparticles | Copper | Silver | Water |
|---|---|---|---|
| 8933 | 10,500 | ||
| /(J.kg.K) | 385 | 235 | 4179 |
| 401 | 429 |
| M | Present Study | Ref. [44] | Present Study | Ref. [44] | |
|---|---|---|---|---|---|
| 0 | |||||
| M | Cu | ||||
|---|---|---|---|---|---|
| 0 | |||||
| 0 | |||||
| 1 | |||||
| 2 | |||||
| 0 | |||||
| 0 | |||||
| M | Ag | ||||
|---|---|---|---|---|---|
| 0 | |||||
| 0 | |||||
| 1 | |||||
| 2 | |||||
| 0 | |||||
| 0 | |||||
| 0 | |||||||
| 1 | |||||||
| 2 | |||||||
| 0 | |||||||
| 0 | |||||||
| 0 | |||||||
| 0 | |||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Divya, S.; Alessa, N.; Eswaramoorthi, S.; Loganathan, K. Thermally Radiative Darcy–Forchheimer Flow of Cu/Ag Nanoliquid in Water Past a Heated Stretchy Sheet with Magnetic and Viscous Dissipation Impacts. Symmetry 2023, 15, 16. https://doi.org/10.3390/sym15010016
Divya S, Alessa N, Eswaramoorthi S, Loganathan K. Thermally Radiative Darcy–Forchheimer Flow of Cu/Ag Nanoliquid in Water Past a Heated Stretchy Sheet with Magnetic and Viscous Dissipation Impacts. Symmetry. 2023; 15(1):16. https://doi.org/10.3390/sym15010016
Chicago/Turabian StyleDivya, S., Nazek Alessa, S. Eswaramoorthi, and Karuppusamy Loganathan. 2023. "Thermally Radiative Darcy–Forchheimer Flow of Cu/Ag Nanoliquid in Water Past a Heated Stretchy Sheet with Magnetic and Viscous Dissipation Impacts" Symmetry 15, no. 1: 16. https://doi.org/10.3390/sym15010016
APA StyleDivya, S., Alessa, N., Eswaramoorthi, S., & Loganathan, K. (2023). Thermally Radiative Darcy–Forchheimer Flow of Cu/Ag Nanoliquid in Water Past a Heated Stretchy Sheet with Magnetic and Viscous Dissipation Impacts. Symmetry, 15(1), 16. https://doi.org/10.3390/sym15010016








