Effects of a Geometrically Realized Early Dark Energy Era on the Spectrum of Primordial Gravitational Waves
Abstract
1. Introduction
2. Gravity Realization of Inflation and Subsequent Eras
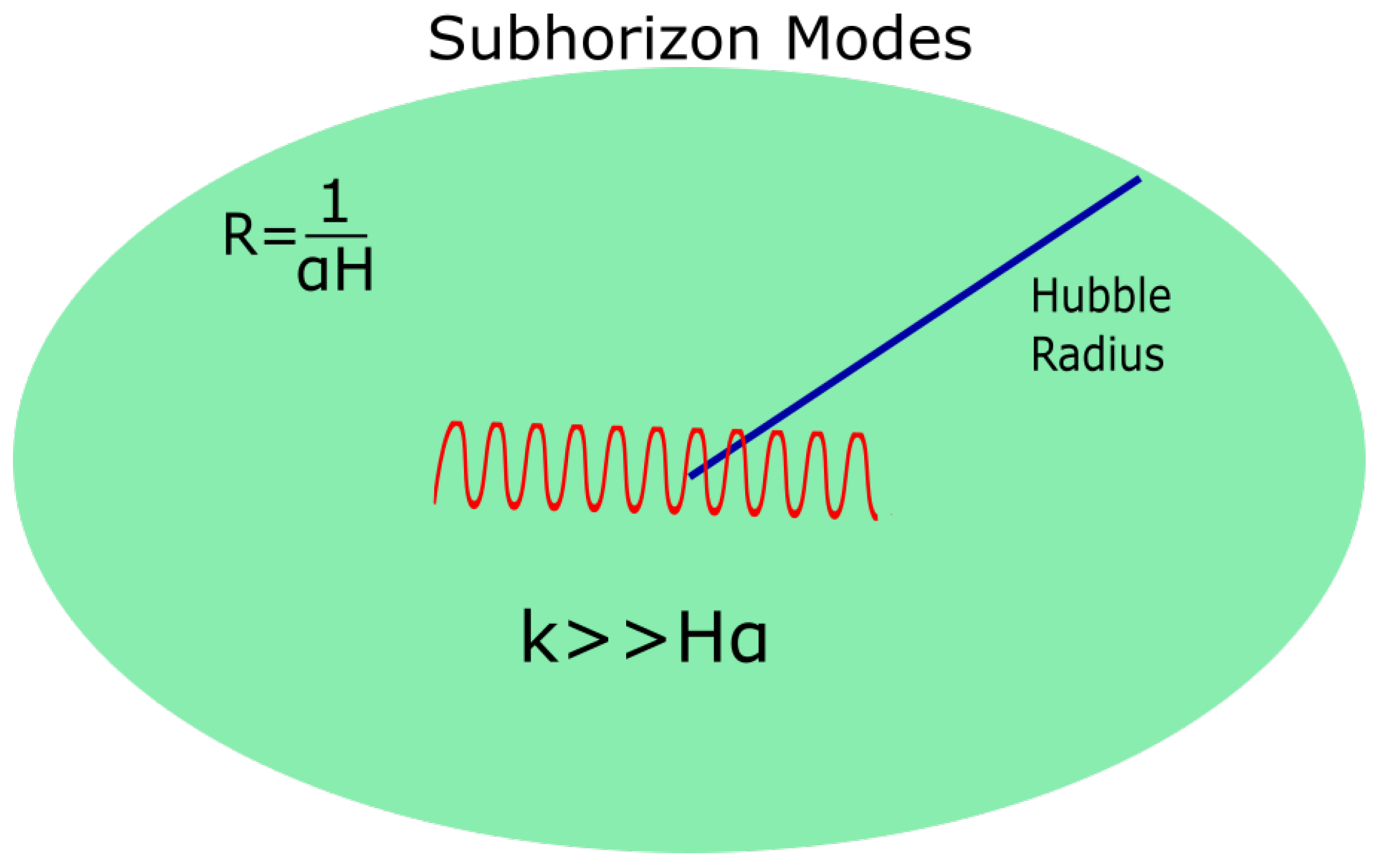
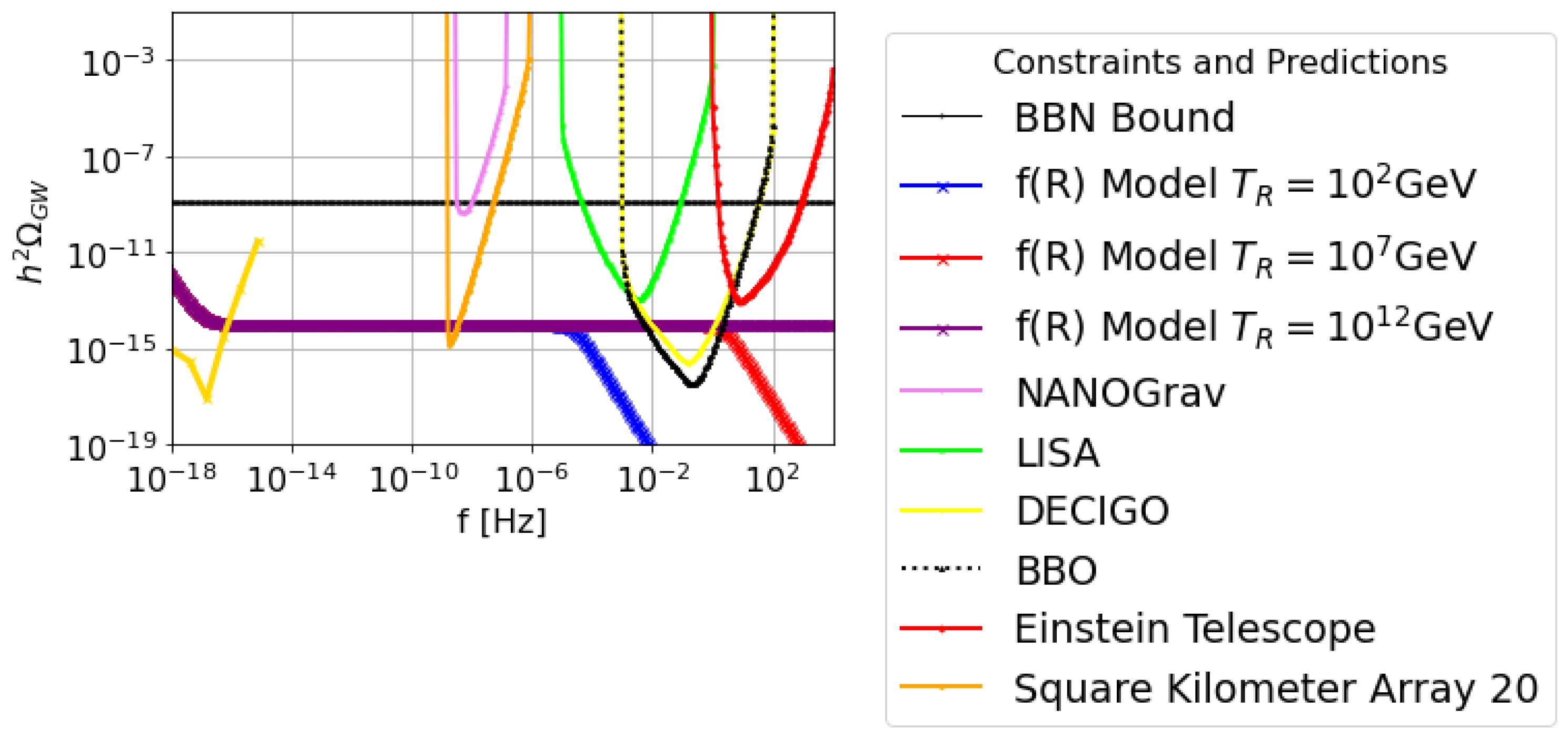
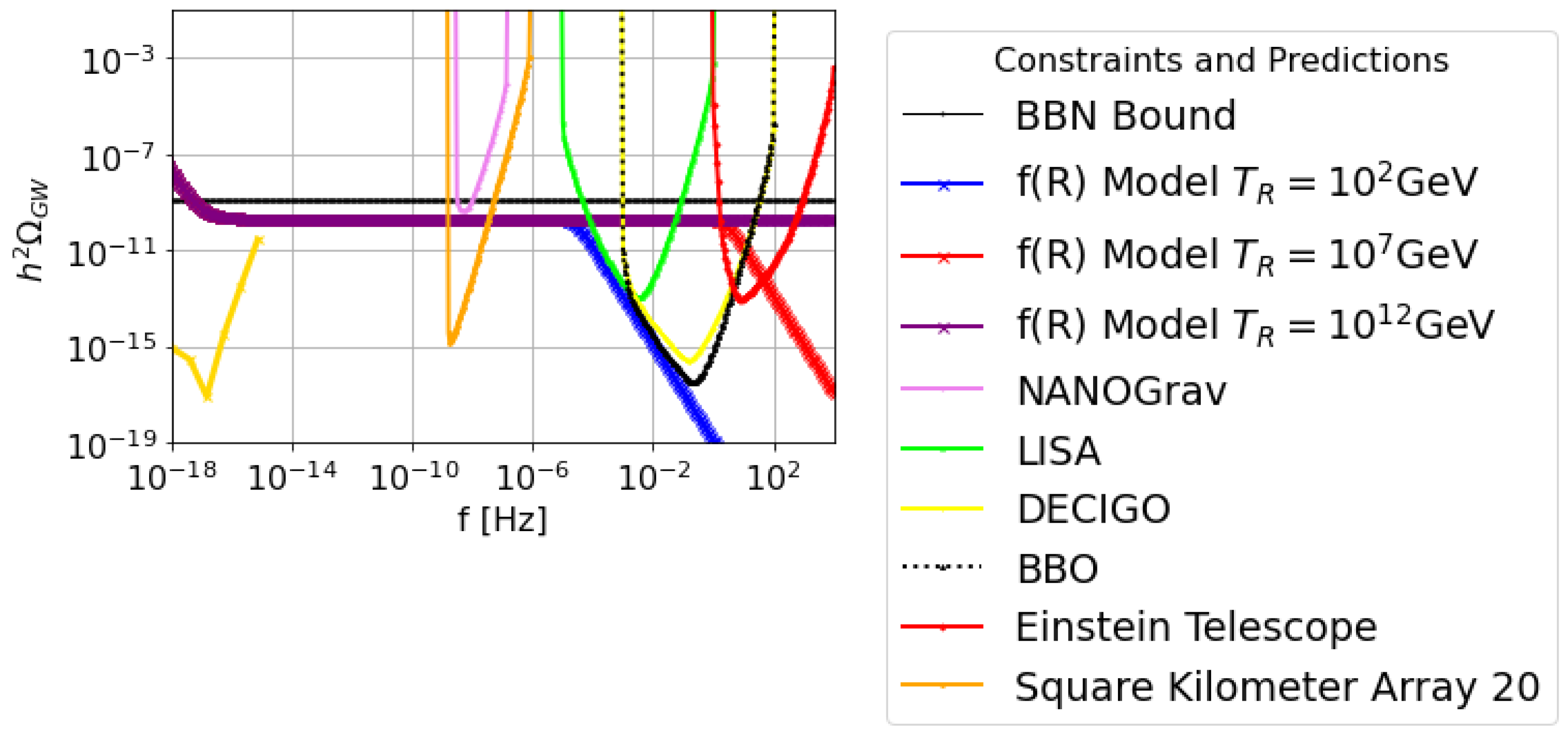
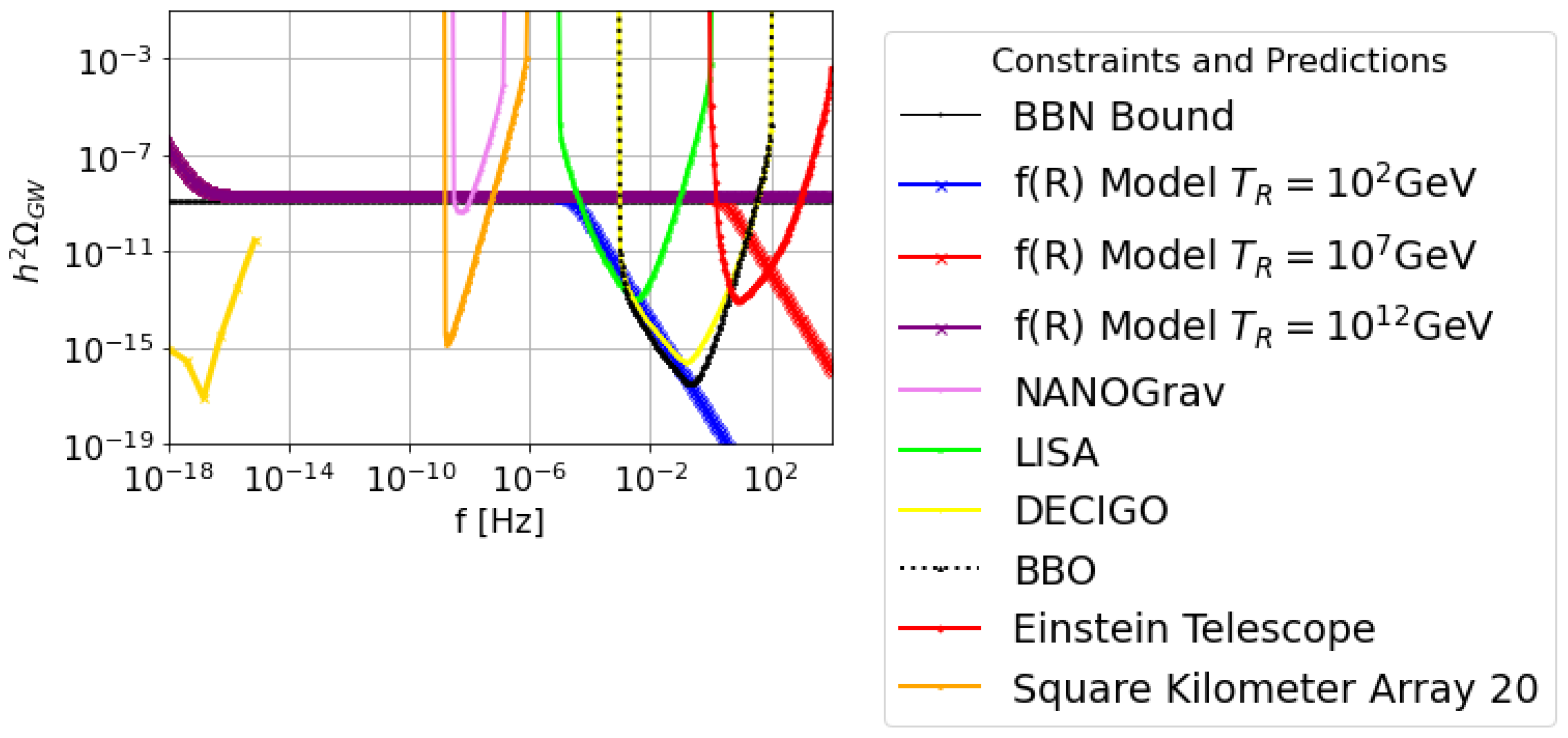
3. Primordial Gravitational Wave Energy Spectrum: The Effects of an Early Dark Energy Phase
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Niedermann, F.; Sloth, M.S. Resolving the Hubble tension with new early dark energy. Phys. Rev. D 2020, 102, 063527. [Google Scholar] [CrossRef]
- Poulin, V.; Smith, T.L.; Karwal, T.; Kamionkowski, M. Early Dark Energy Can Resolve The Hubble Tension. Phys. Rev. Lett. 2019, 122, 221301. [Google Scholar] [CrossRef] [PubMed]
- Karwal, T.; Kamionkowski, M. Dark energy at early times, the Hubble parameter, and the string axiverse. Phys. Rev. D 2016, 94, 103523. [Google Scholar] [CrossRef]
- Oikonomou, V.K. Unifying inflation with early and late dark energy epochs in axion F(R) gravity. Phys. Rev. D 2021, 103, 044036. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D.; Oikonomou, V.K. Unifying Inflation with Early and Late-time Dark Energy in F(R) Gravity. Phys. Dark Univ. 2020, 29, 100602. [Google Scholar] [CrossRef]
- Kamionkowski, M.; Kovetz, E.D. The Quest for B Modes from Inflationary Gravitational Waves. Ann. Rev. Astron. Astrophys. 2016, 54, 227. [Google Scholar] [CrossRef]
- Denissenya, M.; Linder, E.V. Gravity’s Islands: Parametrizing Horndeski Stability. J. Cosmol. Astropart. Phys. 2018, 11, 010. [Google Scholar] [CrossRef]
- Turner, M.S.; White, M.J.; Lidsey, J.E. Tensor perturbations in inflationary models as a probe of cosmology. Phys. Rev. D 1993, 48, 4613–4622. [Google Scholar] [CrossRef]
- Boyle, A.L.; Steinhardt, P.J. Probing the early universe with inflationary gravitational waves. Phys. Rev. D 2008, 77, 063504. [Google Scholar] [CrossRef]
- Zhang, Y.; Yuan, Y.; Zhao, W.; Chen, Y. Relic gravitational waves in the accelerating Universe. Class. Quant. Grav. 2005, 22, 1383. [Google Scholar] [CrossRef]
- Schutz, B.F.; Ricci, F. Gravitational Waves, Sources, and Detectors. arXiv 2010, arXiv:1005.4735. [Google Scholar]
- Sathyaprakash, S.B.; Schutz, F.B. Physics, Astrophysics and Cosmology with Gravitational Waves. Living Rev. Rel. 2009, 12, 2. [Google Scholar] [CrossRef]
- Caprini, C.; Figueroa, D.G. Cosmological Backgrounds of Gravitational Waves. Class. Quant. Grav. 2018, 35, 163001. [Google Scholar] [CrossRef]
- Arutyunov, G.; Heinze, M.; Medina-Rincon, D. Superintegrability of Geodesic Motion on the Sausage Model. J. Phys. A 2017, 50, 244002. [Google Scholar] [CrossRef]
- Kuroyanagi, S.; Chiba, T.; Sugiyama, N. Precision calculations of the gravitational wave background spectrum from inflation. Phys. Rev. D 2009, 79, 103501. [Google Scholar] [CrossRef]
- Clarke, T.J.; Copeland, E.J.; Moss, A. Constraints on primordial gravitational waves from the Cosmic Microwave Background. J. Cosmol. Astropart. Phys. 2020, 10, 002. [Google Scholar] [CrossRef]
- Kuroyanagi, S.; Takahashi, T.; Yokoyama, S. Blue-tilted Tensor Spectrum and Thermal History of the Universe. J. Cosmol. Astropart. Phys. 2015, 2, 003. [Google Scholar] [CrossRef]
- Nakayama, K.; Yokoyama, J. Gravitational Wave Background and Non-Gaussianity as a Probe of the Curvaton Scenario. J. Cosmol. Astropart. Phys. 2010, 1, 010. [Google Scholar] [CrossRef]
- Smith, T.L.; Kamionkowski, M.; Cooray, A. Direct detection of the inflationary gravitational wave background. Phys. Rev. D 2006, 73, 023504. [Google Scholar] [CrossRef]
- Giovannini, M. Thermal history of the plasma and high-frequency Gravitons. Class. Quant. Grav. 2009, 26, 045004. [Google Scholar] [CrossRef]
- Liu, X.J.; Zhao, W.; Zhang, Y.; Zhu, Z.H. Detecting Relic Gravitational Waves by Pulsar Timing Arrays: Effects of Cosmic Phase Transitions and Relativistic Free-Streaming Gases. Phys. Rev. D 2016, 93, 024031. [Google Scholar] [CrossRef]
- Zhao, W.; Zhang, Y.; You, X.P.; Zhu, Z.H. Constraints of relic gravitational waves by pulsar timing arrays: Forecasts for the FAST and SKA projects. Phys. Rev. D 2013, 87, 124012. [Google Scholar] [CrossRef]
- Vagnozzi, S. Implications of the NANOGrav results for inflation. Mon. Not. R. Astron. Soc. 2021, 502, L11–L15. [Google Scholar] [CrossRef]
- Watanabe, Y.; Komatsu, E. Improved Calculation of the Primordial Gravitational Wave Spectrum in the Standard Model. Phys. Rev. D 2006, 73, 123515. [Google Scholar] [CrossRef]
- Kamionkowski, M.; Kosowsky, A.; Turner, M.S. Gravitational radiation from first order phase transitions. Phys. Rev. D 1994, 49, 2837–2851. [Google Scholar] [CrossRef]
- Giarè, W.; Renzi, F. Propagating speed of primordial gravitational waves. Phys. Rev. D 2020, 102, 083530. [Google Scholar] [CrossRef]
- Kuroyanagi, S.; Takahashi, T.; Yokoyama, S. Blue-tilted inflationary tensor spectrum and reheating in the light of NANOGrav results. J. Cosmol. Astropart. Phys. 2021, 1, 071. [Google Scholar] [CrossRef]
- Zhao, W.; Zhang, Y. Relic gravitational waves and their detection. Phys. Rev. D 2006, 74, 043503. [Google Scholar] [CrossRef]
- Nishizawa, A. Generalized framework for testing gravity with gravitational-wave propagation. I. Formulation. Phys. Rev. D 2018, 97, 104037. [Google Scholar] [CrossRef]
- Arai, S.; Nishizawa, A. Generalized framework for testing gravity with gravitational-wave propagation. II. Constraints on Horndeski theory. Phys. Rev. D 2018, 97, 104038. [Google Scholar] [CrossRef]
- Bellini, E.; Sawicki, I. Maximal freedom at minimum cost: Linear large-scale structure in general modifications of gravity. J. Cosmol. Astropart. Phys. 2014, 7, 050. [Google Scholar] [CrossRef]
- Nunes, C.R.; Alves, S.E.M.; de Araujo, N.C.J. Primordial gravitational waves in Horndeski gravity. Phys. Rev. D 2019, 99, 084022. [Google Scholar] [CrossRef]
- D’Agostino, R.; Nunes, R.C. Probing observational bounds on scalar-tensor theories from standard sirens. Phys. Rev. D 2019, 100, 044041. [Google Scholar] [CrossRef]
- Mitra, A.; Mifsud, J.; Mota, D.F.; Parkinson, D. Cosmology with the Einstein Telescope: No Slip Gravity Model and Redshift Specifications. Mon. Not. R. Astron. Soc. 2021, 502, 5563–5575. [Google Scholar] [CrossRef]
- Kuroyanagi, S.; Nakayama, K.; Saito, S. Prospects for determination of thermal history after inflation with future gravitational wave detectors. Phys. Rev. D 2011, 84, 123513. [Google Scholar] [CrossRef]
- Campeti, P.; Komatsu, E.; Poletti, D.; Baccigalupi, C. Measuring the spectrum of primordial gravitational waves with CMB, PTA and Laser Interferometers. J. Cosmol. Astropart. Phys. 2021, 1, 012. [Google Scholar] [CrossRef]
- Nishizawa, A.; Motohashi, H. Constraint on reheating after f(R) inflation from gravitational waves. Phys. Rev. D 2014, 89, 063541. [Google Scholar] [CrossRef]
- Zhao, W. Improved calculation of relic gravitational waves. Chin. Phys. 2007, 16, 2894–2902. [Google Scholar] [CrossRef][Green Version]
- Cheng, W.; Qian, T.; Yu, Q.; Zhou, H.; Zhou, Y.R. Gravitational Wave from Axion-like Particle Inflation. arXiv 2021, arXiv:2107.04242. [Google Scholar]
- Nishizawa, A.; Yagi, K.; Taruya, A.; Tanaka, T. Cosmology with space-based gravitational-wave detectors—Dark energy and primordial gravitational waves. Phys. Rev. D 2012, 85, 044047. [Google Scholar] [CrossRef]
- Chongchitnan, S.; Efstathiou, G. Prospects for direct detection of primordial gravitational waves. Phys. Rev. D 2006, 73, 083511. [Google Scholar] [CrossRef]
- Lasky, P.D.; Mingarelli, C.M.F.; Smith, T.L.; Giblin, J.T.; Reardon, D.J.; Caldwell, R.; Bailes, M.; Bhat, N.D.R.; Burke-Spolaor, S.; Coles, W.; et al. Gravitational-wave cosmology across 29 decades in frequency. Phys. Rev. X 2016, 6, 011035. [Google Scholar] [CrossRef]
- Guzzetti, M.C.; Bartolo, N.; Liguori, M.; Matarrese, S. Gravitational waves from inflation. Riv. Nuovo Cim. 2016, 39, 399–495. [Google Scholar] [CrossRef]
- Ben-Dayan, I.; Keating, B.; Leon, D.; Wolfson, I. Constraints on scalar and tensor spectra from Neff. J. Cosmol. Astropart. Phys. 2019, 6, 007. [Google Scholar] [CrossRef]
- Nakayama, K.; Saito, S.; Suwa, Y.; Yokoyama, J. Probing reheating temperature of the universe with gravitational wave background. J. Cosmol. Astropart. Phys. 2008, 6, 020. [Google Scholar] [CrossRef]
- Capozziello, S.; De Laurentis, M.; Nojiri, S.; Odintsov, S.D. Evolution of gravitons in accelerating cosmologies: The case of extended gravity. Phys. Rev. D 2017, 95, 083524. [Google Scholar] [CrossRef]
- Capozziello, S.; De Laurentis, M.; Nojiri, S.; Odintsov, S.D. f(R) gravity constrained by PPN parameters and stochastic background of gravitational waves. Gen. Rel. Grav. 2009, 41, 2313–2344. [Google Scholar] [CrossRef]
- Capozziello, S.; Corda, C.; De Laurentis, F.M. Massive gravitational waves from f(R) theories of gravity: Potential detection with LISA. Phys. Lett. B 2008, 669, 255–259. [Google Scholar] [CrossRef]
- Cai, R.G.; Fu, C.; Yu, W.W. Parity violation in stochastic gravitational wave background from inflation. arXiv 2021, arXiv:2112.04794. [Google Scholar]
- Cai, R.G.; Pi, S.; Sasaki, M. Gravitational Waves Induced by non-Gaussian Scalar Perturbations. Phys. Rev. Lett. 2019, 122, 201101. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K.; Fronimos, F.P. Quantitative predictions for f(R) gravity primordial gravitational waves. Phys. Dark Univ. 2022, 35, 100950. [Google Scholar] [CrossRef]
- Benetti, M.; Graef, L.L.; Vagnozzi, S. Primordial gravitational waves from NANOGrav: A broken power-law approach. Phys. Rev. D 2022, 105, 043520. [Google Scholar] [CrossRef]
- Lin, J.; Gao, S.; Gong, Y.; Lu, Y.; Wang, Z.; Zhang, F. Primordial black holes and scalar induced secondary gravitational waves from Higgs inflation with non-canonical kinetic term. arXiv 2021, arXiv:2111.01362. [Google Scholar]
- Zhang, F.; Lin, J.; Lu, Y. Double-peaked inflation model: Scalar induced gravitational waves and primordial-black-hole suppression from primordial non-Gaussianity. Phys. Rev. D 2021, 104, 063515. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K. Pre-inflationary bounce effects on primordial gravitational waves of f(R) gravity. Phys. Lett. B 2022, 824, 136817. [Google Scholar] [CrossRef]
- Pritchard, J.R.; Kamionkowski, M. Cosmic microwave background fluctuations from gravitational waves: An Analytic approach. Ann. Phys. 2005, 318, 2–36. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhao, W.; Xia, T.; Yuan, Y. Analytic approach to the CMB polarizations generated by relic gravitational waves. Phys. Rev. D 2006, 74, 083006. [Google Scholar] [CrossRef]
- Baskaran, D.; Grishchuk, L.P.; Polnarev, A.G. Imprints of Relic Gravitational Waves in Cosmic Microwave Background Radiation. Phys. Rev. D 2006, 74, 083008. [Google Scholar] [CrossRef]
- Oikonomou, V.K. Primordial gravitational waves predictions for GW170817-compatible Einstein–Gauss–Bonnet theory. Astropart. Phys. 2022, 141, 102718. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K.; Myrzakulov, R. Spectrum of Primordial Gravitational Waves in Modified Gravities: A Short Overview. Symmetry 2022, 14, 729. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K. Amplification of Primordial Gravitational Waves by a Geometrically Driven non-canonical Reheating Era. arXiv 2022, arXiv:2203.10599. [Google Scholar] [CrossRef]
- Baker, J.; Bellovary, J.; Bender, P.L.; Berti, E.; Caldwell, R.; Camp, J.; Conklin, J.W.; Cornish, N.; Cutler, C.; DeRosa, R.; et al. The Laser Interferometer Space Antenna: Unveiling the Millihertz Gravitational Wave Sky. arXiv 2019, arXiv:1907.06482. [Google Scholar]
- Smith, T.L.; Caldwell, R. LISA for Cosmologists: Calculating the Signal-to-Noise Ratio for Stochastic and Deterministic Sources. Phys. Rev. D 2019, 100, 104055. [Google Scholar] [CrossRef]
- Seto, N.; Kawamura, S.; Nakamura, T. Possibility of direct measurement of the acceleration of the universe using 0.1-Hz band laser interferometer gravitational wave antenna in space. Phys. Rev. Lett. 2001, 87, 221103. [Google Scholar] [CrossRef]
- Kawamura, S.; Ando, M.; Seto, N.; Sato, S.; Musha, M.; Kawano, I.; Yokoyama, J.; Tanaka, T.; Ioka, K.; Akutsu, T.; et al. Current status of space gravitational wave antenna DECIGO and B-DECIGO. arXiv 2020, arXiv:2006.13545. [Google Scholar] [CrossRef]
- Hild, S.; Abernathy, M.; Acernese, F.; Amaro-Seoane, P.; Andersson, N.; Arun, K.; Barone, F.; Barr, B.; Barsuglia, M.; Beker, M.; et al. Sensitivity Studies for Third-Generation Gravitational Wave Observatories. Class. Quant. Grav. 2011, 28, 094013. [Google Scholar] [CrossRef]
- Crowder, J.; Cornish, J.N. Beyond LISA: Exploring future gravitational wave missions. Phys. Rev. D 2005, 72, 083005. [Google Scholar] [CrossRef]
- Smith, T.L.; Caldwell, R. Sensitivity to a Frequency-Dependent Circular Polarization in an Isotropic Stochastic Gravitational Wave Background. Phys. Rev. D 2017, 95, 044036. [Google Scholar] [CrossRef]
- Weltman, A.; Bull, P.; Camera, S.; Kelley, K.; Padmanabhan, H.; Pritchard, J.; Raccanelli, A.; Riemer-Sørensen, S.; Shao, L.; Andrianomena, S.; et al. Fundamental physics with the Square Kilometre Array. Publ. Astron. Soc. Austral. 2020, 37, e002. [Google Scholar] [CrossRef]
- Arzoumanian, Z.; Baker, P.T.; Blumer, H.; Bécsy, B.; Brazier, A.; Brook, P.R.; Burke-Spolaor, S.; Chatterjee, S.; Chen, S.; Cordes, J.M.; et al. The NANOGrav 12.5 yr Data Set: Search for an Isotropic Stochastic Gravitational-wave Background. Astrophys. J. Lett. 2020, 905, L34. [Google Scholar] [CrossRef]
- Pol, N.S.; Taylor, S.R.; Kelley, L.Z.; Vigeland, S.J.; Simon, J.; Chen, S.; Arzoumanian, Z.; Baker, P.T.; Bécsy, B.; Brazier, A.; et al. Astrophysics Milestones for Pulsar Timing Array Gravitational Wave Detection. arXiv 2020, arXiv:2010.11950. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D.; Saez-Gomez, D. Cosmological reconstruction of realistic modified F(R) gravities. Phys. Lett. B 2009, 681, 74. [Google Scholar] [CrossRef]
- Nojiri SOdintsov SD Oikonomou, V.K. Modified Gravity Theories on a Nutshell: Inflation, Bounce and Late-time Evolution. Phys. Rept. 2017, 692, 1. [Google Scholar] [CrossRef]
- Odintsov, S.D.; Oikonomou, V.K. Inflationary attractors in F(R) gravity. Phys. Lett. B 2020, 807, 135576. [Google Scholar] [CrossRef]
- Garcia-Bellido, J. Astrophysics and cosmology. arXiv 1999, arXiv:hep-ph/0004188. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oikonomou, V.K.; Lymperiadou, E.C. Effects of a Geometrically Realized Early Dark Energy Era on the Spectrum of Primordial Gravitational Waves. Symmetry 2022, 14, 1143. https://doi.org/10.3390/sym14061143
Oikonomou VK, Lymperiadou EC. Effects of a Geometrically Realized Early Dark Energy Era on the Spectrum of Primordial Gravitational Waves. Symmetry. 2022; 14(6):1143. https://doi.org/10.3390/sym14061143
Chicago/Turabian StyleOikonomou, Vasilis K., and Eirini C. Lymperiadou. 2022. "Effects of a Geometrically Realized Early Dark Energy Era on the Spectrum of Primordial Gravitational Waves" Symmetry 14, no. 6: 1143. https://doi.org/10.3390/sym14061143
APA StyleOikonomou, V. K., & Lymperiadou, E. C. (2022). Effects of a Geometrically Realized Early Dark Energy Era on the Spectrum of Primordial Gravitational Waves. Symmetry, 14(6), 1143. https://doi.org/10.3390/sym14061143






