Nonlinear Optical Potential with Parity-Time Symmetry in a Coherent Atomic Gas
Abstract
:1. Introduction
2. Model and the Motion Equation for the Scheme
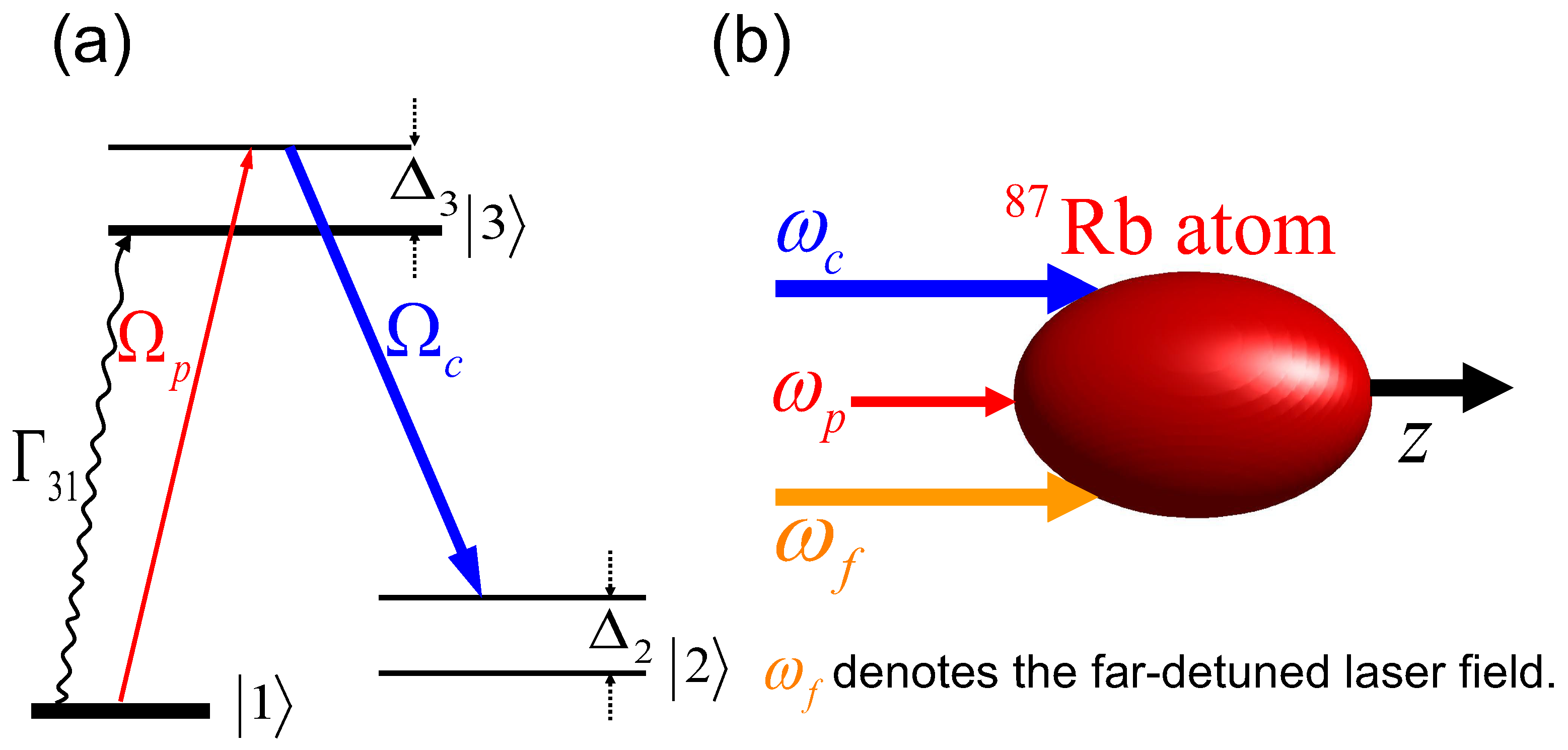
2.1. Model
2.2. Maxwell-Bloch Equations
3. Realization of -Symmetric Potential
3.1. The Probe Field Envelope Equation
3.2. The Design of Symmetric Potential
4. EP and Soliton Solutions
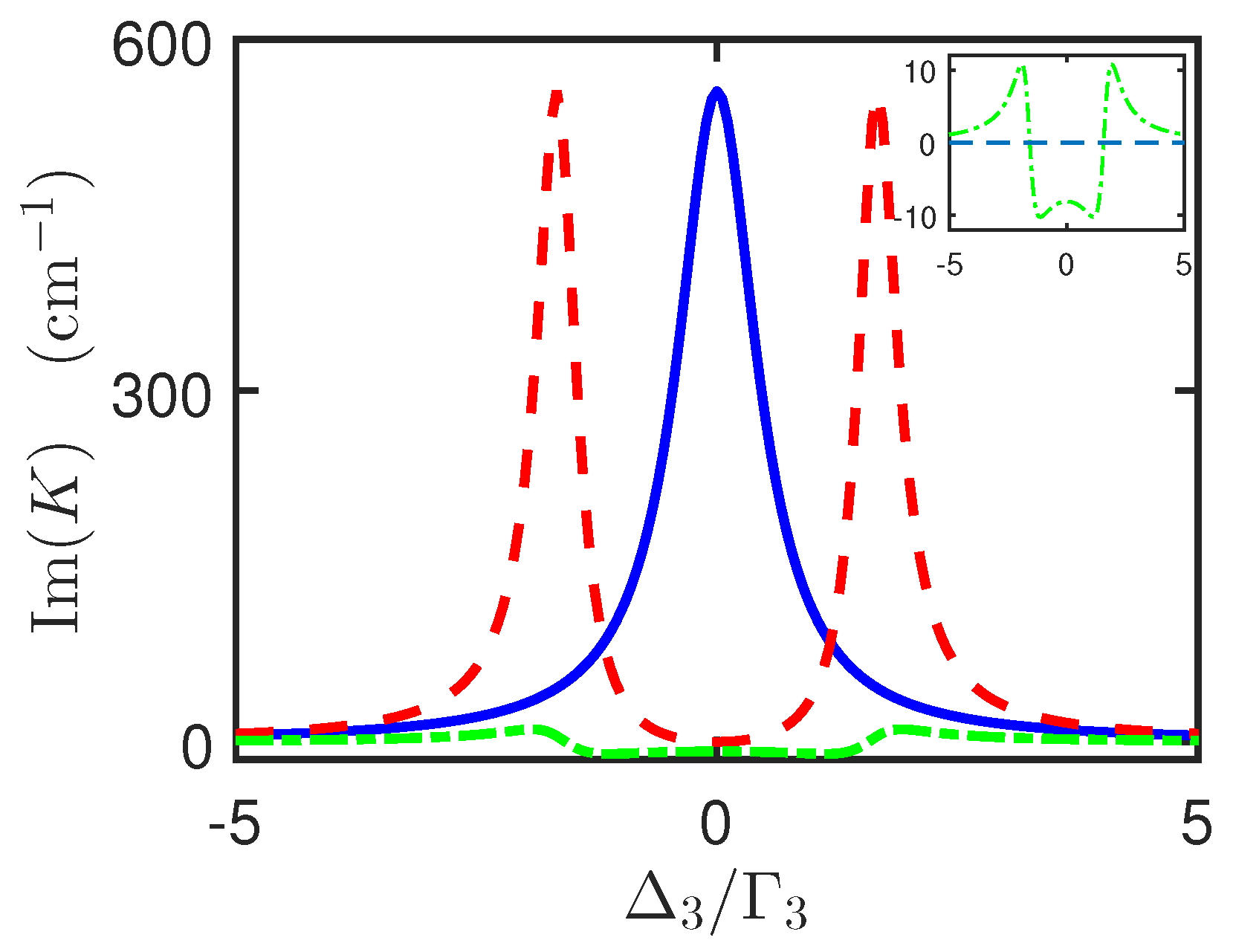
4.1. Property of Linear -Symmetric Potential
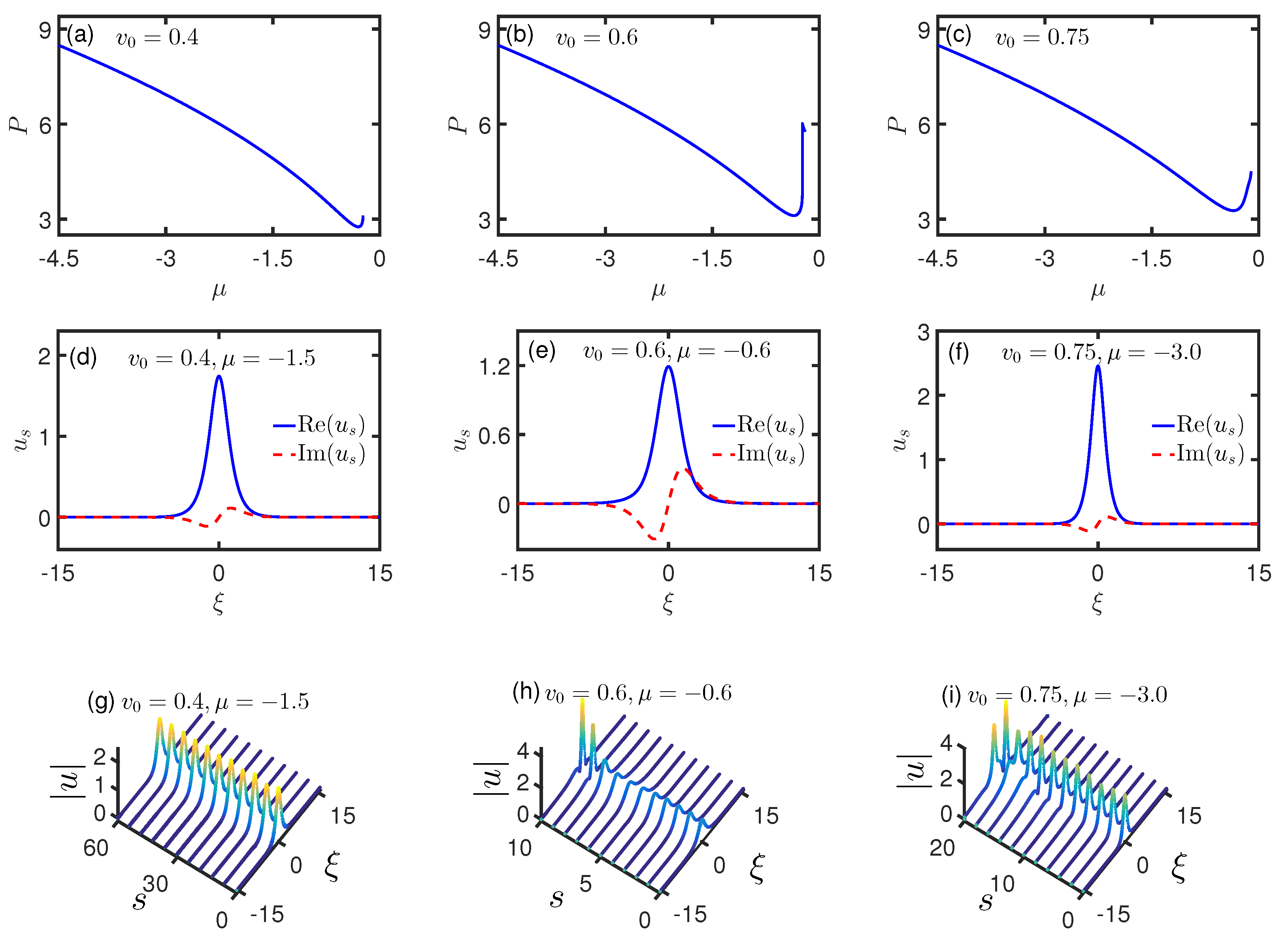
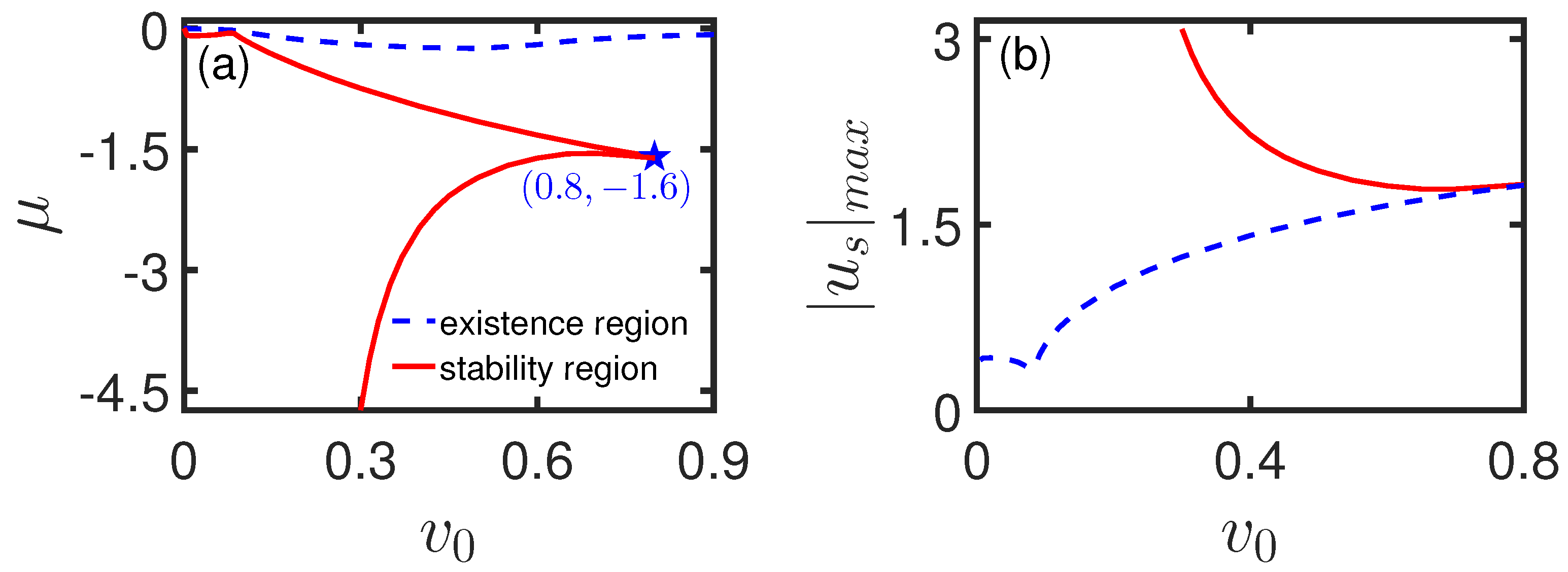
4.2. Property of Nonlinear -Symmetric Potential
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Explicit Expression of Equation (3)
Appendix B. Perturbation Expansion of the MB Equations
References
- Bender, C.M. Making sense of non-Hermitian Hamiltonians. Rep. Prog. Phys. 2008, 70, 947–1018. [Google Scholar] [CrossRef] [Green Version]
- Makris, K.G.; El-Ganainy, R.; Christodoulides, D.N.; Musslimani, Z.H. PT symmetric periodic optical potentials. Int. J. Theor. Phys. 2011, 50, 1019–1041. [Google Scholar] [CrossRef]
- Bittner, S.; Dietz, B.; Günther, U.; Harney, H.L.; Miski-Oglu, M.; Richter, A.; Schäfer, F. PT symmetry and spontaneous symmetry breaking in a microwave billiard. Phys. Rev. Lett. 2012, 108, 024101. [Google Scholar] [CrossRef] [Green Version]
- Rüter, C.E.; Makris, K.R.; El-Ganainy, R.; Christodoulides, D.N.; Segev, M.; Kip, D. Observation of parity-time symmetry in optics. Nat. Phys. 2010, 6, 192–195. [Google Scholar] [CrossRef] [Green Version]
- Regensburger, A.; Bersch, C.; Miri, M.-A.; Onishchukov, G.; Christodoulides, D.N.; Peschel, U. Parity-time synthetic photonic lattices. Nature 2012, 488, 167–171. [Google Scholar] [CrossRef]
- Feng, L.; Xu, Y.-L.; Fegadolli, W.S.; Lu, M.-H.; Oliveira, J.E.B.; Almeida, V.R.; Chen, Y.-F.; Scherer, A. Experimental demonstration of a unidirectional reflectionless parity-time metamaterial at optical frequencies. Nat. Mat. 2013, 12, 108–113. [Google Scholar] [CrossRef]
- Peng, B.; Özdemir, S.K.; Lei, F.; Monifi, F.; Gianfreda, M.; Long, G.L.; Fan, S.; Nori, F.; Bender, C.M.; Yang, L. Parity-time-symmetric whispering-gallery microcavities. Nat. Phys. 2014, 10, 394. [Google Scholar] [CrossRef] [Green Version]
- Chang, L.; Jiang, X.; Hua, S.; Yang, C.; Wen, J.; Jiang, L.; Li, G.; Wang, G.; Xiao, M. Parity-time symmetry and variable optical isolation in active-passive-coupled microresonators. Nat. Photon. 2014, 8, 524. [Google Scholar] [CrossRef]
- Guo, A.; Salamo, G.J.; Duchesne, D.; Morandotti, R.; Volatier-Ravat, M.; Aimez, V.; Sililoglou, G.A.; Christodoulides, D.N. Observation of PT-symmetry breaking in complex optical potentials. Phys. Rev. Lett. 2009, 103, 093902. [Google Scholar] [CrossRef] [Green Version]
- Sun, Y.; Tan, W.; Li, H.; Li, J.; Chen, H. Experimental demonstration of a coherent perfect absorber with PT phase transition. Phys. Rev. Lett. 2014, 112, 143903. [Google Scholar] [CrossRef]
- Lawrence, M.; Xu, N.; Zhang, X.; Cong, L.; Han, J.; Zhang, W.; Zhang, S. Manifestation of PT symmetry breaking in polarization space with terahertz metasurfaces. Phys. Rev. Lett. 2014, 113, 093901. [Google Scholar] [CrossRef] [Green Version]
- Benisty, H.; Degiron, A.; Lupu, A.; De Lustrac, A.; Cheńais, S.; Forget, S.; Besbes, M.; Barbillon, G.; Bruyant, A.; Blaize, S.; et al. Implementation of PT symmetric devices using plasmonics: Principle and applications. Opt. Express 2011, 19, 18004–18019. [Google Scholar] [CrossRef] [Green Version]
- Schindler, J.; Li, A.; Zheng, M.C.; Ellis, F.M.; Kottos, T. Experimental study of active LRC circuits with PT symmetries. Phys. Rev. A 2011, 84, 040101(R). [Google Scholar] [CrossRef] [Green Version]
- Lin, Z.; Ramezani, H.; Eichelkraut, T.; Kottos, T.; Cao, H.; Christodoulides, D.N. Unidirectional invisibility induced by PT-symmetric periodic structures. Phys. Rev. Lett. 2011, 106, 213901. [Google Scholar] [CrossRef] [Green Version]
- Feng, L.; Wong, Z.J.; Ma, R.; Wang, Y.; Zhang, X. Single-mode laser by parity-time symmetry breaking. Science 2014, 346, 972. [Google Scholar] [CrossRef]
- Hodaei, H.; Miri, M.; Heinrich, M.; Christodoulides, D.N.; Khajavikhan, M. Parity-time-symmetric microring laser. Science 2014, 346, 975. [Google Scholar] [CrossRef]
- Hodaei, H.; Hassan, A.U.; Wittek, S.; Garcia-Gracia, H.; El-Ganainy, R.; Christodoulides, D.N.; Khajavikhan, M. Enhanced sensitivity at higher-order exceptional points. Nature 2017, 548, 187. [Google Scholar] [CrossRef]
- Chen, W.; Özdemir, Ş.K.; Zhao, G.; Wiersig, J.; Yang, L. Exceptional points enhance sensing in an optical microcavity. Nature 2017, 548, 192. [Google Scholar] [CrossRef]
- Hang, C.; Huang, G.; Konotop, V.V. PT symmetry with a system of three-level atoms. Phys. Rev. Lett. 2013, 110, 083604. [Google Scholar] [CrossRef] [Green Version]
- Sheng, J.; Miri, M.; Christodoulides, D.N.; Xiao, M. PT-symmetric optical potentials in a coherent atomic medium. Phys. Rev. A 2013, 88, 041803(R). [Google Scholar] [CrossRef] [Green Version]
- Li, H.; Dou, J.; Huang, G. PT symmetry via electromagnetically induced transparency. Opt. Express 2013, 21, 320503. [Google Scholar] [CrossRef] [Green Version]
- Demtröder, W. Laser Spectroscopy: Basic Concepts and Instrumentation, 3rd ed.; Springer: Berlin, Germany, 2003; Chapter 10. [Google Scholar]
- Huang, G.; Deng, L.; Payne, M.G. Dynamics of ultraslow optical solitons in a cold three-state atomic system. Phys. Rev. E 2005, 72, 016617. [Google Scholar] [CrossRef]
- Li, H.-j.; Wu, Y.-p.; Huang, G. Stable weak-light ultraslow spatiotemporal solitons via atomic coherence. Phys. Rev. A 2011, 84, 033816. [Google Scholar] [CrossRef]
- Yang, J. Numerical methods for nonlinear wave equations. In Nonlinear Waves in Integrable and Nonintegrable Systems; Haberman, R., Ed.; SIAM: Philadephia, PA, USA, 2011; Chapter 7. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Polanco Adames, D.A.; Dou, J.; Lin, J.; Zhu, G.; Li, H. Nonlinear Optical Potential with Parity-Time Symmetry in a Coherent Atomic Gas. Symmetry 2022, 14, 1135. https://doi.org/10.3390/sym14061135
Polanco Adames DA, Dou J, Lin J, Zhu G, Li H. Nonlinear Optical Potential with Parity-Time Symmetry in a Coherent Atomic Gas. Symmetry. 2022; 14(6):1135. https://doi.org/10.3390/sym14061135
Chicago/Turabian StylePolanco Adames, Delvi Antonio, Jianpeng Dou, Ji Lin, Gengjun Zhu, and Huijun Li. 2022. "Nonlinear Optical Potential with Parity-Time Symmetry in a Coherent Atomic Gas" Symmetry 14, no. 6: 1135. https://doi.org/10.3390/sym14061135
APA StylePolanco Adames, D. A., Dou, J., Lin, J., Zhu, G., & Li, H. (2022). Nonlinear Optical Potential with Parity-Time Symmetry in a Coherent Atomic Gas. Symmetry, 14(6), 1135. https://doi.org/10.3390/sym14061135





