Resummed Relativistic Dissipative Hydrodynamics
Abstract
:1. Introduction
2. Resummed Dissipative Hydrodynamics in the Conformal Bjorken Limit
Relation to Second-Order Viscous Hydrodynamics in the Small Anisotropy Limit
3. Quasiparticle Anisotropic Hydrodynamics
4. The Equation of State for aHydroQP
5. Evolution and Freezeout in aHydroQP
6. Results and Discussion
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Averbeck, R.; Harris, J.W.; Schenke, B. Heavy-Ion Physics at the LHC. In The Large Hadron Collider: Harvest of Run 1; Schörner-Sadenius, T., Ed.; Springer International Publishing: Cham, Switzerland, 2015; pp. 355–420. [Google Scholar]
- Wang, X.N. (Ed.) Quark-Gluon Plasma 5; World Scientific: Singapore, 2016. [Google Scholar]
- Dexheimer, V.; Noronha, J.; Noronha-Hostler, J.; Ratti, C.; Yunes, N. Future physics perspectives on the equation of state from heavy ion collisions to neutron stars. J. Phys. G 2021, 48, 073001. [Google Scholar] [CrossRef]
- Bazavov, A. An overview of (selected) recent results in finite-temperature lattice QCD. J. Phys. Conf. Ser. 2013, 446, 012011. [Google Scholar] [CrossRef] [Green Version]
- Borsanyi, S. Frontiers of finite temperature lattice QCD. EPJ Web Conf. 2017, 137, 01006. [Google Scholar] [CrossRef] [Green Version]
- Cea, P.; Cosmai, L.; Papa, A. Critical line of 2+1 flavor QCD: Toward the continuum limit. Phys. Rev. D 2016, 93, 014507. [Google Scholar] [CrossRef] [Green Version]
- Bonati, C.; D’Elia, M.; Mariti, M.; Mesiti, M.; Negro, F.; Sanfilippo, F. Curvature of the chiral pseudocritical line in QCD: Continuum extrapolated results. Phys. Rev. D 2015, 92, 054503. [Google Scholar] [CrossRef] [Green Version]
- Bonati, C.; D’Elia, M.; Negro, F.; Sanfilippo, F.; Zambello, K. Curvature of the pseudocritical line in QCD: Taylor expansion matches analytic continuation. Phys. Rev. D 2018, 98, 054510. [Google Scholar] [CrossRef] [Green Version]
- Bonati, C.; D’Elia, M.; Mariti, M.; Mesiti, M.; Negro, F.; Sanfilippo, F. Curvature of the chiral pseudocritical line in QCD. Phys. Rev. D 2014, 90, 114025. [Google Scholar] [CrossRef] [Green Version]
- Borsanyi, S.; Fodor, Z.; Guenther, J.N.; Kara, R.; Katz, S.D.; Parotto, P.; Pasztor, A.; Ratti, C.; Szabo, K.K. QCD Crossover at Finite Chemical Potential from Lattice Simulations. Phys. Rev. Lett. 2020, 125, 052001. [Google Scholar] [CrossRef]
- Bazavov, A.; Ding, H.T.; Hegde, P.; Kaczmarek, O.; Karsch, F.; Karthik, N.; Laermann, E.; Lahiri, A.; Larsen, R.; Li, S.T.; et al. Chiral crossover in QCD at zero and non-zero chemical potentials. Phys. Lett. B 2019, 795, 15–21. [Google Scholar] [CrossRef]
- Toublan, D.; Kogut, J.B. The QCD phase diagram at nonzero baryon, isospin and strangeness chemical potentials: Results from a hadron resonance gas model. Phys. Lett. B 2005, 605, 129–136. [Google Scholar] [CrossRef] [Green Version]
- Endrodi, G.; Fodor, Z.; Katz, S.D.; Szabo, K.K. The QCD phase diagram at nonzero quark density. J. High Energy Phys. 2011, 4, 1–4. [Google Scholar] [CrossRef] [Green Version]
- Bellwied, R.; Borsanyi, S.; Fodor, Z.; Günther, J.; Katz, S.D.; Ratti, C.; Szabo, K.K. The QCD phase diagram from analytic continuation. Phys. Lett. B 2015, 751, 559–564. [Google Scholar] [CrossRef] [Green Version]
- Berges, J.; Borsányi, S. Range of validity of transport equations. Phys. Rev. D 2006, 74, 045022. [Google Scholar] [CrossRef] [Green Version]
- Haque, N.; Strickland, M. Next-to-next-to leading-order hard-thermal-loop perturbation-theory predictions for the curvature of the QCD phase transition line. Phys. Rev. C 2021, 103, 031901. [Google Scholar] [CrossRef]
- Huovinen, P.; Petreczky, P. QCD Equation of State and Hadron Resonance Gas. Nucl. Phys. A 2010, 837, 26–53. [Google Scholar] [CrossRef] [Green Version]
- Alqahtani, M.; Nopoush, M.; Strickland, M. Quasiparticle equation of state for anisotropic hydrodynamics. Phys. Rev. C 2015, 92, 54910. [Google Scholar] [CrossRef] [Green Version]
- Parotto, P.; Bluhm, M.; Mroczek, D.; Nahrgang, M.; Noronha-Hostler, J.; Rajagopal, K.; Ratti, C.; Schäfer, T.; Stephanov, M. QCD equation of state matched to lattice data and exhibiting a critical point singularity. Phys. Rev. C 2020, 101, 34901. [Google Scholar] [CrossRef] [Green Version]
- Jeon, S.; Heinz, U. Introduction to Hydrodynamics. In Quark-Gluon Plasma 5; Wang, X.N., Ed.; World Scientific: Singapore, 2016; pp. 131–187. [Google Scholar]
- Borsányi, S.; Endrődi, G.; Fodor, Z.; Jakovác, A.; Katz, S.D.; Krieg, S.; Ratti, C.; Szabó, K.K. The QCD equation of state with dynamical quarks. J. High Energy Phys. 2010, 2010, 1–33. [Google Scholar] [CrossRef] [Green Version]
- Romatschke, P.; Romatschke, U. Relativistic Fluid Dynamics in and out of Equilibrium; Cambridge Monographs on Mathematical Physics, Cambridge University Press: Cambridge, UK, 2019. [Google Scholar]
- Huovinen, P.; Kolb, P.; Heinz, U.; Ruuskanen, P.; Voloshin, S. Radial and elliptic flow at RHIC: Further predictions. Phys. Lett. B 2001, 503, 58–64. [Google Scholar] [CrossRef] [Green Version]
- Hirano, T.; Tsuda, K. Collective flow and two pion correlations from a relativistic hydrodynamic model with early chemical freeze out. Phys. Rev. 2002, 66, 054905. [Google Scholar] [CrossRef] [Green Version]
- Kolb, P.F.; Heinz, U.W. Hydrodynamic description of ultrarelativistic heavy ion collisions. In Quark-Gluon Plasma 3; World Scientific: Singapore, 2003; pp. 634–714. [Google Scholar]
- Danielewicz, P.; Gyulassy, M. Dissipative Phenomena in Quark Gluon Plasmas. Phys. Rev. D 1985, 31, 53–62. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Policastro, G.; Son, D.T.; Starinets, A.O. The Shear viscosity of strongly coupled N=4 supersymmetric Yang–Mills plasma. Phys. Rev. Lett. 2001, 87, 081601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Muronga, A. Second order dissipative fluid dynamics for ultrarelativistic nuclear collisions. Phys. Rev. Lett. 2002, 88, 062302, Erratum in Phys. Rev. Lett. 2002, 89, 159901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Muronga, A. Causal theories of dissipative relativistic fluid dynamics for nuclear collisions. Phys. Rev. C 2004, 69, 034903. [Google Scholar] [CrossRef] [Green Version]
- Muronga, A.; Rischke, D.H. Evolution of hot, dissipative quark matter in relativistic nuclear collisions. arXiv 2004, arXiv:nucl-th/0407114. [Google Scholar]
- Heinz, U.W.; Song, H.; Chaudhuri, A.K. Dissipative hydrodynamics for viscous relativistic fluids. Phys. Rev. C 2006, 73, 034904. [Google Scholar] [CrossRef] [Green Version]
- Baier, R.; Romatschke, P.; Wiedemann, U.A. Dissipative hydrodynamics and heavy ion collisions. Phys. Rev. C 2006, 73, 064903. [Google Scholar] [CrossRef] [Green Version]
- Romatschke, P.; Romatschke, U. Viscosity Information from Relativistic Nuclear Collisions: How Perfect is the Fluid Observed at RHIC? Phys. Rev. Lett. 2007, 99, 172301. [Google Scholar] [CrossRef] [Green Version]
- Baier, R.; Romatschke, P.; Son, D.T.; Starinets, A.O.; Stephanov, M.A. Relativistic viscous hydrodynamics, conformal invariance, and holography. J. High Energy Phys. 2008, 4, 100. [Google Scholar] [CrossRef]
- Denicol, G.S.; Niemi, H.; Molnar, E.; Rischke, D.H. Derivation of transient relativistic fluid dynamics from the Boltzmann equation. Phys. Rev. D 2015, 85, 114047, Erratum in Phys. Rev. D 2015, 91, 039902. [Google Scholar] [CrossRef] [Green Version]
- Denicol, G.S.; Molnár, E.; Niemi, H.; Rischke, D.H. Derivation of fluid dynamics from kinetic theory with the 14-moment approximation. Eur. Phys. J. A 2012, 48, 170. [Google Scholar] [CrossRef] [Green Version]
- Jaiswal, A. Relativistic dissipative hydrodynamics from kinetic theory with relaxation time approximation. Phys. Rev. C 2013, 87, 051901. [Google Scholar] [CrossRef]
- Jaiswal, A. Relativistic third-order dissipative fluid dynamics from kinetic theory. Phys. Rev. C 2013, 88, 021903. [Google Scholar] [CrossRef] [Green Version]
- Calzetta, E. Hydrodynamic approach to boost invariant free streaming. Phys. Rev. D 2015, 92, 045035. [Google Scholar] [CrossRef] [Green Version]
- Denicol, G.S.; Jeon, S.; Gale, C. Transport Coefficients of Bulk Viscous Pressure in the 14-moment approximation. Phys. Rev. C 2014, 90, 024912. [Google Scholar] [CrossRef] [Green Version]
- Denicol, G.S.; Florkowski, W.; Ryblewski, R.; Strickland, M. Shear-bulk coupling in nonconformal hydrodynamics. Phys. Rev. C 2014, 90, 044905. [Google Scholar] [CrossRef] [Green Version]
- Jaiswal, A.; Ryblewski, R.; Strickland, M. Transport coefficients for bulk viscous evolution in the relaxation time approximation. Phys. Rev. C 2014, 90, 044908. [Google Scholar] [CrossRef] [Green Version]
- Bemfica, F.S.; Disconzi, M.M.; Noronha, J. General-Relativistic Viscous Fluid Dynamics. arXiv 2020, arXiv:2009.11388. [Google Scholar]
- Hoult, R.E.; Kovtun, P. Stable and causal relativistic Navier–Stokes equations. J. High Energy Phys. 2020, 6, 1–5. [Google Scholar] [CrossRef]
- Heinz, U.W.; Bazow, D.; Strickland, M. Viscous hydrodynamics for strongly anisotropic expansion. Nucl. Phys. A 2014, 931, 920–925. [Google Scholar] [CrossRef] [Green Version]
- Florkowski, W.; Ryblewski, R. Highly-anisotropic and strongly-dissipative hydrodynamics for early stages of relativistic heavy-ion collisions. Phys. Rev. C 2011, 83, 034907. [Google Scholar] [CrossRef]
- Martinez, M.; Strickland, M. Dissipative Dynamics of Highly Anisotropic Systems. Nucl. Phys. A 2010, 848, 183–197. [Google Scholar] [CrossRef] [Green Version]
- Ryblewski, R.; Florkowski, W. Highly anisotropic hydrodynamics – discussion of the model assumptions and forms of the initial conditions. Acta Phys. Polon. B 2011, 42, 115–138. [Google Scholar] [CrossRef]
- Florkowski, W.; Ryblewski, R. Projection method for boost-invariant and cylindrically symmetric dissipative hydrodynamics. Phys. Rev. C 2012, 85, 044902. [Google Scholar] [CrossRef] [Green Version]
- Martinez, M.; Ryblewski, R.; Strickland, M. Boost-Invariant (2+1)-dimensional Anisotropic Hydrodynamics. Phys. Rev. C 2012, 85, 064913. [Google Scholar] [CrossRef] [Green Version]
- Ryblewski, R.; Florkowski, W. Highly-anisotropic hydrodynamics in 3+1 space-time dimensions. Phys. Rev. C 2012, 85, 064901. [Google Scholar] [CrossRef] [Green Version]
- Bazow, D.; Heinz, U.W.; Strickland, M. Second-order (2+1)-dimensional anisotropic hydrodynamics. Phys. Rev. C 2014, 90, 054910. [Google Scholar] [CrossRef] [Green Version]
- Tinti, L.; Florkowski, W. Projection method and new formulation of leading-order anisotropic hydrodynamics. Phys. Rev. C 2014, 89, 034907. [Google Scholar] [CrossRef] [Green Version]
- Nopoush, M.; Ryblewski, R.; Strickland, M. Bulk viscous evolution within anisotropic hydrodynamics. Phys. Rev. C 2014, 90, 014908. [Google Scholar] [CrossRef] [Green Version]
- Tinti, L. Anisotropic matching principle for the hydrodynamic expansion. Phys. Rev. C 2016, 94, 044902. [Google Scholar] [CrossRef] [Green Version]
- Bazow, D.; Heinz, U.W.; Martinez, M. Nonconformal viscous anisotropic hydrodynamics. Phys. Rev. C 2015, 91, 064903. [Google Scholar] [CrossRef] [Green Version]
- Strickland, M.; Nopoush, M.; Ryblewski, R. Anisotropic hydrodynamics for conformal Gubser flow. Nucl. Phys. A 2016, 956, 268–271. [Google Scholar] [CrossRef] [Green Version]
- Molnar, E.; Niemi, H.; Rischke, D.H. Derivation of anisotropic dissipative fluid dynamics from the Boltzmann equation. Phys. Rev. D 2016, 93, 114025. [Google Scholar] [CrossRef] [Green Version]
- Molnár, E.; Niemi, H.; Rischke, D.H. Closing the equations of motion of anisotropic fluid dynamics by a judicious choice of a moment of the Boltzmann equation. Phys. Rev. D 2016, 94, 125003. [Google Scholar] [CrossRef] [Green Version]
- Alqahtani, M.; Nopoush, M.; Strickland, M. Quasiparticle anisotropic hydrodynamics for central collisions. Phys. Rev. C 2017, 95, 034906. [Google Scholar] [CrossRef]
- Bluhm, M.; Schäfer, T. Dissipative fluid dynamics for the dilute Fermi gas at unitarity: Anisotropic fluid dynamics. Phys. Rev. A 2015, 92, 043602. [Google Scholar] [CrossRef] [Green Version]
- Bluhm, M.; Schaefer, T. Model-independent determination of the shear viscosity of a trapped unitary Fermi gas: Application to high temperature data. Phys. Rev. Lett. 2016, 116, 115301. [Google Scholar] [CrossRef] [Green Version]
- Alqahtani, M.; Nopoush, M.; Ryblewski, R.; Strickland, M. (3+1)D Quasiparticle Anisotropic Hydrodynamics for Ultrarelativistic Heavy-Ion Collisions. Phys. Rev. Lett. 2017, 119, 042301. [Google Scholar] [CrossRef] [Green Version]
- Alqahtani, M.; Nopoush, M.; Ryblewski, R.; Strickland, M. Anisotropic hydrodynamic modeling of 2.76 TeV Pb-Pb collisions. Phys. Rev. C 2017, 96, 044910. [Google Scholar] [CrossRef] [Green Version]
- Almaalol, D.; Alqahtani, M.; Strickland, M. Anisotropic hydrodynamics with number-conserving kernels. Phys. Rev. C 2019, 99, 014903. [Google Scholar] [CrossRef] [Green Version]
- Almaalol, D.; Strickland, M. Anisotropic hydrodynamics with a scalar collisional kernel. Phys. Rev. C 2018, 97, 044911. [Google Scholar] [CrossRef] [Green Version]
- McNelis, M.; Bazow, D.; Heinz, U. (3+1)-dimensional anisotropic fluid dynamics with a lattice QCD equation of state. Phys. Rev. C 2018, 97, 054912. [Google Scholar] [CrossRef] [Green Version]
- Nopoush, M.; Strickland, M. Including off-diagonal anisotropies in anisotropic hydrodynamics. Phys. Rev. C 2019, 100, 014904. [Google Scholar] [CrossRef] [Green Version]
- Słodkowski, M.; Setniewski, D.; Aszklar, P.; Porter-Sobieraj, J. Modeling the Dynamics of Heavy-Ion Collisions with a Hydrodynamic Model Using a Graphics Processor. Symmetry 2021, 13, 507. [Google Scholar] [CrossRef]
- McNelis, M.; Bazow, D.; Heinz, U. Anisotropic fluid dynamical simulations of heavy-ion collisions. Comput. Phys. Commun. 2021, 267, 108077. [Google Scholar] [CrossRef]
- Romatschke, P.; Strickland, M. Collective modes of an anisotropic quark gluon plasma. Phys. Rev. D 2003, 68, 036004. [Google Scholar] [CrossRef] [Green Version]
- Strickland, M.; Noronha, J.; Denicol, G. Anisotropic nonequilibrium hydrodynamic attractor. Phys. Rev. D 2018, 97, 036020. [Google Scholar] [CrossRef] [Green Version]
- Martinez, M.; McNelis, M.; Heinz, U. Anisotropic fluid dynamics for Gubser flow. Phys. Rev. C 2017, 95, 054907. [Google Scholar] [CrossRef] [Green Version]
- Denicol, G.S.; Koide, T.; Rischke, D.H. Dissipative relativistic fluid dynamics: A new way to derive the equations of motion from kinetic theory. Phys. Rev. Lett. 2010, 105, 162501. [Google Scholar] [CrossRef]
- Denicol, G.S. Kinetic foundations of relativistic dissipative fluid dynamics. J. Phys. G 2014, 41, 124004. [Google Scholar] [CrossRef]
- Alqahtani, M.; Nopoush, M.; Strickland, M. Relativistic anisotropic hydrodynamics. Prog. Part. Nucl. Phys. 2018, 101, 204–248. [Google Scholar] [CrossRef] [Green Version]
- Ryblewski, R. Thermodynamically consistent formulation of quasiparticle viscous hydrodynamics. Acta Phys. Polon. Suppl. 2017, 10, 1073. [Google Scholar] [CrossRef] [Green Version]
- Alqahtani, M.; Strickland, M. Bulk observables at 5.02 TeV using quasiparticle anisotropic hydrodynamics. Eur. Phys. J. C 2021, 81, 1022. [Google Scholar] [CrossRef]
- Chojnacki, M.; Kisiel, A.; Florkowski, W.; Broniowski, W. THERMINATOR 2: THERMal heavy IoN generATOR 2. Comput. Phys. Commun. 2012, 183, 746–773. [Google Scholar] [CrossRef] [Green Version]
- Alqahtani, M.; Nopoush, M.; Ryblewski, R.; Strickland, M. KSU Code Repo. 2021. Available online: http://personal.kent.edu/~mstrick6/code (accessed on 28 December 2021).
- Adler, S.S.; Afanasiev, S.; Aidala, C.; Ajitanand, N.N.; Akiba, Y.; Alexander, J.; Amirikas, R.; Aphecetche, L.; Aronson, S.H.; Averbeck, R.; et al. Identified charged particle spectra and yields in Au+Au collisions at S(NN)**1/2 = 200-GeV. Phys. Rev. C 2004, 69, 034909. [Google Scholar] [CrossRef] [Green Version]
- Almaalol, D.; Alqahtani, M.; Strickland, M. Anisotropic hydrodynamic modeling of 200 GeV Au-Au collisions. Phys. Rev. C 2019, 99, 044902. [Google Scholar] [CrossRef] [Green Version]
- Abelev, B.; ALICE Collaboration. Centrality dependence of π, K, p production in Pb-Pb collisions at = 2.76 TeV. Phys. Rev. C 2013, 88, 044910. [Google Scholar] [CrossRef] [Green Version]
- Acharya, S.; Adamová, D.; Adhya, S.P.; Adler, A.; Adolfsson, J.; Aggarwal, M.M.; Rinella, G.A.; Agnello, M.; Agrawal, N.; Ahammed, Z.; et al. Production of charged pions, kaons, and (anti-)protons in Pb-Pb and inelastic pp collisions at = 5.02 TeV. Phys. Rev. C 2020, 101, 044907. [Google Scholar] [CrossRef]
- Alver, B.; Back, B.B.; Baker, M.D.; Ballintijn, M.; Barton, D.S.; Betts, R.R.; Bickley, A.A.; Bindel, R.; Budzanowski, A.; Busza, W.; et al. Phobos results on charged particle multiplicity and pseudorapidity distributions in Au+Au, Cu+Cu, d+Au, and p+p collisions at ultra-relativistic energies. Phys. Rev. C 2011, 83, 024913. [Google Scholar] [CrossRef] [Green Version]
- Adam, J.; Adamova, D.; Aggarwal, M.M.; Rinella, G.A.; Agnello, M.; Agrawal, N.; Ahammed, Z.; Ahn, S.U.; Aiola, S.; Akindinov, A.; et al. Centrality evolution of the charged-particle pseudorapidity density over a broad pseudorapidity range in Pb-Pb collisions at = 2.76 TeV. Phys. Lett. B 2016, 754, 373–385. [Google Scholar] [CrossRef]
- Adam, J.; Aggarwal, M.M.; Aglieri Rinella, G.; Agrawal, N.; Ahammed, Z.; Ahmad, S.F.; Ahn, S.U.; Aimo, I.; Akindinov, A.; Alam, S.N.; et al. Centrality dependence of the pseudorapidity density distribution for charged particles in Pb-Pb collisions at =5.02 TeV. Phys. Lett. B 2017, 772, 567–577. [Google Scholar] [CrossRef] [Green Version]
- Adare, A.; Afanasiev, S.; Aidala, C.; Ajitanand, N.N.; Akiba, Y.; Al-Bataineh, H.; Alexander, J.; Aoki, K.; Aramaki, Y.; Atomssa, E.T.; et al. Measurement of the higher-order anisotropic flow coefficients for identified hadrons in Au+Au collisions at = 200 GeV. Phys. Rev. C 2016, 93, 051902. [Google Scholar] [CrossRef] [Green Version]
- Abelev, B.B.; Adam, J.; Adamová, D.; Aggarwal, M.M.; Agnello, M.; Agostinelli, A.; Agrawal, N.; Ahammed, Z.; Ahmad, N.; Ahmed, I.; et al. Elliptic flow of identified hadrons in Pb-Pb collisions at =2.76 TeV. J. High Energy Phys. 2015, 6, 190. [Google Scholar] [CrossRef]
- Acharya, S.; Adamová, D.; Adolfsson, J.; Aggarwal, M.M.; Rinella, G.A.; Agnello, M.; Agrawal, N.; Ahammed, Z.; Ahn, S.U.; Aiola, S.; et al. Anisotropic flow of identified particles in Pb-Pb collisions at =5.02 TeV. J. High Energy Phys. 2018, 9, 6. [Google Scholar]
- Alqahtani, M.; Strickland, M. Pion interferometry at 200 GeV using anisotropic hydrodynamics. Phys. Rev. C 2020, 102, 064902. [Google Scholar] [CrossRef]
- Adams, J.; Aggarwal, M.M.; Ahammed, Z.; Amonett, J.; Anderson, B.D.; Arkhipkin, D.; Averichev, G.S.; Badyal, S.K.; Bai, Y.; Balewski, J.; et al. Pion interferometry in Au+Au collisions at S(NN)**(1/2) = 200-GeV. Phys. Rev. C 2005, 71, 044906. [Google Scholar] [CrossRef] [Green Version]
- Moreland, J.S.; Bernhard, J.E.; Bass, S.A. Alternative ansatz to wounded nucleon and binary collision scaling in high-energy nuclear collisions. Phys. Rev. C 2015, 92, 011901. [Google Scholar] [CrossRef] [Green Version]
- Ke, W.; Moreland, J.S.; Bernhard, J.E.; Bass, S.A. Constraints on rapidity-dependent initial conditions from charged particle pseudorapidity densities and two-particle correlations. Phys. Rev. C 2017, 96, 044912. [Google Scholar] [CrossRef] [Green Version]
- Bartels, J.; Golec-Biernat, K.J.; Kowalski, H. A modification of the saturation model: DGLAP evolution. Phys. Rev. D 2002, 66, 014001. [Google Scholar] [CrossRef] [Green Version]
- Kowalski, H.; Teaney, D. An Impact parameter dipole saturation model. Phys. Rev. D 2003, 68, 114005. [Google Scholar] [CrossRef] [Green Version]
- Schenke, B.; Tribedy, P.; Venugopalan, R. Fluctuating Glasma initial conditions and flow in heavy ion collisions. Phys. Rev. Lett. 2012, 108, 252301. [Google Scholar] [CrossRef] [PubMed]
- Chen, S. ISS Repo. 2020. Available online: https://github.com/chunshen1987/iSS (accessed on 28 December 2021).
- Bass, S.A.; Belkacem, M.; Bleicher, M.; Brandstetter, M.; Bravina, L.; Ernst, C.; Gerland, L.; Hofmann, M.; Hofmann, S.; Konopka, J.; et al. Microscopic models for ultrarelativistic heavy ion collisions. Prog. Part. Nucl. Phys. 1998, 41, 255–369. [Google Scholar] [CrossRef] [Green Version]
- Bleicher, M.; Zabrodin, E.; Spieles, C.; Bass, S.A.; Ernst, C.; Soff, S.; Bravina, L.; Belkacem, M.; Weber, H.; Stöcker, H.; et al. Relativistic hadron hadron collisions in the ultrarelativistic quantum molecular dynamics model. J. Phys. G 1999, 25, 1859–1896. [Google Scholar] [CrossRef]
- Weil, J.; Steinberg, V.; Staudenmaier, J.; Pang, L.G.; Oliinychenko, D.; Mohs, J.; Kretz, M.; Kehrenberg, T.; Goldschmidt, A.; Bäuchle, B.; et al. Particle production and equilibrium properties within a new hadron transport approach for heavy-ion collisions. Phys. Rev. C 2016, 94, 054905. [Google Scholar] [CrossRef]
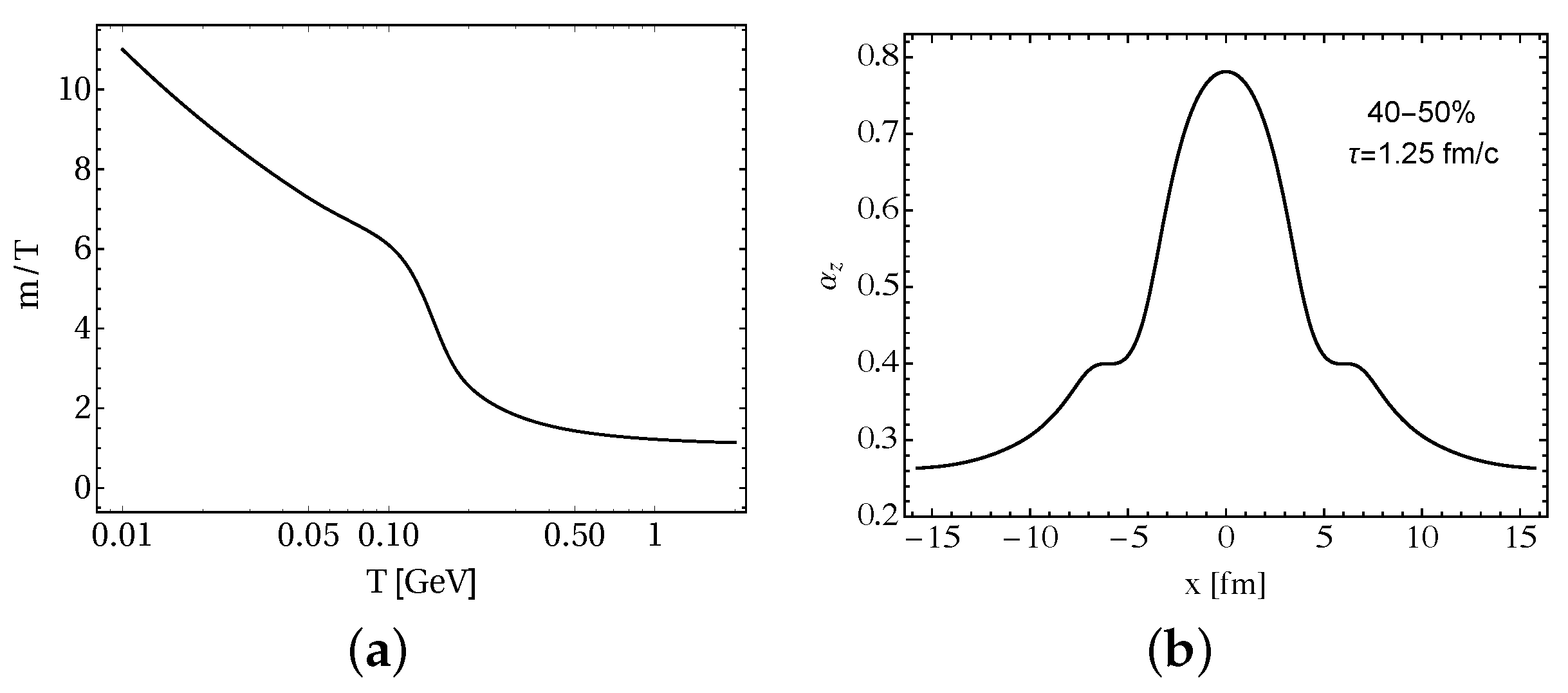
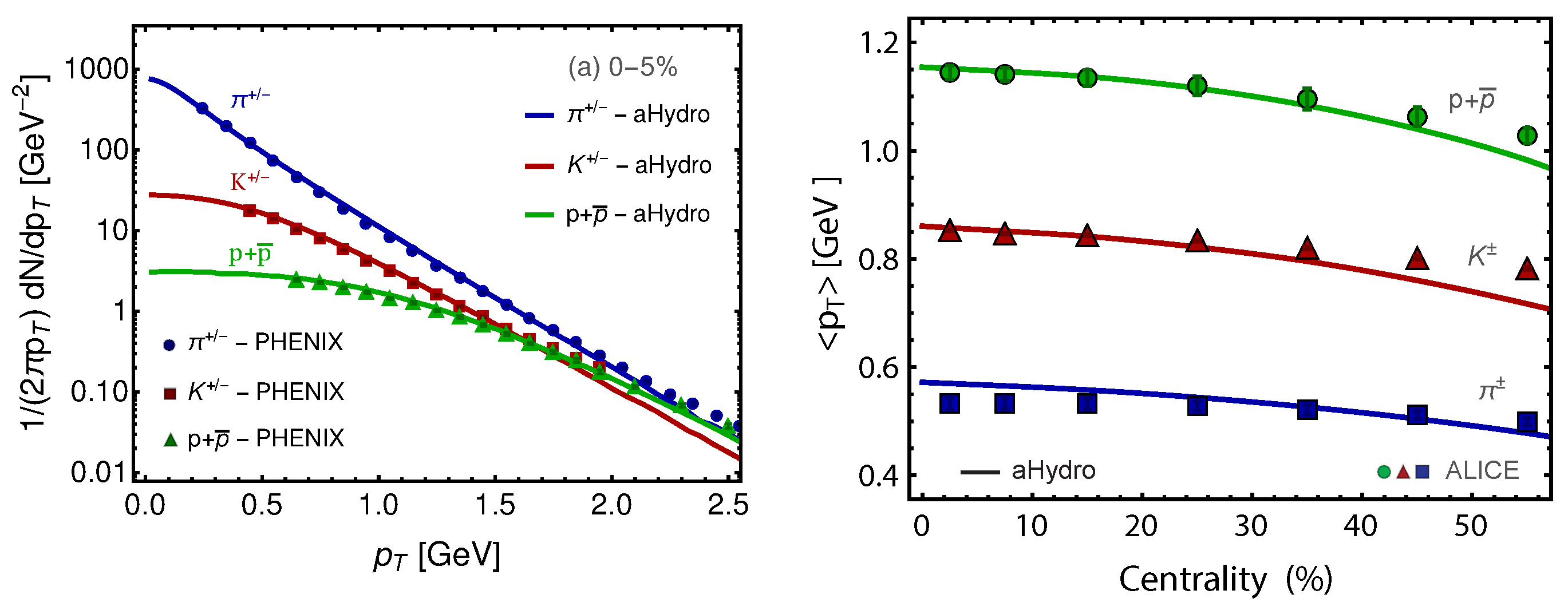
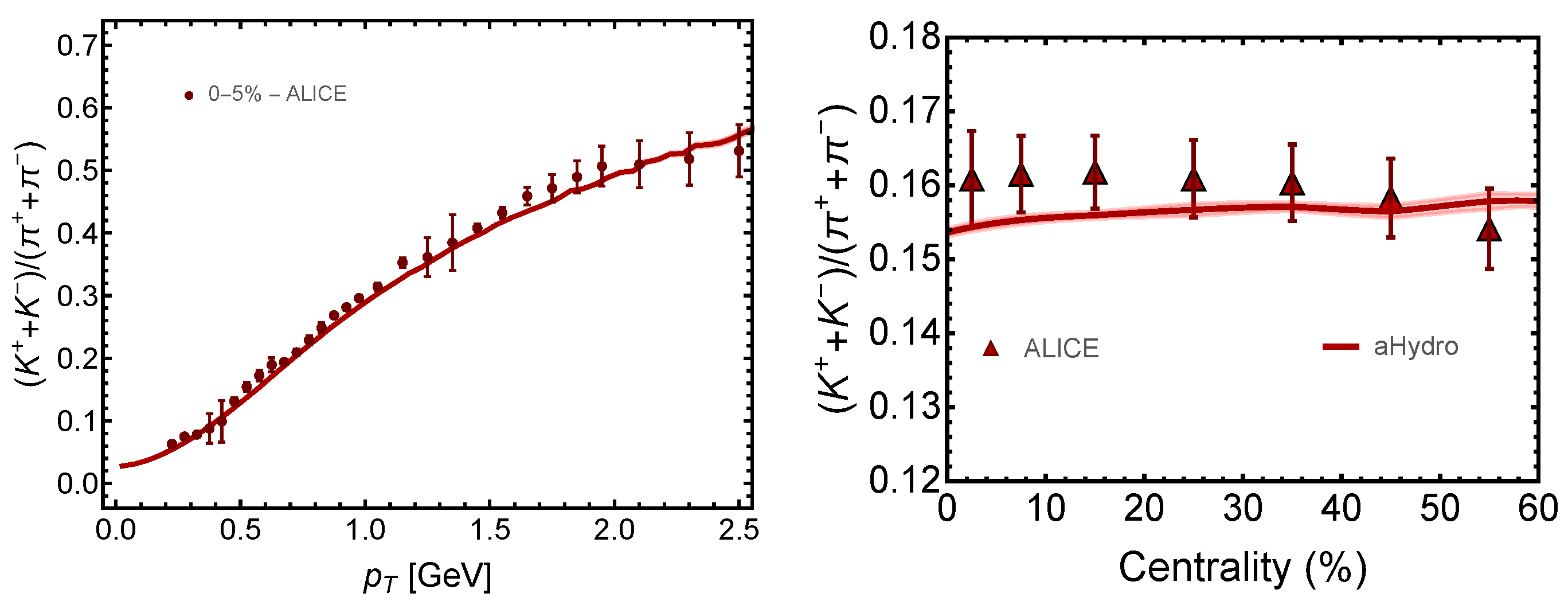
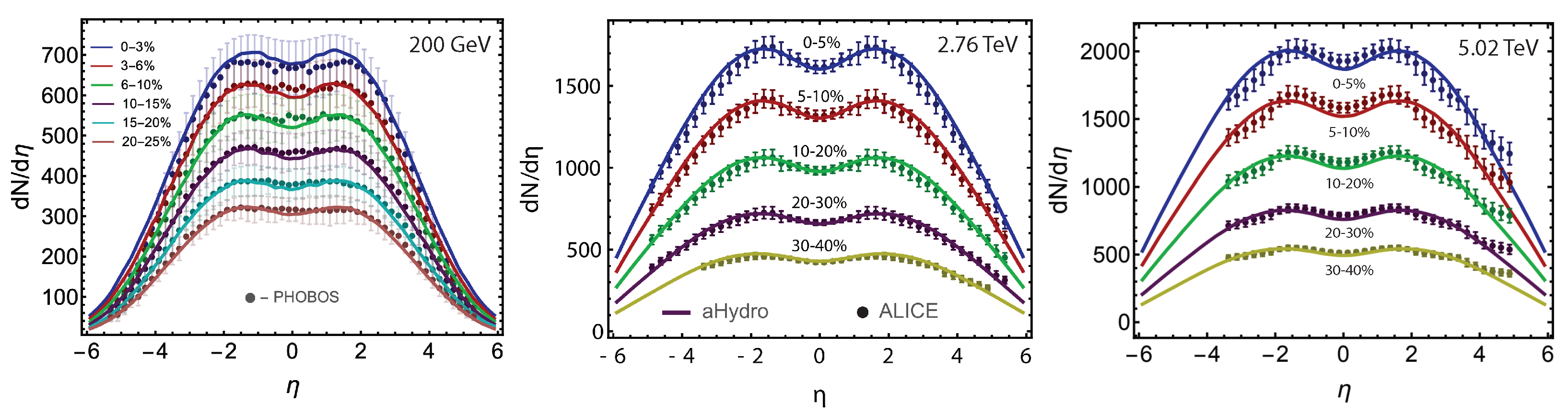
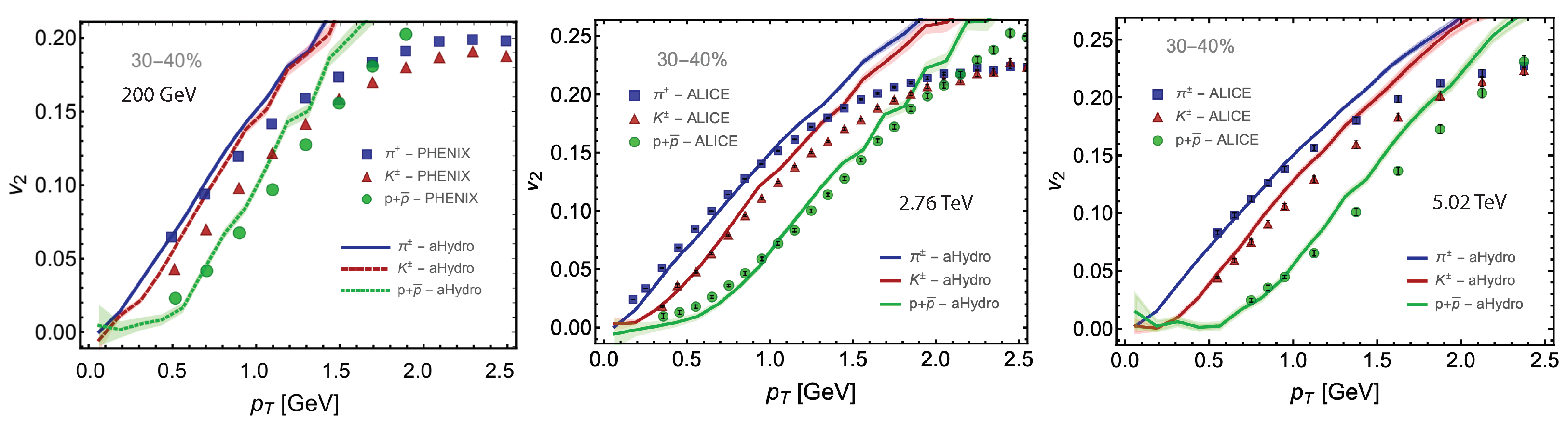
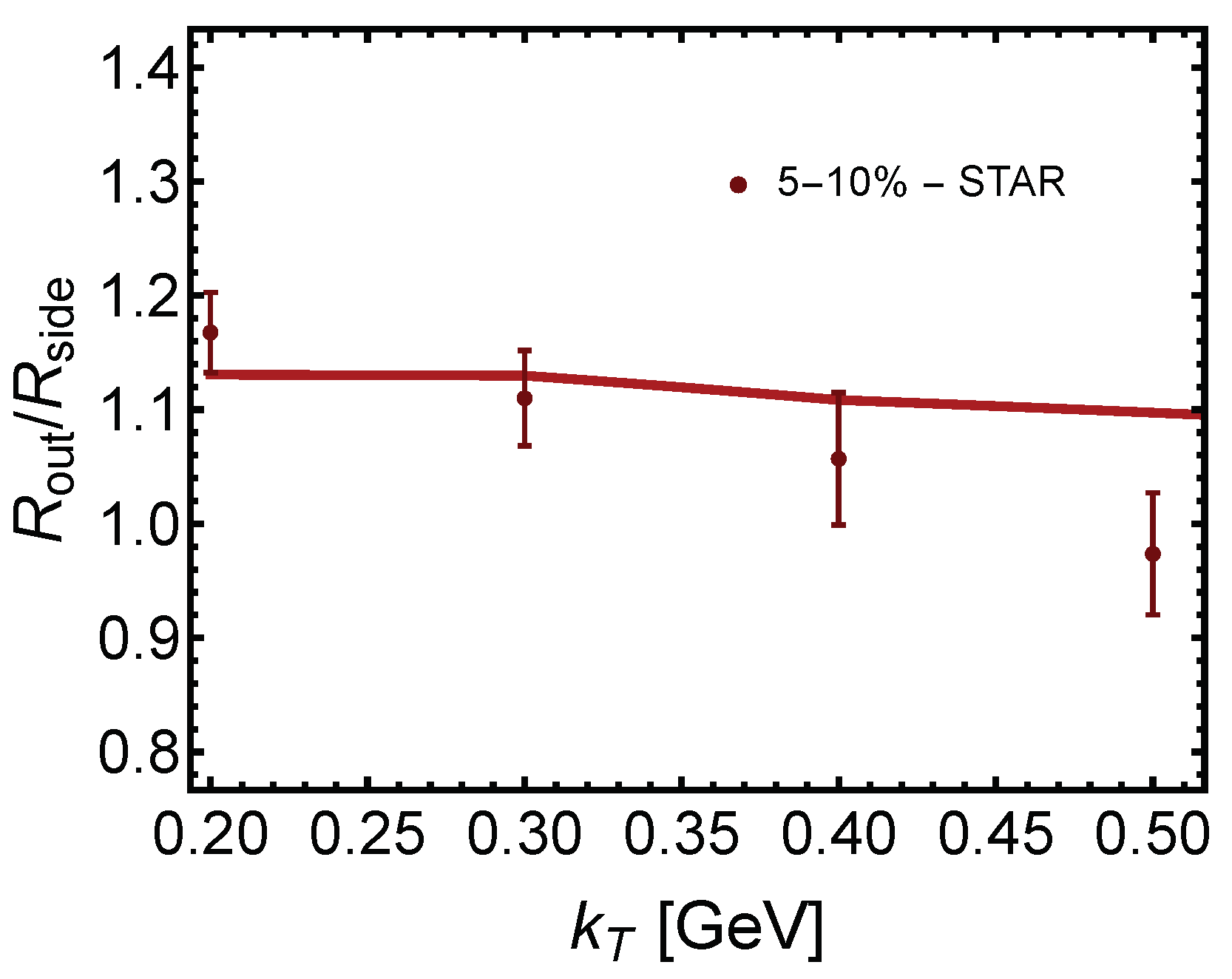
| Collision Energy | [MeV] | |
|---|---|---|
| 200 GeV | 455 | 0.179 |
| 2.76 TeV | 600 | 0.159 |
| 5.02 TeV | 630 | 0.159 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alalawi, H.; Alqahtani, M.; Strickland, M. Resummed Relativistic Dissipative Hydrodynamics. Symmetry 2022, 14, 329. https://doi.org/10.3390/sym14020329
Alalawi H, Alqahtani M, Strickland M. Resummed Relativistic Dissipative Hydrodynamics. Symmetry. 2022; 14(2):329. https://doi.org/10.3390/sym14020329
Chicago/Turabian StyleAlalawi, Huda, Mubarak Alqahtani, and Michael Strickland. 2022. "Resummed Relativistic Dissipative Hydrodynamics" Symmetry 14, no. 2: 329. https://doi.org/10.3390/sym14020329
APA StyleAlalawi, H., Alqahtani, M., & Strickland, M. (2022). Resummed Relativistic Dissipative Hydrodynamics. Symmetry, 14(2), 329. https://doi.org/10.3390/sym14020329






