Fractional View Analysis of Fornberg–Whitham Equations by Using Elzaki Transform
Abstract
:1. Introduction
2. Preliminaries and Model Description
2.1. Preliminaries
2.2. Model Description
3. Methodology of Adomian Decomposition Transform Method
4. Uniqueness and Existence Solution for ADTM
5. Methodology of VITM
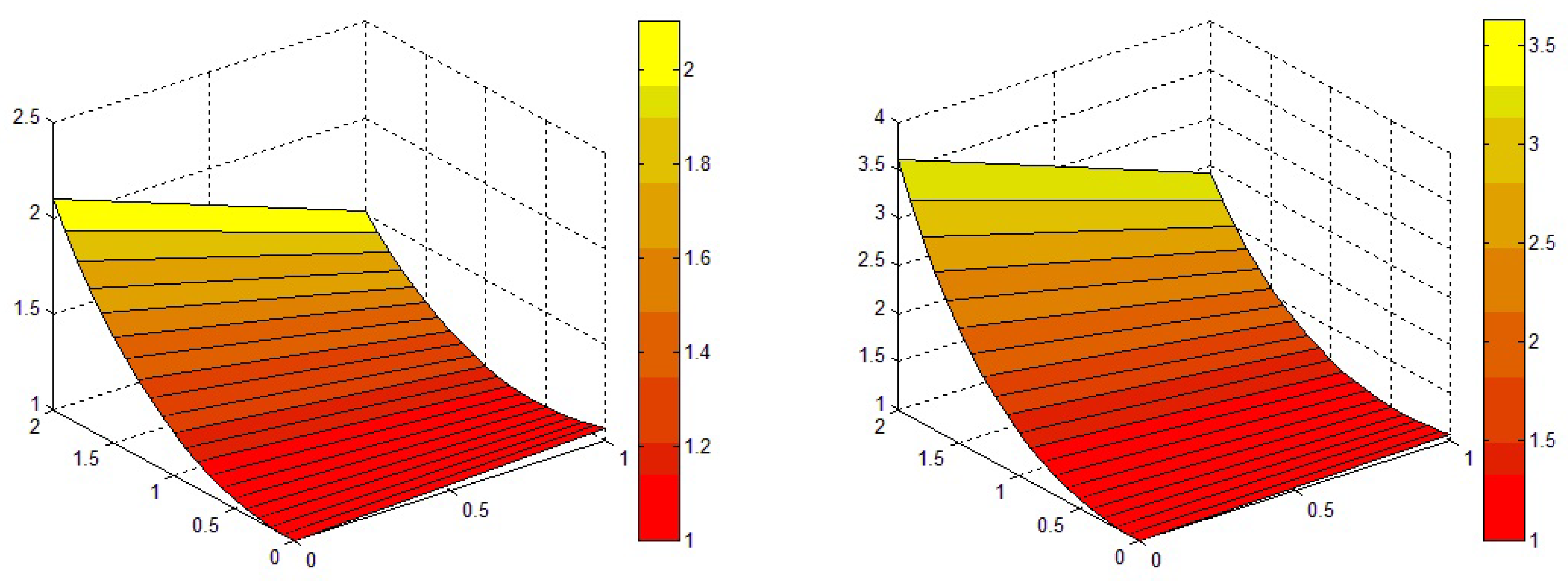
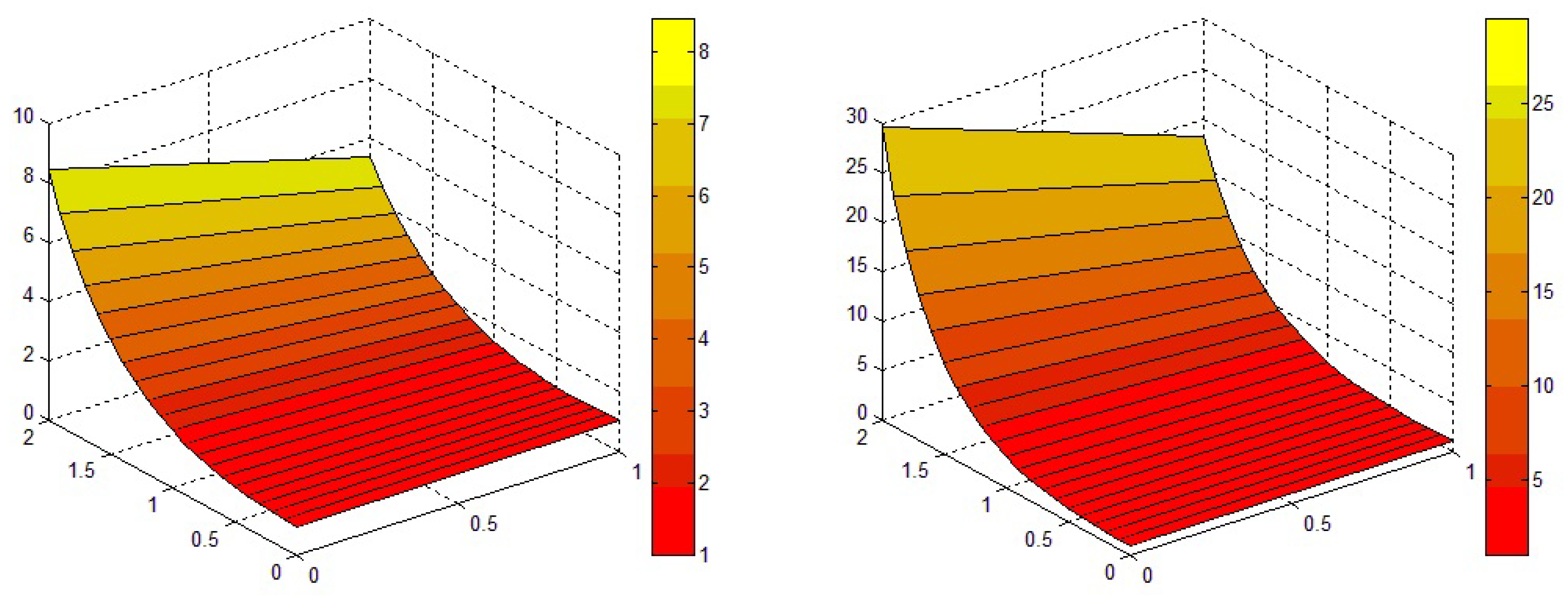
6. Numerical Results
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Reyes-Melo, M.E.; Martinez-Vega, J.J.; Guerrero-Salazar, C.A.; Ortiz-Mendez, U. Application of fractional calculus to modelling of relaxation phenomena of organic dielectric materials. In Proceedings of the 2004 IEEE International Conference on Solid Dielectrics, ICSD 2004, Toulouse, France, 5–9 July 2004; Volume 2, pp. 530–533. [Google Scholar]
- Said, L.A.; Madian, A.H.; Radwan, A.G.; Soliman, A.M. Fractional order oscillator with independent control of phase and frequency. In Proceedings of the 2014 2nd International Conference on Electronic Design (ICED), Penang, Malaysia, 19–21 August 2014; pp. 224–229. [Google Scholar]
- Machado, J.T.; Kiryakova, V.; Mainardi, F. Recent history of fractional calculus. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1140–1153. [Google Scholar] [CrossRef] [Green Version]
- Sabatier, J.A.T.M.J.; Agrawal, O.P.; Machado, J.T. Advances in Fractional Calculus; No. 9; Springer: Dordrecht, The Netherlands, 2007; Volume 4. [Google Scholar]
- Baleanu, D.; Diethelm, K.; Scalas, E.; Trujillo, J.J. Fractional Calculus: Models and Numerical Methods; World Scientific: Singapore, 2012; Volume 3. [Google Scholar]
- Debnath, L. Recent applications of fractional calculus to science and engineering. Int. J. Math. Math. Sci. 2003, 2003, 3413–3442. [Google Scholar] [CrossRef] [Green Version]
- Shah, R.; Saad Alshehry, A.; Weera, W. A semi-analytical method to investigate fractional-order gas dynamics equations by Shehu transform. Symmetry 2022, 14, 1458. [Google Scholar] [CrossRef]
- Mukhtar, S.; Shah, R.; Noor, S. The Numerical Investigation of a Fractional-Order Multi-Dimensional Model of Navier-Stokes Equation via Novel Techniques. Symmetry 2022, 14, 1102. [Google Scholar] [CrossRef]
- Shah, N.A.; Hamed, Y.S.; Abualnaja, K.M.; Chung, J.D.; Khan, A. A comparative analysis of fractional-order kaup-kupershmidt equation within different operators. Symmetry 2022, 14, 986. [Google Scholar] [CrossRef]
- Saad Alshehry, A.; Imran, M.; Khan, A.; Weera, W. Fractional View Analysis of Kuramoto-Sivashinsky Equations with Non-Singular Kernel Operators. Symmetry 2022, 14, 1463. [Google Scholar] [CrossRef]
- Kai, Y.; Chen, S.; Zhang, K.; Yin, Z. Exact solutions and dynamic properties of a nonlinear fourth-order time-fractional partial differential equation. Waves Random Complex Media 2022, 1–12. [Google Scholar] [CrossRef]
- Siryk, S.V.; Salnikov, N.N. Numerical solution of Burgers equation by Petrov-Galerkin method with adaptive weighting functions. J. Autom. Inf. Sci. 2012, 44, 50–67. [Google Scholar] [CrossRef]
- Stynes, M.; Stynes, D. Convection Diffusion Problems: An Introduction to Their Analysis and Numerical Solution; American Mathematical Society: Providence, RI, USA, 2018; Volume 196, 156p. [Google Scholar]
- Siryk, S.V.; Salnikov, N.N. Accuracy and stability of the Petrov-Galerkin method for solving the stationary convection-diffusion equation. Cybern. Syst. Anal. 2014, 50, 278–287. [Google Scholar] [CrossRef]
- Siryk, S.V.; Salnikov, N.N. Analysis of lumped approximations in the finite-element method for convection-diffusion problems. Cybern. Syst. Anal. 2013, 49, 774–784. [Google Scholar] [CrossRef]
- John, V.; Knobloch, P.; Novo, J. Finite elements for scalar convection-dominated equations and incompressible flow problems: A never ending story. Comput. Vis. Sci. 2018, 19, 47–63. [Google Scholar] [CrossRef] [Green Version]
- Siryk, S.V.; Salnikov, N.N. Estimation of the Accuracy of Finite-Element Petrov-Galerkin Method in Integrating the One-Dimensional Stationary Convection-Diffusion-Reaction Equation. Ukr. Math. J. 2015, 67, 1062–1090. [Google Scholar] [CrossRef]
- AbdulRidha, M.W.; Kashkool, H.A. Space-Time Petrov-Discontinuous Galerkin Finite Element Method for Solving Linear Convection Diffusion Problems. J. Phys. Conf. Ser. 2022, 2322, 012007. [Google Scholar] [CrossRef]
- Xu, Y. Similarity solution and heat transfer characteristics for a class of nonlinear convection-diffusion equation with initial value conditions. Math. Probl. Eng. 2019, 2019, 3467276. [Google Scholar] [CrossRef]
- Ahmad, S.; Ullah, A.; Akgul, A.; De la Sen, M. A Novel Homotopy Perturbation Method with Applications to Nonlinear Fractional Order KdV and Burger Equation with Exponential-Decay Kernel. J. Funct. Spaces 2021, 2021, 8770488. [Google Scholar] [CrossRef]
- Sun, H.; Zhang, Y.; Baleanu, D.; Chen, W.; Chen, Y. A new collection of real world applications of fractional calculus in science and engineering. Commun. Nonlinear Sci. Numer. Simul. 2018, 64, 213–231. [Google Scholar] [CrossRef]
- Alderremy, A.A.; Khan, H.; Shah, R.; Aly, S.; Baleanu, D. The Analytical Analysis of Time-Fractional Fornberg Whitham Equations. Mathematics 2020, 8, 987. [Google Scholar] [CrossRef]
- Gupta, P.K.; Singh, M. Homotopy perturbation method for fractional Fornberg-Whitham equation. Comput. Math. Appl. 2011, 61, 250–254. [Google Scholar] [CrossRef]
- Saker, M.G.; Erdogan, F.; Yildirim, A. Variational iteration method for the time fractional Fornberg-Whitham equation. Comput. Math. Appl. 2012, 63, 1382–1388. [Google Scholar] [CrossRef] [Green Version]
- Merdan, M.; Gokdogan, A.; Yildirim, A.; Mohyud-Din, S.T. Numerical simulation of fractional Fornberg-Whitham equation by differential transformation method. Abstr. Appl. Anal. 2012, 2012, 965367. [Google Scholar] [CrossRef]
- Singh, J.; Kumar, D.; Kumar, S. New treatment of fractional Fornberg-Whitham equation via Laplace transform. Ain Shams Eng. J. 2013, 4, 557–562. [Google Scholar] [CrossRef] [Green Version]
- Sakar, M.G.; Ergoren, H. Alternative variational iteration method for solving the time-fractional Fornberg-Whitham equation. Appl. Math. Model. 2015, 39, 3972–3979. [Google Scholar] [CrossRef]
- Al-luhaibi, M.S. An analytical treatment to fractional Fornberg-Whitham equation. Math. Sci. 2017, 11, 1–6. [Google Scholar] [CrossRef] [Green Version]
- Elzaki, T.M. On The New Integral Transform “Elzaki Transform” Fundamental Properties Investigations and Applications. Glob. J. Math. Sci. Theory Pract. 2012, 4, 1–13. [Google Scholar]
- Yasmin, H.; Iqbal, N. A comparative study of the fractional coupled burgers and Hirota-Satsuma KdV equations via analytical techniques. Symmetry 2022, 14, 1364. [Google Scholar] [CrossRef]
- Ziane, D.; Cherif, M.H. Resolution of nonlinear partial differential equations by Elzaki transform decomposition method. J. Approx. Theory Appl. Math. 2015, 5, 17–30. [Google Scholar]
- Shah, N.A.; Chung, J.D. The analytical solution of fractional-order Whitham-Broer-Kaup equations by an Elzaki decomposition method. Numer. Methods Partial. Differ. Equ. 2021. [Google Scholar] [CrossRef]
- Nuruddeen, R.I. Elzaki decomposition method and its applications in solving linear and nonlinear Schrodinger equations. Sohag J. Math. 2017, 4, 1–5. [Google Scholar] [CrossRef]
- Naeem, M.; Azhar, O.F.; Zidan, A.M.; Nonlaopon, K.; Shah, R. Numerical analysis of fractional-order parabolic equations via Elzaki transform. J. Funct. Spaces 2021, 2021, 3484482. [Google Scholar] [CrossRef]
- He, J.-H. Approximate solution of nonlinear differential equations with convolution product nonlinearities. Comput. Methods Appl. Mech. Eng. 1998, 167, 69–73. [Google Scholar] [CrossRef]
- Inokuti, M.; Sekine, H.; Mura, T. General use of the Lagrange multiplier in nonlinear mathematical physics. Var. Method Mech. Solids 1978, 33, 156–162. [Google Scholar]
- Wu, G.-C.; Baleanu, D. Variational iteration method for fractional calculus—A universal approach by Laplace transform. Adv. Differ. Equ. 2013, 2013, 18. [Google Scholar] [CrossRef] [Green Version]
- Anjum, N.; He, J.-H. Laplace transform: Making the variational iteration method easier. Appl. Math. Lett. 2019, 92, 134–138. [Google Scholar] [CrossRef]
- Ziane, D.; Elzaki, T.M.; Cherif, M.H. Elzaki transform combined with variational iteration method for partial differential equations of fractional order. Fundam. J. Math. Appl. 2018, 1, 102–108. [Google Scholar] [CrossRef]
- Hilal, E.; Elzaki, T.M. Solution of nonlinear partial differential equations by new Laplace variational iteration method. J. Funct. Spaces 2014, 2014, 790714. [Google Scholar] [CrossRef] [Green Version]
- Mohamed, M.Z.; Elzaki, T.M.; Algolam, M.S.; Abd Elmohmoud, E.M.; Hamza, A.E. New modified variational iteration Laplace transform method compares Laplace adomian decomposition method for solution time-partial fractional differential equations. J. Appl. Math. 2021, 2021, 6662645. [Google Scholar] [CrossRef]
- Yavuz, M.; Abdeljawad, T. Nonlinear regularized long-wave models with a new integral transformation applied to the fractional derivative with power and Mittag-Leffler kernel. Adv. Differ. Equ. 2020, 2020, 367. [Google Scholar] [CrossRef]
- Elzaki, T.M. The new integral transform Elzaki transform. Glob. J. Pure Appl. Math. 2011, 7, 57–64. [Google Scholar]
- Alderremy, A.A.; Elzaki, T.M.; Chamekh, M. New transform iterative method for solving some Klein-Gordon equations. Results Phys. 2018, 10, 655–659. [Google Scholar] [CrossRef]
- Kim, H. The time shifting theorem and the convolution for Elzaki transform. Int. J. Pure Appl. Math. 2013, 87, 261–271. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haroon, F.; Mukhtar, S.; Shah, R. Fractional View Analysis of Fornberg–Whitham Equations by Using Elzaki Transform. Symmetry 2022, 14, 2118. https://doi.org/10.3390/sym14102118
Haroon F, Mukhtar S, Shah R. Fractional View Analysis of Fornberg–Whitham Equations by Using Elzaki Transform. Symmetry. 2022; 14(10):2118. https://doi.org/10.3390/sym14102118
Chicago/Turabian StyleHaroon, Faisal, Safyan Mukhtar, and Rasool Shah. 2022. "Fractional View Analysis of Fornberg–Whitham Equations by Using Elzaki Transform" Symmetry 14, no. 10: 2118. https://doi.org/10.3390/sym14102118
APA StyleHaroon, F., Mukhtar, S., & Shah, R. (2022). Fractional View Analysis of Fornberg–Whitham Equations by Using Elzaki Transform. Symmetry, 14(10), 2118. https://doi.org/10.3390/sym14102118




