Rapid Geometric Screening of Low-Energy Surfaces in Crystals
Abstract
:1. Introduction
2. Methods
2.1. A Simple Model of Surface Energy
- There are an infinite number of possible surface directions.
- For a given direction, there are an infinite number of possible locations at which to cut the crystal to make a surface.
2.2. Wulff Construction
- 1.
- The crystal’s Wulff shape , alternatively described by:
- (a)
- The set of facets of , encoded as vectors , (with K as small as possible) defining the convex hull . Note that gives the facet k’s unit normal and gives its surface energy.
- (b)
- The set of vertices of , denoted , .
- 2.
- The full orientation-dependence of the surface energy, encoded as a function of a normal unit vector u.
- 1.
- Construct the convex hull of the points and express it in terms of the minimal number of vectors such that .
- 2.
- Compute the convex hull of the points and express it in terms of the minimal number of vectors such that .
- 3.
- For any given unit vector u, compute .
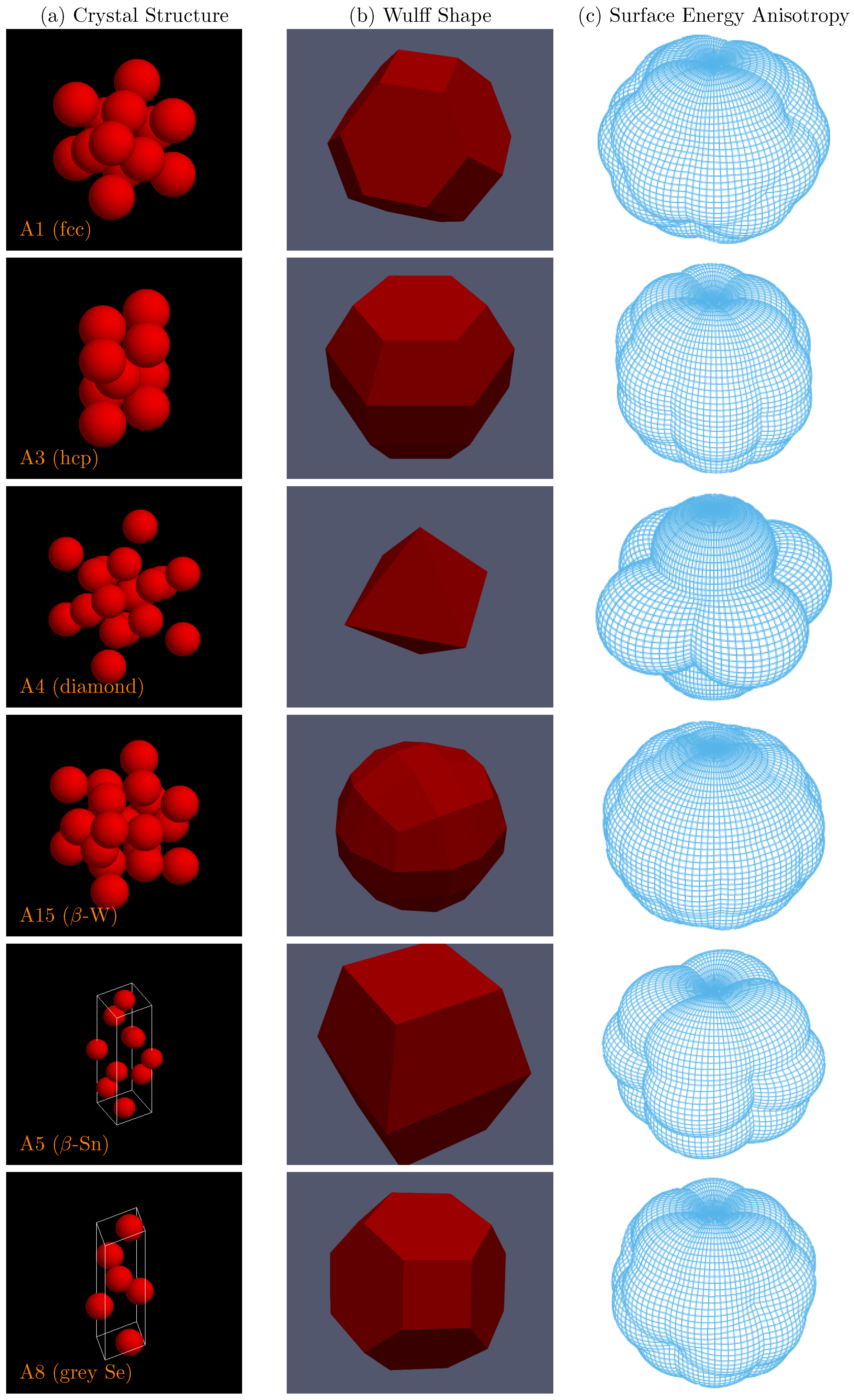
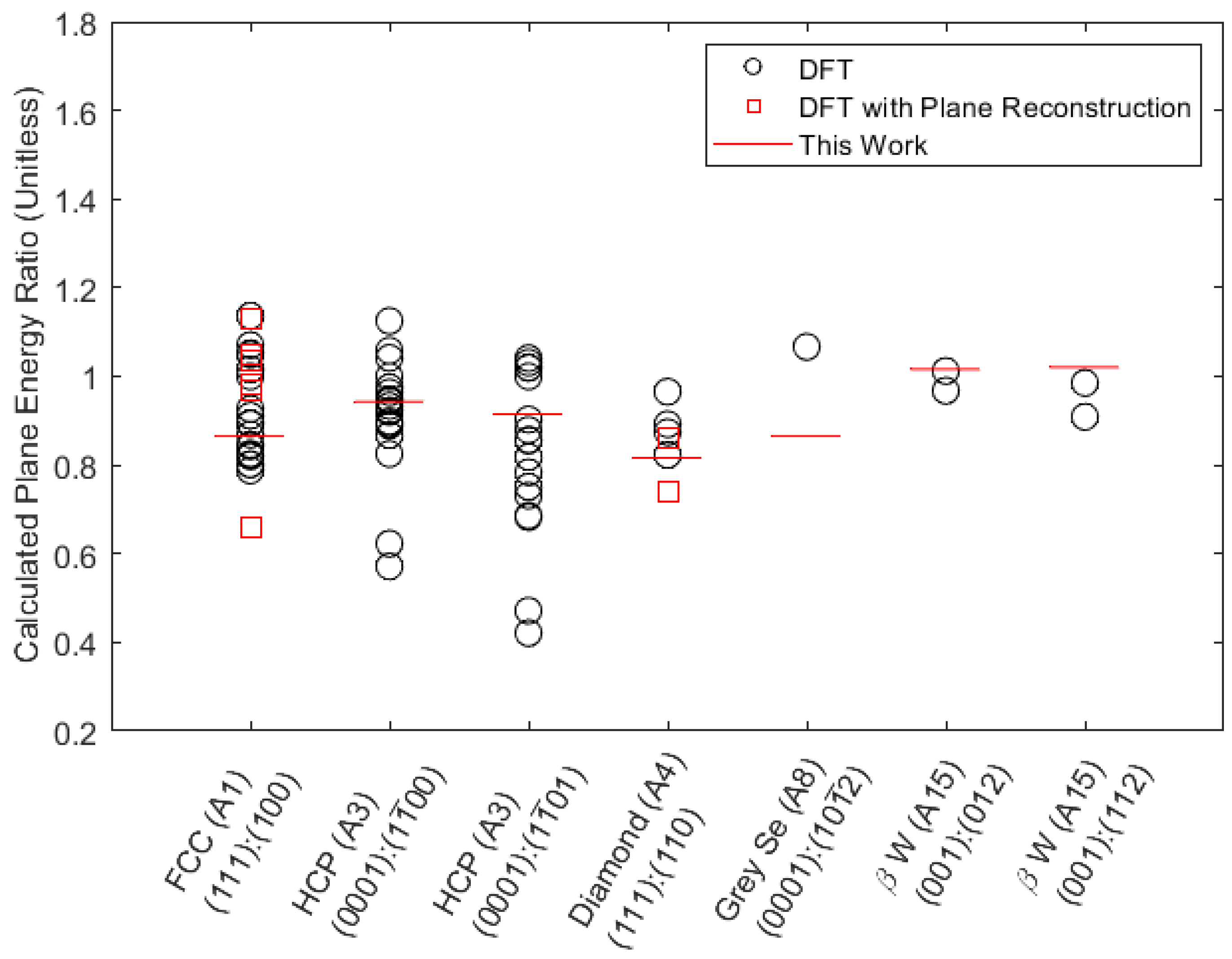
3. Results
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| DFT | Density Functional Theory |
| ATAT | Alloy Theoretic Automated Toolkit |
| FCC | Face-Centered Cubic |
| HCP | Hexagonal Close-Packed |
References
- Kurz, W.; Fisher, D. Fundamentals of Solidification; Trans. Tech.: Aedermannsdorf, Switzerland, 1989. [Google Scholar]
- Jamtveit, B.; Meakin, P. Growth, Dissolution and Pattern Formation in Geosystems; Kluwer: Dordrecht, Germany, 1999. [Google Scholar] [CrossRef]
- Yin, Y.; Alivisatos, A. Colloidal nanocrystal synthesis and the organic–inorganic interface. Nature 2005, 437, 664–670. [Google Scholar] [CrossRef] [Green Version]
- Methfessel, M.; Hennig, D.; Scheffler, M. Trends of the surface relaxations, surface energies, and work functions of the 4d transition metals. Phys. Rev. B 1992, 46, 4816–4829. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tran, R.; Xu, Z.; Radhakrishnan, B.; Winston, D.; Sun, W.; Persson, K.A.; Ong, S.P. Surface energies of elemental crystals. Sci. Data 2016, 3, 160080. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vitos, L.; Ruban, A.V.; Skriver, H.L.; Kollár, J. The surface energy of metals. Surf. Sci. 1998, 411, 186–202. [Google Scholar] [CrossRef]
- Beck, M.J.; van de Walle, A.; Asta, M.D. Surface energetics and structure of the Ge wetting layer on Si (100). Phys. Rev. B 2004, 70, 205337. [Google Scholar] [CrossRef] [Green Version]
- Lanier, C.H.; van de Walle, A.; Erdman, N.; Landree, E.; Warschkow, O.; Kazimirov, A.; Poeppelmeier, K.R.; Zegenhagen, J.; Asta, M.D.; Marks, L.D. The c(6×2) reconstruction on the SrTiO3 (001) Surface. Phys. Rev. B 2007, 76, 045421. [Google Scholar] [CrossRef] [Green Version]
- Chepulskii, R.V.; Butler, W.H.; van de Walle, A.; Curtarolo, S. Surface segregation in nanoparticles from first principles: The case of FePt. Scr. Mater. 2010, 62, 179. [Google Scholar] [CrossRef] [Green Version]
- Galanakis, I.; Bihlmayer, G.; Bellini, V.; Papanikolaou, N.; Zeller, R.; Blügel, S.; Dederichs, P.H. Broken-bond rule for the surface energies of noble metals. Europhys. Lett. (EPL) 2002, 58, 751–757. [Google Scholar] [CrossRef] [Green Version]
- Yang, Z.G.; Enomoto, M. Calculation of the interfacial energy of B1-type carbides and nitrides with austenite. Metall. Mater. Trans. A 2001, 32, 267–274. [Google Scholar] [CrossRef]
- Nicholas, J. Calculation of surface energy as a function of orientation for cubic crystals. Aust. J. Phys. 1968, 21, 21–34. [Google Scholar] [CrossRef] [Green Version]
- Sonderegger, B.; Kozeschnik, E. Generalized Nearest-Neighbor Broken-Bond Analysis of Randomly Oriented Coherent Interfaces in Multicomponent Fcc and Bcc Structures. Metall. Mater. Trans. A 1998, 40, 499–510. [Google Scholar] [CrossRef]
- Mackenzie, J.; Moore, A.; Nicholas, J. Bonds broken at atomically flat crystal surfaces—I: Face-centred and body-centred cubic crystals. J. Phys. Chem. Solids 1962, 23, 185–196. [Google Scholar] [CrossRef]
- Mackenzie, J.; Nicholas, J. Bonds broken at atomically flat crystal surfaces—II: Crystals containing many atoms in a primitive unit cell. J. Phys. Chem. Solids 1962, 23, 197–205. [Google Scholar] [CrossRef]
- van de Walle, A.; Ceder, G. Automating First-Principles Phase Diagram Calculations. J. Phase Equilib. 2002, 23, 348–359. [Google Scholar] [CrossRef] [Green Version]
- Voronoi, G. Nouvelles applications des paramètres continus à la théorie des formes quadratiques: Deuxième Mémoire: Recherches sur les Paralleloèdres Primitifs. J. Reine Angew. Math. 1908, 134, 198. [Google Scholar] [CrossRef]
- Briggs, R.M.; Ciobanu, C.V. Evolutionary approach for finding the atomic structure of steps on stable crystal surfaces. Phys. Rev. B 2007, 75, 195415. [Google Scholar] [CrossRef] [Green Version]
- Chuang, F.; Ciobanu, C.V.; Shenoy, V.; Wang, C.Z.; Ho, K.M. Finding the reconstructions of semiconductor surfaces via a genetic algorithm. Surf. Sci. 2004, 573, L375–L381. [Google Scholar] [CrossRef] [Green Version]
- Oganov, A.R.; Glass, C.W. Crystal structure prediction using ab initio evolutionary techniques: Principles and applications. J. Chem. Phys. 2006, 124, 244704. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hong, Q.J.; Yasi, J.; van de Walle, A. A Tetrahedron-tiling Method for Crystal Structure Prediction. Phys. Rev. Mater. Rapid Commun. 2017, 1, 020801. [Google Scholar] [CrossRef]
- Hoyt, J.J.; Asta, M.; Karma, A. Method for computing the anisotropy of the solid–liquid interfacial free energy. Phys. Rev. Lett 2001, 86, 5530. [Google Scholar] [CrossRef] [PubMed]
- Taylor, J.E. Crystalline Variational Problems. Bull. Am. Math. Soc. 1978, 84, 568–588. [Google Scholar] [CrossRef] [Green Version]
- Cahn, J.; Carter, W. Crystal shapes and phase equilibria: A common mathematical basis. Metall. Mater. Trans. A 1996, 27, 1431–1440. [Google Scholar] [CrossRef]
- Roosen, A.R.; McCormack, R.P.; Carter, W.C. Wulffman: A tool for the calculation and display of crystal shapes. Comput. Mater. Sci. 1998, 11, 16–26. [Google Scholar] [CrossRef]
- van de Walle, A.; Chirranjeevi, B.G.; Demers, S.; Hong, Q.J.; Kowalski, A.; Miljacic, L.; Pomrehn, G.S.; Tiwary, P. Ab initio calculation of anisotropic interfacial excess free energies. Phys. Rev. B 2014, 89, 184101. [Google Scholar] [CrossRef] [Green Version]
- van de Walle, A. Multicomponent multisublattice alloys, nonconfigurational entropy and other additions to the Alloy Theoretic Automated Toolkit. Calphad 2009, 33, 266–278. [Google Scholar] [CrossRef] [Green Version]
- Stekolnikov, A.A.; Furthmüller, J.; Bechstedt, F. Absolute surface energies of group-IV semiconductors: Dependence on orientation and reconstruction. Phys. Rev. B 2002, 65, 115318. [Google Scholar] [CrossRef]
- Jain, A.; Hautier, G.; Moore, C.J.; Ong, S.P.; Fischer, C.C.; Mueller, T.; Persson, K.A.; Ceder, G. A high-throughput infrastructure for density functional theory calculations. Comp. Mater. Sci. 2011, 50, 2295. [Google Scholar] [CrossRef]
- Curtarolo, S.; Hart, G.L.; Nardelli, M.B.; Mingo, N.; Sanvito, S.; Levy, O. The high-throughput highway to computational materials design. Nat. Mater. 2013, 12, 191–201. [Google Scholar] [CrossRef] [PubMed]
- Talapatra, A.; Duong, T.; Son, W.; Gao, H.; Radovic, M.; Arróyave, R. High-throughput combinatorial study of the effect of M site alloying on the solid solution behavior of M2AlC MAX phases. Phys. Rev. B 2016, 94, 104106. [Google Scholar] [CrossRef]
- Saal, J.E.; Kirklin, S.; Aykol, M.; Meredig, B.; Wolverton, C. Materials design and discovery with high-throughput density functional theory: The open quantum materials database (OQMD). JOM 2013, 65, 1501–1509. [Google Scholar] [CrossRef]
- Otis, R.; Liu, Z.K. High-Throughput Thermodynamic Modeling and Uncertainty Quantification for ICME. JOM 2017, 69, 886. [Google Scholar] [CrossRef]
- Curtarolo, S.; Oses, C.; Esters, M.; Hicks, D.; Divilov, S.; Eckert, H.; Friedrich, R.; Mehl, M.J.; Smolyanyuk, A.; Campilongo, X.; et al. Aflow++: A C++ framework for autonomous materials design. arXiv 2022, arXiv:2208.03052. [Google Scholar] [CrossRef]
- van de Walle, A.; Sun, R.; Hong, Q.J.; Kadkhodaei, S. Software tools for high-throughput CALPHAD from first-principles data. Calphad 2017, 58, 70. [Google Scholar] [CrossRef]
- Boettinger, W.J.; Warren, J.A.; Beckermann, C.; Karma, A. Phase-Field Simulation of Solidification. Ann. Rev. Mater. Res. 2002, 32, 163–194. [Google Scholar] [CrossRef]
- Karma, A. Phase Field Methods; Elsevier: Oxford, UK, 2001; Volume 7, pp. 6873–6886. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, H.; van de Walle, A. Rapid Geometric Screening of Low-Energy Surfaces in Crystals. Symmetry 2022, 14, 2067. https://doi.org/10.3390/sym14102067
Liu H, van de Walle A. Rapid Geometric Screening of Low-Energy Surfaces in Crystals. Symmetry. 2022; 14(10):2067. https://doi.org/10.3390/sym14102067
Chicago/Turabian StyleLiu, Helena, and Axel van de Walle. 2022. "Rapid Geometric Screening of Low-Energy Surfaces in Crystals" Symmetry 14, no. 10: 2067. https://doi.org/10.3390/sym14102067
APA StyleLiu, H., & van de Walle, A. (2022). Rapid Geometric Screening of Low-Energy Surfaces in Crystals. Symmetry, 14(10), 2067. https://doi.org/10.3390/sym14102067




