Comparison of Dynamic Performance of an All-Metallic Vibration Isolator by Elliptic Method and Frequency Sweeping Method
Abstract
1. Introduction
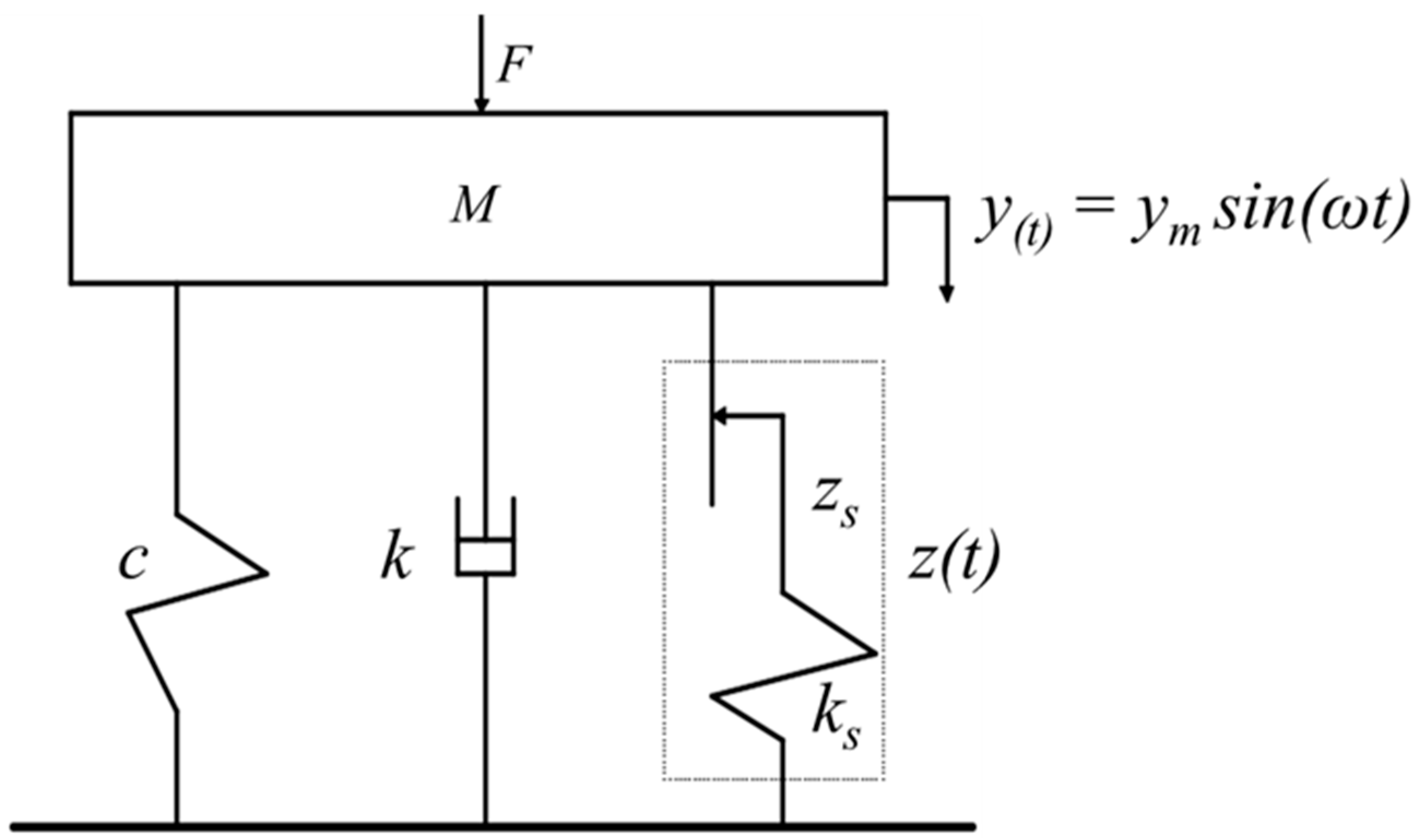
2. Development of an All-Metallic Vibration Isolator
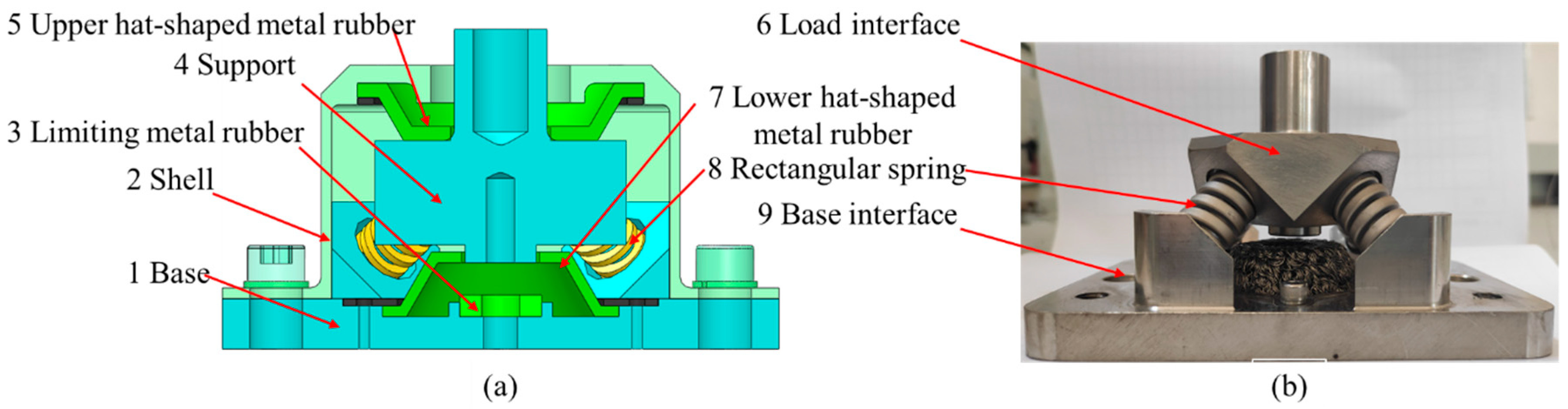
2.1. Structural Design
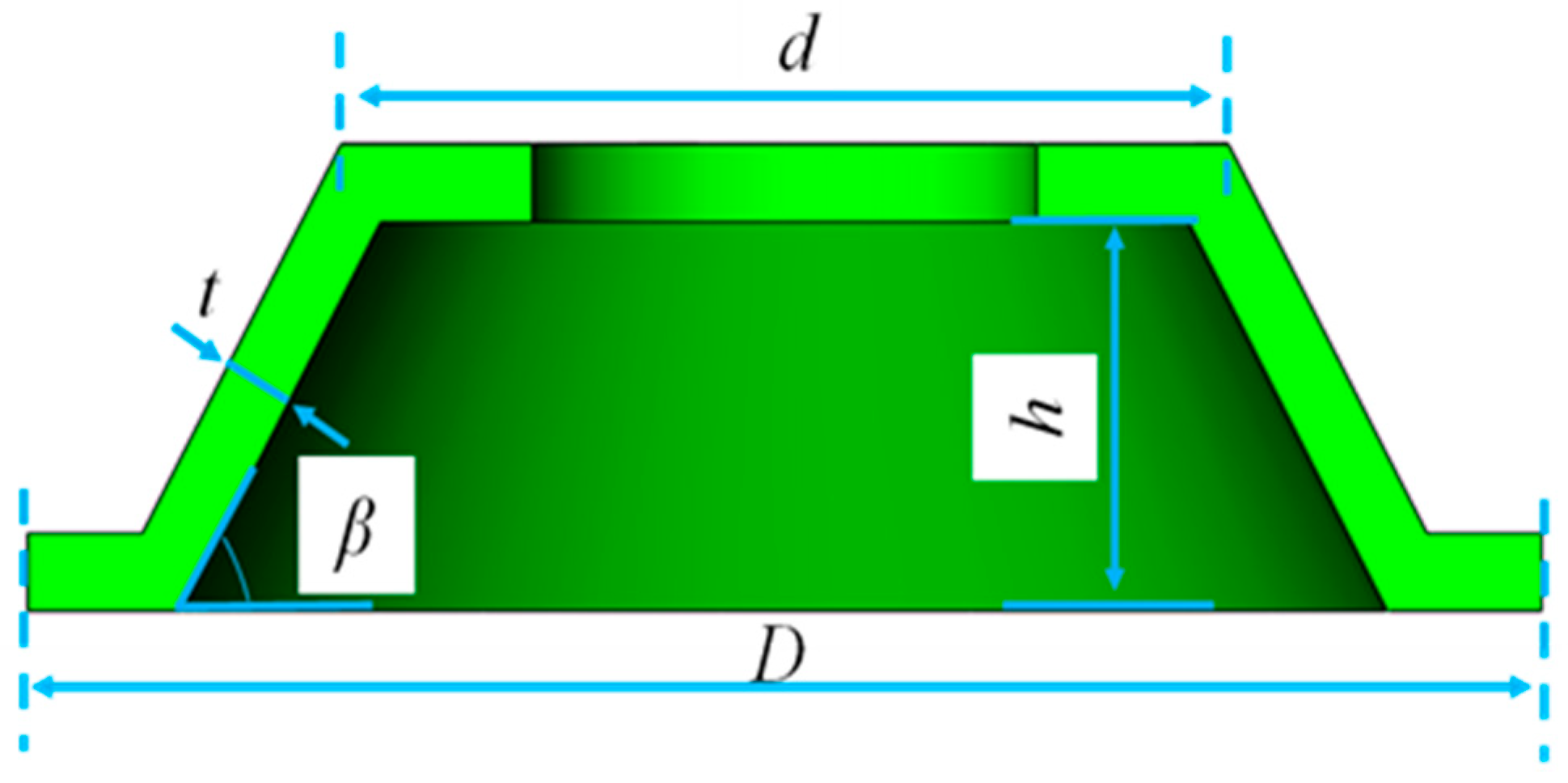
2.2. Material Selection and Main Component Parameters
3. Dynamic Test Methods and Performance Characterization
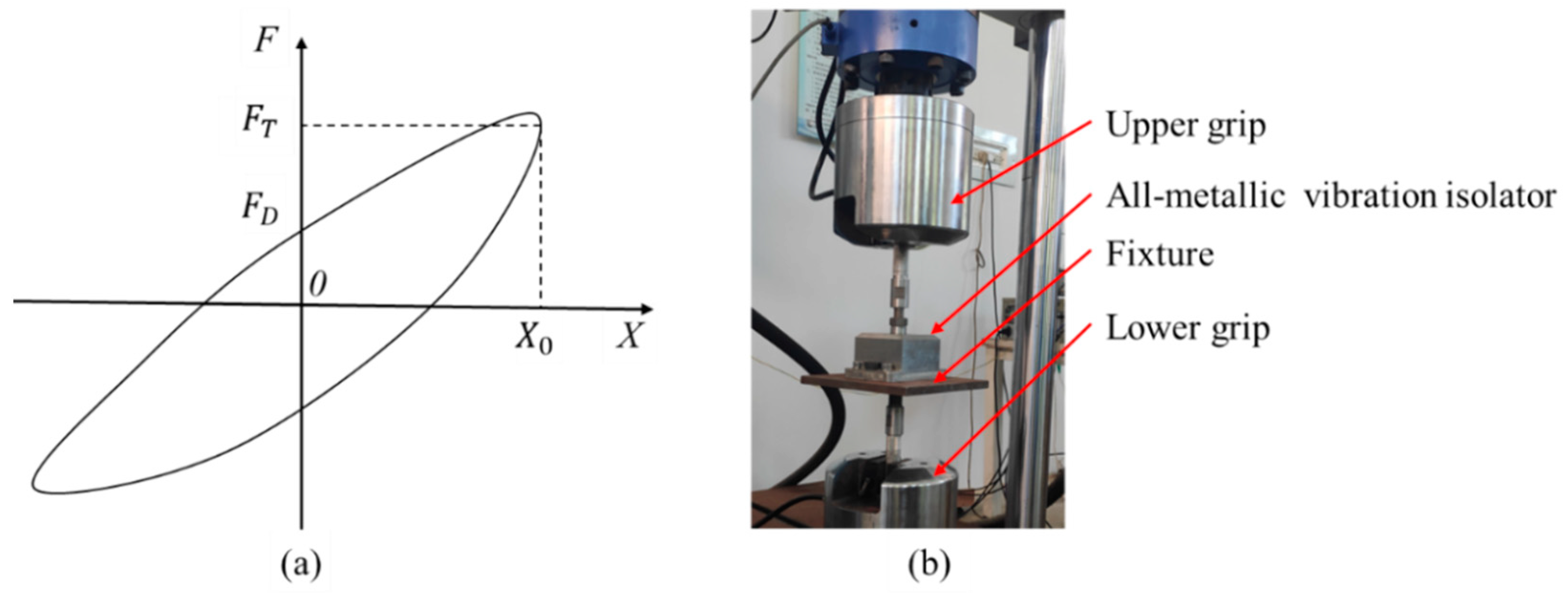
3.1. The Elliptic Method
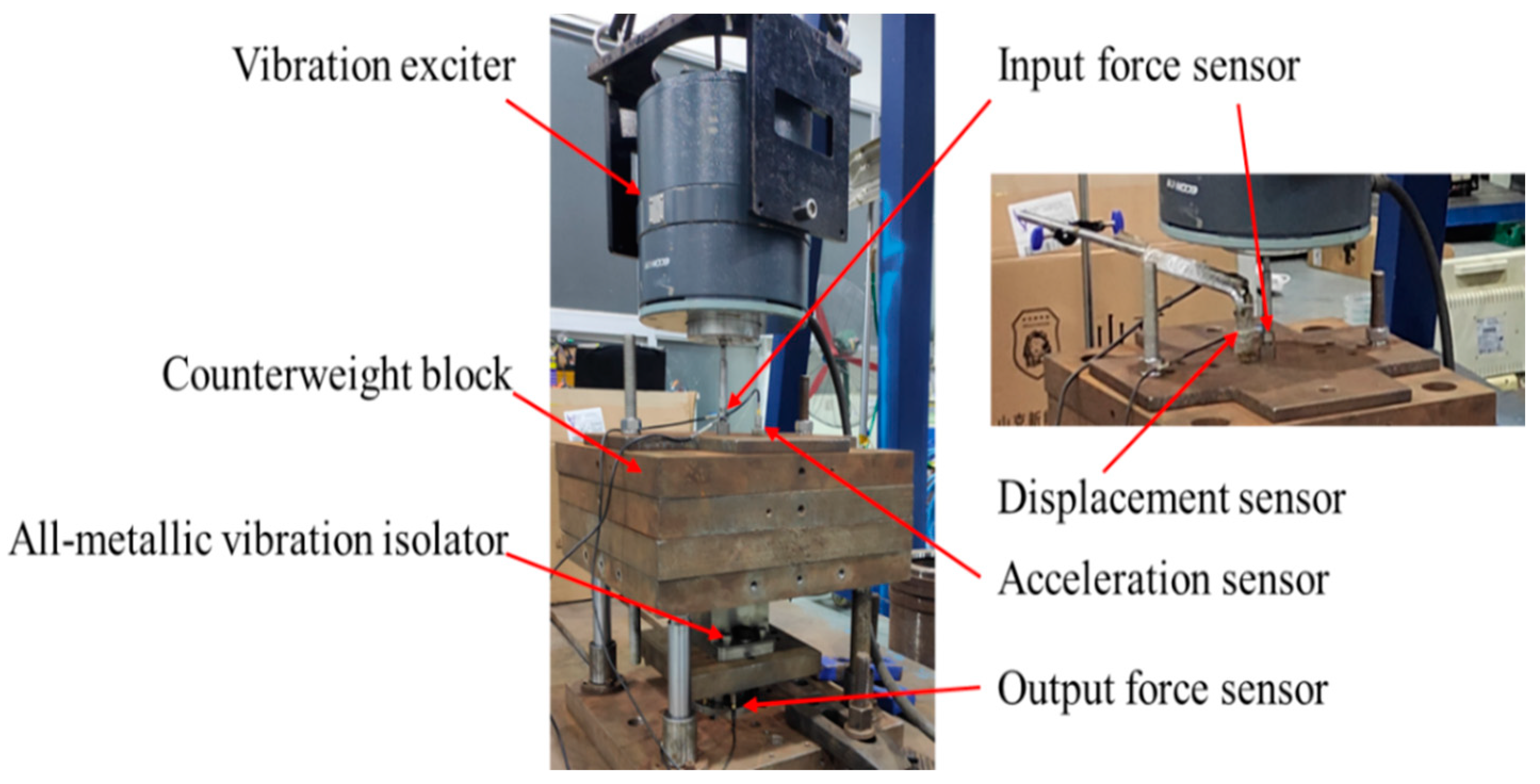
3.2. The Frequency Sweeping Method
3.3. Performance Characterization
3.3.1. Characterization of Dynamic Stiffness
3.3.2. Characterization of Loss Factor
3.3.3. Characterization of Vibration Isolation Performance
4. Results and Discussion
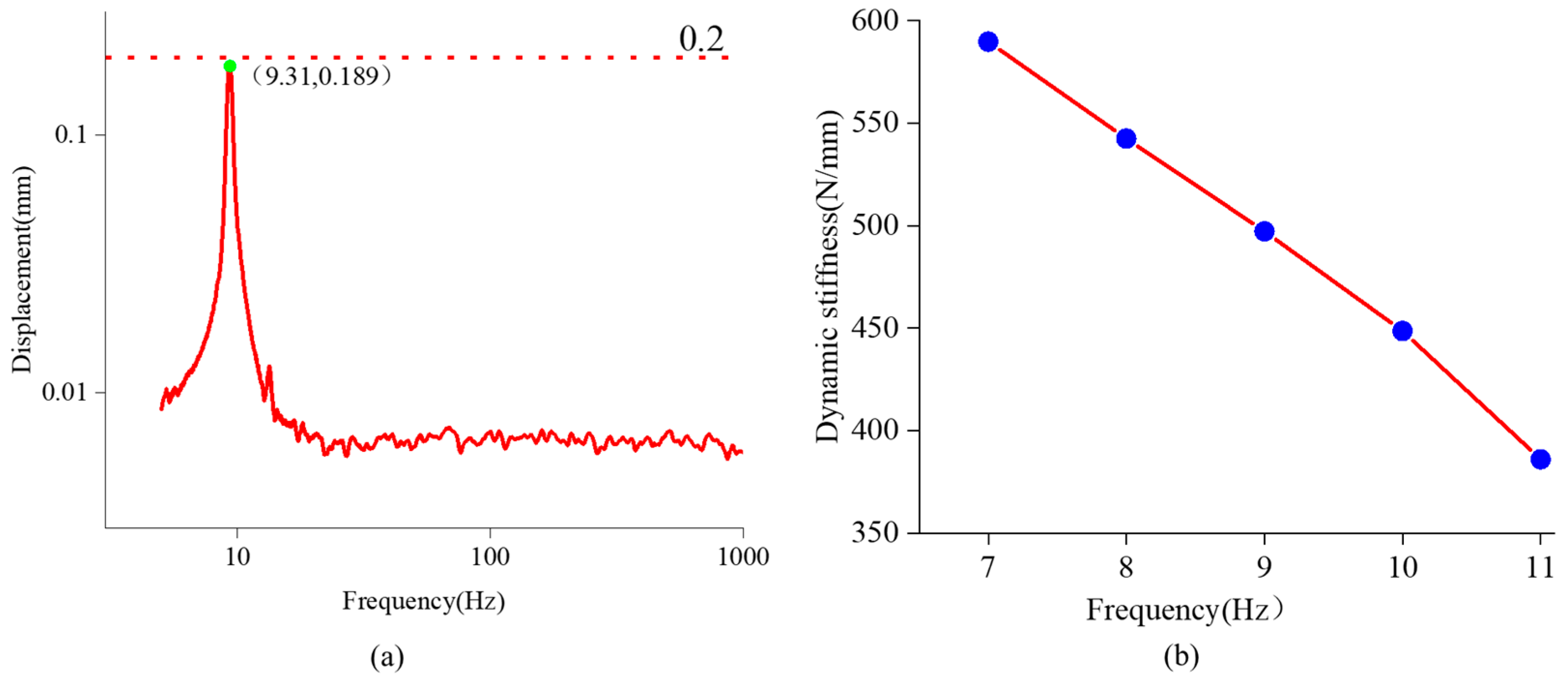
4.1. Effect of the Elliptic Method and the Frequency Sweeping Method on Dynamic Stiffness
4.2. Effect of the Elliptic Method and the Frequency Sweeping Method on Loss Factor
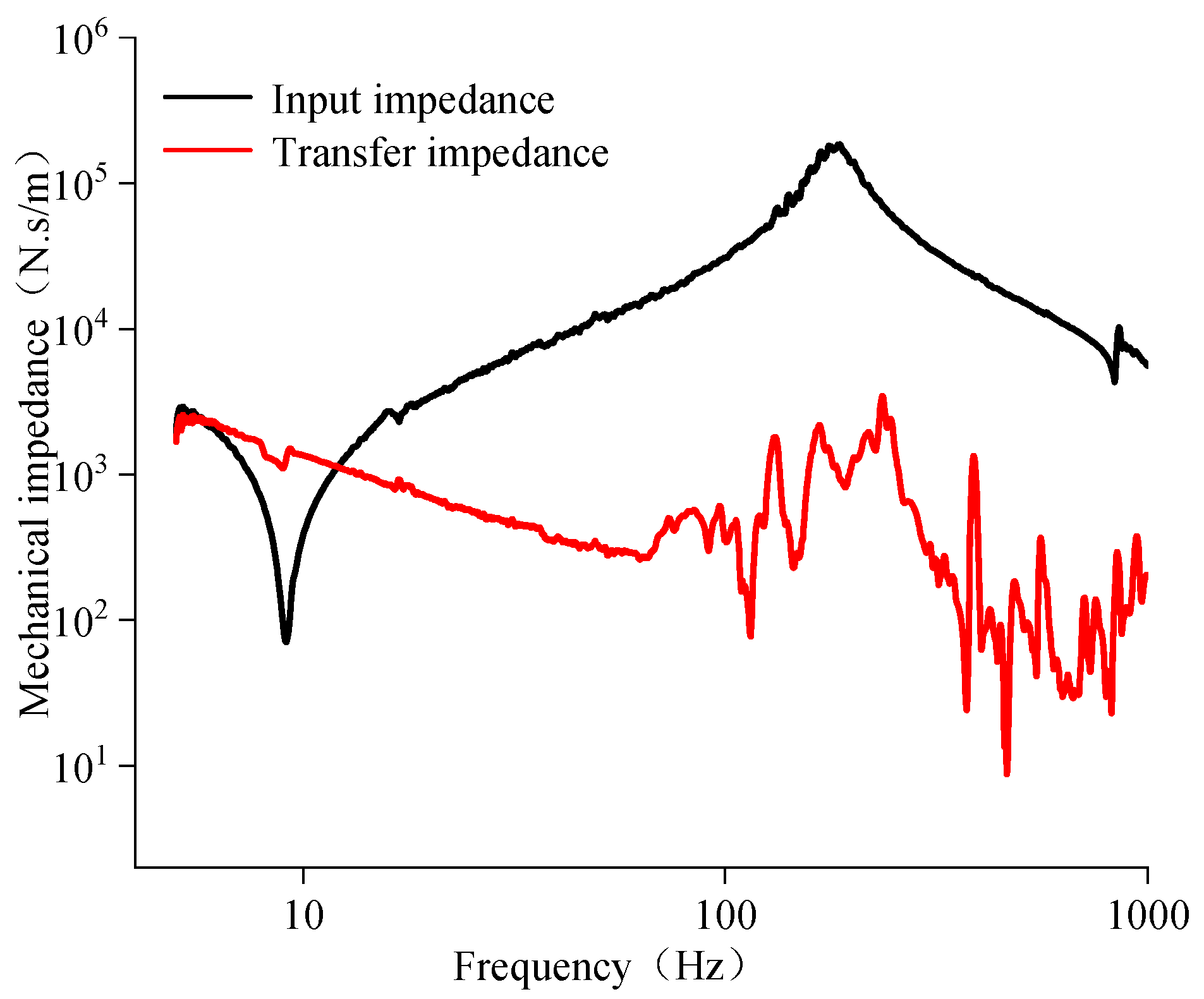
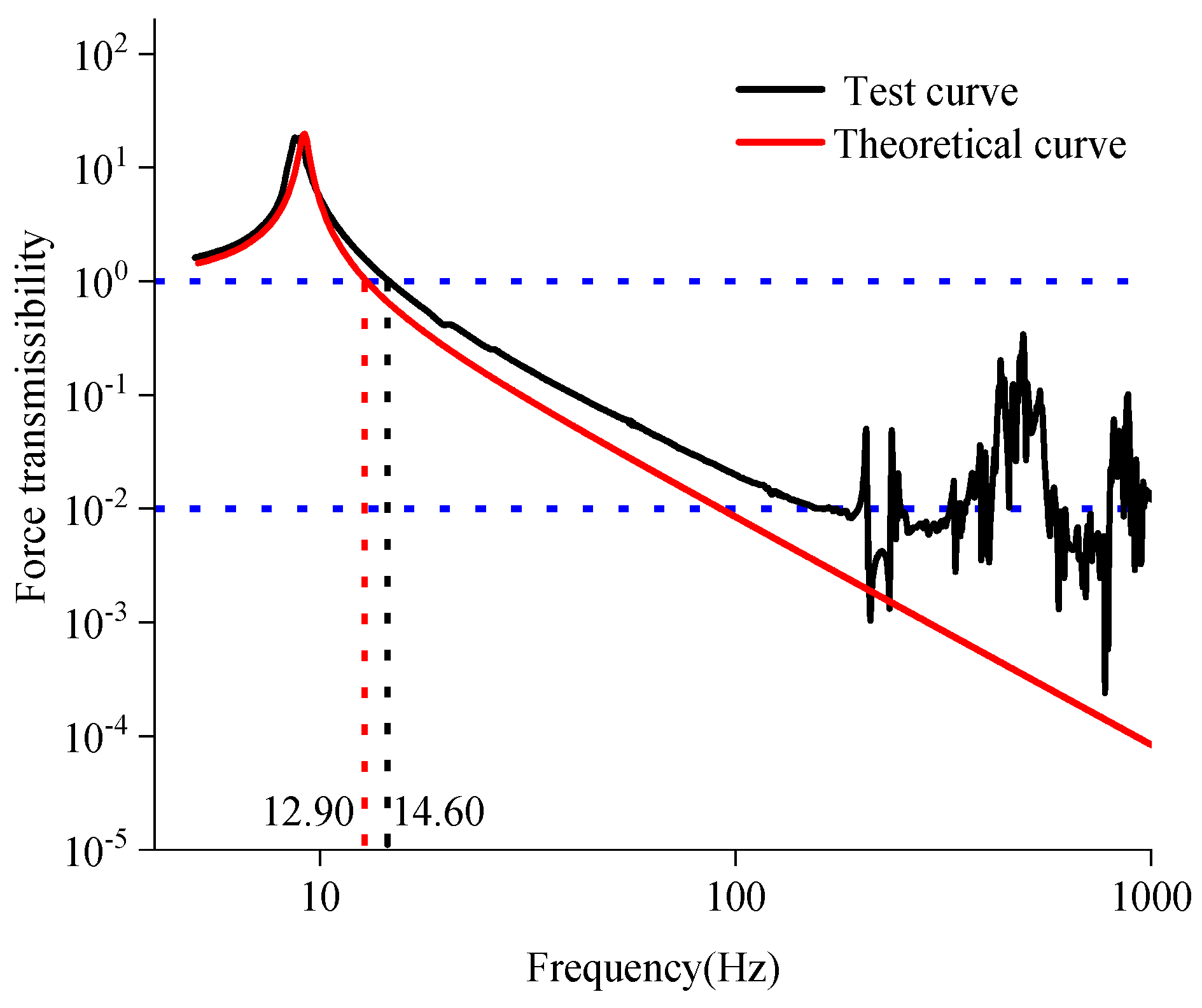
4.3. Analysis of Force Transmissibility Characteristics
5. Conclusions
- (1)
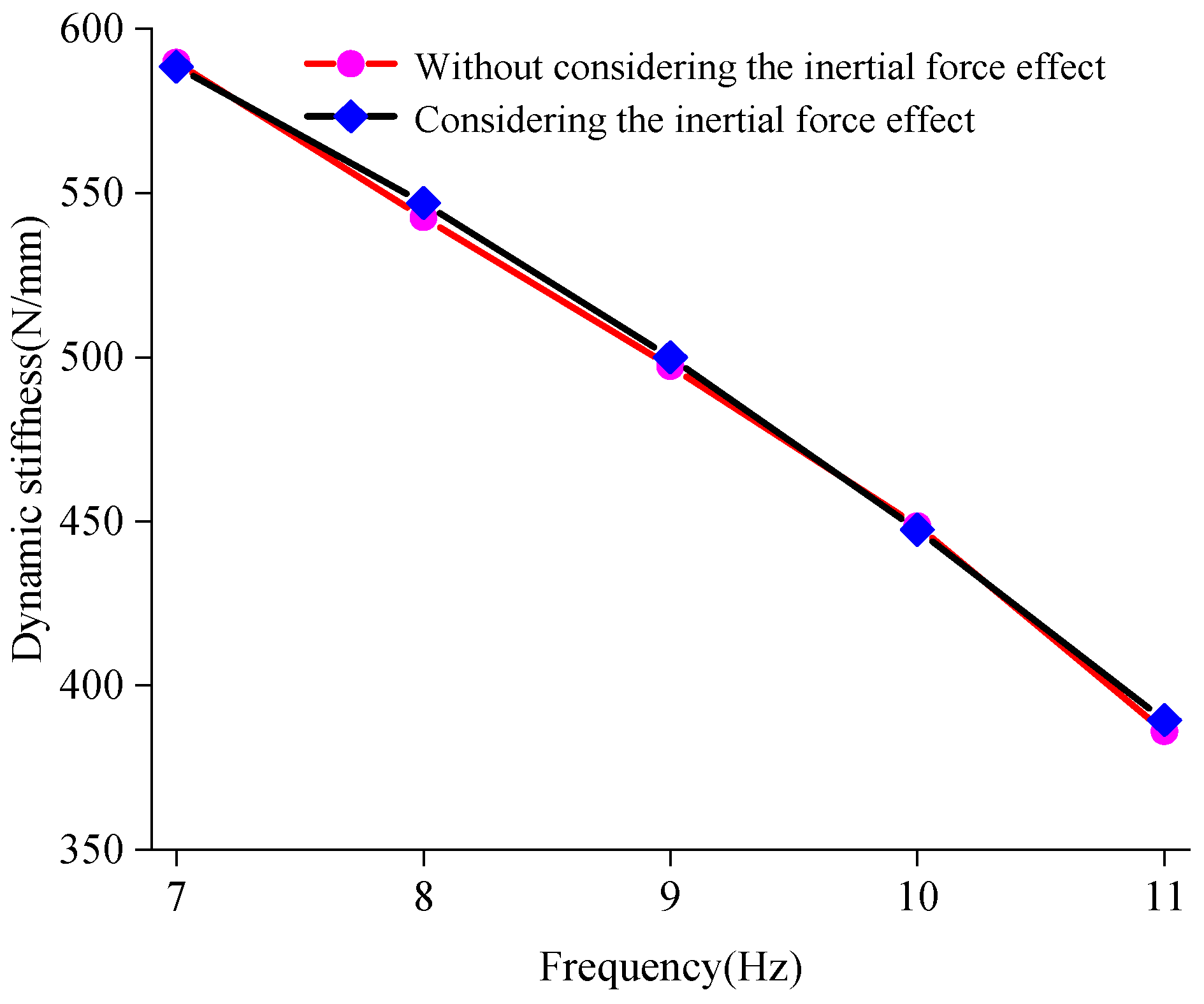
- The dynamic stiffness of the AM-VI calculated by the elliptic method was smaller than that calculated by the frequency sweeping method. However, the loss factor was the opposite. After taking the inertial force effect of the dynamic testing machine into account, the dynamic stiffness calculated by the two methods was similar.
- (2)
- There were significant differences in the dynamic stiffness and the loss factor calculated by two distinct test methodologies. It is suggested that the inertial force effect of the dynamic testing equipment should be considered when the elliptic method is used for dynamic testing.
- (3)
- The AM-VI achieved excellent vibration isolation performance within a broad frequency range. With increased excitation frequency, the force transmissibility reached 0.01.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liu, C.C.; Jing, X.J.; Daley, S.; Li, F.M. Recent advances in micro-vibration isolation. Mech. Syst. Signal Process. 2015, 56–57, 55–80. [Google Scholar] [CrossRef]
- Ibrahim, R.A. Recent advances in nonlinear passive vibration isolators. J. Sound Vib. 2008, 314, 371–452. [Google Scholar] [CrossRef]
- Kamesh, D.; Pandiyan, R.; Ghosal, A. Passive vibration isolation of reaction wheel disturbances using a low frequency flexible space platform. J. Sound Vib. 2012, 331, 1310–1330. [Google Scholar] [CrossRef]
- Kwon, S.C.; Jo, M.S.; Oh, H.U. Experimental validation of fly-wheel passive launch and on-orbit vibration isolation system by using a superelastic SMA mesh washer isolator. Int. J. Aerosp. Eng. 2017, 2017, 1–16. [Google Scholar] [CrossRef]
- Le, T.D.; Ahn, K.K. A vibration isolation system in low frequency excitation region using negative stiffness structure for vehicle seat. J. Sound Vib. 2011, 330, 6311–6335. [Google Scholar] [CrossRef]
- Song, Y.B.; Wen, J.H.; Yu, D.L.; Liu, Y.Z.; Wen, X.S. Reduction of vibration and noise radiation of an underwater vehicle due to propeller forces using periodically layered isolators. J. Sound Vib. 2014, 333, 3031–3043. [Google Scholar] [CrossRef]
- Salvatore, A.; Carboni, B.; Chen, L.Q.; Lacarbonara, W. Nonlinear dynamic response of a wire rope isolator: Experiment, identification and validation. Eng. Struct. 2021, 238, 111121. [Google Scholar] [CrossRef]
- Liu, S.Y.; Su, P.; Yang, L.H.; Feng, X.J.; Liu, H.S. Experimental investigation of a five-spring vibration isolator. J. Phys. Conf. Ser. 2020, 1707, 12007. [Google Scholar] [CrossRef]
- Cao, X.B.; Wei, C.; Liang, J.Q.; Wang, L.X. Design and dynamic analysis of metal rubber isolators between satellite and carrier rocket system. Mech. Sci. 2019, 10, 71–78. [Google Scholar] [CrossRef]
- Jin, S.Z.; Jiang, G.H.; Wu, G.; Chen, B.W.; Zou, X.Y. Simulation calculation and research of double layer metal rubber vibration isolator. Am. J. Sci. Res. Essays 2019, 4, 19. [Google Scholar]
- Xue, X.; Ruan, S.X.; Li, A.X.; Bai, H.B.; Xiao, K. Nonlinear dynamic modelling of two-point and symmetrically supported pipeline brackets with elastic-porous metal rubber damper. Symmetry 2019, 11, 1479. [Google Scholar] [CrossRef]
- Cao, F.L.; Bai, H.B.; Yang, J.C.; Ren, G.Q. Analysis on fatigue damage of metal rubber vibration isolator. Adv. Mater. Res. 2012, 1700, 490–495. [Google Scholar] [CrossRef]
- Yan, H.; Yan, X.; Zhao, Y.L.; Su, H.Y.; Jiang, H.Y.; Yang, D.S. Study on temperature mechanical properties of SMA-MR material dampers. Rare Met. Mater. Eng. 2020, 49, 1140–1143. [Google Scholar]
- Shen, X.; Chen, Y.C.; Wang, J.Q.; Li, J.F.; Chang, L.L.; Sun, Y.Y. Design, experiment and verification of resonant frequency-tunable vibration isolator based on annular metal rubbers and shape memory alloy actuators. J. Vib. Eng. Technol. 2019, 7, 277–289. [Google Scholar] [CrossRef]
- Liu, H.P.; Xiao, K.L.; Lv, Q.; Ma, Y.L. Analysis and experimental study on dynamic characteristics of an integrated quasi-zero stiffness isolator. J. Vib. Acoust.-Trans. ASME. 2022, 144, 1–28. [Google Scholar] [CrossRef]
- Dai, J.G.; Wang, Y.; Wei, M.X.; Zhang, W.W.; Zhu, J.H.; Jin, H.; Jiang, C. Dynamic characteristic analysis of the inerter-based piecewise vibration isolator under base excitation. Acta Mech. 2022, 233, 513–533. [Google Scholar] [CrossRef]
- Wang, Y.; Li, H.X.; Cheng, C.; Ding, H.; Chen, L.Q. Dynamic performance analysis of a mixed-connected inerter-based quasi-zero stiffness vibration isolator. Struct. Control. Health Monit. 2020, 27, e2604. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, J.F.; Ouyang, H.J.; Zheng, X.; Ye, J.P. Vibration analyses of linear isolators incorporating a quasi-zero stiffness and an inerter simultaneously. Vibroengineering Procedia 2020, 32, 69–74. [Google Scholar]
- Roncen, T.; Sinou, J.J.; Lambelin, J.P. Experiments and nonlinear simulations of a rubber isolator subjected to harmonic and random vibrations. J. Sound Vib. 2019, 451, 71–83. [Google Scholar] [CrossRef]
- Tang, B.; Brennan, M.J. A comparison of two nonlinear damping mechanisms in a vibration isolator. J. Sound Vib. 2013, 332, 510–520. [Google Scholar] [CrossRef]
- Xue, X.; Ruan, S.X.; Bai, H.B.; Chen, X.C.; Shao, Y.C.; Lu, C.H. An enhanced constitutive model for the nonlinear mechanical behavior of the elastic-porous metal rubber. Mech. Mater. 2020, 148, 103447. [Google Scholar] [CrossRef]
- Xue, X.; Yang, P.; Shao, Y.C.; Bai, H.B. Manufacture technology and anisotropic behavior of elastic-porous metal rubber. Int. J. Lightweight Mater. Manuf. 2019, 3, 88–99. [Google Scholar]
- Haym, B.; Mark, L.; Nagurka, S.M.H. Mechanical Vibration; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Wang, S.L.; Bai, H.B.; Lu, C.H. The research progress and application expectation of metal rubber vibration isolator. Int. Conf. Mater. 2015, 10, 687–692. [Google Scholar]
- Yerrawar, R.N.; Arakerimath, R.R. Experimental investigations of damping characteristic for MR strut by half-power bandwidth method. J. Meas. Eng. 2018, 6, 163–172. [Google Scholar] [CrossRef]
- Valiente-Blanco, I.; Perez-Diaz, J.L.; Cristache, C. Potential reduction of the inertial mass of tuned vibration absorbers by means of mechanical impedance matching. J. Sound Vib. 2018, 442, 90–107. [Google Scholar] [CrossRef]
- Lu, Z.Q.; Brennan, M.; Ding, H.; Chen, L.Q. High-static-low-dynamic-stiffness vibration isolation enhanced by damping nonlinearit. Sci. China 2019, 62, 1103–1110. [Google Scholar] [CrossRef]
- Lang, Z.Q.; Jing, X.J.; Billings, S.A.; Tomlinson, G.R.; Peng, Z.K. Theoretical study of the effects of nonlinear viscous damping on vibration isolation of SDOF systems. J. Sound Vib. 2009, 323, 352–365. [Google Scholar] [CrossRef]


| Structural Parameter | Values | |
|---|---|---|
| Upper Hat-Shaped Metal Rubber | Lower Hat-Shaped Metal Rubber | |
| Lower surface diameter (D)/mm | 80 | 66 |
| Upper surface diameter (d)/mm | 54.14 | 38.68 |
| Thickness (t)/mm | 4 | 3 |
| Sidewall tilt angle (β)/° | 60 | 60 |
| Height of the inner wall (h)/mm | 12 | 15 |
| Rectangular Spring | Model Specifications | Designed Stiffness | Nominal Stiffness | Actual Stiffness |
|---|---|---|---|---|
| TB20/35 | 242.5 N/mm | 224 N/mm | 214.3 N/mm | |
| Metal rubber | Material | Wire diameter | Coil diameter | Spiral coil pitch |
| 06Cr19Ni10 (304) | 0.3 mm | 3.6 mm | 3.6 mm | |
| Vibration isolator | Load rating | Mass | Base interface | Load interface |
| 2 kN | 4.3 kg | M14 | M18 |
| Dynamic Test Methods | Calculation Model | |
|---|---|---|
| Elliptic method | Without considering the inertial force effect of the dynamic test machine | |
| Considering the inertial force effect of the dynamic test machine | ||
| Sweep frequency method | ||
| Dynamic Test Method | Calculation Model |
|---|---|
| Elliptic method | |
| Sweep frequency method |
| Vibration Isolation Performance Parameter | Calculation Model | |
|---|---|---|
| Impedance | Input impedance | |
| Transfer impedance | ||
| Force transmissibility | ||
| Dynamic Test Method | Frequency (Hz) | Stiffness Dynamic (N/mm) |
|---|---|---|
| Sweep frequency method | 9.31 | 677.52 |
| Elliptic Method | 9 | 497.21 |
| Excitation Frequency | Dynamic Stiffness (N/mm) | |
|---|---|---|
| Considering the Inertial Force Effect | Without Considering the Inertial Force Effect | |
| 7 | 589.74 | 588.42 |
| 8 | 542.46 | 546.97 |
| 9 | 497.21 | 499.99 |
| 10 | 448.6 | 447.48 |
| 11 | 386.03 | 389.45 |
| Dynamic Test Method | Frequency (Hz) | Dynamic Stiffness (N/mm) | |
|---|---|---|---|
| Elliptic Method | The inertial force effect is not considered | 9 | 497.21 |
| The inertial force effect is considered | 9 | 721.05 | |
| Sweep frequency method | 9.31 | 677.52 | |
| Excitation Frequency (Hz) | Loss Factor |
|---|---|
| 7 | 0.075 |
| 8 | 0.079 |
| 9 | 0.083 |
| 10 | 0.073 |
| 11 | 0.078 |
| Dynamic Test Method | Frequency (Hz) | Loss Factor |
|---|---|---|
| Sweep frequency method | 9.31 | 0.060 |
| Elliptic Method | 9 | 0.083 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zou, L.; Zheng, C.; Zheng, Z.; Hu, F.; Shao, Y.; Xue, X. Comparison of Dynamic Performance of an All-Metallic Vibration Isolator by Elliptic Method and Frequency Sweeping Method. Symmetry 2022, 14, 2017. https://doi.org/10.3390/sym14102017
Zou L, Zheng C, Zheng Z, Hu F, Shao Y, Xue X. Comparison of Dynamic Performance of an All-Metallic Vibration Isolator by Elliptic Method and Frequency Sweeping Method. Symmetry. 2022; 14(10):2017. https://doi.org/10.3390/sym14102017
Chicago/Turabian StyleZou, Luming, Chao Zheng, Zhi Zheng, Feng Hu, Yichuan Shao, and Xin Xue. 2022. "Comparison of Dynamic Performance of an All-Metallic Vibration Isolator by Elliptic Method and Frequency Sweeping Method" Symmetry 14, no. 10: 2017. https://doi.org/10.3390/sym14102017
APA StyleZou, L., Zheng, C., Zheng, Z., Hu, F., Shao, Y., & Xue, X. (2022). Comparison of Dynamic Performance of an All-Metallic Vibration Isolator by Elliptic Method and Frequency Sweeping Method. Symmetry, 14(10), 2017. https://doi.org/10.3390/sym14102017







