New Solution of the Sine-Gordon Equation by the Daftardar-Gejji and Jafari Method

Abstract

In this article, the Daftardar-Gejji and Jafari method (DJM) is used to obtain an approximate analytical solution of the sine-Gordon equation. Some examples are solved to demonstrate the accuracy of DJM. A comparison study between DJM, the variational iteration method (VIM), and the exact solution are presented. The comparison of the present symmetrical results with the existing literature is satisfactory.

1. Introduction

Differential equations are highly effective tools used to describe real-life phenomena; in most cases, the numerical or theoretical solutions are difficult to find. In recent years, searching for new methods to solve nonlinear differential equations has received increased attention, see [1,2,3].

In this paper, we will apply the Daftardar-Gejji and Jafari method (DJM) to find the solution of the sine-Gordon nonlinear equation:

$$\begin{array}{c}\hfill u_{tt}-u_{xx}+sin(u)=0.\end{array}$$

(1)

In [4] Herbst et al. used an explicit symplectic method to find numerical results of the sine-Gordon equation. (Wazwaz, 2005) [5] Found new exact solutions to the sine-Gordon equation by using the tanh method. (Kaya, 2003) [6] presented the approximate analytical solution of the sine-Gordon equation by means of the modified decomposition method. (Ray, 2006) [7] applied the modified decomposition method to obtain the solution of coupled sine-Gordon equations. (Batiha et al., 2007) [8] applied the variational iteration method (VIM) to obtain an approximate analytical solution of the sine-Gordon equation.

The Daftardar–Gejji and Jafari method (DJM) was presented by Daftardar-Gejji and Jafari [9]; the DJM was used successfully for solving nonlinear differential equations, see references [10,11,12,13,14,15,16,17,18,19,20,21,22]. DJM has been used to create a new predictor–corrector method [23,24]. In [25,26,27,28,29] Noor et al applied DJM to solve algebraic equations.

In this article, the analytical solution of the sine-Gordon Equation (1) is found by using the DJM. Comparisons with the variational iteration method (VIM) and the exact solution will be presented. The results obtained are symmetrical with VIM and exact solution results.

2. The Daftardar-Gejji and Jafari Method

In this paper, we will discuss the Daftardar-Gejji and Jafari method, and how to use it for solving nonlinear differential equations in the form:

$$\begin{array}{c}\hfill y=f+L(y)+N(y),\end{array}$$

(2)

where L, N are linear and non-linear operators, f is an arbitrary function. The above equation has a solution in the form:

$$\begin{array}{c}\hfill y=\sum _{i=0}^{\infty }{y}_i.\end{array}$$

(3)

Suppose we have,

$$\begin{array}{ccc}\hfill H_0& =& N(y_0),\hfill \end{array}$$

(4)

$$\begin{array}{ccc}\hfill H_m& =& N\left(\sum _{i=0}^m{y}_i\right)-N\left(\sum _{i=0}^{m-1}{y}_i\right).\hfill \end{array}$$

(5)

Then we obtain,

$$\begin{array}{ccc}\hfill H_0& =& N(y_0),\hfill \end{array}$$

(6)

$$\begin{array}{ccc}\hfill H_1& =& N(y_0+y_1)-N(y_0),\hfill \end{array}$$

(7)

$$\begin{array}{ccc}\hfill H_2& =& N(y_0+y_1+y_2)-N(y_0+y_1),\hfill \end{array}$$

(8)

$$\begin{array}{ccc}\hfill H_3& =& N(y_0+y_1+y_2+y_3)-N(y_0+y_1+y_2)+\cdots .\hfill \end{array}$$

(9)

Thus, $N(y)$ is decomposed as:

$$\begin{array}{ccc}\hfill N\left(\sum _{i=0}^{\infty }{y}_i\right)& & =N(y_0)+N(y_0+y_1)-N(y_0)+N(y_0+y_1+y_2)-N(y_0+y_1)\hfill \\ & & +N(y_0+y_1+y_2+y_3)-N(y_0+y_1+y_2)+\cdots .\hfill \end{array}$$

(10)

So, the recurrence relation is as the following form:

$$\begin{array}{ccc}\hfill y_0& =& f\hfill \\ \hfill y_1& =& L(y_0)+H_0\hfill \\ \hfill y_{m+1}& =& L(y_m)+H_m,m=1,2,\cdots .\hfill \end{array}$$

(11)

Since L is linear, then:

$$\begin{array}{c}\hfill \sum _{i=0}^{m}L(y_i)=L\left(\sum _{i=0}^m{y}_i\right).\end{array}$$

(12)

So,

$$\begin{array}{ccc}\hfill \sum _{i=0}^{m+1}{y}_i& =& \sum _{i=0}^{m}L(y_i)+N\left(\sum _{i=0}^m{y}_i\right).\hfill \\ & =& L\left(\sum _{i=0}^m{y}_i\right)+N\left(\sum _{i=0}^m{y}_i\right),m=1,2,\cdots \hfill \end{array}$$

(13)

Thus,

$$\begin{array}{ccc}\hfill \sum _{i=0}^{\infty }{y}_i& =& f+L\left(\sum _{i=0}^{\infty }{y}_i\right)+N\left(\sum _{i=0}^{\infty }{y}_i\right).\hfill \end{array}$$

(14)

We can form the solution in the $k$-term as follows:

$$\begin{array}{c}\hfill y=\sum _{i=0}^{k-1}{y}_i.\end{array}$$

(15)

3. The Method Convergence

Theorem 1.

If N is $C^{(\infty )}$ in a neighborhood of $u_0$ and $\left|\right|N^{(n)}(u_0)\left|\right|\le L$, for any n and for some real $L>0$ and $\left|\right|u_i\left|\right|\le M<\frac{1}{e},i=1,2,\dots ,$ then ${\sum }_{n=0}^{\infty }{H}_n$ is convergent absolutely, $\left|\right|H_n\left|\right|\le L{M}^n{e}^{n-1}(e-1),n=1,2,\dots$.

Proof. 

You can find the detailed proof in [14]. □

Theorem 2.

If N is $C^{(\infty )}$ and $\left|\right|N^{(n)}(u_0)\left|\right|\le M\le e^{-1},\forall n$, then ${\sum }_{n=0}^{\infty }{H}_n$ is convergent absolutely.

Proof. 

You can find the detailed proof in [14]. □

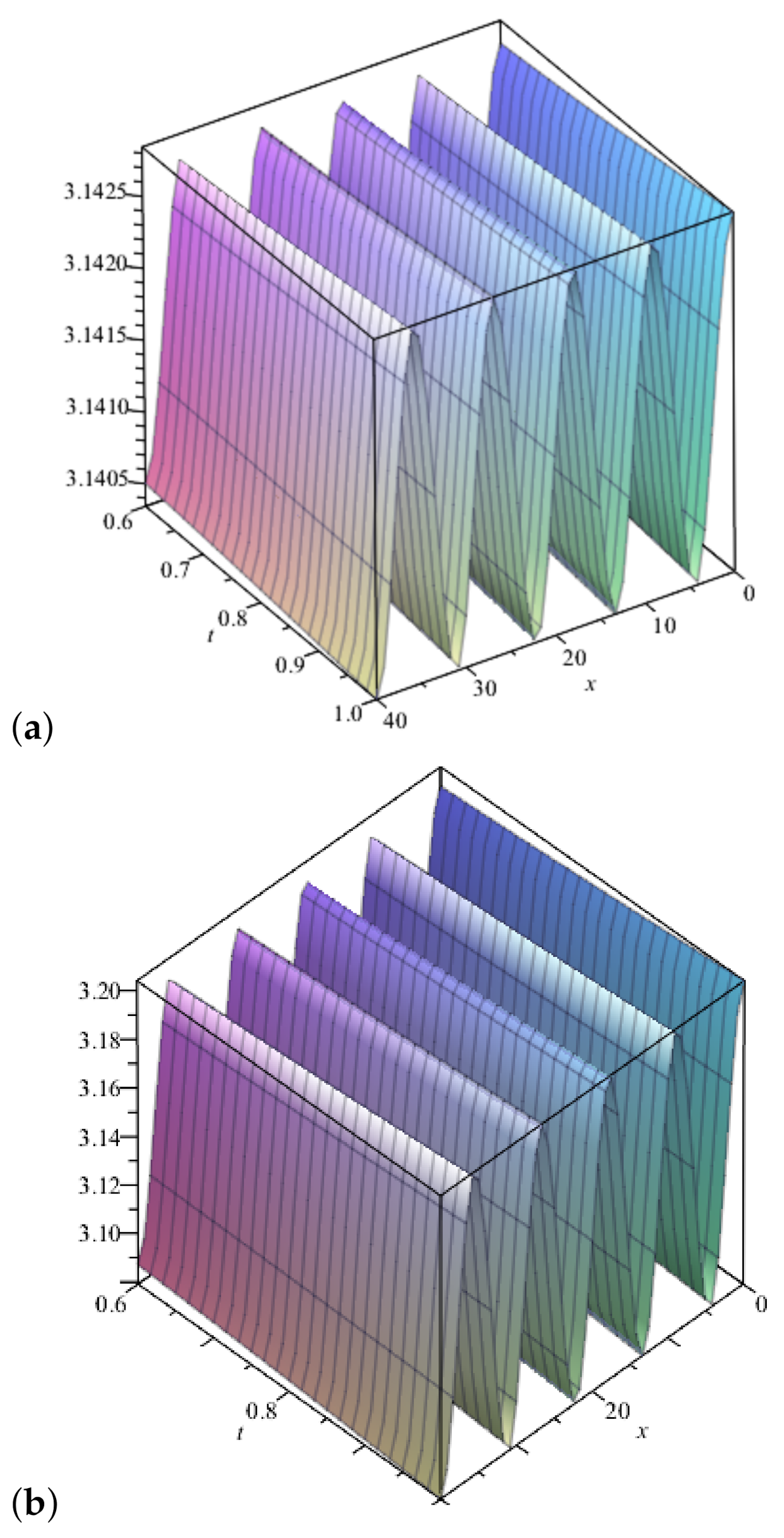
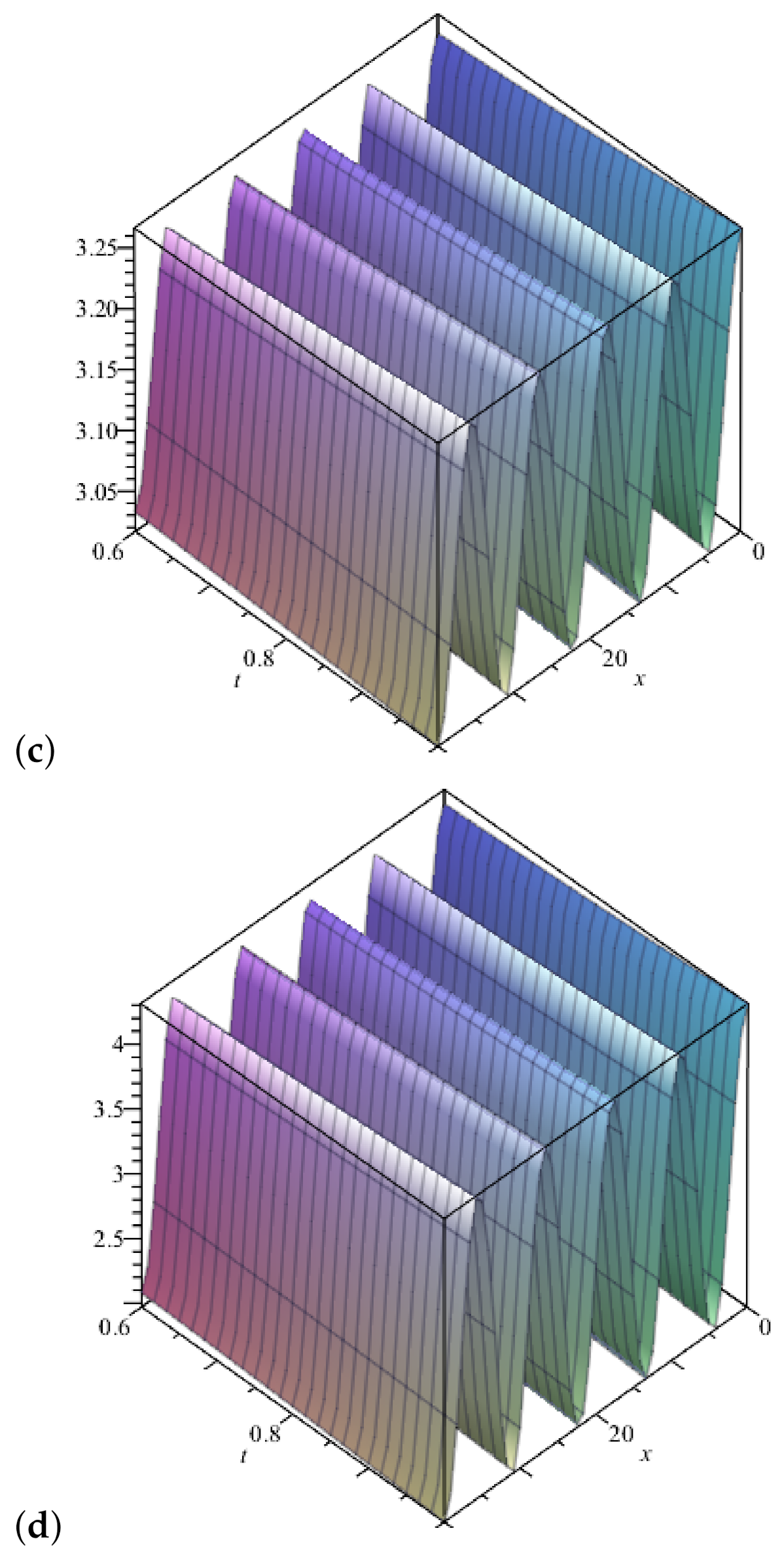
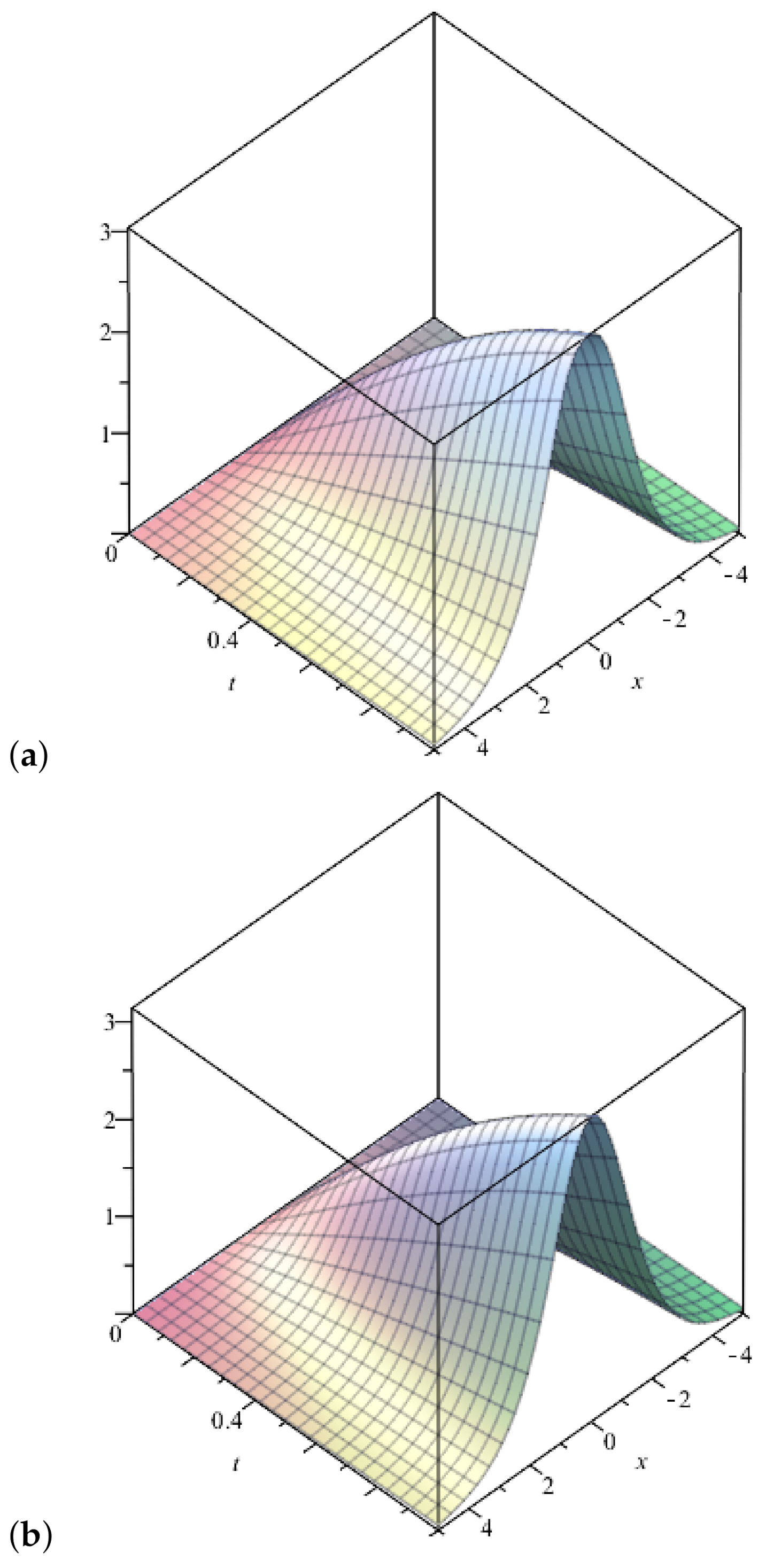
4. Numerical Applications

Here, we will use DJM to find the solution of the sine-Gordon Equation (1).

4.1. Example 1

Example 1.

We will examine the pendulum-like equation in the form:

$$\begin{array}{ccc}\hfill \frac{{\mathrm{d}}^{2}u}{\mathrm{d}{t}^2}& =& sinu,\hfill \end{array}$$

(16)

$$\begin{array}{ccc}\hfill u(0)& =& \pi ,\frac{\mathrm{d}u}{\mathrm{d}t}(0)=-2.\hfill \end{array}$$

(17)

The Equation (16) with initial conditions (17) arises from the sine-Gordon Equation (1) [30].

The implicit solution of Equations (16) and (17) was found to be [30]:

$$\begin{array}{c}\hfill sin\frac{1}{2}u=\mathrm{sech}t.\end{array}$$

(18)

To solve Equation (16) by DJM, we integrate Equation (16) and use Equation (17) to obtain:

$$\begin{array}{c}\hfill u=\pi -2t+{\int }_0^t{\int }_0^{t}sin(u)dtdt.\end{array}$$

(19)

By using algorithm (11) we obtain:

$$\begin{array}{ccc}\hfill u_0& =& \pi -2t,\hfill \\ \hfill u_1& =& t/2-1/4sin\left(2t\right),\hfill \\ & & ⋮\hfill \end{array}$$

Thus,

$$\begin{array}{ccc}\hfill \sum _{i=0}^1{u}_i& =& \pi -\frac{3t}{2}-\frac{sin(2t)}{4}.\hfill \end{array}$$

(20)

Which is the same result as [8] obtained by VIM.

4.2. Example 2

Example 2.

Here, consider the sine-Gordon Equation (1) with ICs: Note, we used Maple 18 with 16 digits.

$$\begin{array}{c}\hfill u(x,0)=\pi +\gamma cos(\beta x),u_t(x,0)=0,\end{array}$$

(21)

where γ is any constant and $\beta =\sqrt{2}/2=0.7071067811865475$.

The sine-Gordon Equation (1) with initial condition (21) is equivalent to the following integral equation:

$$\begin{array}{c}\hfill u=\pi +\gamma cos\left(\beta x\right)+{\int }_0^t{\int }_0^t{u}_{xx}-sin(u)dtdt.\end{array}$$

(22)

In view of the algorithm (11) we find:

$$\begin{array}{ccc}\hfill u_0& =& \pi +\gamma cos\left(\beta x\right),\hfill \\ \hfill u_1& =& 1/2\left(-\gamma cos\left(\beta x\right){\beta }^2+sin\left(\gamma cos\left(\beta x\right)\right)\right)t^2,\hfill \\ & & ⋮\hfill \end{array}$$

(23)

Thus,

$$\begin{array}{c}\hfill \sum _{i=0}^1{u}_i=\pi +\gamma cos\left(\beta x\right)-1/2\left(\gamma cos\left(\beta x\right){\beta }^2-sin\left(\gamma cos\left(\beta x\right)\right)\right)t^2.\end{array}$$

(24)

Which is exactly the same result as [8] found by VIM.

To prove the stability of the DJM, we choose four γ values ($\gamma =0.001$, $\gamma =0.05$, $\gamma =0.1$ and $\gamma =1.0$); these values were chosen by Batiha et al. [8]. Here, Equation (24) was used to draw the graphs in Figure 1.

Figure 1 shows the stability of DJM for different γ values, and the accuracy of DJM for solving the sine-Gordon equation.

4.3. Example 3

Example 3.

Here, obtain the solution of the sine-Gordon Equation (1) with ICs:

$$\begin{array}{c}\hfill u(x,0)=0,u_t(x,0)=4\mathrm{sech}(x).\end{array}$$

(25)

The exact solution is:

$$\begin{array}{c}\hfill u(x,t)=4{tan}^{-1}[t\mathrm{sech}(x)].\end{array}$$

(26)

For solving Equation (1) by DJM, we integrate Equation (1) and use Equation (25) to obtain:

$$\begin{array}{c}\hfill u=4t\mathrm{sech}\left(x\right)+{\int }_0^t{\int }_0^t{u}_{xx}-sin(u)dtdt.\end{array}$$

(27)

By using algorithm (11) we find:

$$\begin{array}{ccc}\hfill u_0& =& 4ts\hfill \\ \hfill u_1& =& \frac{64t^3{s}^3{\left(tanh\left(x\right)\right)}^2-32t^3{s}^3-12cosh\left(x\right)s^{2}t+3sin\left(4ts\right)}{{48s}^2}.\hfill \\ \hfill ⋮\end{array}$$

(28)

where $s=\mathrm{sech}(x)$, thus:

$$\begin{array}{c}\hfill \sum _{i=0}^1{u}_i=4ts+\frac{64t^3{s}^3{\left(tanh\left(x\right)\right)}^2-32t^3{s}^3-12cosh\left(x\right)s^{2}t+3sin\left(4ts\right)}{{48s}^2}.\end{array}$$

(29)

This is exactly the same result as Batiha et al. [8] obtained by VIM. In Figure 2 we show the comparisons between one iteration DJM and the exact solution (26). Figure 2 clearly shows the excellent accuracy of DJM compared to the exact solution.

5. Conclusions

In this article, the DJM was used to obtain the solutions of the sine-Gordon equation with remarkable success. Comparisons with the variation iteration method (VIM) and the exact solution show that DJM is a very promising technique for solving nonlinear partial differential equations. In future research, DJM should be used to solve fractional differential equations.