Balanced Gain-and-Loss Optical Waveguides: Exact Solutions for Guided Modes in Susy-QM
Abstract
1. Introduction
2. Mathematical Physics of Graded-Index Waveguides
2.1. Adding Propagation Constants under Prescription
2.2. Balanced Gain-and-Loss Waveguides
2.2.1. Bi-Orthogonality
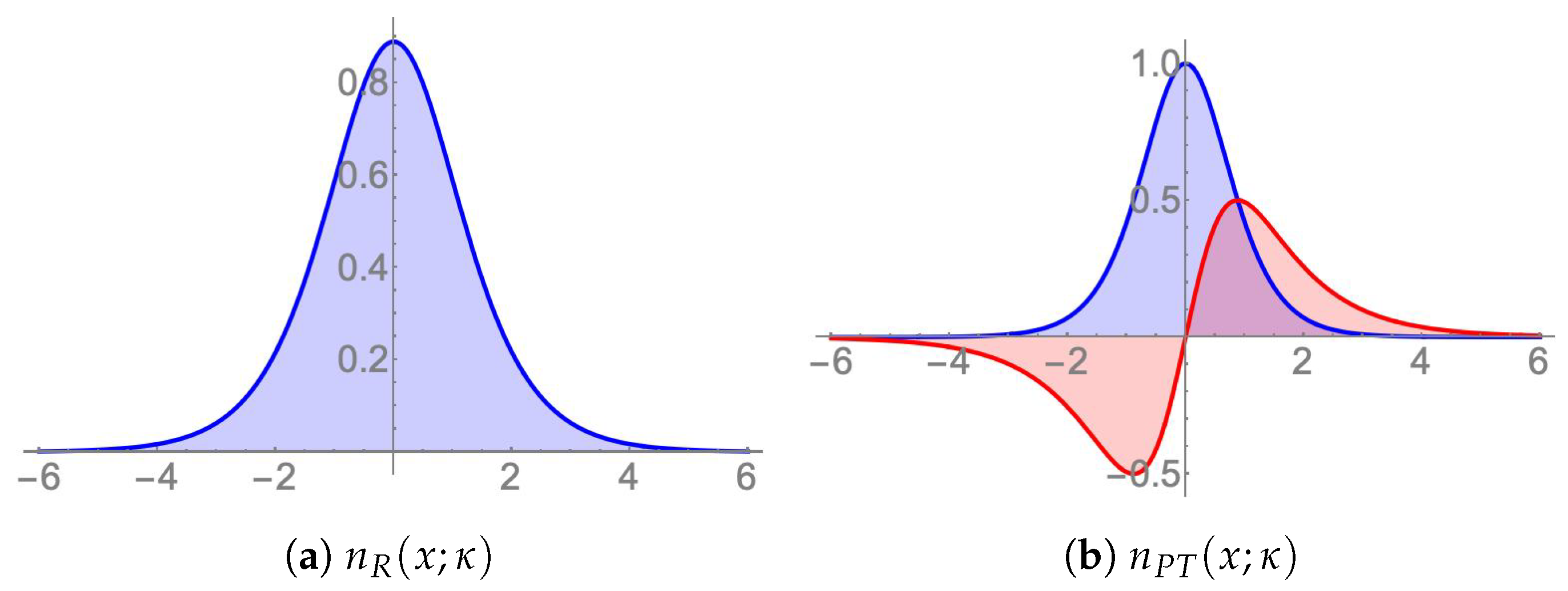
2.2.2. PT-Symmetric Case
2.2.3. Recovering the Real-Valued Case
3. Applications
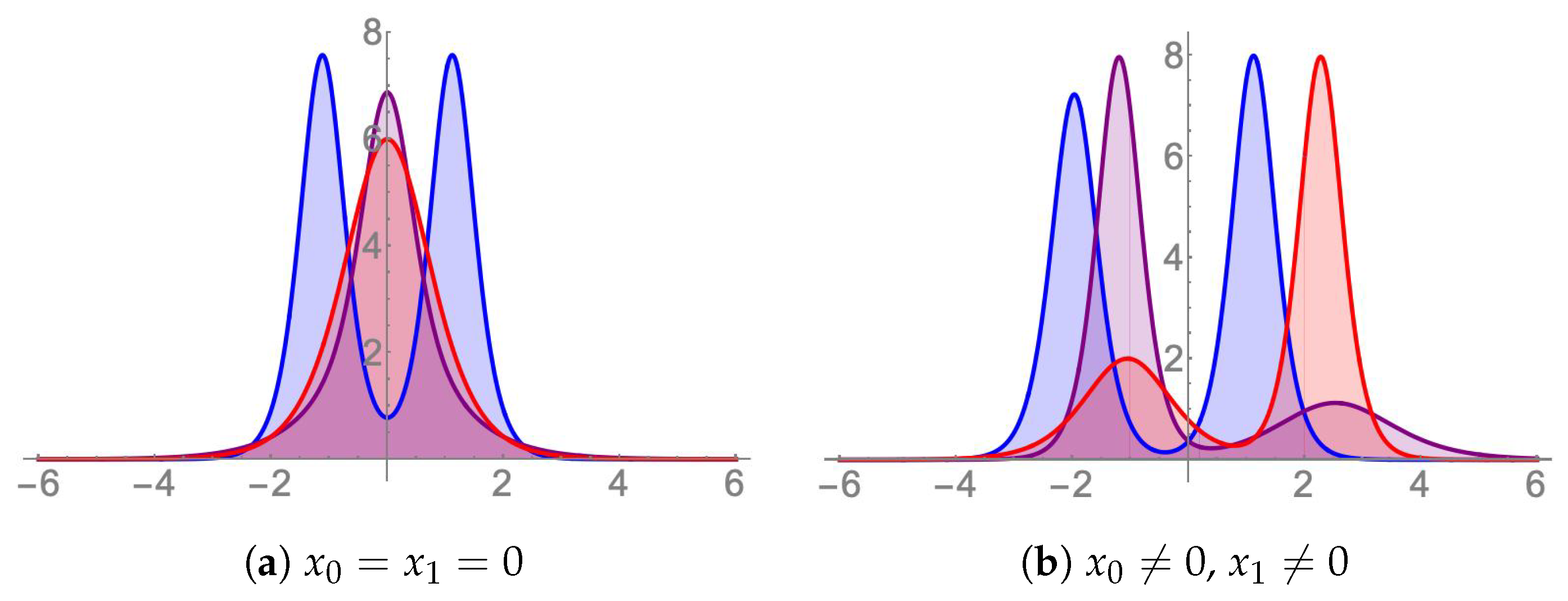
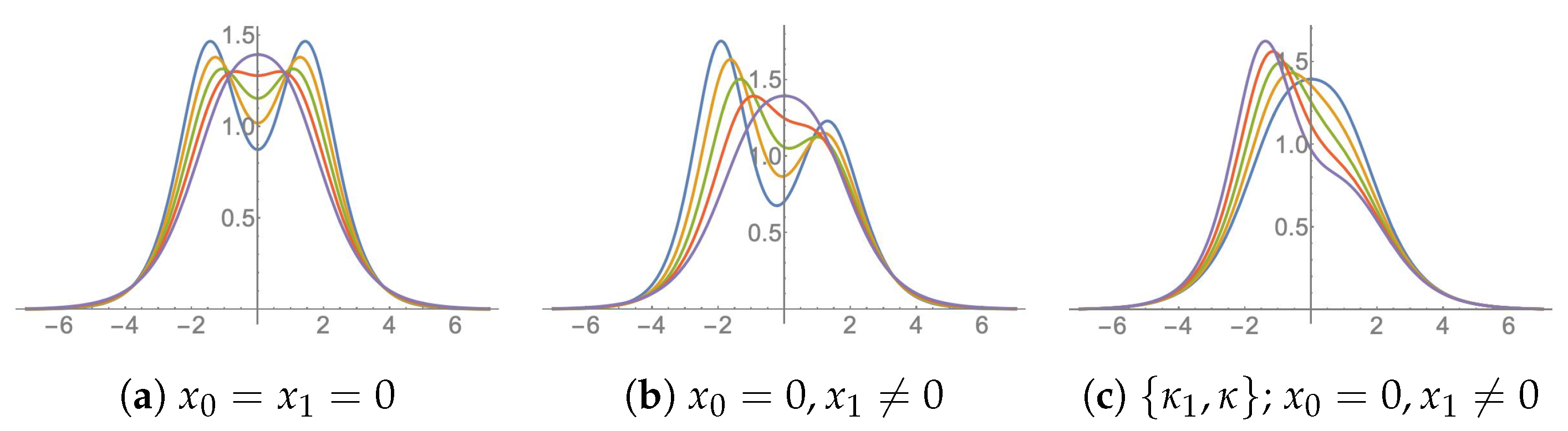
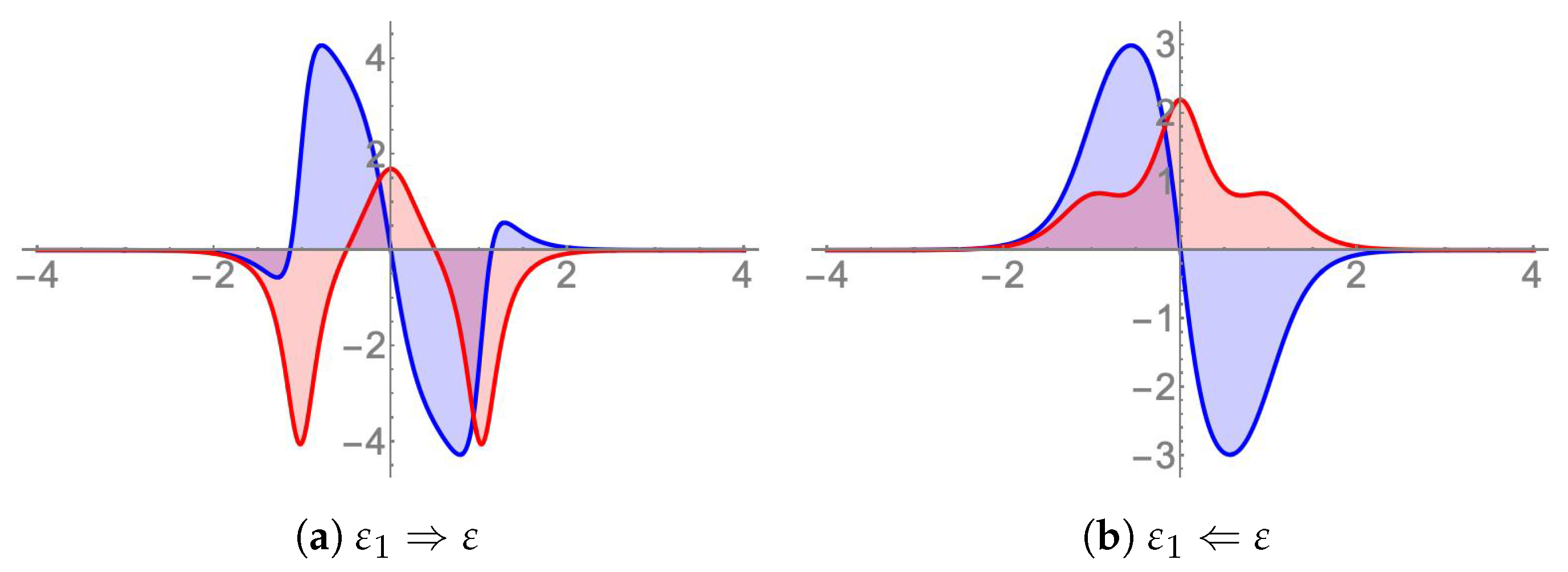
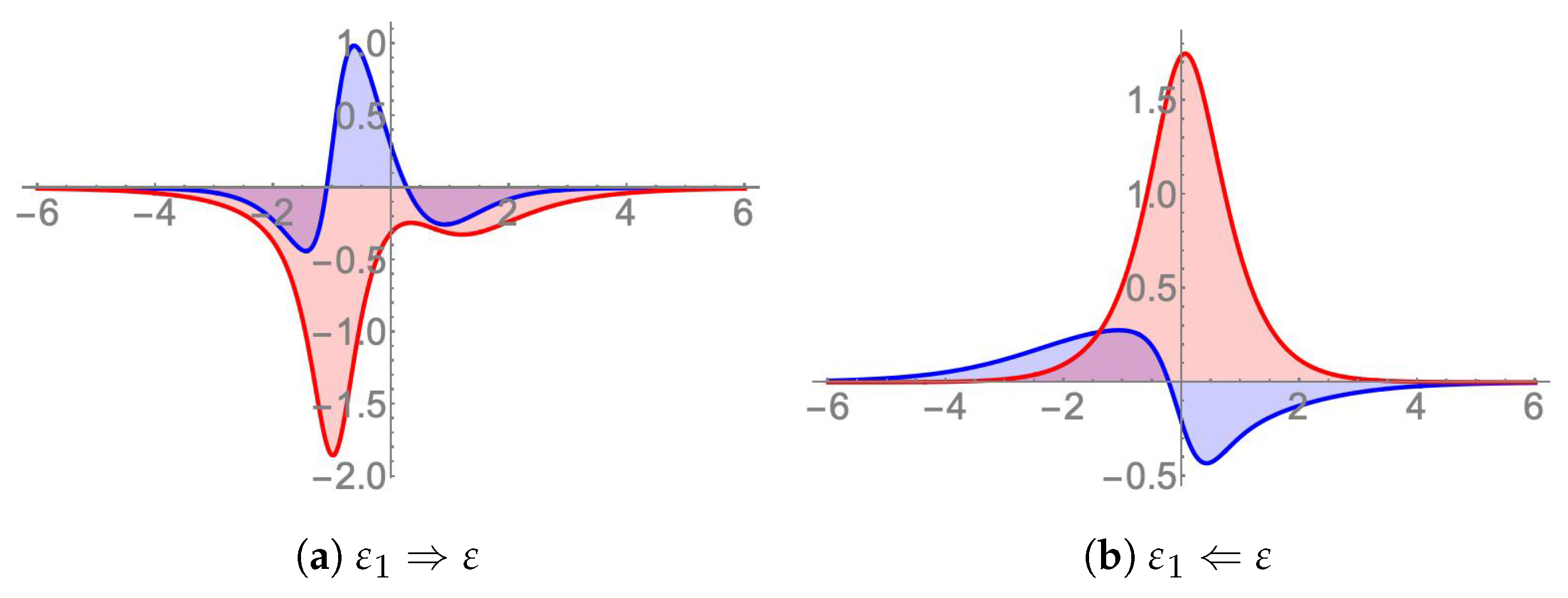
3.1. Adding Guided Modes One at a Time
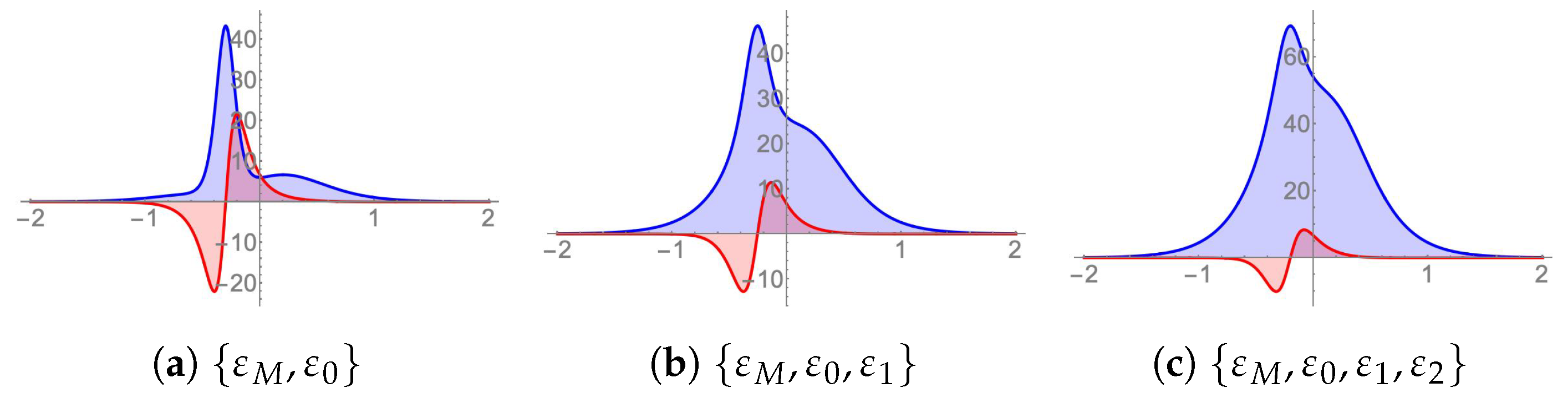
3.2. Manipulating a Set of Guided Modes at Once
4. Discussion of Results and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Supersymmetric Finite-Difference Algorithm
References
- Okoshi, T. Optical Fibers; Academic Press: New York, NY, USA, 1982. [Google Scholar]
- Snyder, A.W.; Love, J.D. Optical Waveguide Theory; Chapman and Hall: New York, NY, USA, 1983. [Google Scholar]
- Okamoto, K. Fundamentals of Optical Waveguides, 2nd ed.; Academic Press: New York, NY, USA, 2006. [Google Scholar]
- Calvo, M.L.; Lakshminarayanan, V. Optical Waveguides. From Theory to Applied Technologies; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Mielnik, B.; Rosas-Ortiz, O. Factorization: Little or great algorithm? J. Phys. A Math. Gen. 2004, 37, 10007. [Google Scholar] [CrossRef]
- Rogers, C.; Schief, W.K. Bäcklund and Darboux Transformations. Geometry and Modern Applications in Soliton Theory; Cambridge Texts in Applied Mathematics; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Witten, E. Dynamical breaking of supersymmetry. Nucl. Phys. B 1981, 188, 513–554. [Google Scholar] [CrossRef]
- Mielnik, B. Factorization method and new potentials with the oscillator spectrum. J. Math. Phys. 1984, 25, 3387–3389. [Google Scholar] [CrossRef]
- Andrianov, A.A.; Borisov, N.V.; Ioffe, M.V. Quantum systems with identical energy spectra. JETP Lett. 1984, 39, 93–97. [Google Scholar]
- Andrianov, A.A.; Borisov, N.V.; Ioffe, M.V. The factorization method and quantum systems with equivalent energy spectra. Phys. Lett. A 1984, 105, 19–22. [Google Scholar] [CrossRef]
- Andrianov, A.A.; Borisov, N.V.; Ioffe, M.V. Factorization method and Darboux transformation for multidimensional Hamiltonians. Theor. Math. Phys. 1984, 61, 1078–1088. [Google Scholar] [CrossRef]
- Nieto, M.M. Relationship between supersymmetry and the inverse method in quantum mechanics. Phys. Lett. B 1984, 145, 208–210. [Google Scholar] [CrossRef]
- Bagchi, B.K. Supersymmetry in Quantum and Classical Mechanics; Chapman and Hall: London, UK; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Cooper, F.; Khare, A.; Sukhatme, U. Supersymmetry in Quantum Mechanics; World Scientific: Singapore, 2001. [Google Scholar]
- Chumakov, S.M.; Wolf, K.B. Supersymmetry Helmholtz optics. Phys. Lett. A 1994, 193, 51–53. [Google Scholar] [CrossRef]
- Razo, R.; Cruz y Cruz, S. New confining optical media generated by Darboux transformations. J. Phys. Conf. Ser. 2019, 1194, 012091. [Google Scholar] [CrossRef]
- Mills, D.W.; Tamil, L.S. Analysis of planar optical waveguides using scattering data. J. Opt. Soc. Am. A 1992, 9, 1769–1778. [Google Scholar] [CrossRef]
- Díaz, J.I.; Negro, J.; Nieto, L.M.; Rosas-Ortiz, O. The supersymmetric modified Pöschl-Teller and delta well potentials. J. Phys. A Math. Gen. 1999, 32, 8447–8460. [Google Scholar] [CrossRef]
- Mielnik, B.; Nieto, L.M.; Rosas-Ortiz, O. The finite difference algorithm for higher order supersymmetry. Phys. Lett. A 2000, 269, 70–78. [Google Scholar] [CrossRef][Green Version]
- May, A.R.; Zervas, M.N. Inverse Scattering Designs of Active Multimode Waveguides with Tailored Modal Gain. IEEE J. Sel. Top. Quant. Electron. 2016, 22, 4401807. [Google Scholar] [CrossRef]
- Bagchi, B. Supersymmetry, reflectionless symmetric potentials and the inverse method. Int. J. Mod. Phys. 1990, 5, 1763–1772. [Google Scholar] [CrossRef]
- Miri, M.A.; Heinrich, M.; Christodoulides, D.N. Supersymmetric Optical Structures. Phys. Rev. Lett. 2013, 110, 233902. [Google Scholar] [CrossRef]
- Miri, M.A.; Heinrich, M.; Christodoulides, D.N. SUSY-inspired one-dimensional transformation optics. Optica 2014, 1, 89–95. [Google Scholar] [CrossRef]
- Heinrich, M.; Miri, M.A.; Stützer, S.; El-Ganainy, R.; Nolte, S.; Szameit, A.; Christodoulides, D.N. Supersymmetric mode converters. Nat. Commun. 2014, 5, 3698. [Google Scholar] [CrossRef] [PubMed]
- Macho, A.; Llorente, R.; García-Meca, C. Supersymmetric Transformations in Optical Fibers. Phys. Rev. Appl. 2018, 9, 014024. [Google Scholar] [CrossRef]
- Bagchi, B.; Ganguly, A.; Bhaumik, D.; Mitra, A. Higher derivative supersymmetry, a modified Crum-Darboux transformation and coherent state. Mod. Phys. Lett. A 1999, 14, 27–34. [Google Scholar] [CrossRef]
- Baye, D.; Lev́ai, G.; Sparenberg, J.M. Phase-equivalent complex potentials. Nucl. Phys. A 1996, 599, 435–456. [Google Scholar] [CrossRef]
- Cannata, F.; Junker, G.; Trost, J. Schrödinger operators with complex potential but real spectrum. Phys. Lett. A 1998, 246, 219–226. [Google Scholar] [CrossRef]
- Andrianov, A.A.; Ioffe, M.V.; Cannata, F.; Dedonder, J.P. Susy quantum mechanics with complex superpotentials and real energy spectra. Int. J. Mod. Phys. A 1999, 14, 2675–2688. [Google Scholar] [CrossRef]
- Bagchi, B.; Mallik, S.; Quesne, C. Generating complex potentials with real eigenvalues in supersymmetric quantum mechanics. Int. J. Mod. Phys. A 2001, 16, 2859–2872. [Google Scholar] [CrossRef]
- Fernández-García, N.; Rosas-Ortiz, O. Gamow-Siegert functions and Darboux-deformed short range potentials. Ann. Phys. 2008, 323, 1397–1414. [Google Scholar] [CrossRef][Green Version]
- Bagchi, B.; Mallik, S.; Quesne, C. Complexified Psusy and Ssusy interpretations of some PT-symmetric Hamiltonians possessing two series of real energy eigenvalues. Int. J. Mod. Phys. A 2002, 17, 51–72. [Google Scholar] [CrossRef]
- El-Ganainy, R.; Makris, K.G.; Christodoulides, D.N.; Musslimani, Z.H. Theory of coupled optical PT-symmetric structures. Opt. Lett. 2007, 32, 2632–2634. [Google Scholar] [CrossRef]
- Rüter, C.E.; Makris, K.G.; El-Ganainy, R.; Christodoulides, D.N.; Segev, M.; Kip, D. Observation of parity-time symmetry in optics. Nat. Phys. 2010, 6, 192–195. [Google Scholar] [CrossRef]
- Bender, C.M.; Boettcher, S. Real spectra in non-Hermitian Hamiltonians having PT-symmetry. Phys. Rev. Lett. 1998, 80, 5243. [Google Scholar] [CrossRef]
- Lévai, G.; Znojil, M. Systematic search for PT-symmetric potentials with real energy spectra. J. Phys. A Math. Gen. 2000, 33, 7165. [Google Scholar] [CrossRef]
- Mostafazadeh, A. Pseudo-Hermiticity versus PT-Symmetry: The necessary condition for the reality of the spectrum of a non-Hermitian Hamiltonian. J. Math. Phys. 2002, 43, 205. [Google Scholar] [CrossRef]
- Mostafazadeh, A. Pseudo-Hermitian representation of Quantum Mechanics. Int. J. Geom. Methods Mod. Phys. 2010, 7, 1191–1306. [Google Scholar] [CrossRef]
- Rosas-Ortiz, O.; Castaños, O.; Schuch, D. New supersymmetry-generated complex potentials with real spectra. J. Phys. A Math. Theor. 2015, 48, 445302. [Google Scholar] [CrossRef]
- Rosas-Ortiz, O.; Zelaya, K. Bi-Orthogonal Approach to Non-Hermitian Hamiltonians with the Oscillator Spectrum: Generalized Coherent States for Nonlinear Algebras. Ann. Phys. 2018, 388, 26–53. [Google Scholar] [CrossRef]
- Blanco-Garcia, Z.; Rosas-Ortiz, O.; Zelaya, K. Interplay between Riccati, Ermakov and Schrödinger equations to produce complex-valued potentials with real energy spectrum. Math. Meth. Appl. Sci. 2019, 42, 4925. [Google Scholar] [CrossRef]
- Zelaya, K.; Dey, S.; Hussin, V.; Rosas-Ortiz, O. Nonclassical States for Non-Hermitian Hamiltonians with the Oscillator Spectrum. Quantum Rep. 2020, 2, 12–38. [Google Scholar] [CrossRef]
- Zelaya, K.; Cruz y Cruz, S.; Rosas-Ortiz, O. On the construction of non-Hermitian Hamiltonians with all-real spectra through supersymmetric algorithms. In Geometric Methods in Physics XXXVIII; Trends in Mathematics; Birkhäuser: Cham, Switzerland, 2020; pp. 283–292. [Google Scholar]
- Bagchi, B.; Yang, J. New families of non-parity-time-symmetric complex potentials with all-real spectra. J. Math. Phys. 2020, 61, 063506. [Google Scholar] [CrossRef]
- Gbur, G.; Makris, K. Introduction to non-Hermitian photonics in complex media: PT-symmetry and beyond. Photonics Res. 2018, 6, PTS1–PTS3. [Google Scholar] [CrossRef]
- Gloge, D.; Marcuse, D. Formal quantum theory of light rays. J. Opt. Soc. Am. 1969, 59, 1629–1631. [Google Scholar] [CrossRef]
- Cruz y Cruz, S.; Rosas-Ortiz, O. Leaky Modes of Waveguides as a Classical Optics Analogy of Quantum Resonances. Adv. Math. Phys. 2015, 2015, 281472. [Google Scholar] [CrossRef]
- Ermakov, V. Second order differential equations. Conditions of complete integrability. Kiev Univ. Izvestia 1880, 9, 1–25. (In Russian). English translation by Harin A.O. in Appl. Anal. Discret. Math. 2008, 2, 123 [Google Scholar] [CrossRef]
- Jaimes-Najera, A.; Rosas-Ortiz, O. Interlace properties for the real and imaginary parts of the wave functions of complex-valued potentials with real spectrum. Ann. Phys. 2017, 376, 126–144. [Google Scholar] [CrossRef]
- Flügge, S. Practical Quantum Mechanics; Springer: New York, NY, USA, 1974. [Google Scholar]
- Cruz y Cruz, S.; Kuru, S.; Negro, J. Classical motion and coherent states for Pöschl-Teller potential. Phys. Lett. A 2008, 372, 1391. [Google Scholar] [CrossRef]
- Cevik, D.; Gadella, M.; Kuru, S.; Negro, J. Resonances and antibound states for the Pöschl-Teller potential: Ladder operators and SUSY partners. Phys. Lett. A 2016, 380, 1600. [Google Scholar] [CrossRef]
- Civitarese, O.; Gadella, M. Coherent Gamow states for the hyperbolic Pöschl-Teller potential. Ann. Phys. 2019, 406, 222. [Google Scholar] [CrossRef]
- Zelaya, K.; Rosas-Ortiz, O. Exact Solutions for Time-Dependent non-Hermitian Oscillators: Classical and Quantum Pictures. Quantum Rep. 2021, 3, 458–472. [Google Scholar] [CrossRef]
- Contreras-Astorga, A.; Jakubský, V. Photonic systems with two-dimensional landscapes of complex refractive index via time-dependent supersymmetry. Phys. Rev. A 2019, 99, 053812. [Google Scholar] [CrossRef]
- Contreras-Astorga, A.; Jakubský, V. Multimode Two-Dimensional PT-Symmetric Waveguides. J. Phys. Conf. Ser. 2020, 1540, 012018. [Google Scholar] [CrossRef]
- Cruz y Cruz, S.; Razo, R. Wave propagation in the presence of a dielectric slab: The paraxial approximation. J. Phys. Conf. Ser. 2015, 624, 012018. [Google Scholar] [CrossRef]
- Rosas-Ortiz, O.; Cruz y Cruz, S. Superpositions of bright and dark solitons supporting the creation of balanced gain-and-loss optical potentials. Math. Meth. Adv. Sci. 2020, 2020. [Google Scholar] [CrossRef]
- Ince, E.L. Ordinary Differential Equations; Dover: New York, NY, USA, 1956. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cruz y Cruz, S.; Romero-Osnaya, A.; Rosas-Ortiz, O. Balanced Gain-and-Loss Optical Waveguides: Exact Solutions for Guided Modes in Susy-QM. Symmetry 2021, 13, 1583. https://doi.org/10.3390/sym13091583
Cruz y Cruz S, Romero-Osnaya A, Rosas-Ortiz O. Balanced Gain-and-Loss Optical Waveguides: Exact Solutions for Guided Modes in Susy-QM. Symmetry. 2021; 13(9):1583. https://doi.org/10.3390/sym13091583
Chicago/Turabian StyleCruz y Cruz, Sara, Alejandro Romero-Osnaya, and Oscar Rosas-Ortiz. 2021. "Balanced Gain-and-Loss Optical Waveguides: Exact Solutions for Guided Modes in Susy-QM" Symmetry 13, no. 9: 1583. https://doi.org/10.3390/sym13091583
APA StyleCruz y Cruz, S., Romero-Osnaya, A., & Rosas-Ortiz, O. (2021). Balanced Gain-and-Loss Optical Waveguides: Exact Solutions for Guided Modes in Susy-QM. Symmetry, 13(9), 1583. https://doi.org/10.3390/sym13091583





