Effect of a Ring onto Values of Eigenvalue–Based Molecular Descriptors
Abstract
:1. Introduction
2. Preparations
3. Results and Discussion
4. Conclusions
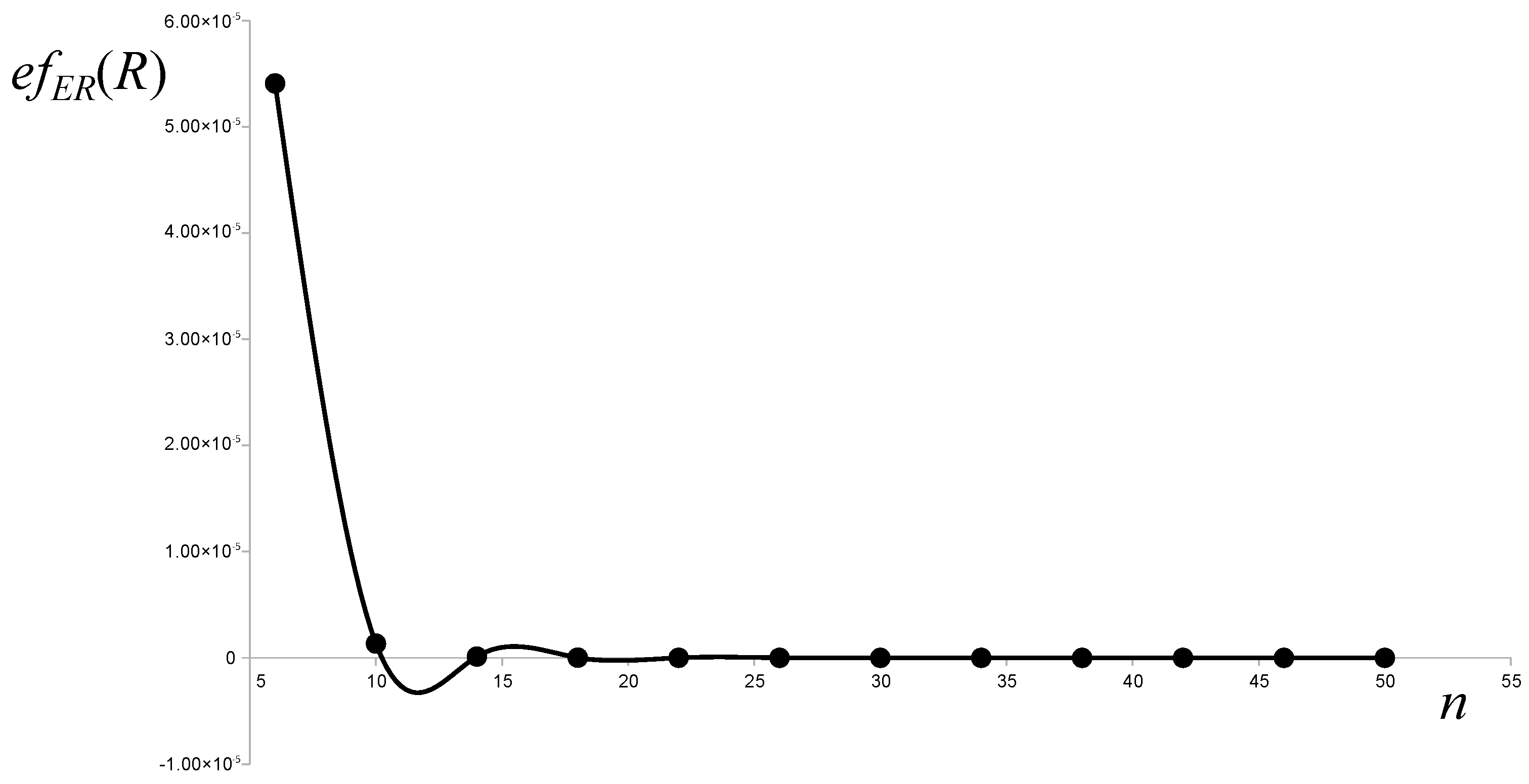
- Enlarging the size of a ring decreases the values of the and . Rings with a size exceeding 12 non-hydrogen atoms do not have . The energy effect varies with the ring size in accordance with the extending Hückel rule.
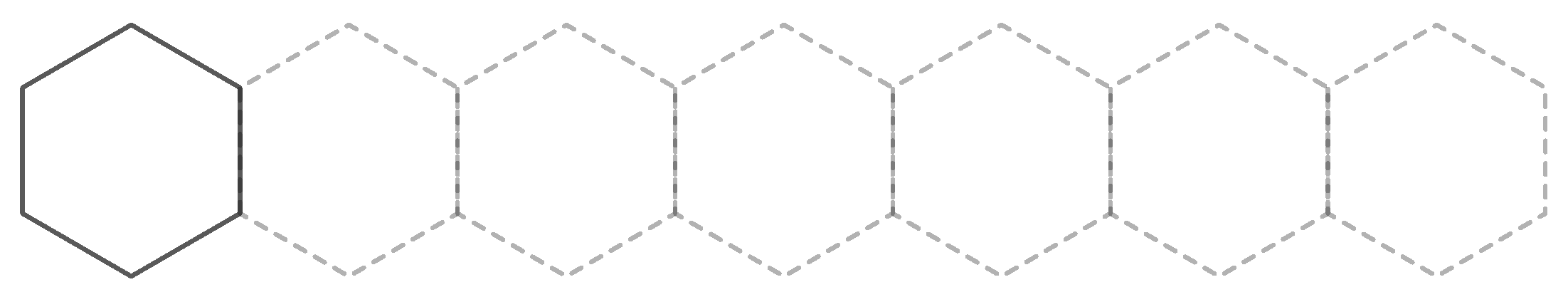
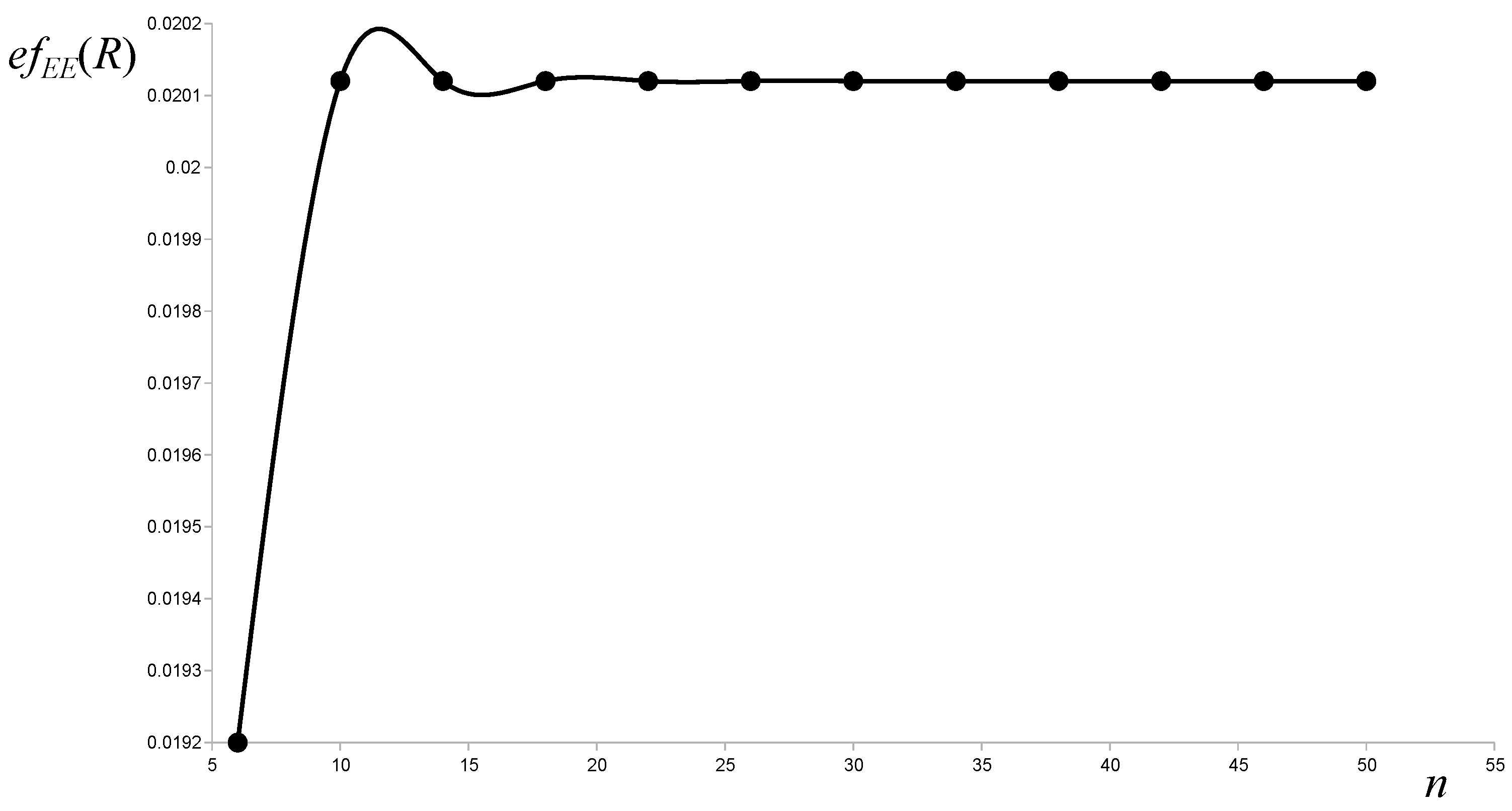
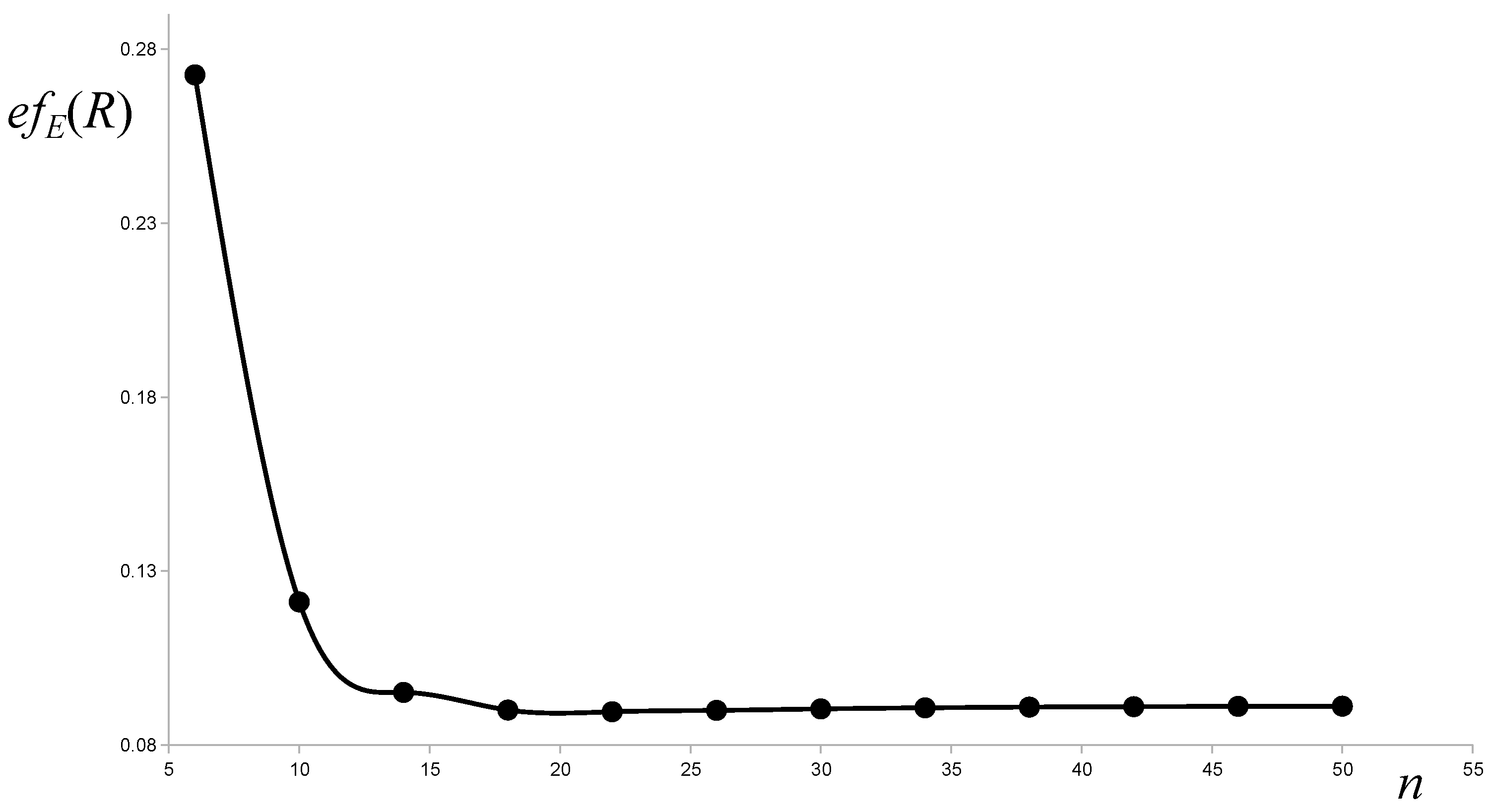
- The size of acene does not affect the . Interestingly, the effect of a ring on the value of the Estrada index is rising from benzene to the first member in acene series (naphthalene). The values of are exponentially decreasing with the size of an acene. The is decreasing for acenes up to 22 carbon atoms, and the negligible rise in its value is detected afterward.
- The third experiment shows that the benzene annelation does not influence the values of and . The results indicate a considerable impact of the benzene annelation on the values of the energy effect.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Todeschini, R.; Consonni, V. Molecular Descriptors for Chemoinformatics; Wiley–VCH: Wheinheim, Germany, 2009. [Google Scholar]
- Dearden, J.C. The use of topological indices in QSAR and QSPR modeling. In Advances in QSAR Modeling–Applications in Pharmaceutical, Chemical, Food, Agricultural and Environmental Sciences; Roy, K., Ed.; Springer: Cham, Switzerland, 2017; pp. 57–88. [Google Scholar] [CrossRef]
- Gálvez, J.; Gálvez-Llompart, M.; García-Domenech, R. Basic concepts and applications of molecular topology to drug design. In Advances in Mathematical Chemistry and Applications; Basak, S.C., Restrepo, G., Villaveces, J.L., Eds.; Bentham Science Pub.: Sharjah, United Arab Emirates, 2014; Volume 1, Chapter 8; pp. 161–195. [Google Scholar]
- Roy, K.; Kar, S.; Das, R.N. A Primer on QSAR/QSPR Modeling: Fundamental Concepts; Springer: Cham, Switzerland, 2015. [Google Scholar] [CrossRef]
- Estrada, E.; Uriarte, E. Recent advances on the role of topological indices in drug discovery research. Curr. Med. Chem. 2001, 8, 1573–1588. [Google Scholar] [CrossRef]
- Ma, Y.; Cao, S.; Shi, Y.; Gutman, I.; Dehmer, M.; Furtula, B. From the connectivity index to various Randić–type descriptors. MATCH Commun. Math. Comput. Chem. 2018, 80, 85–106. [Google Scholar]
- Xu, K.; Liu, M.; Das, K.C.; Gutman, I.; Furtula, B. A survey on graphs extremal with respect to distance–based topological indices. MATCH Commun. Math. Comput. Chem. 2014, 71, 461–508. [Google Scholar]
- Gutman, I.; Ramane, H.S. Research on graph energies in 2019. MATCH Commun. Math. Comput. Chem. 2020, 84, 277–292. [Google Scholar]
- Gutman, I. The energy of graph. Ber. Math. Stat. Sekt. Forschungsz. Graz 1978, 103, 1–22. [Google Scholar]
- Gutman, I.; Furtula, B. Energies of Graphs: Survey, Census, Bibliography; Center Res. SANU & Univ. Kragujevac: Kragujevac, Serbia, 2019. [Google Scholar]
- Li, X.; Shi, Y.; Gutman, I. Graph Energy; Springer: New York, NY, USA, 2012. [Google Scholar] [CrossRef]
- Gutman, I.; Li, X. Energies of Graphs: Theory and Applications; Univ. Kragujevac: Kragujevac, Serbia, 2016. [Google Scholar]
- Redžepović, I.; Furtula, B.; Gutman, I. Relating total π-electron energy of benzenoid hydrocarbons with HOMO and LOMO energies. MATCH Commun. Math. Comput. Chem. 2020, 84, 229–237. [Google Scholar]
- Oboudi, M.R. Bounds for energy of matrices and energy of graphs. MATCH Commun. Math. Comput. Chem. 2020, 84, 377–384. [Google Scholar]
- Hayat, S.; Khan, S. Quality testing of spectrum-based valency descriptors for polycyclic aromatic hydrocarbons with applications. J. Mol. Struct. 2021, 1228, 129789. [Google Scholar] [CrossRef]
- Estrada, E. Characterization of 3D molecular structure. Chem. Phys. Lett. 2000, 319, 713–718. [Google Scholar] [CrossRef]
- Redžepović, I.; Furtula, B. Resolvent energy and Estrada index of benzenoid hydrocarbons. J. Serb. Soc. Comput. Mech. 2020, 2020, 37–44. [Google Scholar] [CrossRef]
- Jahanbani, A.; Karimi, A.T.; Rodriguez, J. Results on the Estrada indices of benzenoid hydrocarbons. Polycyc. Arom. Comp. 2020, 1–18. [Google Scholar] [CrossRef]
- Gutman, I.; Deng, H.; Radenković, S. The Estrada index: An updated survey. In Selected Topics on Applications of Graph Spectra; Cvetković, D., Gutman, I., Eds.; Math. Inst. SASA: Belgrade, Serbia, 2011; pp. 155–174. [Google Scholar]
- Estrada, E.; Rodriguez-Velazquez, J.A. Subgraph centrality in complex networks. Phys. Rev. E 2005, 71, 056103. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shang, Y. Perturbation results for the Estrada index in weighted networks. J. Phys. A Math. Theor. 2011, 44, 075003. [Google Scholar] [CrossRef]
- Gutman, I.; Furtula, B.; Zogić, E.; Glogić, E. Resolvent energy of graphs. MATCH Commun. Math. Comput. Chem. 2016, 75, 279–290. [Google Scholar]
- Redžepović, I.; Furtula, B. On relationships of eigenvalue–based topological molecular descriptors. Acta Chim. Slov. 2020, 67, 312–318. [Google Scholar] [CrossRef] [PubMed]
- Redžepović, I.; Furtula, B. Predictive potential of eigenvalue–based topological molecular descriptors. J. Comput. Aided Mol. Des. 2020, 34, 975–982. [Google Scholar] [CrossRef] [PubMed]
- Redžepović, I.; Furtula, B. Comparative study on structural sensitivity of eigenvalue–based molecular descriptors. J. Math. Chem. 2021, 59, 476–487. [Google Scholar] [CrossRef]
- Das, K.C. Conjectures on resolvent energy of graphs. MATCH Commun. Math. Comput. Chem. 2019, 81, 453–464. [Google Scholar]
- Ghebleh, M.; Kanso, A.; Stevanović, D. On trees with smallest resolvent energy. MATCH Commun. Math. Comput. Chem. 2017, 77, 635–654. [Google Scholar]
- Gök, G.K.; Ashrafi, A.R. Some bounds for the resolvent energy. Appl. Math. Comput. 2021, 397, 125958. [Google Scholar] [CrossRef]
- Zhu, Z. Some extremal properties of the resolvent energy, Estrada and resolvent Estrada indices of graphs. J. Math. Anal. Appl. 2017, 447, 957–970. [Google Scholar] [CrossRef]
- Redžepović, I.; Furtula, B. On degeneracy of A-eigenvalue–based molecular descriptors and r-equienergetic. MATCH Commun. Math. Comput. Chem. 2020, 84, 385–397. [Google Scholar]
- Bosanac, S.; Gutman, I. Effect of a ring on the stability of polycyclic conjugated molecules. Z. Naturforsch. 1977, 32a, 10–12. [Google Scholar] [CrossRef]
- Gutman, I.; Bosanac, S. Quantitative approach to Hückel rule the relations between the cycles of a molecular graph and the thermodynamic stability of a conjugated molecule. Tetrahedron 1977, 33, 1809–1812. [Google Scholar] [CrossRef]
- Gutman, I. Cyclic conjugation energy effects in polycyclic π-electron systems. Monats. Chem. 2005, 136, 1055–1069. [Google Scholar] [CrossRef]
- Gutman, I. Mathematical modeling of chemical phenomena. In Advances in Nonlinear Sciences II–Sciences and Applications; Stevanović Hedrih, K.R., Ed.; Academy of Nonlinear Sciences: Belgrade, Serbia, 2008; pp. 110–136. [Google Scholar]
- Gutman, I.; Polansky, O.E. Mathematical Concepts in Organic Chemistry; Springer: Berlin, Germany, 1986. [Google Scholar] [CrossRef]
- Gutman, I. Cyclic conjugation in dianions: Effect of cycles on the thermodynamic stability of polycyclic conjugated dianions. J. Mol. Struct. (Theochem) 1998, 428, 241–246. [Google Scholar] [CrossRef]
- Fatoorehchi, H.; Gutman, I.; Abolghasemi, H. A combined technique for computation of energy-effect of cycles in conjugated molecules. J. Math. Chem. 2015, 53, 1113–1125. [Google Scholar] [CrossRef]
- Friedman, B. Complex Roots of Polynomial Equations; HardPress Publishing: Houston, TX, USA, 2013. [Google Scholar]
- Harris, C.R.; Millman, K.J.; van der Walt, S.J.; Gommers, R.; Virtanen, P.; Cournapeau, D.; Wieser, E.; Taylor, J.; Berg, S.; Smith, N.J.; et al. Array programming with NumPy. Nature 2020, 585, 357–362. [Google Scholar] [CrossRef]
- Hagberg, A.A.; Schult, D.A.; Swart, P.J. Exploring Network Structure, Dynamics, and Function using NetworkX. In Proceedings of the 7th Python in Science Conference, Pasadena, CA, USA, 21 August 2008; Varoquaux, G., Vaught, T., Millman, J., Eds.; Los Alamos National Lab. (LANL): Los Alamos, NM, USA, 2008; pp. 11–15. Available online: http://conference.scipy.org/proceedings/SciPy2008/paper_2/full_text.pdf (accessed on 16 June 2021).
- Mpmath: A Python Library for Arbitrary-Precision Floating-Point Arithmetic (Version 0.18). 2013. Available online: http://mpmath.org/ (accessed on 16 June 2021).
- Clar, E. The Aromatic Sextet; Wiley: London, UK, 1972. [Google Scholar]






| n | |||
|---|---|---|---|
| 3 | 3.83 | 1.51049 | 0.19701 |
| 4 | 0.44700 | −0.41860 | |
| 5 | 0.10483 | 0.12766 | |
| 6 | 0.02012 | 0.26441 | |
| 7 | 0.00326 | 0.09584 | |
| 8 | 0.00046 | −0.17942 | |
| 9 | 0.07505 | ||
| 10 | 0.19042 | ||
| 11 | 0.05920 | ||
| 12 | −0.11716 | ||
| 13 | 0.0 | 0.04593 | |
| 14 | 0.0 | 0.16718 | |
| 15 | 0.0 | 0.03404 | |
| 16 | 0.0 | −0.09000 | |
| 17 | 0.0 | 0.02277 | |
| 18 | 0.0 | 0.16534 | |
| 19 | 0.0 | −0.10270 | |
| 20 | 0.0 | −0.23598 |
| n | |||
|---|---|---|---|
| 6 | 5.41 | 0.01920 | 0.27259 |
| 10 | 0.02012 | 0.12111 | |
| 14 | 0.02012 | 0.09511 | |
| 18 | 0.02012 | 0.09004 | |
| 22 | 0.02012 | 0.08955 | |
| 26 | 0.02012 | 0.08995 | |
| 30 | 0.02012 | 0.09037 | |
| 34 | 0.02012 | 0.09068 | |
| 38 | 0.02012 | 0.09089 | |
| 42 | 0.02012 | 0.09101 | |
| 46 | 0.02012 | 0.09110 | |
| 50 | 0.02012 | 0.09115 |
| Annelation | |||
|---|---|---|---|
| a | 1.199 | 0.020 | 0.107 |
| b | 1.199 | 0.020 | 0.103 |
| c | 1.201 | 0.021 | 0.043 |
| d | 1.201 | 0.021 | 0.080 |
| e | 1.201 | 0.021 | 0.091 |
| f | 1.201 | 0.022 | 0.067 |
| g | 1.201 | 0.022 | 0.034 |
| h | 1.201 | 0.022 | 0.044 |
| i | 1.201 | 0.022 | 0.043 |
| j | 1.201 | 0.022 | 0.028 |
| k | 1.201 | 0.022 | 0.032 |
| l | 1.201 | 0.022 | 0.032 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Redžepović, I.; Radenković, S.; Furtula, B. Effect of a Ring onto Values of Eigenvalue–Based Molecular Descriptors. Symmetry 2021, 13, 1515. https://doi.org/10.3390/sym13081515
Redžepović I, Radenković S, Furtula B. Effect of a Ring onto Values of Eigenvalue–Based Molecular Descriptors. Symmetry. 2021; 13(8):1515. https://doi.org/10.3390/sym13081515
Chicago/Turabian StyleRedžepović, Izudin, Slavko Radenković, and Boris Furtula. 2021. "Effect of a Ring onto Values of Eigenvalue–Based Molecular Descriptors" Symmetry 13, no. 8: 1515. https://doi.org/10.3390/sym13081515
APA StyleRedžepović, I., Radenković, S., & Furtula, B. (2021). Effect of a Ring onto Values of Eigenvalue–Based Molecular Descriptors. Symmetry, 13(8), 1515. https://doi.org/10.3390/sym13081515







