Molecular Dynamics Simulation of Tolman Length and Interfacial Tension of Symmetric Binary Lennard–Jones Liquid
Abstract
1. Introduction
2. Molecular Dynamics Simulation
2.1. Setting
2.2. Analysis
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tolman, R.C. Consideration of the Gibbs theory of surface tension. J. Chem. Phys. 1948, 16, 758–773. [Google Scholar] [CrossRef]
- Tolman, R.C. The superficial density of matter at a liquid-vapor boundary. J. Chem. Phys. 1949, 17, 118–127. [Google Scholar] [CrossRef]
- Tolman, R.C. The effect of droplet size on surface tension. J. Chem. Phys. 1949, 17, 333–337. [Google Scholar] [CrossRef]
- Kirkwood, J.G.; Buff, F.P. The statistical mechanical theory of surface tension. J. Chem. Phys. 1949, 17, 338–343. [Google Scholar] [CrossRef]
- Buff, F.P.; Kirkwood, J.G. Remarks on the surface tension of small droplets. J. Chem. Phys. 1950, 18, 991–992. [Google Scholar] [CrossRef]
- Koenig, F.O. On the thermodynamic relation between surface tension and curvature. J. Chem. Phys. 1950, 18, 449–459. [Google Scholar] [CrossRef]
- Melrose, J.C. Thermodynamic aspects of capillarity. J. Ind. Eng. Chem. 1968, 60, 53–70. [Google Scholar] [CrossRef]
- Blokhuis, E.M.; Kuipers, J. Thermodynamic expressions for the Tolman length. J. Chem. Phys. 2006, 124, 074701. [Google Scholar] [CrossRef]
- Thompson, S.M.; Gubbins, K.E.; Walton, J.P.R.B.; Chantry, R.A.R.; Rowlinson, J.S. A molecular dynamics study of liquid drops. J. Chem. Phys. 1984, 81, 530–542. [Google Scholar] [CrossRef]
- Shreve, A.P.; Walton, J.P.R.B.; Gubbins, K.E. Liquid drops of polar molecules. J. Chem. Phys. 1986, 85, 2178–2186. [Google Scholar] [CrossRef]
- Kuz, V.A. Evaporation of small drops. J. Appl. Phys. 1991, 69, 7034–7036. [Google Scholar] [CrossRef]
- Blokhuis, E.M.; Bedeaux, D. Pressure tensor of a spherical interface. J. Chem. Phys. 1992, 97, 3576–3586. [Google Scholar] [CrossRef]
- Iwamatsu, M. The surface tension and Tolman’s length of a drop. J. Phys. Condense. Matter 1994, 6, L173–L177. [Google Scholar] [CrossRef]
- Koga, K.; Zeng, X.C.; Shchekin, A.K. Validity of Tolman’s equation: How large should a droplet be? J. Chem. Phys. 1998, 109, 4063–4070. [Google Scholar] [CrossRef]
- Gránásy, L. Semiempirical Van der Waals/Cahn-Hilliard theory: Size dependence of the Tolman length. J. Chem. Phys. 1998, 109, 9660–9663. [Google Scholar] [CrossRef]
- Moody, M.P.; Attard, P. Curvature-dependent surface tension of a growing droplet. Phys. Rev. Lett. 2003, 91, 056104. [Google Scholar] [CrossRef] [PubMed]
- Lu, H.M.; Jiang, Q. Size-dependent surface tension and Tolman’s length of droplets. Langmuir 2005, 21, 779–781. [Google Scholar] [CrossRef] [PubMed]
- Anisimov, M.A. Divergence of Tolman’s length for a droplet near the critical point. Phys. Rev. Lett. 2007, 98, 035702. [Google Scholar] [CrossRef] [PubMed]
- Ashbaugh, H.S. Blowing bubbles in Lennard–Jonesium along the saturation curve. J. Chem. Phys. 2009, 130, 204517. [Google Scholar] [CrossRef]
- Baranov, S.A.; Rekhviashvili, S.S.; Sokurovc, A.A. Some problems in simulation of the thermodynamic properties of droplets. Surf. Eng. Appl. Electrochem. 2019, 55, 286–293. [Google Scholar] [CrossRef]
- Schmelzer, J.W.P.; Abyzov, A.S.; Baidakov, V.G. Entropy and the Tolman parameter in nucleation theory. Entropy 2019, 21, 670. [Google Scholar] [CrossRef]
- Schmelzer, J.W.P.; Abyzov, A.S.; Ferreira, E.B.; Fokin, V.M. Curvature dependence of the surface tension and crystal nucleation in liquids. Int. J. Appl. Glass Sci. 2019, 10, 57–68. [Google Scholar] [CrossRef]
- Kashchiev, D. Nucleation work, surface tension, and Gibbs–Tolman length for nucleus of any size. J. Chem. Phys. 2020, 153, 124509. [Google Scholar] [CrossRef] [PubMed]
- Nijmeijer, M.J.P.; Bakker, A.F.; Bruin, C.; Sikkenk, J.H. A molecular dynamics simulation of the Lennard–Jones liquid-vapor interface. J. Chem. Phys. 1988, 89, 3789–3792. [Google Scholar] [CrossRef]
- Holcomb, C.D.; Clancy, P.; Zollweg, J.A. A critical study of the simulation of the liquid-vapour interface of a Lennard–Jones fluid. Am. J. Physiol. Lung Cell. Mol. Physiol. 1993, 78, 437–459. [Google Scholar] [CrossRef]
- Haye, M.J.; Bruin, C. Molecular dynamics study of the curvature correction to the surface tension. J. Chem. Phys. 1994, 100, 556–558. [Google Scholar] [CrossRef]
- Chen, L.-J. Area dependence of the surface tension of a Lennard–Jones fluid from molecular dynamics simulations. J. Chem. Phys. 1995, 103, 10214–10216. [Google Scholar] [CrossRef]
- Trokhymchuk, A.; Alejandre, J. Computer simulations of liquid/vapor interface in Lennard–Jones fluids: Some questions and answers. J. Chem. Phys. 1999, 111, 8510–8523. [Google Scholar] [CrossRef]
- Kanda, H.; Miyahara, M.; Higashitani, K. Triple point of Lennard–Jones fluid in slit nanopore: Solidification of critical condensate. J. Chem. Phys. 2004, 120, 6173–6179. [Google Scholar] [CrossRef] [PubMed]
- Baidakov, V.G.; Khotienkova, M.N. Surface tension of methane–nitrogen solutions: 2. Description in the framework of the van der Waals gradient theory. Fluid Phase Equilib. 2016, 425, 402–410. [Google Scholar] [CrossRef]
- Brochard, F.; de Gennes, P.G. Phase transitions of binary mixtures in random media. J. Phys. Lett. 1983, 44, L785. [Google Scholar] [CrossRef]
- Nakanishi, H.; Fisher, M.E. Critical point shifts in films. J. Chem. Phys. 1983, 78, 3279–3293. [Google Scholar] [CrossRef]
- De-Gennes, P.G. Liquid-liquid demixing inside a rigid network. Qualitative features. J. Phys. Chem. 1984, 88, 6469–6472. [Google Scholar] [CrossRef]
- Ma, W.-J.; Maritan, A.; Banavar, J.R.; Koplik, J. Dynamics of phase separation of binary fluids. Phys. Rev. A 1992, 45, R5347–R5350. [Google Scholar] [CrossRef] [PubMed]
- Keblinski, P.; Ma, W.-J.; Maritan, A.; Koplik, J.; Banavar, J.R. Molecular dynamics of phase separation in narrow channels. Phys. Rev. E 1993, 47, R2265–R2268. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Chakrabarti, A. Phase separation of binary fluids confined in a cylindrical pore: A molecular dynamics study. Phys. Rev. E 1994, 50, R4290–R4294. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Chakrabarti, A. Phase separation of binary fluids in porous media: Asymmetries in pore geometry and fluid composition. Phys. Rev. E 1995, 52, 2736–2741. [Google Scholar] [CrossRef]
- Laradji, M.; Toxvaerd, S.; Mouritsen, O.G. Molecular dynamics simulation of spinodal decomposition in three-dimensional binary fluids. Phys. Rev. Lett. 1996, 77, 2253–2256. [Google Scholar] [CrossRef]
- Velasco, E.; Toxvaerd, S. Phase separation in two-dimensional binary fluids: A molecular dynamics study. Phys. Rev. E 1996, 54, R605–R610. [Google Scholar] [CrossRef]
- Sliwinska-Bartkowiak, M.; Sowers, S.L.; Gubbins, K.E. Liquid−liquid phase equilibria in porous materials. Langmuir 1997, 13, 1182–1188. [Google Scholar] [CrossRef]
- Gelb, L.D.; Gubbins, K.E.; Radhakrishnan, R.; Sliwinska-Bartkowiak, M. Phase separation in confined systems. Rep. Prog. Phys. Phys. Soc. 1999, 62, 1573–1659. [Google Scholar] [CrossRef]
- Das, S.K.; Puri, S.; Horbach, J.; Binder, K. Molecular dynamics study of phase separation kinetics in thin films. Phys. Rev. Lett. 2006, 96, 016107. [Google Scholar] [CrossRef]
- Bucior, K.; Yelash, L.; Binder, K. Phase separation of an asymmetric binary-fluid mixture confined in a nanoscopic slit pore: Molecular-dynamics simulations. Phys. Rev. E 2008, 77, 051602. [Google Scholar] [CrossRef]
- Patrick, W.A.; Jones, D.C. Studies in the adsorption from solution from the standpoint of capillarity. I. J. Phys. Chem. 1925, 29, 1–10. [Google Scholar] [CrossRef]
- Patrick, W.A.; Eberman, N.F. Studies in the adsorption from solution from the standpoint of capillarity. II. J. Phys. Chem. 1925, 29, 220–228. [Google Scholar] [CrossRef]
- Miyahara, M.; Kato, M.; Okazaki, M. Liquid-phase capillary condensation and adsorption isotherm. AIChE J. 1994, 40, 1549–1557. [Google Scholar] [CrossRef]
- Miyahara, M.; Suzuki, K.; Okazaki, M. Capillary phase separation in solvent dehydration by hygroscopic porous adsorbents. J. Chem. Eng. Jpn. 1997, 30, 683–690. [Google Scholar] [CrossRef][Green Version]
- Díaz-Herrera, E.; Moreno-Razo, J.A.; Ramírez-Santiago, G. Wetting phenomenon in the liquid-vapor phase coexistence of a partially miscible Lennard–Jones binary mixture. Phys. Rev. E 2004, 70, 051601. [Google Scholar] [CrossRef]
- Diaz-Herrera, E.; Ramirez-Santiago, G.; Moreno-Razo, J.A. Phase and interfacial behavior of partially miscible symmetric Lennard–Jones binary mixtures. J. Chem. Phys. 2005, 123, 184507. [Google Scholar] [CrossRef]
- Kanda, H.; Makino, H. Liquid-liquid phase separation of binary Lennard–Jones fluid in slit nanopores. Adsorption 2008, 14, 485–491. [Google Scholar] [CrossRef][Green Version]
- Stephan, S.; Hasse, H. Influence of dispersive long-range interactions on properties of vapour–liquid equilibria and interfaces of binary Lennard–Jones mixtures. Mol. Phys. 2020, 118, e1699185. [Google Scholar] [CrossRef]
- van der Meer, B.; Prymidis, V.; Dijkstra, M.; Filion, L. Predicting the phase behavior of mixtures of active spherical particles. J. Chem. Phys. 2020, 152, 144901. [Google Scholar] [CrossRef]
- Kofke, D.A. Direct evaluation of phase coexistence by molecular simulation via integration along the saturation line. J. Chem. Phys. 1993, 98, 4149–4162. [Google Scholar] [CrossRef]
- del Río, E.M.; de Miguel, E. Computer simulation study of the free surfaces of a liquid crystal model. Phys. Rev. E 1997, 55, 2916–2924. [Google Scholar] [CrossRef]
- Guggenheim, E.A. The principle of corresponding states. J. Chem. Phys. 1945, 13, 253. [Google Scholar] [CrossRef]
- Smit, B. Phase diagrams of Lennard–Jones fluids. J. Chem. Phys. 1992, 96, 8639–8640. [Google Scholar] [CrossRef]
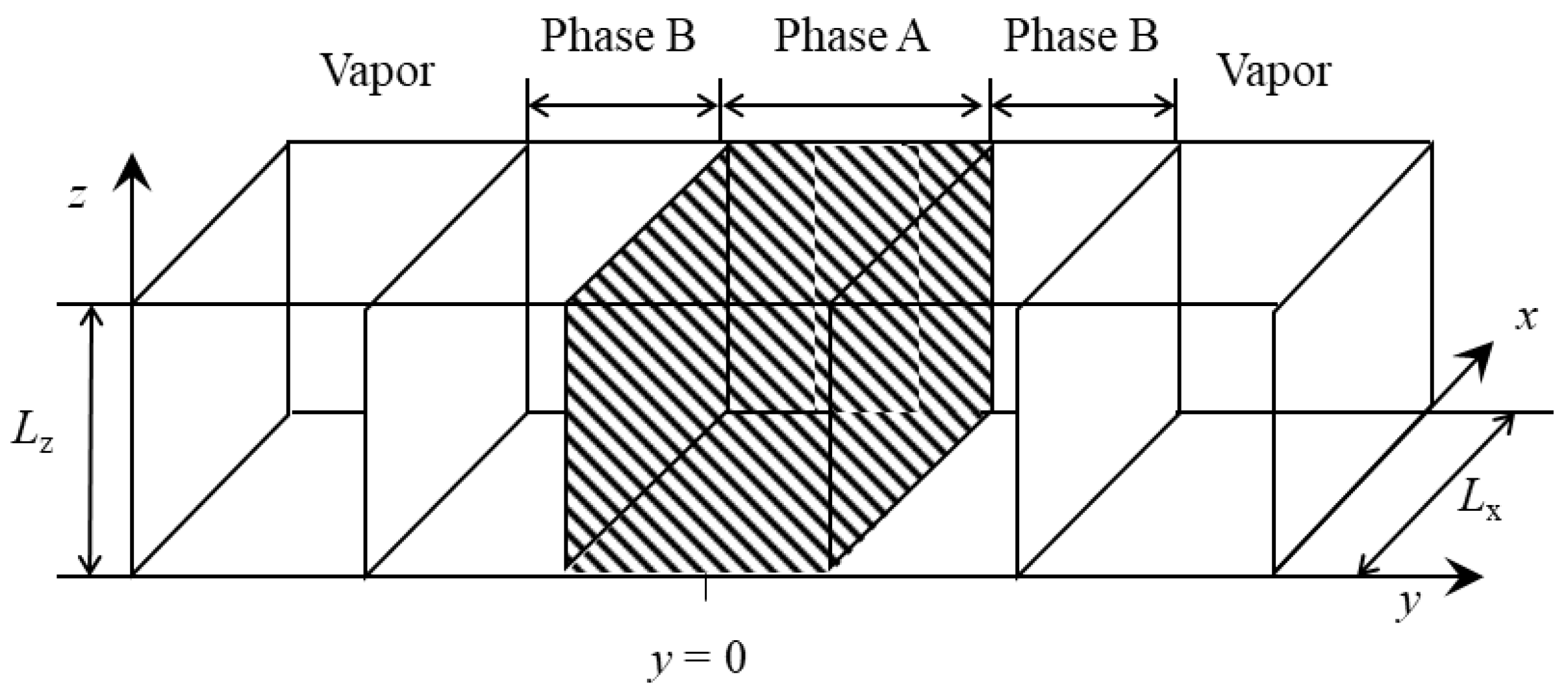


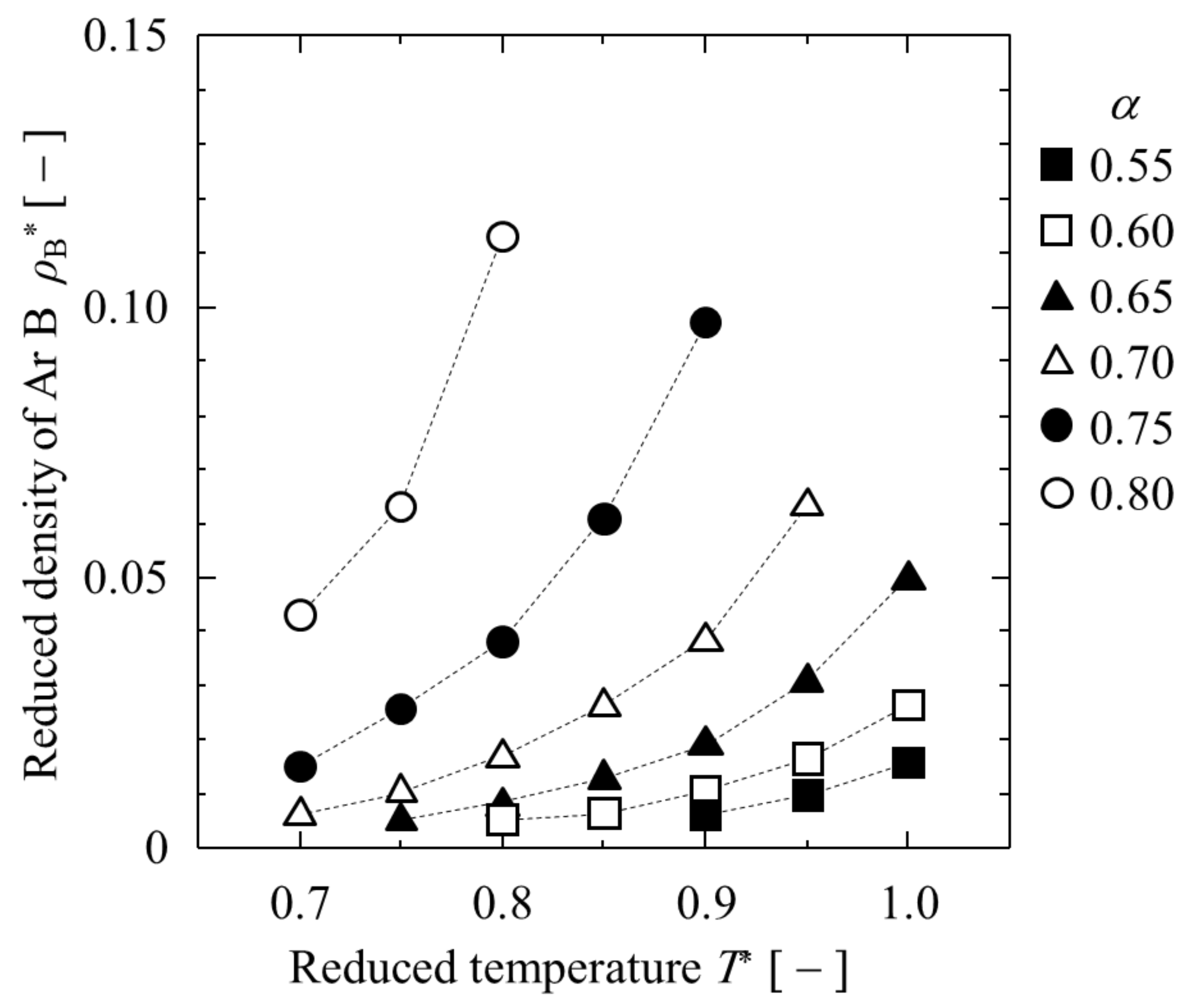
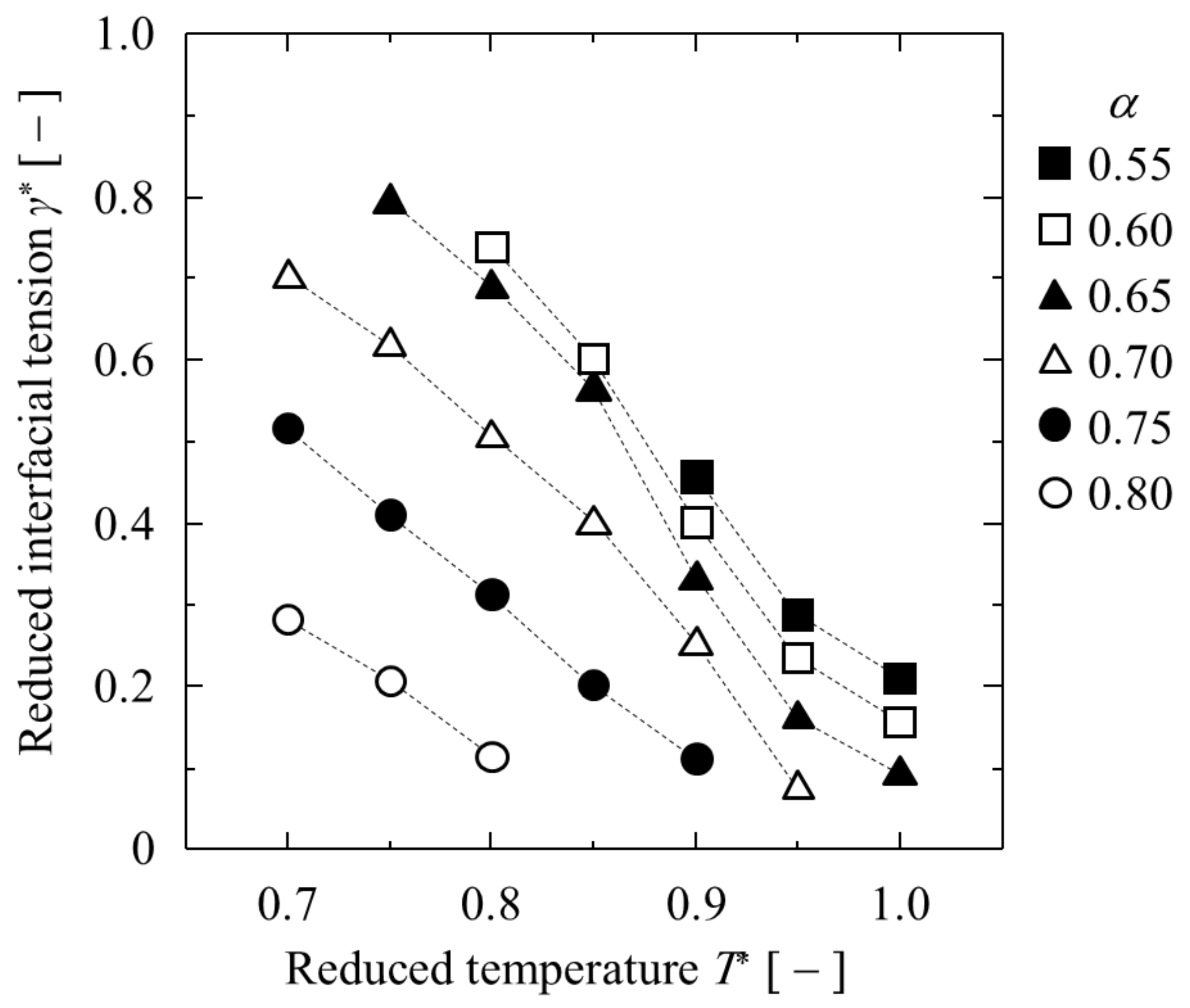
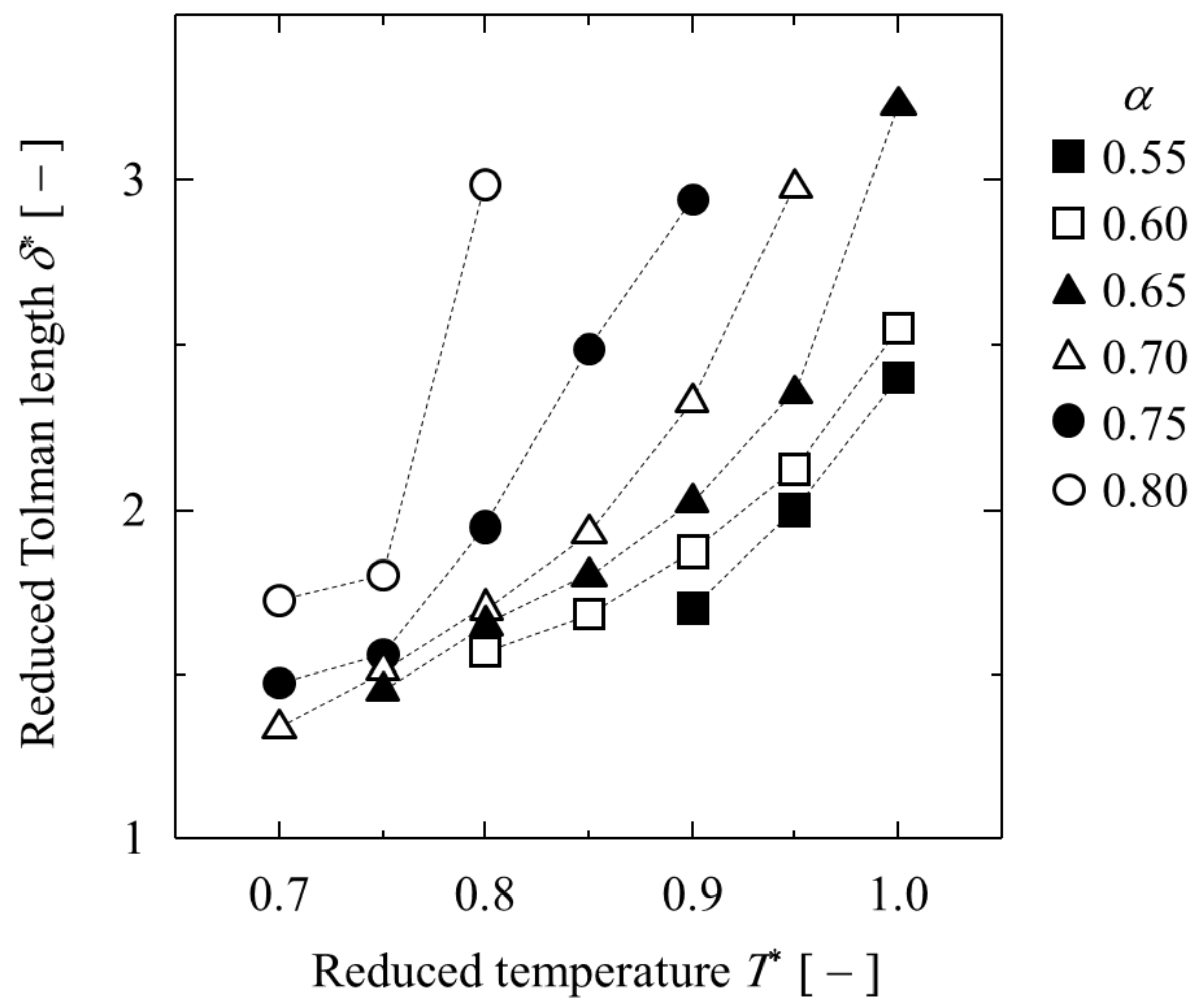
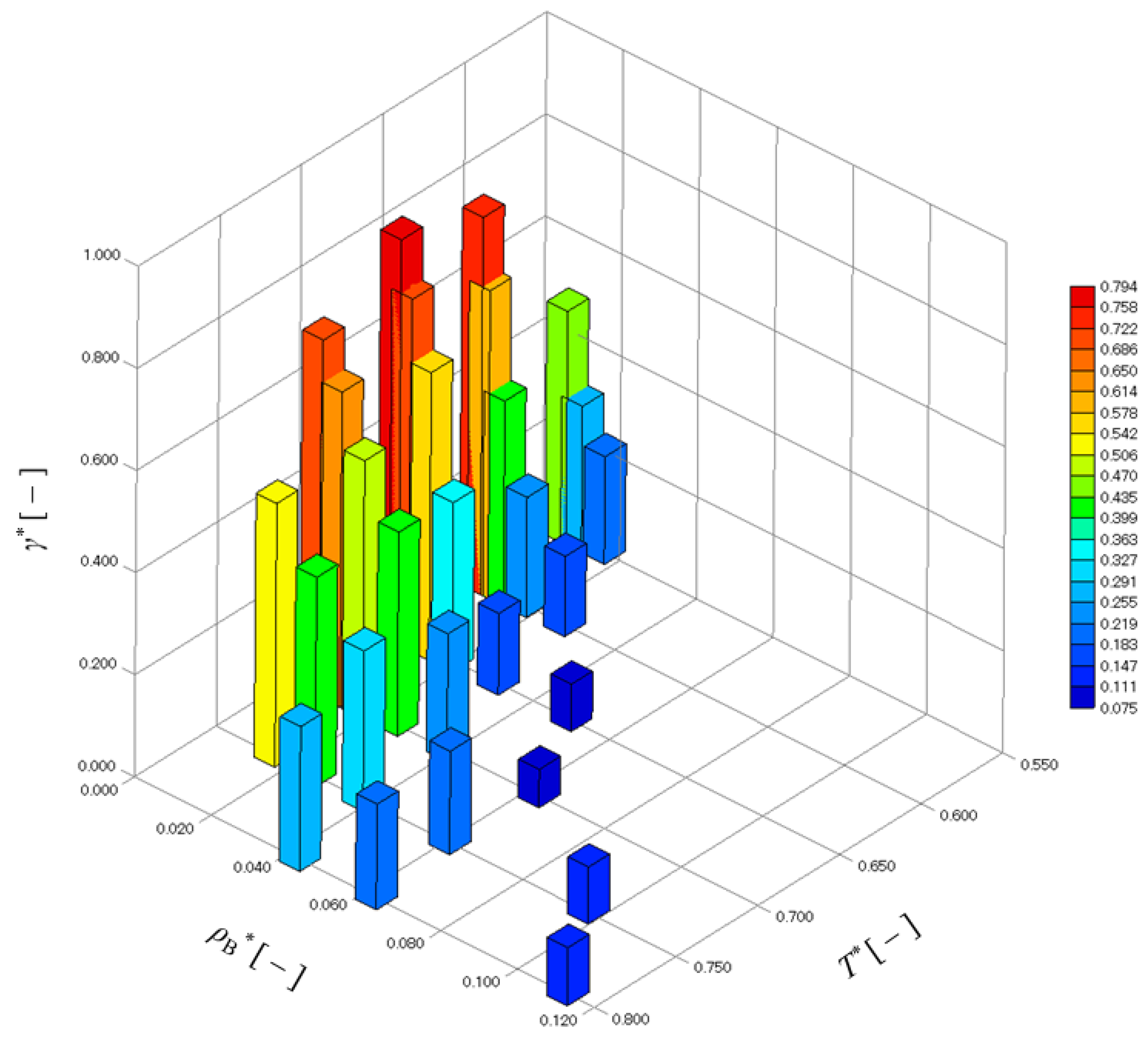
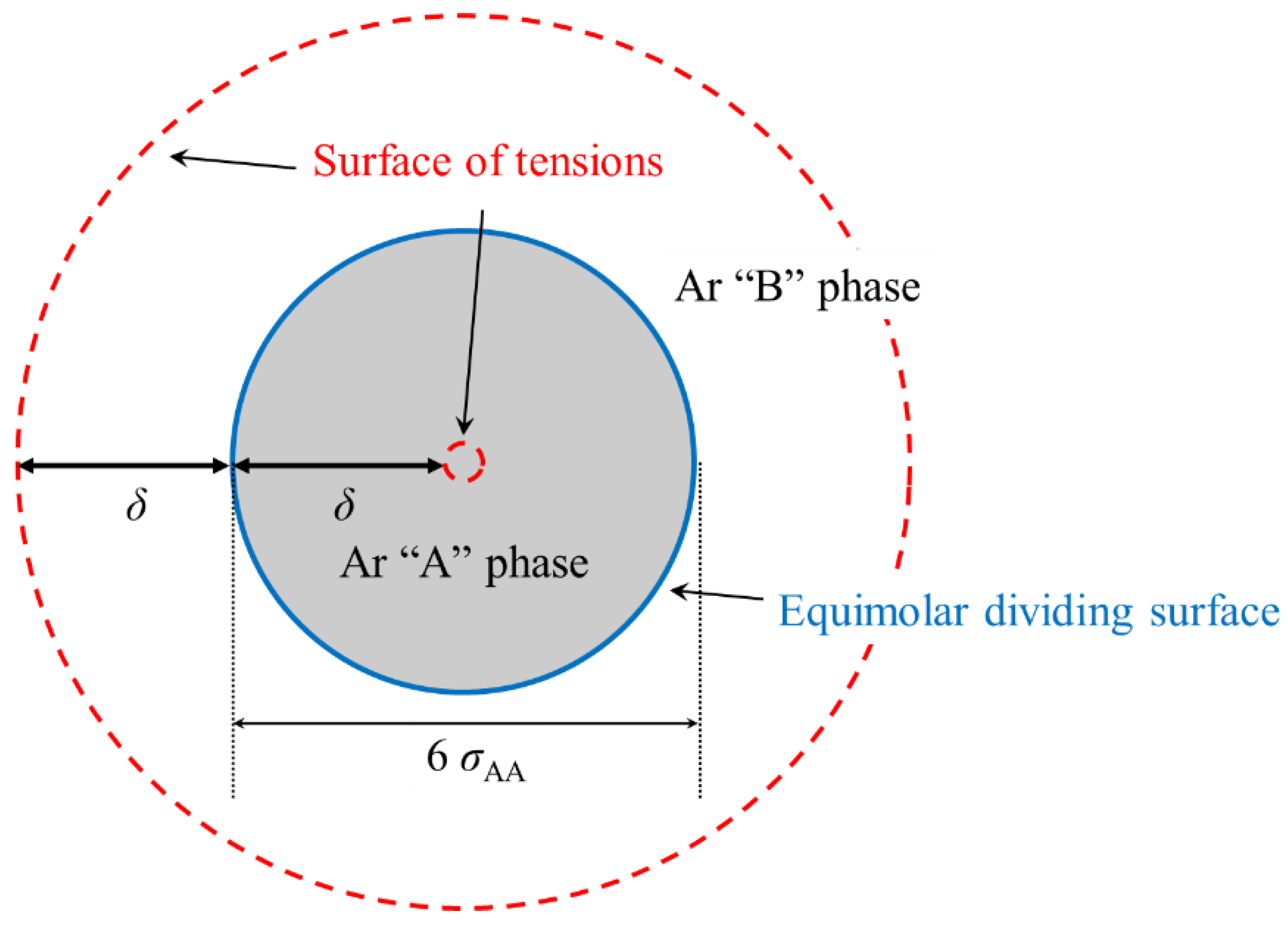
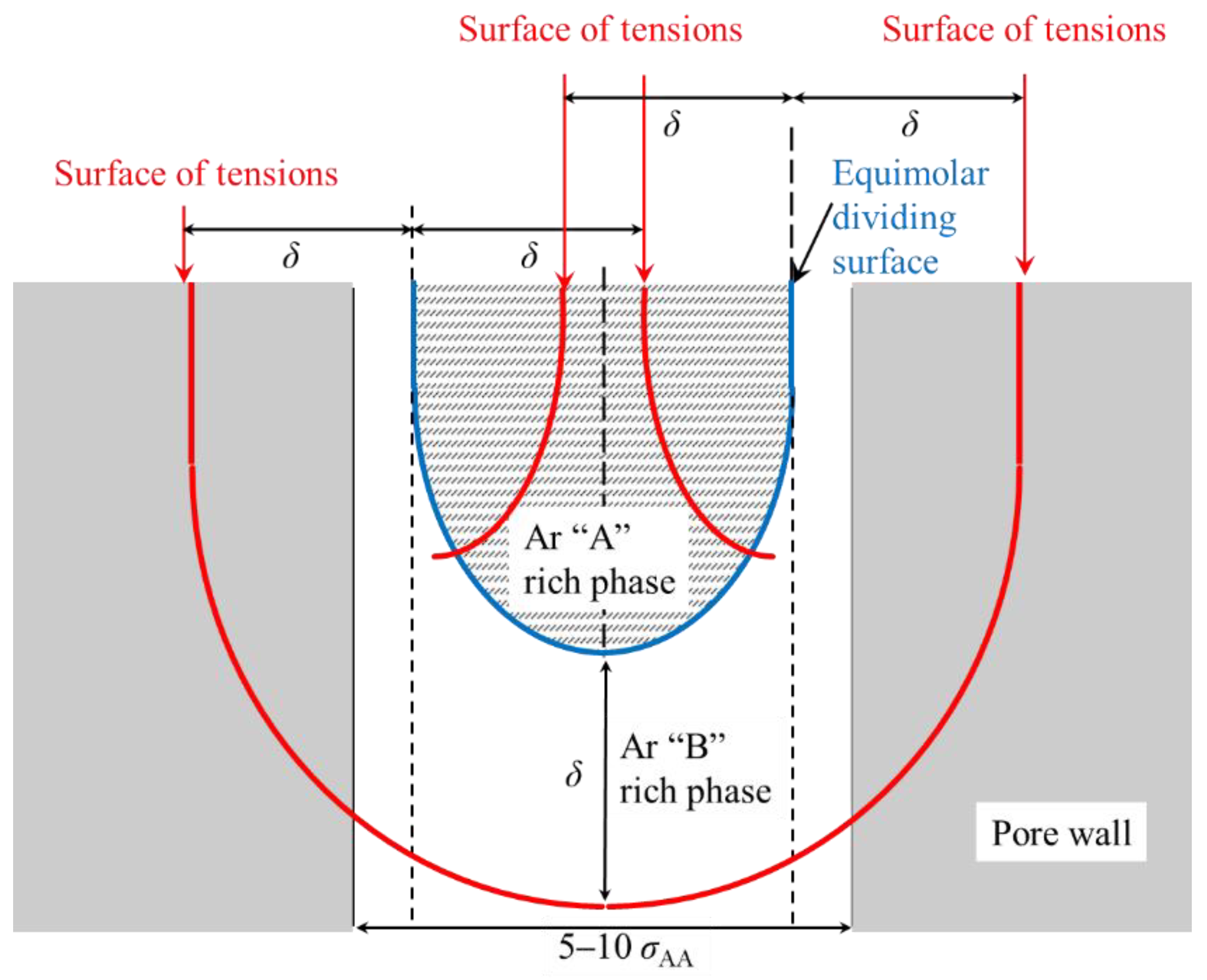
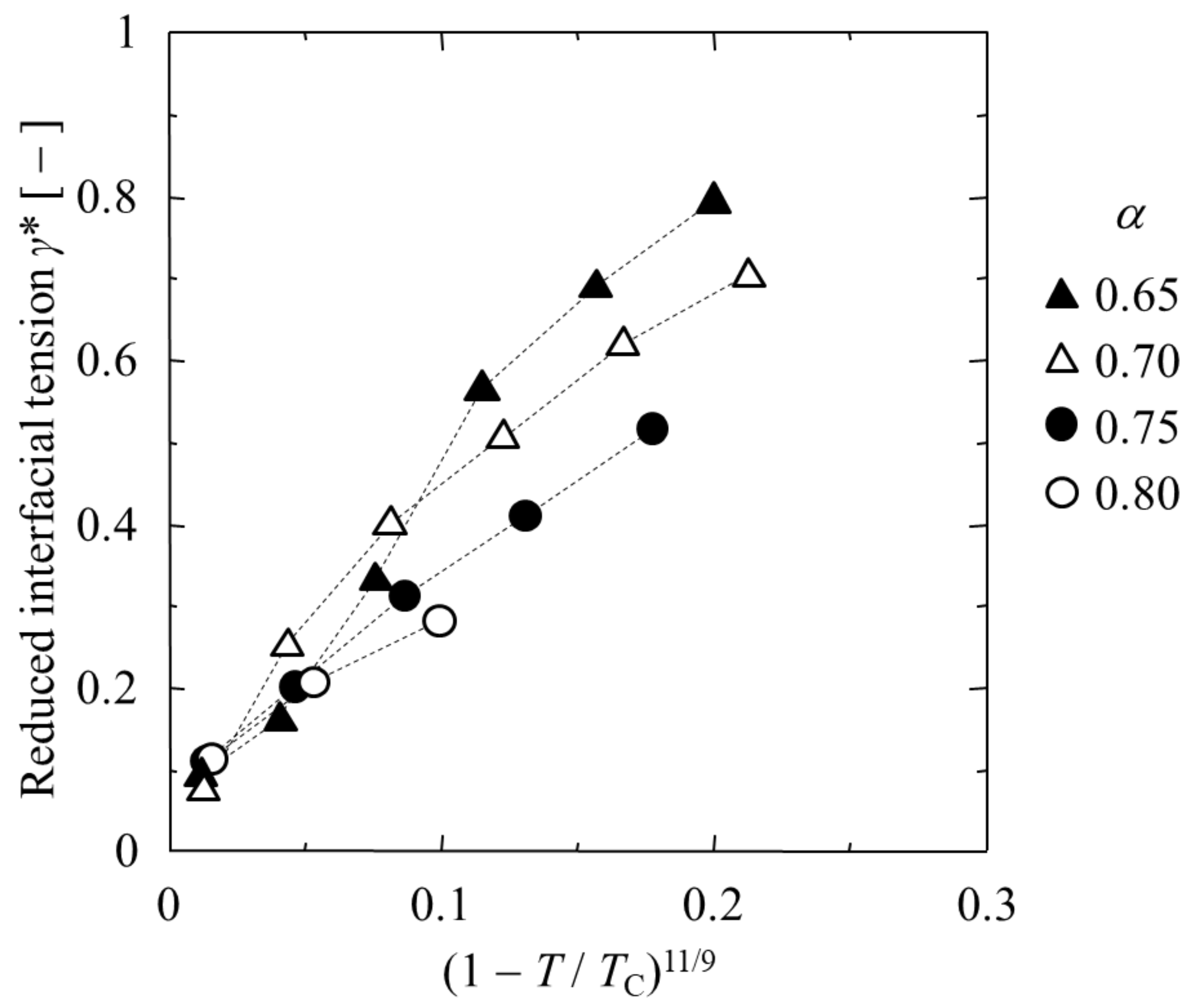
| α | T* | γ* | δ* | ||
|---|---|---|---|---|---|
| 0.85 | 0.70 | Complete mixture | |||
| 0.80 | 0.85 | Complete mixture | |||
| 0.80 | 0.660 | 0.113 | 0.114 | 2.99 | |
| 0.75 | 0.743 | 0.0632 | 0.207 | 1.80 | |
| 0.70 | 0.785 | 0.0432 | 0.283 | 1.73 | |
| 0.75 | 0.95 | Complete mixture | |||
| 0.90 | 0.615 | 0.0971 | 0.112 | 2.94 | |
| 0.85 | 0.690 | 0.0610 | 0.203 | 2.49 | |
| 0.80 | 0.745 | 0.384 | 0.314 | 1.95 | |
| 0.75 | 0.783 | 0.259 | 0.411 | 1.56 | |
| 0.70 | 0.813 | 0.154 | 0.517 | 1.48 | |
| 0.65 | 1.05 | Complete mixture | |||
| 1.00 | 0.614 | 0.0498 | 0.093 | 3.23 | |
| 0.95 | 0.675 | 0.0308 | 0.160 | 2.35 | |
| 0.90 | 0.721 | 0.0191 | 0.332 | 2.03 | |
| 0.85 | 0.752 | 0.0129 | 0.564 | 1.80 | |
| 0.80 | 0.783 | 0.0079 | 0.689 | 1.65 | |
| 0.75 | 0.808 | 0.0052 | 0.794 | 1.45 | |
| 0.70 | Complete separation | ||||
| 0.60 | 1.05 | Complete mixture | |||
| 1.00 | 0.651 | 0.0266 | 0.157 | 2.55 | |
| 0.95 | 0.697 | 0.0166 | 0.235 | 2.13 | |
| 0.90 | 0.730 | 0.0108 | 0.401 | 1.88 | |
| 0.85 | 0.756 | 0.0065 | 0.602 | 1.68 | |
| 0.80 | 0.793 | 0.0054 | 0.738 | 1.57 | |
| 0.75 | Complete separation | ||||
| 0.55 | 1.05 | Complete mixture | |||
| 1.00 | 0.664 | 0.0160 | 0.211 | 2.40 | |
| 0.95 | 0.704 | 0.0100 | 0.287 | 2.00 | |
| 0.90 | 0.737 | 0.0061 | 0.458 | 1.70 | |
| 0.85 | Complete separation | ||||
| 0.50 | 1.05 | Complete separation | |||
| 1.00 | Complete separation | ||||
| 0.95 | Complete separation | ||||
| 0.90 | Complete separation | ||||
| 0.85 | Complete separation | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kanda, H.; Wahyudiono; Goto, M. Molecular Dynamics Simulation of Tolman Length and Interfacial Tension of Symmetric Binary Lennard–Jones Liquid. Symmetry 2021, 13, 1376. https://doi.org/10.3390/sym13081376
Kanda H, Wahyudiono, Goto M. Molecular Dynamics Simulation of Tolman Length and Interfacial Tension of Symmetric Binary Lennard–Jones Liquid. Symmetry. 2021; 13(8):1376. https://doi.org/10.3390/sym13081376
Chicago/Turabian StyleKanda, Hideki, Wahyudiono, and Motonobu Goto. 2021. "Molecular Dynamics Simulation of Tolman Length and Interfacial Tension of Symmetric Binary Lennard–Jones Liquid" Symmetry 13, no. 8: 1376. https://doi.org/10.3390/sym13081376
APA StyleKanda, H., Wahyudiono, & Goto, M. (2021). Molecular Dynamics Simulation of Tolman Length and Interfacial Tension of Symmetric Binary Lennard–Jones Liquid. Symmetry, 13(8), 1376. https://doi.org/10.3390/sym13081376








