Some Symmetry Identities for Carlitz’s Type Degenerate Twisted (p,q)-Euler Polynomials Related to Alternating Twisted (p,q)-Sums

Abstract

In this paper, we define a new form of Carlitz’s type degenerate twisted $(p,q)$-Euler numbers and polynomials by generalizing the degenerate Euler numbers and polynomials, Carlitz’s type degenerate q-Euler numbers and polynomials. Some interesting identities, explicit formulas, symmetric properties, a connection with Carlitz’s type degenerate twisted $(p,q)$-Euler numbers and polynomials are obtained. Finally, we investigate the zeros of the Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials by using computer.

1. Introduction

Many mathematicians have been working in the fields of the degenerate Euler numbers and polynomials, degenerate Bernoulli numbers and polynomials, degenerate tangent numbers and polynomials, degenerate Genocchi numbers and polynomials, degenerate Stirling numbers, and special polynomials (see [1,2,3,4,5,6,7,8]). In recent years, we have been studied some properties and symmetry identiities of the degenerate Carlitz-type $(p,q)$-Euler numbers and polynomials, $(p,q)$-Euler zeta function, higher-order generalized twisted $(h,q)$-Euler polynomials, Dirichlet-type multiple twisted $(h,q)$-l-function, twisted $(h,p,q)$-Euler polynomials, and degenerate Carlitz-type q-Euler numbers and polynomial (see [9,10,11,12,13,14,15]).

In this paper we define a new form of Carlitz’s type degenerate twisted $(p,q)$-Euler numbers and polynomials and study some theories of the Carlitz’s type degenerate twisted $(p,q)$-Euler numbers and polynomials.

Throughout this paper, we always make use of the following classical notations: $\mathbb{N}$ denotes the set of natural numbers, $\mathbb{Z}$ denotes the set of integers, ${\mathbb{Z}}_0=\mathbb{N}\cup \left\{0\right\}$ denotes the set of nonnegative integers, $\mathbb{R}$ denotes the set of real numbers, and $\mathbb{C}$ denotes the set of complex numbers.

We recall that the degenerate Euler numbers ${\mathcal{E}}_n(\mu )$ and Euler polynomials ${\mathcal{E}}_n(z,\mu )$, which are defined by generating functions like following (see [2,3,4])

$${\displaystyle \sum _{n=0}^{\infty }}{\mathcal{E}}_n(\mu )\frac{t^n}{n!}=\frac{2}{{(1+\mu t)}^{\frac{1}{\mu }}+1},$$

and

$${\displaystyle \sum _{n=0}^{\infty }}{\mathcal{E}}_n(z,\mu )\frac{t^n}{n!}=\frac{2}{{(1+\mu t)}^{\frac{1}{\mu }}+1}{(1+\mu t)}^{\frac{z}{\mu }},$$

respectively.

We remind that well-known Stirling numbers of the first kind $S_1(n,j)$ and the second kind $S_2(n,j)$ are defined by this (see [2,4,6])

$${(z)}_n={\displaystyle \sum _{j=0}^n}{S}_1(n,j)z^j\mathrm{and}{z}^n={\displaystyle \sum _{j=0}^n}{S}_2(n,j){(z)}_j,$$

respectively. Here ${(z)}_j=z(z-1)\cdots (z-j+1)$. The numbers $S_2(n,j)$ is like this

$${\displaystyle \sum _{n=j}^{\infty }}{S}_2(n,j){\displaystyle \frac{t^n}{n!}}={\displaystyle \frac{{(e^t-1)}^j}{j!}}.$$

We also have

$${\displaystyle \sum _{n=j}^{\infty }}{S}_1(n,j){\displaystyle \frac{t^n}{n!}}={\displaystyle \frac{{(\log (1+t))}^j}{j!}}.$$

The generalized falling factorial ${(z|\mu )}_n$ with increment $\mu$ is defined by

$${(z|\mu )}_n={\displaystyle \prod _{k=0}^{n-1}}(z-\mu k)$$

for positive integer n, with ${(z|\mu )}_0=1$; as we know,

$${(z|\mu )}_n={\displaystyle \sum _{k=0}^n}{S}_1(n,k){\mu }^{n-k}{z}^k.$$

${(z|\mu )}_n={\mu }^n{({\mu }^{-1}z|1)}_n$ for $\mu \ne 0$. Clearly ${(z|0)}_n=z^n$. The binomial theorem is this for a variable z,

$${(1+\mu t)}^{z/\mu }={\displaystyle \sum _{n=0}^{\infty }}{(z|\mu )}_n{\displaystyle \frac{t^n}{n!}}.$$

For $z\in \mathbb{C}$, the $(p,q)$-number is defined by

$${[z]}_{p,q}=\frac{p^z-q^z}{p-q},(p\ne q).$$

The $(p,q)$-number is a natural generalization of the q-number, that is

$$\underset{p\to 1}{\lim }{[z]}_{p,q}={[z]}_q=\frac{1-q^z}{1-q},q\ne 1.$$

By using $(p,q)$-number, we define a new form of Carlitz’s type degenerate twisted $(p,q)$-Euler numbers and polynomials, which generalized the previously known numbers and polynomials, including the degenerate Euler numbers and polynomials, Carlitz’s type degenerate q-Euler numbers and polynomials (see [2,3,6,9]). We begin by recalling here the Carlitz’s type twisted $(p,q)$-Euler numbers and polynomials (see [13]). Let $\zeta$ be rth root of 1 and $\zeta \ne 1$ (see [13,14,16]).

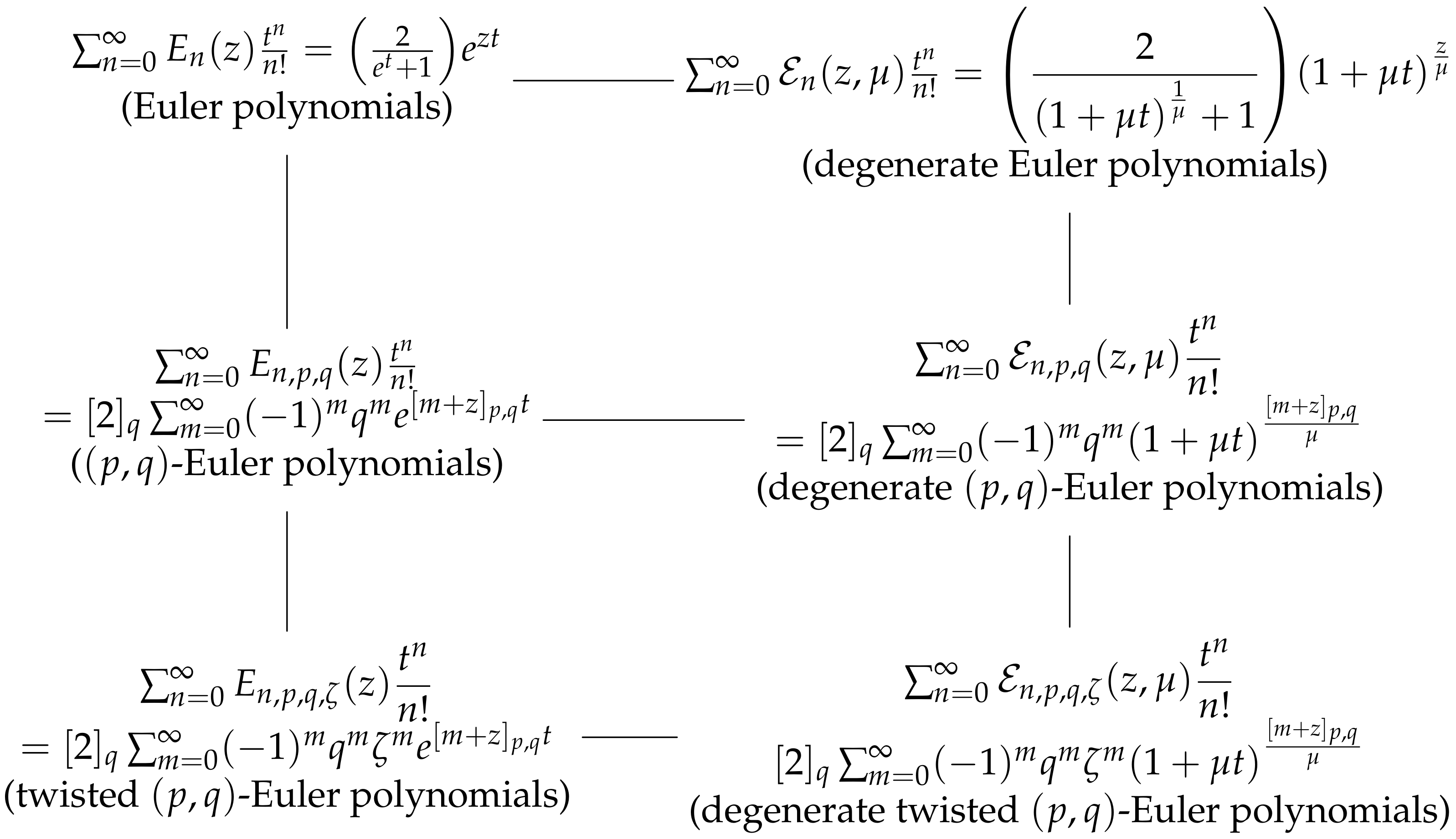
Definition 1.

The Carlitz’s type twisted $(p,q)$-Euler polynomials $E_{n,p,q,\zeta }(z)$ are defined by means of the generating function

$${\displaystyle \sum _{n=0}^{\infty }}{E}_{n,p,q,\zeta }(z)\frac{t^n}{n!}={[2]}_q{\displaystyle \sum _{m=0}^{\infty }}{(-1)}^m{q}^m{\zeta }^m{e}^{{[m+z]}_{p,q}t}.$$

and their values at $z=0$ are called the Carlitz’s type twisted $(p,q)$-Euler numbers and denoted $E_{n,p,q,\zeta }$.

Here is a brief introduction to the history for the reader. The following diagram shows the variations of the different types of Euler polynomials, degenerate Euler polynomials, $(p,q)$-Euler polynomials, degenerate $(p,q)$-Euler polynomials, and twisted $(p,q)$-Euler polynomials. Those polynomials in the first row and the second row of the diagram are studied by Carlitz [1,2], Cenkci, and Howard [3,4,5], Young [6], Hwang and Ryoo [9], Ryoo [10,11], Simsek [16], and Srivastava [17], respectively. The motivation of this paper is to investigate some interesting symmetric identities and explicit identities for Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials in the third row of the diagram.

Many generalizations of these numbers and polynomials can be found in the literature (see [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17]). Based on this idea, we generalize the Carlitz’s type twisted $(p,q)$-Euler numbers $E_{n,p,q,\zeta }$ and polynomials $E_{n,p,q,\zeta }(z)$, degenertae $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q}(\mu )$ and polynomials ${\mathcal{E}}_{n,p,q}(z,\mu )$, and degenerate q-Euler numbers ${\mathcal{E}}_{n,q}(\mu )$ and polynomials ${\mathcal{E}}_{n,q}(z,\mu )$. It follows that we define the following the Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ and polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$.

In the following section, we define a new form of Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ and polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$. After that we will investigate some their properties. In Section 2, Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ and polynomials ${\mathcal{E}}_{n,p,,\zeta }(z,\mu )$ are defined. We derive some of their relevant properties. In Section 3, first, we derive the symmetric properties for Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ and polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$. Finally, we investigate the zeros of the Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ and polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$ by using computer.

2. Carlitz’s Type Degenerate Twisted (p,q)-Euler Numbers and Polynomials

In this section, we define a new form of Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ and polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$, and provide some of their relevant properties. Let $\zeta$ be rth root of 1 and $\zeta \ne 1$ and $\lambda \in \mathbb{R}\setminus \left\{0\right\}$ (see [13,14,16]). Firstly, we construct the Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ and polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$ as follows:

Definition 2.

For $0<q<p\le 1$, the Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ and polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$ are defined by means of the generating functions

$${\displaystyle \sum _{n=0}^{\infty }}{\mathcal{E}}_{n,p,q,\zeta }(\mu )\frac{t^n}{n!}={[2]}_q{\displaystyle \sum _{m=0}^{\infty }}{(-1)}^m{q}^m{\zeta }^m{(1+\mu t)}^{{\displaystyle \frac{{[m]}_{p,q}}{\mu }}}.$$

(1)

and

$${\displaystyle \sum _{n=0}^{\infty }}{\mathcal{E}}_{n,p,q,\zeta }(z,\mu )\frac{t^n}{n!}={[2]}_q{\displaystyle \sum _{m=0}^{\infty }}{(-1)}^m{q}^m{\zeta }^m{(1+\mu t)}^{{\displaystyle \frac{{[m+z]}_{p,q}}{\mu }}},$$

(2)

respectively.

The Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ can be determined explicitly. A few of them are

$$\begin{array}{cc}\hfill {\mathcal{E}}_{0,p,q,\zeta }(\mu )=& {\displaystyle \frac{{[2]}_q}{1+\zeta q}},\hfill \\ \hfill {\mathcal{E}}_{1,p,q,\zeta }(\mu )=& {\displaystyle \frac{{[2]}_q}{(p-q)(1+\zeta pq)}}-{\displaystyle \frac{{[2]}_q}{(p-q)(1+\zeta q^2)}},\hfill \\ \hfill {\mathcal{E}}_{2,p,q,\zeta }(\mu )=& -{\displaystyle \frac{{[2]}_q\mu }{(p-q)(1+\zeta pq)}}+{\displaystyle \frac{{[2]}_q}{{(p-q)}^2(1+\zeta p^{2}q)}}+{\displaystyle \frac{{[2]}_q\mu }{(p-q)(1+\zeta q^2)}}\hfill \\ & -{\displaystyle \frac{2{[2]}_q}{{(p-q)}^2(1+\zeta p{q}^2)}}+{\displaystyle \frac{{[2]}_q}{{(p-q)}^2(1+\zeta q^3)}},\hfill \\ \hfill {\mathcal{E}}_{3,p,q,\zeta }(\mu )=& {\displaystyle \frac{2{[2]}_q{\mu }^2}{(p-q)(1+\zeta pq)}}-{\displaystyle \frac{3{[2]}_q\mu }{{(p-q)}^2(1+\zeta p^{2}q)}}+{\displaystyle \frac{{[2]}_q}{{(p-q)}^3(1+\zeta p^{3}q)}}-{\displaystyle \frac{2{[2]}_q{\mu }^2}{(p-q)(1+\zeta q^2)}}\hfill \\ & +{\displaystyle \frac{6{[2]}_q\mu }{{(p-q)}^2(1+\zeta p{q}^2)}}-{\displaystyle \frac{3{[2]}_q}{{(p-q)}^3(1+\zeta p^2{q}^2)}}-{\displaystyle \frac{3{[2]}_q\mu }{{(p-q)}^2(1+\zeta q^3)}}\hfill \\ & +{\displaystyle \frac{3{[2]}_q}{{(p-q)}^3(1+\zeta p{q}^3)}}-{\displaystyle \frac{{[2]}_q}{{(p-q)}^3(1+\zeta q^4)}}.\hfill \end{array}$$

Setting $p=1$ in (1) and (2), we can obtain the corresponding definitions for the Carlitz’s type degenerate twisted q-Euler number ${\mathcal{E}}_{n,q,\zeta }(\mu )$ and q-Euler polynomials ${\mathcal{E}}_{n,q,\zeta }(z,\mu )$, respectively (see [13]). Obviously, if we put $p=1$, then we have

$${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )={\mathcal{E}}_{n,q,\zeta }(z,\mu ),{\mathcal{E}}_{n,p,q,\zeta }(\mu )={\mathcal{E}}_{n,q,\zeta }(\mu ).$$

Putting $p=1$ and $\zeta =1$, we have

$$\underset{q\to 1}{\lim }{\mathcal{E}}_{n,p,q,\zeta }(z,\mu )={\mathcal{E}}_n(z,\mu ),\underset{q\to 1}{\lim }{\mathcal{E}}_{n,p,q,\zeta }(\mu )={\mathcal{E}}_n(\mu ).$$

Theorem 1.

For $n\in {\mathbb{Z}}_0$, we have

$$\begin{array}{cc}\hfill {\mathcal{E}}_{n,p,q,\zeta }(z,\mu )& ={[2]}_q{\displaystyle \sum _{l=0}^n}{\displaystyle \sum _{j=0}^l}{\displaystyle \frac{S_1(n,l){\mu }^{n-l}\left(\genfrac{}{}{0pt}{}{l}{j}\right){(-1)}^j{q}^{zj}{p}^{z(l-j)}}{{(p-q)}^l}}{\displaystyle \frac{1}{1+\zeta q^{j+1}{p}^{l-j}}}\hfill \\ & ={[2]}_q{\displaystyle \sum _{m=0}^{\infty }}{\displaystyle \sum _{l=0}^n}{S}_1(n,l){\mu }^{n-l}{(-1)}^m{q}^m{\zeta }^m{[z+m]}_{p,q}^l.\hfill \end{array}$$

Proof. 

Since

$$\begin{array}{cc}\hfill {(1+\mu t)}^{{\displaystyle \frac{{[z+2y]}_{p,q}}{\mu }}}& =e^{{\displaystyle \frac{{[z+2y]}_{p,q}}{\mu }}\log (1+\mu t)}\hfill \\ & ={\displaystyle \sum _{n=0}^{\infty }}{\left({\displaystyle \frac{{[z+2y]}_{p,q}}{\mu }}\right)}^n{\displaystyle \frac{{(\log (1+\mu t))}^n}{n!}}\hfill \\ & ={\displaystyle \sum _{n=0}^{\infty }}\left({\displaystyle \sum _{m=0}^n}{S}_1(n,m){\mu }^{n-m}{[z+2y]}_{p,q}^m\right){\displaystyle \frac{t^n}{n!}},\hfill \end{array}$$

(3)

we have

$$\begin{array}{c}{\displaystyle \sum _{n=0}^{\infty }}{\mathcal{E}}_{n,p,q,\zeta }(z,\mu )\frac{t^n}{n!}\hfill \\ ={[2]}_q{\displaystyle \sum _{m=0}^{\infty }}{(-1)}^m{q}^m{\zeta }^m{(1+\mu t)}^{{\displaystyle \frac{{[m+z]}_{p,q}}{\mu }}}\hfill \\ ={[2]}_q{\displaystyle \sum _{m=0}^{\infty }}{(-1)}^m{q}^m{\zeta }^m{\displaystyle \sum _{n=0}^{\infty }}{\displaystyle \sum _{l=0}^n}{S}_1(n,l){\mu }^{n-l}{\displaystyle \frac{{\sum }_{j=0}^l\left(\genfrac{}{}{0pt}{}{l}{j}\right){(-1)}^j{p}^{(z+m)(l-j)}{q}^{(z+m)j}}{{(p-q)}^l}}\frac{t^n}{n!}\hfill \\ ={\displaystyle \sum _{n=0}^{\infty }}\left({[2]}_q{\displaystyle \sum _{l=0}^n}{\displaystyle \sum _{j=0}^l}{\displaystyle \frac{S_1(n,l){\mu }^{n-l}\left(\genfrac{}{}{0pt}{}{l}{j}\right){(-1)}^j{q}^{zj}{p}^{z(l-j)}}{{(p-q)}^l}}{\displaystyle \frac{1}{1+\zeta q^{j+1}{p}^{l-j}}}\right)\frac{t^n}{n!}.\hfill \end{array}$$

(4)

By comparing the coefficients $\frac{t^n}{n!}$ in the above equation, the proof is completed. □

Theorem 2.

For $n\in {\mathbb{Z}}_0$, we have

$$E_{n,p,q,\zeta }(z)={\displaystyle \sum _{n=0}^m}{\mathcal{E}}_{n,p,q,\zeta }(z,\mu ){\mu }^{m-n}{S}_2(m,n).$$

Proof. 

By replacing t by ${\displaystyle \frac{e^{\mu t}-1}{\mu }}$ in (2), we have

$$\begin{array}{cc}\hfill E_{n,p,q,\zeta }(z)& ={\displaystyle \sum _{n=0}^{\infty }}{\mathcal{E}}_{n,p,q,\zeta }(z,\mu ){\left({\displaystyle \frac{e^{\mu t}-1}{\mu }}\right)}^n{\displaystyle \frac{1}{n!}}\hfill \\ & ={\displaystyle \sum _{n=0}^{\infty }}{\mathcal{E}}_{n,p,q,\zeta }(z,\mu ){\mu }^{-n}{\displaystyle \sum _{m=n}^{\infty }}{S}_2(m,n){\mu }^m{\displaystyle \frac{t^m}{m!}}\hfill \\ & ={\displaystyle \sum _{m=0}^{\infty }}\left({\displaystyle \sum _{n=0}^m}{\mathcal{E}}_{n,p,q,\zeta }(z,\mu ){\mu }^{m-n}{S}_2(m,n)\right){\displaystyle \frac{t^m}{m!}}.\hfill \end{array}$$

(5)

Thus, by (5), the proof is completed. □

Theorem 3.

For $m\in {\mathbb{Z}}_0$, we have

$${\mathcal{E}}_{m,p,q,\zeta }(z,\mu )={\displaystyle \sum _{k=0}^m}{E}_{k,p,q,\zeta }(z){\mu }^{m-k}{S}_1(m,k)$$

Proof. 

By replacing t by $\log {(1+\mu t)}^{1/\mu }$ in Definition 1, we have

$$\begin{array}{cc}\hfill {\displaystyle \sum _{n=0}^{\infty }}{E}_{n,p,q,\zeta }(z){\left(\log {(1+\mu t)}^{1/\mu }\right)}^n{\displaystyle \frac{1}{n!}}& ={[2]}_q{\displaystyle \sum _{m=0}^{\infty }}{(-1)}^m{q}^m{\zeta }^m{(1+\mu t)}^{{\displaystyle \frac{{[m+z]}_{p,q}}{\mu }}}\hfill \\ & ={\displaystyle \sum _{m=0}^{\infty }}{\mathcal{E}}_{m,p,q,\zeta }(z,\mu ){\displaystyle \frac{t^m}{m!}},\hfill \end{array}$$

(6)

and

$${\displaystyle \sum _{n=0}^{\infty }}{E}_{n,p,q,\zeta }(z){\left(\log {(1+\mu t)}^{1/\mu }\right)}^n{\displaystyle \frac{1}{n!}}={\displaystyle \sum _{m=0}^{\infty }}\left({\displaystyle \sum _{n=0}^m}{E}_{n,p,q,\zeta }(z){\mu }^{m-n}{S}_1(m,n)\right){\displaystyle \frac{t^m}{m!}}.$$

(7)

Thus, by (6) and (7), the proof is completed. □

We intriduce the $(p,q)$-analogue of the generalized falling factorial ${(z|\mu )}_n$ with increment $\mu$. The $(p,q)$-generalized falling factorial ${({[z]}_{p,q}|\mu )}_n$ with increment $\mu$ is defined by

$${({[z]}_{p,q}|\mu )}_n={\displaystyle \prod _{k=0}^{n-1}}({[z]}_{p,q}-\mu k)$$

for positive integer n, with the convention ${({[z]}_{p,q}|\mu )}_0=1$.

Theorem 4.

For $n\in {\mathbb{Z}}_0$, we have

$${\displaystyle \sum _{l=0}^{n-1}}{(-1)}^l{q}^l{\zeta }^l{({[l]}_{p,q}|\mu )}_m={\displaystyle \frac{{(-1)}^{n+1}{q}^n{\zeta }^n{\mathcal{E}}_{m,p,q,\zeta }(n,\mu )+{\mathcal{E}}_{m,p,q,\zeta }(\mu )}{{[2]}_q}}.$$

Proof. 

By (1) and (2), we get

$$\begin{array}{c}-{[2]}_q{(-1)}^n{q}^n{\zeta }^n{\displaystyle \sum _{l=0}^{\infty }}{(-1)}^l{q}^l{\zeta }^l{(1+\mu t)}^{{\displaystyle \frac{{[l+n]}_{p,q}}{\mu }}}+{[2]}_q{\displaystyle \sum _{l=0}^{\infty }}{(-1)}^l{q}^l{\zeta }^l{(1+\mu t)}^{{\displaystyle \frac{{[l+n]}_{p,q}}{\mu }}}\hfill \\ ={[2]}_q{\displaystyle \sum _{l=0}^{n-1}}{(-1)}^l{q}^l{\zeta }^l{(1+\mu t)}^{{\displaystyle \frac{{[l]}_{p,q}}{\mu }}}.\hfill \end{array}$$

(8)

Hence, by (8), we also have

$$\begin{array}{c}{(-1)}^{n+1}{q}^n{\zeta }^n{\displaystyle \sum _{m=0}^{\infty }}{\mathcal{E}}_{m,p,q,\zeta }(n,\mu ){\displaystyle \frac{t^m}{m!}}+{\displaystyle \sum _{m=0}^{\infty }}{\mathcal{E}}_{m,p,q,\zeta }(\mu ){\displaystyle \frac{t^m}{m!}}\hfill \\ ={\displaystyle \sum _{m=0}^{\infty }}\left({[2]}_q{\displaystyle \sum _{l=0}^{n-1}}{(-1)}^l{q}^l{\zeta }^l{({[l]}_{p,q}|\mu )}_m\right){\displaystyle \frac{t^m}{m!}}.\hfill \end{array}$$

(9)

By comparing the coefficients $\frac{t^m}{m!}$ on both sides of (9), the proof is completed. □

Theorem 5.

For $n\in {\mathbb{Z}}_0$, we have

$${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )={\displaystyle \sum _{k=0}^n}{\displaystyle \sum _{l=0}^k}\left(\genfrac{}{}{0pt}{}{n}{k}\right){(p^m{[z]}_{p,q}|\mu )}_{n-k}{\mu }^{k-l}{q}^{zl}{S}_1(k,l)E_{l,p,q,\zeta }.$$

Proof. 

We observe that

$$\begin{array}{cc}\hfill {\left(1+\mu t\right)}^{{\displaystyle \frac{{[z+y]}_{p,q}}{\mu }}}& ={(1+\mu t)}^{{\displaystyle \frac{p^y{[z]}_{p,q}}{\mu }}}{(1+\mu t)}^{{\displaystyle \frac{q^z{[y]}_{p,q}}{\mu }}}\hfill \\ & ={\displaystyle \sum _{m=0}^{\infty }}{(p^y{[z]}_{p,q}|\mu )}_m{\displaystyle \frac{t^m}{m!}}{e}^{\log {(1+\mu t)}^{{\displaystyle \frac{q^z{[y]}_{p,q}}{\mu }}}}\hfill \\ & ={\displaystyle \sum _{m=0}^{\infty }}{(p^y{[z]}_{p,q}|\mu )}_m{\displaystyle \frac{t^m}{m!}}{\displaystyle \sum _{l=0}^{\infty }}{\left({\displaystyle \frac{q^z{[y]}_{p,q}}{\mu }}\right)}^l{\displaystyle \frac{\log {(1+\mu t)}^l}{l!}}\hfill \\ & ={\displaystyle \sum _{m=0}^{\infty }}{(p^y{[z]}_{p,q}|\mu )}_m{\displaystyle \frac{t^m}{m!}}{\displaystyle \sum _{l=0}^{\infty }}{\left({\displaystyle \frac{q^z{[y]}_{p,q}}{\mu }}\right)}^l{\displaystyle \sum _{k=l}^{\infty }}{S}_1(k,l){\mu }^k{\displaystyle \frac{t^k}{k!}}\hfill \\ & ={\displaystyle \sum _{n=0}^{\infty }}\left({\displaystyle \sum _{k=0}^n}{\displaystyle \sum _{l=0}^k}\left(\genfrac{}{}{0pt}{}{n}{k}\right){(p^y{[z]}_{p,q}|\mu )}_{n-k}{\mu }^{k-l}{q}^{zl}{[y]}_{p,q}^l{S}_1(k,l)\right){\displaystyle \frac{t^n}{n!}}.\hfill \end{array}$$

(10)

By (2),

$$\begin{array}{cc}\hfill {\displaystyle \sum _{n=0}^{\infty }}{\mathcal{E}}_{n,p,q,\zeta }(z,\mu )\frac{t^n}{n!}& ={[2]}_q{\displaystyle \sum _{m=0}^{\infty }}{(-1)}^m{q}^m{\zeta }^m{(1+\mu t)}^{{\displaystyle \frac{{[m+z]}_{p,q}}{\mu }}}\hfill \\ & ={[2]}_q{\displaystyle \sum _{m=0}^{\infty }}{(-1)}^m{q}^m{\zeta }^m{\displaystyle \sum _{n=0}^{\infty }}\left({\displaystyle \sum _{k=0}^n}{\displaystyle \sum _{l=0}^k}\left(\genfrac{}{}{0pt}{}{n}{k}\right){(p^m{[z]}_{p,q}|\mu )}_{n-k}{\mu }^{k-l}{q}^{zl}{[m]}_{p,q}^l{S}_1(k,l)\right){\displaystyle \frac{t^n}{n!}}\hfill \\ & ={\displaystyle \sum _{n=0}^{\infty }}\left({\displaystyle \sum _{k=0}^n}{\displaystyle \sum _{l=0}^k}\left(\genfrac{}{}{0pt}{}{n}{k}\right){(p^m{[z]}_{p,q}|\mu )}_{n-k}{\mu }^{k-l}{q}^{zl}{S}_1(k,l)E_{l,p,q,\zeta }\right){\displaystyle \frac{t^n}{n!}},\hfill \end{array}$$

So the proof is completed. □

3. Symmetric Properties about Carlitz’s Type Degenerate Twisted (p,q)-Euler Numbers and Polynomials

In this section, we are going to obtain the main results of Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ and polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$. We also establish some interesting symmetric identities for Carlitz’s type degenerate twisted $(p,q)$-Euler numbers ${\mathcal{E}}_{n,p,q,\zeta }(\mu )$ and polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$.

Theorem 6.

Let a and b be odd positive integers. Then one has

$$\begin{array}{c}{[2]}_{q^a}{[b]}_{p,q}^n{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\mathcal{E}}_{n,p^b,q^b,{\zeta }^b}\left(az+\frac{ai}{b},{\displaystyle \frac{\mu }{{[b]}_{p,q}}}\right)\hfill \\ ={[2]}_{q^b}{[a]}_{p,q}^n{\displaystyle \sum _{j=0}^{a-1}}{(-1)}^j{q}^{bj}{\zeta }^{bj}{\mathcal{E}}_{n,p^a,q^a,{\zeta }^a}\left(bz+\frac{bi}{a},{\displaystyle \frac{\mu }{{[a]}_{p,q}}}\right).\hfill \end{array}$$

Proof. 

Observe that ${[zy]}_{p,q}={[z]}_{p^y,q^y}{[y]}_{p,q}$ for any $z,y\in \mathbb{C}$.

By substitute $az+\frac{ai}{b}$ for z in Definition 2, replace p by $p^b$, replace q by $q^b$, and replace $\zeta$ by ${\zeta }^b$, respectively, we derive

$$\begin{array}{c}{\displaystyle \sum _{n=0}^{\infty }}\left({[2]}_{q^a}{[b]}_{p,q}^n{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\mathcal{E}}_{n,p^b,q^b,{\zeta }^b}\left(az+\frac{ai}{b},{\displaystyle \frac{\mu }{{[b]}_{p,q}}}\right)\right){\displaystyle \frac{t^n}{n!}}\hfill \\ ={[2]}_{q^a}{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\displaystyle \sum _{n=0}^{\infty }}{\mathcal{E}}_{n,p^b,q^b,{\zeta }^b}\left(az+\frac{ai}{b},{\displaystyle \frac{\mu }{{[b]}_{p,q}}}\right){\displaystyle \frac{{({[b]}_{p,q}t)}^n}{n!}}\hfill \\ ={[2]}_{q^a}{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{[2]}_{q^b}{\displaystyle \sum _{n=0}^{\infty }}{(-1)}^n{q}^{bn}{\zeta }^{bn}{\left(1+{\displaystyle \frac{\mu }{{[b]}_{p,q}}}{[b]}_{p,q}t\right)}^{{\displaystyle \frac{{[az+\frac{ai}{b}+n]}_{p^b,q^b}}{\frac{\mu }{{[b]}_{p,q}}}}}\hfill \\ ={[2]}_{q^a}{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{[2]}_{q^b}{\displaystyle \sum _{n=0}^{\infty }}{(-1)}^n{q}^{bn}{\zeta }^{bn}{\left(1+\mu t\right)}^{{\displaystyle \frac{{[abz+ai+nb]}_{p,q}}{\mu }}}.\hfill \end{array}$$

Since for any non-negative integer n and odd positive integer a, there exist unique non-negative integer r such that $n=ar+j$ with $0\le j\le a-1$. So this can be written as

$$\begin{array}{l}{[2]}_{q^a}{[2]}_{q^b}{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\displaystyle \sum _{n=0}^{\infty }}{(-1)}^n{q}^{bn}{\zeta }^{bn}\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\times {\left(1+\mu t\right)}^{{\displaystyle \frac{{[abz+ai+nb]}_{p,q}}{\mu }}}.\\ ={[2]}_{q^a}{[2]}_{q^b}{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\displaystyle \sum _{\begin{array}{c}ar+j=0\\ 0\le j\le a-1\end{array}}^{\infty }}{(-1)}^{ar+j}{q}^{b(ar+j)}{\zeta }^{b(ar+j)}\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\times {\left(1+\mu t\right)}^{{\displaystyle \frac{{[abz+ai+(ar+j)b]}_{p,q}}{\mu }}}.\\ ={[2]}_{q^a}{[2]}_{q^b}{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\displaystyle \sum _{j=0}^{a-1}}{\displaystyle \sum _{r=0}^{\infty }}{(-1)}^{ar}{(-1)}^j{q}^{bar}{q}^{bj}{\zeta }^{bar}{\zeta }^{bj}\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\times {\left(1+\mu t\right)}^{{\displaystyle \frac{{[abz+ai+abr+bj]}_{p,q}}{\mu }}}\\ ={[2]}_{q^a}{[2]}_{q^b}{\displaystyle \sum _{i=0}^{b-1}}{\displaystyle \sum _{j=0}^{a-1}}{\displaystyle \sum _{r=0}^{\infty }}{(-1)}^i{(-1)}^r{(-1)}^j{q}^{ai}{q}^{bar}{q}^{bj}{\zeta }^{ai}{\zeta }^{bar}{\zeta }^{bj}\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\times {\left(1+\mu t\right)}^{{\displaystyle \frac{{[abz+ai+abr+bj]}_{p,q}}{\mu }}}.\end{array}$$

It follows from the above equation that

$$\begin{array}{l}{\displaystyle \sum _{n=0}^{\infty }}\left({[2]}_{q^b}{[b]}_{p,q}^n{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\mathcal{E}}_{n,p^b,q^b,{\zeta }^b}\left(az+\frac{ai}{b},{\displaystyle \frac{\mu }{{[b]}_{p,q}}}\right)\right){\displaystyle \frac{t^n}{n!}}\\ ={[2]}_{q^a}{[2]}_{q^b}{\displaystyle \sum _{i=0}^{b-1}}{\displaystyle \sum _{j=0}^{a-1}}{\displaystyle \sum _{r=0}^{\infty }}{(-1)}^i{(-1)}^r{(-1)}^j{q}^{ai}{q}^{bar}{q}^{bj}{\zeta }^{ai}{\zeta }^{bar}{\zeta }^{bj}\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\times {\left(1+\mu t\right)}^{{\displaystyle \frac{{[abz+ai+abr+bj]}_{p,q}}{\mu }}}.\end{array}$$

(11)

From the similar method, we can obtain that

$$\begin{array}{l}{\displaystyle \sum _{n=0}^{\infty }}\left({[2]}_{q^b}{[a]}_{p,q}^n{\displaystyle \sum _{i=0}^{a-1}}{(-1)}^i{q}^{bi}{\zeta }^{bi}{\mathcal{E}}_{n,p^a,q^a,{\zeta }^a}\left(bz+\frac{bi}{a},{\displaystyle \frac{\mu }{{[a]}_{p,q}}}\right)\right){\displaystyle \frac{t^n}{n!}}\\ ={[2]}_{q^a}{[2]}_{q^b}{\displaystyle \sum _{i=0}^{a-1}}{\displaystyle \sum _{j=0}^{b-1}}{\displaystyle \sum _{r=0}^{\infty }}{(-1)}^i{(-1)}^r{(-1)}^j{q}^{bi}{q}^{aar}{q}^{aj}{\zeta }^{bi}{\zeta }^{bar}{\zeta }^{aj}\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\times {\left(1+\mu t\right)}^{{\displaystyle \frac{{[abz+bi+abr+aj]}_{p,q}}{\mu }}}.\end{array}$$

(12)

Thus, from (11) and (12), the proof is completed. □

It follows that we show some special cases of Theorem 6. Setting $b=1$ in Theorem 6, we have the multiplication theorem for the Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$.

Corollary 1.

Let a be odd positive integer. Then one has

$${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )={\displaystyle \frac{{[2]}_q}{{[2]}_{q^a}}}{[a]}_{p,q}^n{\displaystyle \sum _{j=0}^{a-1}}{(-1)}^j{q}^j{\zeta }^j{\mathcal{E}}_{n,p^a,q^a,{\zeta }^a}\left({\displaystyle \frac{z+i}{a}},{\displaystyle \frac{\mu }{{[a]}_{p,q}}}\right).$$

(13)

Let $z=0$ in Theorem 6, we have the following corollary.

Corollary 2.

Let a and b be odd positive integers. Then it has

$$\begin{array}{l}{[2]}_{q^a}{[b]}_{p,q}^n{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\mathcal{E}}_{n,p^b,q^b,{\zeta }^b}\left(\frac{ai}{b},{\displaystyle \frac{\mu }{{[b]}_{p,q}}}\right)\\ ={[2]}_{q^b}{[a]}_{p,q}^n{\displaystyle \sum _{j=0}^{a-1}}{(-1)}^j{q}^{bj}{\zeta }^{bj}{\mathcal{E}}_{n,p^a,q^a,{\zeta }^a}\left(\frac{bj}{a},{\displaystyle \frac{\mu }{{[a]}_{p,q}}}\right).\end{array}$$

By Theorem 3 and Corollary 2, we have the below theorem.

Theorem 7.

Let a and b be odd positive integers. Then

$$\begin{array}{l}{\displaystyle \sum _{l=0}^n}{S}_1(n,l){\mu }^{n-l}{[b]}_{p,q}^l{[2]}_{q^a}{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{E}_{l,p^b,q^b,{\zeta }^b}\left(\frac{a}{b}i\right)\\ ={\displaystyle \sum _{l=0}^n}{S}_1(n,l){\mu }^{n-l}{[a]}_{p,q}^l{[2]}_{q^b}{\displaystyle \sum _{j=0}^{a-1}}{(-1)}^j{q}^{bj}{\zeta }^{bj}{E}_{l,p^a,q^a,{\zeta }^a}\left(\frac{b}{a}j\right).\end{array}$$

We get another result by applying the addition theorem about the Carlitz-type twisted $(h,p,q)$-Euler polynomials $E_{n,p,q,\zeta }^{(h)}(x)$ (see [14]).

Theorem 8.

Let a and b be odd positive integers. Then we have

$$\begin{array}{l}{[2]}_{q^a}{\displaystyle \sum _{l=0}^n}{\displaystyle \sum _{k=0}^l}\left(\genfrac{}{}{0pt}{}{l}{k}\right)S_1(n,l){\mu }^{n-l}{p}^{abzk}{[a]}_{p,q}^k{[b]}_{p,q}^{l-k}{E}_{l-k,p^b,q^b,{\zeta }^b}^{(k)}(az)S_{l,k,p^a,q^a,{\zeta }^a}(b)\\ ={[2]}_{q^b}{\displaystyle \sum _{l=0}^n}{\displaystyle \sum _{k=0}^l}\left(\genfrac{}{}{0pt}{}{l}{k}\right)S_1(n,l){\mu }^{n-l}{p}^{abzk}{[b]}_{p,q}^k{[a]}_{p,q}^{l-k}{E}_{l-k,p^a,q^a,{\zeta }^a}^{(k)}(bz)S_{l,k,p^b,q^b,{\zeta }^b}(a),\end{array}$$

where $S_{l,k,p,q,\zeta }(a)={\sum }_{i=0}^{a-1}{(-1)}^i{q}^{(l-k+1)i}{\zeta }^i{[i]}_{p,q}^k$ is called as the alternating twisted $(p,q)$-sums.

Proof. 

From (3), Theorem 3, and Theorem 6, we have

$$\begin{array}{l}{[2]}_{q^a}{[b]}_{p,q}^n{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\mathcal{E}}_{n,p^b,q^b,{\zeta }^b}\left(az+\frac{ai}{b},{\displaystyle \frac{\mu }{{[b]}_{p,q}}}\right)\\ ={[2]}_{q^a}{[b]}_{p,q}^n{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\displaystyle \sum _{l=0}^n}{E}_{l,p^b,q^b,{\zeta }^b}\left(az+\frac{ai}{b}\right){\left({\displaystyle \frac{\mu }{{[b]}_{p,q}}}\right)}^{n-l}{S}_1(n,l)\\ ={[2]}_{q^a}{\displaystyle \sum _{l=0}^n}{S}_1(n,l){\mu }^{n-l}{[b]}_{p,q}^l{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\zeta }^{ai}{\displaystyle \sum _{k=0}^l}\left(\genfrac{}{}{0pt}{}{l}{k}\right)q^{a(l-k)i}{p}^{abzk}\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\times E_{l-k,p^b,q^b,{\zeta }^b}^{(k)}(az){\left(\frac{{[a]}_{p,q}}{{[b]}_{p,q}}\right)}^k{[i]}_{p^a,q^a}^k\\ ={[2]}_{q^a}{\displaystyle \sum _{l=0}^n}{S}_1(n,l){\mu }^{n-l}{\displaystyle \sum _{k=0}^l}\left(\genfrac{}{}{0pt}{}{l}{k}\right)p^{abzk}{[a]}_{p,q}^k{[b]}_{p,q}^{l-k}{E}_{l-k,p^b,q^b,{\zeta }^b}^{(k)}(az)\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\times {\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{q}^{(l-k)ai}{\zeta }^{ai}{[i]}_{p^a,q^a}^k.\end{array}$$

Therefore, we induce that

$$\begin{array}{l}{[2]}_{q^a}{[b]}_{p,q}^n{\displaystyle \sum _{i=0}^{b-1}}{(-1)}^i{q}^{ai}{\mathcal{E}}_{n,p^b,q^b,{\zeta }^b}\left(az+\frac{ai}{b},{\displaystyle \frac{\mu }{{[b]}_{p,q}}}\right)\\ ={\displaystyle \sum _{l=0}^n}{\displaystyle \sum _{k=0}^l}\left(\genfrac{}{}{0pt}{}{l}{k}\right)S_1(n,l){\mu }^{n-l}{p}^{abzk}{[2]}_{q^a}{[a]}_{p,q}^k{[b]}_{p,q}^{l-k}{E}_{l-k,p^b,q^b,{\zeta }^b}^{(k)}(az)S_{l,k,p^a,q^a,{\zeta }^a}(b),\end{array}$$

(14)

and

$$\begin{array}{l}{[2]}_{q^b}{[a]}_{p,q}^n{\displaystyle \sum _{j=0}^{a-1}}{(-1)}^j{q}^{bj}{\mathcal{E}}_{n,p^a,q^a,{\zeta }^a}\left(bx+\frac{bj}{a},{\displaystyle \frac{\lambda }{{[a]}_{p,q}}}\right)\\ ={\displaystyle \sum _{l=0}^n}{\displaystyle \sum _{k=0}^l}\left(\genfrac{}{}{0pt}{}{l}{k}\right)S_1(n,l){\mu }^{n-l}{p}^{abzk}{[2]}_{q^b}{[b]}_{p,q}^k{[a]}_{p,q}^{l-k}{E}_{l-k,p^a,q^a,{\zeta }^a}^{(k)}(bz)S_{l,k,p^b,q^b,{\zeta }^b}(a).\end{array}$$

(15)

By (14) and (15), we make the desired symmetric identity. □

In particular, the case $a=3$ in Corollary 1 gives the triplication formula for Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials

$$\begin{array}{l}{\mathcal{E}}_{n,p^3,q^3,{\zeta }^3}\left({\displaystyle \frac{z}{3}},{\displaystyle \frac{\mu }{{[3]}_{p,q}}}\right)+q^2{\zeta }^2{\mathcal{E}}_{n,p^3,q^3,{\zeta }^3}\left({\displaystyle \frac{z+2}{3}},{\displaystyle \frac{\mu }{{[3]}_{p,q}}}\right)\\ ={\displaystyle \frac{{[2]}_{q^3}}{{[2]}_q{[3]}_{p,q}^n}}{\mathcal{E}}_{n,p,q,\zeta }(z,\mu )+q\zeta {\mathcal{E}}_{n,p^3,q^3,{\zeta }^3}\left({\displaystyle \frac{z+1}{3}},{\displaystyle \frac{\mu }{{[3]}_{p,q}}}\right).\end{array}$$

(16)

Setting $p=1$ in (13) and (16) leads to the familiar multiplication theorem for the Carlitz’s type degenerate twisted q-Euler polynomials

$${\mathcal{E}}_{n,q,\zeta }(z,\mu )={\displaystyle \frac{{[2]}_q{[a]}_q^n}{{[2]}_{q^a}}}{\displaystyle \sum _{j=0}^{a-1}}{(-1)}^j{q}^j{\zeta }^j{\mathcal{E}}_{n,q^a,{\zeta }^a}\left({\displaystyle \frac{z+i}{a}},{\displaystyle \frac{\mu }{{[a]}_q}}\right).$$

(17)

and the triplication formula for Carlitz’s type degenerate twisted q-Euler polynomials

$$\begin{array}{l}{\mathcal{E}}_{n,q^3,{\zeta }^3}\left({\displaystyle \frac{z}{3}},{\displaystyle \frac{\mu }{{[3]}_q}}\right)+q^2{\zeta }^2{\mathcal{E}}_{n,q^3,{\zeta }^3}\left({\displaystyle \frac{z+2}{3}},{\displaystyle \frac{\mu }{{[3]}_q}}\right)\\ ={\displaystyle \frac{{[2]}_{q^3}}{{[2]}_q{[3]}_q^n}}{\mathcal{E}}_{n,q,\zeta }(z,\mu )+q\zeta {\mathcal{E}}_{n,q^3,{\zeta }^3}\left({\displaystyle \frac{z+1}{3}},{\displaystyle \frac{\mu }{{[3]}_q}}\right).\end{array}$$

(18)

Letting $q\to 1$ in (17) and (18) leads to the familiar multiplication theorem for the degenerate twisted Euler polynomials

$${\mathcal{E}}_{n,\zeta }(z,\mu )=a^n{\displaystyle \sum _{j=0}^{a-1}}{(-1)}^j{\zeta }^j{\mathcal{E}}_{n,{\zeta }^a}\left({\displaystyle \frac{z+i}{a}},{\displaystyle \frac{\mu }{a}}\right)$$

(19)

and the triplication formula for degenerate twisted Euler polynomials

$${\mathcal{E}}_{n,{\zeta }^3}\left({\displaystyle \frac{z}{3}},{\displaystyle \frac{\mu }{3}}\right)+{\zeta }^2{\mathcal{E}}_{n,{\zeta }^3}\left({\displaystyle \frac{z+2}{3}},{\displaystyle \frac{\mu }{3}}\right)={\displaystyle \frac{1}{3^n}}{\mathcal{E}}_{n,\zeta }(z,\mu )+\zeta {\mathcal{E}}_{n,{\zeta }^3}\left({\displaystyle \frac{z+1}{3}},{\displaystyle \frac{\mu }{3}}\right).$$

(20)

Letting $\zeta =1$ in (19) and (20) leads to the familiar multiplication theorem for the degenerate Euler polynomials

$${\mathcal{E}}_n(z,\mu )=a^n{\displaystyle \sum _{j=0}^{a-1}}{(-1)}^j{\mathcal{E}}_n\left({\displaystyle \frac{z+i}{a}},{\displaystyle \frac{\mu }{a}}\right)$$

(21)

and the triplication formula for degenerate Euler polynomials

$${\mathcal{E}}_n\left({\displaystyle \frac{z}{3}},{\displaystyle \frac{\mu }{3}}\right)+{\mathcal{E}}_n\left({\displaystyle \frac{z+2}{3}},{\displaystyle \frac{\mu }{3}}\right)={\displaystyle \frac{1}{3^n}}{\mathcal{E}}_n(z,\mu )+{\mathcal{E}}_n\left({\displaystyle \frac{z+1}{3}},{\displaystyle \frac{\mu }{3}}\right).$$

(22)

Letting $\mu \to 0$ in (21) and (22) leads to the familiar multiplication theorem for the Euler polynomials

$$E_n(z)=a^n{\displaystyle \sum _{j=0}^{a-1}}{(-1)}^j{E}_n\left({\displaystyle \frac{z+i}{a}}\right)$$

and the triplication formula for Euler polynomials

$$E_n(z)=3^n{E}_n\left({\displaystyle \frac{z}{3}}\right)-3^n{E}_n\left({\displaystyle \frac{z+1}{3}}\right)+3^n{E}_n\left({\displaystyle \frac{z+2}{3}}\right).$$

4. Zeros of the Carlitz’s Type Degenerate Twisted (p,q)-Euler Numbers and Polynomials

In this section, we demonstrate the benefit of using numerical investigation to support theoretical prediction and to observe novel, interesting pattern of the solutions of the Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$. The Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )$ can be determined explicitly. A few of them are

$$\begin{array}{cc}\hfill {\mathcal{E}}_{0,p,q,\zeta }(z,\mu )& ={\displaystyle \frac{{[2]}_q}{1+\zeta q}},\hfill \\ \hfill {\mathcal{E}}_{1,p,q,\zeta }(z,\mu )& ={\displaystyle \frac{{[2]}_q{p}^z}{(p-q)(1+\zeta pq)}}-{\displaystyle \frac{{[2]}_q{q}^z}{(p-q)(1+\zeta q^2)}},\hfill \end{array}$$

$$\begin{array}{cc}\hfill {\mathcal{E}}_{2,p,q,\zeta }(z,\mu )=& -{\displaystyle \frac{{[2]}_q\mu p^z}{(p-q)(1+\zeta pq)}}+{\displaystyle \frac{{[2]}_q{p}^{2z}}{{(p-q)}^2(1+\zeta p^{2}q)}}+{\displaystyle \frac{{[2]}_q\mu q^z}{(p-q)(1+\zeta q^2)}}\hfill \\ & -{\displaystyle \frac{{[2]}_{q}2p^z{q}^z}{{(p-q)}^2(1+\zeta p{q}^2)}}+{\displaystyle \frac{{[2]}_q{q}^{2z}}{{(p-q)}^2(1+\zeta q^3)}},\hfill \\ \hfill {\mathcal{E}}_{3,p,q,\zeta }(z,\mu )=& {\displaystyle \frac{2{[2]}_q{p}^z{\mu }^2}{(p-q)(1+\zeta pq)}}-{\displaystyle \frac{3{[2]}_q{p}^{2z}\mu }{{(p-q)}^2(1+\zeta p^{2}q)}}+{\displaystyle \frac{{[2]}_q{p}^{3z}}{{(p-q)}^3(1+\zeta p^{3}q)}}-{\displaystyle \frac{2{[2]}_q{q}^z{\mu }^2}{(p-q)(1+\zeta q^2)}}\hfill \\ & +{\displaystyle \frac{6{[2]}_q{p}^z{q}^z\mu }{{(p-q)}^2(1+\zeta p{q}^2)}}-{\displaystyle \frac{3{[2]}_q{p}^{2z}{q}^z}{{(p-q)}^3(1+\zeta p^2{q}^2)}}-{\displaystyle \frac{3{[2]}_q{q}^{2z}\mu }{{(p-q)}^2(1+\zeta q^3)}}\hfill \\ & +{\displaystyle \frac{3{[2]}_q{p}^z{q}^{2z}}{{(p-q)}^3(1+\zeta p{q}^3)}}-{\displaystyle \frac{{[2]}_q{q}^{3z}}{{(p-q)}^3(1+\zeta q^4)}}.\hfill \end{array}$$

Our numerical results for approximate solutions of complex zeros of ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$ are displayed (

Table 1. Numbers of real and complex zeros of ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$, $\mu \to 0$.

	$\mathit{p}=1/2,\mathit{q}=1/10,\mathit{\zeta }={\mathit{e}}^{2\mathit{\pi }\mathit{i}}$		$\mathit{p}=1/2,\mathit{q}=1/10,\mathit{\zeta }={\mathit{e}}^{\frac{\mathit{\pi }\mathit{i}}{2}}$
Degree n	Real Zeros	Complex Zeros	Real Zeros	Complex Zeros
1	1	0	0	1
2	*	*	*	*
3	1	2	0	3
4	*	*	*	*
5	1	4	0	5
6	2	4	0	6
7	1	6	0	7
8	*	*	*	*
9	1	8	0	9
10	2	8	0	10

and

Table 2. Approximate solutions of ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0,p=1/2,q=1/10,z\in \mathbb{R}$.

Degree n	z
1	0.0241325
3	0.133545
5	0.194723
6	−0.141066, 0.21479
7	0.230656
9	0.254116
10	−0.160295, 0.263028
11	0.270618

).

The ∗ mark in Table 1 means that there is no solution of ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$. As a result of numerical experiments, it was found that ther is no solution of ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$ for $p=1/2,q=1/10,n=2^k,k=1,2,3,\dots$ We hope to verify that there is no solution of ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$ for $p=1/2,q=1/10,n=2^k,k=1,2,3,\dots$ We can see a regular pattern of the roots of the ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$ and also hope to verify the same kind of regular structure of the roots of the ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$ (Table 1).

We investigate the zeros of the ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$ by using a computer. We plot the zeros of the ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$ for $z\in \mathbb{C}$ (Figure 1).

In Figure 1 (top-left), we choose $n=10,p=1/2,$$q=1/10,\mu \to 0,$ and $\zeta =e^{2\pi i}.$ In Figure 1 (top-right), we choose $n=10,p=1/2,$$q=1/10,\mu \to 0,$ and $\zeta =e^{\frac{\pi i}{2}}.$ In Figure 1 (bottom-left), we choose $n=20,p=1/2,$$q=1/10,\mu \to 0,$ and $\zeta =e^{2\pi i}.$ In Figure 1 (bottom-right), we choose $n=20,p=1/2,$$q=1/10,\mu \to 0,$ and $\zeta =e^{\frac{\pi i}{2}}.$ The ● mark in Figure 1 means the distribution of zeros of ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$. We observed that zeros of the ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$ have no $Re(x)=a$ reflection symmetry for $a\in \mathbb{R}$.

Next, we calculated an approximate solution satisfying ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$ for $z\in \mathbb{R}$. The results are given in Table 2.

In Figure 1, the pink dots represent the distribution of zeros.

In Table 2, we choose $\mu \to 0,$ and $\zeta =e^{2\pi i}.$

5. Conclusions and Discussion

In our previous paper [13], we studied some identities of symmetry on the Carlitz-type twisted $(p,q)$-Euler numbers and polynomials. The motivation of this paper is to construct the Carlitz’s type degenerate twisted $(p,q)$-Euler numbers and polynomials. We also investigate some explicit identities for the Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials in the third row of the diagram at page 3. Therefore, we construct the Carlitz’s type degenerate twisted $(p,q)$-Euler numbers and polynomials in the Definition 2 and obtained the formulas (Theorems 1 and 5), distribution relation (Corollary 1). In Theorems 6 and 7, we gave some symmetry identities for the Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials. We also obtained the explicit identities related with the Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials, Carlitz’s type wisted $(h,p,q)$-Euler polynomials, the alternating twisted $(p,q)$-sums, and Stirling numbers (see Theorems 7 and 8). Finally, we observed novel, interesting pattern of the solutions of the Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$.

If we can give a theoretical prediction via numerical experiments by finding a regular pattern for the roots of the Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$, we look forward to contributing to research related to the Carlitz’s type degenerate twisted $(p,q)$-Euler polynomials ${\mathcal{E}}_{n,p,q,\zeta }(z,\mu )=0$ in applied mathematics, mathematical physics, and engineering.