Quantum Particle on Dual Weight Lattice in Weyl Alcove
Abstract
1. Introduction
2. Dual-Weight Fourier–Weyl Transforms
2.1. Weyl Groups and Invariant Shifted Lattices
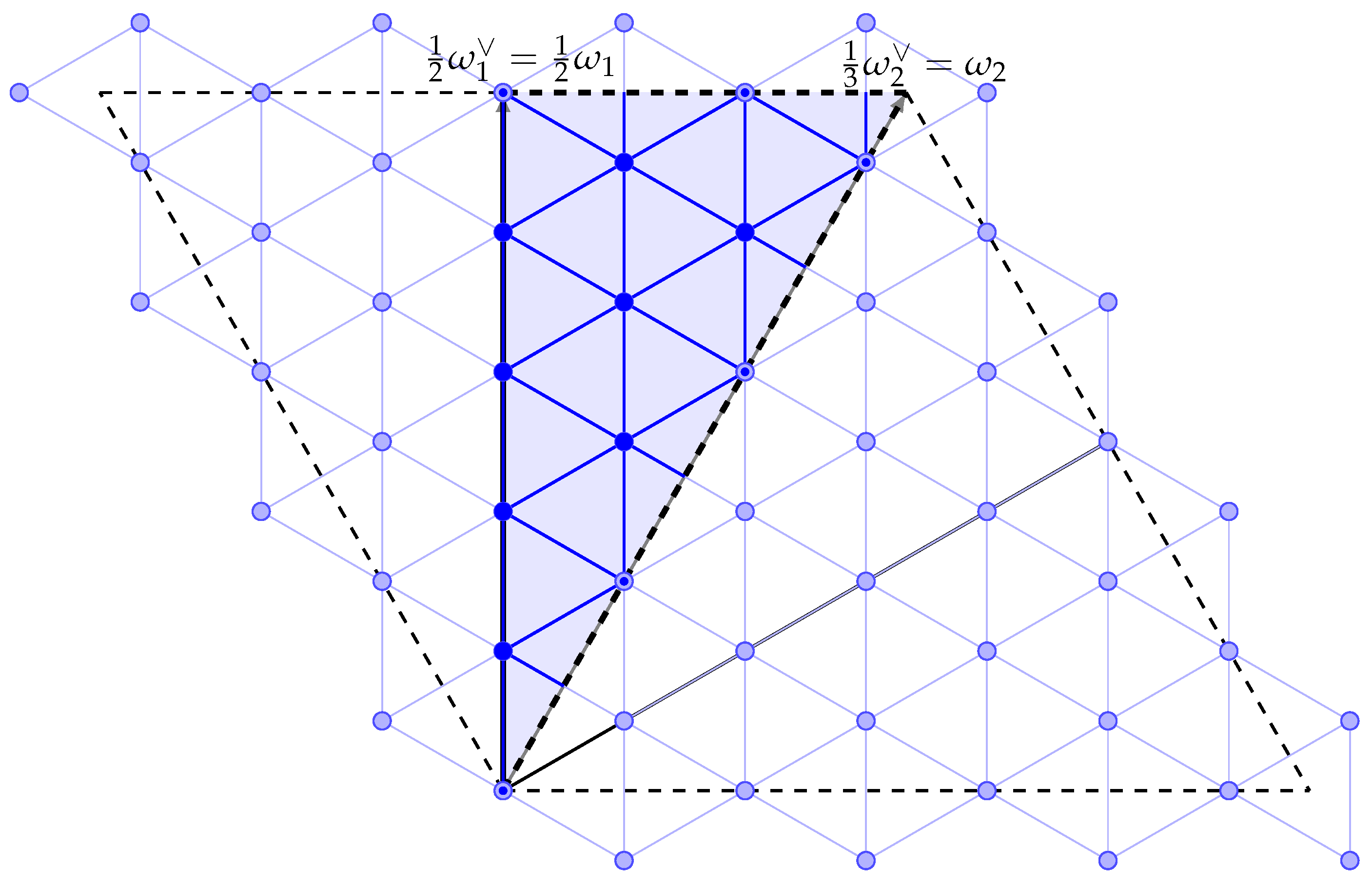
2.2. Signed Fundamental Domains
2.3. Signed Dual Fundamental Domains
2.4. Dual-Weight Discretization of Weyl Orbit Functions
3. Dual Weight Lattice Models
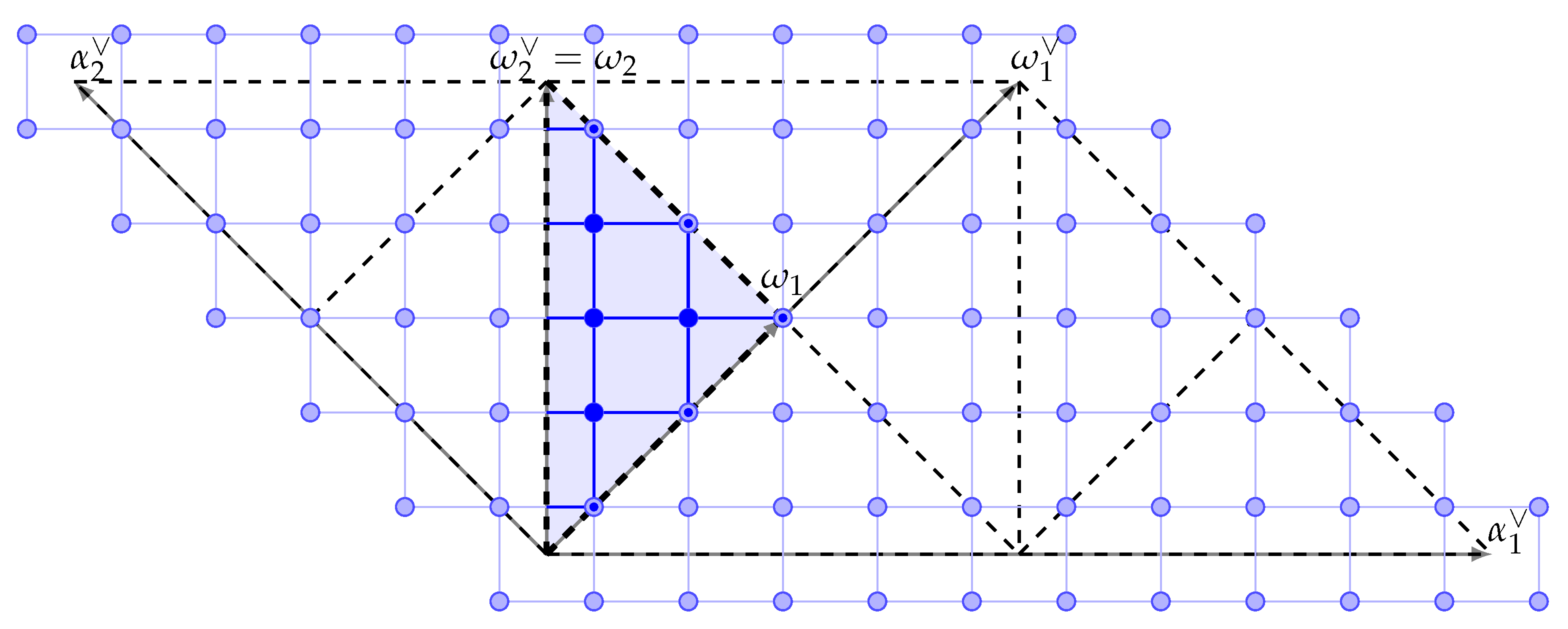
3.1. Dual-Weight Dots
3.2. Schrödinger Equations
3.3. Time Evolution
4. Dual Weight Lattice Models of and
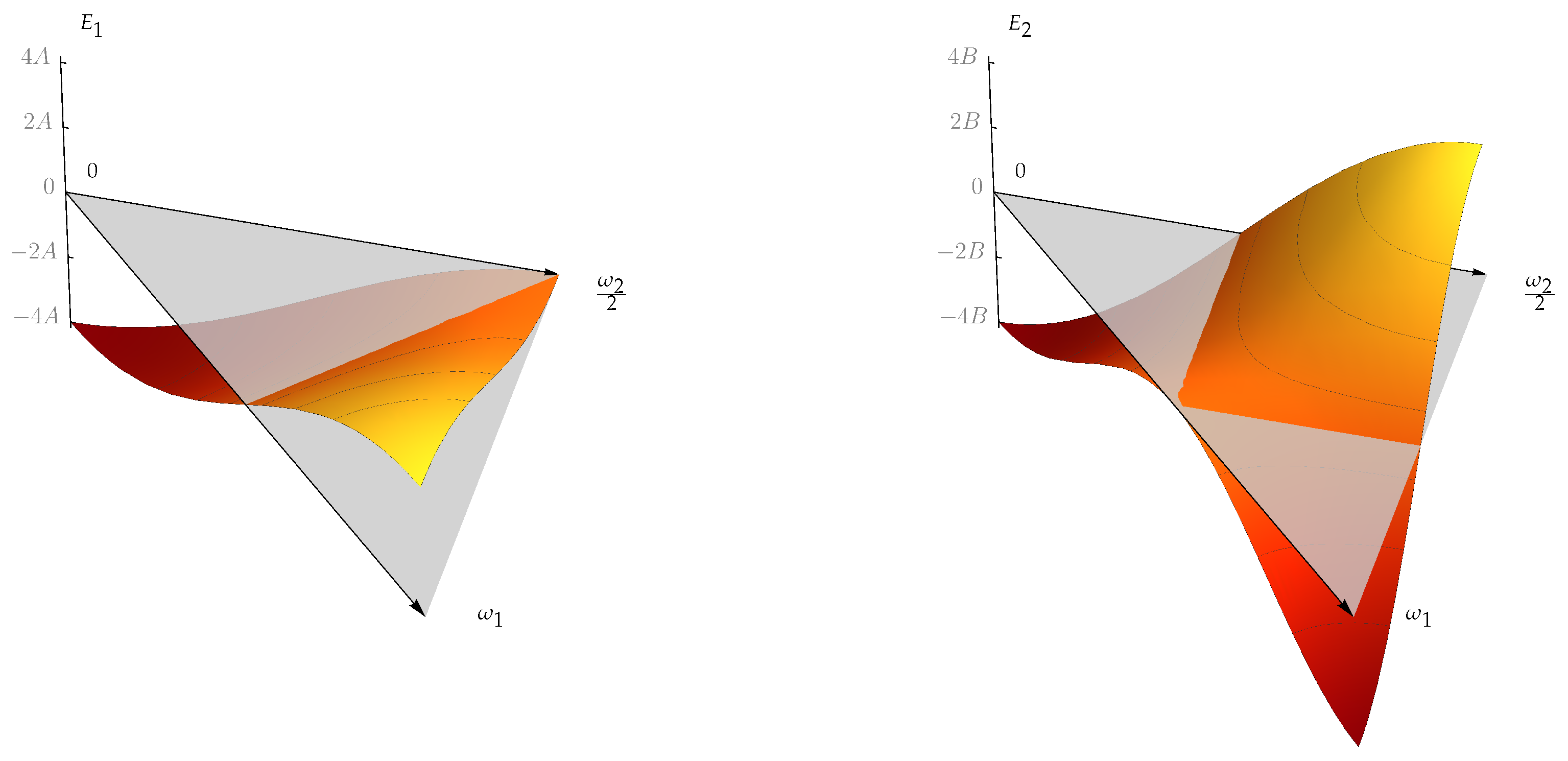
4.1. Case
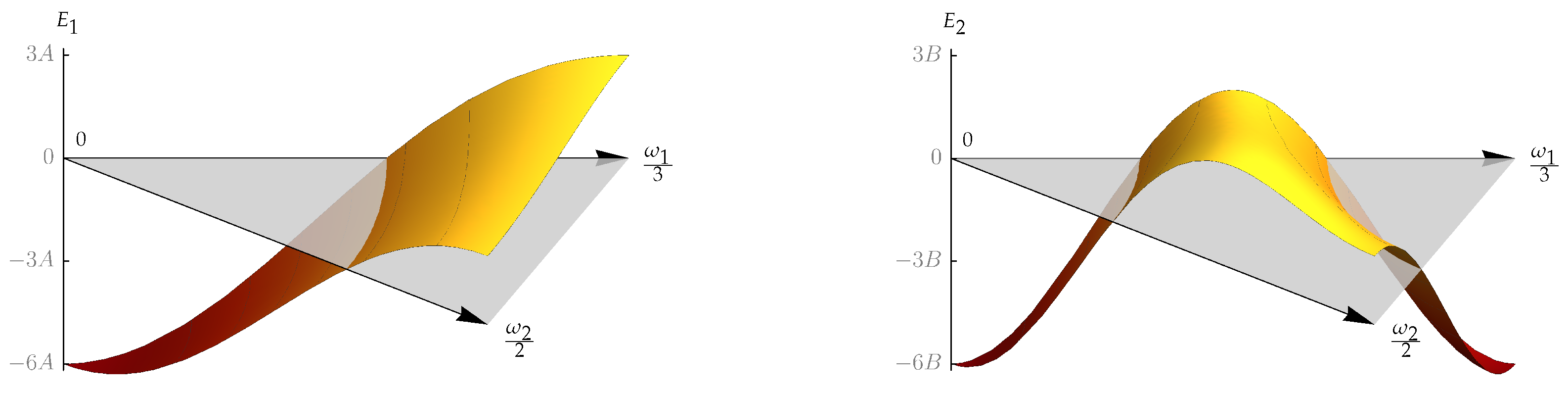
4.2. Case
5. Conclusions
- The developed one-particle dual-weight discrete quantum billiard systems describe the non-relativistic quantum particle propagating on the dots , which comprise finitely-many positions located inside the scaled closure of the Weyl alcove . The precise arrangements (15) and (16) of the Dirichlet and Neumann walls and that realise the quantum trapping of the particle and coincide with the dual-root billiards [1] constitute the boundaries of the simplex . Any predetermined admissible dual-weight hopping function , which encodes the amplitude propagation to the neighbouring positions, directly provides explicit formulas for the eigenenergies of the systems (59) via its Fourier transform by the symmetric Weyl orbit sums over its finite dominant support . The vectors of the orthonormal momentum basis, , , determined independently on the dual-weight hopping function by their explicit form (56) constitute solutions of the time-independent Schrödinger Equation (58). The time evolution of the dual-weight quantum systems from any normalised initial state vector given in the position basis |a〉, is exactly determined (71).
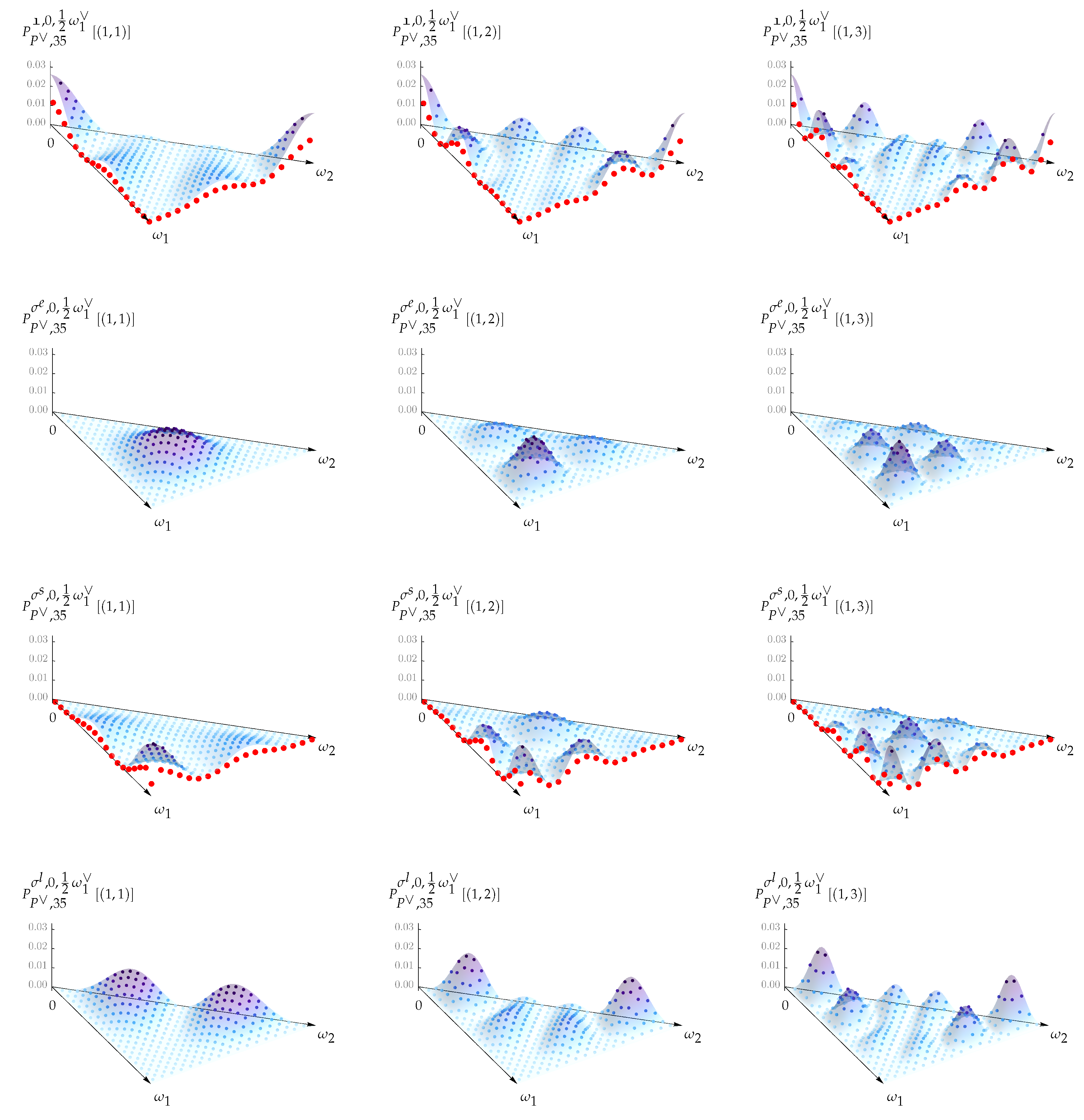
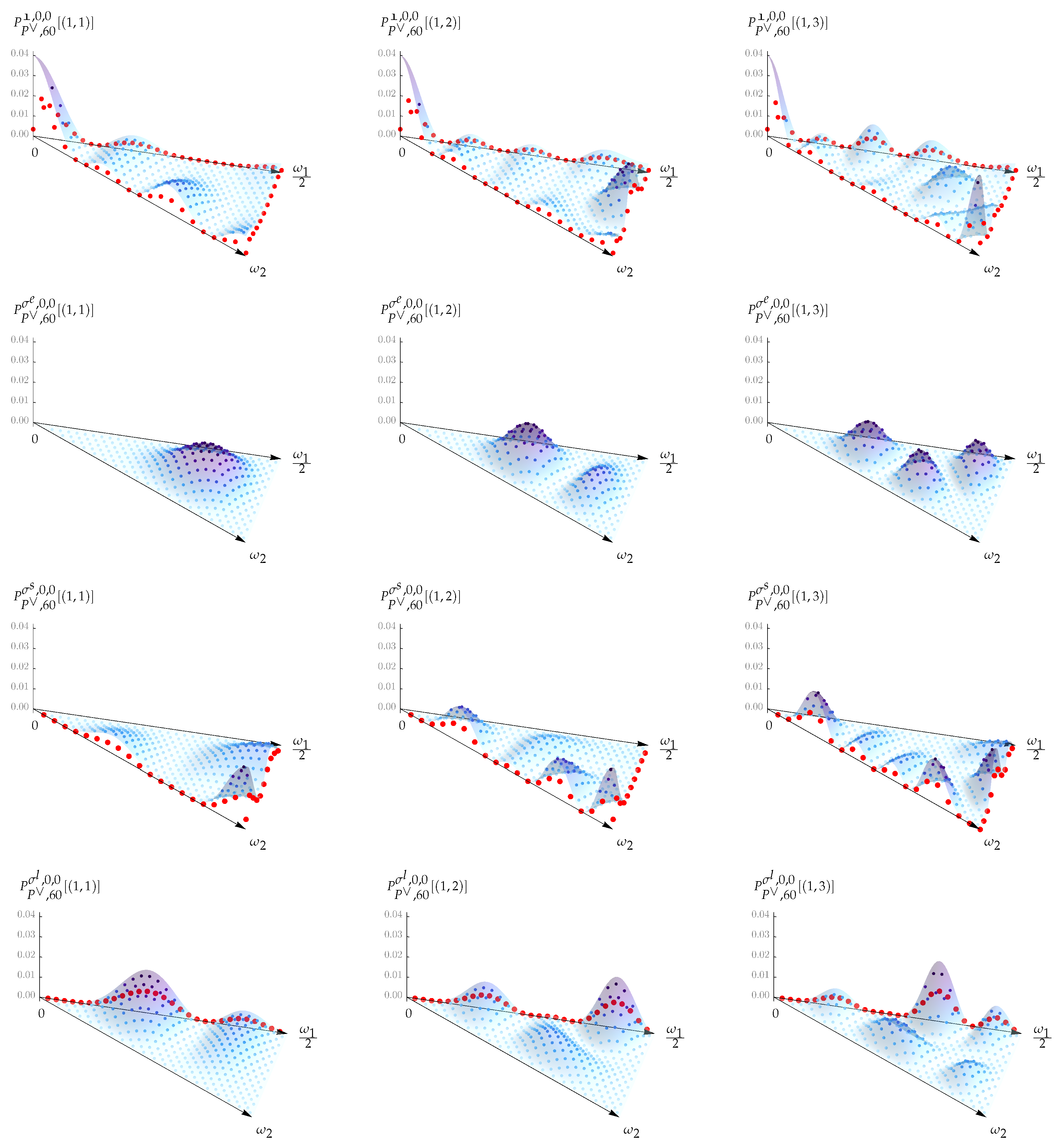
- The presence of the affine Weyl group orbits of the target positions in the coupling sets (51) represent the first essential symmetry component for implementing the interactions enforced by the boundaries of . Secondly, the addition of the sign -function (13) values over the affine-reflected positions in the coupling set counts the number and type of amplitude reflections between the source position and the target position . The -function generalises the sign functions from [40] that are necessary for describing the Galois symmetries of Weyl orbit functions. The square roots of the stabiliser ε-functions (7) present as factors in defining relation of the dual-weight hopping operators matrix elements in the position basis (52) and manifest a direct consequence of the weighted discrete orthogonality relations (39). The ε-function subsequently straightforwardly regulates the probabilities (74) of finding the particle in a stationary state on the Neumann walls of the simplex . The Neumann boundary effect, which is observed similarly in dual-root models [1], is pointedly evident in Figure 3 and Figure 6.
- Considering an electron as the quantum particle propagating in the current discrete quantum systems, a novel class of the tight-binding models [3] with the electron propagating among atoms positioned at the points of the dual-weight dot is obtained. The hopping integrals [15] between the coupled neighbouring positions in the atomic lattice, which might be estimated from theoretical considerations and/or fine-tuned experimentally, directly enter the present models as the values of the dual-weight hopping function . Analogously to the dual-root models, the physical interpretation of the dual-weight models coincides with the inductively developed electron propagation in a crystal lattice [41]. Since the dual-weight Fourier–Weyl transforms of the current one-dimensional model of a linear crystal specialise to the four types I–IV of the discrete cosine and sine transforms [18,25], the current stationary state vectors represent boundary-dependent (anti)symmetric alternatives to the periodic exponential solutions [41]. Moreover, the discrete Hamiltonian approach used for dual-weight and dual-root models produces strictly defined boundary-dependent forms of the energy spectra (59).
- Similarly to the dual-root models, the dual-weight models employ the generalised dual-weight Fourier–Weyl transforms (41) to construct the momentum basis (56) together with the stationary states (70) and time-evolutions (71). The utilisation of the weight lattice transforms [23] as well as the dual-weight E-transforms [42] for the description of analogous discrete quantum systems deserves further study. Potentially resulting in the generalisation of the present models to the prominent honeycomb-type (pseudo)lattices [17,32], the intricate composition of the weight and root lattice transforms demands a specific construction of extension coefficients of the extended Weyl orbit functions [43]. The calculation of the extension coefficients is determined by the desired form of product-to-sum decomposition formulas (33), which characterise the coupling of the considered (pseudo)lattice model. Since the extended Weyl orbit function approach potentially represents alternative description to the (pseudo)spinor wavefunctions approach [17], the Fourier–Weyl transforms induced by the extended Weyl orbit functions, together with the discrete symmetry analysis of the associated quantum systems, deserve further study.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Brus, A.; Hrivnák, J.; Motlochová, L. Quantum particle on dual root lattice in Weyl alcove. J. Phys. A Math. Theor. 2021, 54, 095202. [Google Scholar] [CrossRef]
- Lindsay, D.M.; Wang, Y.; George, T.F. The Hückel model for small metal clusters. IV. Orbital properties and cohesive energies for model clusters of up to several hundred atoms. J. Clust. Sci. 1990, 1, 107–126. [Google Scholar] [CrossRef]
- Manninen, M. Models of Metal Clusters and Quantum Dots. In Atomic Clusters and Nanoparticles; Guet, C., Hobza, P., Speigelman, F., David, F., Eds.; Springer: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Fernández-Hurtado, V.; Mur-Petit, J.; García-Ripoll, J.J.; Molina, R.A. Lattice scars: Surviving in an open discrete billiard. New J. Phys. 2014, 16, 035005. [Google Scholar] [CrossRef]
- Miao, F.; Wijeratne, S.; Zhang, Y.; Coskun, U.C.; Bao, W.; Lau, C.N. Phase-Coherent Transport in Graphene Quantum Billiards. Science 2007, 317, 1530–1533. [Google Scholar] [CrossRef]
- McDonald, S.W.; Kaufman, A.N. Wave chaos in the stadium: Statistical properties of short-wave solutions of the Helmholtz equation. Phys. Rev. A 1988, 37, 3067–3086. [Google Scholar] [CrossRef]
- Howard, P.J.; O’Mahony, P.F. The behaviour of resonances in Hecke triangular billiards under deformation. J. Phys. A Math. Theor. 2007, 40, 9275. [Google Scholar] [CrossRef]
- Spina, M.E.; Saraceno, M. Quantum spectra of triangular billiards on the sphere. J. Phys. A Math. Gen. 2001, 34, 2549. [Google Scholar] [CrossRef][Green Version]
- Martins Quintela, M.F.C.; Lopes dos Santos, J.M.B. A polynomial approach to the spectrum of Dirac–Weyl polygonal Billiards. J. Phys. Condens. Matter 2021, 33, 035901. [Google Scholar] [CrossRef]
- Gaddah, W. A Lie group approach to the Schrödinger equation for a particle in an equilateral triangular infinite well. Eur. J. Phys. 2013, 34, 1175. [Google Scholar] [CrossRef]
- Gaddah, W. Exact solutions to the Dirac equation for equilateral triangular billiard systems. J. Phys. A Math. Theor. 2018, 51, 385304. [Google Scholar] [CrossRef]
- Schachner, H.C.; Obermair, G.M. Quantum billiards in the shape of right triangles. Z. Phys. B 1994, 95, 113–119. [Google Scholar] [CrossRef]
- Mandarino, A.; Linowski, T.; Życzkowski, K. Bipartite unitary gates and billiard dynamics in the Weyl chamber. Phys. Rev. A 2018, 98, 012335. [Google Scholar] [CrossRef]
- Krimer, D.O.; Khomeriki, R. Realization of discrete quantum billiards in a two-dimensional optical lattice. Phys. Rev. A 2011, 84, 041807. [Google Scholar] [CrossRef]
- Güçlü, A.D.; Potasz, P.; Korkusinski, M.; Hawrylak, P. Graphene Quantum Dots; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Hrivnák, J.; Motlochová, L. Graphene Dots via Discretizations of Weyl-Orbit Functions. In Lie Theory and Its Applications in Physics, Proceedings of Lie Theory and Its Applications in Physics, Varna, Bulgaria, June 2019; Dobrev, V., Ed.; Springer: Singapore, 2020; pp. 407–413. [Google Scholar]
- Rozhkov, A.V.; Nori, F. Exact wave functions for an electron on a graphene triangular quantum dot. Phys. Rev. B 2010, 81, 155401. [Google Scholar] [CrossRef]
- Czyżycki, T.; Hrivnák, J. Generalized discrete orbit function transforms of affine Weyl groups. J. Math. Phys. 2014, 55, 113508. [Google Scholar] [CrossRef]
- Hrivnák, J.; Motlochová, L.; Patera, J. On discretization of tori of compact simple Lie groups II. J. Phys. A 2012, 45, 255201. [Google Scholar] [CrossRef]
- Hrivnák, J.; Patera, J. On discretization of tori of compact simple Lie groups. J. Phys. A Math. Theor. 2009, 42, 385208. [Google Scholar] [CrossRef]
- Czyżycki, T.; Hrivnák, J.; Motlochová, L. Generalized Dual-Root Lattice Transforms of Affine Weyl Groups. Symmetry 2020, 12, 1018. [Google Scholar] [CrossRef]
- Hrivnák, J.; Motlochová, L. Dual-root lattice discretization of Weyl orbit functions. J. Fourier Anal. Appl. 2019, 25, 2521–2569. [Google Scholar] [CrossRef]
- Hrivnák, J.; Walton, M.A. Weight-Lattice Discretization of Weyl-Orbit Functions. J. Math. Phys. 2016, 57, 083512. [Google Scholar] [CrossRef]
- Li, H.; Xu, Y. Discrete Fourier analysis on fundamental domain and simplex of Ad lattice in d-variables. J. Fourier Anal. Appl. 2010, 16, 383–433. [Google Scholar] [CrossRef]
- Britanak, V.; Rao, K.; Yip, P. Discrete Cosine and Sine Transforms: General Properties, Fast Algorithms and Integer Approximations; Elsevier/Academic Press: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Humphreys, J.E. Reflection Groups and Coxeter Groups; Cambridge Studies in Advanced Mathematics 29; Cambridge University Press: Cambridge, UK, 1990. [Google Scholar]
- Moody, R.V.; Motlochová, L.; Patera, J. Gaussian cubature arising from hybrid characters of simple Lie groups. J. Fourier Anal. Appl. 2014, 20, 1257–1290. [Google Scholar] [CrossRef]
- Klimyk, A.U.; Patera, J. Orbit functions. SIGMA 2006, 2, 006. [Google Scholar]
- Montangero, S.; Frustaglia, D.; Calarco, T.; Fazio, R. Quantum billiards in optical lattices. Europhys. Lett. 2009, 88, 30006. [Google Scholar] [CrossRef]
- Alhassid, Y. The statistical theory of quantum dots. Rev. Mod. Phys. 2000, 72, 895–968. [Google Scholar] [CrossRef]
- Mounet, N.; Gibertini, M.; Schwaller, P.; Campi, D.; Merkys, A.; Marrazzo, A.; Sohier, T.; Castelli, I.E.; Cepellotti, A.; Pizzi, G.; et al. Two-dimensional materials from high-throughput computational exfoliation of experimentally known compounds. Nature Nanotech. 2018, 13, 246–252. [Google Scholar] [CrossRef]
- Drissi, L.B.; Saidi, E.H.; Bousmina, M. Graphene, Lattice Field Theory and Symmetries. J. Math. Phys. 2011, 52, 022306. [Google Scholar] [CrossRef]
- Bloch, I.; Dalibard, J.; Nascimbène, S. Quantum simulations with ultracold quantum gases. Nat. Phys. 2012, 8, 267–276. [Google Scholar] [CrossRef]
- Harshman, N.L.; Olshanii, M.; Dehkharghani, A.S.; Volosniev, A.G.; Jackson, S.G.; Zinner, N.T. Integrable Families of Hard-Core Particles with Unequal Masses in a One-Dimensional Harmonic Trap. Phys. Rev. X 2017, 7, 041001. [Google Scholar]
- Tolar, J.; Chadzitaskos, G. Feynman’s path integral and mutually unbiased bases. J. Phys. A Math. Theor. 2009, 42, 245306. [Google Scholar] [CrossRef]
- Politi, A.; Cryan, M.J.; Rarity, J.G.; Yu, S.; O’Brien, J.L. Silica-on-Silicon Waveguide Quantum Circuits. Science 2008, 320, 646–649. [Google Scholar] [CrossRef] [PubMed]
- Bourbaki, N. Groupes et Algèbres de Lie, Chapiters IV, V, VI; Hermann: Paris, France, 1968. [Google Scholar]
- Vinberg, E.B.; Onishchik, A.L. Lie Groups and Lie Algebras; Springer: New York, NY, USA, 1994. [Google Scholar]
- Klimyk, A.U.; Patera, J. Antisymmetric orbit functions. SIGMA 2007, 3, 023. [Google Scholar] [CrossRef]
- Hrivnák, J.; Walton, M.A. Discretized Weyl-orbit functions: Modified multiplication and Galois symmetry. J. Phys. A Math. Theor. 2015, 48, 175205. [Google Scholar] [CrossRef][Green Version]
- Feynman, R.P.; Leighton, R.B.; Sand, M. The Feynman Lectures on Physics: Volume III; Basic Books: New York, NY, USA, 2010. [Google Scholar]
- Hrivnák, J.; Juránek, M. On E-Discretization of Tori of Compact Simple Lie Groups. II. J. Math. Phys. 2017, 58, 103504. [Google Scholar] [CrossRef]
- Hrivnák, J.; Motlochová, L. Discrete cosine and sine transforms generalized to honeycomb lattice. J. Math. Phys. 2018, 59, 063503. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brus, A.; Hrivnák, J.; Motlochová, L. Quantum Particle on Dual Weight Lattice in Weyl Alcove. Symmetry 2021, 13, 1338. https://doi.org/10.3390/sym13081338
Brus A, Hrivnák J, Motlochová L. Quantum Particle on Dual Weight Lattice in Weyl Alcove. Symmetry. 2021; 13(8):1338. https://doi.org/10.3390/sym13081338
Chicago/Turabian StyleBrus, Adam, Jiří Hrivnák, and Lenka Motlochová. 2021. "Quantum Particle on Dual Weight Lattice in Weyl Alcove" Symmetry 13, no. 8: 1338. https://doi.org/10.3390/sym13081338
APA StyleBrus, A., Hrivnák, J., & Motlochová, L. (2021). Quantum Particle on Dual Weight Lattice in Weyl Alcove. Symmetry, 13(8), 1338. https://doi.org/10.3390/sym13081338





