A Generalized Rayleigh Family of Distributions Based on the Modified Slash Model
Abstract
:1. Introduction
2. The New Model
2.1. Stochastic Representation
- Let . Then, T can be expressed as , where and are independent.
- If then T follows a canonic modified slashed generalized Rayleigh (CMSGR) distribution, and we can write , where and are independent. This is denoted as .
- Generate .
- Generate .
- Compute .
2.2. Probability Density Function
2.3. Reliability Analysis
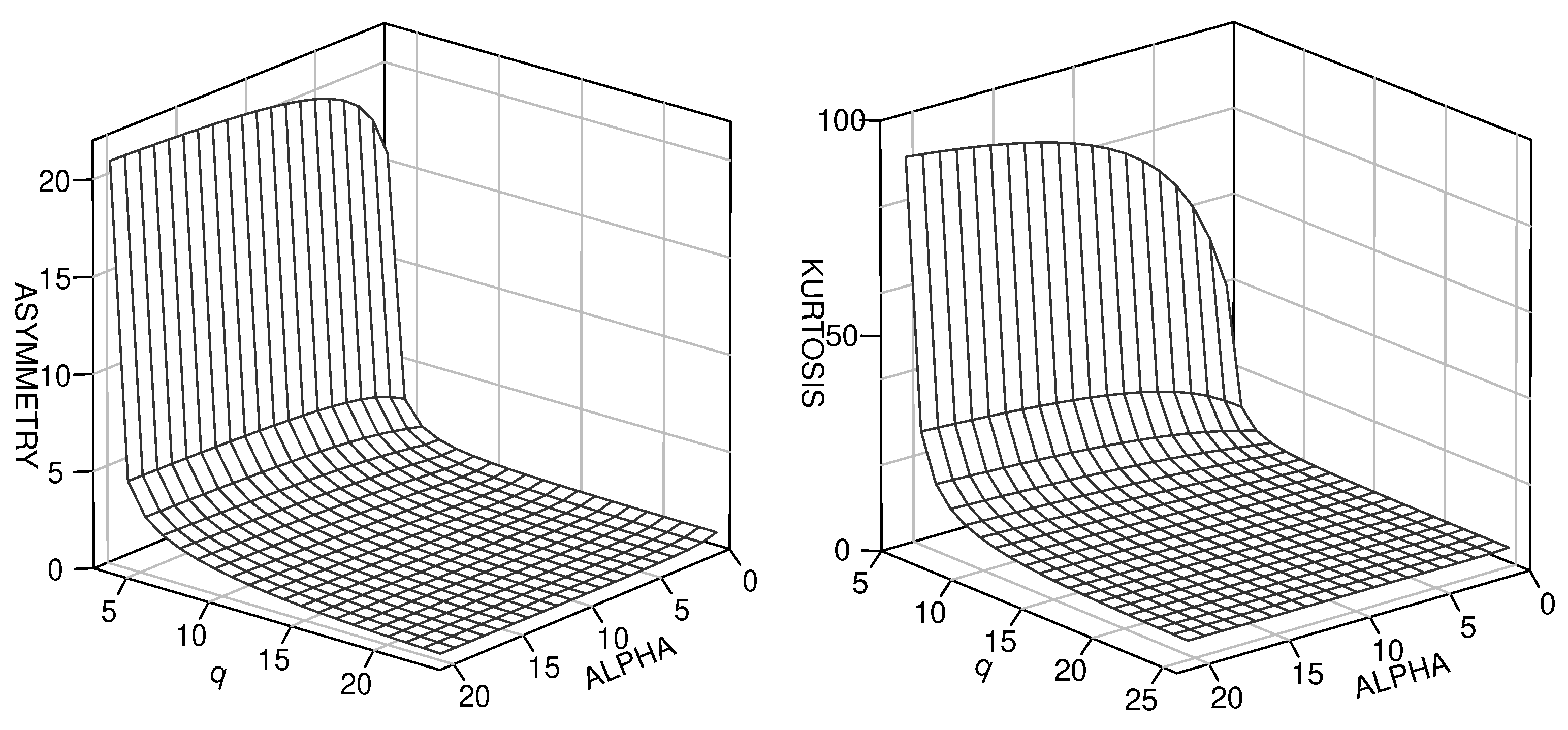
2.4. Moments
3. The MSGR Family of Distributions
4. Parameter Estimation
4.1. Method of Moments Estimators
4.2. Maximum Likelihood Estimation
4.3. Maximum Likelihood Estimation via the EM Algorithm
4.4. Simulation Study
5. Illustrations
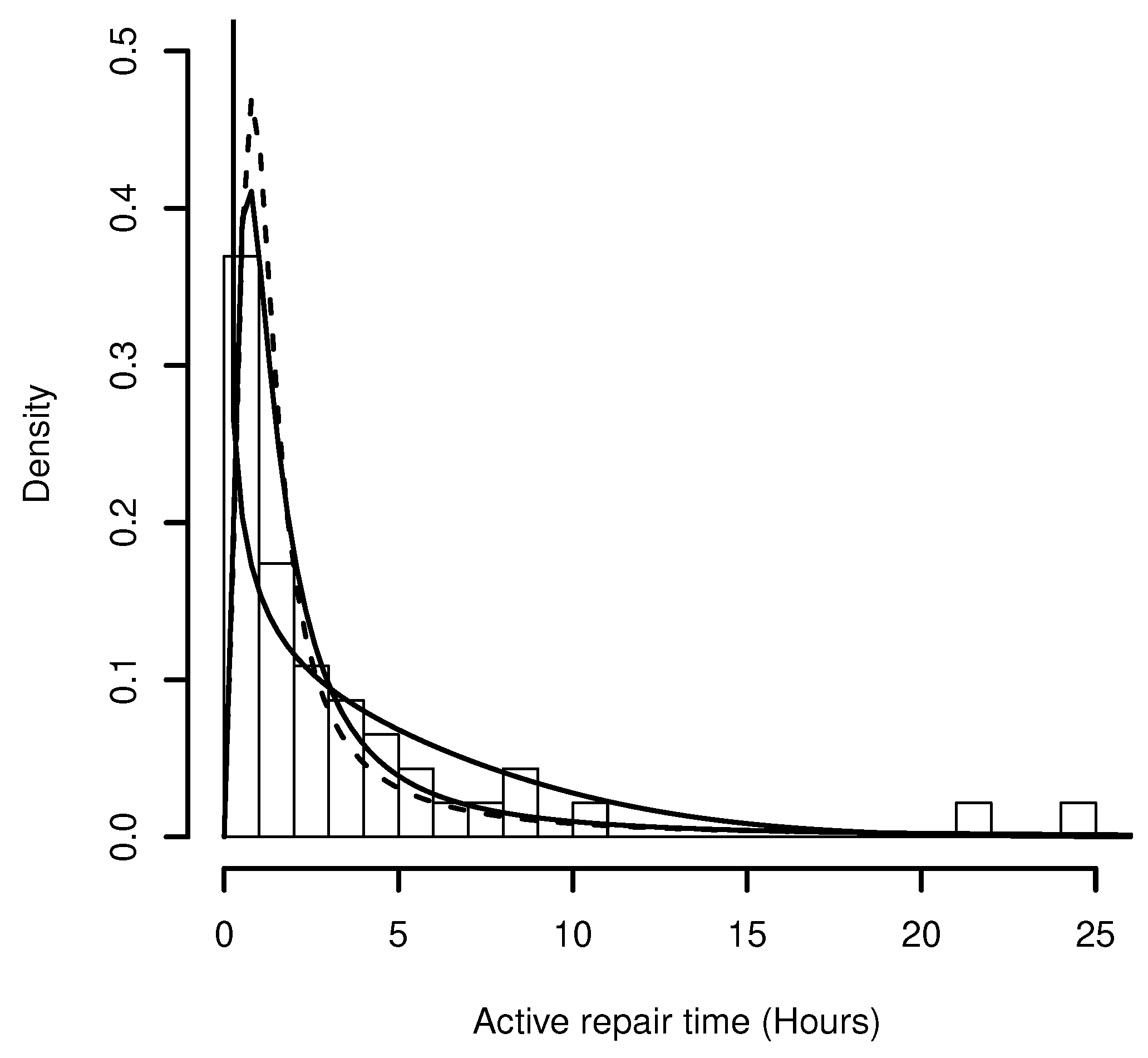
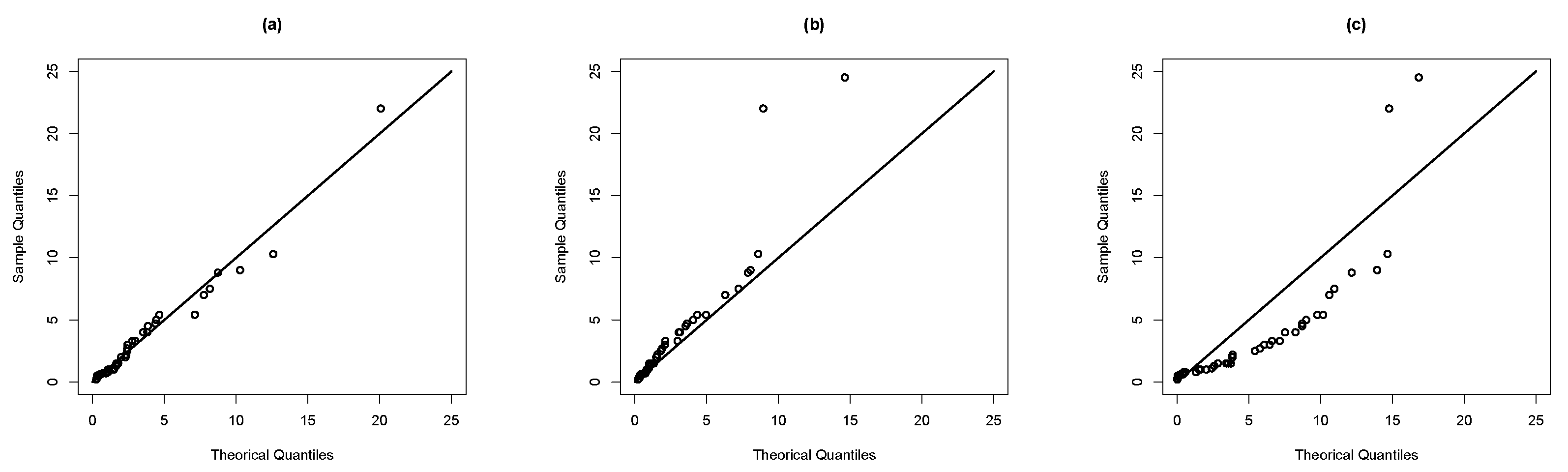
5.1. Active Repair Time Data
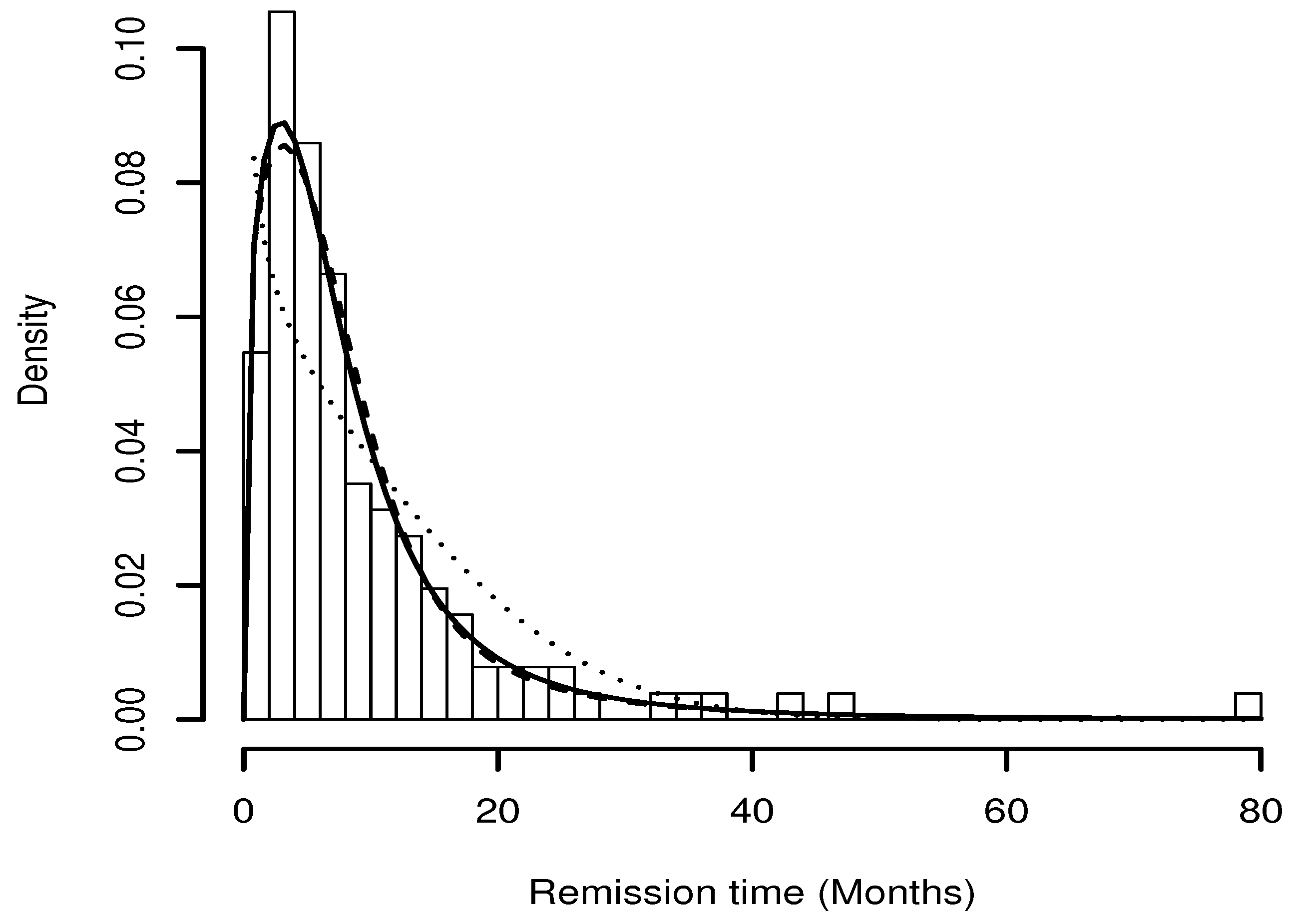
5.2. Remission Time Data
6. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Vodă, V.G. Inferential procedures on a generalized Rayleigh variate I. Apl. Mat. 1976, 21, 395–412. [Google Scholar]
- Siddiqui, M.M. Some problems connected with Rayleigh distributions. J. Res. Natl. Bur. Stand. Sect. D Radio Propag. 1962, 66, 167–174. [Google Scholar] [CrossRef]
- Hirano, K. Rayleigh distribution. In Encyclopedia of Statistical Sciences; Kotz, S., Johnson, N.L., Read, C.B., Eds.; Wiley: New York, NY, USA, 1986; pp. 647–649. [Google Scholar]
- Abramowitz, M.; Stegun, I.E. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th ed.; National Bureau of Standars: Washington, DC, USA, 1970.
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products; Academic Press: San Diego, CA, USA, 2000. [Google Scholar]
- Gómez, H.W.; Quintana, F.A.; Torres, F.J. A new family of slash-distributions with elliptical contours. Stat. Probab. Lett. 2007, 77, 717–725. [Google Scholar] [CrossRef]
- Reyes, J.; Barranco-Chamorro, I.; Gómez, H.W. Generalized modified slash distribution with applications. Commun. Stat. Theory Methods 2020, 49, 2025–2048. [Google Scholar] [CrossRef]
- Iriarte, Y.A.; Vilca, F.; Varela, H.; Gómez, H.W. Slashed generalized Rayleigh distribution. Commun. Stat. Theory Methods 2017, 46, 4686–4699. [Google Scholar] [CrossRef]
- Olmos, N.M.; Varela, H.; Gómez, H.W.; Bolfarine, H. An extension of the half-normal distribution. Stat. Pap. 2012, 53, 875–886. [Google Scholar] [CrossRef]
- Reyes, J.; Gómez, H.W.; Bolfarine, H. Modified slash distribution. Statistics 2013, 47, 929–941. [Google Scholar] [CrossRef]
- Stacy, E. A generalization of the gamma distribution. Ann. Math. Stat. 1962, 33, 1187–1192. [Google Scholar] [CrossRef]
- Rahman, M.S.; Gupta, R.P. Family of transformed chi-square distributions. Commun. Stat. Theory Methods 1992, 22, 135–146. [Google Scholar] [CrossRef]
- Hwang, L.C. Asymptotic Non-Deficiency of Some Procedures for a Particular Exponential Family Under Relative LINEX Loss. Seq. Anal. 2014, 33, 345–359. [Google Scholar] [CrossRef]
- Kudryavtsev, A.; Shestakov, O. Asymptotically normal estimators for the parameters of the gamma-exponential distribution. Mathematics 2021, 9, 273. [Google Scholar] [CrossRef]
- Reyes, J.; Barranco-Chamorro, I.; Gallardo, D.I.; Gómez, H.W. Generalized Modified Slash Birnbaum—Saunders Distribution. Symmetry 2018, 10, 724. [Google Scholar] [CrossRef] [Green Version]
- Zörnig, P. On Generalized Slash Distributions: Representation by Hypergeometric Functions. Stats 2019, 2, 26. [Google Scholar] [CrossRef] [Green Version]
- Prudnikov, A.; Brychkov, Y.A.; Marichev, O.I. Integrals and Series, Vols. 1, 2 and 3; Gordon and Breach Science Publishers: Amsterdam, The Netherlands, 1986. [Google Scholar]
- Casella, G.; Berger, R.L. Statistical Inference, 2nd ed.; Duxbury Advanced Series; Duxbury-Thomson Learning: Pacific Grove, CA, USA, 2002. [Google Scholar]
- Polovko, A. Fundamentals of Reliability Theory; Academic Press: San Diego, CA, USA, 1968. [Google Scholar]
- Lawless, J.F. Statistical Models and Methods for Lifetime Data, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2003. [Google Scholar]
- Sarti, A.; Corsi, C.; Mazzini, E.; Lamberti, C. Maximum likelihood segmentation of ultrasound images with Rayleigh distribution. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2005, 52, 947–960. [Google Scholar] [CrossRef] [Green Version]
- Kalaiselvi, S.; Loganathan, A.; Vijayaraghavan, R. Bayesian Reliability Sampling Plans under the Conditions of Rayleigh-Inverse-Rayleigh Distribution. Econ. Qual. Control 2014, 29, 29–38. [Google Scholar] [CrossRef]
- Dhaundiyal, A.; Singh, S. Approximations to the Non-Isothermal Distributed Activation Energy Model for Biomass Pyrolysis Using the Rayleigh Distribution. Cta Technol. Agric. 2017, 20, 78–84. [Google Scholar] [CrossRef] [Green Version]
- Kuruoglu, E.; Zerubia, J. Modeling SAR Images with a Generalization of the Rayleigh Distribution. IEEE Trans. Image Process. 2004, 13, 527–533. [Google Scholar] [CrossRef]
- Barranco-Chamorro, I.; Moreno-Rebollo, J.L.; Pascual-Acosta, A.; Enguix-González, A. An Overview of Asymptotic Properties of Estimators in Truncated Distributions. Commun. Stat. Theory Methods 2007, 36, 2351–2366. [Google Scholar] [CrossRef]
- Lehmann, E. Elements of Large-Sample Theory; Springer: New York, NY, USA, 1999. [Google Scholar]
- Iriarte, Y.A.; Castillo, N.O.; Bolfarine, H.; Gómez, H.W. Modified slashed-Rayleigh distribution. Commun. Stat. Theory Methods 2018, 47, 3220–3233. [Google Scholar] [CrossRef]
- Olmos, N.M.; Venegas, O.; Gómez, Y.M.; Iriarte, Y.A. An Asymmetric Distribution with Heavy Tails and Its Expectation-Maximization (EM) Algorithm Implementation. Symmetry 2019, 11, 1150. [Google Scholar] [CrossRef] [Green Version]
- Hogg, R.; Tanis, E. Probability and Statistical Inference; MacMillan Publishing: New York, NY, USA, 1993. [Google Scholar]
- Bekker, A.; Roux, J. Reliability characteristics of the Maxwell distribution: A Bayes estimation study. Commun. Stat. Theory Methods 2005, 34, 2169–2178. [Google Scholar] [CrossRef]
- Johnson, N.L.; Kotz, S.; Balakrishnan, N. Continuous Univariate Distributions, 2nd ed.; John Wiley & Sons Inc.: New York, NY, USA, 1995; Volume 2. [Google Scholar]
- Dempster, A.; Laird, N.; Rubim, D. Maximum likelihood from incomplete data via the EM algorithm (with discussion). J. R. Stat. Soc. Ser. B 1977, 39, 1–38. [Google Scholar]
- R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2021. [Google Scholar]
- Chhikara, R.; Folks, J. The inverse Gaussian distribution as a lifetime model. Technometrics 1977, 19, 461–468. [Google Scholar] [CrossRef]
- Akaike, H. A new look at the statistical model identification. IEE Trans. Autom. Control 1974, 19, 716–723. [Google Scholar] [CrossRef]
- Schwarz, G. Estimating the dimension of a model. Ann. Stat. 1978, 6, 461–464. [Google Scholar] [CrossRef]
- Lee, E.T.; Wang, J. Statistical Methods for Survival Data Analysis; John Wiley & Sons: Hoboken, NJ, USA, 2003. [Google Scholar]





| q | (SD) | (SD) | (SD) | |||
|---|---|---|---|---|---|---|
| 1 | −0.5 | 2 | 1.249 (0.897) | −0.459 (0.233) | 2.586 (1.101) | |
| 1 | −0.5 | 3 | 1.183 (0.674) | −0.435 (0.171) | 3.442 (1.615) | |
| 1 | −0.5 | 4 | 1.117 (0.543) | −0.468 (0.132) | 4.275 (1.568) | |
| 1 | −0.5 | 3 | 1.172 (0.830) | 0.597 (0.517) | 3.137 (1.471) | |
| 1 | 0.0 | 3 | 1.182 (0.842) | 0.099 (0.274) | 3.116 (0.977) | |
| 1 | 3.0 | 3 | 1.245 (0.897) | 3.208 (1.811) | 3.258 (0.793) | |
| 1 | 2.0 | 3 | 1.296 (1.098) | 2.269 (1.607) | 3.369 (1.816) | |
| 2 | 2.0 | 3 | 2.433 (1.659) | 2.240 (1.356) | 3.197 (0.918) | |
| 3 | 2.0 | 3 | 3.212 (2.365) | 2.084 (1.206) | 3.247 (0.861) | |
| 1 | −0.5 | 2 | 1.223 (0.888) | −0.472 (0.105) | 2.151 (0.662) | |
| 1 | −0.5 | 3 | 1.137 (0.551) | −0.478 (0.082) | 3.225 (1.021) | |
| 1 | −0.5 | 4 | 1.093 (0.497) | −0.481 (0.074) | 4.095 (1.261) | |
| 1 | −0.5 | 3 | 1.096 (0.498) | 0.591 (0.401) | 3.135 (0.666) | |
| 1 | 0.0 | 3 | 1.131 (0.539) | 0.057 (0.252) | 3.064 (0.672) | |
| 1 | 3.0 | 3 | 1.239 (0.821) | 2.828 (0.998) | 3.138 (0.555) | |
| 1 | 2.0 | 3 | 1.276 (0.821) | 2.250 (1.115) | 3.117 (0.566) | |
| 2 | 2.0 | 3 | 2.217 (0.957) | 2.237 (0.990) | 3.107 (0.598) | |
| 3 | 2.0 | 3 | 2.848 (0.923) | 2.068 (0.841) | 3.135 (0.617) | |
| 1 | −0.5 | 2 | 1.127 (0.562) | −0.475 (0.096) | 2.075 (0.451) | |
| 1 | −0.5 | 3 | 1.072 (0.467) | −0.497 (0.062) | 3.018 (0.736) | |
| 1 | −0.5 | 4 | 1.016 (0.387) | −0.501 (0.055) | 4.051 (0.742) | |
| 1 | −0.5 | 3 | 1.044 (0.388) | 0.504 (0.301) | 3.070 (0.598) | |
| 1 | 0.0 | 3 | 1.010 (0.376) | 0.010 (0.168) | 3.046 (0.585) | |
| 1 | 3.0 | 3 | 0.994 (0.443) | 2.944 (0.890) | 3.080 (0.481) | |
| 1 | 2.0 | 3 | 1.144 (0.643) | 2.156 (0.514) | 3.078 (0.462) | |
| 2 | 2.0 | 3 | 2.036 (0.792) | 2.015 (0.764) | 3.081 (0.498) | |
| 3 | 2.0 | 3 | 3.087 (0.746) | 2.061 (0.680) | 3.092 (0.511) | |
| n | ||||
|---|---|---|---|---|
| 46 | 3.606 | 24.445 | 2.794 | 11.294 |
| Parmeters | GR (s.e) | SGR (s.e) | MSGR (s.e) |
|---|---|---|---|
| 0.008 (0.002) | 2.121 (2.316) | 2.772 (0.132) | |
| −0.696 (0.049) | 0.241 (0.640) | 0.731 (0.091) | |
| - | 0.859 (0.202) | 1.182 (0.080) | |
| Log-likelihood | 111.041 | 101.888 | 100.292 |
| AIC | 226.083 | 209.776 | 206.585 |
| BIC | 229.740 | 215.262 | 212.071 |
| KS | 0.173 | 0.152 | 0.108 |
| n | ||||
|---|---|---|---|---|
| 128 | 9.365 | 110.425 | 3.286 | 18.483 |
| Parameters | GR (s.e) | SGR (s.e) | MSGR (s.e) |
|---|---|---|---|
| 0.002 (0.0002) | 0.019 (0.007) | 0.023 (0.009) | |
| −0.610 (0.035) | −0.390 (0.086) | −0.355 (0.100) | |
| - | 1.839 (0.381) | 2.213 (0.402) | |
| Log-likelihood | −426.824 | −410.183 | −409.695 |
| AIC | 859.648 | 826.366 | 825.391 |
| BIC | 868.204 | 834.922 | 833.947 |
| KS | 0.156 | 0.070 | 0.062 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barranco-Chamorro, I.; Iriarte, Y.A.; Gómez, Y.M.; Astorga, J.M.; Gómez, H.W. A Generalized Rayleigh Family of Distributions Based on the Modified Slash Model. Symmetry 2021, 13, 1226. https://doi.org/10.3390/sym13071226
Barranco-Chamorro I, Iriarte YA, Gómez YM, Astorga JM, Gómez HW. A Generalized Rayleigh Family of Distributions Based on the Modified Slash Model. Symmetry. 2021; 13(7):1226. https://doi.org/10.3390/sym13071226
Chicago/Turabian StyleBarranco-Chamorro, Inmaculada, Yuri A. Iriarte, Yolanda M. Gómez, Juan M. Astorga, and Héctor W. Gómez. 2021. "A Generalized Rayleigh Family of Distributions Based on the Modified Slash Model" Symmetry 13, no. 7: 1226. https://doi.org/10.3390/sym13071226
APA StyleBarranco-Chamorro, I., Iriarte, Y. A., Gómez, Y. M., Astorga, J. M., & Gómez, H. W. (2021). A Generalized Rayleigh Family of Distributions Based on the Modified Slash Model. Symmetry, 13(7), 1226. https://doi.org/10.3390/sym13071226








