Relation between Quantum Walks with Tails and Quantum Walks with Sinks on Finite Graphs

Abstract

We connect the Grover walk with sinks to the Grover walk with tails. The survival probability of the Grover walk with sinks in the long time limit is characterized by the centered generalized eigenspace of the Grover walk with tails. The centered eigenspace of the Grover walk is the attractor eigenspace of the Grover walk with sinks. It is described by the persistent eigenspace of the underlying random walk whose support has no overlap to the boundaries of the graph and combinatorial flow in graph theory.

1. Introduction

A simple random walker on a finite and connected graph starting from any vertex hits an arbitrary vertex in a finite time. This fact implies that, if we consider a subset of the vertices of this graph as sinks, where the random walker is absorbed, then the survival probability of the random walk in the long time limit converges to zero. However, for quantum walks (QW) [1], the situation is more complicated and the survival probability depends in general on the graph, coin operator, and the initial state of the walk. For a two-state quantum walk on a finite line with sinks on both ends and a non-trivial coin, the survival probability is also zero, as shown by the studies of the corresponding absorption problem [2,3,4,5]. However, for a three-state quantum walk with the Grover coin [6], the survival probability on a finite line is non-vanishing [7] due to the existence of trapped states. These are the eigenstates of the unitary evolution operator which do not have a support on the sinks. Trapped states crucially affect the efficiency of quantum transport [8] and lead to counter-intuitive effects, e.g., the transport efficiency can be improved by increasing the distance between the initial vertex and the sink [9,10]. We find a similar phenomena to this quantum walk model in the experiment on the energy transfer of the dressed photon [11] through the nanoparticles distributed in a finite three-dimensional grid [12]. The output signal intensity increases when the depth direction is larger. Although, when the depth is deeper, a lot of “detours" newly appear to reach to the position of the output from the classical point of view, the output signal intensity of the dressed photon becomes stronger. The existence of trapped states also results in infinite hitting times [13,14].

In this paper, we analyze such counter-intuitive phenomena for the Grover walk on a general connected graph using spectral analysis. The Grover walk is an induced quantum walk of the random walk from the viewpoint of the spectral mapping theorem [15].

To this end, first, we connect the Grover walk with sinks to the Grover walk with tails. The tails are the semi-infinite paths attached to a finite and connected graph. We call the set of vertices connecting to the tails the boundary. The Grover walk with tail was introduced by [16,17] in terms of the scattering theory. If we set some appropriate bounded initial state so that the support is included in the tail, the existence of the fixed point of the dynamical system induced by the Grover walk with tails is shown, and the stable generalized eigenspace ${\mathcal{H}}_s$, in which the dynamical system lives, is orthogonal to the centered generalized eigenspace ${\mathcal{H}}_c$ [18] at every time step [19]. The centered generalized eigenspace is generated by the generalized eigenvectors of the principal submatrix of the time evolution operator of the Grover walk with respect to the internal graph, and all the corresponding absolute values of the eigenvalues are 1. This eigenstate is equivalent to the attractor space [8] of the Grover walk with sink. Indeed, we show that the stationary state of the Grover walk with sink is attracted to this centered generalized eigenstate. Secondly, we characterize this centered generalized eigenspace using the persistent eigenspace of the underlying random walk whose supports have no overlaps to the boundary, also using the concept of “flow” from graph theory. From this result, we see that the existence of the persistent eigenspace of the underlying random walk significantly influences the asymptotic behavior of the corresponding Grover walk, although it has little effect on the asymptotic behavior of the random walk itself. Moreover, we clarify that the graph structure which constructs the symmetric or anti-symmetric flow satisfying the Kirchhoff’s law contributes to the non-zero survival probability of the Grover walk, as suggested in [8,15].

This paper is organized as follows. In Section 2, we prepare the notations of graphs and give the definition of the Grover walk and the boundary operators which are related to the chain. In Section 3, we give the definition of the Grover walk on a graph with sinks. In Section 4, a necessary and sufficient condition for the surviving of the Grover walk is described. In Section 5, we give an example. Section 6 is devoted to the relation between the Grover walk with sink and the Grover walk with tail. In Section 7, we partially characterize the centered generalized eigenspace using the concept of flow from graph theory.

2. Preliminary

2.1. Graph Notation

Let $G=(V,A)$ be a connected and symmetric digraph such that an arc $a\in A$ if and only if its inverse arc $\overline{a}\in A$. The origin and terminal vertices of $a\in A$ are denoted by $o\left(a\right)\in V$ and $t\left(a\right)\in V$, respectively. Assume that G has no multiple arcs. If $t\left(a\right)=o\left(a\right)$, we call such an arc a the self-loop. In this paper, we regard $\overline{a}=a$ for any self-loops. We denote $A_{\sigma }$ as the set of all self-loops. The degree of $v\in V$ is defined by

$$deg\left(v\right)=\left|\right\{a\in A\left|t\right(a)=v\}|.$$

The support edge of $a\in A\setminus A_{\sigma }$ is denoted by $\left|a\right|$ with $\left|a\right|=|\overline{a}|$. The set of (non-directed) edges is

$$E=\left\{\right|a\left|\right|a\in A\setminus A_{\sigma }\}.$$

A walk in G is a sequence of arcs such that $p=(a_0,a_1,\cdots ,a_{r-1})$ with $t\left(a_j\right)=o\left(a_{j+1}\right)$ for any $j=0,\cdots ,r-2$, which may have the same arcs in p. The cycle in G is a subgraph of G which is isomorphic to a sequence of arcs $(a_0,a_1,\cdots ,a_{r-1})$ ($r\ge 3$) satisfying $t\left(a_j\right)=o\left(a_{j+1}\right)$ with $a_j\ne {\overline{a}}_{j+1}$ for any $j=0,\cdots ,r-1$, where the subscript is the modulus of r. We identify $(a_k,a_{k+1},\cdots ,a_{k+r-1})$ with $(a_0,a_1,\cdots ,a_{r-1})$ for $k\in \mathbb{Z}$. The spanning tree of G is a connected subtree of G covering all vertices of G. A fundamental cycle induced by the spanning tree is the cycle in G generated by recovering an arc which is outside of the spanning tree to the spanning tree. There are two choices of orientations for each support of the fundamental cycle, but we choose only one of them as the representative. Fixing a spanning tree, we denote the set of fundamental cycles by $\Gamma$. Then, the cardinality of $\Gamma$ is $\left|E\right|-\left|V\right|+1=:b_1$. We call $b_1$ the first Betti number.

2.2. Definition of the Grover Walk

Let $\Omega$ be a discrete set. The vector space whose standard basis is labeled by each element of $\Omega$ is denoted by ${\mathbb{C}}^{\Omega }$. The standard basis is denoted by ${\delta }_{\omega }^{(\Omega )}$ ($\omega \in \Omega$), i.e.,

$${\delta }_{\omega }^{(\Omega )}\left({\omega }^{\prime }\right)=\left\{\begin{array}{cc}1\hfill & :\omega ={\omega }^{\prime },\hfill \\ 0\hfill & :\mathrm{otherwise}.\hfill \end{array}\right.$$

Throughout this paper, the inner product is standard, i.e.,

$${\langle \psi ,\varphi \rangle }_{\Omega }=\sum _{\omega \in \Omega }\overline{\psi }\left(\omega \right)\varphi \left(\omega \right),$$

for any $\psi ,\varphi \in {\mathbb{C}}^{\Omega }$, and the norm is defined by

$${\left|\right|\psi \left|\right|}_{\Omega }=\sqrt{{\langle \psi ,\psi \rangle }_{\Omega }}.$$

For any $\psi \in {\mathbb{C}}^{\Omega }$, the support of $\psi$ is defined by

$$supp\left(\psi \right):=\{\omega \in \Omega |\psi \left(\omega \right)\ne 0\}.$$

For subspaces $M,N\subset {\mathbb{C}}^{\Omega }$, the relation

$${\mathbb{C}}^{\Omega }=M\oplus N,$$

means that M and N are complementary spaces in ${\mathbb{C}}^{\Omega }$, i.e., for any $f\in {\mathbb{C}}^{\Omega }$, $g\in M$ and $h\in N$ are uniquely determined such that $f=g+h$, which means, if $u^{\prime }+u^{″}=0$ for some $u^{\prime }\in {\Omega }^{\prime }$ and $u^{″}\in {\Omega }^{″}$, then $u^{\prime }$ and $u^{″}$ must be $u^{\prime }=u^{″}=0$. Note that ${\langle g,h\rangle }_{\Omega }\ne 0$ in general, i.e., M and N are not necessarily orthogonal subspaces. Especially in this paper, we treat an operator which is a submatrix of a unitary operator, and we are not ensured that it is a normal operator. The vector space describing the whole system of the Grover walk is ${\mathbb{C}}^A$. The time evolution operator of the Grover walk on G is defined by

$$\left(U_G\psi \right)\left(a\right)=-\psi \left(\overline{a}\right)+\frac{2}{deg\left(o\right(a\left)\right)}\sum _{t\left(b\right)=o\left(a\right)}\psi \left(b\right)$$

for any $\psi \in {\mathbb{C}}^A$ and $a\in A$. Note that, since $U_G$ is a unitary operator on ${\mathbb{C}}^A$, $U_G$ preserves the ${\ell }^2$ norm, i.e., $\left|\right|U_G{\psi \left|\right|}_A^2={\left|\right|\psi \left|\right|}_A^2$. Let ${\psi }_n\in {\mathbb{C}}^A$ be the nth iteration of the Grover walk ${\psi }_n=U_G{\psi }_{n-1}$ ($n\ge 1$) with the initial state ${\psi }_0$. Then, the probability distribution at time n, ${\mu }_n:V\to [0,1]$, can be defined by

$${\mu }_n\left(v\right)=\sum _{t\left(a\right)=v}{\left|{\psi }_n\left(a\right)\right|}^2$$

if the norm of the initial state is unity. Our interest is the asymptotic behavior of the sequence of probabilities ${\mu }_n$ and also of amplitudes ${\psi }_n$ on the graph comparing with the behavior of the corresponding random walk.

2.3. Boundary Operators

Let $G=(V,A)$ be the original graph. The set of sinks is denoted by $V_s\subset V$. The subgraph of G; $G_0=(V_0,A_0)$, is defined by

$$V_0=V\setminus V_s,A_0=\{a\in A|t\left(a\right),o\left(a\right)\notin V_s\}.$$

The set of self-loops in $G_0$ is denoted by $A_{0,\sigma }\subset A_0$ (see Figure 1). The set of the fundamental cycles in $G_0$ is denoted by $\Gamma$ hereafter. The set of boundary vertices of $G_0$ is defined by

$$\delta G_0=\left\{o\left(a\right)\right|a\in A,o\left(a\right)\in V\setminus V_s,t\left(a\right)\in V_s\}.$$

This means that $\delta G_0$ consists of the origins of arcs flowing into the sinks. Under the above settings of graphs, let us now prepare some notations to show our main theorem.

Definition 1.

Let $\mathrm{deg}\left(u\right)$ be the degree of u in the original graph G. Let $G_0=(V_0,A_0)$ be the subgraph as above. Then, the boundary operators $d_1:{\mathbb{C}}^{A_0}\to {\mathbb{C}}^{V_0}$ and ${\partial }_2:{\mathbb{C}}^{\Gamma }\to {\mathbb{C}}^{A_0}$ are denoted by

$$\left(d_1\psi \right)\left(v\right)=\frac{1}{\sqrt{\mathrm{deg}\left(v\right)}}\sum _{t\left(a\right)=v}\psi \left(a\right),({\partial }_2\Psi )\left(a\right)=\sum _{a\in A\left(c\right)\subset A_0}\Psi \left(c\right),$$

respectively, for any $\psi \in {\mathbb{C}}^A$, $\Psi \in {\mathbb{C}}^{\Gamma }$ and $v\in V_0$, $a\in A_0$. Here, $A\left(c\right)$ is the set of arcs of $c\in \Gamma$.

The boundary operator $d_1$ has the following matrix representation

$${\left(d_1\right)}_{u,a}=\left\{\begin{array}{cc}1/\sqrt{\mathrm{deg}\left(u\right)}\hfill & :t\left(a\right)=u,\hfill \\ 0\hfill & :\mathrm{otherwise},\hfill \end{array}\right.$$

while the boundary operator ${\partial }_2$ has the following matrix representation

$${\left({\partial }_2\right)}_{a,c}=\left\{\begin{array}{cc}1\hfill & :a\in A\left(c\right),\hfill \\ 0\hfill & :\mathrm{otherwise}.\hfill \end{array}\right.$$

Note that $\mathrm{deg}\left(u\right)$ is the degree of G; thus, if $u\in \delta G_0$, then $\mathrm{deg}\left(u\right)$ is greater than the degree in $G_0$. The adjoint operators of $d_1$ and ${\partial }_2$ are defined by

$${\langle f,d_1\psi \rangle }_{V_0}={\langle d_1^{\ast }f,\psi \rangle }_{A_0},{\langle \psi ,{\partial }_2\Psi \rangle }_{A_0}={\langle {\partial }_2^{\ast }\psi ,\Psi \rangle }_{\Gamma }$$

which imply

$$\left(d_1^{\ast }f\right)\left(a\right)=f\left(t\left(a\right)\right),\left({\partial }_2^{\ast }\psi \right)\left(c\right)=\sum _{a\in A\left(c\right)}\psi \left(a\right).$$

Let $S:{\mathbb{C}}^{A_0}\to {\mathbb{C}}^{A_0}$ be a unitary operator defined by $\left(S\psi \right)\left(a\right)=\psi \left(\overline{a}\right)$. We prove that the composition of $d_1(I-S)\circ {\partial }_2$ is identically equal to zero as follows.

Lemma 1.

Let $d_1$ and ${\partial }_2$ be the above. Then, we have

$$d_1(I-S){\partial }_2=0.$$

Proof. 

For any $c\in \Gamma$, let ${\delta }_c^{(\Gamma )}\in {\mathbb{C}}^{\Gamma }$ be the delta function, i.e.,

$${\delta }_c^{(\Gamma )}\left(c^{\prime }\right)=\left\{\begin{array}{cc}1\hfill & :c=c^{\prime },\hfill \\ 0\hfill & :c\ne c^{\prime }.\hfill \end{array}\right.$$

Then, it is enough to see that $d_1(I-S){\partial }_2{\delta }_c^{(\Gamma )}=0$ for any $c\in \Gamma$. Indeed, we find

$$\begin{array}{cc}\hfill d_1(I-S){\partial }_2{\delta }_c^{(\Gamma )}& =d_1(\sum _{a\in A\left(c\right)}{\delta }_a^{\left(A\right)}-\sum _{a\in A\left(c\right)}{\delta }_{\overline{a}}^{\left(A\right)})\hfill \\ \hfill & =\sum _{a\in A\left(c\right)}\frac{1}{\sqrt{\mathrm{deg}\left(t\right(a\left)\right)}}{\delta }_{t\left(a\right)}^{\left(V\right)}-\sum _{a\in A\left(c\right)}\frac{1}{\sqrt{\mathrm{deg}\left(t\left(\overline{a}\right)\right)}}{\delta }_{t\left(\overline{a}\right)}^{\left(V\right)}\hfill \\ \hfill & =0,\hfill \end{array}$$

which is the desired conclusion. □

Let us set the function ${\xi }_c^{(+)}$ induced by $c\in \Gamma$ by

$${\xi }_c^{(+)}:=(I-S){\partial }_2{\delta }_c^{(\Gamma )}.$$

In other words, $\mathrm{supp}\left({\xi }_c^{(+)}\right)=A\left(c\right)\cup A\left(\overline{c}\right)$ and

$$\left({\xi }_c^{(+)}\right)\left(a\right)=\left\{\begin{array}{cc}1\hfill & :a\in A\left(c\right),\hfill \\ -1\hfill & :\overline{a}\in A\left(c\right),\hfill \\ 0\hfill & :\mathrm{otherwise}.\hfill \end{array}\right.$$

The function ${\xi }_c^{(+)}$ represents the fundamental cycle c. Let us introduce ${\chi }_S:{\mathbb{C}}^A\to {\mathbb{C}}^{A_0}$ by

$$\left({\chi }_S\varphi \right)\left(a\right)=\varphi \left(a\right)$$

for all $a\in A_0$. The adjoint ${\chi }_S^{\ast }:{\mathbb{C}}^{A_0}\to {\mathbb{C}}^A$ is described by

$$\left({\chi }_S^{\ast }f\right)\left(a\right)=\left\{\begin{array}{cc}f\left(a\right)\hfill & :a\in A_0,\hfill \\ 0\hfill & :\mathrm{otherwise}.\hfill \end{array}\right.$$

A matrix representation of ${\chi }_S$ is expressed as follows:

$${\chi }_S\cong \left[I_{A_0}\right|0],$$

which is a $|A_0|\times |A|$ matrix. The function ${\xi }_c^{(+)}$ satisfies the following properties:

Proposition 1.

For any fundamental cycle c in $G_0\subset G$, we have ${\chi }_S^{\ast }{\xi }_c^{(+)}\in ker(1-U_G)$.

Proof. 

The following direct computation gives the consequence:

$$\begin{array}{cc}\hfill \left(U_G{\chi }_S^{\ast }{\xi }_c^{(+)}\right)\left(a\right)& =-\left({\chi }_S^{\ast }{\xi }_c^{(+)}\right)\left(\overline{a}\right)+\frac{2}{\mathrm{deg}\left(o\right(a\left)\right)}\sum _{t\left(b\right)=o\left(a\right)}\left({\chi }_S^{\ast }{\xi }_c^{(+)}\right)\left(b\right)\hfill \\ \hfill & =\left({\chi }_S^{\ast }{\xi }_c^{(+)}\right)\left(a\right)+\frac{2}{\sqrt{\mathrm{deg}\left(o\right(a\left)\right)}}\left(d_1{\chi }_S^{\ast }{\xi }_c^{(+)}\right)\left(o\left(a\right)\right)\hfill \\ \hfill & =\left({\chi }_S^{\ast }{\xi }_c^{(+)}\right)\left(a\right).\hfill \end{array}$$

Here, the first equality derives from the definition of $U_G$. In the second equality, since supp$\left({\xi }_c^{(+)}\right)\subset A_0\subset A$ and the summation of RHS in the first equality is essentially the same as the one over $A_0$, we can apply the definition of $d_1$ to this. We use Lemma 1 in the last equality. □

We set $\mathcal{K}\subset {\mathbb{C}}^{A_0}$ by

$$\mathcal{K}=\mathrm{span}\left\{{\chi }_S{\xi }_c^{(+)}\right|c\in \Gamma \subset G_0\}.$$

(1)

The self-adjoint operator

$$T:=\left({\chi }_S{d}_1\right)S{\left({\chi }_S{d}_1\right)}^{\ast }$$

on ${\mathbb{C}}^{A_0}$ is similar to the transition probability operator $P^{\prime }$ with the Dirichlet boundary condition on $\delta V_0$; i.e.,

$$P^{\prime }=D^{-1/2}T{D}^{1/2},$$

where $\left(Df\right)=\mathrm{deg}\left(u\right)f\left(u\right)$. Here, the matrix representation of $P^{\prime }$ is described by

$${\left(P^{\prime }\right)}_{u,v}:={\langle {\delta }_u^{\left(V_0\right)},P^{\prime }{\delta }_v^{\left(V_0\right)}\rangle }_{V_0}=\left\{\begin{array}{cc}1/\mathrm{deg}\left(u\right)\hfill & :\mathrm{if}u\mathrm{and}v\mathrm{are}\mathrm{connected},\hfill \\ 0\hfill & :\mathrm{otherwise},\hfill \end{array}\right.$$

for any $u,v\in V_0$. If $Tf=xf$ and $Tg=yg$ ($x\ne y$), then we find the orthogonality such that

$$\langle (1-e^{iarccosx}S)d_1^{\ast }f,(1-e^{-iarccosy}S)d_1^{\ast }g\rangle =0,$$

$$\langle (1-e^{iarccosx}S)d_1^{\ast }f,(1-e^{iarccosy}S)d_1^{\ast }g\rangle =0,$$

$$\langle (1-e^{iarccosx}S)d_1^{\ast }f,(1-e^{-iarccosy}S)d_1^{\ast }g\rangle =0.$$

Then, we set $\mathcal{T}\subset {\mathbb{C}}^{A_0}$ by

$$\mathcal{T}=\underset{\left|\lambda \right|=1}{⨁}\left\{(1-\lambda S)d_1^{\ast }f\right|f\in ker((\lambda +{\lambda }^{-1})/2-T),supp\left(f\right)\subset V_0\setminus \delta V_0\}.$$

(2)

This is the subspace of ${\mathbb{C}}^{A_0}$ lifted up from the eigenfunctions in ${\mathbb{C}}^{V_0}$ of the Dirichlet cut random walk T by $(1-\lambda S)d_1^{\ast }f$. It is shown that $\mathrm{Spec}\left(E\right)\subset \mathbb{D}$, where $\mathbb{D}$ is the unit disc $\{z\in \mathbb{C}|\left|z\right|\le 1\}$ in Proposition 3, and $\mathcal{T}={\oplus }_{\left|\lambda \right|=1,\lambda \ne \pm 1}ker(\lambda -E)$, where $E:={\chi }_S{U}_G{\chi }_S^{\ast }$ in Lemma 3.

3. Definition of the Grover Walk on Graphs with Sinks

Let $G=(V,A)$ be a finite and connected graph with sinks $V_s=\{v_1,\cdots ,v_q\}\subset V$. We consider the subgraph $G_0=(V_0,A_0)$ as defined in Section 2.3. Assume that $G_0$ is connected. For simplicity, in this paper, we consider the initial state of the Grover walk ${\varphi }_0$ that satisfies the condition supp$\left({\varphi }_0\right)\subset A_0$. (If we consider general initial state ${\varphi }_0^{\prime }$ such that supp$\left({\varphi }_0^{\prime }\right)\cap (A\setminus A_0)\ne \varnothing$, replacing ${\varphi }_0^{\prime }$ into ${\varphi }_0={\varphi }_1^{\prime }$, we can reproduce the QW with this initial state after $n\ge 1$ by our setting.) The time evolution of the Grover walk with sinks $V_s$ with such an initial state ${\varphi }_0$ is defined by

$${\varphi }_n\left(a\right)=\left\{\begin{array}{cc}\left(U_G{\varphi }_{n-1}\right)\left(a\right)\hfill & :t\left(a\right)\in V\setminus V_s,\hfill \\ 0\hfill & :t\left(a\right)\in V_s,\hfill \end{array}\right.$$

(3)

This means that a quantum walker at a sink falls into a pit trap. We are interested in the survival probability of the Grover walk defined by

$$\gamma :=\underset{n\to \infty }{lim}\sum _{a\in A}{\left|{\varphi }_n\left(a\right)\right|}^2.$$

It is the probability that the quantum walker remains in the graph without falling into the sinks forever. Considering the corresponding isotropic random walk with sinks such that

$$p_n\left(v\right)=\left\{\begin{array}{cc}\left(P{p}_{n-1}\right)\left(v\right)\hfill & :v\in V\setminus V_s,\hfill \\ 0\hfill & :v\in V_s,\hfill \end{array}\right.$$

we find that its survival probability is zero,

$${\gamma }^{RW}:=\underset{n\to \infty }{lim}\sum _{v\in V}{p}_n\left(v\right)=0,$$

because the first hitting time of a random walk to an arbitrary vertex for a finite graph is finite. On the other hand, in the case of the Grover walk, the survival probability becomes positive, up to the initial state. In this paper, we clarify a necessary and sufficient condition for $\gamma >0$.

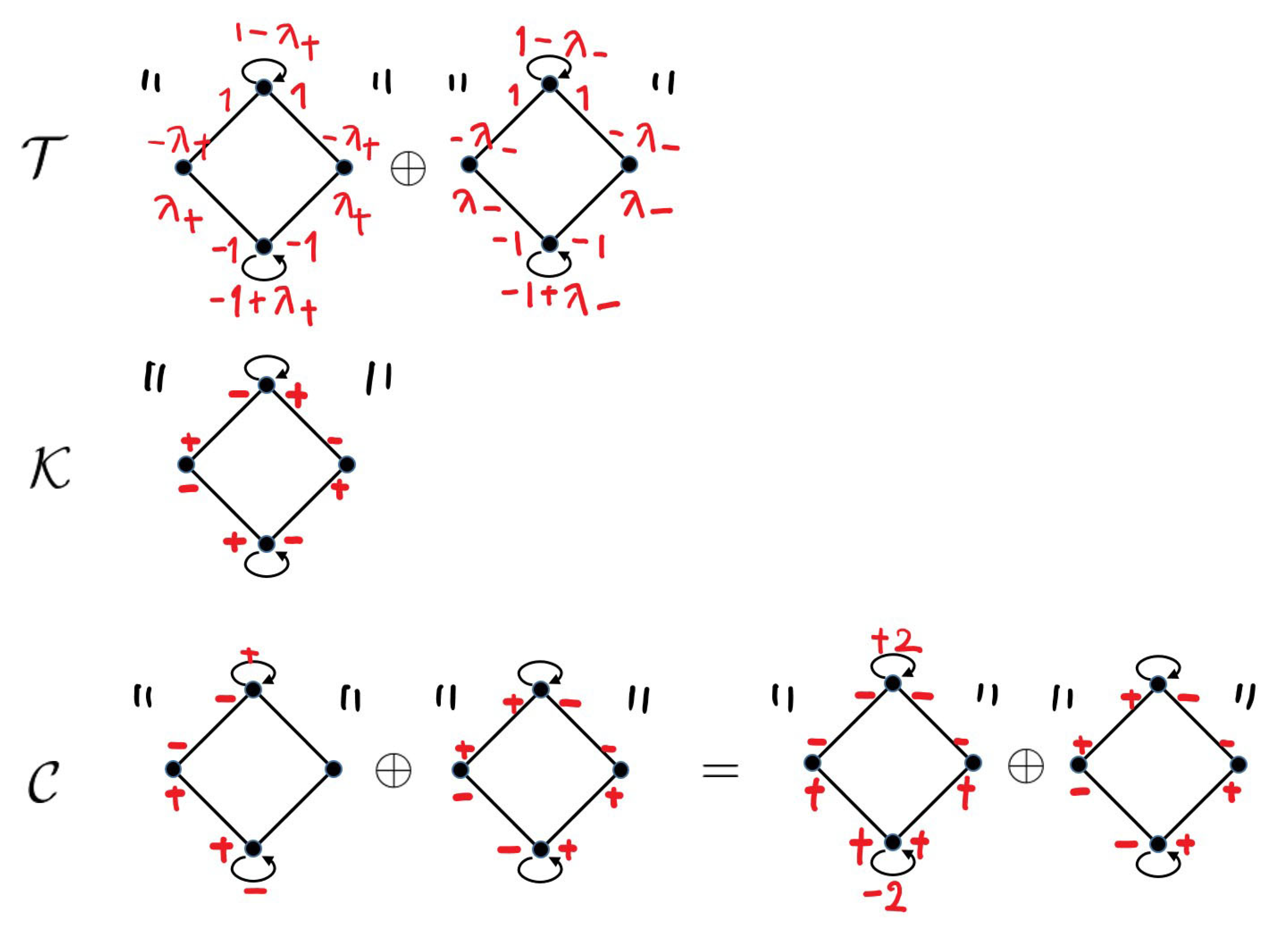
4. Main Theorem

We consider the case study on $G_0$ by

Case A: 

$A_{0,\sigma }=\varnothing$ and $G_0$ is a bipartite graph;

Case B: 

$A_{0,\sigma }=\varnothing$ and $G_0$ is a non-bipartite graph;

Case C: 

$A_{0,\sigma }\ne \varnothing$ and $G_0\setminus A_{0,\sigma }$ is a bipartite graph;

Case D: 

$A_{0,\sigma }\ne \varnothing$ and $G_0\setminus A_{0,\sigma }$ is a non-bipartite graph.

For a subspace $\mathcal{H}\subset {\mathbb{C}}^{A_0}$, the projection operator onto $\mathcal{H}$ is denoted by ${\Pi }_{\mathcal{H}}$. Then, we obtain the following theorem.

Theorem 1.

Let ${\varphi }_n$ be the nth iteration of the Grover walk on $G=(V,A)$ with sinks. Let the survival probability at time n be defined by

$${\gamma }_n=\sum _{a\in A}{\left|\left({\varphi }_n\right)\right|}^2.$$

The subspaces $\mathcal{A},\mathcal{B},\mathcal{C},\mathcal{D}$ of ${\mathbb{C}}^{A_0}$ are defined in (7),..., (10), respectively. Then, we have

1. 

${lim}_{n\to \infty }{\gamma }_n=\gamma$ exists.

2. 

The survival probability γ is expressed by

$$\gamma =\left|\right|{\Pi }_{\mathcal{T}}{\chi }_S{\varphi }_0{\left|\right|}^2+\left|\right|{\Pi }_{\mathcal{K}}{\chi }_S{\varphi }_0{\left|\right|}^2+\left\{\begin{array}{cc}\left|\right|{\Pi }_{\mathcal{A}}{\chi }_S{\varphi }_0{\left|\right|}^2\hfill & :\mathit{Case}A\hfill \\ \left|\right|{\Pi }_{\mathcal{B}}{\chi }_S{\varphi }_0{\left|\right|}^2\hfill & :\mathit{Case}B\hfill \\ \left|\right|{\Pi }_{\mathcal{C}}{\chi }_S{\varphi }_0{\left|\right|}^2\hfill & :\mathit{Case}C\hfill \\ \left|\right|{\Pi }_{\mathcal{D}}{\chi }_S{\varphi }_0{\left|\right|}^2\hfill & :\mathit{Case}D\hfill \end{array}\right.$$

Proof. 

Part 1 of Theorem 1 is obtained by the consequences of Proposition 3 and Part 2 derives from Propositions 5 and 6. □

From this theorem, we obtain useful sufficient conditions for non-zero survival probability as follows.

Corollary 1.

Assume $G_0$ is a finite and connected graph. If $G_0$ is not a tree or $G_0$ has more than two self-loops, then $\gamma >0$.

Remark 1.

The eigenspaces $\mathcal{A},\mathcal{B},\mathcal{C},\mathcal{D}$ correspond to the p-attractors defined in [8].

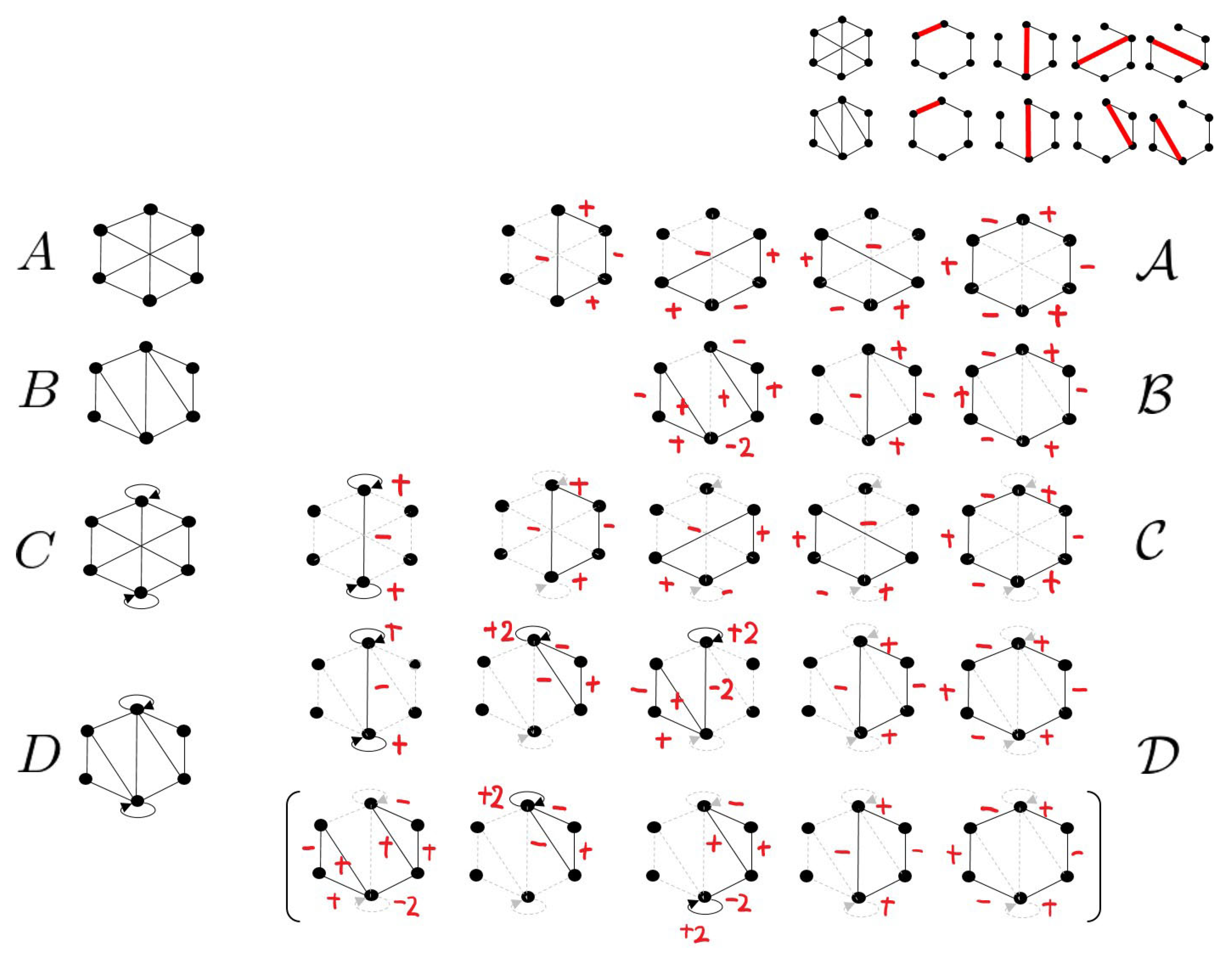
5. Example

Let us consider a simple example in Figure 1. $G_0=(V_0,A_0)$ with $V_0=\{1,2,3,4\}$ and $A_0=\{a_1,a_2,a_3,a_4,{\overline{a}}_1,{\overline{a}}_2,{\overline{a}}_3,{\overline{a}}_4,b_1,b_2\}$, where $a_1$ has the origin 1 and the terminus 2; $a_2$ has the origin 2 and the terminus 3; $a_3$ has the origin 1 and the terminus 4; $a_4$ has the origin 1 and the terminus 1; and $b_1$ and $b_2$ are the self loops on 1 and 3, respectively.

This graph fits into Case C. Thus, let q be the closed walk by $q=(a_1,a_2,a_3,a_4)$ and $q^{\prime }$ be the walk between two selfloops by $(b_1,a_1,a_2,b_2)$. Then, ${\xi }_q^{(+)}$, and the functions defined by (6) and Definition 2 are given by

$$\begin{array}{cc}\hfill {\xi }_q^{(+)}& =({\delta }_{a_1}+{\delta }_{a_2}+{\delta }_{a_3}+{\delta }_{a_4})-({\delta }_{{\overline{a}}_1}+{\delta }_{{\overline{a}}_2}+{\delta }_{{\overline{a}}_3}+{\delta }_{{\overline{a}}_4},)\hfill \\ \hfill {\xi }_q^{(-)}& =({\delta }_{a_1}+{\delta }_{{\overline{a}}_1})-({\delta }_{a_2}+{\delta }_{{\overline{a}}_2})+({\delta }_{a_3}+{\delta }_{{\overline{a}}_3})-({\delta }_{a_4}+{\delta }_{{\overline{a}}_4}),\hfill \\ \hfill {\eta }_{b_1-b_2}& ={\delta }_{b_1}-({\delta }_{a_1}+{\delta }_{{\overline{a}}_1})+({\delta }_{a_2}+{\delta }_{{\overline{a}}_2})-{\delta }_{b_2}.\hfill \end{array}$$

The matrix representation of the self adjoint operator T is expressed by

$$T=\frac{1}{3}\left[\begin{array}{cccc}1& 1& 0& 1\\ 1& 0& 1& 0\\ 0& 1& 1& 1\\ 1& 0& 1& 0\end{array}\right].$$

The eigenvector of T which has no overlaps to $\delta V_0=\{2,4\}$ is easily obtained by

$$f={[1,0,-1,0]}^{\top }$$

which satisfies $Tf=(1/3)f$. Here, the symbol “⊤” is the transpose. The eigenfunctions lifted up to ${\mathbb{C}}^A$ from f is

$$\left({\phi }_{\pm }\right)\left(a\right)=f\left(t\left(a\right)\right)-{\lambda }_{\pm }f\left(o\left(a\right)\right)$$

by (2), where

$${\lambda }_{\pm }=\frac{1}{3}(1\pm i\sqrt{8})=e^{\pm i\theta },\theta =arccos\frac{1}{3}.$$

Then, we have

$$\begin{array}{c}\hfill {\phi }_{\pm }\left(a_1\right)=-{\lambda }_{\pm },{\phi }_{\pm }\left(a_2\right)=-1,{\phi }_{\pm }\left(a_3\right)={\lambda }_{\pm },{\phi }_{\pm }\left(a_4\right)=1,\\ \hfill {\phi }_{\pm }\left({\overline{a}}_1\right)=1,{\phi }_{\pm }\left({\overline{a}}_2\right)={\lambda }_{\pm },{\phi }_{\pm }\left({\overline{a}}_3\right)=-1,{\phi }_{\pm }\left({\overline{a}}_4\right)=-{\lambda }_{\pm },\\ \hfill {\phi }_{\pm }\left(b_1\right)=1-{\lambda }_{\pm },{\phi }_{\pm }\left(b_2\right)=-1+{\lambda }_{\pm }.\end{array}$$

It holds that $E{\phi }_{\pm }={\lambda }_{\pm }{\phi }_{\pm }$. We obtain

$$\begin{array}{cc}\hfill \mathcal{T}& =\mathbb{C}{\phi }_{+}\oplus \mathbb{C}{\phi }_{-},\hfill \\ \hfill \mathcal{K}& =\mathbb{C}{\xi }_{(a_1,a_2,a_3,a_4)}^{(+)},\hfill \\ \hfill \mathcal{C}& =\mathbb{C}{\xi }_{(a_1,a_2,a_3,a_4)}^{(-)}\oplus \mathbb{C}{\eta }_{b_1-b_2}.\hfill \end{array}$$

After the Gram–Schmidt procedure to $\mathcal{C}$, we have

$$\mathcal{C}=\mathbb{C}{\xi }_{(a_1,a_2,a_3,a_4)}^{(-)}\oplus \mathbb{C}({\eta }_{b_1-b_2}+{\eta }_{b_1-b_2}^{\prime }).$$

Here, we denote

$${\eta }_{b_1-b_2}^{\prime }={\delta }_{b_1}-({\delta }_{a_4}+{\delta }_{{\overline{a}}_4})+({\delta }_{a_3}+{\delta }_{{\overline{a}}_3})-{\delta }_{b_2}$$

(see Figure 2). We express the functions ${\phi }_{\pm }$, ${\xi }_{(a_1,a_2,a_3,a_4)}^{(+)}$, ${\eta }_{b_1-b_2}$, ${\eta }_{b_1-b_2}^{\prime }$, ${\eta }_{b_1-b_2}+{\eta }_{b_1-b_2}^{\prime }$ by weighted sub-digraphs of $G_0$. Then, the time evolution of the asymptotic dynamics of this quantum walk is described by

$$\begin{array}{c}{U}^n\sim \frac{1}{8}|{\xi }_{(a_1,a_2,a_3,a_4)}^{(+)}\rangle \langle {\xi }_{(a_1,a_2,a_3,a_4)}^{(+)}|\hfill \\ +{(-1)}^n\left(\frac{1}{8}|{\xi }_{(a_1,a_2,a_3,a_4)}^{(-)}\rangle \langle {\xi }_{(a_1,a_2,a_3,a_4)}^{(-)}|+\frac{1}{16}|{\eta }_{b_1-b_2}+{\eta }_{b_1-b_2}^{\prime }\rangle \langle {\eta }_{b_1-b_2}+{\eta }_{b_1-b_2}^{\prime }|\right)\\ \hfill +e^{in\theta }\frac{3}{32}|{\phi }_{+}\rangle \langle {\phi }_{+}|+e^{-in\theta }\frac{3}{32}|{\phi }_{-}\rangle \langle {\phi }_{-}|.\end{array}$$

(4)

Finally, for example, if the initial state is ${\phi }_0={\delta }_{b_1}$, then the survival probability can be computed by

$$\begin{array}{cc}\hfill \gamma & =||{\Pi }_{\mathcal{T}}{\phi }_0{||}^2+||{\Pi }_{\mathcal{K}}{\phi }_0{||}^2+||{\Pi }_{\mathcal{C}}{\phi }_0{||}^2\hfill \\ \hfill & =\frac{1}{16}{|\langle {\eta }_{b_1-b_2}+{\eta }_{b_1-b_2}^{\prime },{\phi }_0\rangle |}^2+\frac{3}{32}{|\langle {\phi }_{+},{\phi }_0\rangle |}^2+\frac{3}{32}{|\langle {\phi }_{-},{\phi }_0\rangle |}^2\hfill \\ \hfill & =\frac{1}{16}{|2|}^2+\frac{3}{32}|1-{\lambda }_{+}{|}^2+\frac{3}{32}{|1-{\lambda }_{-}|}^2\hfill \\ \hfill & =1/2.\hfill \end{array}$$

The second equality derives from the fact that the orthonormalized eigenvectors in the centered generalized eigenspace which have an overlap with the self-loop $b_1$ are given by $(1/4)({\eta }_{b_1-b_2}+{\eta }_{b_1-b_2}^{\prime })$ and $\sqrt{3/32}{\phi }_{\pm }$.

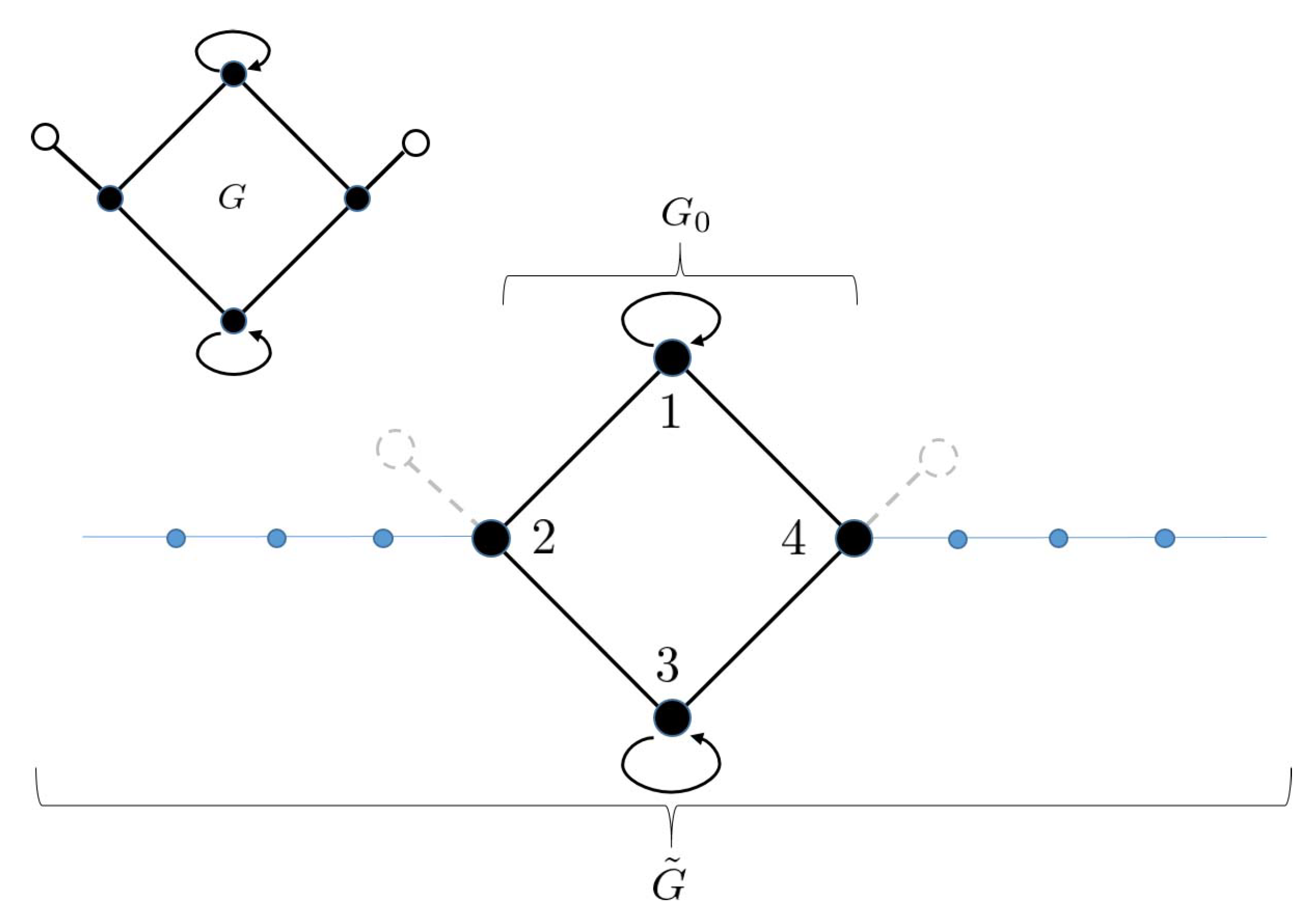
6. Relation between Grover Walk with Sinks and Grover Walk with Tails

6.1. Grover Walk on Graphs with Tails

Let $G=(V,A)$ be a finite and connected graph with the set of sinks $V_s\subset V$. We introduce the infinite graph $\tilde{G}=(\tilde{V},\tilde{A})$ by adding the semi-infinite paths to each vertex of $\delta V=\{v_1,\cdots ,v_r\}$, that is,

$$\begin{array}{cc}\hfill \tilde{V}& =(V\setminus V_s)\cup \left({\cup }_{j=1}^{r}V\left({\mathbb{P}}_j\right)\right),\hfill \\ \hfill \tilde{A}& ={\cup }_{j=1}^{r}A\left({\mathbb{P}}_j\right)\cup \left(A\setminus \{a\in A|t\left(a\right)\in V_{s}oro\left(a\right)\in V_s\}\right).\hfill \end{array}$$

Here, ${\mathbb{P}}_i$s are the semi-infinite paths named the tail whose origin vertex is identified with $v_i$ ($i=1,\cdots ,r$) (see Figure 1). Recall that $G_0=(V_0,A_0)$ is the subgraph of G eliminating the sinks $V_s$. Recall also that ${\chi }_S:{\mathbb{C}}^A\to {\mathbb{C}}^{A_0}$ is

$$\left({\chi }_S\varphi \right)\left(a\right)=\varphi \left(a\right)$$

for all $a\in A_0$. In the same way, we newly introduce ${\chi }_T:{\mathbb{C}}^{\tilde{A}}\to {\mathbb{C}}^{A_0}$ by

$$\left({\chi }_T\varphi \right)\left(a\right)=\varphi \left(a\right)$$

for all $a\in A_0$. The adjoint ${\chi }_T^{\ast }:{\mathbb{C}}^{A_0}\to {\mathbb{C}}^{\tilde{A}}$ is

$$\left({\chi }_T^{\ast }f\right)\left(a\right)=\left\{\begin{array}{cc}f\left(a\right)\hfill & :a\in A_0,\hfill \\ 0\hfill & :\mathrm{otherwise}.\hfill \end{array}\right.$$

The only difference between ${\chi }_S$ and ${\chi }_T$ is the domain. A matrix representation of ${\chi }_T$ is

$${\chi }_T\cong \left[I_{A_0}\right|0]$$

which is a $|A_0|\times \infty$ matrix because $|\tilde{A}\setminus A_0|=\infty$. The following theorem was proven by [19].

Theorem 2

([19]). Let $\tilde{G}=(\tilde{V},\tilde{A})$ be the graph with infinite tails ${\left\{{\mathbb{P}}_j\right\}}_{j=1}^r$ induced by $G_0$ and its boundaries $\delta V_0$. Assume the initial state ${\psi }_0$ is

$${\psi }_0\left(a\right)=\left\{\begin{array}{cc}{\alpha }_1\hfill & :a\in A\left({\mathbb{P}}_1\right),\mathrm{dist}(o\left(a\right),v_1)>\mathrm{dist}(t\left(a\right),v_1),\hfill \\ ⋮\hfill \\ {\alpha }_r\hfill & :a\in A\left({\mathbb{P}}_r\right),\mathrm{dist}(o\left(a\right),v_r)>\mathrm{dist}(t\left(a\right),v_r),\hfill \\ 0\hfill & :\mathit{otherwise}.\hfill \end{array}\right.$$

Then, ${lim}_{n\to \infty }{\psi }_n\left(a\right)=:{\psi }_{\infty }\left(a\right)$ exists and ${\psi }_{\infty }\left(a\right)$ is expressed by

$${\psi }_{\infty }\left(a\right)=\frac{{\alpha }_1+\cdots +{\alpha }_r}{r}+\mathrm{j}\left(a\right).$$

Here, $\mathrm{j}(·)$ is the electric current flow on the electric circuit assigned the resistance value 1 at each edge, that is, $\mathrm{j}(·)$ satisfies the following properties:

$$\begin{array}{cc}\hfill d_1\mathrm{j}& =0,\mathrm{j}\left(\overline{a}\right)=-\mathrm{j}\left(a\right)\left({\mathrm{Kirchhoff}}^{\prime }\mathrm{s}\mathrm{current}\mathrm{law}\right)\hfill \\ \hfill {\partial }_2^{\ast }\mathrm{j}& =0\left({\mathrm{Kirchhoff}}^{\prime }\mathrm{s}\mathrm{voltage}\mathrm{law}\right)\hfill \end{array}$$

with the boundary conditions

$$\mathrm{j}\left(e_i\right)={\alpha }_i-\frac{{\alpha }_1+\cdots +{\alpha }_r}{r}$$

(5)

for any $e_i$ ($i=1,\cdots ,r$) such that $t\left(e_i\right)=v_j$ and $o\left(e_i\right)\in V\left({\mathbb{P}}_i\right)$.

Remark 2.

The stationary state ${\psi }_{\infty }$ satisfies the equation

$${\psi }_{\infty }\left(a\right)=\left(U_{\tilde{G}}{\psi }_{\infty }\right)\left(a\right)$$

for any $a\in A$ and ${\psi }_{\infty }\in {\ell }^{\infty }$, however $\left|\right|{\psi }_{\infty }{\left|\right|}_{\tilde{A}}=\infty$.

Remark 3.

The function ${\xi }_c^{(+)}=(1-S){\partial }_2{\delta }_c^{(\Gamma )}$ also satisfies

$${\chi }_T^{\ast }{\xi }_c^{(+)}\left(a\right)=\left(U_{\tilde{G}}{\chi }_T^{\ast }{\xi }_c^{(+)}\right)\left(a\right)$$

and Kirchhoff’s current and voltage laws if the internal graph $G_0$ is not a tree, while it does not satisfy the boundary condition (5) because the support of this function ${\chi }_T^{\ast }{\xi }_c^{(+)}$ has no overlaps to the tails but is included in the fundamental cycle c in the internal graph $G_0$.

6.2. Relation between Grover Walk with Sinks and Grover Walk with Tails

Let us consider the Grover walk on G with sinks $V_s$ and with the initial state ${\psi }_0^{\left(S\right)}\in {\mathbb{C}}^A$. We describe $U_G$ as the time evolution operator of Grover walk on G. The nth iteration of this walk following (3) is denoted by ${\psi }_n^{\left(S\right)}$. Let us also consider the Grover walk on $\tilde{G}$ with the tails and with the “same” initial state

$${\psi }_0^{\left(T\right)}\left(a\right)=\left\{\begin{array}{cc}{\psi }_0^{\left(S\right)}\left(a\right)\hfill & :a\in A_0,\hfill \\ 0\hfill & :\mathrm{otherwise}.\hfill \end{array}\right.$$

Note that the initial state ${\psi }_0^{\left(S\right)}$ is different from the one in the setting of Theorem 2. Putting the time evolution operator on $\tilde{G}$ by $U_{\tilde{G}}$, we denote the nth iteration of this walk by ${\psi }_n^{\left(T\right)}=U_{\tilde{G}}{\psi }_{n-1}^{\left(T\right)}$. Then, we obtain a simple but important relation between QW with sinks and QW with tails.

Lemma 2.

Let the setting of the QW with sinks and QW with tails be as the above. Then, for any time step n, we have

$${\chi }_S{\psi }_n^{\left(S\right)}={\chi }_T{\psi }_n^{\left(T\right)}.$$

Proof. 

The initial state of ${\chi }_S{\psi }_0^{\left(S\right)}$ coincides with ${\chi }_T{\psi }_0^{\left(T\right)}$ because of the setting. Note that ${\chi }_J^{\ast }{\chi }_J$ is the projection operator onto ${\mathbb{C}}^{A_0}$ while ${\chi }_J{\chi }_J^{\ast }$ is the identity operator on ${\mathbb{C}}^{A_0}$$(J\in \{S,T\left\}\right)$. Since ${\psi }_n^{\left(S\right)}\left(a\right)=0$ for any $a\in V_s$, we have

$$(1-{\chi }_S^{\ast }{\chi }_S){\psi }_n^{\left(S\right)}=0$$

for any $n\in \mathbb{N}$. Then, putting ${\chi }_S{\psi }_n^{\left(S\right)}=:{\varphi }_n^{\left(S\right)}$ and ${\chi }_S{U}_G{\chi }_S^{\ast }=:E$, we have

$$\begin{array}{cc}\hfill {\varphi }_n^{\left(S\right)}& ={\chi }_S{\psi }_n^{\left(S\right)}={\chi }_S{U}_G{\psi }_{n-1}^{\left(S\right)}\hfill \\ \hfill & ={\chi }_S{U}_G({\chi }_S^{\ast }{\chi }_S+(1-{\chi }_S^{\ast }{\chi }_S)){\psi }_{n-1}^{\left(S\right)}\hfill \\ \hfill & =E{\varphi }_{n-1}^{\left(S\right)}+\left({\chi }_S{U}_G(1-{\chi }_S^{\ast }{\chi }_S)\right){\psi }_{n-1}^{\left(S\right)}\hfill \\ \hfill & =E{\varphi }_{n-1}^{\left(S\right)}.\hfill \end{array}$$

It is easy to see that $E={\chi }_S{U}_G{\chi }_S^{\ast }={\chi }_T{U}_{\tilde{G}}{\chi }_T^{\ast }$. Since the support of the initial state is included in the internal graph, the inflow never comes into the internal graph from the tail for any time n, which implies

$$\left({\chi }_T{U}_{\tilde{G}}(1-{\chi }_T^{\ast }{\chi }_T)\right){\psi }_n^{\left(T\right)}=0.$$

It holds that $E={\chi }_S\tilde{U}{\chi }_S^{\ast }={\chi }_T{U}_{\tilde{G}}{\chi }_T^{\ast }$. Then, putting ${\varphi }_n^{\left(T\right)}:={\chi }_T{\psi }_n^{\left(T\right)}$, in the same way as ${\psi }_n^{\left(S\right)}$, we have

$$\begin{array}{cc}\hfill {\varphi }_n^{\left(T\right)}& ={\chi }_T{U}_{\tilde{G}}({\chi }_T^{\ast }{\chi }_T+(1-{\chi }_T^{\ast }{\chi }_T)){\psi }_{n-1}^{\left(T\right)}\hfill \\ \hfill & =E{\varphi }_{n-1}^{\left(T\right)}.\hfill \end{array}$$

Therefore, ${\chi }_S{\psi }_n^{\left(S\right)}$ and ${\chi }_T{\psi }_n^{\left(T\right)}$ follow the same recurrence and have the same initial state which means ${\chi }_S{\psi }_n^{\left(S\right)}={\chi }_T{\psi }_n^{\left(T\right)}$ for any $n\in \mathbb{N}$. □

Corollary 2.

Let the initial state for the Grover walk with sinks be ${\varphi }_0$ with supp$\left({\varphi }_0\right)\subset A_0$. The survival probability γ can be expressed by

$$\gamma =\left|\right|{\varphi }_0{\left|\right|}_A^2-\sum _{n=0}^{\infty }{\tau }_n,$$

where ${\tau }_n$ is the outflow of the QW with tails from the internal graph $G_0$, i.e.,

$${\tau }_n=\sum _{o\left(a\right)\in \delta V,t\left(a\right)\notin A_0}{\left|\left(U_{\tilde{G}}{\chi }_T^{\ast }{\varphi }_{n-1}^{\left(T\right)}\right)\left(a\right)\right|}^2$$

Remark 4.

The time evolution for ${\varphi }_n^{\left(T\right)}$ is given by

$${\varphi }_n^{\left(T\right)}=E{\varphi }_{n-1}^{\left(T\right)}+\rho ,$$

where $\rho ={\chi }_T{U}_{\tilde{G}}{\psi }_0^{\left(T\right)}$. In this case, the inflow is $\rho =0$. On the other hand, in the setting of Theorem 2, ρ is given by a nonzero constant vector.

Let us now consider a QW with tails with a general initial state ${\Psi }_0\in {\mathbb{C}}^{\tilde{A}}$ on $\tilde{G}$. We denote $\nu ={\chi }_T{\Psi }_0$ and $\rho ={\chi }_T{U}_{\tilde{G}}(1-{\chi }^{\ast }\chi ){\Psi }_0$. We summarize the relation between a QW with sinks and a QW for the setting of Theorem 2 in

Table 1. Relatiion beteween QWs with tails and sinks.

	$\mathit{\rho }$	$\mathit{\nu }$	State in ${\mathit{G}}_0$
QW with tails in the setting of Theorem 2 [19]	$\ne 0$	$=0$	$\in {\mathcal{H}}_s$ (for any n)
QW with sinks	$=0$	$\ne 0$	$\in {\mathcal{H}}_c$ (asymptotically)

from the viewpoint of a QW with tails.

7. Centered Generalized Eigenspace of E for the Grover Walk Case

7.1. The Stationary States from the Viewpoint of the Centered Generalized Eigenspace

From the above discussion, we see the importance of the spectral decomposition

$$E={\chi }_S{U}_G{\chi }_S^{\ast }={\chi }_T{U}_{\tilde{G}}{\chi }_T^{\ast },$$

to obtain both limit behaviors. The operator E is no longer a unitary operator, and, moreover, it is not ensured that it is diagonalizable. The centered generalized eigenspace of E is defined by

$${\mathcal{H}}_c:=\{\psi \in {\mathbb{C}}^{A_0}|\exists m\ge 1\mathrm{and}\exists |\lambda |=1\mathrm{such}\mathrm{that}(E^m-\lambda )\psi =0\}$$

Let ${\mathcal{H}}_s$ be defined by

$${\mathbb{C}}^{A_0}={\mathcal{H}}_c\oplus {\mathcal{H}}_s.$$

Here, “⊕” means ${\mathcal{H}}_c$ and ${\mathcal{H}}_s$ are complementary spaces, that is, if $u_c+u_v=0$ for some $u_c\in {\mathcal{H}}_c$ and $u_v\in {\mathcal{H}}_s$, then $u_c$ and $u_v$ must be $u_c=u_v=0$. Note that, since E is not a normal operator on a vector space ${\mathcal{H}}_c\oplus {\mathcal{H}}_s$, it seems that in general $\langle u_c,u_v\rangle \ne 0$ for $u\in {\mathcal{H}}_c$ and ${\mathcal{H}}_s\in N$. However, we can see some important properties of the spectrum of E in the following proposition.

Proposition 2

([19]).

1. 

For any $\lambda \in \mathrm{Spec}\left(E\right)$, it holds that $\left|\lambda \right|\le 1$, i.e.,

$${\mathcal{H}}_s=\left\{\psi \right|\exists m\in {\mathbb{N},\exists \left|\lambda \right|<1,(U-\lambda )}^m\psi )=0\}.$$

2. 

Let $P_c$ be the projection operator on ${\mathcal{H}}_c$ along with ${\mathcal{H}}_s$; that is, $P_{c}E=E{P}_c$ and $P_c^2=P_c$. Then, $P_c$ is the orthogonal projection onto ${\mathcal{H}}_c$, i.e., $P_c=P_c^{\ast }$.

3. 

The operator E acts as a unitary operator on ${\mathcal{H}}_c$, that is, ${\mathcal{H}}_c={\oplus }_{\left|\lambda \right|=1}ker(\lambda -E)$ and $U_G{\chi }_S^{\ast }\phi =\lambda {\chi }_S^{\ast }\phi$ for any $\phi \in ker(\lambda -E)$ with $\left|\lambda \right|=1$.

We call ${\mathcal{H}}_c$ and ${\mathcal{H}}_s$ the centered eingenspace and the stable eigenspace [18], respectively.

Corollary 3.

For any$\psi \in {\mathcal{H}}_s$and$\varphi \in {\mathcal{H}}_c$, it holds that$\langle \psi ,\varphi \rangle =0$.

Now, let us see the stationary states from the viewpoint of the orthogonal decomposition of ${\mathcal{H}}_c\oplus {\mathcal{H}}_s$.

Proposition 3.

1. 

The state ${\chi }_T{\psi }_n$ in Theorem 2 belongs to ${\mathcal{H}}_s$ for any time step $n\in \mathbb{N}$.

2. 

The state of QW with sinks, ${\chi }_S{\varphi }_n$, asymptotically belongs to ${\mathcal{H}}_c$ in the long time limit n.

Proof. 

The inflow $\rho ={\chi }^{\ast }U{\psi }_0$ is orthogonal to ${\mathcal{H}}_c$ by a direct consequence of Lemma 3.5 in [19], which implies $E^n\rho \in {\mathcal{H}}_s$ for any $n\in \mathbb{N}$ by Proposition 2. Since the stationary state of Part 1 is described by the limit of the following recurrence

$${\chi }_T{\psi }_n=E{\chi }_T{\psi }_{n-1}+\rho ,{\chi }_T{\psi }_0=0,$$

we obtain the conclusion of Part 1. On the other hand, let us consider the proof of Part 2 in the following. The time evolution in $G_0$ obeys ${\chi }_S{\varphi }_n=E{\chi }_S{\varphi }_{n-1}$. The overlap of ${\chi }_S{\varphi }_n$ to the space ${\mathcal{H}}_s$ decreases more quickly than polynomial times because all the absolute values of the generalized eigenvalues of ${\mathcal{H}}_s$ are strictly less than 1 (see Proposition 4 for more detailed order of the convergence). Then, only the contribution of the centered eigenspace, whose eigenvalues lie on the unit circle in the complex plain, remains in the long time limit. □

Let $W=P_{c}E=E{P}_c=P_{c}E{P}_c$ be the operator restricted to the centered eigenspace ${\mathcal{H}}_c$. Then, we have

$$\underset{n\to \infty }{lim}\left|{\chi }_S{\varphi }_n\left(a\right)-W^n{\chi }_S{\varphi }_0\left(a\right)\right|=0$$

for any $a\in A_0$ uniformly by Proposition 3. This means that, in the long time limit, the time evolution is reduced to W, which is a unitary operator on ${\mathcal{H}}_c$.

Proposition 4.

The survival probability is re-expressed by

$$\gamma =\left|\right|P_c{\chi }_S{\varphi }_0{\left|\right|}^2.$$

The convergence speed ($f\left(n\right)=O\left(g\right(n\left)\right)$ means ${lim}_{n\to \infty }|f\left(n\right)/g\left(n\right)|<\infty$ if the limit exists) is estimated by $O\left(n^{\kappa }{r}_{max}^n\right)$, where $\kappa =dim{\mathcal{H}}_s$, $r_{max}=max\left\{\right|\lambda |;\lambda \in \mathrm{Spec}\left(E\right),\left|\lambda \right|<1\}$.

Proof. 

Putting $E(1-P_c)=W^{\prime }$, we have

$$W+W^{\prime }=E,W{W}^{\prime }=0,$$

by Proposition 2 (2). Note that the operator $E^n$ is similar to

$$\underset{\lambda \in \mathrm{Spec}\left(E\right)}{⨁}{J}^n(\lambda ;k_{\lambda })$$

with some natural numbers $k_{\lambda }$s. Here, $J(\lambda ;k)$ is the k-dimensional matrix by

$$J(\lambda ;k)=\left[\begin{array}{ccccc}\lambda & 1& & & \\ & \lambda & 1& & \\ & & \ddots & \ddots & \\ & & & \ddots & 1\\ & & & & \lambda \end{array}\right].$$

We obtain that the survival probability at each time n is described by

$$\begin{array}{cc}\hfill {\gamma }_n& =\left|\right|U_G{\chi }_S^{\ast }{E}^{n-1}{\chi }_S{\varphi }_0{\left|\right|}^2\hfill \\ \hfill & =\left|\right|U_G{\chi }_S^{\ast }(W^{n-1}+{W^{\prime }}^{n-1}){\chi }_S{\varphi }_0{\left|\right|}^2\hfill \\ \hfill & =\left|\right|(W^{n-1}+{W^{\prime }}^{n-1}){\chi }_S{\varphi }_0{\left|\right|}^2\hfill \\ \hfill & =\left|\right|W^{n-1}{\chi }_S{\varphi }_0{\left|\right|}^2+\left|\right|{W^{\prime }}^{n-1}{\chi }_S{\varphi }_0{\left|\right|}^2.\hfill \end{array}$$

In the third equality, we use the fact that $U_G$ is unitary; the last equality follows from Corollary 3. The second term decreases to zero by Proposition 2 (2) with the convergence speed at least $O\left(n^{\kappa }{r}_{max}^n\right)$ because the Jordan matrix $J(\lambda ;k)$ can be estimated by $J{(\lambda ;k)}^n=O(n^k{\left|\lambda \right|}^n)$. Hence, we find for ${\gamma }_n$

$$\begin{array}{cc}\hfill {\gamma }_n& =\left|\right|W^{n-1}{\chi }_S{\varphi }_0{\left|\right|}^2+O\left(n^{\kappa }{r}_{max}^n\right)(n>>1)\hfill \\ \hfill & =\left|\right|W^{n-1}{P}_c{\chi }_S{\varphi }_0{\left|\right|}^2+O\left(n^{\kappa }{r}_{max}^n\right)\hfill \\ \hfill & =\left|\right|P_c{\chi }_S{\varphi }_0{\left|\right|}^2+O\left(n^{\kappa }{r}_{max}^n\right),\hfill \end{array}$$

where in the second equality we use that $W=W{P}_c$ and the last equality follows from Proposition 2 (3). □

Therefore, the characterization of ${\mathcal{H}}_c$ is important to obtain the asymptotic behavior of ${\varphi }_n$.

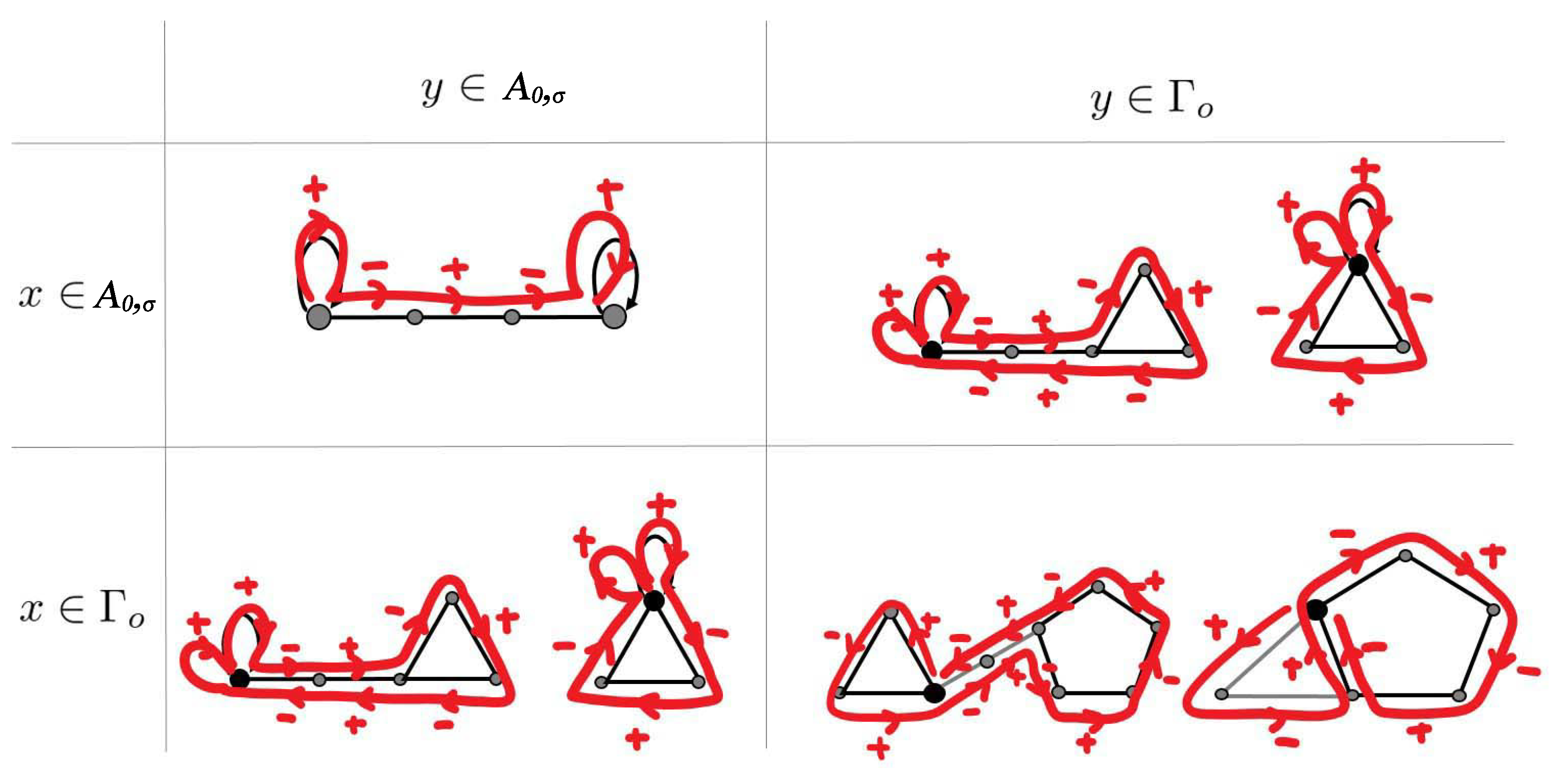
7.2. Characterization of Centered Generalized Eigenspace by Graph Notations

The centered generalized eigenspace of E can be rewritten by using the boundary operator $d_1$ and the self-adjoint operator $T=d_{1}S{d}_1^{\ast }$ as follows.

Lemma 3

([19]). Assume $\lambda \in \mathrm{Spec}\left(E\right)$ with $\left|\lambda \right|=1$. Then, we have

1. 

$\lambda =\pm 1$ if and only if $ker(\lambda -E)=ker(-\lambda -S)\cap ker{d}_1$.

2. 

$\lambda \ne \pm 1$ if and only if supp$\left(g\right)\subset V_0\setminus \delta V_0$ for any $g\in ker((\lambda +{\lambda }^{-1})/2-T)\ne 0$.

In the following, we consider the characterization of $ker(\pm 1-E)$ using some walks on graph $G_0$ up to the situations of the graph (Cases (A)–(D)). First, we prepare the following notations. For each support edge $e\in E_0$, there are two arcs a and $\overline{a}$ such that $\left|a\right|=|\overline{a}|$. Let us choose one of the arcs from each $e\in E_0$ and denote $A_{+}$ as the set of selected arcs. Then, $|A_{+}|=|E_0|$ and $a\in A_{+}$ if and only if $\overline{a}\notin A_{+}$ holds. We set $A_{rep}=A_{0,\sigma }\cup A_{+}$. Let us introduce the map $\iota :{\mathbb{C}}^{A_0}\to {\mathbb{C}}^{A_{rep}}$ defined by $\left(\iota \psi \right)\left(a\right)=\psi \left(a\right)$ for any $\psi \in {\mathbb{C}}^{A_0}$ and $a\in A_{rep}$.

Let us define the boundary operator ${\partial }_{+}:{\mathbb{C}}^{A_{rep}}\to {\mathbb{C}}^{V_0}$ by

$$\left({\partial }_{+}\phi \right)\left(u\right)=\sum _{t\left(a\right)=u\mathrm{in}{A}_{+}}\phi \left(a\right)-\sum _{o\left(a\right)=u\mathrm{in}{A}_{+}}\phi \left(a\right)$$

for any $\phi \in {\mathbb{C}}^{A_{rep}}$ and $u\in V_0$. On the other hand, let us also define the boundary operator ${\partial }_{-}:{\mathbb{C}}^{A_{rep}}\to {\mathbb{C}}^{V_0}$ by

$$\left({\partial }_{-}\phi \right)\left(u\right)=\left\{\begin{array}{cc}{\displaystyle \sum _{t\left(a\right)=u}}\phi \left(a\right)+{\displaystyle \sum _{o\left(a\right)=u}}\phi \left(a\right)\hfill & :u\mathrm{has}\mathrm{no}\mathrm{selfloop},\hfill \\ {\displaystyle \sum _{t\left(a\right)=u}}\phi \left(a\right)+{\displaystyle \sum _{o\left(a\right)=u}}\phi \left(a\right)-\phi \left(a_s\right)\hfill & :u\mathrm{has}\mathrm{a}\mathrm{selfloop}{a}_s,\hfill \end{array}\right.$$

for any $\phi \in {\mathbb{C}}^{A_{rep}}$ and $u\in V_0$. We obtain the following lemma.

Lemma 4.

Let $G_0=(V_0,A_0)$ be a graph with self-loops. We set $E_0$ as the set of support edges of $A_0\setminus A_{0,\sigma }$ such that $E_0=\left\{\right|a\left|\right|a\in A_0\setminus A_{0,\sigma }\}$. Then, we have

$$dim[ker(1-E)]=|E_0|-|V_0|+1,$$

$$dim[ker(1+E)]=\left\{\begin{array}{cc}|E_0|-|V_0|+1\hfill & :\mathit{Case}A,\hfill \\ |E_0|-|V_0|\hfill & :\mathit{Case}B,\hfill \\ |E_0|-|V_0|+|A_{0,\sigma }|\hfill & :\mathit{Cases}C\mathit{and}D,\hfill \end{array}\right.$$

Proof. 

Note that, if $\psi \in ker(1+S)$, then $\psi \left(\overline{a}\right)=-\psi \left(a\right)$ for any $a\in A_{+}$, and, if $\psi \in ker\left(d\right)$, then ${\sum }_{t\left(a\right)=u}\psi \left(a\right)=0$ for any $u\in V_0$. We remark that, since $\left(S\psi \right)\left(a_s\right)=\psi \left(a_s\right)$ for any $a_s\in A_{0,\sigma }$, we have $\psi \left(a_s\right)=0$ if $\psi \in ker(1+S)$. Therefore, if $\psi \in ker(1+S)\cap ker\left(d\right)$, then

$$\sum _{t\left(a\right)=u\mathrm{in}{A}_{+}}\left(\iota \psi \right)\left(a\right)-\sum _{o\left(a\right)=u\mathrm{in}{A}_{+}}\left(\iota \psi \right)\left(a\right)=\left({\partial }_{+}\iota \psi \right)\left(u\right)=0$$

holds. Then, $ker(1+S)\cap kerd$ is isomorphic to $\{\phi \in ker{\partial }_{+}|supp\left(\phi \right)\subset A_{+}\}$. Let us consider $ker{\partial }_{+}$. By the definition of ${\partial }_{+}$, we have ${\partial }_{+}{\delta }_a^{\left(A_{rep}\right)}=0$ for any $a\in A_s$. Hence, we should eliminate the subspace of $ker{\partial }_{+}$ induced by the self-loops. The dimension of this subspace is $|A_{0,\sigma }|$. The adjoint operator ${\partial }_{+}^{\ast }:{\mathbb{C}}^{V_0}\to {\mathbb{C}}^{A_{+}}$ of ${\partial }_{+}$ is described by

$$\left({\partial }_{+}^{\ast }f\right)\left(a\right)=f\left(t\left(a\right)\right)-f\left(o\left(a\right)\right),$$

for any $f\in {\mathbb{C}}^{V_0}$ and $a\in A_{rep}$. If ${\partial }_{+}^{\ast }f=0$ holds, then $f\left(t\right(a\left)\right)=f\left(o\right(a\left)\right)$ for any $a\in A_{+}$. This means $f\left(u\right)=c$ for any $u\in V_0$ with some non-zero constant c. Thus, $dimker\left({\partial }_{+}^{\ast }\right)=1$. Therefore, the fundamental theorem of linear algebra (for a linear map $g:X\to Y$, $dimkerg=dimX-dimY+dimker{g}^{\ast }$) implies

$$\begin{array}{cc}\hfill dimker(1+S)\cap kerd& =dimker\left({\partial }_{+}\right)-\left|A_{0,\sigma }\right|\hfill \\ \hfill & =\left(\right|A_{rep}|-|V_0|+1)-|A_{0,\sigma }|\hfill \\ \hfill & =|E_0|-|V_0|+1.\hfill \end{array}$$

Next, let us consider $dim(ker(1-S)\cap ker{d}_1)$. Note that, if $\psi \in ker(1-S)$, then $\psi \left(\overline{a}\right)={\psi }_{(}a)$. Assume that $\psi \in ker(1-S)\cap ker\left(d_1\right)$; then,

$$\sum _{t\left(a\right)=u}\left(\iota \psi \right)\left(a\right)=0\mathrm{for}\mathrm{any}u\in V_0,$$

which is equivalent to

$${\partial }_{-}\iota \psi =0.$$

The adjoint of ${\partial }_{-}$ is described by

$$\left({\partial }_{-}^{\ast }f\right)\left(a\right)=\left\{\begin{array}{cc}f\left(t\right(a\left)\right)+f\left(o\right(a\left)\right)\hfill & :a\in A_{+},\hfill \\ f\left(t\right(a\left)\right)\hfill & :a\in A_{0,\sigma }.\hfill \end{array}\right.$$

Let us consider $f\in ker\left({\partial }_{-}^{\ast }\right)$ in the cases for both $A_{0,\sigma }=\varnothing$ and $A_{0,\sigma }\ne \varnothing$.

$A_{0,\sigma }=\varnothing$ case:

If $G_0$ is a bipartite graph, then we can decompose the vertex set V into $X\cup Y$, where every edge connects a vertex in X to one in Y. Then, $f\left(x\right)=k$ for any $x\in X$ and $f\left(y\right)=-k$ for any $y\in Y$ with some nonzero constant k. Hence, $dimker\left({\partial }^{\ast }\right)=1$ if $A_{0,\sigma }=\varnothing$ and $G_0$ is bipartite. On the other hand, if $G_0$ is non-bipartite, then there must exist an odd length fundamental cycle $c=(a_0,a_1,\cdots ,a_{2m})$. We have that

$$f\left(o\left(a_1\right)\right)=-f\left(o\left(a_2\right)\right)=f\left(o\left(a_3\right)\right)=\cdots =-f\left(o\left(a_{2r}\right)\right)=f\left(o\left(a_0\right)\right)=-f\left(o\left(a_1\right)\right).$$

Then, $f\left(u\right)=0$ for any $u\in V\left(c\right)$. Since $G_0$ is connected, the value 0 is inherited to the other vertices by $f\left(t\right(a\left)\right)=-f\left(o\right(a\left)\right)$. After all, we have $f=0$, which implies $ker\left({\partial }_{-}^{\ast }\right)=0$ if $A_{0,\sigma }=\varnothing$ and $G_0$ is non-bipartite.

$A_{0,\sigma }\ne \varnothing$ case: Since $\left({\partial }_{-}^{\ast }f\right)\left(a\right)=f\left(t\left(a\right)\right)=0$ if $a\in A_{0,\sigma }$, then f takes the value 0 at the other vertices since $f\left(t\right(a\left)\right)=-f\left(o\right(a\left)\right)$ for any $a\in A_{+}$, which implies $ker\left({\partial }_{+}^{\ast }\right)=0$ if $A_{0,\sigma }\ne \varnothing$.

After all, by the fundamental theorem of the linear algebra,

$$\begin{array}{cc}\hfill dimker{\partial }_{-}& =|A_{rep}|-|V_0|+\left\{\begin{array}{cc}1\hfill & :A_s=\varnothing ,G_0\mathrm{is}\mathrm{bipartite}.\hfill \\ 0\hfill & :\mathrm{otherwise}.\hfill \end{array}\right.\hfill \end{array}$$

Noting that $|A_{rep}|=|E_0|+|A_{0,\sigma }|$, we obtain the desired conclusion. □

In the following, let us find linearly independent eigenfunctions of $ker(\pm 1-E)$ using some concepts from graph theory. A walk p in $G_0$ is a sequence $p=(a_0,a_1,\cdots ,a_r)$ of arcs with $t\left(a_j\right)=o\left(a_{j+1}\right)$ ($j=0,1,\cdots ,r-1$), which may contain repeated arcs as defined in Section 2.1. We set $\{a_0,a_1,\cdots ,a_r\}=:A\left(p\right)$, and similarly $\overline{A}\left(p\right)=\{{\overline{a}}_0,\cdots ,{\overline{a}}_r\}$ as multi sets.

We describe ${\tilde{\xi }}_p^{(\pm )}:\{a_0,\cdots ,a_r\}\cup \{{\overline{a}}_0,\cdots ,{\overline{a}}_r\}\to \{\pm 1\}$ by

$${\tilde{\xi }}_p^{(+)}\left(a\right)=\left\{\begin{array}{cc}1\hfill & :a\in A\left(p\right),\hfill \\ -1\hfill & :\overline{a}\in A\left(p\right),\hfill \end{array}\right.$$

$${\tilde{\xi }}_p^{(-)}\left(a\right)=\left\{\begin{array}{cc}1\hfill & :\left|a\right|\in \left\{\right|a_j\left|\right|j\mathrm{is}\mathrm{even}\},\hfill \\ -1\hfill & :\left|a\right|\in \left\{\right|a_j\left|\right|j\mathrm{is}\mathrm{odd}\}.\hfill \end{array}\right.$$

Then, we set the functions ${\xi }_p^{(\pm )}\in {\mathbb{C}}^A$ by

$${\xi }_p^{(\pm )}\left(a\right)=\left\{\begin{array}{cc}\sum _{b:a=b}{\tilde{\xi }}_p^{(\pm )}\left(b\right)\hfill & :a\in A\left(p\right)\cup \overline{A}\left(p\right),\hfill \\ 0\hfill & :\mathrm{otherwise}.\hfill \end{array}\right.$$

(6)

Now, we are ready to show the following proposition for $ker(1-E)$.

Proposition 5.

Let ${\xi }_c^{(+)}$ be defined as (6). Then, we have

$$ker(1-E)=\mathrm{span}\left\{{\xi }_c^{(+)}\right|c\in \Gamma \}.$$

Proof. 

By the definition of ${\xi }_c^{(+)}$, we have ${\xi }_c^{(+)}\in ker{d}_1\cap ker(1-S)$, which implies ${\xi }_c^{(+)}\in ker(1-E)$ by Lemma 3. We show the linear independence of ${\left\{{\xi }_c^{(+)}\right\}}_{c\in \Gamma }$. Let us set $\Gamma =\{c_1,\cdots ,c_r\}$ and ${\xi }_j:={\xi }_{c_j}^{(+)}$ ($j=1,\cdots ,r$) induced by the spanning tree $\mathbb{T}\subset G$. Assume that

$${\beta }_1{\xi }_1+\cdots +{\beta }_r{\xi }_r=0.$$

Put $a_r\in A_0\left(c_r\right)\cap (A_0\setminus A\left(\mathbb{T}\right))$. From the definition of the fundamental cycle, we have

$${\beta }_1{\xi }_1\left(a_r\right)+\cdots +{\beta }_r{\xi }_r\left(a_r\right)={\beta }_r=0.$$

In the same way, let $a_{r-1}\in A\left(c_{r-1}\right)\cap (A_0\setminus A\left(\mathbb{T}\right))$; then,

$${\beta }_1{\xi }_1\left(a_r\right)+\cdots +{\beta }_{r-1}{\xi }_{r-1}\left(a_{r-1}\right)={\beta }_{r-1}=0.$$

Then, using it recursively, we obtain ${\beta }_1=\cdots ={\beta }_r=0$, which means ${\xi }_j$s are linearly independent.

Then, $dim\left(\mathcal{K}\right)=|\Gamma |=|E_0|-|V_0|+1$. By Lemma 4, we reach the conclusion. □

Define ${\Gamma }_o,{\Gamma }_e\subset \Gamma$ as the set of odd and even length fundamental cycles. In the following, to obtain a characterization of $ker(1+E)=ker(1-S)\cap ker\left(d_1\right)$, we construct the function ${\eta }_{x,y}\in ker(1-S)\cap ker\left(d_1\right)$, which is determined by $x,y\in A_{0,\sigma }\cup {\Gamma }_o$. The main idea to construct such a function is as follows. By the definition of ${\xi }_q^{(-)}$ for any walk q, ${\xi }_q^{(-)}\in ker(1-S)$. This is equivalent to assigning the symbols “+” and “−” alternatively to each edge along the walk q. If the walk c is an even length cycle, then a symbol on each edge of c is different from the ones on the neighbor’s edges; this means

$$\sum _{t\left(a\right)=u}{\xi }_c^{(-)}\left(a\right)=0,$$

for every u. Then, ${\xi }_c^{(-)}\in ker\left(d_1\right)\cap ker(1-S)$ holds. On the other hand, if the walk $c=(b_1,\cdots ,b_r)$ is an odd length cycle, then a “frustration” appears at $u:=o\left(b_1\right)$; i.e.,

$$\sum _{t\left(a\right)=u}{\xi }_c^{(-)}\left(a\right)=2.$$

There are two ways to vanish this frustration: the first is to make a cancellation by another frustration induced by another odd cycle $c^{\prime }$ and the second is to push the frustration to a self-loop. That is the reason the domains of x and y are $A_{0,\sigma }\cup {\Gamma }_o$. We give more precise explanations of the constructions as follows. See also Figure 3.

Definition 2.

Construction of${\eta }_{x,y}\in {\mathbb{C}}^{A_0}$:

The function ${\eta }_{x,y}$ is described by ${\xi }_q^{(-)}$ induced by a walk depending on the indexes of $x,y$. In this paper, we consider four cases of the domains of x and y: (1) $x\in {\Gamma }_o$, $y\in {\Gamma }_o$; (2) $x\in A_{\sigma }$, $y\in A_{\sigma }$; (3) $x\in A_{\sigma }$, $y\in {\Gamma }_o$; and (4) $x\in {\Gamma }_o$, $y\in A_{\sigma }$.

1. 

$x\in {\Gamma }_o$, $y\in {\Gamma }_o$ case:

If $G_0$ is a bipartite graph, let us fix an odd length fundamental cycle $c_{\ast }=(a_0,\cdots ,a_{r-1})\in {\Gamma }_o$ and pick up another $c\in {\Gamma }_o=(b_0,\cdots ,b_{s-1})$. We set the following walk q and define the function on ${\mathbb{C}}^{A_0}$; ${\xi }_q^{(-)}=:\overline{a}{a}_{c_{\ast }-c}$, induced by $c_{\ast },c\in {\Gamma }_o$:

(a) 

$c_0\cap c\ne \varnothing$ case: We set q as the shortest closed walk starting from a vertex $u_0\in V\left(c_0\right)\cap V\left(c\right)$ and visiting all the vertices of $V\left(c_0\right)$ and $V\left(c\right)$; that is, $q=(a_i,\cdots ,a_{i+r},b_j,\cdots ,b_{s+j})$. Here, $o\left(a_i\right)=o\left(b_j\right)=u_0$ and the suffices are modulus of r and s.

(b) 

$c_0\cap c=\varnothing$ case: Let us fix the shortest path between $c_0$ and c by $p=(p_1,\cdots ,p_t)$. Denoting the vertex in $V\left(c_{\ast }\right)$ connecting to p by $u_0\in V\left(c_{\ast }\right)$, we set q by the shortest closed walk q starting from $u_{\ast }$ and visiting all the vertices; that is, $q=(a_i,\cdots ,a_{r+i},p_0\cdots ,p_t,b_j\cdots ,b_{s+j},{\overline{p}}_t\cdots ,{\overline{p}}_1)$, where $o\left(a_i\right)=t\left(a_{r+i}\right)=o\left(p_1\right)=u_0$, $t\left(p_t\right)=o\left(b_j\right)=t\left(b_{s+j}\right)$.

Note that, by the definition of the fundamental cycle, the intersection $c_0\cap c$ is a path in Case (1). Since $G_0$ is connected, there is a path connecting $c_{\ast }$ to c and we fix such a path for every pair of $(c_{\ast },c)$ in Case (2).

2. 

$x\in A_{\sigma }$ and $y\in A_{\sigma }$ case:

If the number of self-loops $|A_{\sigma }|\ge 2$, let us fix a self-loop $a_{\ast }$ from $A_{\sigma }$ and a path between $a_{\ast }$ to each $a\in A_{\sigma }\setminus \left\{a_{\ast }\right\}$. Let us denote the path between $a_{\ast }$ and a by $p=(p_1,\cdots ,p_t)$. Then, we set the walk from $a_{\ast }$ to a by $q=(a_{\ast },p_1,\cdots ,p_t,a)$ and ${\xi }_q^{(-)}=:{\eta }_{a_{\ast }-a}$.

3. 

$x\in A_{\sigma }$ and $y\in {\Gamma }_o$ case:

If $|A_{\sigma }|\ge 1$ and $G\setminus A_{\sigma }$ is a non-bipartite graph, let us fix a self-loop $a_{\ast }$ and pick up an odd cycle $c=(b_1,\cdots ,b_t)\in {\Gamma }_o$; if the self-loop $o\left(a_{\ast }\right)\in V\left(c\right)$, we set the walk starting from $a_{\ast }$ visiting all the vertices $V\left(c\right)$ and returning back to $a_{\ast }$ by $q=(a_{\ast },b_1,\cdots ,b_t,a_{\ast })$; and, for $o\left(a_{\ast }\right)\notin V\left(c\right)$, let us fix a path $p=(p_1,\cdots ,p_t)$ between $o\left(a_{\ast }\right)$ and $o\left(b_1\right)$ and set the walk starting from $a_{\ast }$ visiting all the vertices $V\left(p\right)\cup V\left(c\right)$ and returning back to $a_{\ast }$; $q=(a_{\ast },p_1,\cdots ,p_t,b_0\cdots ,b_t,{\overline{p}}_t,\cdots ,{\overline{p}}_1,a_{\ast })$. Then, we set ${\xi }_q^{(-)}=:{\eta }_{a_{\ast },c}$.

4. 

$x\in {\Gamma }_o$ and $y\in A_{\sigma }$ case:

Let us fix an odd length fundamental cycle $c_{\ast }\in {\Gamma }_o=(b_1,\cdots ,b_{s-1})$ and pick up a self-loop $a\in A_{\sigma }$. Let us set a short length path p between $o\left(a\right)$ and $o\left(b_1\right)$. Then, we consider the same walk q as in Case (3) and set ${\xi }_q^{(-)}=:{\eta }_{c_{\ast },a}.$

By the construction, we have ${\eta }_{x,y}\in ker(1-S)\cap ker\left(d_1\right)$. Using the function ${\eta }_{x,y}$, we obtain the following characterization of $ker(-1-E)$.

Proposition 6.

Let ${\xi }_c^{(-)}$ be defined by (6) and ${\eta }_{x,y}$ be the above. Let us fix $a_{\ast }\in A_{\sigma }$ and $c_{\ast }\in {\Gamma }_o$. Then, we have

$$ker(1+E)=\left\{\begin{array}{cc}\mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in \Gamma \}\hfill & :\mathit{Case}\left(A\right),\hfill \\ \mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in {\Gamma }_e\}\oplus \mathrm{span}\left\{{\eta }_{c_{\ast }-c}\right|c\in {\Gamma }_o\setminus \left\{c_{\ast }\right\}\}\hfill & :\mathit{Case}\left(B\right),\hfill \\ \mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in \Gamma \}\oplus \mathrm{span}\left\{{\eta }_{a_{\ast }-a}\right|a\in A_{0,\sigma }\setminus \left\{a_{\ast }\right\}\}\hfill & :\mathit{Case}\left(C\right),\hfill \\ \mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in {\Gamma }_e\}\oplus \mathrm{span}\left\{{\eta }_{a_{\ast }-y}\right|y\in {\Gamma }_o\cup (A_{0,\sigma }\setminus \left\{a_{\ast }\right\})\}\hfill & :\mathit{Case}\left(D\right).\hfill \end{array}\right.$$

Proof. 

We put

$$\begin{array}{cc}\hfill \mathcal{A}& :=\mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in \Gamma \},\hfill \end{array}$$

(7)

$$\begin{array}{cc}\hfill \mathcal{B}& :=\mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in {\Gamma }_e\}\oplus \mathrm{span}\left\{{\eta }_{c_{\ast }-c}\right|c\in {\Gamma }_o\setminus \left\{c_{\ast }\right\}\},\hfill \end{array}$$

(8)

$$\begin{array}{cc}\hfill \mathcal{C}& :=\mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in \Gamma \}\oplus \mathrm{span}\left\{{\eta }_{a_{\ast }-a}\right|a\in A_{0,\sigma }\setminus \left\{a_{\ast }\right\}\},\hfill \end{array}$$

(9)

$$\begin{array}{cc}\hfill \mathcal{D}& :=\mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in {\Gamma }_e\}\oplus \mathrm{span}\left\{{\eta }_{a_{\ast }-y}\right|y\in {\Gamma }_o\cup (A_{0,\sigma }\setminus \left\{a_{\ast }\right\})\}\hfill \end{array}$$

(10)

(see also Figure 4). From the construction of ${\eta }_{x,y}$ and ${\xi }_c^{(-)}$, the linear independence is immediately obtained. Let us check the dimensions for each case.

In Case (A),

$$dim\left(\mathcal{A}\right)=|\Gamma |=|E_0|-|V_0|+1.$$

In Case (B),

$$dim\left(\mathcal{B}\right)=|{\Gamma }_e|+(|{\Gamma }_o|-1)=|E_0|-|V_0|.$$

In Case (C),

$$dim\left(\mathcal{C}\right)=|\Gamma |+\left(\right|A_{0,\sigma }|-1)=|E_0|-|V_0|+|A_{0,\sigma }|.$$

In Case (D),

$$dim\left(\mathcal{D}\right)=|{\Gamma }_e|+(|{\Gamma }_o|-1)+\left(\right|A_{0,\sigma }|-1)=|E_0|-|V_0|+|A_{0,\sigma }|.$$

By Lemma 4, we reach the conclusion. □

Remark 5.

“$M\oplus N$” in Proposition 6 means that M and N are just complementary spaces; the orthogonality is not ensured in general.

Remark 6.

If $|{\Gamma }_o|=1$ in Case (B), we have $\mathcal{B}=\mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in {\Gamma }_e\}$. If $|A_{0,\sigma }|=1$ in Case (C), we have $\mathcal{C}=\mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in \Gamma \}$.

Remark 7.

The subspace $\mathcal{D}$ can be re-expressed by

$$\mathcal{D}=\mathrm{span}\left\{{\xi }_c^{(-)}\right|c\in {\Gamma }_e\}\oplus \mathrm{span}\left\{{\eta }_{c_{\ast }-y}\right|y\in ({\Gamma }_o\setminus \left\{c_{\ast }\right\})\cup A_{0,\sigma }\}.$$

8. Conclusions

We investigated the Grover walk on a finite graph G with sinks using its connection with the walk on the graph $G_0$ with tails. It was shown that the centered generalized eigenspace of the Grover walk with tails corresponds to the attractor space of the Grover walk with sinks, i.e., it contains all trapped states which do not contribute to the transport of the quantum walker into the sink. Consequently, the attractor space of the Grover walk with sinks can be characterized using the persistent eigenspace of the underlying random walk whose supports have no overlaps to the boundary and the concept of “flow” from graph theory. In particular, we constructed linearly independent basis vectors of the attractor space using the properties of fundamental cycles of $G_0$. The attractor space can be divided into subspaces $\mathcal{T}$ and $\mathcal{K}$, corresponding to the eigenvalues $\lambda \ne \pm 1$ and $\lambda =1$, respectively, and an additional subspace which belongs to the eigenvalue $\lambda =-1$. While the basis of $\mathcal{T}$ and $\mathcal{K}$ can be constructed using the same procedure for all finite connected graphs $G_0$, for the last subspace, we provided a construction based on case separation, depending on if the graph is bipartite or not and if it involves self-loops.

The use of fundamental cycles allowed us to considerably expand the results previously found in the literature, which are often limited to planar graphs. The derived construction of the attractor space enables better understanding of the quantum transport models on graphs. In addition, our results reveal that the attractor space can contain subspaces of eigenvalues different from $\lambda =\pm 1$. In such a case, the evolution of the Grover walk with sink will have more complex asymptotic cycle. In fact, the example presented in Section 5 exhibits an infinite asymptotic cycle, since the phase $\theta$ of the eigenvalues ${\lambda }_{\pm }\ne \pm 1$ is not a rational multiple of $\pi$. This feature is missing, e.g., in the Grover walk on dynamically percolated graphs with sinks, where the evolution converges to a steady state.