Novel Approach for Third-Party Reverse Logistic Provider Selection Process under Linear Diophantine Fuzzy Prioritized Aggregation Operators
Abstract
:1. Introduction
2. Preliminaries
- (1)
- ;
- (2)
- ;
- (3)
- .
- (i) :
- If then ,
- (ii) :
- If then ,
- (iii) :
- If then,
- (a) :
- If then ,
- (b) :
- If then ,
- (c) :
- If then .
- ;
- ;
- ;
- .
- ;
- ;
- .
3. Linear Diophantine Fuzzy Prioritized Aggregation Operators
3.1. LDFPWA Operator
- 1 .
- 2 .
- 3 .
- 4 .
3.2. LDFPWG Operator
- 1 .
- 2 .
- 3 .
- 4 .
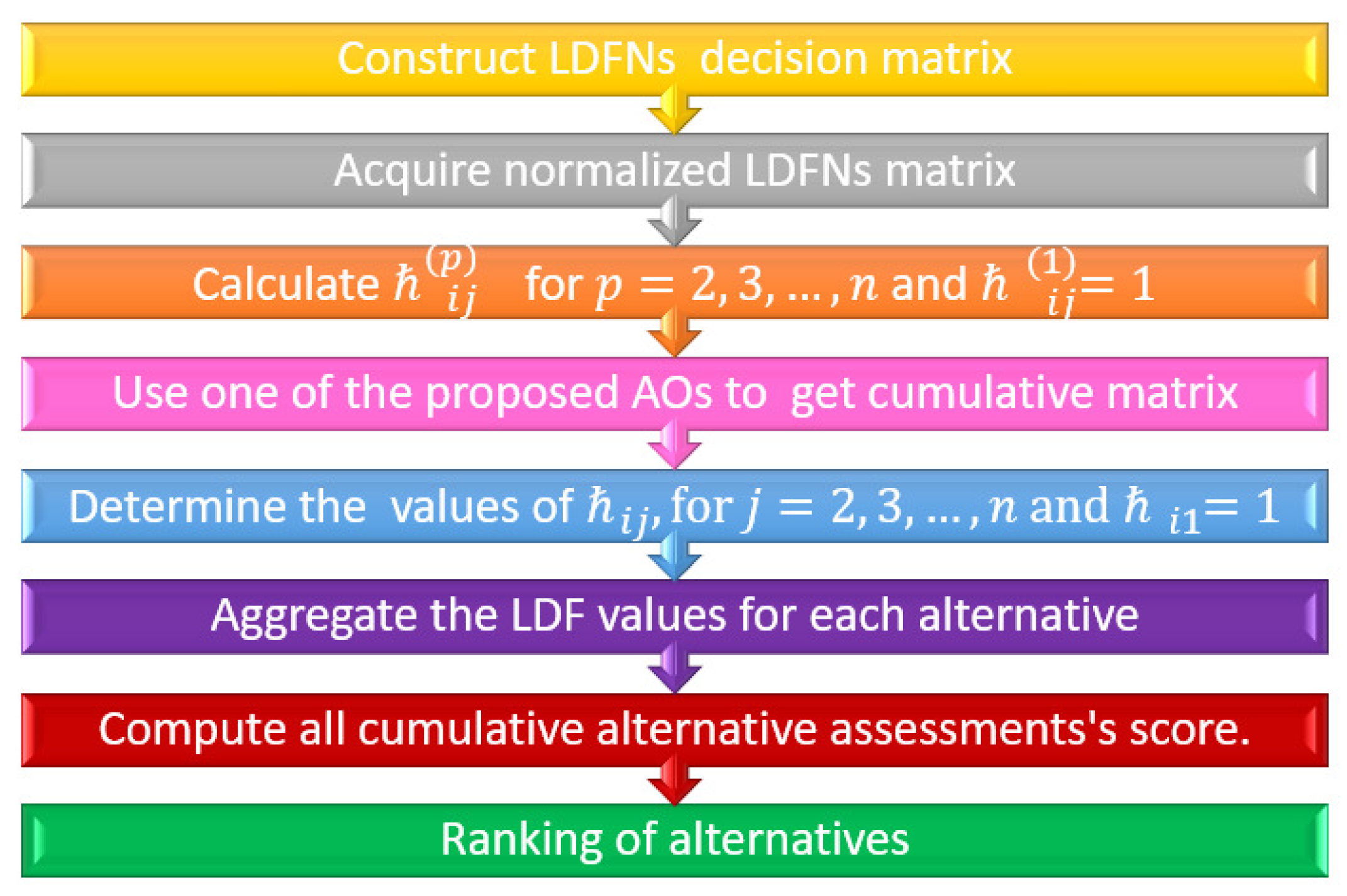
4. Proposed Methodology
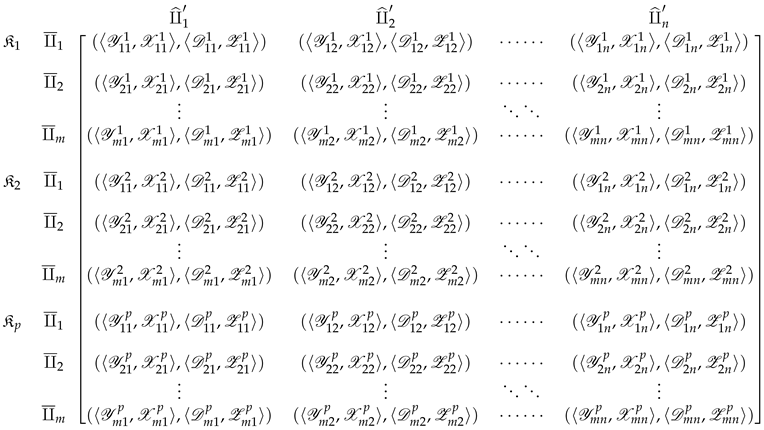
5. Case Study
5.1. Numerical Example

5.2. Comparative Analysis
6. Conclusions and Future Studies
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef] [Green Version]
- Atanassov, K.T. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Atanassov, K.T. Geometrical interpretation of the elemets of the intuitionistic fuzzy objects. Int. J. Bioautom. 1986, 20 (Suppl. 1), S27–S42. [Google Scholar]
- Yager, R.R.; Abbasov, A.M. Pythagorean membership grades, complex numbers, and decision making. Int. J. Intell. Syst. 2013, 28, 436–452. [Google Scholar] [CrossRef]
- Yager, R.R. Pythagorean fuzzy subsets. In Proceedings of the IFSA World Congress and NAFIPS Annual Meeting (IFSA/NAFIPS), Edmonton, AB, Canada, 24–28 June 2013; pp. 57–61. [Google Scholar]
- Yager, R.R. Pythagorean membership grades in multi criteria decision-making. IEEE Trans. Fuzzy Syst. 2014, 22, 958–965. [Google Scholar] [CrossRef]
- Yager, R.R. Generalized orthopair fuzzy sets. IEEE Trans. Fuzzy Syst. 2017, 25, 1222–1230. [Google Scholar] [CrossRef]
- Alcantud, J.C.R.; Garcia, G.S. A new criterion for soft set based decision making problems under incomplete information. Int. J. Comput. Intell. Syst. 2017, 10, 394–404. [Google Scholar] [CrossRef] [Green Version]
- Xu, Z.S. Intuitionistic fuzzy aggregation operators. IEEE Transections Fuzzy Syst. 2007, 15, 1179–1187. [Google Scholar]
- Xu, Z.S.; Yager, R.R. Some geometric aggregation operators based on intuitionistic fuzzy sets. Int. J. Gen. Syst. 2006, 35, 417–433. [Google Scholar] [CrossRef]
- Hashmi, M.R.; Riaz, M.; Smarandache, F. m-polar Neutrosophic Topology with Applications to Multi-Criteria Decision-Making in Medical Diagnosis and Clustering Analysis. Int. J. Fuzzy Syst. 2020, 22, 273–292. [Google Scholar] [CrossRef]
- Wang, W.; Liu, X. Intuitionistic fuzzy information aggregation using Einstein operators. IEEE Transections Fuzzy Syst. 2012, 20, 923–938. [Google Scholar] [CrossRef]
- Zhang, H.Y.; Wang, J.Q.; Chen, X.H. Interval neutrosophic sets and their applications in multi-criteria decision making problems. Sci. World J. 2014, 2014, 645953. [Google Scholar]
- Zhao, H.; Xu, Z.S.; Ni, M.F.; Lui, S.S. Generalized aggregation operators for intuitionistic fuzzy sets. Int. J. Intell. Syst. 2010, 25, 1–30. [Google Scholar] [CrossRef]
- Garg, H. A new generalized Pythagorean fuzzy information aggregation using Einstein operators and its applications to decision-making. Int. J. Intell. Syst. 2016, 31, 886–920. [Google Scholar] [CrossRef]
- Mahmood, T.; Ullah, K.; Khan, Q.; Jan, N. An approach toward decision-making and medical diagnosis problems using the concept of spherical fuzzy sets. Neural Comput. Appl. 2019, 31, 7041–7053. [Google Scholar] [CrossRef]
- Feng, F.; Liang, M.; Fujita, H.; Yager, R.R.; Liu, X. Lexicographic orders of intuitionistic fuzzy values and their relationships. Mathematics 2019, 7, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Akram, M.; Yaqoob, N.; Ali, G.; Chammam, W. Extensions of Dombi aggregation operators for decision making under m-polar fuzzy information. J. Math. 2020, 6, 1–20. [Google Scholar] [CrossRef]
- Akram, M.; Shahzadi, G.; Peng, X. Extension of Einstein Geometric Operators to Multi-Attribute Decision Making under q-Rung Orthopair Fuzzy Information. Available online: https://link.springer.com/article/10.1007 (accessed on 20 May 2021).
- Peng, X.D.; Yang, Y. Some results for Pythagorean fuzzy sets. Int. J. Intell. Syst. 2015, 30, 1133–1160. [Google Scholar] [CrossRef]
- Zhang, X.L.; Xu, Z.S. Extension of TOPSIS to multiple criteria decision making with Pythagorean fuzzy sets. Int. J. Intell. Syst. 2014, 29, 1061–1078. [Google Scholar] [CrossRef]
- Karaaslan, F.; Ozlu, S. Correlation coefficients of dual type-2 hesitant fuzzy sets and their applications in clustering analysis. Int. J. Intell. Syst. 2020, 35, 1200–1229. [Google Scholar] [CrossRef]
- Sitara, M.; Akram, M.; Riaz, M. Decision-making analysis based on q-rung picture fuzzy graph structures. J. Appl. Math. Comput. 2021. [Google Scholar] [CrossRef]
- Riaz, M.; Hashmi, M.R. Linear Diophantine fuzzy set and its applications towards multi-attribute decision making problems. J. Intell. Fuzzy Syst. 2019, 37, 5417–5439. [Google Scholar] [CrossRef]
- Kamaci, H. Linear Diophantine fuzzy algebraic structures. J. Ambient. Intell. Humaniz. Comput. 2021. [Google Scholar] [CrossRef]
- Ayub, S.; Shabir, M.; Riaz, M.; Aslam, M.; Chinram, R. Linear Diophantine fuzzy relations and their algebraic properties with decision making. Symmetry 2021, 13, 945. [Google Scholar] [CrossRef]
- Almagrabi, A.O.; Abdullah, S.; Shams, M. A new approach to q-linear Diophantine fuzzy emergency decision support system for COVID19. J. Ambient. Intell. Humanoized Comput. 2021. [Google Scholar] [CrossRef]
- Pamucar, D. Normalized weighted Geometric Dombi Bonferoni Mean Operator with interval grey numbers: Application in multicriteria decision making. Rep. Mech. Eng. 2020, 1, 44–52. [Google Scholar] [CrossRef]
- Pamucar, D.; Jankovic, A. The application of the hybrid interval rough weighted Power-Heronian operator in multi-criteria decision making. Oper. Res. Eng. Sci. Theory Appl. 2020, 3, 54–73. [Google Scholar] [CrossRef]
- Riaz, M.; Salabun, W.; Farid, H.M.A.; Ali, N.; Watróbski, J. A robust q-rung orthopair fuzzy information aggregation using Einstein operations with application to sustainable energy planning decision management. Energies 2020, 13, 2125. [Google Scholar] [CrossRef]
- Riaz, M.; Pamucar, D.; Farid, H.M.A.; Hashmi, M.R. q-Rung Orthopair Fuzzy Prioritized Aggregation Operators and Their Application Green Supplier Chain Management. Symmetry 2020, 12, 976. [Google Scholar] [CrossRef]
- Riaz, M.; Farid, H.M.A.; Karaaslan, F.; Hashmi, M.R. Some q-rung orthopair fuzzy hybrid aggregation operators and TOPSIS method for multi-attribute decision-making. J. Intell. Fuzzy Syst. 2020, 39, 1227–1241. [Google Scholar] [CrossRef]
- Riaz, M.; Farid, H.M.A.; Kalsoom, H.; Pamucar, D.; Chu, Y.M. A Robust q-rung orthopair fuzzy Einstein prioritized aggregation operators with application towards MCGDM. Symmetry 2020, 12, 1058. [Google Scholar] [CrossRef]
- Riaz, M.; Garg, H.; Farid, H.M.A.; Chinram, R. Multi-criteria decision making based on bipolar picture fuzzy operators and new distance measures. Comput. Model. Eng. Sci. 2021, 127, 771–800. [Google Scholar] [CrossRef]
- Yager, R.R. Prioritized aggregation operators. Int. J. Approx. Reason. 2008, 48, 263–274. [Google Scholar] [CrossRef] [Green Version]
- Ali, Z.; Mahmood, T.; Ullah, K.; Khan, Q. Einstein Geometric Aggregation Operators using a Novel Complex Interval-valued Pythagorean Fuzzy Setting with Application in Green Supplier Chain Management. Rep. Mech. Eng. 2021, 2, 105–134. [Google Scholar] [CrossRef]
- Ramakrishnan, K.R.; Chakraborty, S. A cloud TOPSIS model for green supplier selection. Facta Univ. Ser. Mech. Eng. 2020, 18, 375–397. [Google Scholar]
- Petrovic, G.; Mihajlovic, J.; Cojbasic, Z.; Madic, M.; Marinkovic, D. Comparison of three fuzzy MCDM methods for solving the supplier selection problem. Facta Univ. Ser. Mech. Eng. 2019, 17, 455–469. [Google Scholar] [CrossRef]
- Badi, I.; Pamucar, D. Supplier selection for steelmaking company by using combined Grey-MARCOS methods. Decis. Mak. Appl. Manag. Eng. 2020, 3, 37–48. [Google Scholar] [CrossRef]
- Karamasa, C.; Demir, E.; Memis, S.; Korucuk, S. Weighting the factors affecting logistics outsourcing. Decis. Mak. Appl. Manag. Eng. 2021, 4, 19–32. [Google Scholar] [CrossRef]
- Aguezzoul, A. Third-Party Logistics Selection Problem: A Literature Review on Criteria and Methods. Omega 2014, 49, 69–78. [Google Scholar] [CrossRef]
- Prahinski, C.; Kocabasoglu, C. Empirical research opportunities in reverse supply chains. Omega 2006, 34, 519–532. [Google Scholar] [CrossRef]
- Zareinejad, M.; Javanmard, H. Evaluation and selection of a third-party reverse logistics provider using ANP and IFG-MCDM methodology. Life Sci. J. 2013, 10, 350–355. [Google Scholar]
- Saen, R.F. A new model for selecting third-party reverse logistics providers in the presence of multiple dual-role factors. Int. J. Adv. Manuf. Technol. 2010, 46, 405–410. [Google Scholar] [CrossRef] [Green Version]
- Yang, D.H.; Kim, S.; Nam, C.; Min, J.W. Developing a decision model for business process outsourcing. Comput. Oper. Res. 2007, 34, 3769–3778. [Google Scholar] [CrossRef]
- Hsiao, H.; Kemp, R.G.M.; der Vorst, J.G.A.V.; Omta, S.O. A classification of logistic outsourcing levels and their impact on service performance: Evidence from the food processing industry. Int. J. Prod. Econ. 2010, 124, 75–86. [Google Scholar] [CrossRef]
- Jabbour, A.B.L.; Latan, C.J.C.; Teixeira, A.A.; Oliveira, J.H.C. Quality management, environmental management maturity, green supply chain practices and green performance of Brazilian companies with ISO 14001 certification: Direct and indirect effects. Transp. Res. Logist. Transp. Rev. 2014, 67, 39–51. [Google Scholar] [CrossRef]
- Pamucar, D.; Chatterjee, K.; Zavadskas, E.K. Assessment of third-party logistics provider using multi-criteria decision-making approach based on interval rough numbers. Comput. Ind. Eng. 2019, 127, 383–407. [Google Scholar] [CrossRef]
- Yayla, A.Y.; Oztekin, A.; Gumus, A.T.; Gunasekaran, A. A hybrid data analytic methodology for 3PL transportation provider evaluation using fuzzy multi-criteria decision making. Int. J. Prod. Res. 2015, 53, 6097–6113. [Google Scholar] [CrossRef]
- Roy, J.; Adhikary, K.; Kar, S.; Pamucar, D. A rough strength relational DEMATEL model for analysing the key success factors of hospital service quality. Decis. Making Appl. Manag. Eng. 2018, 1, 121–142. [Google Scholar] [CrossRef]
- Zarbakhshnia, N.; Soleimani, H.; Ghaderi, H. Sustainable thirdparty reverse logistics provider evaluation and selection using fuzzy SWARA and developed fuzzy COPRAS in the presence of risk criteria. Appl. Soft Comput. 2018, 65, 307–319. [Google Scholar] [CrossRef]
- Liu, A.; Ji, X.; Lu, H.; Liu, H. The selection of 3PRLs on self-service mobile recycling machine: Interval-valued Pythagorean hesitant fuzzy best-worst multi-criteria group decision-making. J. Clean. Prod. 2019, 230, 734–750. [Google Scholar] [CrossRef]
- Bai, C.; Sarkis, J. Integrating and extending data and decision tools for sustainable third-party reverse logistics provider selection. Oper. Res. Comput. 2019, 110, 188–207. [Google Scholar] [CrossRef]
- Zarbakhshnia, N.; Wu, Y.; Govindan, K.; Soleimani, H. A novel hybrid multiple attribute decision-making approach for outsourcing sustainable reverse logistics. J. Clean. Prod. 2020, 242, 118461. [Google Scholar] [CrossRef]
- Zhang, X.; Su, T. The dominance degree-based heterogeneous linguistic decision-making technique for sustainable S3PRLP selection. Complexity 2020, 2020, 6102036. [Google Scholar]
- Efendigil, T.; Önüt, S.; Kongar, E. A Holistic Approach for Selecting a Third-Party Reverse Logistics Provider in the Presence of Vagueness. Comput. Ind. Eng. 2008, 54, 269–287. [Google Scholar] [CrossRef]
- Cheng, Y.; Lee, F. Outsourcing Reverse Logistics of High-Tech Manufacturing Firms by using a Systematic Decision-Making Approach: TFT-LCD Sector in Taiwan. Ind. Mark. Manag. 2010, 39, 1111–1119. [Google Scholar] [CrossRef]
- Ho, W.; He, T.; Lee, C.K.M. Emrouznejad, A. Strategic Logistics Outsourcing: An Integrated QFD and Fuzzy AHP Approach. Expert Syst. Appl. 2012, 39, 10841–10850. [Google Scholar] [CrossRef]
- Chai, J.; Liu, J.N.; Ngai, E.W. Application of Decision-Making Techniques in Supplier Selection: A Systematic Review of Literature. Expert Syst. Appl. 2013, 40, 3872–3885. [Google Scholar] [CrossRef]
- Govindan, K.; Pokharel, S.; Kumar, P.S. A hybrid approach using ISM and fuzzy TOPSIS for the selection of reverse logistics provider. Resour. Conserv. Recycl. 2009, 54, 28–36. [Google Scholar]
- Boyson, S.; Corsi, T.; Dresner, M.; Rabinovich, E. Managing third party logistics relationships: What does it take. J. Bus. Logist. 1999, 20, 73–100. [Google Scholar]
- Langley, C.J.; Allen, O.R.; Tyndall, O.R. Third-Party Logistics Study Results and Finding of the 2002 Seventh Annual Study; Georgia Institute of Technology: Atlanta, GA, USA, 2002; Unpublished Report. [Google Scholar]
- Meade, L.; Sarkis, J. A conceptual model for selecting and evaluating third-party reverse logistics providers. Supply Chain. 2002, 7, 283–295. [Google Scholar] [CrossRef]
- Gunasekaran, A.; Patel, C.; McGaughey, R.E. A framework for supply chain performance measurement. Int. J. Prod. Econ. 2004, 87, 333–347. [Google Scholar] [CrossRef]
- Stock, O.N.; Oreis, N.P.; Kasarda, J.D. Logistics strategy and structure a conceptual framework. Int. J. Oper. Prod. Manag. 1998, 18, 37–52. [Google Scholar] [CrossRef]
- Ha, S.H.; Krishnan, R. A hybrid approach to supplier selection for the maintenance of a competitive supply chain. Expert Syst. Appl. 2008, 34, 1303–1311. [Google Scholar] [CrossRef]
- Amin, S.H.; Zhang, G. An integrated model for closed-loop supply chain configuration and supplier selection: Multi-objective approach. Expert Syst. Appl. 2012, 39, 6782–6791. [Google Scholar] [CrossRef]
- Darvish, M.; Yasaei, M.; Saeedi, A. Application of graph theory and matrix methods for contractor ranking. Int. J. Proj. Manag. 2009, 27, 610–619. [Google Scholar] [CrossRef]
- Amin, S.H.; Razmi, J. An integrated fuzzy model for supplier management: A case study of ISP selection and evaluation. Expert Syst. Appl. 2009, 36, 8639–8648. [Google Scholar] [CrossRef]
- Chen, Y.J. Structured methodology for supplier selection and evaluation in a supply chain. Inf. Sci. 2011, 181, 1651–1670. [Google Scholar] [CrossRef]
- Mavi, R.K.; Goh, M.; Zarbakhshnia, N. Sustainable third party reverse logistic provider selection with fuzzy SWARA and fuzzy MOORA in plastic industry. Int. J. Adv. Manuf. Technol. 2017, 91, 2401–2418. [Google Scholar] [CrossRef]
- Li, Y.L.; Ying, C.S.; Chin, K.S.; Yang, H.T.; Xu, J. Third party reverse logistics provider selection approach based on hybrid-information MCDM and cumulative prospect theory. J. Clean. Prod. 2018, 195, 573–584. [Google Scholar] [CrossRef]
- Kuo, R.J.; Wang, Y.C.; Tien, F.C. Integration of artificial neural network andMADA methods for green supplier selection. J. Clean. Prod. 2010, 18, 1161–1170. [Google Scholar] [CrossRef]
- Amindoust, A.; Ahmed, S.; Saghafinia, A.; Bahreininejad, A. Sustainable supplier selection: A ranking model based on fuzzy inference system. Appl. Soft Comput. 2012, 12, 1668–1677. [Google Scholar] [CrossRef]
- Shen, L.; Olfat, L.; Govindan, K.; Khodaverdi, R.; Diabatd, A. A fuzzy multi criteria approach for evaluating green supplier’s performance in green supply chain with linguistic preferences. Resour. Conserv. Recycl. 2013, 74, 170–179. [Google Scholar] [CrossRef]
- Govindan, K.; Khodaverdi, R.; Jafarian, A. A fuzzy multi criteria approach for measuring sustainability performance of a supplier based on triple bottom line approach. J. Clean. Prod. 2013, 47, 345–354. [Google Scholar] [CrossRef]
- Kannan, D.; Khodaverdi, R.; Olfat, L.; Jafarian, A.; Diabat, A. Integrated fuzzy multi criteria decision making method and multiobjective programming approach for supplier selection and order allocation in a green supply chain. J. Clean. Prod. 2013, 47, 355–366. [Google Scholar] [CrossRef]
- Jabbour, A.B.; Jabbour, C.; Govindan, K.; Kannan, D.; Arantes, A.F. Mixed methodology to analyze the relationship between maturity of environmental management and the adoption of green supply chain management in Brazil. Resour. Conserv. Recycl. 2014, 92, 255–267. [Google Scholar] [CrossRef]
- Kannan, D.; Garg, K.; Jha, P.C.; Diabat, A. Integrating disassembly line balancing in the planning of a reverse logistics network from the perspective of a third party provider. Ann. Oper. Res. 2017, 253, 353–376. [Google Scholar] [CrossRef]
- Sen, D.K.; Datta, S.; Mahapatra, S.S. Decision support framework for selection of 3PL providers: Dominance-based approach in combination with grey set theory. Int. J. Inf. Technol. Decis. Mak. 2017, 16, 25–57. [Google Scholar] [CrossRef]
- Xu, Z.S.; Yager, R.R. Intuitionistic Fuzzy Bonferroni Means. IEEE Trans. Syst. 2011, 41, 568–578. [Google Scholar]
- Xu, Z.S.; Xia, M.M. Induced generalized intuitionistic fuzzy operators. Knowl. Based Syst. 2011, 24, 197–209. [Google Scholar] [CrossRef]

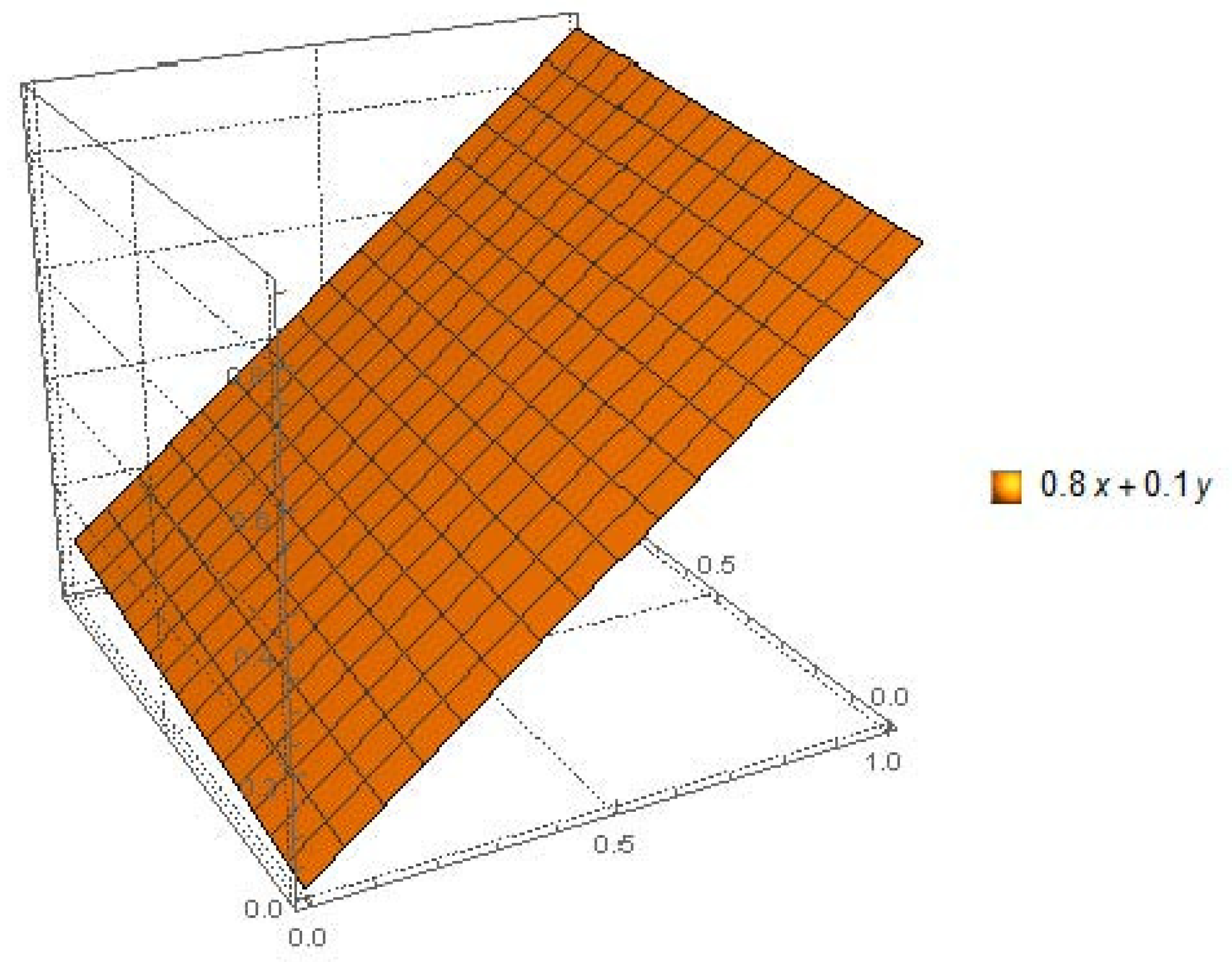
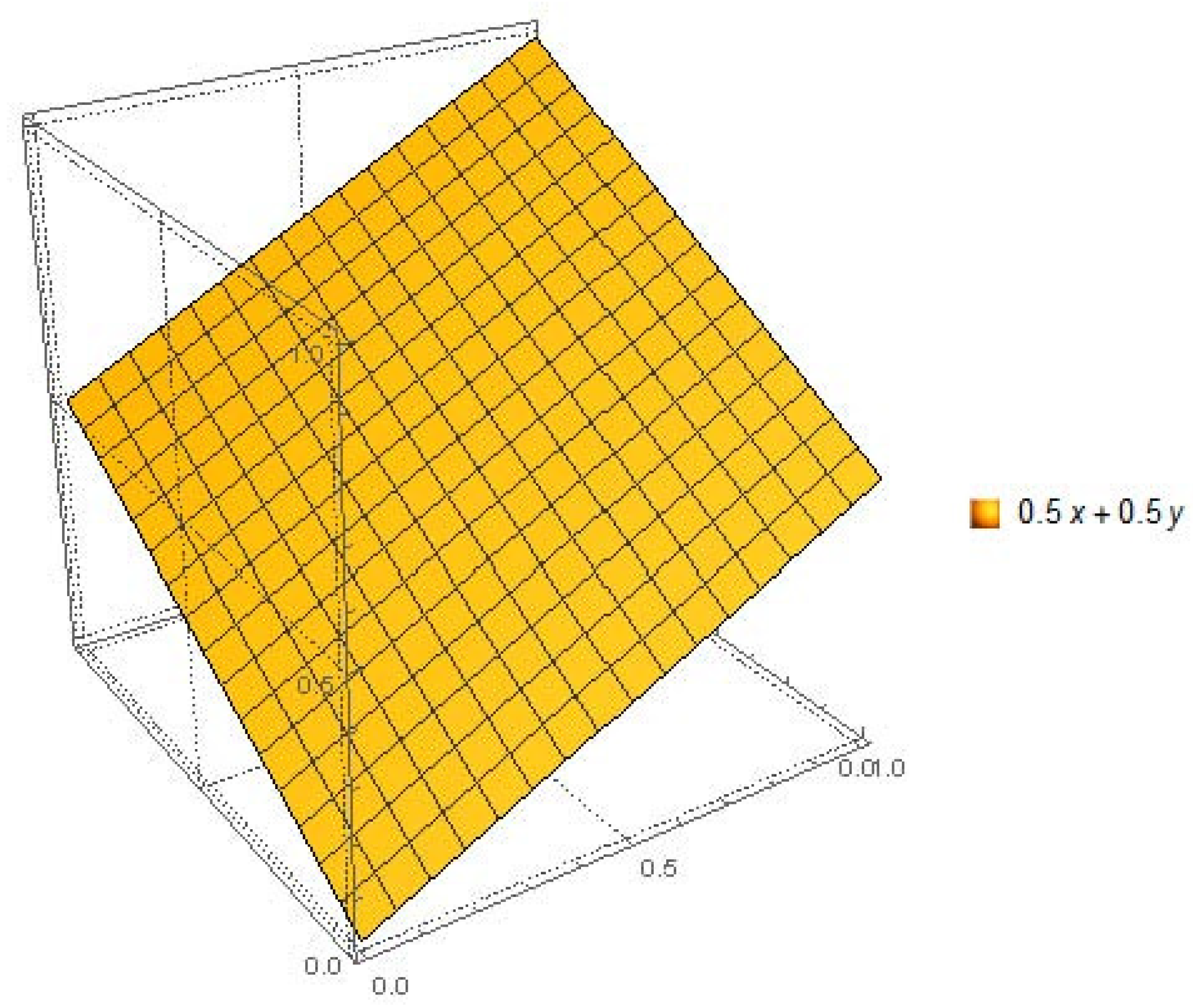

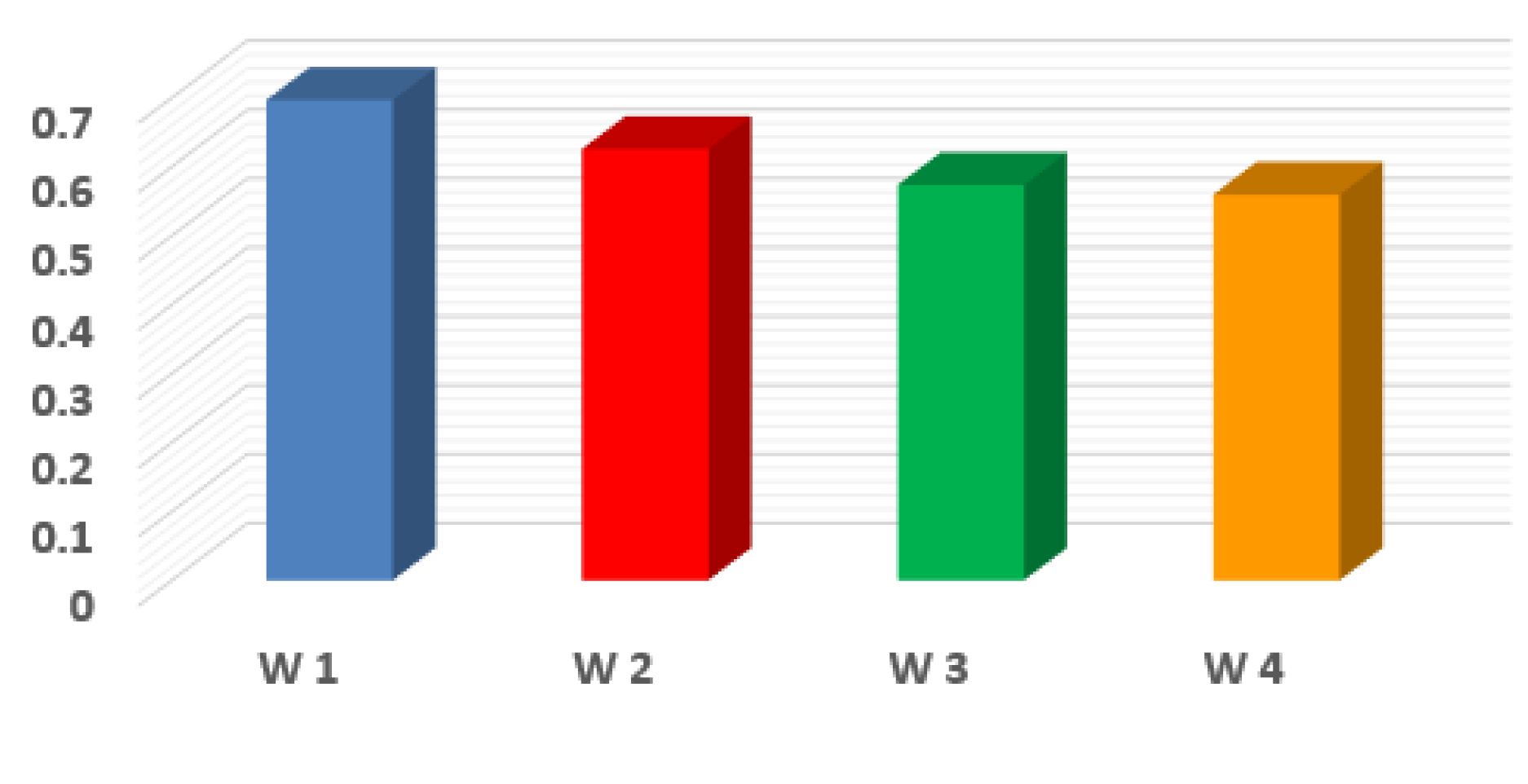
| Concepts | Remarks |
|---|---|
| Fuzzy sets [1] | It does not consider NMD. |
| IFSs [2] | It cannot be applied if for some . |
| PFSs [5,6] | It cannot be applied if for some . |
| q-ROFSs [7] | It is inapplicable for smaller “q” values. with , or if for some . |
| LDFSs [24] | (1) It can handle all situations where IFS, PFS, and q-ROFS cannot be used; (2) it takes a parameterizations approach and operates under the control of reference parameters; (3) MD and NMD can be taken in free manner from . |
| Criteria | Literature | Nature |
|---|---|---|
| Cost () | Govindan et al. [60], Boyson et al. [61], Langley et al. [62] | Non-beneficial |
| Meade and Sarkis [63], Gunasekaran et al. [64], Efendigil et al. [56] | ||
| Stock et al. [65], Ha and Krishnan [66] | ||
| Experience () | Ha and Krishnan [66], Amin and Zhang [67], Darvish et al. [68], | Beneficial |
| Amin and Razmi [69], Saen [44], Chen [70] | ||
| Quality () | Govindan et al. [60], Boyson et al. [61], Stock et al. [65], | |
| Saen [44], Mavi et al. [71], Li et al. [72] | Beneficial | |
| Eco-design | Kuo et al. [73], Amindoust et al. [74], Shen et al. [75] | Beneficial |
| production () | Govindan et al. [76], Kannan et al. [77], Jabbour et al. [78] | |
| Reputation () | Saen [44], Mavi et al. [71], Kannan et al. [79], Sen et al. [80] | Beneficial |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Riaz, M.; Farid, H.M.A.; Aslam, M.; Pamucar, D.; Bozanić, D. Novel Approach for Third-Party Reverse Logistic Provider Selection Process under Linear Diophantine Fuzzy Prioritized Aggregation Operators. Symmetry 2021, 13, 1152. https://doi.org/10.3390/sym13071152
Riaz M, Farid HMA, Aslam M, Pamucar D, Bozanić D. Novel Approach for Third-Party Reverse Logistic Provider Selection Process under Linear Diophantine Fuzzy Prioritized Aggregation Operators. Symmetry. 2021; 13(7):1152. https://doi.org/10.3390/sym13071152
Chicago/Turabian StyleRiaz, Muhammad, Hafiz Muhammad Athar Farid, Muhammad Aslam, Dragan Pamucar, and Darko Bozanić. 2021. "Novel Approach for Third-Party Reverse Logistic Provider Selection Process under Linear Diophantine Fuzzy Prioritized Aggregation Operators" Symmetry 13, no. 7: 1152. https://doi.org/10.3390/sym13071152
APA StyleRiaz, M., Farid, H. M. A., Aslam, M., Pamucar, D., & Bozanić, D. (2021). Novel Approach for Third-Party Reverse Logistic Provider Selection Process under Linear Diophantine Fuzzy Prioritized Aggregation Operators. Symmetry, 13(7), 1152. https://doi.org/10.3390/sym13071152









