Monte Carlo Simulation of a Modified Chi Distribution Considering Asymmetry in the Generating Functions: Application to the Study of Health-Related Variables
Abstract
1. Introduction
2. Methodology
2.1. Generalities on the Chi Distribution
2.2. Monte Carlo Simulations for Non-Gaussian Generating Distributions
2.3. Measurement of Goodness of Fit between Data and Models
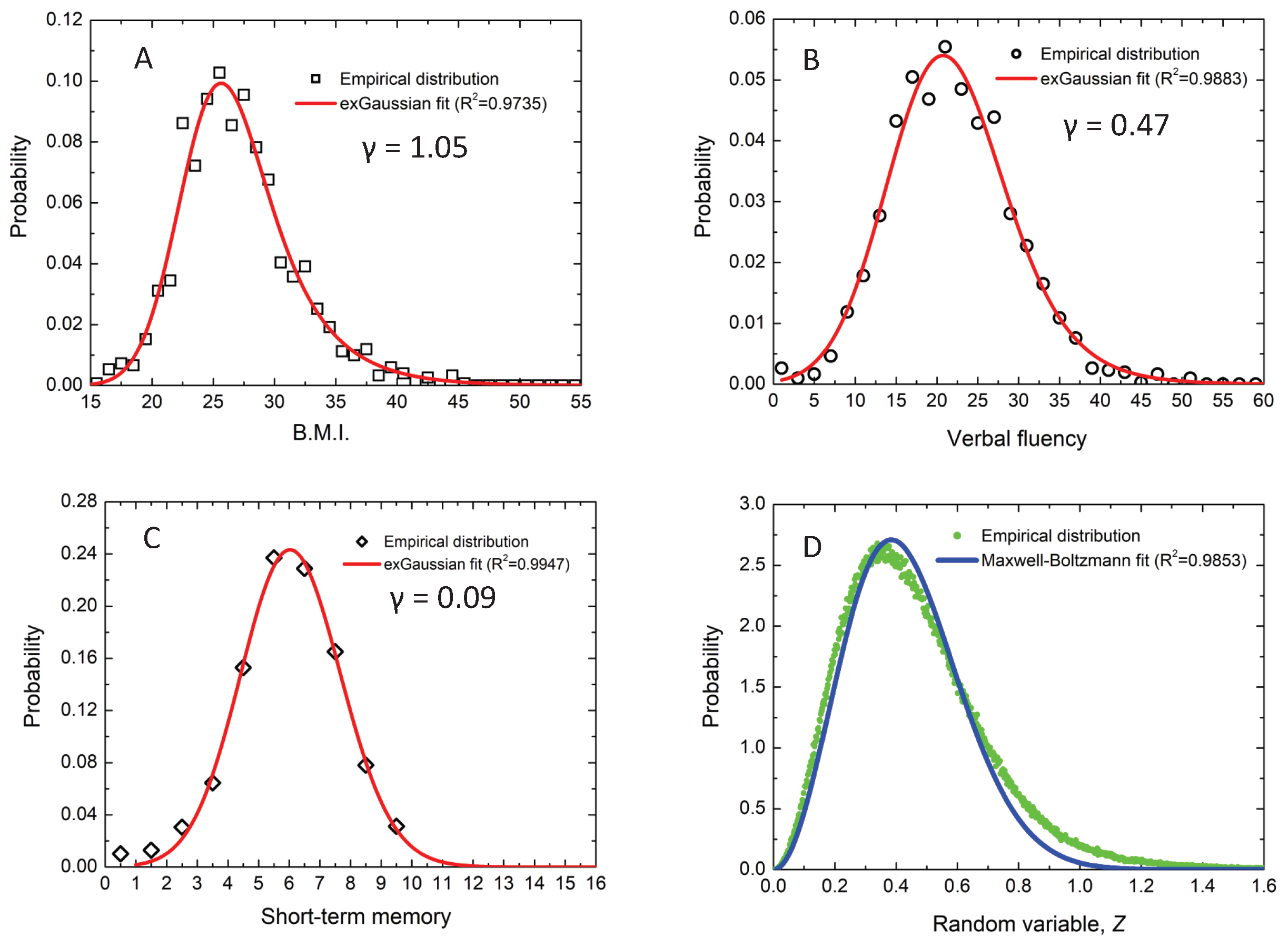
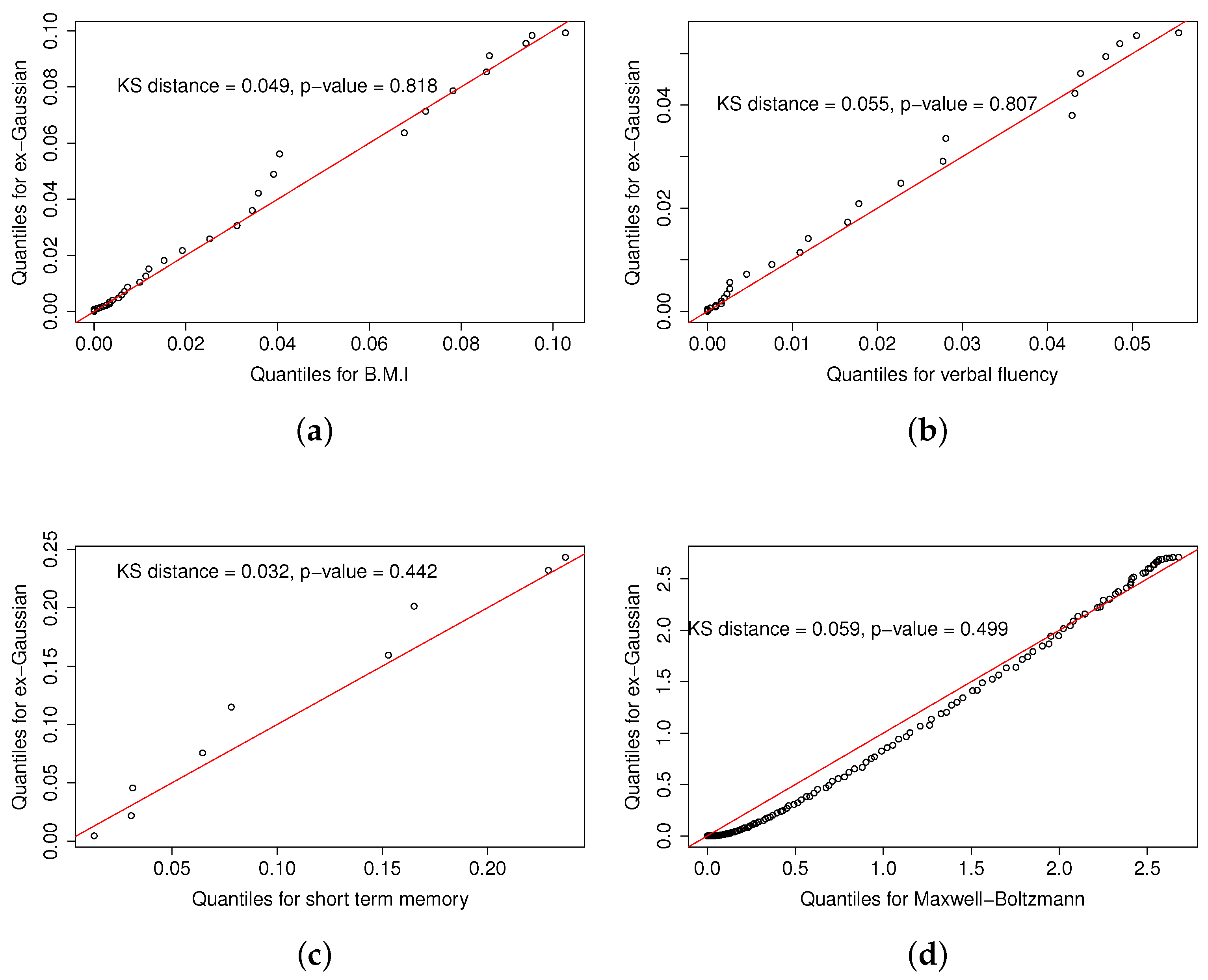
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Montgomery, D.C.; Runger, G.C. Applied Statistics and Probability for Engineers, 6th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2014. [Google Scholar]
- Pearson, K. On the criterion that a given system of deviations from the probable in the case of a correlated system of variables is such that it can be reasonably supposed to have arisen from random sampling. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1900, 50, 157–175. [Google Scholar] [CrossRef]
- Fisher, R. On the Interpretation of χ2 from Contingency Tables, and the Calculation of P. J. R. Stat. Soc. 1922, 85, 87–94. [Google Scholar] [CrossRef]
- Fisher, R.A. The Conditions Under Which χ2 Measures the Discrepancy Between Observation and Hypothesis. J. R. Stat. Soc. 1924, 87, 442–450. [Google Scholar]
- Bolboacă, S.D.; Jäntschi, L.; Sestraş, A.F.; Sestraş, R.E.; Pamfil, D.C. Pearson-Fisher Chi-Square Statistic Revisited. Information 2011, 2, 528–545. [Google Scholar] [CrossRef]
- Castro-Palacio, J.C.; Fernández-de-Córdoba, P.; Isidro, J.M.; Navarro-Pardo, E.; Selva-Aquilar, R. Aguilar. Percentile study of Chi distribution. Application to response time data. Mathematics 2020, 8, 514. [Google Scholar] [CrossRef]
- Castro-Palacio, J.C.; Navarro-Pardo, E.; Isidro, J.M.; Sahu, S.; Fernández-de-Córdoba, P. Brain reaction times. Linking individual and collective behavior through Physics modeling. Symmetry 2021, 13, 451. [Google Scholar] [CrossRef]
- Iglesias-Martínez, M.E.; Hernaiz-Guijarro, M.; Castro-Palacio, J.C.; Fernández-de-Córdoba, P.; Isidro, J.M.; Navarro-Pardo, E. Machinery Failure Approach and Spectral Analysis to Study the Reaction Time Dynamics over Consecutive Visual Stimuli: An Entropy-Based Model. Mathematics 2020, 8, 1979. [Google Scholar] [CrossRef]
- Castro-Palacio, J.C.; Isidro, J.M.; Navarro-Pardo, E.; Velázquez-Abad, L.; Fernández-de-Córdoba, P. Monte Carlo Simulation of a Modified Chi Distribution with Unequal Variances in the Generating Gaussians. A Discrete Methodology to Study Collective Response Times. Mathematics 2021, 9, 77. [Google Scholar] [CrossRef]
- Moret-Tatay, C.; Gamermann, D.; Navarro-Pardo, E.; Fernández-de-Córdoba, P. ExGUtils: A Python Package for Statistical Analysis with the ex-Gaussian Probability Density. Front. Psycol. 2018, 9, 612. [Google Scholar] [CrossRef]
- Ratclif, R.; Murdock, B.B. Retrieval processes in recognition memory. Psychol. Rev. 1976, 83, 190. [Google Scholar] [CrossRef]
- Gmehlin, D.; Fuermaier, A.B.M.; Walther, S.; Debelak, R.; Rentrop, M.; Westermann, C.; Sharma, A.; Tucha, L.; Koerts, J.; Tucha, O.; et al. Intraindividual variability in inhibitory function in adults with ADHD. An ex-Gaussian approach. PLoS ONE 2014, 9, e112298. [Google Scholar] [CrossRef] [PubMed]
- Adamo, N.; Hodsoll, J.; Asherson, P.; Buitelaar, J.K.; Kuntsi, J. Ex-Gaussian, frequency and reward analyses reveal specificity of reaction time fluctuations to ADHD and not autism traits. J. Abnorm. Child Psychol. 2019, 47, 557. [Google Scholar] [CrossRef] [PubMed]
- Xavier Castellanos, F.; Sonuga-Barke, E.J.S.; Scheres, A.; Di Martino, A.; Hyde, C.; Walters, J.R. Varieties of Attention-Defficit/Hyperactivity Disorder-Related Intra-Individual Variability. Biol. Psychiatry 2005, 57, 1416. [Google Scholar] [CrossRef]
- Navarro-Pardo, E.; Navarro-Prados, A.B.; Gamermann, D.; Moret-Tatay, C. Differences between young and old university students on a lexical decision task: Evidence through an ex-gaussian approach. J. Gen. Psychol. 2013, 140, 251–268. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Moret-Tatay, C.; Moreno-Cid, A.; de Lima Argimon, I.I.; Quarti Irigaray, T.; Szczerbinski, M.; Murphy, M.; Vázquez-Martínez, A.; Vázquez-Molina, J.; Sáiz-Mauleón, B.; Navarro-Pardo, E.; et al. The effects of age and emotional valence on recognition memory: An ex-Gaussian components analysis. Scand. J. Psychol. 2014, 55, 420–426. [Google Scholar] [CrossRef]
- Hernáiz-Guijarro, M.; Castro-Palacio, J.C.; Navarro-Pardo, E.; Isidro, J.M.; Fernández-de-Córdoba, P. A probabilistic classification procedure based on response time analysis towards a quick pre-diagnosis of student’s attention deficit. Mathematics 2019, 7, 473. [Google Scholar] [CrossRef]
- Li, J.A. Simplified Exponentially Modified Gaussian Function for Modeling Chromatographic Peaks. J. Chromatogr. Sci. 1995, 33, 568–572. [Google Scholar] [CrossRef]
- Gradshteyn, I.; Ryzhik, I. Table of Integrals, Series and Products; Academic Press: Boston, MA, USA, 2007. [Google Scholar]
- Tolman, R. The Principles of Statistical Mechanics; Dover Publications Inc.: New York, NY, USA, 2003. [Google Scholar]
- Boltzmann, L. Studies on the balance of kinetic energy between moving material points. Wiener Berichte 1868, 58, 517–560. [Google Scholar]
- Fodor, M.P.; Bolboacă, S.D.; Jäntschi, L. Distribution of Molecules by Kinetic Energy Revisited. Bull. UASVM Hortic. 2013, 70, 10–18. [Google Scholar]
- Levenberg, K. A Method for the Solution of Certain Non-Linear Problems in Least Squares. Q. Appl. Math. 1944, 2, 164–168. [Google Scholar] [CrossRef]
- Marquardt, D. An Algorithm for Least-Squares Estimation of Nonlinear Parameters. J. Soc. Ind. Appl. Math. 1963, 11, 431–441. [Google Scholar] [CrossRef]
- Devore, J.L. Probability and Statistics for Engineering and the Sciences; Cengage Learning: Boston, MA, USA, 2011. [Google Scholar]
- Conover, W.J. Practical Nonparametric Statistics; Wiley: New York, NY, USA, 1999. [Google Scholar]
- Kolmogoroff, A. Confidence Limits for an Unknown Distribution Function. Ann. Math. Stat. 1941, 12, 461–463. [Google Scholar] [CrossRef]
- Jäntschi, L. A Test Detecting the Outliers for Continuous Distributions Based on the Cumulative Distribution Function of the Data Being Tested. Symmetry 2019, 11, 835. [Google Scholar] [CrossRef]
- Jäntschi, L. Detecting Extreme Values with Order Statistics in Samples from Continuous Distributions. Mathematics 2020, 8, 216. [Google Scholar] [CrossRef]
- Wilk, M.B.; Gnanadesikan, R. Probability plotting methods for the analysis of data. Biom. Biom. Trust 1968, 55, 1–17. [Google Scholar] [CrossRef]
- Börsch-Supan, A. Survey of Health, Ageing and Retirement in Europe (SHARE) Wave 7; Release Version: 7.1.1; SHARE-ERIC: München, Germany, 2020. [Google Scholar]
- Bergmann, M.; Scherpenzeel, A.; Börsch-Supan, A. SHARE Wave 7 Methodology: Panel Innovations and Life Histories; Munich Center for the Economics of Aging (MEA): München, Germany, 2019. [Google Scholar]
- Börsch-Supan, A.; Brandt, M.; Hunkler, C.; Kneip, T.; Korbmacher, J.; Malter, F.; Schaan, B.; Stuck, S.; Zuber, S. Data Resource Profile: The Survey of Health, Ageing and Retirement in Europe (SHARE). Int. J. Epidemiol. 2013, 42, 992–1001. [Google Scholar] [CrossRef]
- Fernandez-de-las-Penas, C.; Fernandez-Munoz, J.J.; Palacios-Cena, M.; Navarro-Pardo, E. Sleep disturbances in tension-type headache and migraine. Ther. Adv. Neurol. Disord. 2017, 11, 1–6. [Google Scholar] [CrossRef]
- Viguer, P.; Carlos Melendez, J.; Valencia, S.; Cantero, M.-J.; Navarro, E. Grandparent-Grandchild Relationships from the Children’s Perspective: Shared Activities and Socialization Styles. Span. J. Psychol. 2010, 13, 708–717. [Google Scholar] [CrossRef]

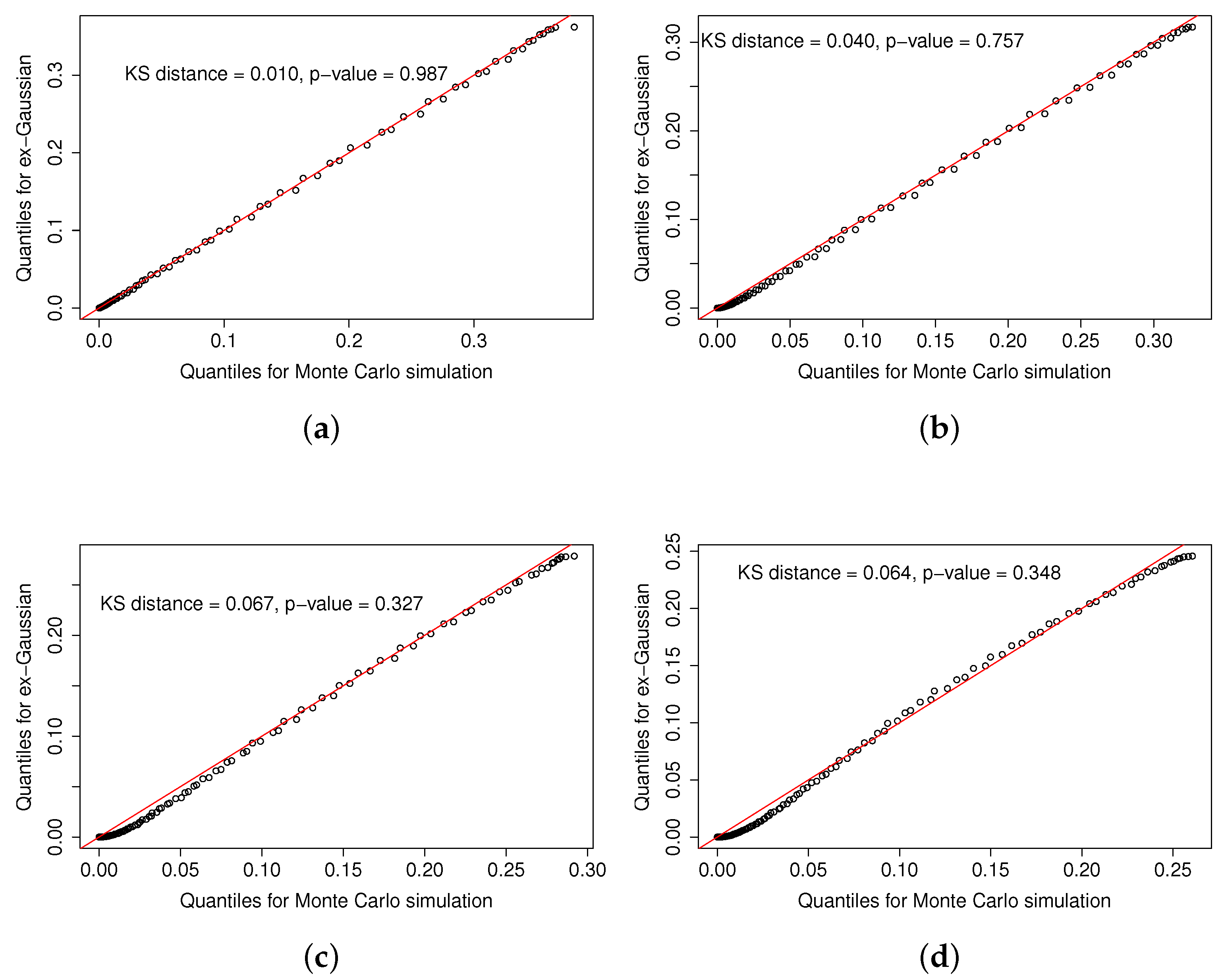
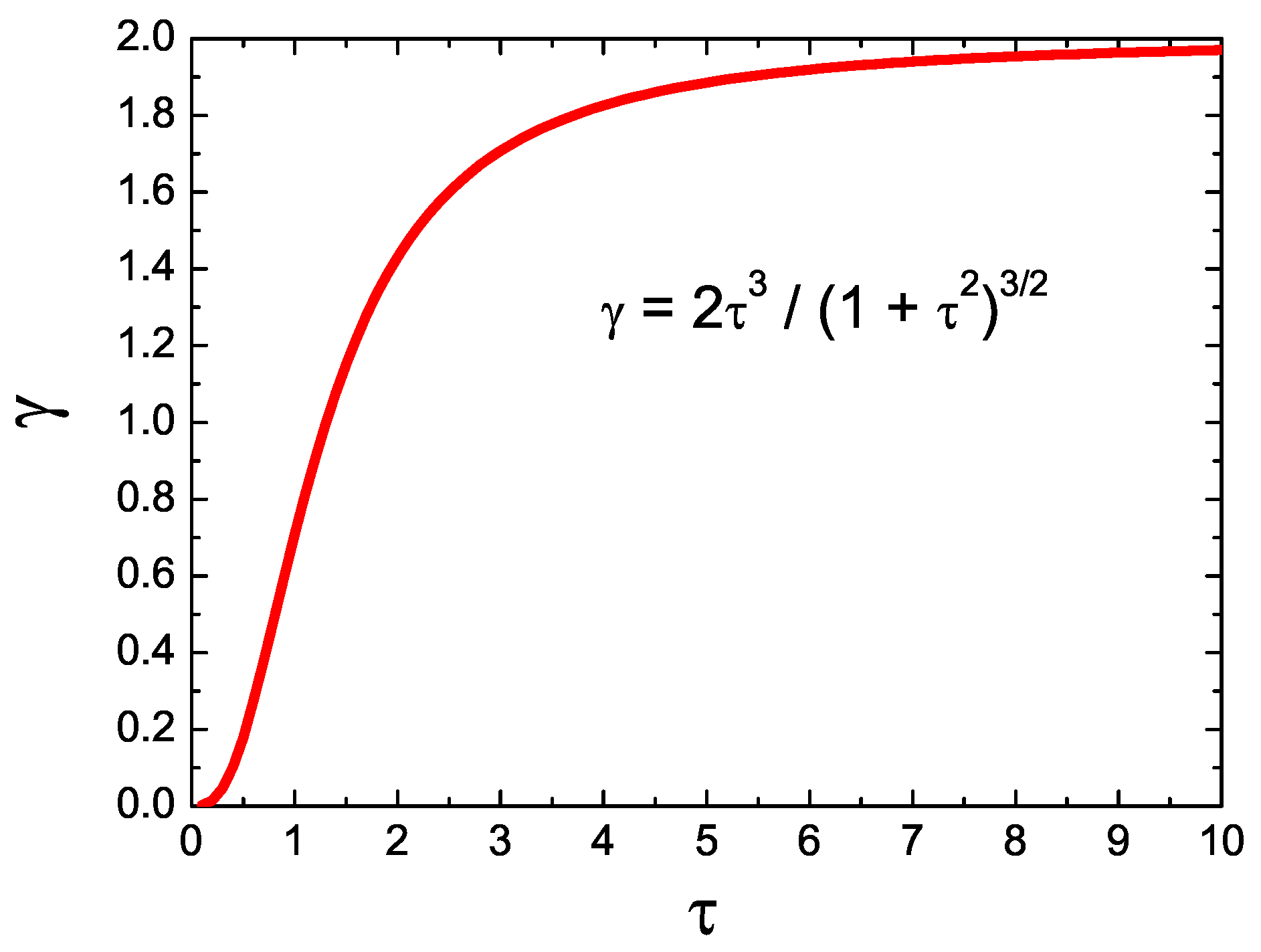
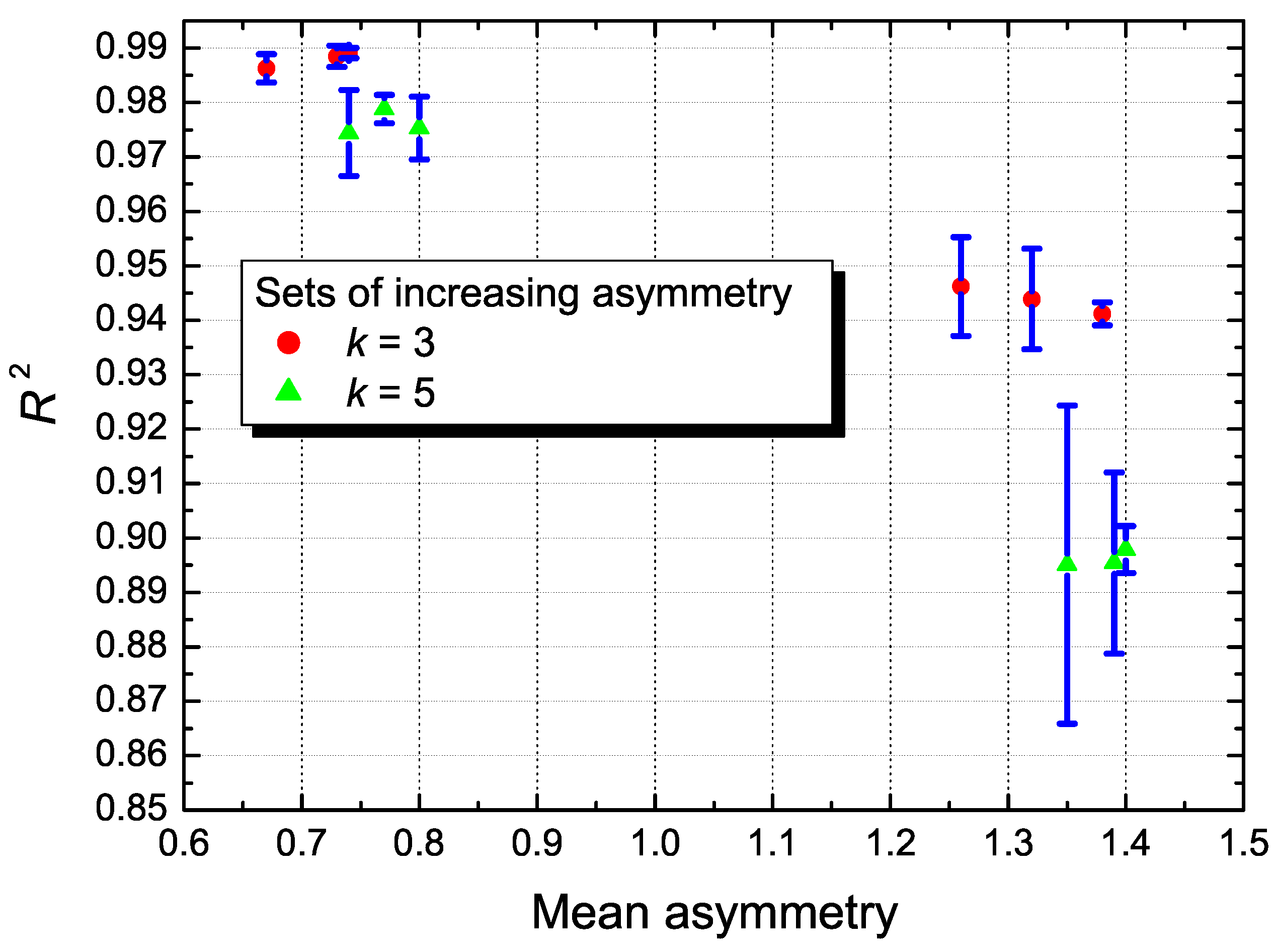
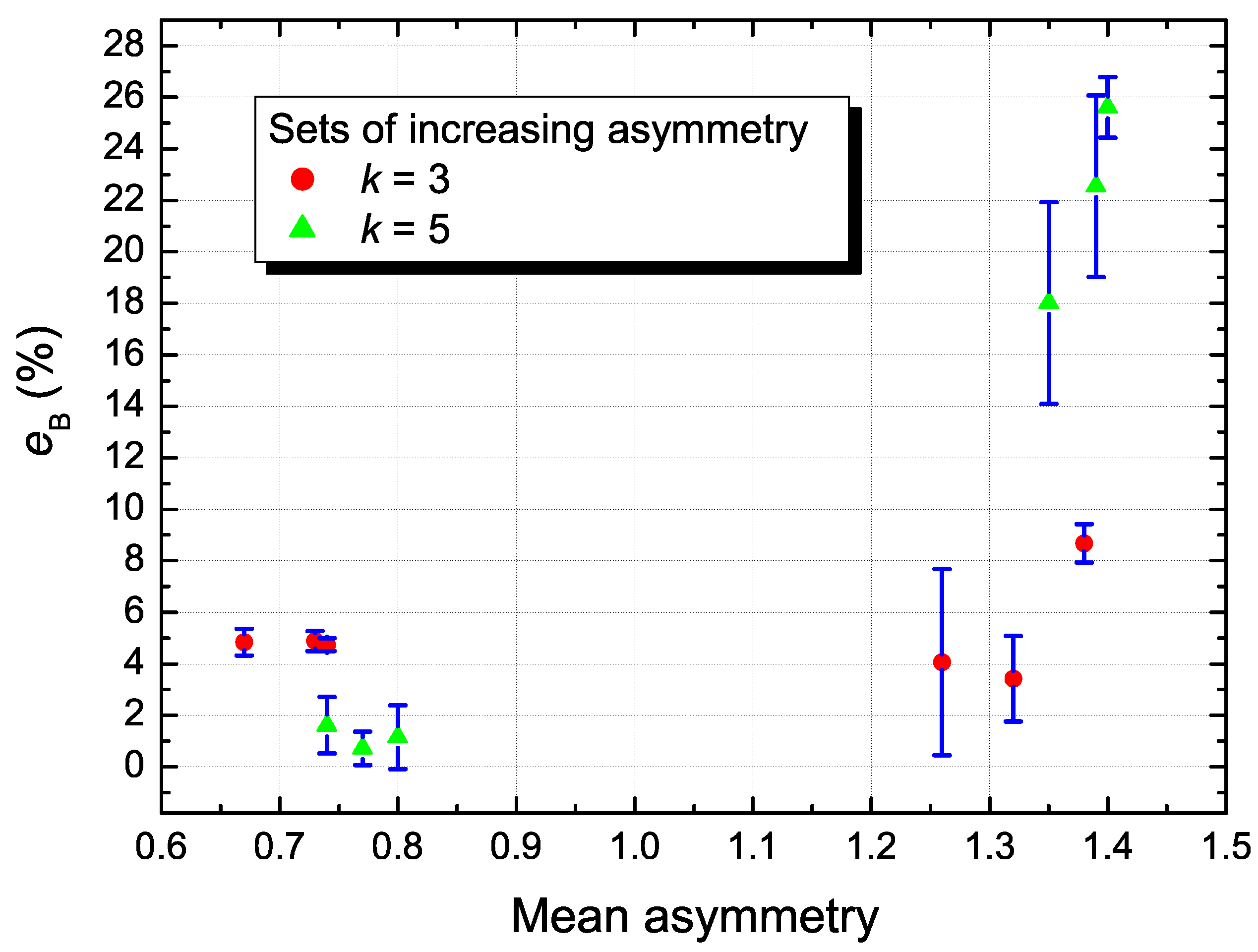
| Set | τ1 | τ2 | τ3 | eτ (%) | 〈γ〉 | 〈〉 | 〈 | ||
|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.5 | 0.8 | 1.45 | 97.44 | 0.67 | 5.27 | 5.00 | 0.9863 | 4.84 |
| 0.5 | 1 | 1.45 | 97.44 | 5.12 | 4.87 | ||||
| 0.5 | 1.2 | 1.45 | 97.44 | 5.26 | 5.04 | ||||
| 2 | 0.7 | 0.9 | 1.4 | 66.67 | 0.73 | 4.55 | 4.31 | 0.9885 | 4.89 |
| 0.7 | 1 | 1.4 | 66.67 | 4.14 | 3.94 | ||||
| 0.7 | 1.2 | 1.4 | 66.67 | 4.25 | 4.07 | ||||
| 3 | 0.9 | 0.95 | 1.2 | 28.57 | 0.74 | 4.14 | 3.93 | 0.9891 | 4.74 |
| 0.9 | 1 | 1.2 | 28.57 | 3.97 | 3.79 | ||||
| 0.9 | 1.1 | 1.2 | 28.57 | 4.02 | 3.84 | ||||
| 4 | 1 | 1.5 | 2.8 | 94.74 | 1.26 | 3.44 | 3.60 | 0.9462 | 4.06 |
| 1 | 2 | 2.8 | 94.74 | 3.26 | 3.51 | ||||
| 1 | 2.5 | 2.8 | 94.74 | 3.47 | 3.48 | ||||
| 5 | 1.3 | 1.5 | 2.5 | 63.16 | 1.32 | 3.52 | 3.58 | 0.9439 | 3.43 |
| 1.3 | 2 | 2.5 | 63.16 | 3.32 | 3.49 | ||||
| 1.3 | 2.3 | 2.5 | 63.16 | 3.59 | 3.72 | ||||
| 6 | 1.7 | 1.8 | 2.1 | 21.05 | 1.38 | 3.02 | 3.28 | 0.9412 | 8.68 |
| 1.7 | 1.9 | 2.1 | 21.05 | 3.01 | 3.28 | ||||
| 1.7 | 2 | 2.1 | 21.05 | 2.94 | 3.24 |
| Set | (%) | 〈〉 | 〈〉 | 〈〉 | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.5 | 0.8 | 0.9 | 1.0 | 1.45 | 97.44 | 0.74 | 4.55 | 4.50 | 0.9744 | 1.61 |
| 0.5 | 1.0 | 1.1 | 1.2 | 1.45 | 4.48 | 4.53 | |||||
| 0.5 | 1.2 | 1.3 | 1.4 | 1.45 | 4.69 | 4.83 | |||||
| 2 | 0.7 | 0.9 | 1.0 | 1.2 | 1.4 | 66.67 | 0.80 | 4.15 | 4.14 | 0.9753 | 1.15 |
| 0.7 | 1.0 | 1.1 | 1.2 | 1.4 | 3.91 | 3.94 | |||||
| 0.7 | 1.2 | 1.3 | 1.35 | 1.4 | 4.05 | 4.15 | |||||
| 3 | 0.9 | 0.95 | 1.0 | 1.1 | 1.2 | 28.57 | 0.77 | 3.85 | 3.84 | 0.9788 | 0.72 |
| 0.9 | 1.0 | 1.1 | 1.15 | 1.2 | 3.77 | 3.80 | |||||
| 0.9 | 1.1 | 1.15 | 1.16 | 1.2 | 3.79 | 3.84 | |||||
| 4 | 1.0 | 1.5 | 1.6 | 1.8 | 2.8 | 94.74 | 1.35 | 3.21 | 3.68 | 0.8951 | 18.01 |
| 1.0 | 2.0 | 2.2 | 2.4 | 2.8 | 3.14 | 3.88 | |||||
| 1.0 | 2.5 | 2.6 | 2.7 | 2.8 | 4.02 | 4.88 | |||||
| 5 | 1.3 | 1.5 | 1.8 | 2.0 | 2.5 | 63.16 | 1.39 | 3.19 | 3.84 | 0.8954 | 22.55 |
| 1.3 | 2.0 | 2.1 | 2.2 | 2.5 | 3.05 | 3.90 | |||||
| 1.3 | 2.3 | 2.35 | 2.4 | 2.5 | 3.30 | 4.24 | |||||
| 6 | 1.7 | 1.8 | 1.9 | 2.0 | 2.1 | 21.05 | 1.40 | 2.89 | 3.70 | 0.8979 | 25.61 |
| 1.7 | 1.9 | 1.95 | 2.0 | 2.1 | 2.90 | 3.72 | |||||
| 1.7 | 2.0 | 2.05 | 2.08 | 2.1 | 2.84 | 3.73 |
| Variable | |||||||
|---|---|---|---|---|---|---|---|
| B.M.I | 23.274 | 0.235 | 2.8314 | 0.1971 | 3.8610 | 0.4428 | 0.9735 |
| Verbal fluency | 17.063 | 0.485 | 6.1891 | 0.2964 | 4.8408 | 0.7148 | 0.9883 |
| Short term mem. | 5.4995 | 0.4108 | 1.5475 | 0.1351 | 0.58533 | 0.48266 | 0.9947 |
| MB parameter | 0.07370 | 0.00025 | 0.9853 | 0.07918 | 7.17 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ortigosa, N.; Orellana-Panchame, M.; Castro-Palacio, J.C.; Córdoba, P.F.d.; Isidro, J.M. Monte Carlo Simulation of a Modified Chi Distribution Considering Asymmetry in the Generating Functions: Application to the Study of Health-Related Variables. Symmetry 2021, 13, 924. https://doi.org/10.3390/sym13060924
Ortigosa N, Orellana-Panchame M, Castro-Palacio JC, Córdoba PFd, Isidro JM. Monte Carlo Simulation of a Modified Chi Distribution Considering Asymmetry in the Generating Functions: Application to the Study of Health-Related Variables. Symmetry. 2021; 13(6):924. https://doi.org/10.3390/sym13060924
Chicago/Turabian StyleOrtigosa, Nuria, Marcos Orellana-Panchame, Juan Carlos Castro-Palacio, Pedro Fernández de Córdoba, and J. M. Isidro. 2021. "Monte Carlo Simulation of a Modified Chi Distribution Considering Asymmetry in the Generating Functions: Application to the Study of Health-Related Variables" Symmetry 13, no. 6: 924. https://doi.org/10.3390/sym13060924
APA StyleOrtigosa, N., Orellana-Panchame, M., Castro-Palacio, J. C., Córdoba, P. F. d., & Isidro, J. M. (2021). Monte Carlo Simulation of a Modified Chi Distribution Considering Asymmetry in the Generating Functions: Application to the Study of Health-Related Variables. Symmetry, 13(6), 924. https://doi.org/10.3390/sym13060924









