Lie Symmetry Analysis, Conservation Laws, Power Series Solutions, and Convergence Analysis of Time Fractional Generalized Drinfeld-Sokolov Systems
Abstract
1. Introduction
2. Preliminaries for Symmetry Analysis
- (i)
- and satisfy the following expressions:and
- (ii)
- u = and are also solutions of (3) and (4), respectively.
3. Lie Symmetry Analysis and Reduction of Time Fractional GDSS
4. Conservation Laws for the Time Fractional GDSS
- i:
- ∈ (0,1)
- ii:
- ∈ (1,2)andwhereand .
5. Series Solutions of Equations (29) and (30)
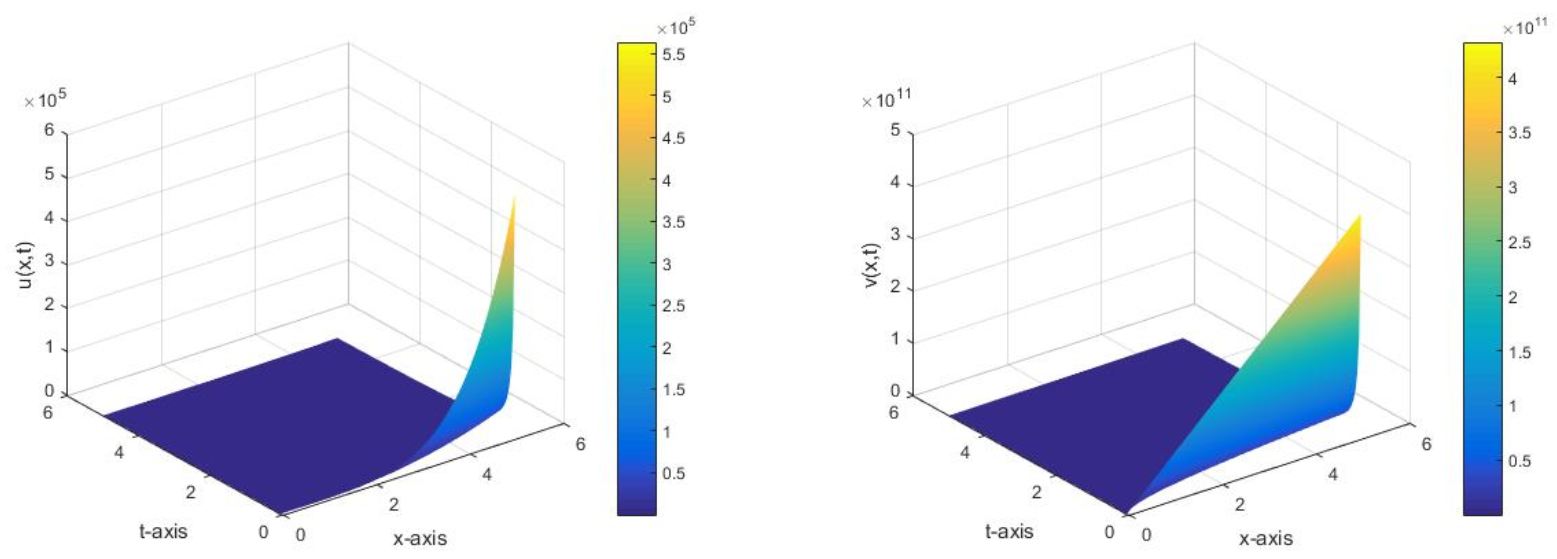
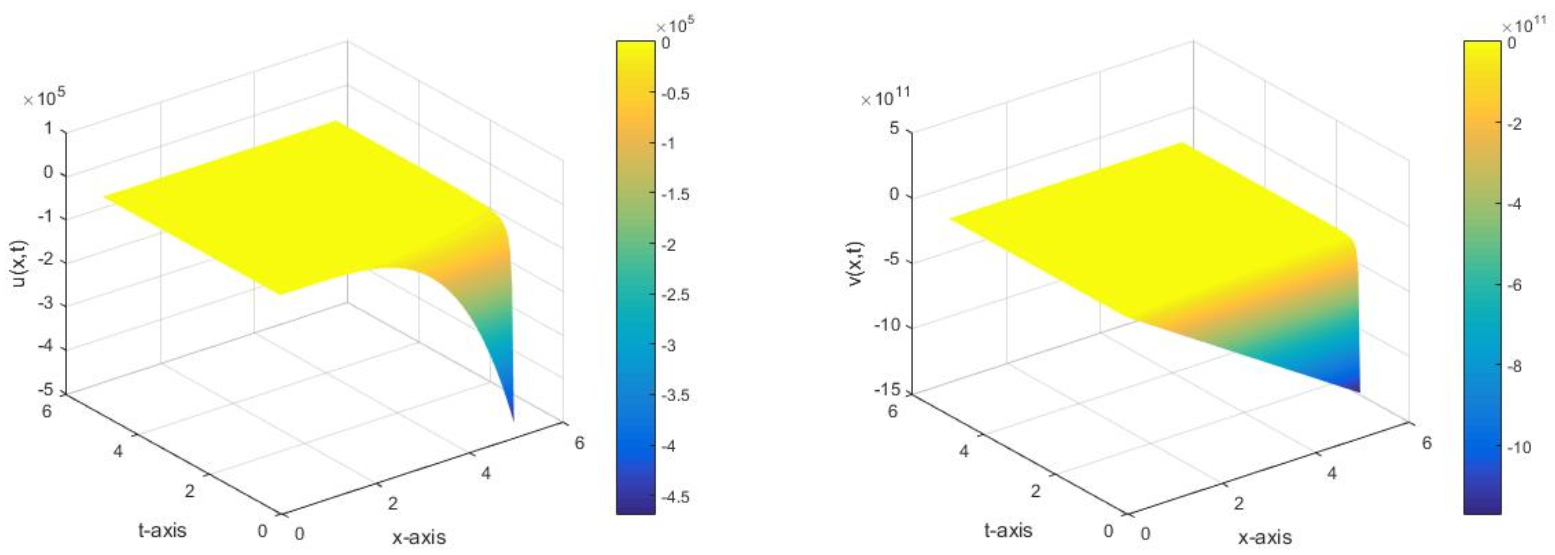
6. Convergence Analysis of the Power Series Solution
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Diethelm, K. The Analysis of Fractional Differential Equations; Springer: Berlin, Germany, 2010. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction To the Fractional Calculus and Fractional Differential Equations; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Oldham, K.B.; Spanier, J. The Fractional Calculus; Academic Press: San Diego, CA, USA, 1974. [Google Scholar]
- Guy, J. Lagrange characteristic method for solving a class of nonlinear partial differential equations of fractional order. Appl. Math. Lett. 2006, 19, 873–880. [Google Scholar] [CrossRef]
- El-Sayed, A.; Gaber, M. The Adomian decomposition method for solving partial differential equations of fractal order in finite domains. Phys. Lett. A 2006, 359, 175–182. [Google Scholar] [CrossRef]
- Baleanu, D.; Inc, M.; Yusuf, A.; Aliyu, A.I. Institute of Space Sciences Lie symmetry analysis, exact solutions and conservation laws for the time fractional modified Zakharov–Kuznetsov equation. Nonlinear Anal. Model. Control. 2017, 22, 861–876. [Google Scholar] [CrossRef]
- Jumarie, G. Cauchy’s integral formula via the modified Riemann–Liouville derivative for analytic functions of fractional order. Appl. Math. Lett. 2010, 23, 1444–1450. [Google Scholar] [CrossRef]
- Sardar, A.; Husnine, S.M.; Rizvi, S.T.R.; Younis, M.; Ali, K. Multiple travelling wave solutions for electrical transmission line model. Nonlinear Dyn. 2015, 82, 1317–1324. [Google Scholar] [CrossRef]
- Ali, S.; Rizvi, S.T.R.; Younis, M. Traveling wave solutions for nonlinear dispersive water-wave systems with time-dependent coefficients. Nonlinear Dyn. 2015, 82, 1755–1762. [Google Scholar] [CrossRef]
- Cheemaa, N.; Younis, M. New and more exact traveling wave solutions to integrable (2 + 1)-dimensional Maccari system. Nonlinear Dyn. 2016, 83, 1395–1401. [Google Scholar] [CrossRef]
- Cheemaa, N.; Younis, M. New and more general traveling wave solutions for nonlinear Schrodinger equation. Waves Random Complex Media 2016, 26, 84–91. [Google Scholar] [CrossRef]
- Deng, W. Finite Element Method for the Space and Time Fractional Fokker–Planck Equation. SIAM J. Numer. Anal. 2009, 47, 204–226. [Google Scholar] [CrossRef]
- Zhang, S. Application of Exp-function method to a KdV equation with variable coefficients. Phys. Lett. A 2007, 365, 448–453. [Google Scholar] [CrossRef]
- Zhang, X.; Zhao, J.; Liu, J.; Tang, B. Homotopy perturbation method for two dimensional time-fractional wave equation. Appl. Math. Model. 2014, 38, 5545–5552. [Google Scholar] [CrossRef]
- Fadravi, H.H.; Nik, H.S.; Buzhabadi, R. Homotopy Analysis Method for Solving Foam Drainage Equation with Space- and Time-Fractional Derivatives. Int. J. Differ. Equ. 2011, 2011, 1–12. [Google Scholar] [CrossRef]
- Gurefe, Y.; Sonmezoglu, A.; Misirli, E. Application of the trial equation method for solving some nonlinear evolution equations arising in mathematical physics. Pramana 2011, 77, 1023–1029. [Google Scholar] [CrossRef]
- Zheng, B.; Wen, C. Exact solutions for fractional partial differential equations by a new fractional sub-equation method. Adv. Differ. Equ. 2013, 2013, 199. [Google Scholar] [CrossRef]
- Kumar, S.; Kour, B.; Yao, S.-W.; Inc, M.; Osman, M. Invariance Analysis, Exact Solution and Conservation Laws of (2 + 1) Dim Fractional Kadomtsev-Petviashvili (KP) System. Symmetry 2021, 13, 477. [Google Scholar] [CrossRef]
- Kour, B.; Kumar, S. Space time fractional Drinfel’d-Sokolov-Wilson system with time-dependent variable coefficients: Symmetry analysis, power series solutions and conservation laws. Eur. Phys. J. Plus 2019, 134, 1–15. [Google Scholar] [CrossRef]
- Lukashchuk, S.Y. Symmetry reduction and invariant solutions for nonlinear fractional diffusion equation with a source term. Ufim. Mat. Zhurnal 2016, 8, 111–122. [Google Scholar] [CrossRef]
- Lie, S. Theorie der Transformationsgruppen II; Written with the help of Friedrich Engel; BG Teubner: Leipzig, Germany, 1890. [Google Scholar]
- Bluman, G.W.; Kumei, S. Symmetries and Differential Equations; Springer Science and Business Media: Berlin, Germany, 2013; Volume 81. [Google Scholar]
- Ibragimov, N.K. Elementary Lie Group Analysis and Ordinary Differential Equations; Wiley: New York, NY, USA, 1999; Volume 197. [Google Scholar]
- Clarkson, P.A.; Kruskal, M.D. New similarity reductions of the Boussinesq equation. J. Math. Phys. 1989, 30, 2201–2213. [Google Scholar] [CrossRef]
- Clarkson, P.A. New similarity solutions for the modified Boussinesq equation. J. Phys. A Math. Gen. 1989, 22, 2355–2367. [Google Scholar] [CrossRef]
- Noether, E. Invariant variation problems. Transp. Theory Stat. Phys. 1971, 1, 186–207. [Google Scholar] [CrossRef]
- Atanacković, T.M.; Konjik, S.; Pilipović, S.; Simić, S. Variational problems with fractional derivatives: Invariance conditions and Noether’s theorem. Nonlinear Anal. Theory Methods Appl. 2009, 71, 1504–1517. [Google Scholar] [CrossRef]
- Ibragimov, N.H. A new conservation theorem. J. Math. Anal. Appl. 2007, 333, 311–328. [Google Scholar] [CrossRef]
- Lukashchuk, S.Y. Conservation laws for time-fractional sub diffusion and diffusion-wave equations. Nonlinear Dyn. 2015, 80, 791–802. [Google Scholar] [CrossRef]
- Coclite, G.M.; Dipierro, S.; Maddalena, F.; Valdinoci, E. Singularity Formation in Fractional Burgers’ Equations. J. Nonlinear Sci. 2020, 30, 1285–1305. [Google Scholar] [CrossRef]
- Kirane, M.; Malik, S.A. The profile of blowing-up solutions to a nonlinear system of fractional differential equations. Nonlinear Anal. Theory Methods Appl. 2010, 73, 3723–3736. [Google Scholar] [CrossRef]
- Miškinis, P. Some properties of fractional burgers equation. Math. Model. Anal. 2002, 7, 151–158. [Google Scholar] [CrossRef][Green Version]
- Sahoo, S.; Ray, S.S. Invariant analysis with conservation laws for the time fractional Drinfeld–Sokolov–Satsuma–Hirota equations. Chaos Solitons Fractals 2017, 104, 725–733. [Google Scholar] [CrossRef]
- Jumarie, G. Modified Riemann-Liouville derivative and fractional Taylor series of nondifferentiable functions further results. Comput. Math. Appl. 2006, 51, 1367–1376. [Google Scholar] [CrossRef]
- Gazizov, R.K.; A Kasatkin, A.; Lukashchuk, S.Y. Symmetry properties of fractional diffusion equations. Phys. Scr. 2009, T136, 14–16. [Google Scholar] [CrossRef]
- Kiryakova, V.S. Generalized Fractional Calculus and Applications; CRC Press: Boca Raton, FL, USA, 1993. [Google Scholar]
- Huang, Q.; Zhdanov, R. Symmetries and exact solutions of the time fractional Harry-Dym equation with Riemann–Liouville derivative. Phys. A Stat. Mech. Its Appl. 2014, 409, 110–118. [Google Scholar] [CrossRef]
- Wang, G.-W.; Xu, T.-Z. Invariant analysis and exact solutions of nonlinear time fractional Sharma–Tasso–Olver equation by Lie group analysis. Nonlinear Dyn. 2013, 76, 571–580. [Google Scholar] [CrossRef]
- Gazizov, R.; Ibragimov, N.; Lukashchuk, S. Nonlinear self-adjointness, conservation laws and exact solutions of time-fractional Kompaneets equations. Commun. Nonlinear Sci. Numer. Simul. 2015, 23, 153–163. [Google Scholar] [CrossRef]
- Hashemi, M. Group analysis and exact solutions of the time fractional Fokker–Planck equation. Phys. A Stat. Mech. Its Appl. 2015, 417, 141–149. [Google Scholar] [CrossRef]
- Nuseir, A.S.; Al-Hasson, A. Power series solution for nonlinear system of partial differential equations. Appl. Math. Sci. 2012, 6, 5147–5159. [Google Scholar]
- Rudin, W. Principles of Mathematical Analysis; McGraw-Hill: New York, NY, USA, 1976; Volume 3. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gülşen, S.; Yao, S.-W.; Inc, M. Lie Symmetry Analysis, Conservation Laws, Power Series Solutions, and Convergence Analysis of Time Fractional Generalized Drinfeld-Sokolov Systems. Symmetry 2021, 13, 874. https://doi.org/10.3390/sym13050874
Gülşen S, Yao S-W, Inc M. Lie Symmetry Analysis, Conservation Laws, Power Series Solutions, and Convergence Analysis of Time Fractional Generalized Drinfeld-Sokolov Systems. Symmetry. 2021; 13(5):874. https://doi.org/10.3390/sym13050874
Chicago/Turabian StyleGülşen, Selahattin, Shao-Wen Yao, and Mustafa Inc. 2021. "Lie Symmetry Analysis, Conservation Laws, Power Series Solutions, and Convergence Analysis of Time Fractional Generalized Drinfeld-Sokolov Systems" Symmetry 13, no. 5: 874. https://doi.org/10.3390/sym13050874
APA StyleGülşen, S., Yao, S.-W., & Inc, M. (2021). Lie Symmetry Analysis, Conservation Laws, Power Series Solutions, and Convergence Analysis of Time Fractional Generalized Drinfeld-Sokolov Systems. Symmetry, 13(5), 874. https://doi.org/10.3390/sym13050874





