Lorentz Symmetry Group, Retardation and Energy Transformations in a Relativistic Engine
Abstract
1. Introduction
2. Energy and Momentum Conservation
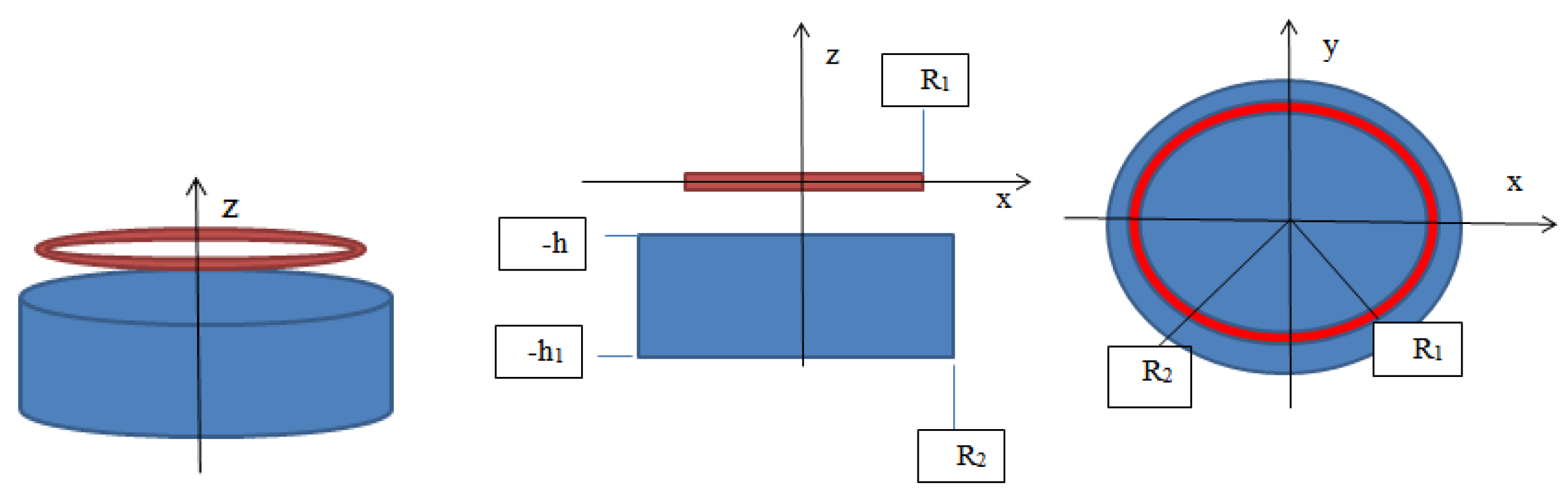
3. The Case of Two Currents Loops of Arbitrary Geometry
3.1. Proposed Experiment
The Problem of Current Switching
4. Field Energy
5. Field Energy of a System of Two Current Loops
5.1. Normalization
5.2.
5.3.
5.4.
5.5.
5.6.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Energy Balance from n = 0 to n = 4
Appendix A.1. n = 0
Appendix A.1.1. Power
Appendix A.1.2. Field Energy
Appendix A.1.3. Poynting Vector
Appendix A.1.4. Intermediate Account
Appendix A.2. n = 1
Appendix A.2.1. Power
Appendix A.2.2. Field Energy
Appendix A.2.3. Poynting Vector
Appendix A.2.4. Intermediate Account
Appendix A.3. n = 2
Appendix A.3.1. Power
Appendix A.3.2. Field Energy
Appendix A.3.3. Poynting Vector
Appendix A.3.4. Intermediate Account
Appendix A.4. n = 3
Appendix A.4.1. Power
Appendix A.4.2. Field Energy
Appendix A.4.3. Poynting Vector
Appendix A.4.4. Intermediate Account
Appendix A.5. n = 4
Appendix A.5.1. Power
Appendix A.5.2. Field Energy
Appendix A.5.3. Poynting Vector
Appendix A.5.4. Intermediate Account
Appendix B. On the Nullification of a Certain Integral
Appendix C. Asymptotic Values
Appendix D. Q Function Evaluation
Appendix E. Asymptotic Form of
Appendix F. Evaluating PFb
References
- Einstein, A. On the Electrodynamics of Moving Bodies. Ann. Phys. 1905, 17, 891–921. [Google Scholar] [CrossRef]
- Maxwell, J.C. A dynamical theory of the electromagnetic field. Philos. Trans. R. Soc. Lond. 1865, 155, 459–512. [Google Scholar]
- Jackson, J.D. Classical Electrodynamics, 3rd. ed.; Wiley: New York, NY, USA, 1999. [Google Scholar]
- Feynman, R.P.; Leighton, R.B.; Sands, M.L. Feynman Lectures on Physics, Revised 50th Anniversary Edition; Basic Books: New Yrok, NY, USA, 2011. [Google Scholar]
- Heaviside, O. On the Electromagnetic Effects due to the Motion of Electrification through a Dielectric. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1889, 27, 324–339. [Google Scholar] [CrossRef]
- Newton, I. Philosophiae Naturalis Principia Mathematica; Benjamin Motte: London, UK, 1687. [Google Scholar]
- Goldstein, H.; Poole, C.P., Jr.; Safko, J.L. Classical Mechanics, 3rd ed.; Pearson: London, UK, 2001. [Google Scholar]
- Tuval, M.; Yahalom, A. Momentum Conservation in a Relativistic Engine. Eur. Phys. J. Plus 2016, 131, 374. [Google Scholar] [CrossRef]
- Pryce, M.H.L. The mass-centre in the restricted theory of relativity and its connexion with the quantum theory of elementary particles. Proc. Roy. Soc. Lond. Ser. A 1948, 195, 62–81. [Google Scholar]
- SBosanac, S.D. Dynamics of Particles and the Electromagnetic Field; World Scientific: Singapore, 2005; Chapter 6. [Google Scholar]
- Mansuripur, M. Trouble with the Lorentz Law of Force: Incompatibility with Special Relativity and Momentum Conservation. Phys. Rev. Lett. 2012, 108, 193901. [Google Scholar] [CrossRef] [PubMed]
- Griffiths, D.J.; Heald, M.A. Time dependent Generalizations of the Biot-Savart and Coulomb laws. Am. J. Phys. 1991, 59, 111–117. [Google Scholar] [CrossRef]
- Jefimenko, O.D. Electricity and Magnetism, Appleton-Century Crofts, New York, 1966, 2nd ed.; Electret Scientific: Star City, WV, USA, 1989. [Google Scholar]
- Tuval, M.; Yahalom, A. Newton’s Third Law in the Framework of Special Relativity. Eur. Phys. J. Plus 2014, 129, 240. [Google Scholar] [CrossRef]
- Tuval, M.; Yahalom, A. A Permanent Magnet Relativistic Engine. In Proceedings of the Ninth International Conference on Materials Technologies and Modeling (MMT-2016), Ariel, Israel, 5–29 July 2016. [Google Scholar]
- Yahalom, A. Retardation in Special Relativity and the Design of a Relativistic Motor. Acta Phys. Pol. A 2017, 131, 1285–1288. [Google Scholar] [CrossRef]
- Yahalom, A. Preliminary Energy Considerations in a Relativistic Engine. In Proceedings of the Israeli-Russian Bi-National Workshop, The Optimization of Composition, Structure and Properties of Metals, Oxides, Composites, Nano- and Amorphous Materials, Ariel, Israel, 28–31 August 2017; pp. 203–213. [Google Scholar]
- Rajput, S.; Yahalom, A. Preliminary Magnetic Energy Considerations in a Relativistic Engine: Mutual Inductance vs. Kinetic Terms. In Proceedings of the 2018 IEEE International Conference on the Science of Electrical Engineering in Israel (ICSEE), Eilat, Israel, 12–14 December 2018; pp. 1–5. [Google Scholar] [CrossRef]
- Rajput, S.; Yahalom, A. Material Engineering and Design of a Relativistic Engine: How to Avoid Radiation Losses. Adv. Eng. Forum 2020, 36, 126–131. [Google Scholar] [CrossRef]

| n | |
|---|---|
| 0 | −7.18 |
| 1 | −6.74 |
| 2 | −4.94 |
| 3 | 0 |
| 4 | 11.97 |
| 5 | 39.48 |
| 6 | 101.03 |
| Parameter | Value |
|---|---|
| 1000 | |
| 1000 | |
| 100 A | |
| 100 A | |
| h | 0.1 m |
| 0.3 ns | |
| 10 ns |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rajput, S.; Yahalom, A.; Qin, H. Lorentz Symmetry Group, Retardation and Energy Transformations in a Relativistic Engine. Symmetry 2021, 13, 420. https://doi.org/10.3390/sym13030420
Rajput S, Yahalom A, Qin H. Lorentz Symmetry Group, Retardation and Energy Transformations in a Relativistic Engine. Symmetry. 2021; 13(3):420. https://doi.org/10.3390/sym13030420
Chicago/Turabian StyleRajput, Shailendra, Asher Yahalom, and Hong Qin. 2021. "Lorentz Symmetry Group, Retardation and Energy Transformations in a Relativistic Engine" Symmetry 13, no. 3: 420. https://doi.org/10.3390/sym13030420
APA StyleRajput, S., Yahalom, A., & Qin, H. (2021). Lorentz Symmetry Group, Retardation and Energy Transformations in a Relativistic Engine. Symmetry, 13(3), 420. https://doi.org/10.3390/sym13030420








