Spatial Dependence of the Dipolar Interaction between Quantum Dots and Organic Molecules Probed by Two-Color Sum-Frequency Generation Spectroscopy
Abstract
1. Introduction
2. Materials and Methods
3. Results
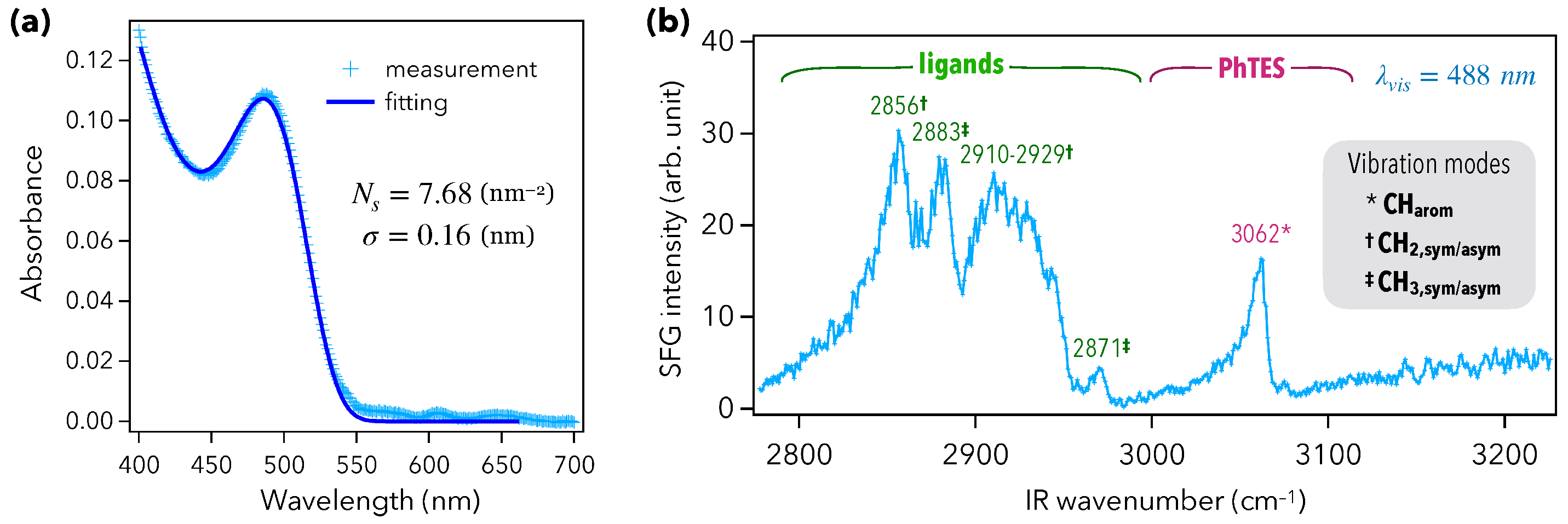
3.1. Structural and Chemical Characterizations of the QD/Molecule Interface
3.2. Vibroelectronic Correlation between QDs and Aromatic Rings
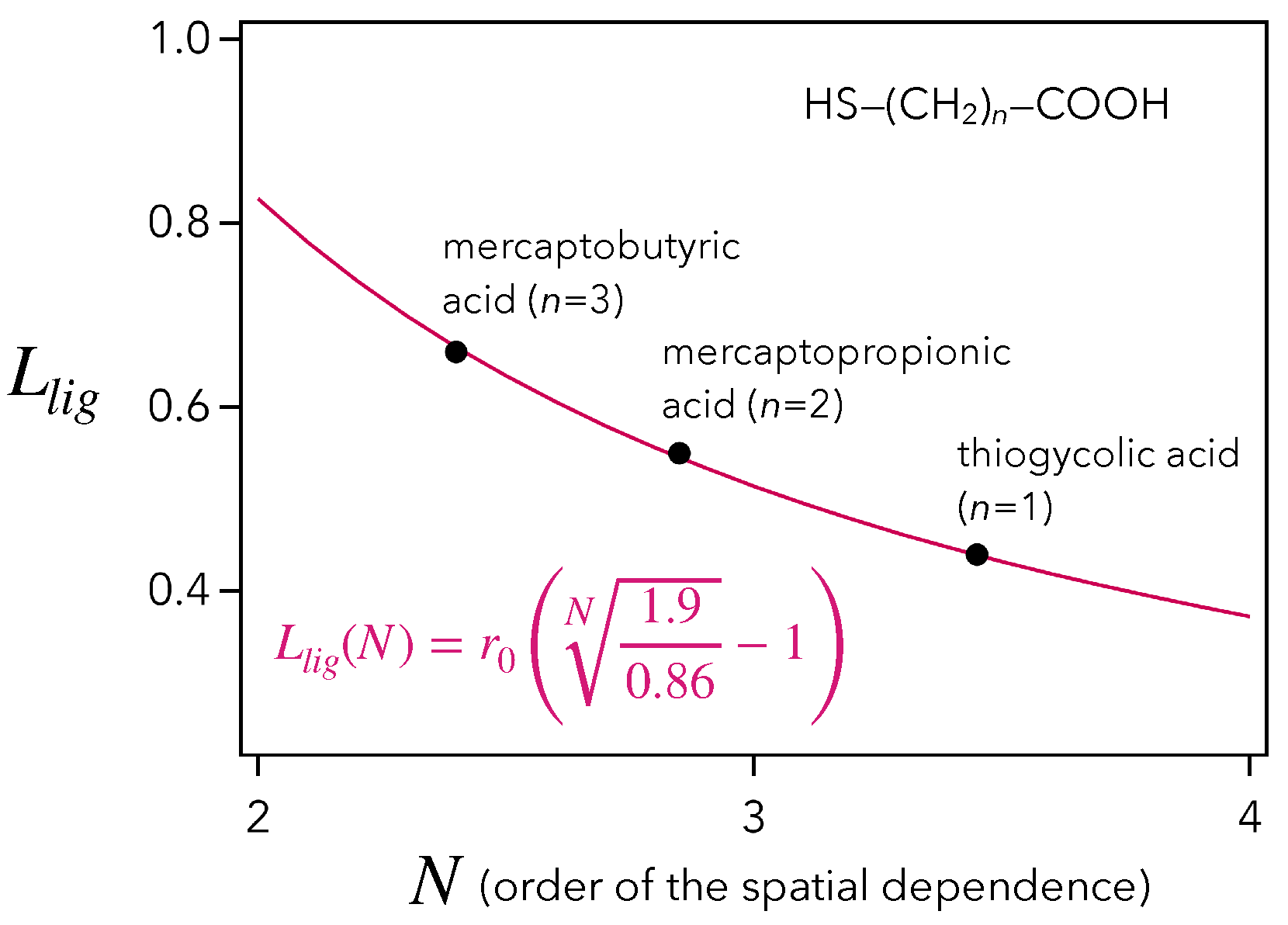
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| QD | Quantum Dot |
| 2C-SFG | Two-color Sum-Frequency Generation |
| ATR | Attenuated Total Reflection |
| PhTES | Triethoxyphenylsilane |
| APTES | 3-aminopropyltriethoxysilane |
References
- Brus, L.E. Electron-electron and electron-hole interactions in small semiconductor crystallites: The size dependence of the lowest excited electronic states. J. Chem. Phys. 1984, 80, 4403–4409. [Google Scholar] [CrossRef]
- Masumoto, Y.; Sonobe, K. Size-dependent energy levels of CdTe quantum dots. Phys. Rev. B 1997, 56, 9734–9737. [Google Scholar] [CrossRef]
- Wang, X.; Yu, W.W.; Zhang, J.; Aldana, J.; Peng, X.; Xiao, M. Photoluminescence upconversion in colloidal CdTe quantum dots. Phys. Rev. B 2003, 68, 125318–125323. [Google Scholar] [CrossRef]
- Wuister, S.F.; Swart, I.; van Driel, F.; Hickey, S.G.; de Mello Donegá, C. Highly Luminescent Water-Soluble CdTe Quantum Dots. Nano Lett. 2003, 3, 503–507. [Google Scholar] [CrossRef]
- Sapra, S.; Sarma, D.D. Evolution of the electronic structure with size in II-VI semiconductor nanocrystals. Phys. Rev. B 2004, 69, 125304–125310. [Google Scholar] [CrossRef]
- Law, M.; Beard, M.C.; Choi, S.; Luther, J.M.; Hanna, M.C.; Nozik, A.J. Determining the Internal Quantum Efficiency of PbSe Nanocrystal Solar Cells with the Aid of an Optical Model. Nano Lett. 2008, 8, 3904–3910. [Google Scholar] [CrossRef] [PubMed]
- Zhao, H.; Fan, Z.; Liang, H.; Selopal, G.S.; Gonfa, B.A.; Jin, L.; Soudi, A.; Cui, D.; Enrichi, F.; Natile, M.M.; et al. Controlling photoinduced electron transfer from PbS@CdS core@shell quantum dots to metal oxide nanostructured thin films. Nanoscale 2014, 6, 7004. [Google Scholar] [CrossRef]
- Cao, S.W.; Yuan, Y.P.; Fang, J.; Shahjamali, M.M.; Boey, F.Y.C.; Barber, J.; Loo, S.C.J.; Xue, C. In-situ growth of CdS quantum dots on g-C3N4 nanosheets for highly efficient photocatalytic hydrogen generation under visible light irradiation. Int. J. Hydrog. Energy 2013, 38, 1258–1266. [Google Scholar] [CrossRef]
- Xiao, F.X.; Miao, J.; Liu, B. Layer-by-Layer Self-Assembly of CdS Quantum Dots/Graphene Nanosheets Hybrid Films for Photoelectrochemical and Photocatalytic Applications. J. Am. Chem. Soc. 2014, 136, 1559–1569. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, H.; Navarro-Pardo, F.; Selopal, G.S.; Sun, S.; Wang, Z.M.; Zhao, H.; Rosei, F. Hybrid surface passivation of PbS/CdS quantum dots for efficient photoelectrochemical hydrogen generation. Appl. Surf. Sci. 2020, 530, 147252. [Google Scholar] [CrossRef]
- Heuff, R.F.; Swift, J.L.; Cramb, D.T. Fluorescence correlation spectroscopy using quantum dots: Advances, challenges and opportunities. Phys. Chem. Chem. Phys. 2007, 9, 1870–1880. [Google Scholar] [CrossRef]
- Hottechamps, J.; Noblet, T.; Brans, A.; Humbert, C.; Dreesen, L. How Quantum Dots Aggregation Enhances Förster Resonant Energy Tranfer. ChemPhysChem 2020, 21, 853–862. [Google Scholar] [CrossRef] [PubMed]
- Medintz, I.L.; Uyeda, H.T.; Goldman, E.R.; Mattoussi, H. Quantum dot bioconjugates for imaging, labelling and sensing. Nat. Mater. 2005, 4, 435–446. [Google Scholar] [CrossRef]
- Yong, K.T.; Law, W.C.; Roy, I.; Jing, Z.; Huang, H.; Swihart, M.T.; Prasad, P.N. Aqueous phase synthesis of CdTe quantum dots for biophotonics. J. Biophotonics 2011, 4, 9–20. [Google Scholar] [CrossRef]
- Li, J.; Zhu, J.J. Quantum dots for fluorescent biosensing and bio-imaging applications. Analyst 2013, 138, 2506–2515. [Google Scholar] [CrossRef]
- Tyrakowski, C.M.; Snee, P.T. A primer on synthesis, water-solubilization, and functionalization of quantum dots, their use as biological sensing agents, and present status. Phys. Chem. Chem. Phys. 2014, 16, 837–855. [Google Scholar] [CrossRef]
- Wegner, K.D.; Hildebrandt, N. Quantum dots: Bright and versatile in vitro and in vivo fluorescence imaging biosensors. Chem. Soc. Rev. 2015, 44, 4792–4834. [Google Scholar] [CrossRef]
- Dubertret, B. Quantum dots: DNA detectives. Nat. Mater. 2005, 4, 797–798. [Google Scholar] [CrossRef] [PubMed]
- Liu, P.; Wang, Q.; Li, X. Studies on CdSe/L-cysteine Quantum Dots Synthesized in Aqueous Solution for Biological Labeling. J. Phys. Chem. C 2009, 113, 7670–7676. [Google Scholar] [CrossRef]
- Zahavy, E.; Freeman, E.; Lustig, S.; Keysary, A.; Yitzhaki, S. Double Labeling and Simultaneous Detection of B- and T Cells Using Fluorescent Nano-Crystal (q-dots) in Paraffin-Embedded Tissues. J. Fluoresc. 2005, 15, 661. [Google Scholar] [CrossRef] [PubMed]
- Goldman, E.R.; Clapp, A.R.; Anderson, G.P.; Uyeda, H.; Mauro, J.M.; Medintz, I.L.; Mattoussi, H. Multiplexed Toxin Analysis Using Four Colors of Quantum Dot Fluororeagents. Anal. Chem. 2004, 76, 684–688. [Google Scholar] [CrossRef] [PubMed]
- Susha, A.S.; Javier, A.M.; Parak, W.J.; Rogach, A.L. Luminescent CdTe nanocrystals as ion probes and pH sensors in aqueous solutions. Colloids Surfaces A Physicochem. Eng. Asp. 2006, 281, 40–43. [Google Scholar] [CrossRef]
- Generalova, A.N.; Oleinikov, V.A.; Zarifullina, M.M.; Lankina, E.V.; Sizova, S.V.; Artemyev, M.V.; Zubov, V.P. Optical sensing quantum dot-labeled polyacrolein particles prepared by layer-by-layer deposition technique. J. Colloid Interface Sci. 2011, 357, 265–272. [Google Scholar] [CrossRef]
- Mrad, R.; Poggi, M.; Chaâbane, R.B.; Negrerie, M. Role of surface defects in colloidal cadmium selenide (CdSe) nanocrystals in the specificity of fluorescence quenching by metal cations. J. Colloid Interface Sci. 2020, 571, 368–377. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Zhou, Z.; Yang, B. The Influence of Carboxyl Groups on the Photoluminescence of Mercaptocarboxylic Acid-Stabilized CdTe Nanoparticles. J. Phys. Chem. B 2003, 107, 8–13. [Google Scholar] [CrossRef]
- Frederick, M.T.; Amin, V.A.; Weiss, E.A. Optical Properties of Strongly Coupled Quantum Dot-Ligand Systems. J. Phys. Chem. Lett. 2013, 4, 634–640. [Google Scholar] [CrossRef]
- Liang, Y.; Thorne, J.E.; Parkinson, B.A. Controlling the Electronic Coupling between CdSe Quantum Dots and Thiol Capping Ligands via pH and Ligand Selection. Langmuir 2012, 28, 11072–11077. [Google Scholar] [CrossRef] [PubMed]
- Jin, S.; Harris, R.D.; Lau, B.; Aruda, K.O.; Amin, V.A.; Weiss, E.A. Enhanced Rate of Radiative Decay in CdSe Quantum Dots upon Adsorption of an Exciton-Delocalizing Ligand. Nano Lett. 2014, 14, 5323–5328. [Google Scholar] [CrossRef] [PubMed]
- Noblet, T.; Dreesen, L.; Boujday, S.; Méthivier, C.; Busson, B.; Tadjeddine, A.; Humbert, C. Semiconductor quantum dots reveal dipolar coupling from exciton to ligand vibration. Commun. Chem. 2018, 1, 76. [Google Scholar] [CrossRef]
- Noblet, T.; Boujday, S.; Méthivier, C.; Erard, M.; Hottechamps, J.; Busson, B.; Humbert, C. Two-Dimensional Layers of Colloidal CdTe Quantum Dots: Assembly, Optical Properties, and Vibroelectronic Coupling. J. Phys. Chem. C 2020, 124, 25873–25883. [Google Scholar] [CrossRef]
- Humbert, C.; Noblet, T. A Unified Mathematical Formalism for First to Third Order Dielectric Response of Matter: Application to Surface-Specific Two-Colour Vibrational Optical Spectroscopy. Symmetry 2021, 13, 153. [Google Scholar] [CrossRef]
- Barbillon, G.; Noblet, T.; Busson, B.; Tadjeddine, A.; Humbert, C. Localised detection of thiophenol with gold nanotriangles highly structured as honeycombs by nonlinear Sum Frequency Generation spectroscopy. J. Mater. Sci. 2018, 53, 4554–4562. [Google Scholar] [CrossRef]
- Noblet, T.; Dreesen, L.; Hottechamps, J.; Humbert, C. A global method for handling fluorescence spectra at high concentration derived from the competition between emission and absorption of colloidal CdTe quantum dots. Phys. Chem. Chem. Phys. 2017, 19, 26559–26565. [Google Scholar] [CrossRef]
- Dalstein, L.; Haddada, M.B.; Barbillon, G.; Humbert, C.; Tadjeddine, A.; Boujday, S.; Busson, B. Revealing the Interplay between Adsorbed Molecular Layers and Gold Nanoparticles by Linear and Nonlinear Optical Properties. J. Phys. Chem. C 2015, 119, 17146–17155. [Google Scholar] [CrossRef]
- Humbert, C.; Pluchery, O.; Lacaze, E.; Tadjeddine, A.; Busson, B. Optical spectroscopy of functionalized gold nanoparticles assemblies as a function of the surface coverage. Gold Bull. 2013, 46, 299–309. [Google Scholar] [CrossRef]
- Tourillon, G.; Dreesen, L.; Volcke, C.; Sartenaer, Y.; Thiry, P.A.; Peremans, A. Total internal reflection sum-frequency generation spectroscopy and dense gold nanoparticles monolayer: A route for probing adsorbed molecules. Nanotechnology 2007, 18, 415301–415307. [Google Scholar] [CrossRef]
- Chen, F.; Gozdzialski, L.; Hung, K.K.; Stege, U.; Hore, D.K. Assessing the Molecular Specificity and Orientation Sensitivity of Infrared, Raman, and Vibrational Sum-Frequency Spectra. Symmetry 2021, 13, 42. [Google Scholar] [CrossRef]
- Zhuang, X.; Miranda, P.B.; Kim, D.; Shen, Y.R. Mapping molecular orientation and conformation interfaces by surface nonlinear optics. Phys. Rev. B 1999, 59, 12632–12640. [Google Scholar] [CrossRef]
- Sperling, R.A.; Parak, W.J. Surface modification, functionalization and bioconjugation of colloidal inorganic nanoparticles. Phil. Trans. R. Soc. A 2010, 368, 1333–1383. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Noblet, T.; Dreesen, L.; Tadjeddine, A.; Humbert, C. Spatial Dependence of the Dipolar Interaction between Quantum Dots and Organic Molecules Probed by Two-Color Sum-Frequency Generation Spectroscopy. Symmetry 2021, 13, 294. https://doi.org/10.3390/sym13020294
Noblet T, Dreesen L, Tadjeddine A, Humbert C. Spatial Dependence of the Dipolar Interaction between Quantum Dots and Organic Molecules Probed by Two-Color Sum-Frequency Generation Spectroscopy. Symmetry. 2021; 13(2):294. https://doi.org/10.3390/sym13020294
Chicago/Turabian StyleNoblet, Thomas, Laurent Dreesen, Abderrahmane Tadjeddine, and Christophe Humbert. 2021. "Spatial Dependence of the Dipolar Interaction between Quantum Dots and Organic Molecules Probed by Two-Color Sum-Frequency Generation Spectroscopy" Symmetry 13, no. 2: 294. https://doi.org/10.3390/sym13020294
APA StyleNoblet, T., Dreesen, L., Tadjeddine, A., & Humbert, C. (2021). Spatial Dependence of the Dipolar Interaction between Quantum Dots and Organic Molecules Probed by Two-Color Sum-Frequency Generation Spectroscopy. Symmetry, 13(2), 294. https://doi.org/10.3390/sym13020294






