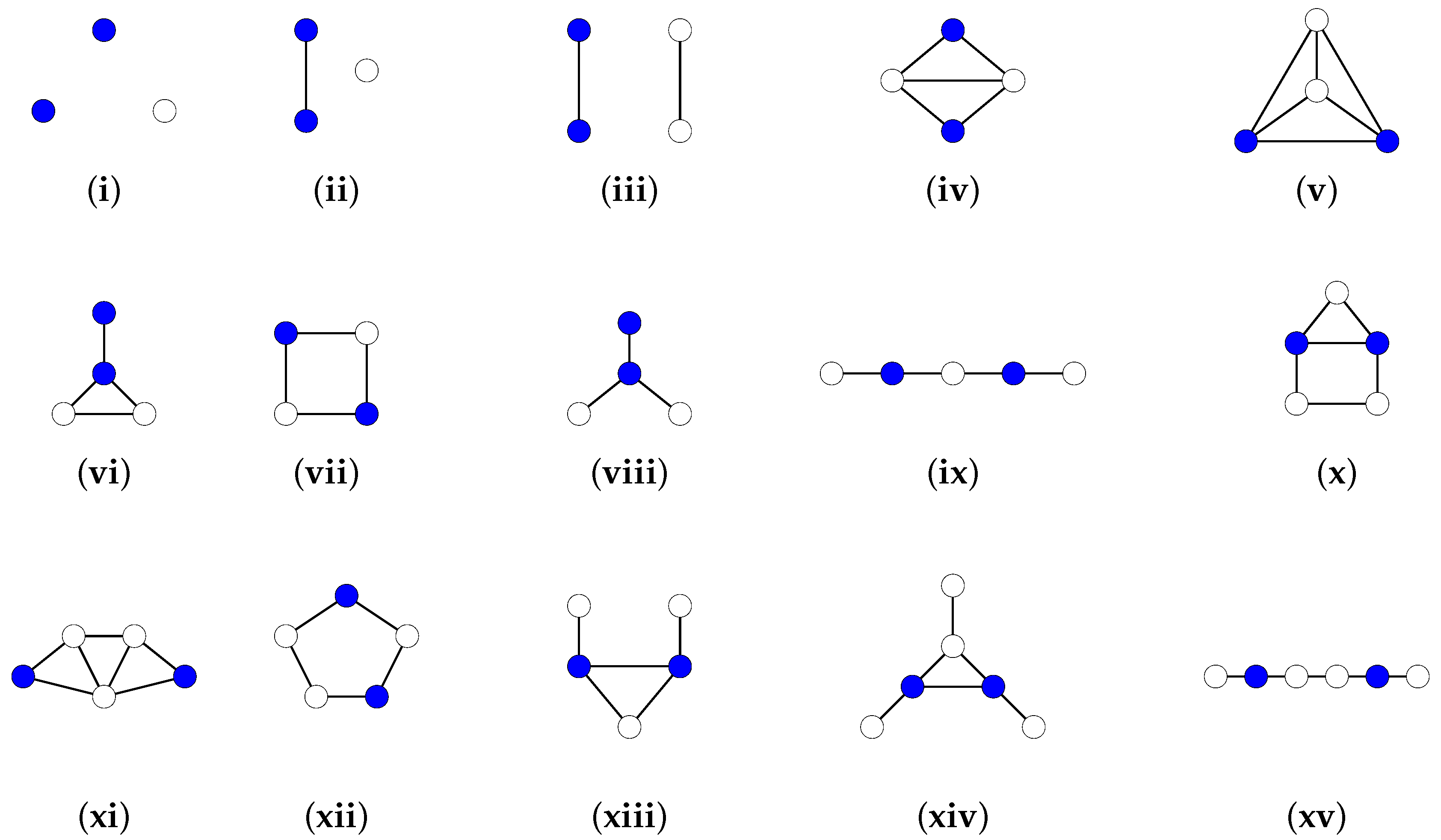
It is not difficult to verify that each of these graphs has a failed zero forcing number of 2. For the sake of completeness, we include the details. For each graph, the larger vertices give a lower bound for the failed zero forcing number of 2. For the disconnected graphs (i)–(iii), the upper bound for follows since coloring any three vertices blue forces all of the vertices to be colored blue. For the five connected graphs with four vertices (iv)–(viii) the upper bound follows since . For the two paths (ix) and (xv), the coloring of any three vertices blue will result in either a vertex of degree 1 being colored blue or two adjacent vertices colored blue, both of which will force all of the vertices in the graph to be blue. For the graph of coloring any three vertices blue will mean that two adjacent vertices were colored blue, which will force the remaining vertices in the graph. For the house graph (x) note that coloring any three of the vertices in the graph blue will either include two vertices of the triangle or three vertices in the both of which will force all vertices in the graph to be blue. For the gem fan graph (xii) with five vertices, coloring three vertices blue will either include the vertex of degree four and two other vertices, or three vertices of degree two or three, both of which will force all vertices in the graph to be blue. For the graph consisting of a triangle and two pendant edges (xiii) note that coloring any three vertices blue will either include the three vertices of degree greater than 1, one vertex of degree 1, and two vertices of degree greater than 1, or two vertices of degree 1, all of which will force all vertices to be colored blue. Finally for the triangle with three pendant edges (xiv), coloring any three vertices blue will either include all three vertices of degree greater than 1, or at least one vertex of degree 1 and two other vertices that are either degree 1 or greater than 1, all of which will force all vertices to be blue.
We next present a series of lemmas that will be used to prove Theorem 1.
This theorem can be applied to determine all disconnected graphs with a particular failed zero forcing number. We show the result for the case where .
In the next three lemmas we identify families of graphs that have a failed zero forcing number of at least 3. These will be used to prove Theorem 1.
2.1. Graphs with Six Vertices: Exception Cases
We will consider the 112 connected graphs with six vertices. We will refer to the table and numbering found in [
8]. For convenience, we explicitly show each graph in each of the individual cases.
Out of 112 different graphs, there were 16 cases where there does not exist three blue vertices, none of which are true blue. We will refer to these as our exception cases. We will show that each of the exception cases with six vertices can either be extended to graphs with seven, eight, or nine vertices where there are three blue vertices with none of which are true blue, or the extension includes a pendant V, which would be ruled out by Lemma 6. In each of the cases below when identifying the three blue vertices, none of which are true blue, it can be assumed that all other vertices can be colored white.
This will not only show that they have a failed zero forcing number of at least 3, but they can be extended indefinitely by adding vertices and edges and the resulting supergraph will also have a failed zero forcing number of at least 3.
These graphs are the exceptions: 54, 58, 59, 60, 76, 77, 80, 85, 86, 87, 96, 98, 102, 103, 105, and 112, each of which we will present individually. Graph 54: Please refer to
Figure 5.
We begin by extending graph 54 by one vertex v and connecting it to each vertex in G. Notice there will be no case where v is adjacent to or . This is because connecting a vertex to either one will create a cherry structure and that case is covered by our cherry lemma. Our goal with extending is to find no true blue vertex within the graph. It is also important to notice that in each case, we are able to find a failed zero forcing number of three.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be colored blue. If v is adjacent to , then and can be colored blue. If v is adjacent to , then and can be colored blue.
If v is adjacent to only then G contains a pendant cherry and is removed from consideration by Lemma 6.
If v is adjacent to only then G contains a pendant cherry and is removed from consideration by Lemma 6.
If v is adjacent to and then and can be colored blue.
Since all cases can be extended indefinitely, this implies that graph 54 can be extended indefinitely without being forced with
. Graph 58: Please refer to
Figure 6.
Let be the vertex of degree 1 and let be the neighbor of ; is the vertex of degree 3 that is adjacent to , , and . Let be the vertex of degree 3 that is adjacent to ; vertices and both have degree 2. We consider extending the graph by adding a new vertex v.
If v is adjacent to then and can be colored blue.
If v is adjacent to then and can be colored blue.
If v is adjacent to then and can be colored blue.
If v is adjacent to and then and can be colored blue.
In all of the above cases, we are left without any true blue vertices and thus we can extend these graphs indefinitely with .
If v is adjacent to only then the graph contains a cherry and is removed from consideration by Lemma 6.
The only problem cases is where
v is adjacent to
or
(but not both). Here we can find a failed zero forcing set
S of size 3 by selecting
,
, and
, however we may not be able to extend
S to larger graphs. Without loss of generality assume that
v is adjacent to
. We consider adding a new vertex
u with possible neighbors. We note that
u cannot have degree 1 and be adjacent to
or
since this would create a cherry and this case would be ruled out by Lemma 6. If
u is adjacent to both
or
then we can color
u,
, and
blue. If
u is adjacent to
v then we could color
v,
, and
blue. If
u is adjacent to
then we could color
,
, and
blue. If
u is adjacent to
then we could color
, and
blue. If
u is adjacent to
then we could color
, and
blue. If
u is adjacent to
then we could color
, and
blue. Graph 59: Please refer to
Figure 7.
Let be the vertex of degree 1, be the vertex of degree 4 that is adjacent to , be the vertex of degree 2 that is adjacent to , be the other vertex of degree 4, be the vertex of degree 3 that is adjacent to and , and be the vertex of degree 2 that is adjacent to and . Graph 59 follows with the case of graph 58. We can extend along any vertex without any true blue vertices with the only exception being when a new vertex is adjacent to vertex . Similarly to graph 58 however, upon any additional extension, there are no issues arising when all the vertices are reshuffled.
For the sake of completeness we include the details. We consider adding a new vertex
v and possible neighbors of
v. The vertex
v cannot have degree 1 and be adjacent to
since this would create a cherry. In each case of the remaining cases we can find a coloring with 3 blue vertices, none of which are true blue vertices, and all of the remaining vertices including
v will be colored white. If
v is adjacent to
, then
, and
can be colored blue. If
v is adjacent to
, then
, and
can be colored blue. If
v is adjacent to
then
and
can be colored blue. If
v is adjacent to
, then
,
and
can be colored blue. The only problem case is if
v is adjacent to
. Here we can find a failed zero forcing set
S of size 3 with vertices
,
, and
, but we many not be able to extend this graph. Here we consider adding another vertex
u. The vertex
u cannot have degree 1 and be adjacent to either
or
since this would create a cherry and we can remove it from consideration by Lemma 2.6. If
u is adjacent to
v, then we color
v,
, and
blue. If
u is adjacent to
, then we color
,
, and
blue. If
u is adjacent to
, then we color
,
, and
blue. If
u is adjacent to
, then we color
,
, and
blue. If
u is adjacent to
, then we color
,
, and
blue. If
u is adjacent to both
and
then we color
u,
, and
blue. Graph 60: Please refer to
Figure 8.
Graph 60 has at least one true blue vertex but all extensions along graph 60 result in
and no true blue vertices. Let
be the vertex of degree 1,
be the neighbor of
,
be the vertex of degree 4,
be the vertex of degree 3 that is adjacent to
and
,
be the vertex of degree 3 adjacent to
, and let
be the vertex of degree 2. We consider adding another vertex
v and possible neighbors of
v. First if
v has degree 1 then it cannot be adjacent to
since this would create a cherry. In each of the remaining cases we can find a coloring with 3 blue vertices, none of which are true blue vertices, and all of the remaining vertices including
v will be colored white. If
v is adjacent to
then we can color
,
, and
blue, if
v is adjacent to
then
,
, and
can be colored blue. If
v is adjacent to
then
,
and
can be colored blue and if
v is adjacent to
then
,
and
can be colored blue, and if
v is adjacent to
then
,
and
can be colored blue. Graph 76: Please refer to
Figure 9.
We will show that all but two extensions of G to a graph with 7 vertices will have no true blue vertex of degree 1. Let v denote the new vertex. Note that extensions that would result in a cherry are neglected as those cases are resolved by Lemma 6. We have the following cases
If v is adjacent to only, we color , , and blue and v, , and white.
We consider cases of adding a new vertex v.
If v is adjacent to , we color , , and blue.
If v is adjacent to , we color , , and blue.
If v is adjacent to or only, then this case can be removed by Lemma 6.
If v is adjacent to both and only, then color v, , and blue.
The only problem cases is where v is adjacent to or .
Without loss of generality, we assume v is adjacent to . Then we consider adding another vertex u.
If u is adjacent to v then color v, , and blue.
If u is adjacent to then color , , and blue.
If u is adjacent to then color , , and blue.
If u is adjacent to then color , , and blue.
If u is adjacent to , , or only then this case can be removed by Lemma 6.
If u is adjacent to , , and then we can color , , and blue.
If u is adjacent to and only then we can color , , and blue.
If u is adjacent to and only then we can color u, , and blue.
If u is adjacent to and only then we can color , , and blue.
Since in each of these extensions we have three blue vertices none of which are true blue, we can extend these graphs indefinitely. Graph 77: Please refer to
Figure 10. Suppose we add a new vertex called
v.
If v is adjacent to or only then we can remove this case by Lemma 6. Our goal with extending is to find no true blue vertex. If we do have a true blue, we must then extend further.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be colored blue.
There are two cases in which we cannot not obtain three non-true blue vertices. The first is when v is adjacent to and the second is when v is adjacent to both and .
Case 1. If v is adjacent to , then and v can be filled. For this case, we must extend further since there is a true blue vertex. Suppose we add a second vertex called u.
If u is adjacent to v then , and can be colored blue.
If u is adjacent to then , and can be colored blue.
If u is adjacent to then , and can be colored blue.
If u is adjacent to then , and can be colored blue.
If u is adjacent to , or only then we can remove this case by Lemma 6.
If u is adjacent to and then , and can be colored blue.
If u is adjacent to and then , and can be colored blue.
If u is adjacent to and then , and ccan be colored blue.
Case 2. If v is adjacent to both and then we consider adding another vertex w.
If w is adjacent to v then , and can be colored blue.
If w is adjacent to then , and can be colored blue.
If w is adjacent to then , and can be colored blue.
If w is adjacent to then , and can be colored blue.
If w is adjacent to then , and can be colored blue.
The cases where w is adjacent to or only can be removed from consideration by Lemma 6.
If w is adjacent to and then , and can be colored blue.
Thus, we can extend all cases indefinitely without ever forcing G. Since each case is extendable, then graph 77 is extendable.
Notice there is no case where v is adjacent to or . This is because connecting a vertex to either one would create a cherry structure and that case is covered elsewhere. It is also important to notice that in each case, we are able to find a failed zero forcing number of three.
Every blue vertex has two white neighbors, so we can add any number of edges or vertices to any of these graphs without forcing G. This is because the three blue vertices will always be adjacent to two white neighbors and cannot force any other vertex. Thus, for graph 77.
Graph 80: Please refer to
Figure 11. It will be helpful to refer to
Figure 11. Let the vertex on the far left be
. Let the vertex adjacent to
be
. Let the top vertex adjacent to
be
and the bottom be
. Let the vertex that is adjacent to both
and
on the right be
. Furthermore, finally, let the vertex on the far right side be
. We begin by extending graph 80 by one vertex,
v and connecting
v to every vertex in
G. We can rule out the case where
v is adjacent to
or
only by Lemma 6. If
v is adjacent to both
and
then then we can color vertices
v,
, and
blue and all other vertices white. This is because connecting a vertex to either would create a cherry structure and that case is covered elsewhere.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then , and can be colored blue.
In all cases, there are no true blue vertices. Thus, all cases are extendable and this implies graph 80 can be extended indefinitely. It is also important to notice that in each case, we are able to find a failed zero forcing number of three. Graph 85: Please refer to
Figure 12.
We will show that all but two extensions of G to a graph with 7 vertices have no true blue vertex of degree 1. Let v denote the new vertex. Note that extensions that would result in a cherry are neglected as those cases are resolved by Lemma 6. We consider the following cases.
If v is adjacent to only, we color , , and blue.
If v is adjacent to only, we color , , and blue.
If v is adjacent to only, then , , and can be colored blue.
If v is adjacent to only then this case can be removed by Lemma 2.6.
The only problems are where v is adjacent to or .
First, we consider the case where v is adjacent to .
Then we add another vertex u.
If u is adjacent to or only then this case can be removed by Lemma 2.6.
If u is adjacent to and , then u, , and can be colored blue.
If u is adjacent to v, then v, , and can be colored blue.
If u is adjacent to , then , , and can be colored blue.
If u is adjacent to , then , , and can be colored blue.
If u is adjacent to , then , , and can be colored blue.
If u is adjacent to , then , , and can be colored blue.
First, we consider the case where v is adjacent to .
Then we add another vertex w.
If w is adjacent to v, then v, , and can be colored blue.
If w is adjacent to , then , , and can be colored blue.
If w is adjacent to , then , , and can be colored blue.
If w is adjacent to , then , , and can be colored blue.
If w is adjacent to , then , , and can be colored blue.
If w is adjacent to or only then this case can be removed by Lemma 2.6.
If
w is adjacent to
and
, then
u,
, and
can be colored blue. Graph 86: Please refer to
Figure 13.
We will show that all extensions of G to a graph with 7 vertices have no true blue vertices of degree 1. Let v be the new vertex. Let be the vertex of degree 1, be the vertex of degree 2 that is adjacent to , be the vertex of degree 3 that is adjacent to and on the left of , be the vertex of degree 2 that is adjacent to , be the vertex of degree 2 that is adjacent to , and be the vertex of degree 3 that is adjacent to . We have the following cases.
If v is adjacent to only, we color , , and blue.
If v is adjacent to only, the resulting graph contains a cherry. So we color v, white, and color the remaining vertices blue.
If v is adjacent to only, we color , , and blue.
If v is adjacent to only, we color , , and blue.
The remaining cases of connecting
v to
G are analogous to one of the previous four. Furthermore, note that in each case where the resulting graph does not contain a cherry, we can always color
v white and add edges between
v and any subset of the remaining vertices without affecting the number of blue vertices. Graph 87: Please refer to
Figure 14.
All but one of the single vertex extensions of G is either a cherry or suitable for extension by Lemma 3. Let be the only vertex of degree one that is adjacent to ; is adjacent to and ; will be adjacent to and and will be adjacent to and .
If v is adjacent to , we can fill in , , and .
If v is adjacent to , it creates a cherry and this case can be discarded.
If v is adjacent to , we can fill in , , and .
If v is adjacent to , we can fill in , , and .
If v is adjacent to , we can fill in , , and .
If v is adjacent to , we must have a true blue vertex and thus this graph must be extended further. We can then add in an additional vertex, u.
If u is adjacent to , we can fill in , , and .
If u is adjacent to , it again creates a cherry and we can discard this case.
If u is adjacent to , we can fill in , , and .
If u is adjacent to , we can fill in , , and .
If u is adjacent to , we get another cherry and thus another discarded case.
If u is adjacent to , we can fill in , , and .
If u is adjacent to v, then we can fill v, , and .
In every case, we see a suitable coloring for extension by Lemma 3. Thus, we may extend graph 87 to a super-graph
H, and by Lemma 3,
. Graph 96: Please refer to
Figure 15.
We will show that any extension of G to a graph with 7 vertices will have at most one true blue vertex. Let the vertices of the triangle be and which are adjacent to vertices of degree 1, and , respectively.
Consider the addition of a new vertex v. We first consider if v is adjacent to or . Without loss of generality assume v is adjacent to . Then we color and blue and and white. If v is adjacent to one of the vertices or only then we apply Lemma 6. If v is adjacent to all of the vertices and then we can color and blue. The only case that is a problem is where v is adjacent to exactly two of the vertices and . Without loss of generality we assume that v is adjacent to and .
Here we add another vertex u.
If u is adjacent to , , and then these cases are similar to the previous cases involving v,
If u is adjacent to v then we can color v, , and blue.
If u is adjacent to exactly one of , , and then this case can be removed by Lemma 6.
If u is adjacent to and only then we can color u, v, and blue.
If u is adjacent to and only then we can color , , and blue.
If u is adjacent to and only then we can color , , and blue.
If
u is adjacent to
,
, and
then we can color
,
, and
blue. Graph 98: Please refer to
Figure 16.
We begin by extending graph 98 by one vertex, v and connecting v to every vertex in G.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be colored blue.
The case where v is adjacent to , , or only can be removed by Lemma 6.
If v is adjacent to and , then and can be colored blue.
If v is adjacent to and , then and can be colored blue.
If v is adjacent to and , then and can be colored blue.
We can see in all cases there are no true blue vertices. Thus, all cases are extendable and this implies graph 98 can be extended indefinitely. It is also important to notice that in each case, we are able to find a failed zero forcing set of size three. Graph 102: Please refer to
Figure 17.
We begin by extending graph 102 by one vertex, v and connecting v to every vertex in G. Due to the symmetry of the graph, we can ignore the case where v is adjacent to . Notice there will be no case where v is adjacent to or . This is because connecting a vertex to either one will create a cherry structure, and this is covered by Lemma 6.
We have to consider the case where v is adjacent to both and . In this case, we have to add a new vertex u.
If u is adjacent to v, then , and can be colored blue.
If u is adjacent to , then , and can be colored blue.
If u is adjacent to , then and can be colored blue. If u is adjacent to , then and can be colored blue. If u is adjacent to , then and can be colored blue. If u is adjacent to or only then this can be removed by Lemma 6.
If u is adjacent to and then and can be colored blue.
If v is adjacent to , then and can be colored blue. Due to the symmetry of the graph, this case is the same as when v is adjacent to . So it can be ignored. However, this case has a true blue and so it must be extended. Suppose we add a new vertex u.
If u is adjacent to , then and can be filled.
If u is adjacent to , then and can be filled. Here vertex u is true blue, so we must extend further. Suppose we add another vertex called w.
If w is adjacent to , then and can be filled.
The only problem case left is if w is adjacent to only. This would create a with a pendant edge at each vertex.
Here we can add another vertex x.
If x is adjacent to v, then and can be colored blue.
If x is adjacent to w, then and can be colored blue. If x is adjacent to , then and can be colored blue.
If x is adjacent to , then and can be colored blue.
If x is adjacent to , , , and only then this case can be removed by Lemma 6.
If x is adjacent to and , then and can be colored blue.
If x is adjacent to and , then and can be colored blue.
If x is adjacent to and , then and can be colored blue.
If x is adjacent to and , then and can be colored blue.
Notice there are no true blue vertices and that in all cases. Since there are no true blue vertices, then this case is now extendable. Since each case is extendable, then graph 102 can be extended indefinitely without being forced.
Graph 103: Please refer to
Figure 18. We begin by extending graph 103 by one vertex,
v, and connecting
v to every vertex in G. Notice there will be no case where
v is adjacent to
or
. This is because connecting a vertex to either would create a cherry structure and that case is covered by the cherry lemma. It is also important to notice that in each case and sub-case, we are able to find a failed zero forcing number of three. Our goal with extending is to find no true blue vertex within the graph. Due to the symmetry of the graph, we can ignore the cases where
v is adjacent to
and
.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then this reverts to the extension of Graph 102 where the added vertex v is adjacent to .
Since there are no more true blue vertices, then this case is resolved and can be extended indefinitely. Since each case is extendable, this implies that graph 103 can also be extended indefinitely without being forced. Graph 105: Please refer to
Figure 19.
We begin by extending graph 105 by one vertex, v and connecting v to every vertex in G. Notice there will be no case where v is adjacent to . This is because adding an extension to would create a cherry structure and this case is covered by the cherry lemma. It is also important to notice that in each case, we are able to find a failed zero forcing number of three. Our goal with extending is to find no true blue vertex within the graph.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be colored blue.
If v is adjacent to , then and can be filled.
Notice the last two cases have a true blue vertex. Without loss of generality assume that v is adjacent to .
Suppose we add a new vertex u.
If u is adjacent to or only then this case can be removed by Lemma 6.
If u is adjacent to v, then and can be colored blue.
If u is adjacent to , then and can be colored blue.
If u is adjacent to , then and can be colored blue.
If u is adjacent to and , then and can be colored blue.
If u is adjacent to , then and can be colored blue.
If u is adjacent to , then and can be colored blue.
Since there are no more true blue vertices in these sub-cases, then this case is resolved and can be extended indefinitely. Since each case is extendable, this implies that graph 105 can be extended indefinitely without being forced and .
: Any extension of to a graph with 7 vertices will have at most one true blue vertex.
We consider adding a vertex v to this graph. If v is adjacent to then we can color vertex v white and color vertices and blue to create a failed zero forcing set of size 3. If v is adjacent to then we can color vertex v white and color vertices and blue to create a failed zero forcing set of size 3. If v is not adjacent to either or then v must be adjacent to one or more of the vertices or . We consider different cases, each showing that we can create a failed zero forcing set of size 3 with at most one true blue vertex. We consider a series of different cases.
If v is adjacent to only one of the vertices , , , or then this creates a pendant V which can be removed from consideration by Lemma 6.
If v is adjacent to and only then we color and blue.
If v is adjacent to and only then we color and blue.
If v is adjacent to and only then we color and blue.
If v is adjacent to and only then we color and blue.
If v is adjacent to and only then we color , and blue.
If v is adjacent to and only then we color and blue.
If v is adjacent to and only, then we color , and blue.
The only problem case is where v is adjacent to both and . In this case we add a new vertex u.
If u is adjacent to v then we color and blue.
If u is adjacent to then we color and blue.
If u is adjacent to then we color and blue.
If u is adjacent to then we color and blue.
If u is adjacent to then we color and blue.
If u is adjacent to or only then this case can be removed from consideration by Lemma 6.
If u is adjacent to and then we color u, v, and blue.