Synchrotron Radiation in Periodic Magnetic Fields of FEL Undulators—Theoretical Analysis for Experiments
Abstract
1. Introduction
2. Spontaneous UR intensity and Spectrum Distortions
3. Analysis of the Harmonic Generation in Some FEL Experiments
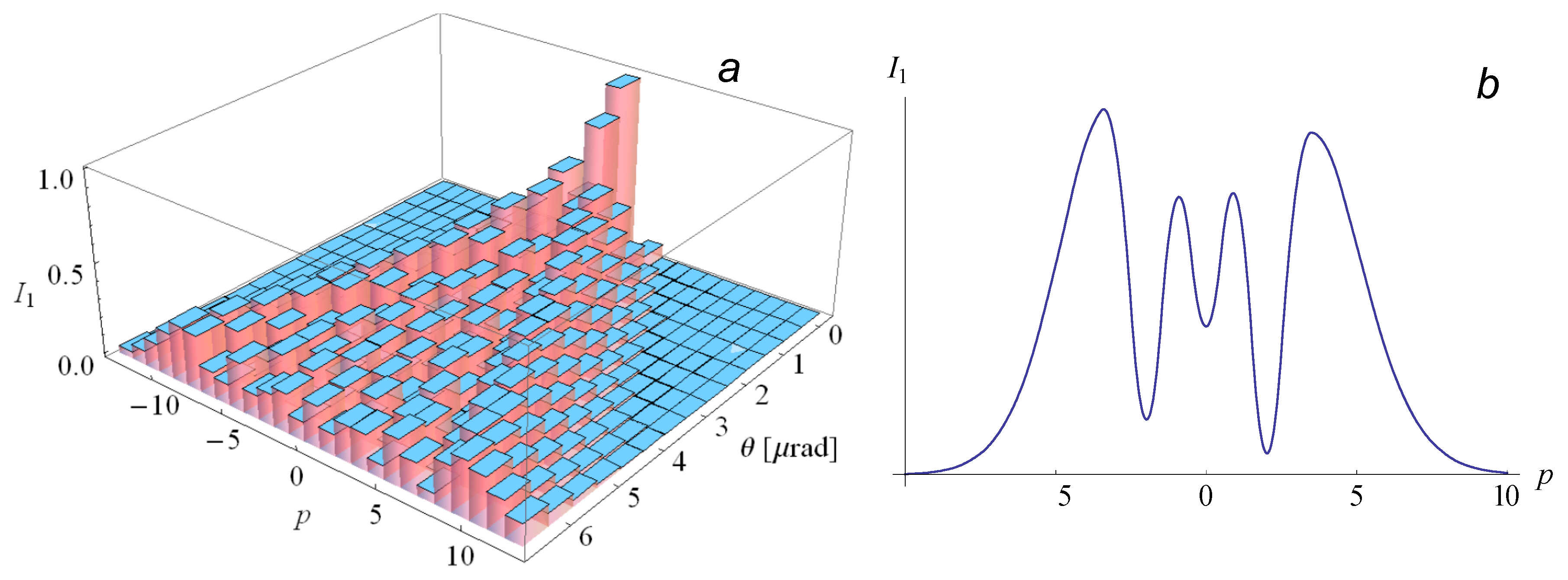
3.1. SACLA FEL Experiment
3.2. POHANG FEL X-ray Experiments
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Phenomenological Model of Harmonic Power Evolution in High-Gain FELs
References
- Ginzburg, V.L. On the radiation of microradiowaves and their absorbtion in the air. Isvestia Akad. Nauk. SSSR (Fizika) 1947, 11, 1651. [Google Scholar]
- Motz, H.; Thon, W.; Whitehurst, R.N.J. Experiments on radiation by fast electron beams. Appl. Phys. 1953, 24, 826. [Google Scholar] [CrossRef]
- McNeil, B.W.J.; Thompson, N.R. X-ray free-electron lasers. Nat. Photonics 2010, 4, 814. [Google Scholar] [CrossRef]
- Pellegrini, C.; Marinelli, A.; Reiche, S. The physics of X-ray free-electron lasers. Rev. Mod. Phys. 2016, 88, 015006. [Google Scholar] [CrossRef]
- Schmüser, P.; Dohlus, M.; Rossbach, J.; Behrens, C. Free-Electron Lasers in the Ultraviolet and X-ray Regime. In Springer Tracts in Modern Physics; Springer: Cham, Switzerland, 2014; Volume 258. [Google Scholar]
- Huang, Z.; Kim, K.J. Review of X-ray free-electron laser theory. Phys. Rev. ST-AB 2007, 10, 034801. [Google Scholar] [CrossRef]
- Margaritondo, G.; Ribic, P.R. A simplified description of X-ray free-electron lasers. J. Synchrotron Rad. 2011, 18, 101–108. [Google Scholar] [CrossRef] [PubMed]
- Margaritondo, G. Synchrotron light: A success story over six decades. Riv. Nuovo Cim. 2017, 40, 411–471. [Google Scholar]
- Bagrov, V.G.; Bisnovaty-Kogan, G.S.; Bordovitsyn, V.A.; Borisov, A.V.; Dorofeev, O.F.; Ya, E.V.; Pivovarov, Y.L.; Shorokhov, O.V.; Zhukovsky, V.C. Synchrotron Radiation Theory and Its Development; Bordovitsyn, V.A., Ed.; Word Scientific: Singapore, 1999; p. 447. [Google Scholar]
- Margaritondo, G. Characteristics and Properties of Synchrotron Radiation. In Synchrotron Radiation; Mobilio, S., Boscherini, F., Meneghini, C., Eds.; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Dattoli, G.; Ottaviani, P.L. Semi-analytical models of free electron laser saturation. Opt. Commun. 2002, 204, 283–297. [Google Scholar] [CrossRef]
- Zhukovsky, K.; Kalitenko, A. Phenomenological and numerical analysis of power evolution and bunching in single-pass X-ray FELs. J. Synchrotron Rad. 2019, 26, 159–169. [Google Scholar] [CrossRef] [PubMed]
- Zhukovsky, K.; Kalitenko, A. Analysis of harmonic generation in planar undulators in single-pass free electron lasers. Russ. Phys. J. 2019, 61, 153–160. [Google Scholar]
- Zhukovsky, K.; Kalitenko, A. Phenomenological and numerical analysis of power evolution and bunching in single-pass X-ray FELs. Erratum. J. Synchrotron Rad. 2019, 26, 605–606. [Google Scholar] [CrossRef] [PubMed]
- Zhukovsky, K. Two-frequency undulator in a short SASE FEL for angstrom wavelengths. J. Opt. 2018, 20, 095003. [Google Scholar] [CrossRef]
- Zhukovsky, K. Analysis of harmonic generation in planar and elliptic bi-harmonic undulators and FELs. Results Phys. 2019, 13, 102248. [Google Scholar] [CrossRef]
- Zhukovsky, K.V. Analytical Description of Nonlinear Harmonic Generation Close to the Saturation Region in Free Electron Lasers. Mosc. Univ. Phys. Bull. 2019, 74, 480–487. [Google Scholar] [CrossRef]
- Emma, P. First lasing of the LCLS X-ray FEL at 1.5 Å. In Proceedings of the PAC09, Vancouver, BC, Canada, 4–8 May 2009. [Google Scholar]
- Emma, P.; Akre, R.; Arthur, J.; Bionta, R.; Bostedt, C.; Bozek, J.; Brachmann, A.; Bucksbaum, P.; Coffee, R.; Decker, F.-J.; et al. First lasing and operation of an angstrom-wavelength free-electron laser. Nat. Photonics 2010, 4, 641–647. [Google Scholar] [CrossRef]
- Ratner, D.; Brachmann, A.; Decker, F.J.; Ding, Y.; Dowell, D.; Emma, P.; Frisch, J.; Huang, Z.; Iverson, R.; Krzywinski, J.; et al. Second and third harmonic measurements at the linac coherent light source. Phys. Rev. ST-AB 2011, 14, 060701. [Google Scholar] [CrossRef]
- Biedron, S.G.; Dejusa, R.J.; Huanga, Z.; Miltona, S.V.; Sajaeva, V.; Berga, W.; Borlanda, M.; den Hartoga, P.K.; Erdmanna, M.; Fawleyc, W.M.; et al. Measurements of nonlinear harmonic generation at the Advanced Photon Source’s SASE FEL. Nucl. Instrum. Meth. Phys. Res. 2002, 483, 94–100. [Google Scholar] [CrossRef]
- Milton, S.V.; Gluskin, E.; Arnold, N.D.; Benson, C.; Berg, W.; Biedron, S.G.; Borland, M.; Chae, Y.C.; Dejus, R.J.; den Hartog, P.K.; et al. Exponential Gain and Saturation of a Self-AmpliÞed Spontaneous Emission Free-Electron Laser. Science 2011, 292, 2037–2041. [Google Scholar] [CrossRef]
- Huang, Z.; Kim, K.-J. Nonlinear Harmonic Generation of Coherent Amplification and Self-Amplified Spontaneous Emission. Nucl. Instr. Meth. 2001, 475, 112. [Google Scholar] [CrossRef]
- Zhukovsky, K.V. Effect of the 3rd undulator field harmonic on spontaneous and stimulated undulator radiation. J. Synchrotron Rad. 2019, 26, 1481–1488. [Google Scholar] [CrossRef]
- Zhukovsky, K.V. Generation of UR Harmonics in Undulators with Multiperiodic Fields. Russ. Phys. J. 2019, 62, 1043–1053. [Google Scholar] [CrossRef]
- Alexeev, V.I.; Bessonov, E.G. On some methods of generating circularly polarized hard undulator radiation. Nucl. Instr. Meth. 1991, 308, 140. [Google Scholar]
- Dattoli, G.; Mikhailin, V.V.; Ottaviani, P.L.; Zhukovsky, K. Two-frequency undulator and harmonic generation by an ultrarelativistic electron. J. Appl. Phys. 2006, 100, 084507. [Google Scholar] [CrossRef]
- Zhukovsky, K. Analytical account for a planar undulator performance in a constant magnetic field. J. Electromagn. Waves Appl. 2014, 28, 1869–1887. [Google Scholar] [CrossRef]
- Dattoli, G.; Mikhailin, V.V.; Zhukovsky, K. Undulator radiation in a periodic magnetic field with a constant component. J. Appl. Phys. 2008, 104, 124507-1–124507-8. [Google Scholar] [CrossRef]
- Dattoli, G.; Mikhailin, V.V.; Zhukovsky, K.V. Influence of a constant magnetic field on the radiation of a planar undulator. Mosc. Univ. Phys. Bull. 2009, 64, 507–512. [Google Scholar] [CrossRef]
- Zhukovsky, K.; Srivastava, H. Operational solution of non-integer ordinary and evolution-type partial differential equations. Axioms 2016, 5, 29. [Google Scholar] [CrossRef]
- Zhukovsky, K.V. Solving evolutionary-type differential equations and physical problems using the operator method. Theor. Math. Phys. 2017, 190, 52–68. [Google Scholar] [CrossRef]
- Zhukovsky, K.V. Operational solution for some types of second order differential equations and for relevant physical problems. J. Math. Anal. Appl. 2017, 446, 628–647. [Google Scholar] [CrossRef]
- Dattoli, G.; Srivastava, H.M.; Zhukovsky, K.V. Orthogonality properties of the Hermite and related polynomials. J. Comput. Appl. Math. 2005, 182, 165–172. [Google Scholar] [CrossRef]
- Dattoli, G.; Srivastava, H.M.; Zhukovsky, K. Operational Methods and Differential Equations with Applications to Initial-Value problems. Appl. Math. Comput. 2007, 184, 979–1001. [Google Scholar] [CrossRef]
- Zhukovsky, K.V. The operational solution of fractional-order differential equations, as well as Black-Scholes and heat-conduction equations. Mosc. Univ. Phys. Bull. 2016, 71, 237–244. [Google Scholar] [CrossRef]
- Zhukovsky, K. Operational Approach and Solutions of Hyperbolic Heat Conduction Equations. Axioms 2016, 5, 28. [Google Scholar] [CrossRef]
- Gould, H.W.; Hopper, A.T. Operational formulas connected with two generalizations of Hermite polynomials. Duke Math. J. 1962, 29, 51–63. [Google Scholar] [CrossRef]
- Kang, H.-S.; Chang-Ki, M.; Hoon, H.; Changbum, K.; Haeryong, Y.; Gyujin, K.; Inhyuk, N.; Soung, Y.B.; Hyo-Jin, C.; Geonyeong, M.; et al. Hard X-ray free-electron laser with femtosecondscale timing jitter. Nat. Photonics 2017, 11, 708–713. [Google Scholar] [CrossRef]
- Alferov, D.F.; Bashmakov Yu, A.; Bessonov, E.G. Undulator radiation. Sov. Phys. Tech. Phys. 1974, 18, 1336. [Google Scholar]
- Alferov, D.F.; Bashmakov, U.A.; Cherenkov, P.A. Radiation from relativistic electrons in a magnetic undulator. Usp. Fis. Nauk. 1989, 32, 200. [Google Scholar]
- Vinokurov, N.A.; Levichev, E.B. Undulators and wigglers for the production of radiation and other applications. Phys. Usp. 2015, 58, 917–939. [Google Scholar] [CrossRef]
- Dattoli, G.; Renieri, A.; Torre, A. Lectures on the Free Electron Laser Theory and Related Topics; World Scientific: Singapo, 1993. [Google Scholar]
- Prakash, B.; Huse, V.; Gehlot, M.; Mishra, G.; Mishra, S. Analysis of spectral properties of harmonic undulator radiation of anelectromagnet undulator. Optik 2016, 127, 1639–1643. [Google Scholar] [CrossRef]
- Saldin, E.L.; Schneidmiller, E.A.; Yurkov, M.V. The Physics of Free Electron Lasers; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Bonifacio, R.; Pellegrini, C.; Narducci, L. Collective instabilities and high-gain regime in a free electron laser. Opt. Commun. 1984, 50, 373. [Google Scholar] [CrossRef]
- Bonifacio, R.; De Salvo, L.; Pierini, P. Large harmonic bunching in a high-gain free-electron laser. Nucl. Instrum. A 1990, 293, 627. [Google Scholar] [CrossRef]
- Huang, Z.; Kim, K.-J. Three-dimensional analysis of harmonic generation in high-gain free-electron lasers. Phys. Rev. E 2000, 62, 7295. [Google Scholar] [CrossRef] [PubMed]
- Saldin, E.L.; Schneidmiller, E.A.; Yurkov, M.V. Study of a noise degradation of amplification process in a multistage HGHG FEL. Opt. Commun. 2002, 202, 169–187. [Google Scholar] [CrossRef]
- Shatan, T.; Yu, L.-H. High-gain harmonic generation free-electron laser with variable wavelength. Phys. Rev. E 2005, 71, 046501. [Google Scholar] [CrossRef] [PubMed]
- Dattoli, G.; Giannessi, L.; Ottaviani, P.L.; Ronsivalle, C. Semi-analytical model of self-amplified spontaneous-emission free-electron lasers, including diffraction and pulse-propagation effects. J. Appl. Phys. 2004, 95, 3206–3210. [Google Scholar] [CrossRef]
- Dattoli, G.; Ottaviani, P.L.; Pagnutti, S. Nonlinear harmonic generation in high-gain free-electron lasers. J. Appl. Phys. 2005, 97, 113102. [Google Scholar] [CrossRef]
- Dattoli, G.; Ottaviani, P.L.; Pagnutti, S. Booklet for FEL Design; ENEA Pubblicazioni: Frascati, Italy, 2007. [Google Scholar]
- Zhukovsky, K.V. Generation of Coherent Radiation in the Near X-ray Band by a Cascade FEL with a Two-Frequency Undulator. Mosc. Univ. Phys. Bull. 2018, 73, 364–371. [Google Scholar] [CrossRef]
- Zhukovsky, K. Compact single-pass X-ray FEL with harmonic multiplication cascades. Opt. Commun. 2018, 418, 57–64. [Google Scholar] [CrossRef]
- Zhukovsky, K. Soft X-ray generation in cascade SASE FEL with two-frequency undulator. EPL 2017, 119, 34002. [Google Scholar] [CrossRef]
- Zhukovsky, K.V. Generation of X-Ray Radiation in Free-Electron Lasers with Two-Frequency Undulators. Russ. Phys. J. 2018, 60, 1630–1637. [Google Scholar] [CrossRef]
- Zhukovsky, K. Generation of coherent soft X-ray radiation in short FEL with harmonic multiplication cascades and two-frequency undulator. J. Appl. Phys. 2017, 122, 233103. [Google Scholar] [CrossRef]
- Zhukovsky, K. High-harmonic X-ray undulator radiation for nanoscale-wavelength free-electron lasers. J. Phys. D 2017, 50, 505601. [Google Scholar] [CrossRef]
- Zhukovsky, K.; Potapov, I. Two-frequency undulator usage in compact self-amplified spontaneous emission free electron laser in Roentgen range. Laser Part. Beams 2017, 35, 326. [Google Scholar] [CrossRef]
- Tetsuya, I.; Hideki, A.; Takao, A.; Yoshihiro, A.; Noriyoshi, A.; Teruhiko, B.; Hiroyasu, E.; Kenji, F.; Toru, F.; Yukito, F.; et al. A compact X-ray free-electron laser emitting in the sub-ångström region. Nat. Photonics 2012, 6, 540–544. [Google Scholar]
- Owada, S.; Togawa, K.; Inagaki, T.; Hara, T.; Tanaka, T.; Joti, Y.; Koyama, T.; Nakajima, K.; Ohashi, H.; Senba, Y.; et al. Soft X-ray free-electron laser beamline at SACLA: The light source, photon beamline and experimental station. J. Synchrotron Rad. 2018, 25, 282–288. [Google Scholar] [CrossRef]
- Ego, H. RF system of the SPring-8 upgrade project. In Proceedings of the IPAC2016, Busan, Korea, 8−13 May 2016. [Google Scholar]
- Tono, K.; Hara, T.; Yabashi, M.; Tanaka, H. Multiple-beamline operation of SACLA. J. Synchrotron Rad. 2019, 26, 595–602. [Google Scholar] [CrossRef]
- Ichiro, I. Generation of narrow-band X-ray free-electron laser via reflection self-seeding. Nat. Photonics 2019, 13, 319–322. [Google Scholar]
- Tono, K.; Togashi, T.; Inubushi, Y.; Sato, T.; Katayama, T.; Ogawa, K.; Ohashi, H.; Kimura, H.; Takahashi, S.; Takeshita, K.; et al. Beamline, experimental stations and photon beam diagnostics for the hard X-ray free electron laser of SACLA. New J. Phys. 2013, 15, 083035. [Google Scholar] [CrossRef]
- Yabashi, M. Overview of the SACLA facility. J. Synchrotron Rad. 2015, 22, 477–484. [Google Scholar] [CrossRef]
- Yabashi, M.; Tanaka, H.; Tono, K.; Ishikawa, T. Status of the SACLA Facility. Appl. Sci. 2017, 7, 604. [Google Scholar] [CrossRef]
- Takashi, T.; Shunji, G.; Toru, H.; Takaki, H.; Haruhiko, O.; Kazuaki, T.; Makina, Y.; Hitoshi, T. Undulator commissioning by characterization of radiation in X-ray free electron lasers. Phys. Rev. ST-AB 2012, 15, 110701. [Google Scholar]
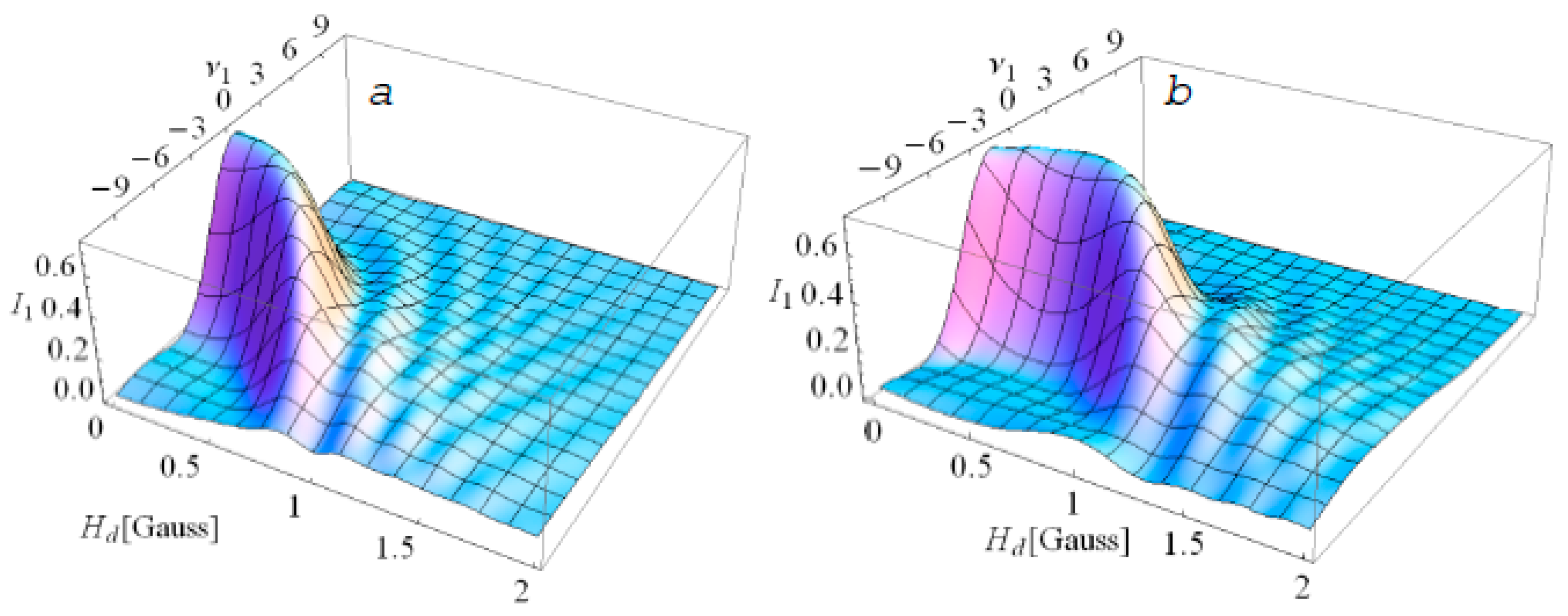


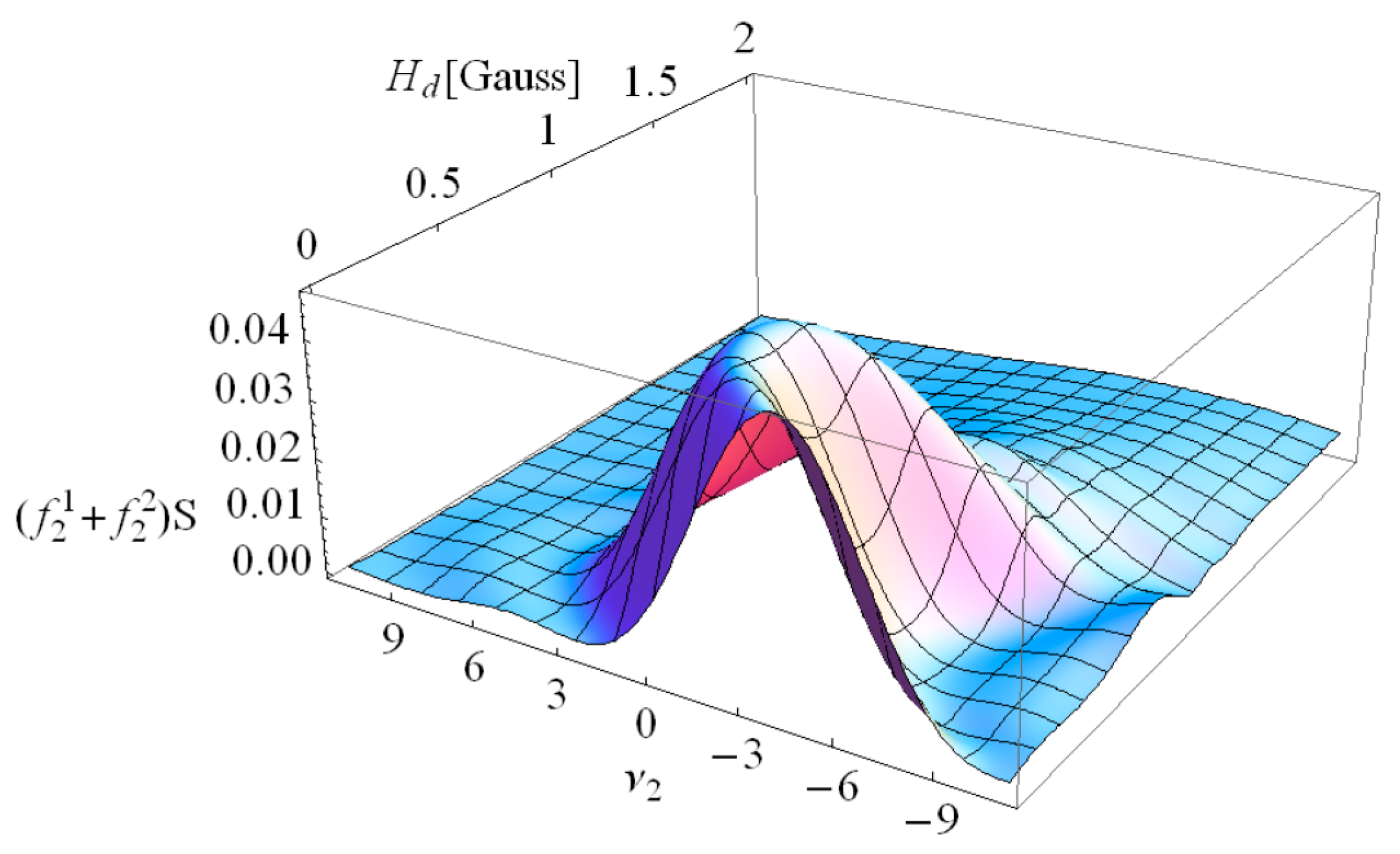

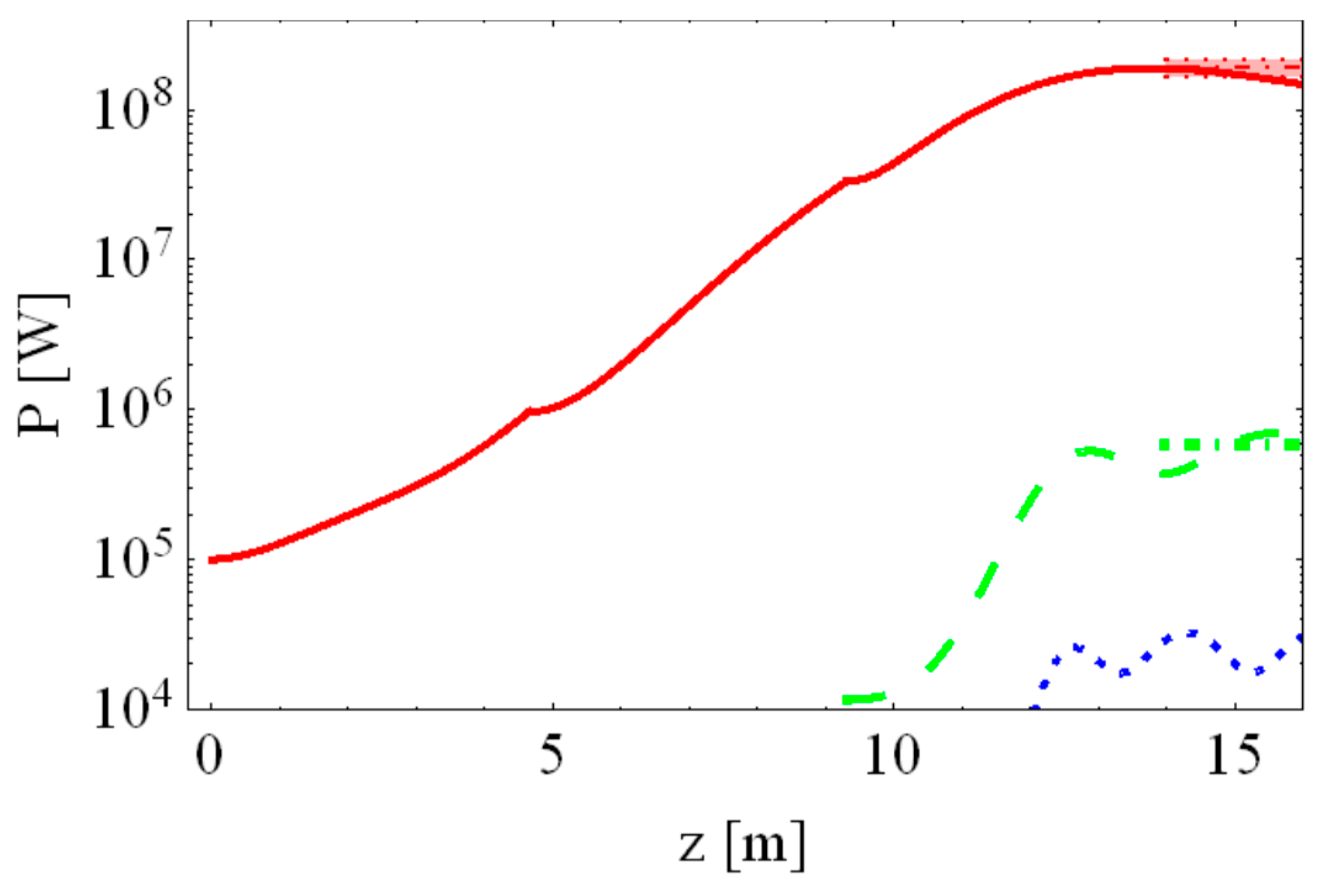

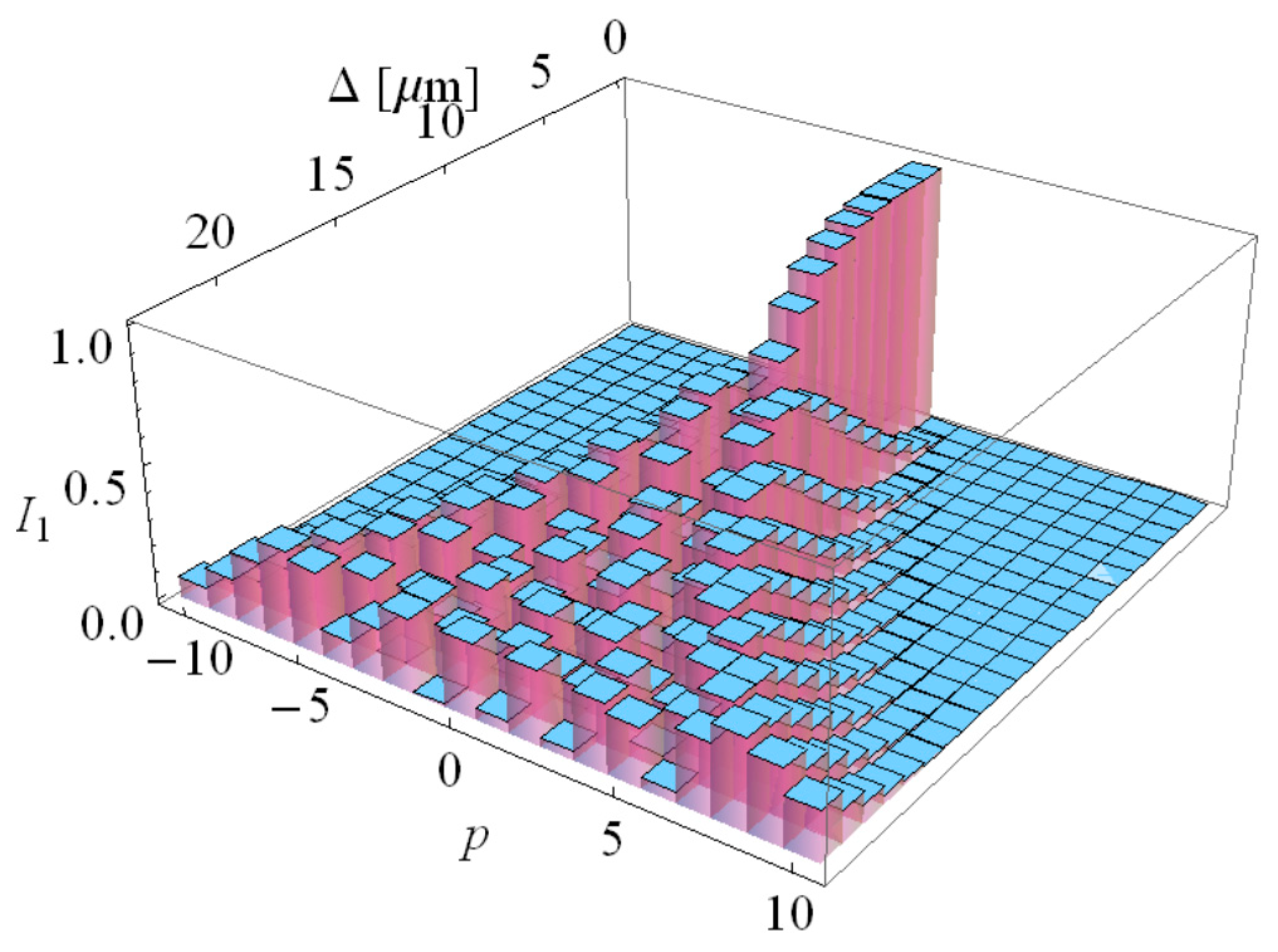
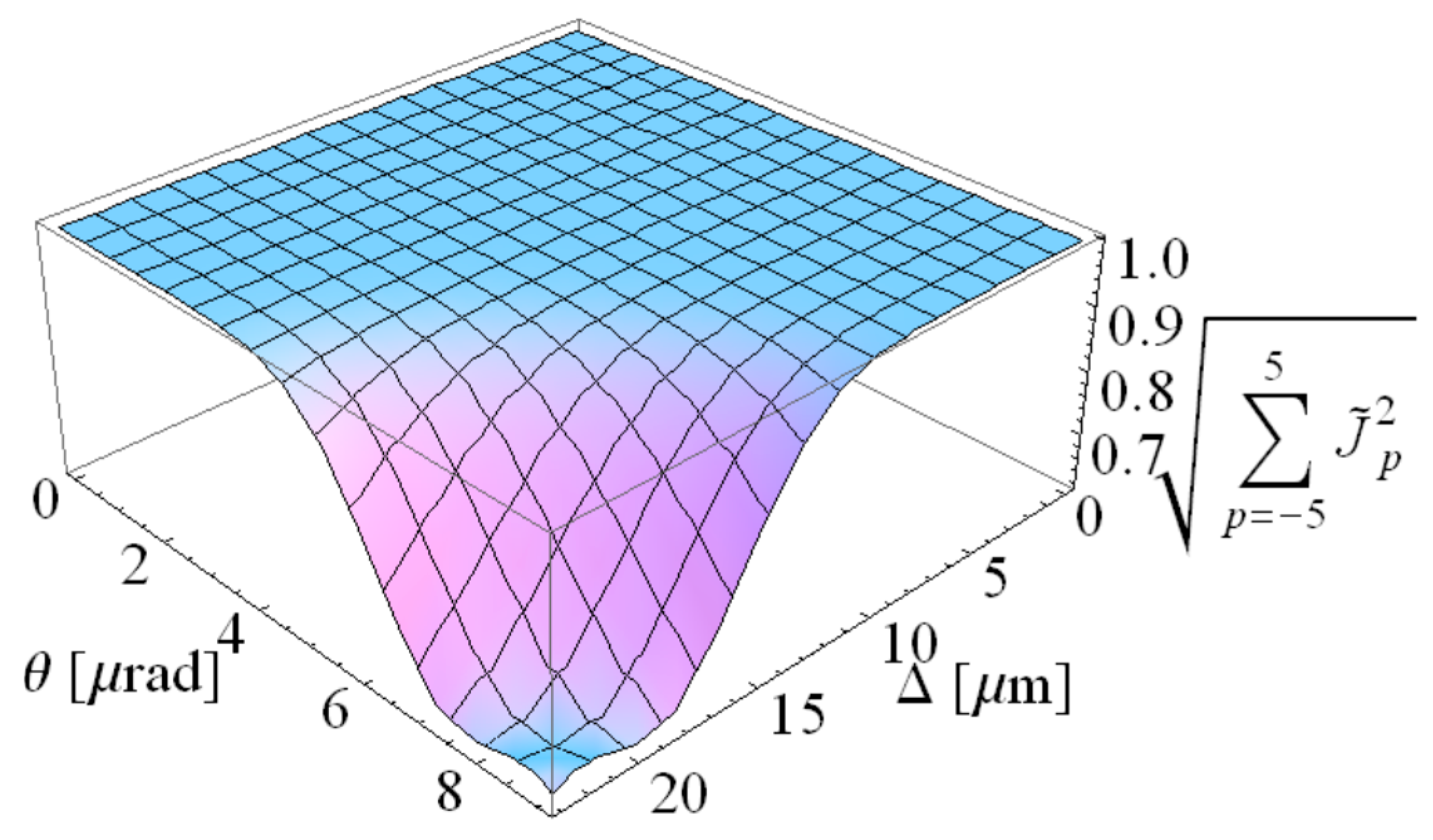

| Beam parameters: relativistic factor γ = 1526, beam power PE = 234 GW, current I0 = 300 A, current density J = 2.9 × 1010 A/m2, beam section ∑ = 1.03×10−8 m2, emittances μm, βx = 6 m, βy = 4 m, beam size ≈40 μm, divergence ≈ 8 μrad, ~40 μrad, γθ ≈ 0.06, energy spread (per slice) σe = 1.6 × 10−3 | |||||
| Undulator parameters: k = 2.1, λu = 1.8 cm, N = 259, section length 4.66 m | |||||
| Calculated FEL properties: saturated length Ls = 13 m, gain length Lgain = 1.1 m, radiation beam size ≅ 36 μm | |||||
| Harmonic number | n = 1 | n = 2 | n = 3 | n = 4 | n = 5 |
| Bessel coefficient fn | 0.79 | 0.09 | 0.32 | 0.09 | 0.18 |
| Pierce parameter | 0.0015 | 0.0003 | 0.0008 | 0.0003 | 0.0006 |
| Harmonic wavelength λn, nm | 12.4 | 6.2 | 4.1 | 3.1 | 2.5 |
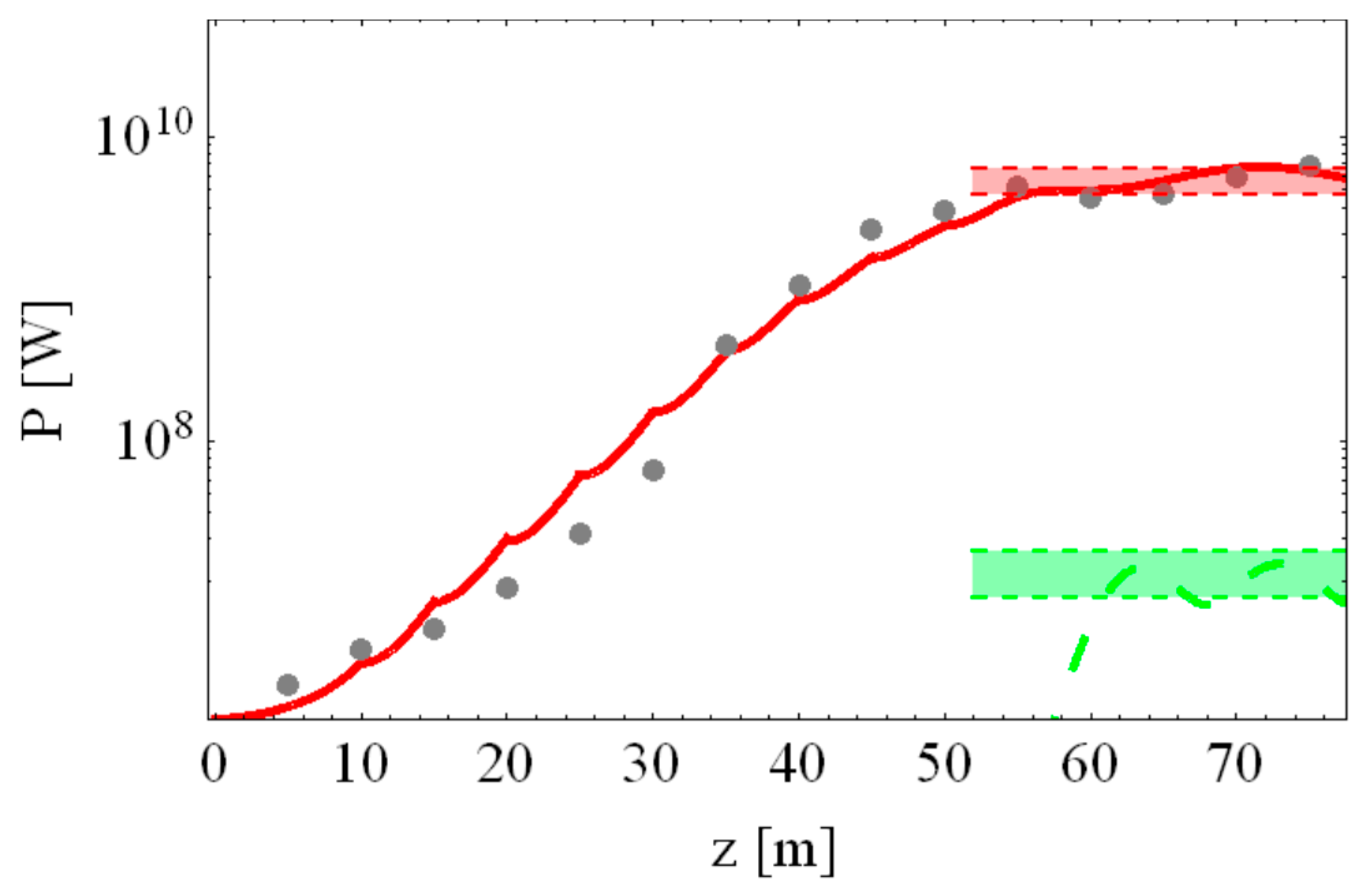
| Saturated power PF,n,W | 1.9 × 108 | — | 6 × 105 | — | 3 × 104 |
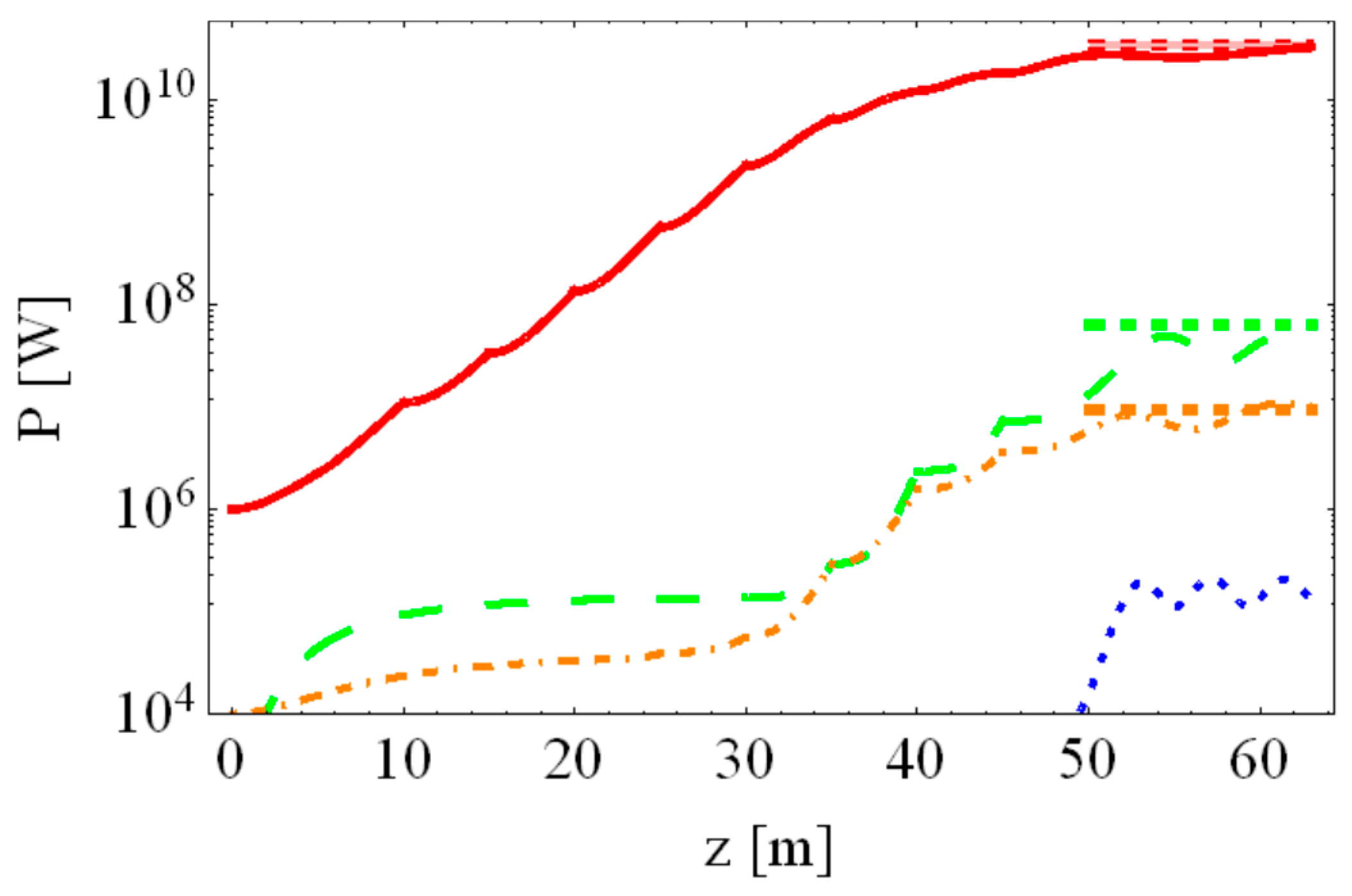
| Beam parameters: relativistic factor γ = 15264, beam power PE = 78 TW, current I0 = 10 kA, current density J = 3.04×1012 A/m2, beam section ∑ = 3.29×10−9 m2, emittances μm, βx,y = 20m, beam size ≈ 22μm, divergence ≈ 1.1 μrad, ~ 9 μrad, γθ ≈ 0.14, energy spread σe = 0.926 × 10−3 | |||||
| Undulator parameters: k = 2.1, λu = 1.8 cm, N = 277, section length 4.66 m | |||||
| Calculated FEL properties: saturated length Ls = 38 m, gain length Lgain = 2.6 m, radiation beam size ≅ 11 μm | |||||
| Harmonic number | n = 1 | n = 2 | n = 3 | n = 4 | n = 5 |
| Bessel coefficient fn | 0.79 | 0.19 | 0.27 | 0.19 | 0.11 |
| Pierce parameter | 0.00075 | 0.0003 | 0.00037 | 0.0003 | 0.0002 |
| Harmonic wavelength λn, nm | 12.4 | 6.2 | 4.1 | 3.1 | 2.5 |
| Saturated power PF,n,W | 1.9 × 1010 | 9 × 106 | 5 × 107 | 5 × 106 | 1.6 × 105 |
| Beam parameters: γ = 5870, beam power PE = 6.60 TW, current I0 = 2.2 kA, current density J = 1.246 × 1011 A/m2, beam section ∑ = 1.766 × 10−8 m2, emittances = 0.55 μm, β = 30 m, beam size = 53 μm, divergence ≈ 1.8 μrad, ≈ 15 μrad, energy spread σe = 0.5 × 10−3 | |||||
| Undulator parameters: k = 2, λu = 3.5 cm, section length 5 m | |||||
| Calculated FEL properties: saturated length Ls = 31 m, gain length Lgain = 2.0 m, radiation beam size ≈0.29 mm | |||||
| Harmonic number | n = 1 | n = 2 | n = 3 | n = 4 | n = 5 |
| Bessel coefficient fn | 0.80 | 0.13 | 0.32 | 0.13 | 0.16 |
| Pierce parameter | 0.0010 | 0.0003 | 0.0005 | 0.0003 | 0.0003 |
| Harmonic wavelength λn, nm | 1.52 | 0.76 | 0.51 | 0.38 | 0.30 |
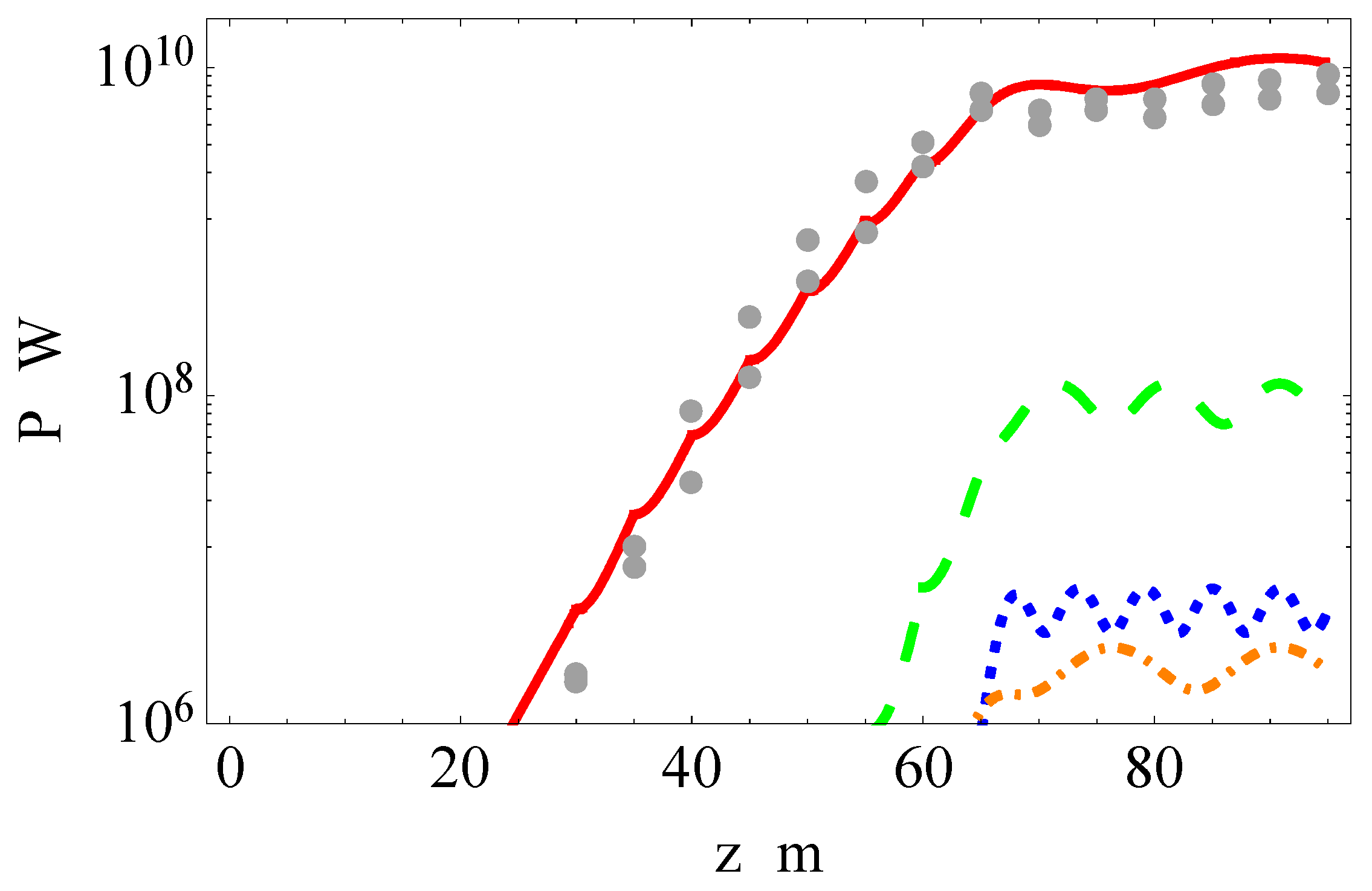
| Saturated power PF,n,W | 8.2 × 109 | 3.2 × 106 | 5.4 × 107 | 1.6 × 106 | 2.0 × 106 |
| Beam parameters: γ = 15,660, beam power PE = 20.0 TW, current I0 = 2,5 kA, current density J = 3.16 × 1011 A/m2, beam section ∑ = 7.91 × 10−9 m2, emittances μm, β ≈ 36 m, beam size = 35 μm, divergence ≈ 1 μrad, ≈4.5 μrad, energy spread σe = 0.18 × 10−3 | |||||
| Undulator parameters: k = 1.87, λu = 2.571 cm, section length 5 m | |||||
| Calculated FEL properties: saturated length Ls ~ 55 m, gain length Lgain = 3.4 m, radiation beam size ≈15 μm | |||||
| Harmonic number | n = 1 | n = 2 | n = 3 | n = 4 | n = 5 |
| Bessel coefficient fn | 0.82 | 0.09 | 0.31 | 0.09 | 0.16 |
| Pierce parameter | 0.0004 | 0.00009 | 0.0002 | 0.00009 | 0.00014 |
| Harmonic wavelength λn, nm | 0.144 | 0.072 | 0.048 | 0.036 | 0.029 |
| Saturated power PF,n,W | 1.0 × 1010 | 2.0 × 106 | 1.0 × 108 | 1.0 × 106 | 6.0 × 106 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhukovsky, K. Synchrotron Radiation in Periodic Magnetic Fields of FEL Undulators—Theoretical Analysis for Experiments. Symmetry 2020, 12, 1258. https://doi.org/10.3390/sym12081258
Zhukovsky K. Synchrotron Radiation in Periodic Magnetic Fields of FEL Undulators—Theoretical Analysis for Experiments. Symmetry. 2020; 12(8):1258. https://doi.org/10.3390/sym12081258
Chicago/Turabian StyleZhukovsky, Konstantin. 2020. "Synchrotron Radiation in Periodic Magnetic Fields of FEL Undulators—Theoretical Analysis for Experiments" Symmetry 12, no. 8: 1258. https://doi.org/10.3390/sym12081258
APA StyleZhukovsky, K. (2020). Synchrotron Radiation in Periodic Magnetic Fields of FEL Undulators—Theoretical Analysis for Experiments. Symmetry, 12(8), 1258. https://doi.org/10.3390/sym12081258




